Identification of Damping of Spruce Wood (Picea abies) under Various Levels of Moisture Content Using Time-Scale Decomposition
Abstract
:1. Introduction
2. Materials and Methods
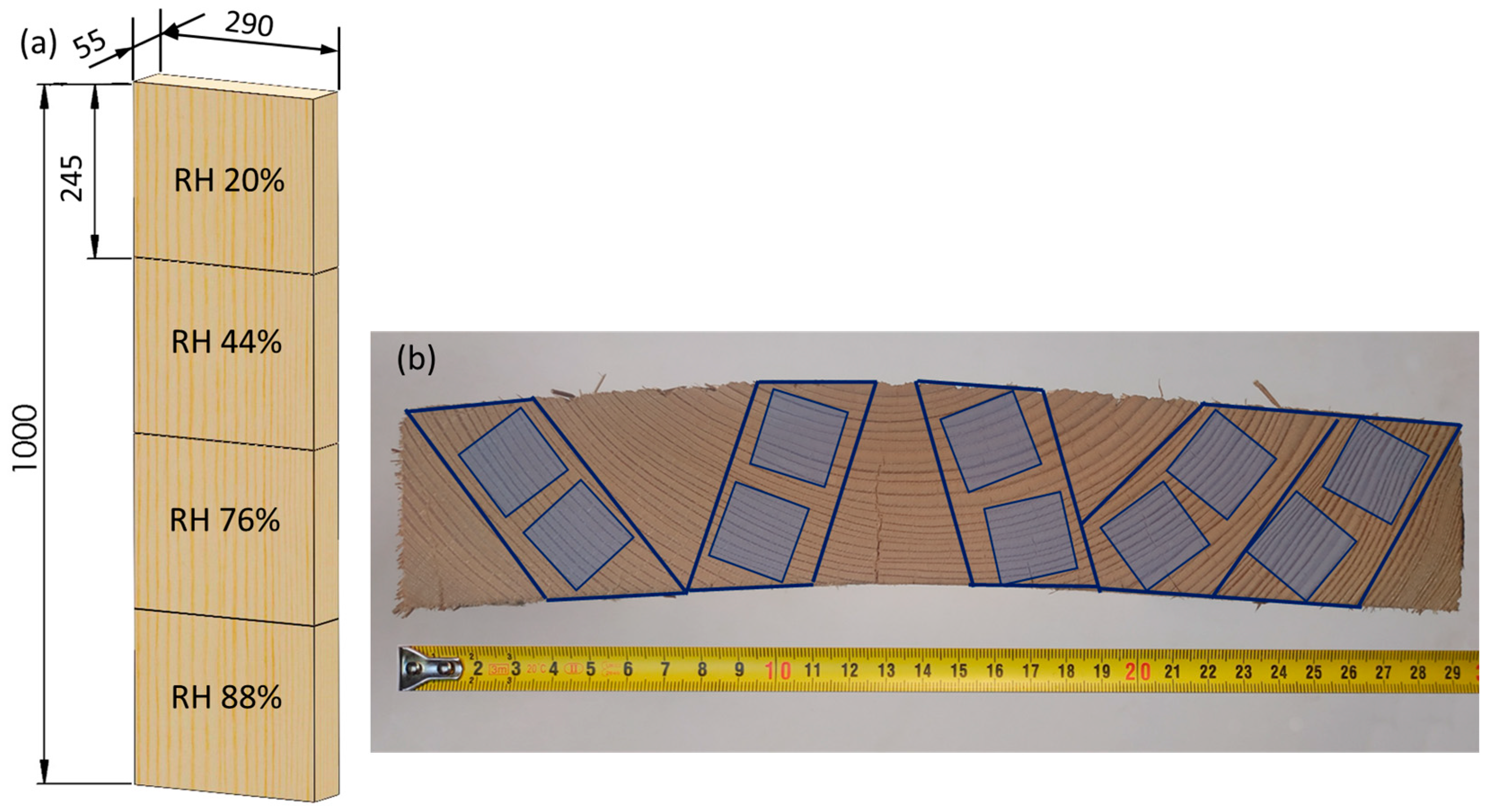
2.1. Specimen Preparation
2.2. Experimental Procedures
2.2.1. Vibration Measurements
2.2.2. Damping Determination Based on Fitting the Oscillation Envelope
2.2.3. Damping Determination Based on Wavelet Transform
2.3. Statistical Analysis
3. Results and Discussion
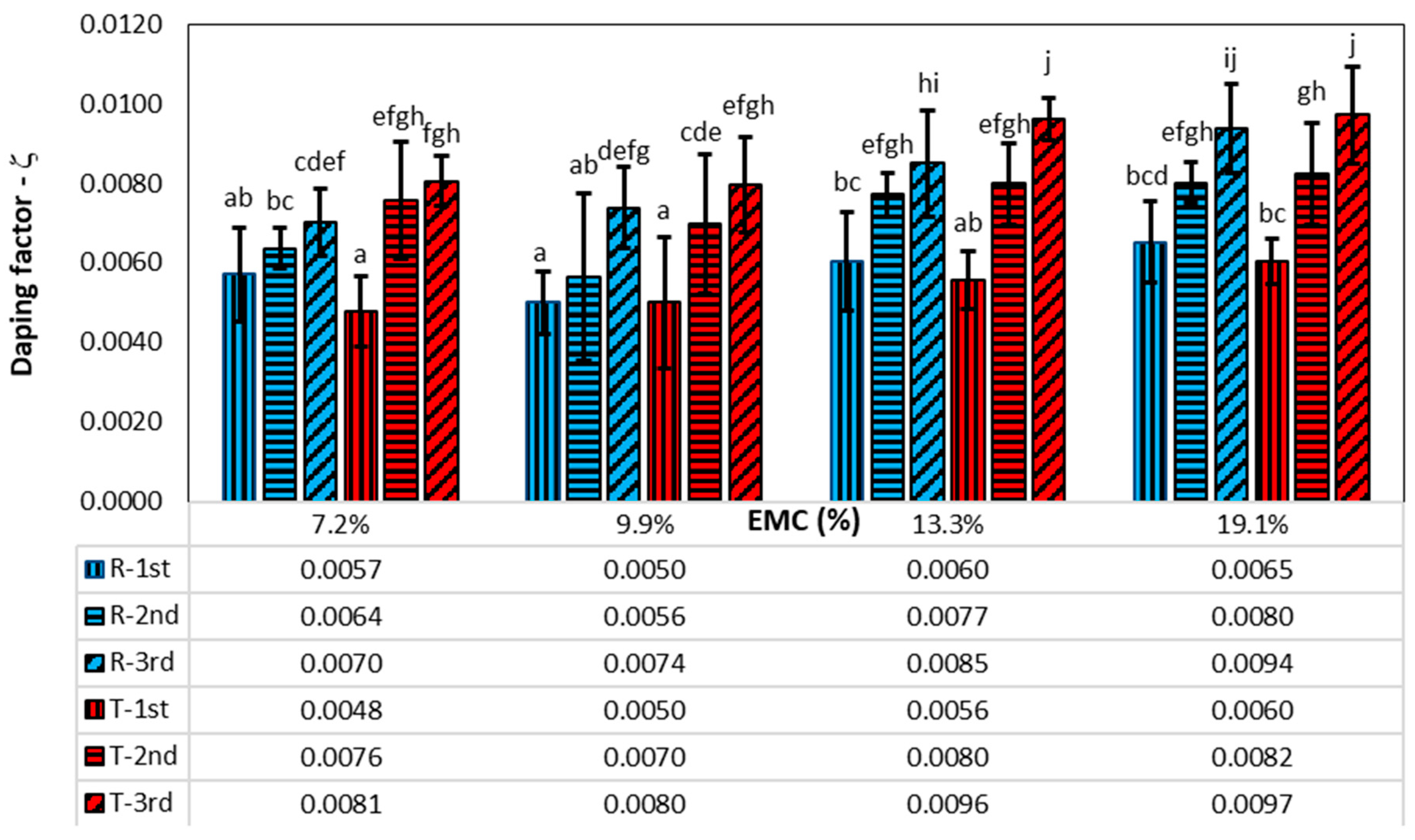
3.1. Damping Factor in 1st Vibration Mode
3.2. Damping Factor in Higher Vibration Modes
4. Conclusions
- The damping factor determined with the wavelet transform is more accurate than the logarithmic decrement or the envelope fitting method when coupling with other modes is present since the wavelet transform allows the decoupling of MDOF systems into single modes and serves as a filter that also filters out the noise added during the measurement.
- The damping factor increases with the EMC for all vibration modes and vibration directions.
- The damping factors for the first vibration mode, determined by fitting the envelope, showed, on average, 15.9% higher values than the damping factors determined with the WT method, which is mainly due to the higher amplitudes resulting from the coupling with other vibration modes.
- The damping factor in the first vibration mode is, on average, 9% higher in the radial direction than in the tangential direction, while the opposite is true for the higher vibration modes. Thus, the damping factor in the tangential direction is higher than in the radial direction by 10% and 8.8% for the second and third vibration modes, respectively.
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Thomson, W.T. Theory of Vibration with Applications, 4th ed.; CRC Press: London, UK, 2018; p. 558. [Google Scholar] [CrossRef]
- Piersol, A.; Paez, T. Harris’ Shock and Vibration Handbook, 6th, ed.; McGraw Hill: New York, NY, USA, 2009. [Google Scholar]
- Zhang, L.; Tiemann, A.; Zhang, T.; Gauthier, T.; Hsu, K.; Mahamid, M.; Moniruzzaman, P.K.; Ozevin, D. Nondestructive assessment of cross-laminated timber using non-contact transverse vibration and ultrasonic testing. Eur. J. Wood Wood Prod. 2021, 79, 335–347. [Google Scholar] [CrossRef]
- Brémaud, I. Acoustical properties of wood in string instruments soundboards and tuned idiophones: Biological and cultural diversity. J. Acoust. Soc. Am. 2012, 131, 807–818. [Google Scholar] [CrossRef] [PubMed]
- Brémaud, I.; El Kaïm, Y.; Guibal, D.; Minato, K.; Thibaut, B.; Gril, J. Characterisation and categorisation of the diversity in viscoelastic vibrational properties between 98 wood types. Ann. For. Sci. 2012, 69, 373–386. [Google Scholar] [CrossRef]
- Brémaud, I.; Gril, J. Moisture content dependence of anisotropic vibrational properties of wood at quasi equilibrium: Analytical review and multi-trajectories experiments. Holzforschung 2021, 75, 313–327. [Google Scholar] [CrossRef]
- Ahmed, S.A.; Adamopoulos, S. Acoustic properties of modified wood under different humid conditions and their relevance for musical instruments. Appl. Acoust. 2018, 140, 92–99. [Google Scholar] [CrossRef]
- Zauer, M.; Kowalewski, A.; Sproßmann, R.; Stonjek, H.; Wagenführ, A. Thermal modification of European beech at relatively mild temperatures for the use in electric bass guitars. Eur. J. Wood Wood Prod. 2016, 74, 43–48. [Google Scholar] [CrossRef]
- Mania, P.; Skrodzka, E. Modal parameters of resonant spruce wood (Picea abies L.) after thermal treatment. J. King Saud Univ.-Sci. 2020, 32, 1152–1156. [Google Scholar] [CrossRef]
- Danihelová, A.; Vidholdová, Z.; Gergeľ, T.; Kružlicová, L.S.; Pástor, M. Thermal Modification of Spruce and Maple Wood for Special Wood Products. Polymers 2022, 14, 2813. [Google Scholar] [CrossRef] [PubMed]
- Stanciu, M.D.; Sova, D.; Savin, A.; Ilias, N.; Gorbacheva, G.A. Physical and mechanical properties of ammonia-treated black locust wood. Polymers 2020, 12, 377. [Google Scholar] [CrossRef]
- Buchelt, B.; Krüger, R.; Wagenführ, A. The vibrational properties of native and thermally modified wood in dependence on its moisture content. Eur. J. Wood Wood Prod. 2023, 81, 947–956. [Google Scholar] [CrossRef]
- Wegst, U.G.K. Wood for sound. Am. J. Bot. 2006, 93, 1439–1448. [Google Scholar] [CrossRef] [PubMed]
- Merhar, M.; Humar, M. The influence of wood modification on transfer function of a violin bridge. Drv. Ind. 2020, 71, 163–169. [Google Scholar] [CrossRef]
- Sproßmann, R.; Zauer, M.; Wagenführ, A. Characterization of acoustic and mechanical properties of common tropical woods used in classical guitars. Results Phys. 2017, 7, 1737–1742. [Google Scholar] [CrossRef]
- Gurău, L.; Timar, M.C.; Coșereanu, C.; Cosnita, M.; Stanciu, M.D. Aging of Wood for Musical Instruments: Analysis of Changes in Color, Surface Morphology, Chemical, and Physical-Acoustical Properties during UV and Thermal Exposure. Polymers 2023, 15, 1794. [Google Scholar] [CrossRef] [PubMed]
- Mihalcica, M.; Stanciu, M.D.; Teodorescu, H.D.; Iftimie, N. Evaluation of viscous-elastic properties of resonance maple wood. Int. J. Mod. Manuf. Technol. 2022, 14, 145–150. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, J.; Wang, Z.; Hua, F.; He, S.; Lu, B.; Wang, X.; Zhang, X.; Leng, W. Microstructural and Thermo-Mechanical Characterization of Furfurylated Douglas Fir. Polymers 2022, 14, 4641. [Google Scholar] [CrossRef] [PubMed]
- Staszewski, W.J. Identification of Damping in Mdof Systems Using Time-Scale Decomposition. J. Sound Vib. 1997, 203, 283–305. [Google Scholar] [CrossRef]
- Morlet, J. Sampling theory and wave propagation. In Issues in Acoustic Signal/Image Processing and Recognition; Springer: New York, NY, USA, 1983; pp. 233–261. [Google Scholar] [CrossRef]
- Chui, C.K. An Introduction to Wavelets, 1st ed.; Academic Press: Cambridge, MA, USA, 1992. [Google Scholar]
- Ruzzene, M.; Fasana, A.; Garibaldi, L.; Piombo, B. Natural frequencies and dampings identification using wavelet transform: Application to real data. Mech. Syst. Signal Process. 1997, 11, 207–218. [Google Scholar] [CrossRef]
- Tomac, I.; Slavič, J. Morlet-wave-based modal identification in the time domain. Mech. Syst. Signal Process. 2023, 192, 110243. [Google Scholar] [CrossRef]
- Chandra, N.H.; Sekhar, A.S. Nonlinear damping identification in rotors using wavelet transform. Mech. Mach. Theory 2016, 100, 170–183. [Google Scholar] [CrossRef]
- Chen, S.-L.; Liu, J.-J.; Lai, H.-C. Wavelet analysis for identification of damping ratios and natural frequencies. J. Sound Vib. 2009, 323, 130–147. [Google Scholar] [CrossRef]
- Wang, S.; Zhao, W.; Zhang, G.; Xu, H.; Du, Y. Identification of structural parameters from free vibration data using Gabor wavelet transform. Mech. Syst. Signal Process. 2021, 147, 107122. [Google Scholar] [CrossRef]
- Perez-Ramirez, C.A.; Amezquita-Sanchez, J.P.; Adeli, H.; Valtierra-Rodriguez, M.; Camarena-Martinez, D.; Romero-Troncoso, R.J. New methodology for modal parameters identification of smart civil structures using ambient vibrations and synchrosqueezed wavelet transform. Eng. Appl. Artif. Intell. 2016, 48, 1–12. [Google Scholar] [CrossRef]
- Ashory, M.R.; Khatibi, M.M.; Jafari, M.; Malekjafarian, A. Determination of mode shapes using wavelet transform of free vibration data. Arch. Appl. Mech. 2013, 83, 907–921. [Google Scholar] [CrossRef]
- Slavič, J.; Simonovski, I.; Boltežar, M. Damping identification using a continuous wavelet transform: Application to real data. J. Sound Vib. 2003, 262, 291–307. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A Practical Guide to Wavelet Analysis. Bulletin of the American Meteorological Society 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Daubechies, I. The Wavelet Transform, Time-Frequency Localization and Signal Analysis. IEEE Trans. Inf. Theory 1990, 36, 961–1005. [Google Scholar] [CrossRef]
- Tchamitchian, P.; Torresani, B. Ridge and skeleton extraction from the wavelet transform. In Proceedings of the CBMS-NSF Conference W‘avelets and Their Applications’, Lowell, MA, USA, 11–15 June 1991. [Google Scholar]
- Obataya, E. Effects of natural and artificial ageing on the physical and acoustic properties of wood in musical instruments. J. Cult. Herit. 2017, 27, S63–S69. [Google Scholar] [CrossRef]








| Type III Sum of Squares | df | Mean Square | F | p | |
|---|---|---|---|---|---|
| Determination method | 3.75 × 10−5 | 1 | 3.75 × 10−5 | 21.019 | 0.000 |
| Vibration direction | 2.86 × 10−5 | 1 | 2.86 × 10−5 | 16.041 | 0.000 |
| EMC | 5.90 × 10−5 | 3 | 1.97 × 10−5 | 11.006 | 0.000 |
| Source | Type III Sum of Squares | df | Mean Square | F | p |
|---|---|---|---|---|---|
| Vibration mode | 0.000306 | 2 | 0.000153 | 120.487 | 0.000 |
| Vibration direction | 6.99 × 10−6 | 1 | 6.99 × 10−6 | 5.510 | 0.020 |
| EMC | 0.000105 | 3 | 3.5 × 10−5 | 27.624 | 0.000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Merhar, M. Identification of Damping of Spruce Wood (Picea abies) under Various Levels of Moisture Content Using Time-Scale Decomposition. Polymers 2024, 16, 1313. https://doi.org/10.3390/polym16101313
Merhar M. Identification of Damping of Spruce Wood (Picea abies) under Various Levels of Moisture Content Using Time-Scale Decomposition. Polymers. 2024; 16(10):1313. https://doi.org/10.3390/polym16101313
Chicago/Turabian StyleMerhar, Miran. 2024. "Identification of Damping of Spruce Wood (Picea abies) under Various Levels of Moisture Content Using Time-Scale Decomposition" Polymers 16, no. 10: 1313. https://doi.org/10.3390/polym16101313






