Entropy Generation in a Couple Stress Fluid Flow Through a Vertical Channel Filled with Saturated Porous Media
Abstract
:1. Introduction
2. Mathematical Model


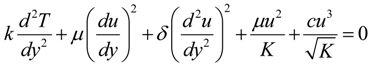
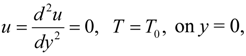



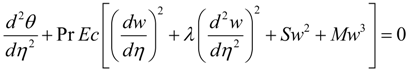
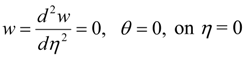

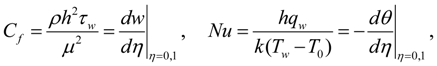
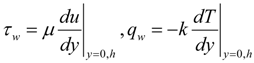
3. Entropy Analysis
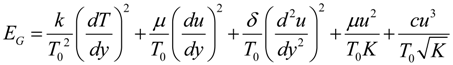
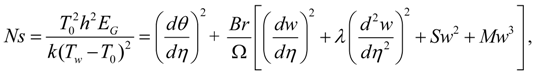
 is the temperature difference parameter and Br = EcPr is the Brinkmann number. The Bejan number Be is defined as:
is the temperature difference parameter and Br = EcPr is the Brinkmann number. The Bejan number Be is defined as:
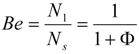
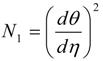 (Heat transfer Irreversibility),
(Heat transfer Irreversibility), 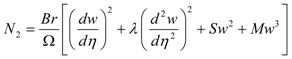 (Irreversibility due to viscous dissipation, couple stresses and porous media),
(Irreversibility due to viscous dissipation, couple stresses and porous media), 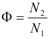 (Irreversibility ratio).
(Irreversibility ratio). . While Be = 0 represents the limit case of fluid friction, couple stress and porous media dominated irreversibility, Be = 1 corresponds to the limit case of heat transfer dominated irreversibility. The contribution of both heat transfer and fluid friction to entropy production in the flow system is the same when Be = 0.5.
. While Be = 0 represents the limit case of fluid friction, couple stress and porous media dominated irreversibility, Be = 1 corresponds to the limit case of heat transfer dominated irreversibility. The contribution of both heat transfer and fluid friction to entropy production in the flow system is the same when Be = 0.5.4. Results and Discussion
4.1. Variation of Parameters on Velocity Profile
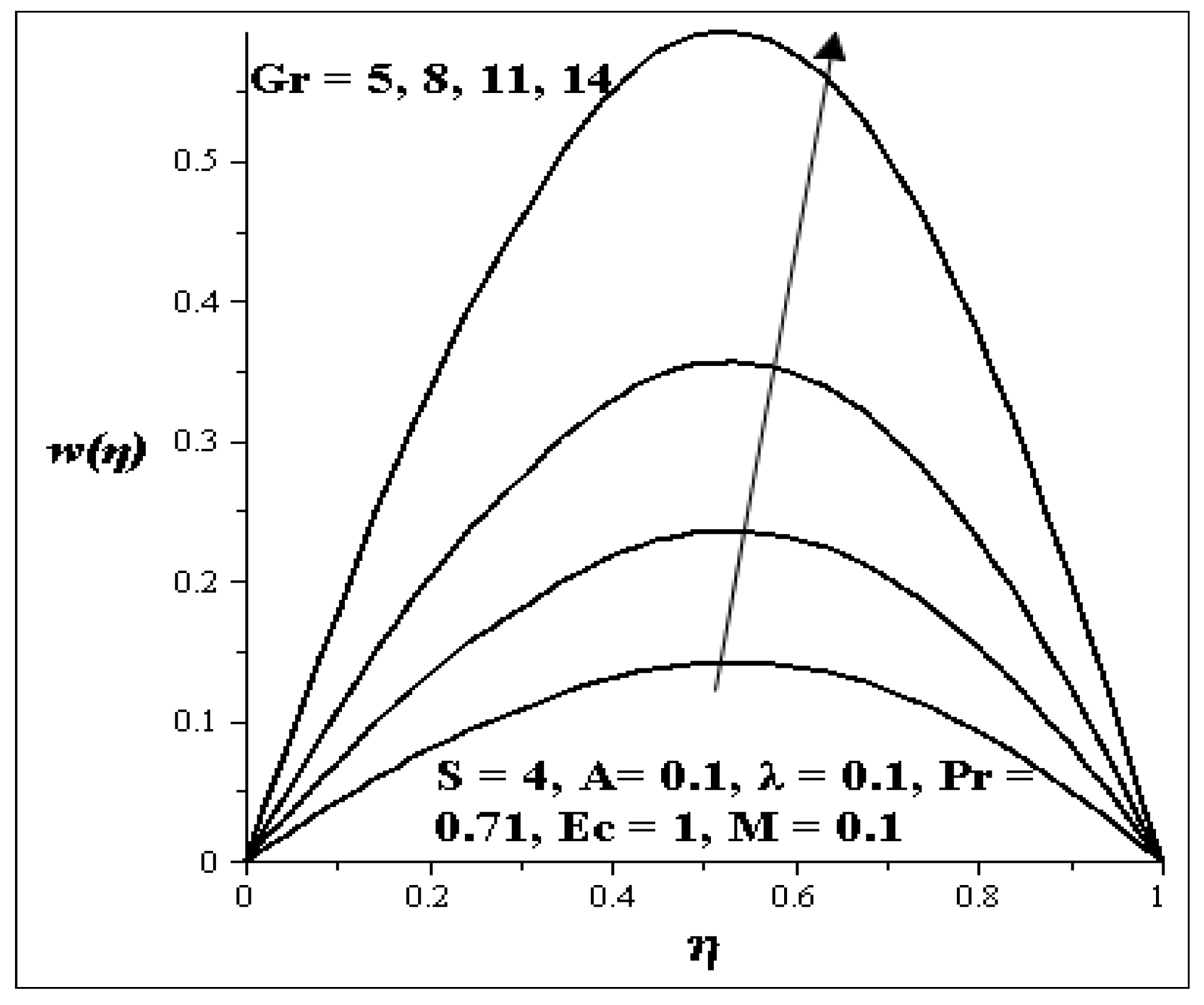
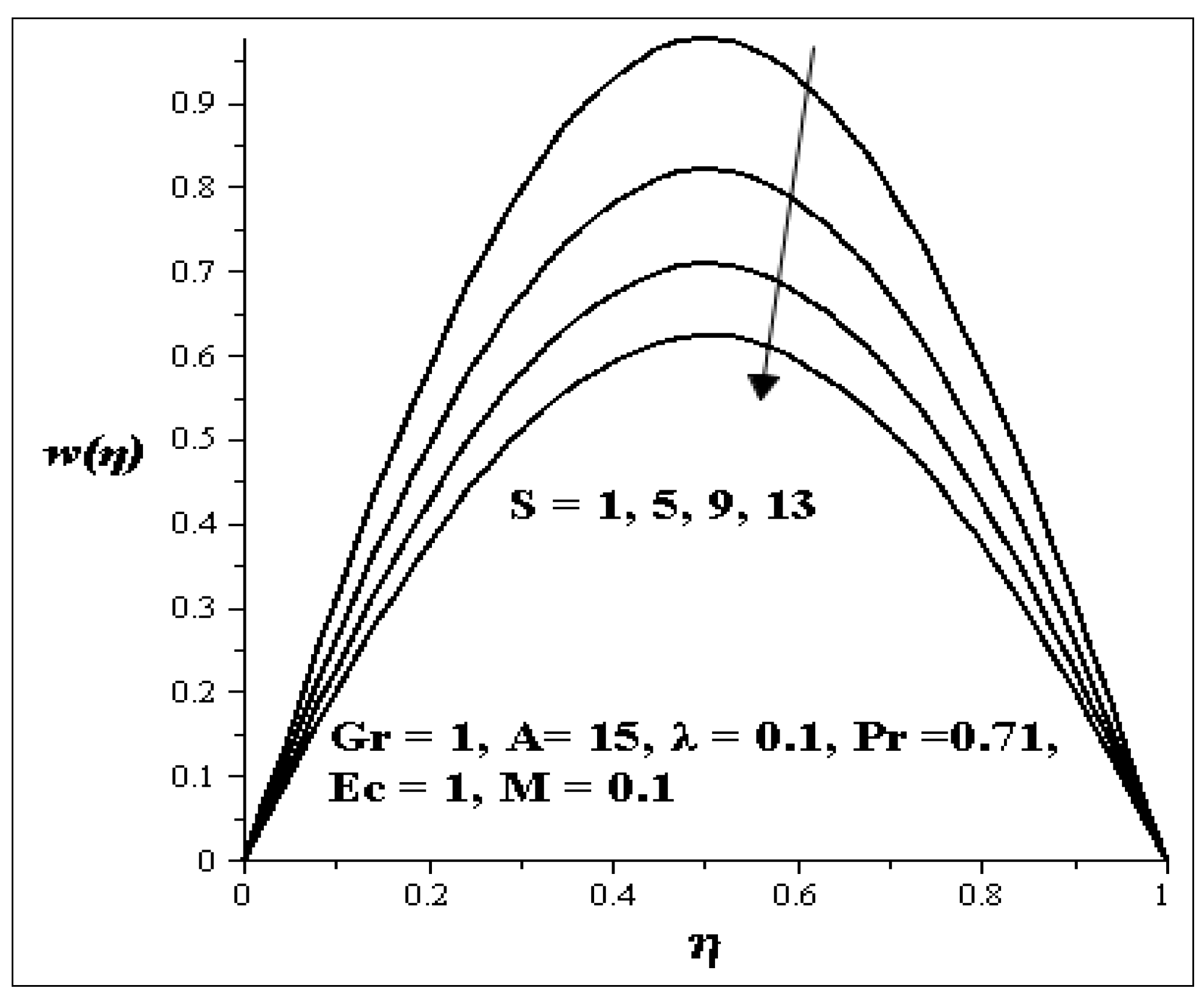
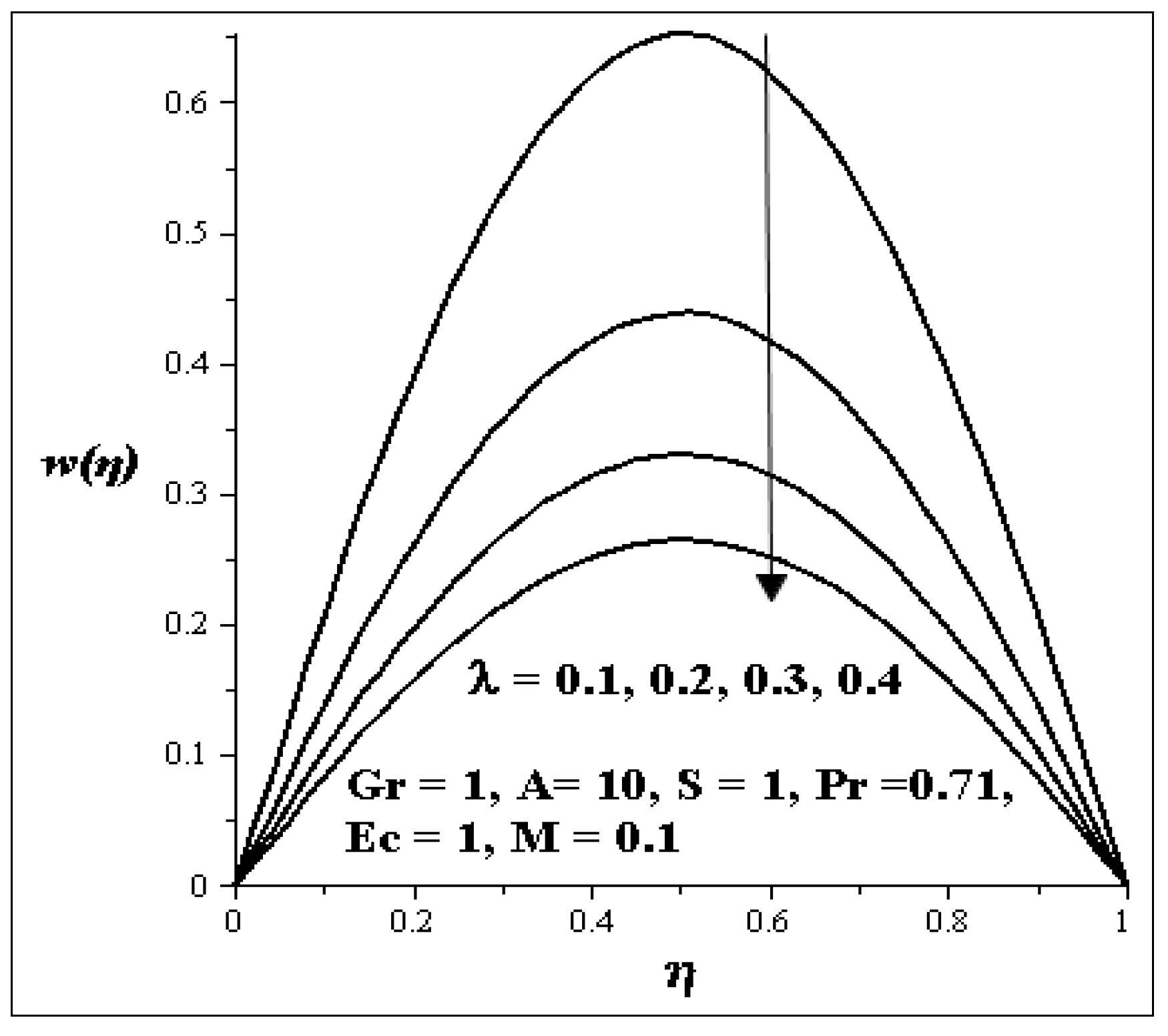


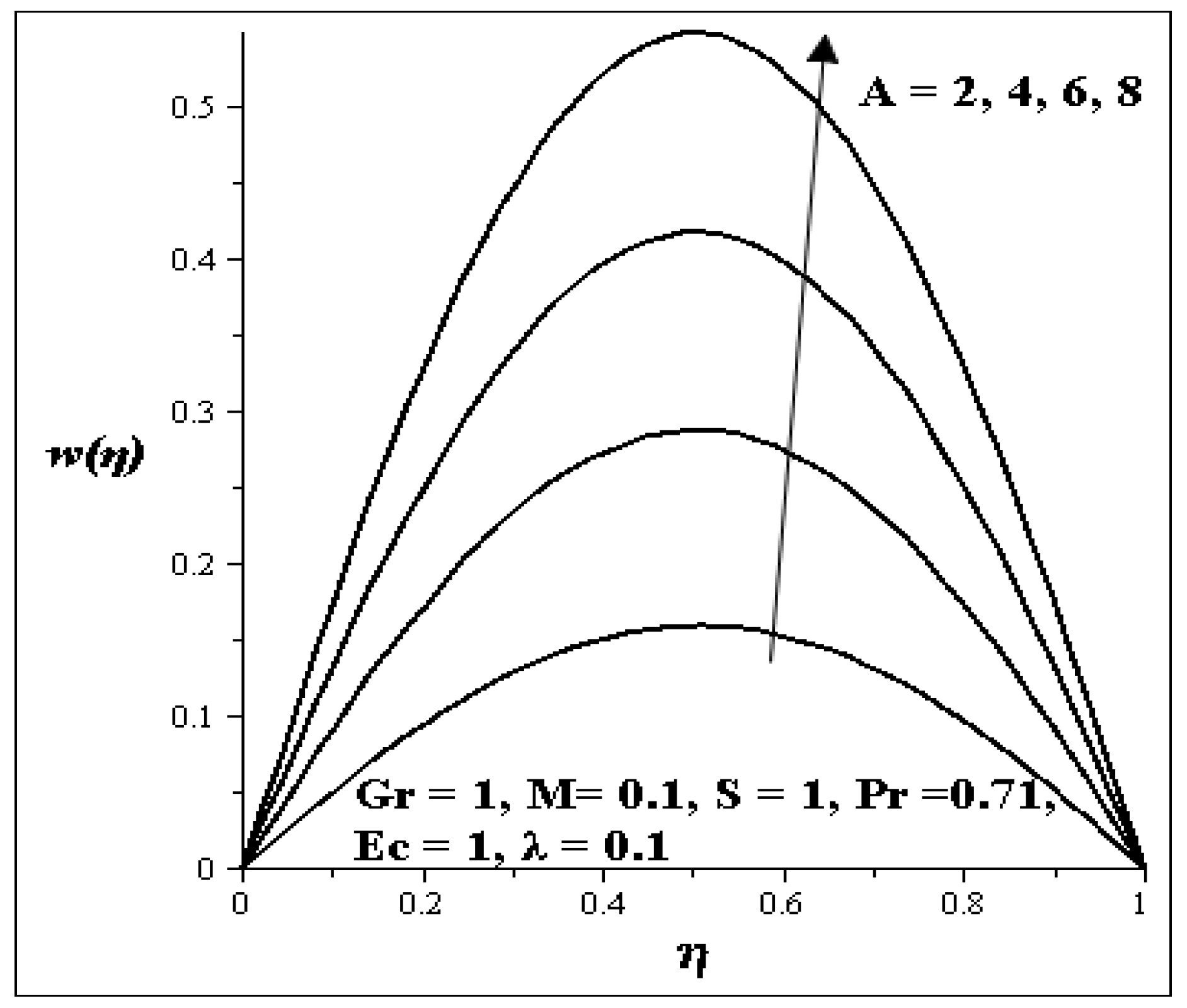
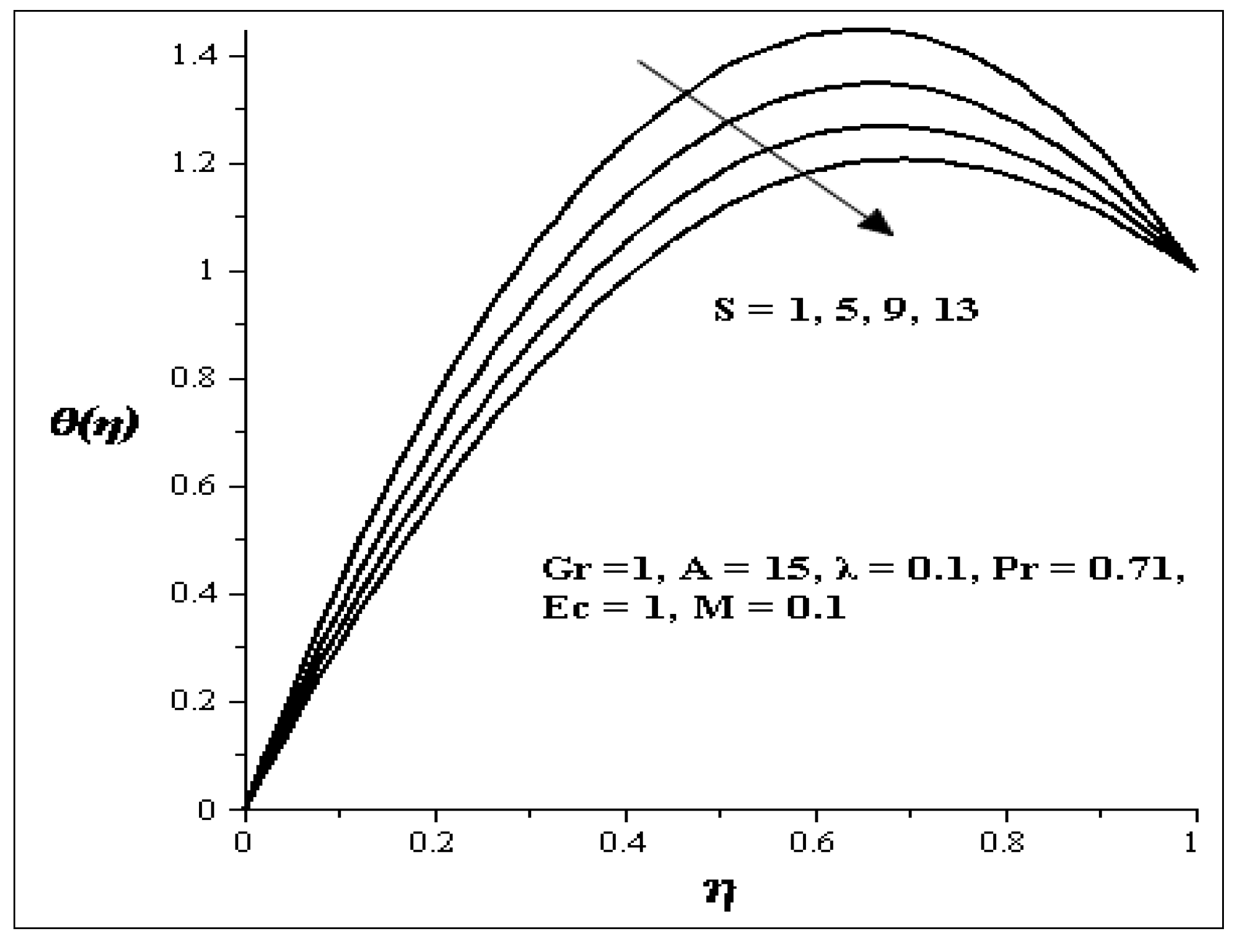
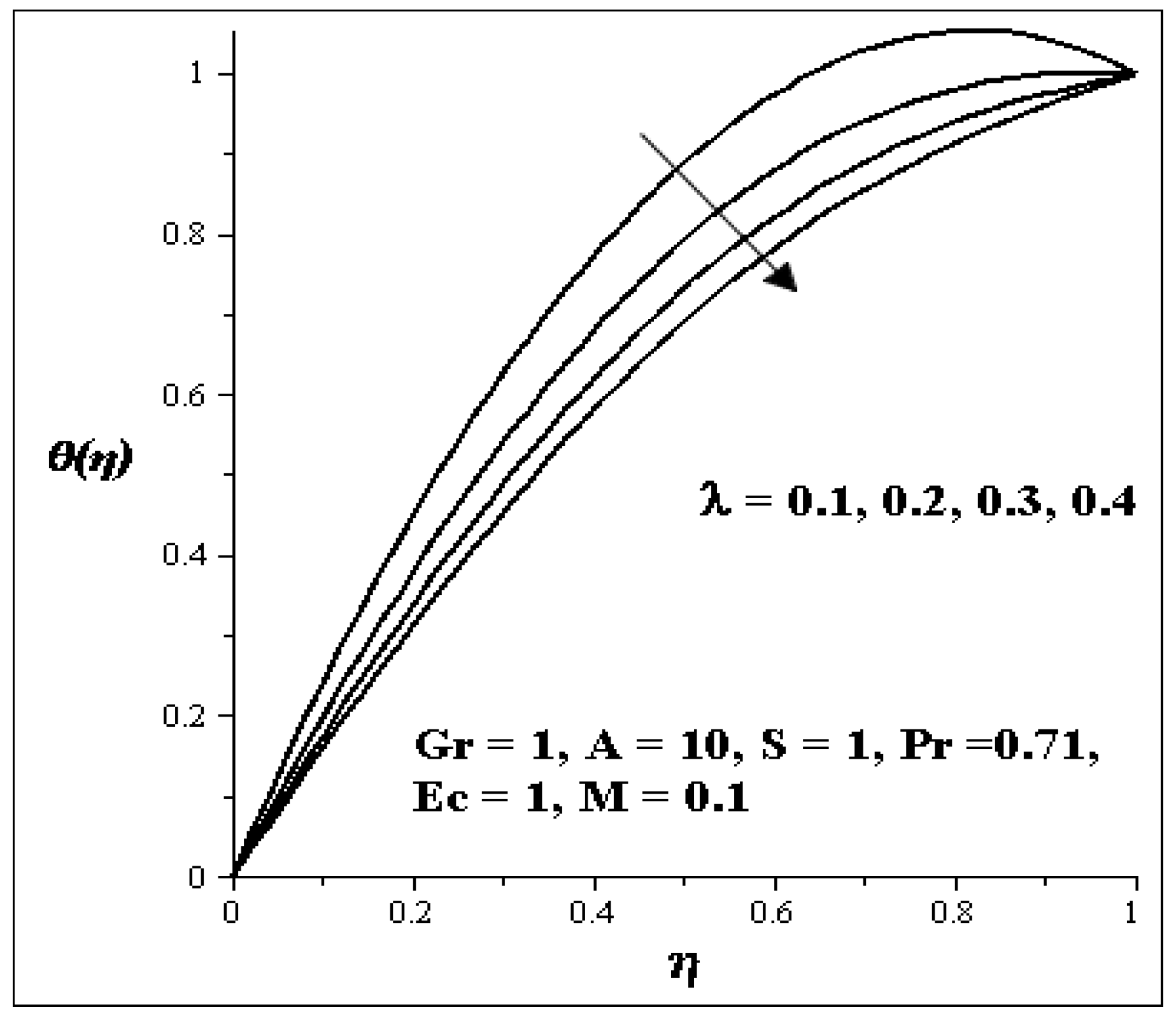
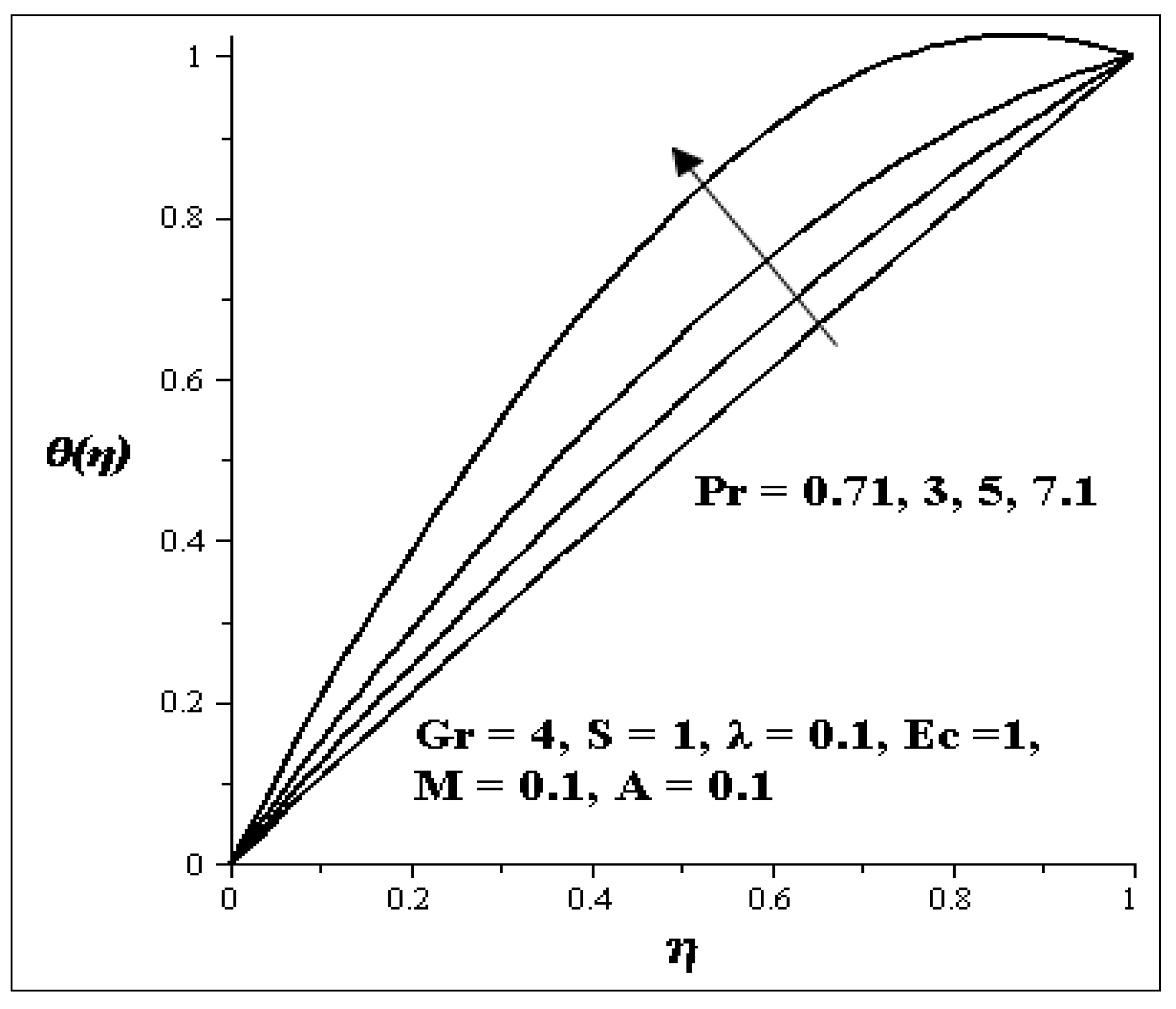
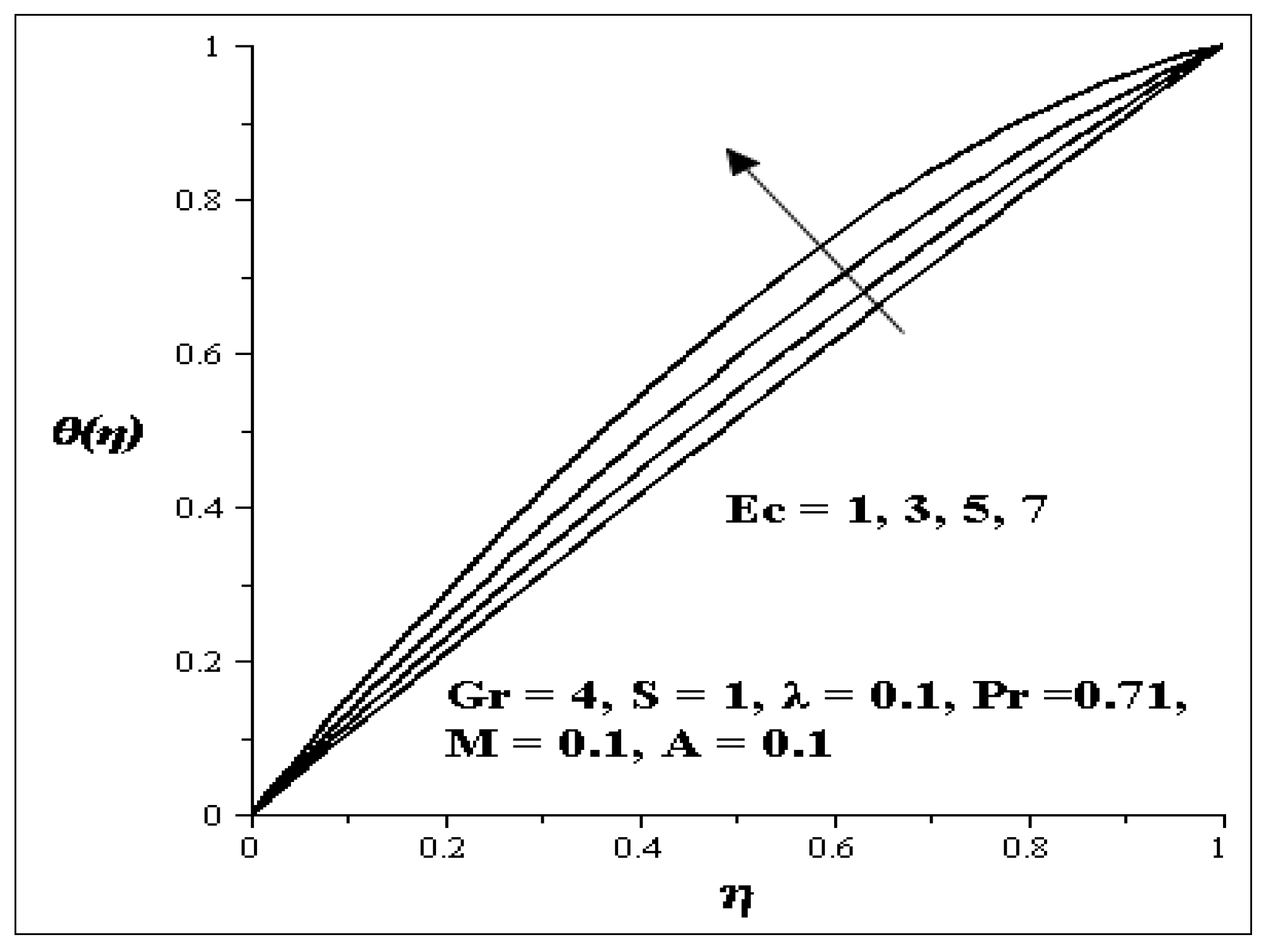
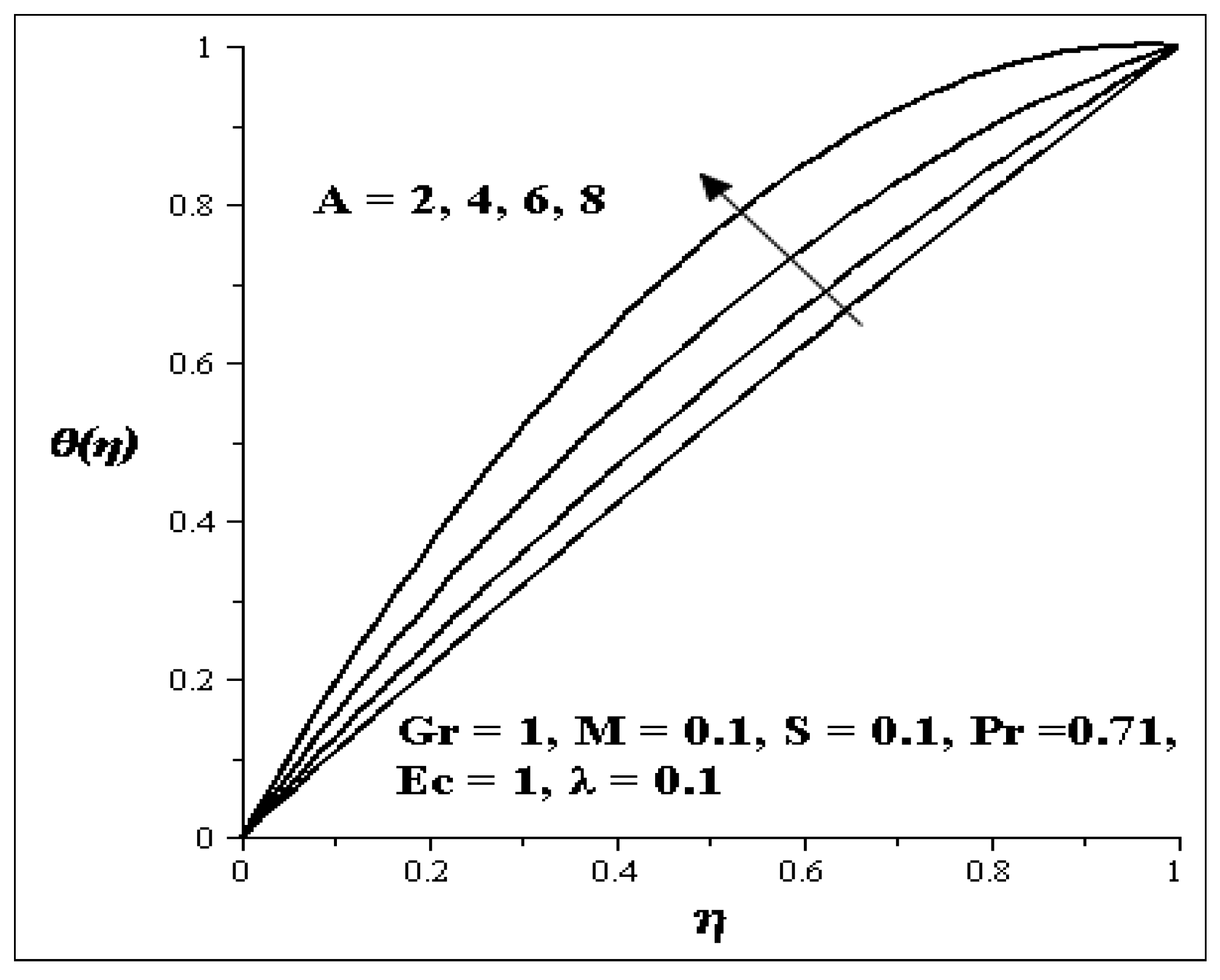
4.2. Variation of Parameters on Temperature Profile


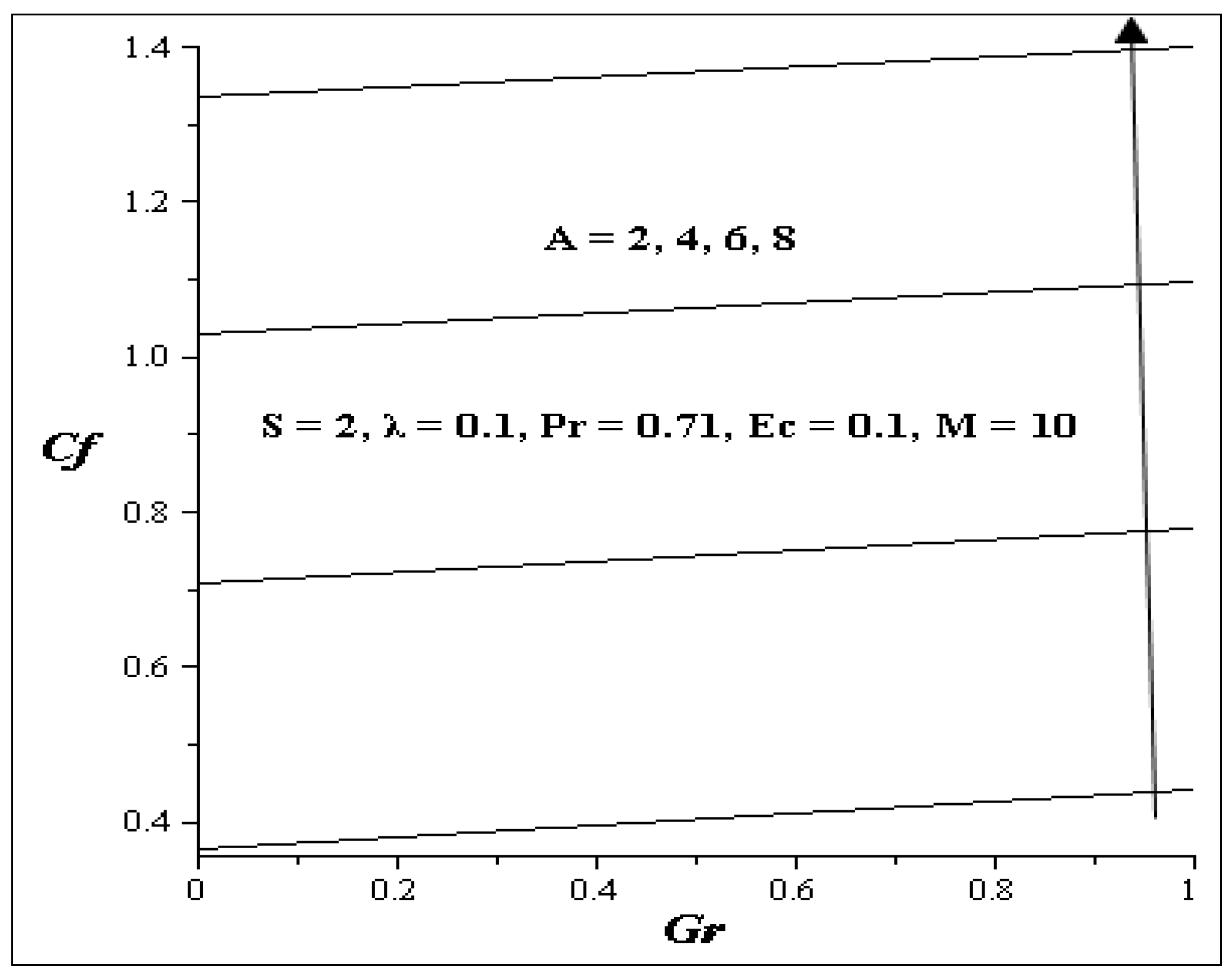
4.3. Variation of Parameters on Skin Friction

4.4. Variation of Parameters on Nusselt Number


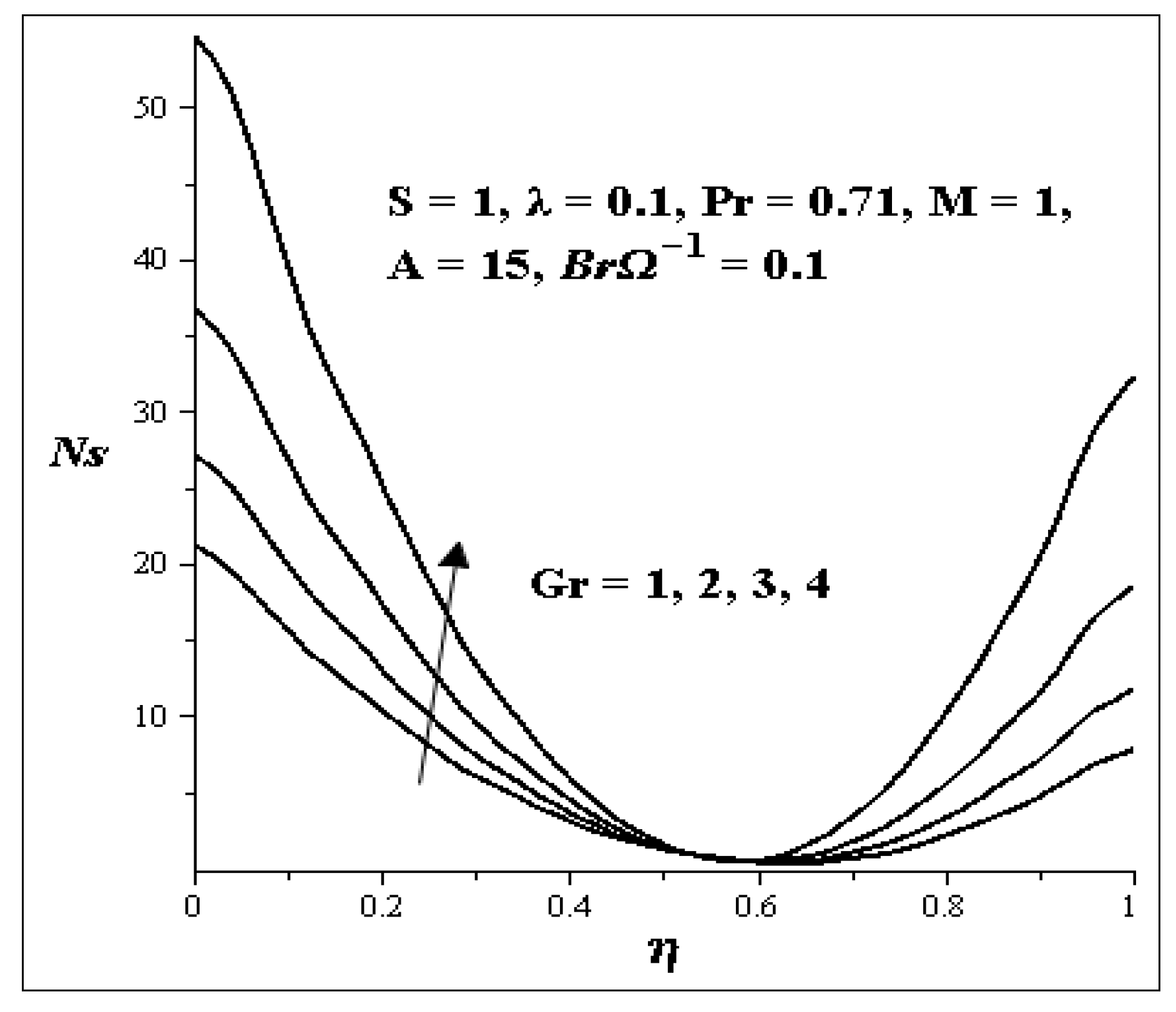
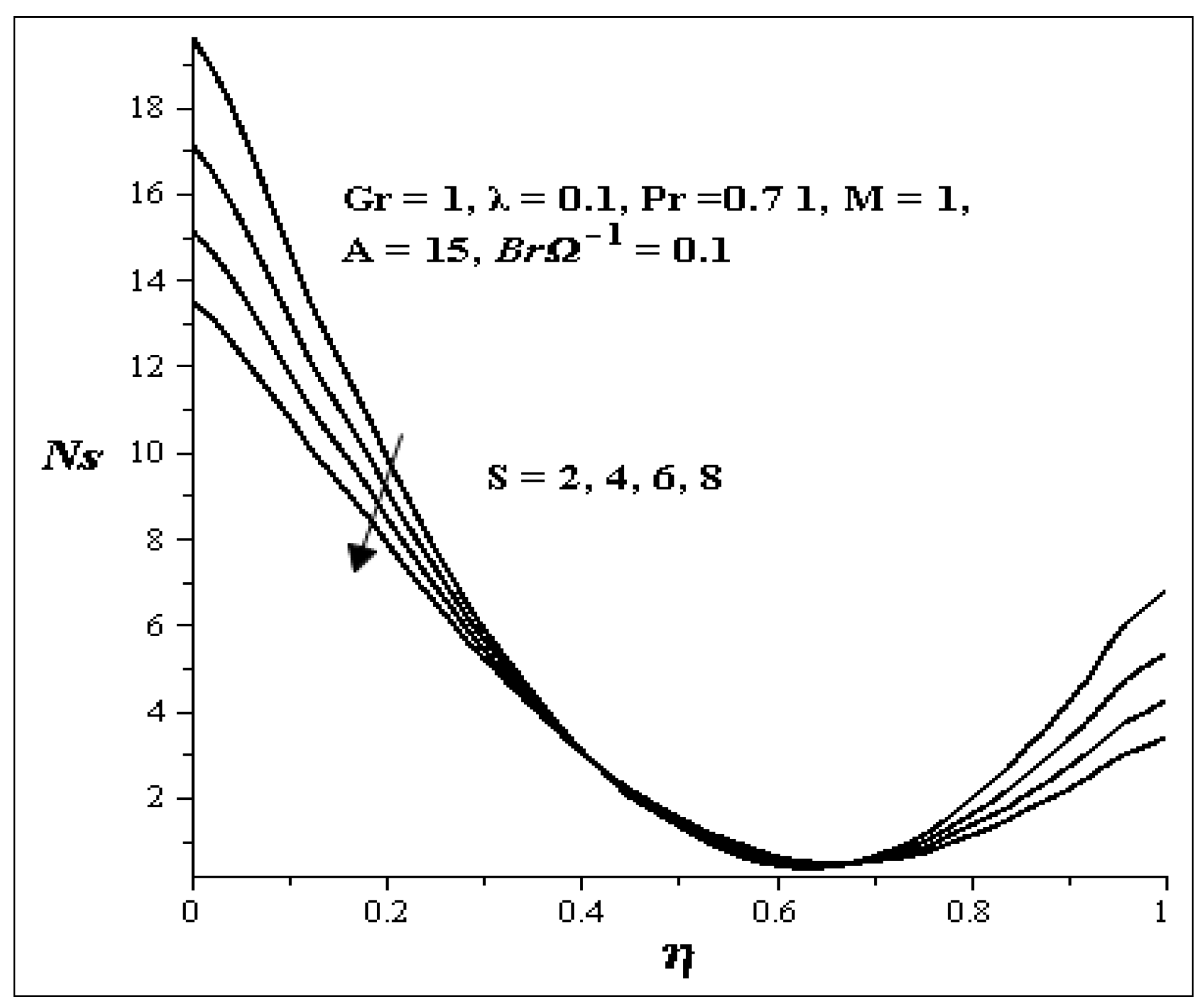
4.4. Effect of Various Parameters on Entropy Generation Profiles
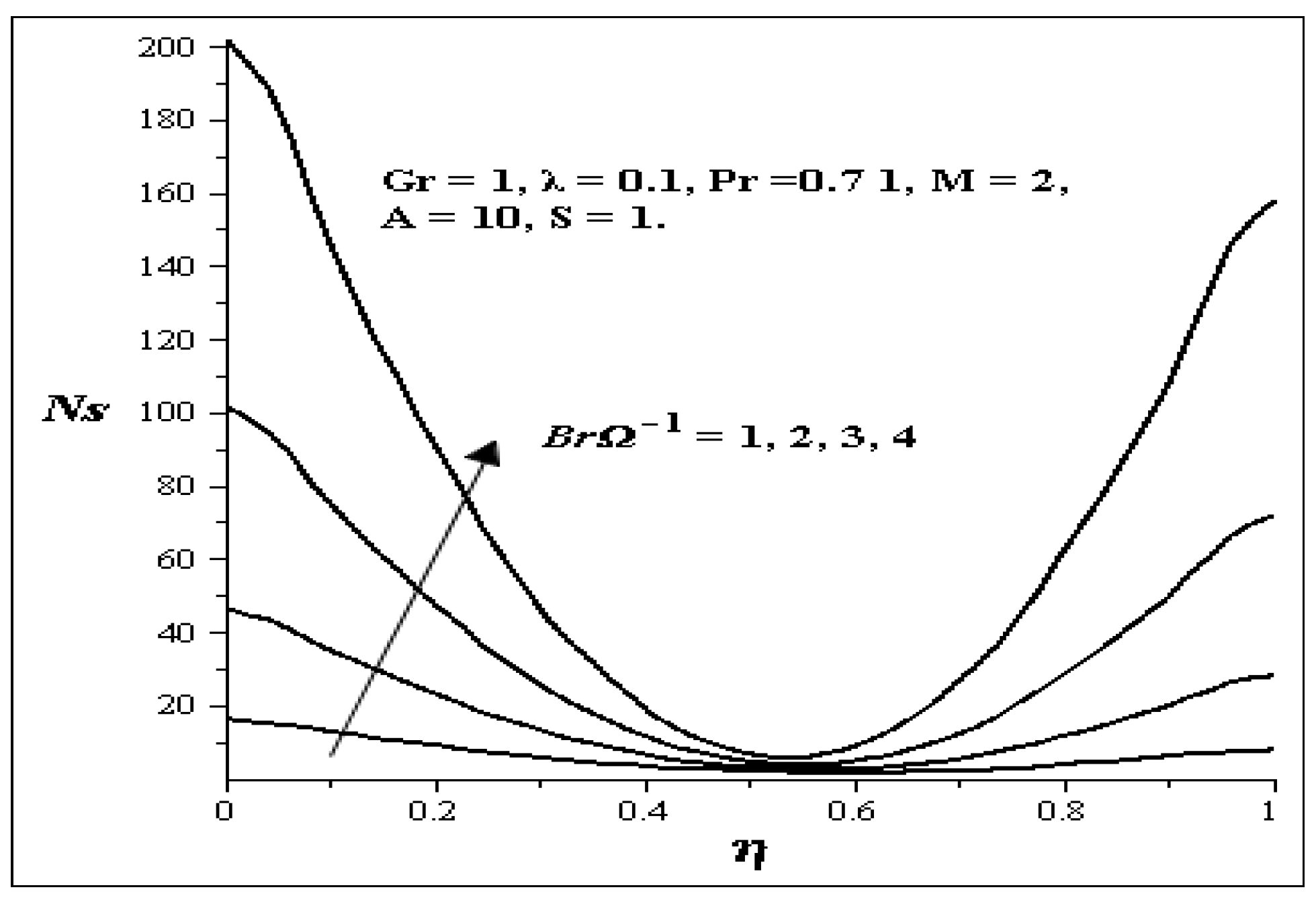
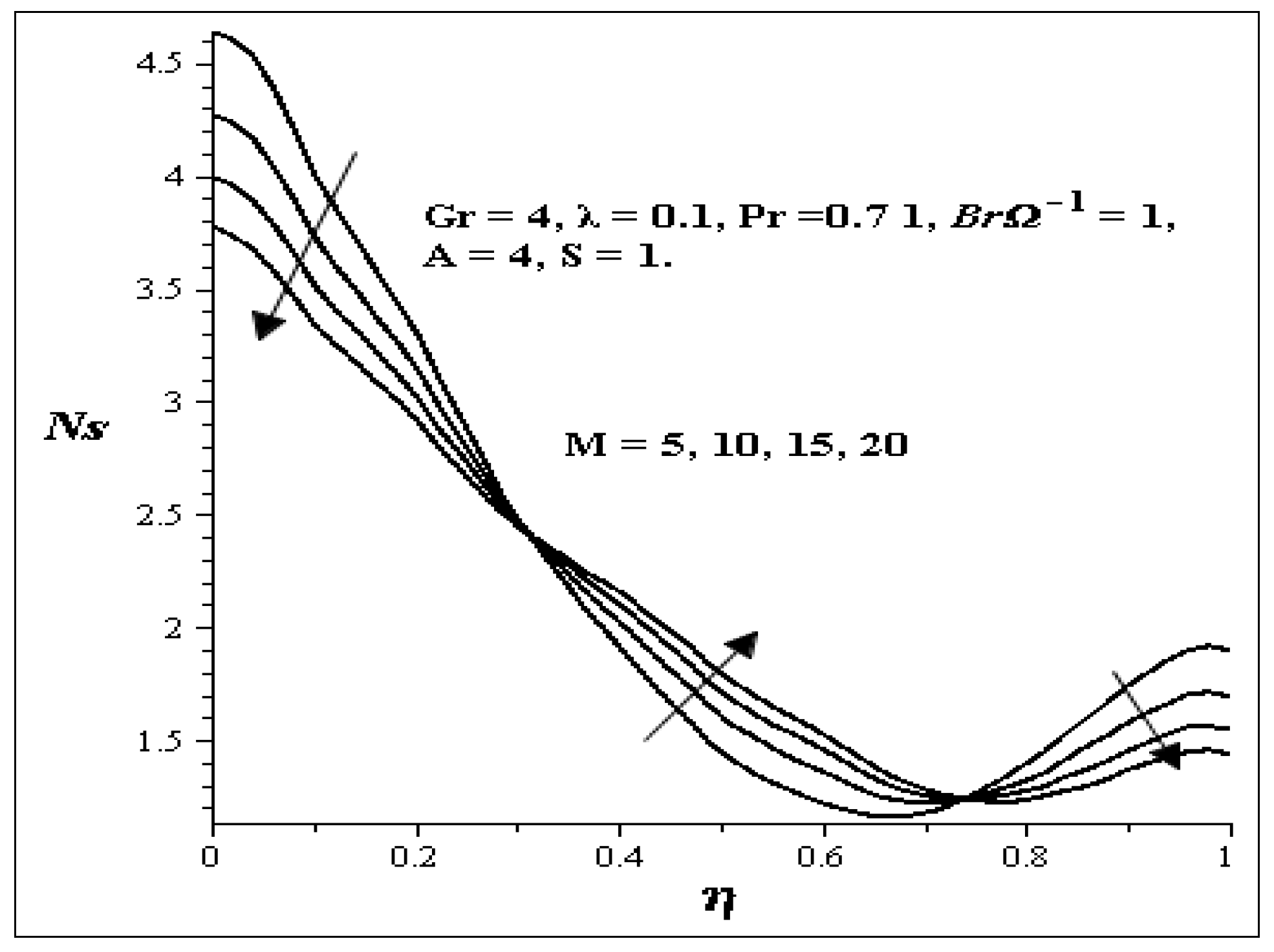
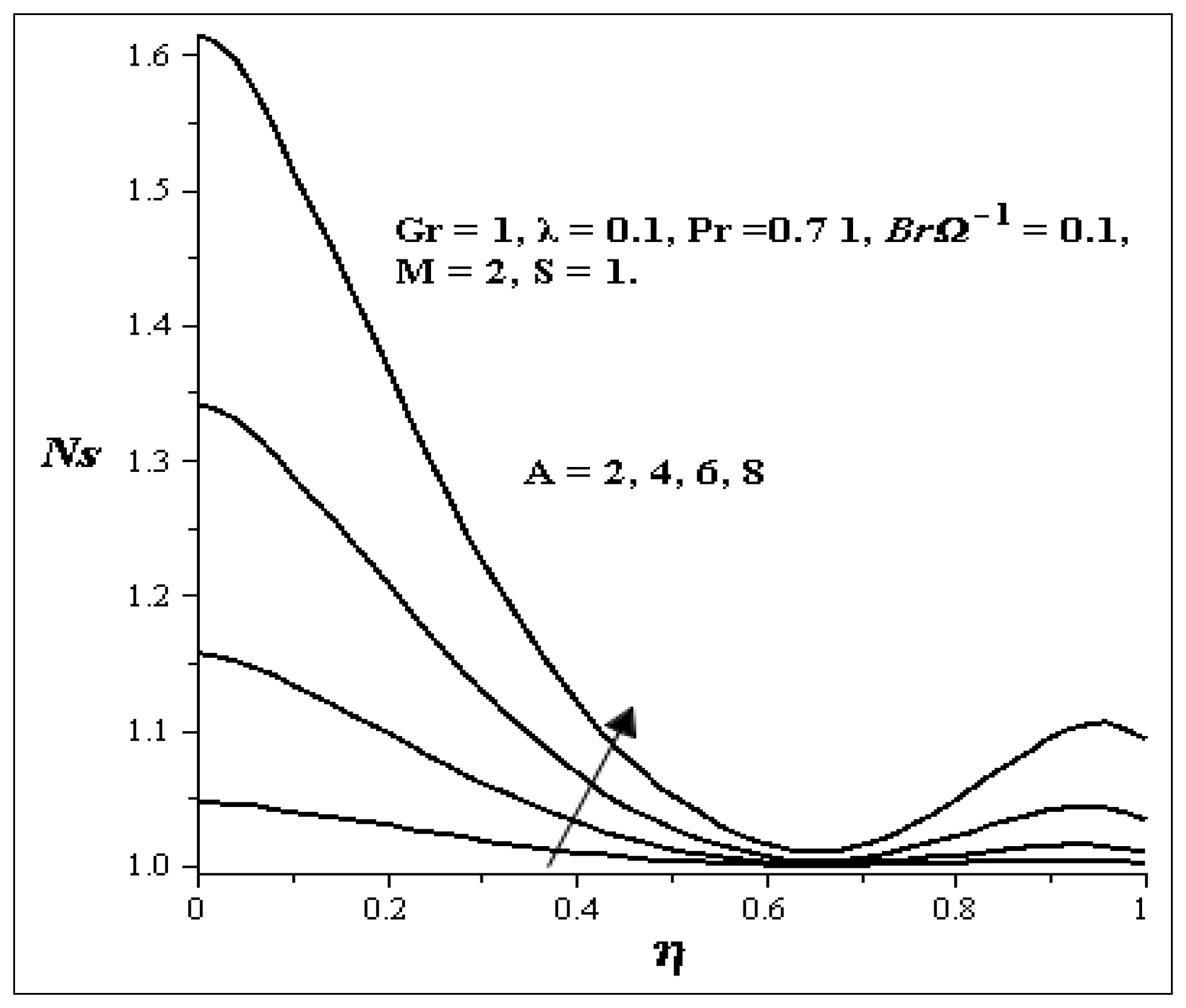
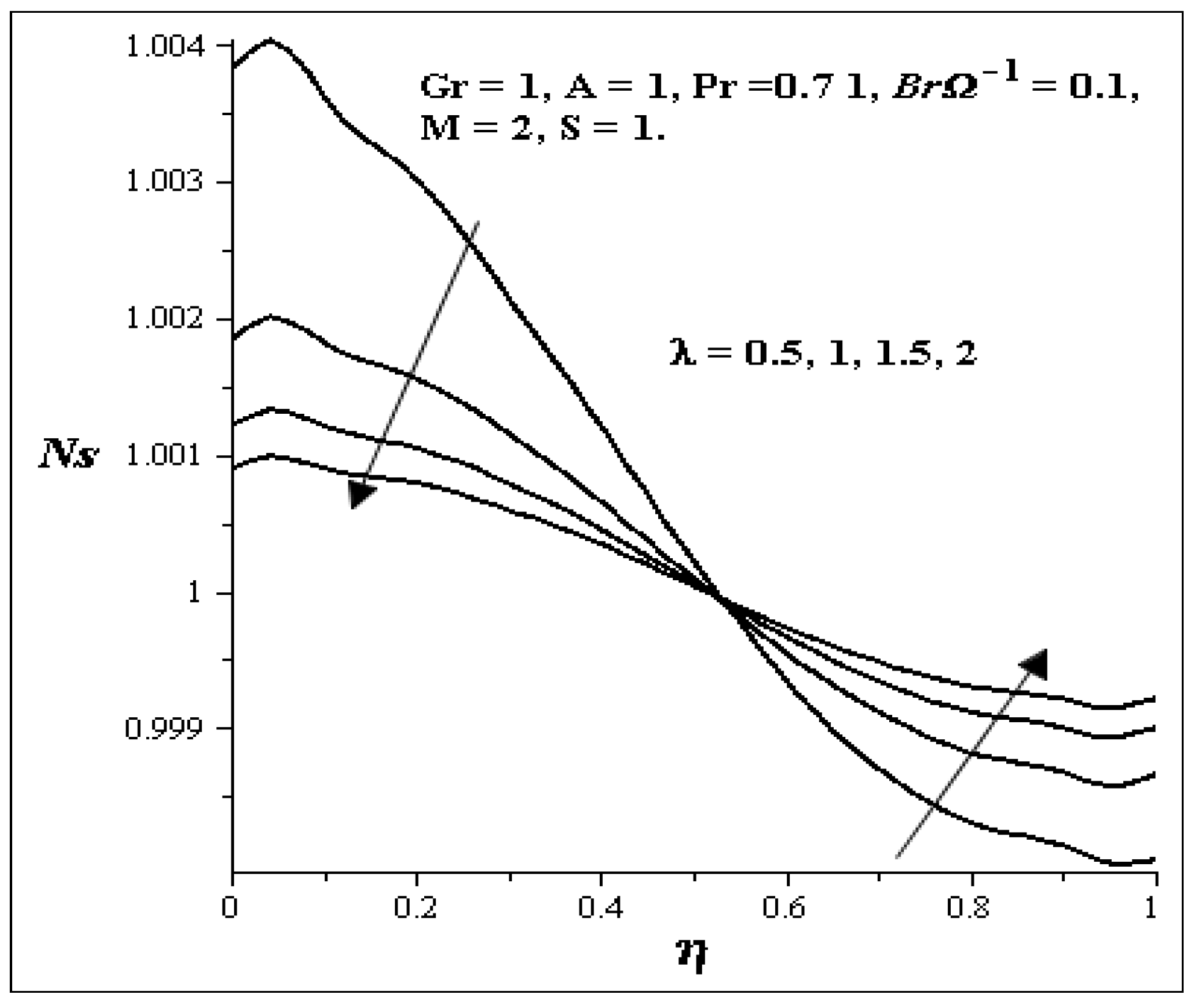
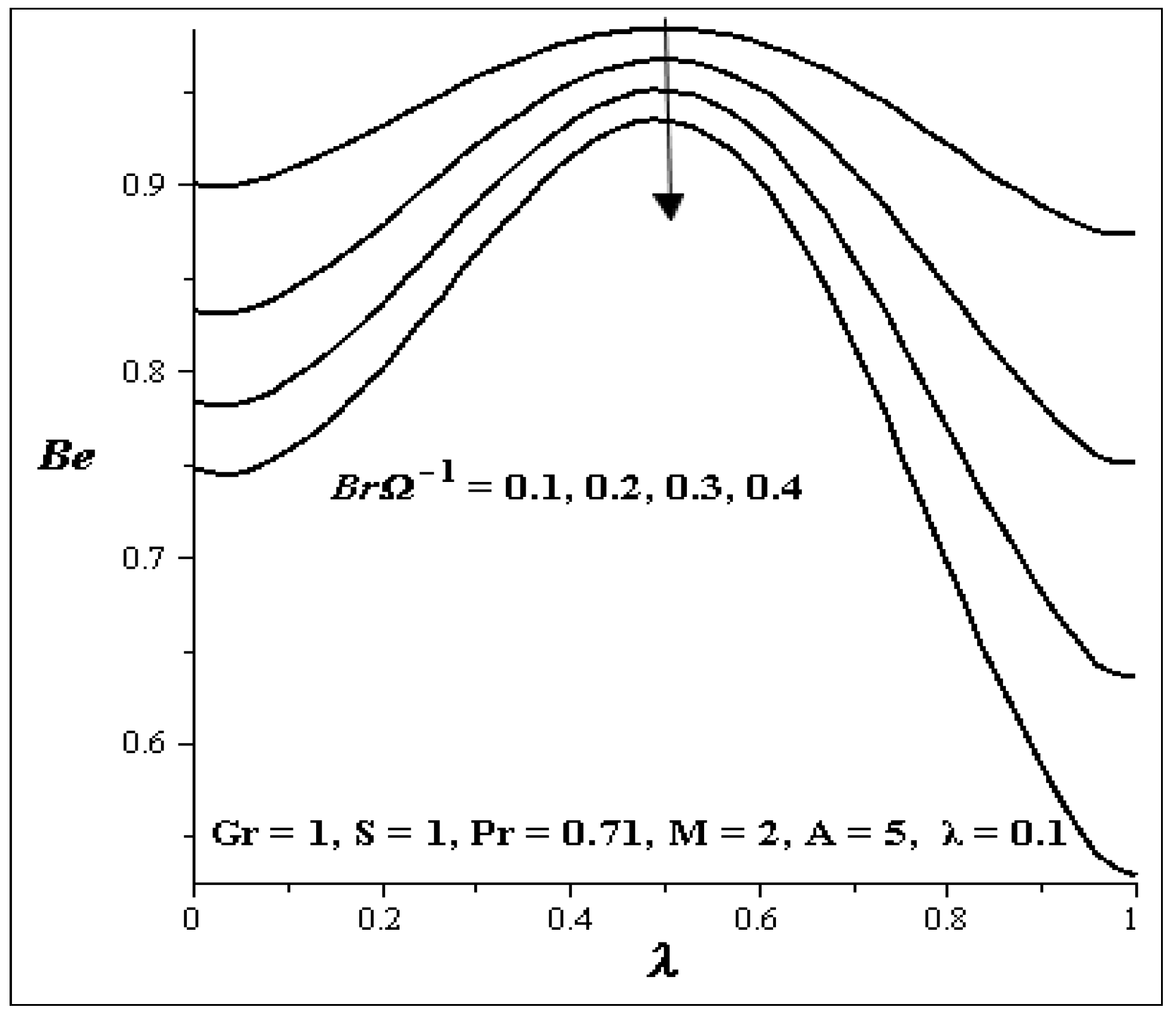
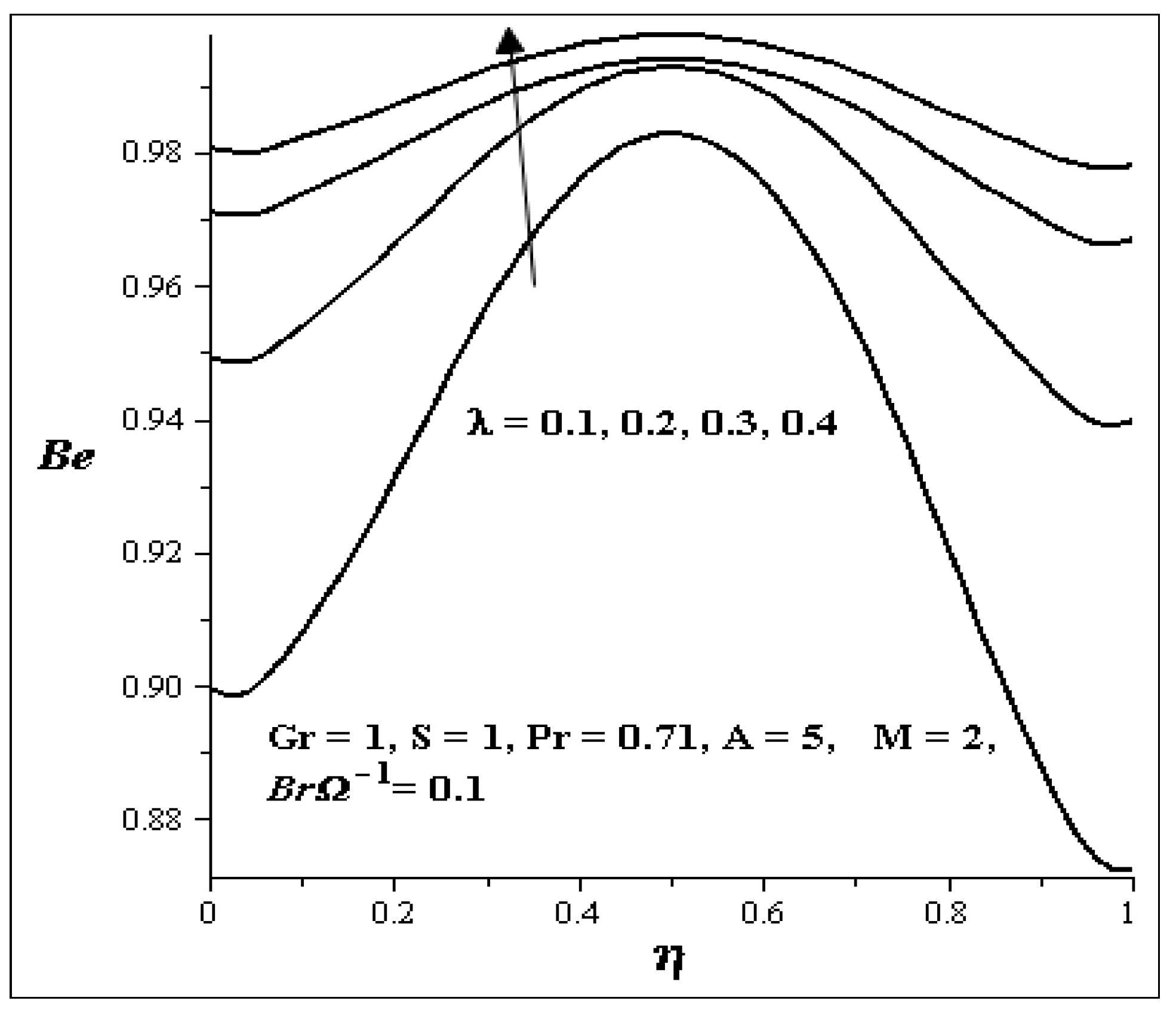
4.5. Effect of Different Parameters on Bejan Number




5. Conclusions
- The velocity profiles in general are parabolic in nature. Increases in Gr, Pr and A increase the velocity profile, while increases in M, S and λ decrease the velocity profile.
- An increase in Gr, Pr, Ec and A increases the temperature profile, while increases in S, M and λ decrease the temperature profile.
- An increase in M verses S decreases the skin friction, while an increase in A versus Gr increases the skin friction.
- The Nusselt number increases with increasing A versus S, while it decreases with an increase in M versus S.
- Entropy generation increases with increases in Gr, BrΩ−1 and A, while it decreases with an increase in S. As M increases, its decreases at both walls, but increases in the centre of the channel.
- Increases in Gr, A and BrΩ−1 decrease the Bejan number across the flow, while an increase in λ increases the Bejan number across the flow. Increases in M and S; increase the Bejan number at both walls, but at the centre of the channel, the Bejan number decreases.
Conflicts of Interest
References
- Stokes, V.K. Couple stresses in fluid. Phys. Fluids 1966, 9, 1709–1715. [Google Scholar] [CrossRef]
- Stokes, V.K. Theories of Fluids with Microstructure: An Introduction; Springer: New York, NY, USA, 1984. [Google Scholar]
- Srinivasacharya, D.; Srikanth, D. Effect of couple stresses on the flow in a constricted annulus. Arch. Appl. Mech. 2008, 78, 251–257. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer: New York, NY, USA, 2006. [Google Scholar]
- Postelnicu, A. Influence of a magnetic field on heat and mass transfer by natural convection from vertical surfaces in porous media considering Soret and Dufour effects. Int. J. Heat Mass Trans. 2004, 47, 1467–1475. [Google Scholar] [CrossRef]
- Makinde, O.D.; Mhone, P.Y. On temporal stability analysis for hydromagnetic flow in a channel filled with a saturated porous medium. Flow Turbul. Combust. 2009, 83, 21–32. [Google Scholar] [CrossRef]
- Makinde, O.D.; Aziz, A. MHD mixed convection from a vertical plate embedded in a porous medium with a convective boundary condition. Int. J. Therm. Sci. 2010, 49, 1813–1820. [Google Scholar] [CrossRef]
- Makinde, O.D.; Chinyoka, T.; Rundora, L. Unsteady flow of a reactive variable viscosity non-Newtonian fluid through a porous saturated medium with asymmetric convective boundary conditions. Comp. Math. Appl. 2011, 62, 3343–3352. [Google Scholar] [CrossRef]
- Bejan, A. Second-law analysis in heat transfer and thermal design. Adv. Heat Trans. 1982, 15, 1–58. [Google Scholar]
- Bejan, A. Entropy Generation Minimization; CRC: Boca Raton, FL, USA, 1996. [Google Scholar]
- Wood, L.C. Thermodynamics of Fluid Systems; Oxford University Press: Oxford, UK, 1975. [Google Scholar]
- Ozkol, I.; Komurgoz, G.; Arikoglu, A. Entropy generation in the laminar natural convection from a constant temperature vertical plate in an infinite fluid. J. Power Energy 2007, 221, 609–616. [Google Scholar] [CrossRef]
- Mahmud, S.; Fraser, R.A. Mixed convection-radiation interaction in a vertical porous channel: Entropy generation. Energy 2003, 28, 1557–1577. [Google Scholar] [CrossRef]
- Tasnim, S.M.; Mahmud, S.; Mamum, M.A.H. Entropy generation in a porous channel with hydromagetic effect. Int. J. Exergy 2002, 3, 300–308. [Google Scholar] [CrossRef]
- Chauhan, D.S.; Kumar, V. Heat transfer and entropy generation during compressible fluid flow in a channel partially filled with porous medium. Int. J. Energy Tech. 2011, 3, 1–10. [Google Scholar]
- Eegunjobi, A.S.; Makinde, O.D. Combined effect of buoyancy force and Navier slip on entropy generation in a vertical porous channel. Entropy 2012, 33, 692–698. [Google Scholar] [CrossRef]
- Chen, S.; Liu, Z.H.; Bao, S.; Zheng, C.G. Natural convection and entropy generation in a vertically concentric annular space. Int. J. Therm. Sci. 2010, 49, 2439–2452. [Google Scholar] [CrossRef]
- Chen, S. Entropy generation of double-diffusive convection in the presence of rotation. Appl. Math. Comput. 2011, 217, 8575–8597. [Google Scholar] [CrossRef]
- Cebeci, T.; Bradshaw, P. Physical and Computational Aspects of Convective Heat Transfer; Springer: New York, NY, USA, 1988. [Google Scholar]
© 2013 by the authors; licensee Molecular Diversity Preservation International, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Makinde, O.D.; Eegunjobi, A.S. Entropy Generation in a Couple Stress Fluid Flow Through a Vertical Channel Filled with Saturated Porous Media. Entropy 2013, 15, 4589-4606. https://doi.org/10.3390/e15114589
Makinde OD, Eegunjobi AS. Entropy Generation in a Couple Stress Fluid Flow Through a Vertical Channel Filled with Saturated Porous Media. Entropy. 2013; 15(11):4589-4606. https://doi.org/10.3390/e15114589
Chicago/Turabian StyleMakinde, Oluwole Daniel, and Adetayo Samuel Eegunjobi. 2013. "Entropy Generation in a Couple Stress Fluid Flow Through a Vertical Channel Filled with Saturated Porous Media" Entropy 15, no. 11: 4589-4606. https://doi.org/10.3390/e15114589




