On the Entropy of a Class of Irreversible Processes

Abstract

We review a recent technique for determining the entropy change accompanying certain classes of irreversible processes involving changes in the state of a system anchored to a reservoir. Time is introduced as a parameter to specify the corresponding entropy evolution of the system. The procedural details are outlined and their relation to the standard treatment of irreversible processes is examined.

1. Introduction

1.1. Generalities

This paper presents a review of recent work on time-dependent irreversible processes that differ from more conventional methodologies. It is hoped thereby to provide a more unified approach to topics that usually are treated as disparate subjects.

Thermodynamics is ordinarily applied to systems at equilibrium and subject to reversible processes. However, while considering the Second Law one often confronts the necessity of introducing irreversible processes that follow non-equilibrium paths. This ordinarily requires the introduction of a Taylor series expansion about the quiescent state, to approximate the properties of systems away from equilibrium. Necessarily then, the departures from equilibrium must be “small”. The extent to which such departures can be applied to characterize any situation can only be decided by experiment. In recent times this approach has been extended to encompass more drastic departures from equilibrium, an approach that comes under the heading of extended nonequilibrium thermodynamics.

Here we review a different approach to irreversible processes entirely within the realm of classical thermodynamics, and thus amenable to simple mathematical operations. For a certain class of processes enumerated below we allow the entire system to follow paths well removed from equilibrium.

The present discussion has many historical antecedents. The extension of thermodynamics to nonequilibrium states dates back to Clausius (1850–1865), who first introduced the concept of “uncompensated transformations” for the difference between entropy changes accompanying irreversible as opposed to reversible processes. A similar characterization was developed by Natanson in 1896 [1]. Jaumann and Lohr (1911–1918) [2,3,4] were among the first to develop a microscopic theory of irreversible thermodynamics, in which they linked the flow of matter, energy, entropy, chemical species and other dissipative processes to the presence of generalized forces. The resulting transport theory leads to fundamental laws discovered earlier through empirical observation: Fourier’s law, Fick’s Law, Ohm’s law, and so on. Later developments, principally by Meixner [5], de Groot & Mazur [6], Prigogine [7], Haase [8] and their schools then led to the development of phenomenological equations governing several classes of irreversible phenomena. In Extended Irreversible Thermodynamics the assumption of local equilibrium has been abandoned and replaced by the use of fluxes as the fundamental state variables, whence the linear phenomenological equations have been superseded by first order time evolution equations. Thereby memory, nonlinear and nonlocal effects have been successfully incorporated into a coherent description of such nonlinear phenomena. Detailed expositions are provided in References [9,10,11,12,13,14].

The subsequent discussion has its origins in the work of De Donder [15] who related “non-compensated heat” in a chemical reaction directly to the corresponding affinity function. Later work on time-dependent irreversible processes has been reviewed by Bejan [16] and was brought up to date in a review by Lucia and Grazzini [17,18]. As emphasized in both publications, time must be introduced as an important variable in the specification of irreversible processes. This view has been adopted in the present approach, with emphasis placed on the time evolution of irreversible processes rather than on setting up time-dependent differential equations and optimization of available work in engineering applications, which has been the goal of the majority of publications in the area. The publications that come closest to the present approach are probably those of Salamon, Band, and Kafri [19], Band, Kafri, and Salamon, [20], Ondrechen, Rubin, and Band [21], who sketched the use of time as a parameter in specifying the course of an irreversible process, again, with an emphasis on optimization of available work.

1.2. Basic Assumptions

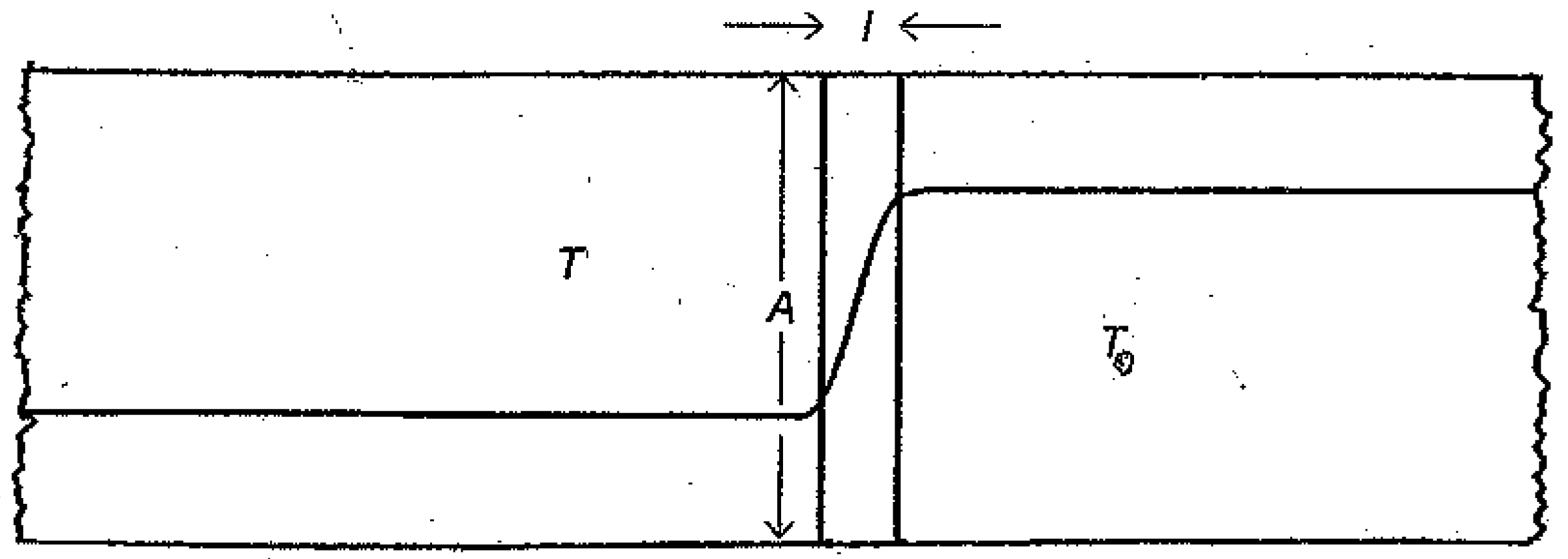
We now consider an alternative approach to irreversible processes by examining a system in contact with a reservoir, the compound unit being isolated from the rest of the universe. A sketch of the isolated unit is shown in Figure 1. The reservoir is assumed to be large in extent and maintained well mixed so that any change in its configuration occurs reversibly while maintaining all intensive variables constant, even in its interactions with the system undergoing irreversible changes. This assumption, commonly employed, is crucial, so as to maintain a simple mathematical framework for later developments. The reservoir is linked to the system via an intervening moveable junction that is a poor thermal conductor, also allowing for the slow diffusion of chemical species between the compartments. As indicated in the diagram, the temperature T, pressure P, and chemical potential μ of the system differ from the corresponding T₀, P₀, μ₀ of the reservoir. During the interaction the system is assumed to undergo slow physical and chemical changes, so as to allow for a uniform change in all its properties. This process will be designated as a quasistatic irreversible process (QSIP). Thus, while the temperature T₀ of the reservoir remains fixed, the temperature T of the system is forced to change sufficiently slowly so as to keep T essentially uniform over almost the entire volume of the system. The temperature change then occurs solely within the boundary region. Similar considerations apply to other intensive variables. The two compartments are thus not in equilibrium with each other; instead, one can maintain arbitrarily large differences in intensive variables. We specify the resulting thermodynamic relations under such conditions and determine the operating characteristics in terms of thermodynamic equilibrium variables. These idealizations may be approached by choosing a system that has a fast relaxation time, and is small in the lateral extension away from the junction. Alternatively, one may consider a set of small subsystems dispersed within the reservoir that undergo identical changes simultaneously.

Figure 1. Temperature Profile for a System at Temperature T Attached to a Reservoir at Temperature T₀ via a Junction of Cross Section A and Length l.

Figure 1. Temperature Profile for a System at Temperature T Attached to a Reservoir at Temperature T₀ via a Junction of Cross Section A and Length l.

Under the above assumptions the temperature of relevance is always that of the reservoir and is thus well defined. If one wishes to relax the assumption of a uniform temperature distribution in the system one needs to consider the extent to which the concept of temperature remains applicable for large departures of the system from equilibrium. This matter has been addressed in several studies [22,23,24], where it is shown that the concept of temperature remains relevant and does not depart significantly from the temperature of the applicable equilibrium state. This in principle allows one to investigate cases where the temperature of the system is no longer uniform. The resulting complications are not addressed here.

1.3. Fundamentals

Before proceeding it is important to go back to fundamentals involving the concept of entropy S. Conventionally, following Clausius, one posits that in an incremental heat transfer đQ the incremental change in entropy is subject to the relation dS ≥ đQ/T. Similarly, for cyclic processes one encounters the inequality in the form ${\oint }^{\text{}}\mathsf{đ}Q/T$$\le 0.$ This introduces several difficulties. It is troublesome to manipulate inequalities in any subsequent mathematical operations, and the existence of a bound furnishes no information on the actual value that prevails. Furthermore, state functions in differential form such as dE = TdS − PdV apply solely to reversible processes. Here we generalize these functions so as to render them applicable to QSIPs, and we also recover several well established results in a unified approach.

1.4. Entropy Changes during Processes

We begin a study of entropy changes of QSIPs in the isolated compound system. Consider a system that in its interactions with a reservoir undergoes a reversible change from state A to state B, at a uniform temperature T. The entropy change of the compound system in an incremental step is specified by:

d_aS_u ≡ dS(T,V,n_i) + d_aS₀(T,V₀,n_0i) = 0

(1)

where conventional notation was employed; the zero subscript refers to the reservoir. Now let the same step be executed irreversibly, with the two components at different temperatures; normally this is recorded via the inequality:

d_bS_u ≡ dS(T,V,n_i) + d_bS₀(T₀,V₀,n_0i) > 0

(2)

To eliminate the inequality we introduce a deficit function, commonly known as the entropy dissipation function, đθ > 0, such that:

d_bS_u ≡ dS(T,V,n_i) + d_bS₀(T₀,V₀,n_0i) − đθ = 0

(3)

So far đθ is simply a bookkeeping device, but it acquires meaning when we subtract Equation (1) from Equation (3) to write:

d_bS₀(T₀,V₀,n_0i) - d_aS₀(T,V₀,n_0i) = đθ

(4)

whence đθ represents the difference in entropy change of the surroundings when the system is allowed to undergo the same process irreversibly as opposed to reversibly.

In the isolated compound unit all of the heat absorbed (released) by the system is transferred out of (into) the reservoir. Thus, during the reversible (r) operation the entropy changes of the system and surroundings are related by:

dS ≡ d_rS = đ_rQ/T = −d_aS₀

(5)

while the irreversible (i) operation entails an entropy change:

d_bS₀ = đ_rQ₀/T₀ = − đ_iQ/T₀

(6)

where we invoked the reversibility of all processes in the reservoir. On inserting Equations (5) and (6) into Equation (3) we obtain a relation between heat transfers during the reversible and irreversible operations:

đ_iQ = (T₀/T) đ_rQ − T₀đθ = T₀ (dS – đθ) < (T₀/T)đ_rQ

(7)

or in alternative form as:

đ_iQ = đ_rQ + (T₀/T − 1) đ_rQ − T₀đθ

(8)

Equation (7) duplicates the expression derived by Tolman and Fine (1948) [25] by a different method.

For heat flow into the system đ_iQ and đ_rQ are both positive and T < T₀. The inequality in Equation (7) must hold even for the limiting case T → T₀⁻. This leads directly to the inequality set:

đ_iQ < đ_rQ < (T₀/T)đ_rQ

(9)

which represents one statement of the Clausius inequality. For the reverse flow both đ_iQ and đ_rQ are negative and T > T₀; then Equation (9) represents a string of increasingly less negative quantities.

Equation (7) may be turned around to read:

dS = đ_iQ/T₀ + đθ ≥ đ_iQ/T₀

(10)

which shows explicitly that in irreversible processes the total entropy change in the system is only partially accounted for by the transfer of heat. Note that it is the well established temperature of the reservoir that enters the expression. For adiabatic processes in isolation, δS = đθ > 0; entropy can only increase, a process that continues until quiescence is reached, at which stage the entropy is at a maximum with respect to the applied constraints. All this obviously is in accord with well-established principles.

If we apply the đ_iQ < đ_rQ inequality to Equation (8) we require the last two terms on the right to be negative, so that:

đθ > (T₀/T – 1) đ_rQ/T₀ > 0

(11)

where the lower bound is always positive, since for T/T₀ < 1, đ_rQ > 0, and for T/T₀ > 1, đ_rQ < 0. Thus, Equation (11) yields a lower positive bound on đθ.

The performance of work W may be analyzed on the basis of the First Law in the formulation:

dE = đ_rQ + đ_rW = đ_iQ + đ_iW

(12)

On substituting from Equations (7) or (8) we find that:

đ_iW = đ_rW − (T₀/T − 1) đ_rQ + T₀đθ = đ_rW − (T₀ – T)dS + T₀đθ

(13)

Equation (13) represents a variant of the Gouy-Stodola theorem.

Comparison with Equations (8) and (11) shows that đ_iW > đ_rW, which is another well established principle.

We may solve Equation (13) for:

đθ = (đ_iW − đ_rW)/T₀ + (1 – T/T₀)dS.

(14)

This finally provides one method for determining đθ through measurements of work performance for the same process under actual as opposed to reversible operating conditions; also needed are measurements or calculations of the entropy change under those conditions.

In the absence of work performance Equation (14) reduces to:

đθ = (1/T – 1/T₀) đ_rQ

(15)

which was derived elsewhere by Kestin by different means [26]. The first term in Equation (14) was obtained Bejan who employed a different approach [27,28]. Equation (14) further provides a thermodynamic background for the theoretical work of Jarzynski [29] who related the average of repeated executions of irreversible microscopic processes to thermodynamic equilibrium processes. That work was confined to processes executed at constant temperature; the above relation shows how this analysis may be extended.

We next set up functions of state that incorporate irreversible processes; these can then be used to specify đθ for processes under a variety of operating conditions.

1.5. The Energy

We begin by applying the First Law to the operation of the reservoir, in its interactions with the system that involve heat exchange, mechanical work, and transfer of matter. For the corresponding reversible operation we write in conventional notation:

dE₀ = T₀dS₀ − P₀dV₀ + Σ_iμ_0idn_0i

(16)

for the energy of the surroundings. The energy of the system is found by noting that in the isolated compound unit dE + dE₀ = 0. Further, when the systems undergoes irreversible processes we use Equation (3) to replace dS­₀ in Equation (16) by d_bS₀ = −dS + đθ. On the assumption that the isolated compound system is maintained at constant volume and fixed composition we may also set dV₀ = −dV, and dn_0i = −dn_i, so that the energy differential of the system is given as:

dE = T₀dS − P₀dV + Σ_iμ_0idn_i − T₀đθ

(17)

This relation, involving different arguments, was derived by Kestin [26]. The intensive variables are those of the surroundings and are therefore well defined, even under irreversible processes within the system; dS, dV, dn_i are the control variables; đθ may be specified by Equation (14), or by expressions developed shortly, or by techniques reviewed below. Thus, dE is well defined for QSIPs. If work other than mechanical is involved, relevant terms must be added to Equation (17) as a product of intensive variables appropriate to the relevant work reservoir, and corresponding extensive variables for the system proper.

It is instructive to rewrite Equation (17) in the form:

dE = (T₀ – T)dS − (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i + TdS − PdV + Σ_iμ_idn_i − T₀đθ

(18)

where the variables lacking the subscript zero refer to the properties of the system. Under reversible operations the first three terms and the last term drop out, and one recovers the conventional formulation. Since E is a function of state we next invoke the relation dE = TdS − PdV_. + Σ_iμ_idn_i for reversible operations, and subtract this relation from Equation (18), which allows us to solve for:

T₀đθ = (T₀ – T)dS − (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i

(19)

The quantities on the right are experimentally accessible; hence, the above equation permits the determination of đθ in terms of S, V, and n_i as the control variables. The above relation is not immediately useful since the specification of dE involves the use of the state function dS as a control variable. This problem is addressed as follows:

1.6. The Helmholtz Free Energy

To construct the Helmholtz energy we introduce the definition A = E – TS, which sensibly involves the temperature T of the system. This transforms the independent variable from S to T. Based on Equation (18) we find that:

dA = (T₀ – T)dS – (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i – SdT – PdV + Σ_iμ_idn_i – T₀đθ.

(20)

This must be rewritten in terms of T, V, n_i as the applicable control variables. For this purpose we now express entropy in terms of these quantities: S = S(T,V,n_i), with the differential form:

dS = (∂S/∂T)_V,ni dT + (∂S/∂V)_T,ni dV + Σ_i(∂S/∂n_i)_T,V,ni≠j dn_i

(21)

Substitution in Equation (20) then yields:

dA = (T₀ – T)[(∂S/∂T)_V,nidT + (∂S/∂V)_T,nidV + Σ_i(∂S/∂n_i)_T,V,ni≠jdn_i] − (P₀ – P)dV
+ Σ_i(μ_0i – μ_i)dn_i − SdT - PdV + Σ_iμ_idn_i – T₀đθ

(20)

We now introduce the replacements (∂S/∂T)_V,ni = C_V/T, and (∂S/∂V)_T,ni = (∂P/∂T)_V,ni, where C_V is the heat capacity at constant volume and composition, and where the appropriate Maxwell relation has been introduced.

Also, we use the mathematical identity ${\left(\partial P/\partial T\right)}_{V,n_i}=-{\left(\partial V/\partial T\right)}_{P,n_i}/{\left(\partial V/\partial P\right)}_{T,n_i}$ and then replace the numerator and denominator by - αV and by - βV, where α and β are the isobaric coefficient of expansion and the isothermal compressibility respectively. We also set ${\left(\partial S/\partial n_i\right)}_{T,V,n_{j\ne i}}\equiv {\widehat{S}}_i$ as the differential entropy at constant temperature, volume and mole number of species j ≠ i. Then:

dA = (T₀ – T)[(CV/T)dT + (α/β) dV + Σ_i dn_i] – (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i – SdT – PdV + Σ_iμ_idn_i – T₀đθ

(21)

which relates the differential of the Helmholtz function under non-equilibrium conditions to changes in T, V, and the n_i. The associated coefficients involve measurable quantities. Again, under reversible operations dA = - SdT - PdV + Σ_iμ_idn; when this relation is subtracted from (23) we may solve for:

T₀đθ = (T₀ – T)[(C_V/T)dT + (α/β) dV + Σ_i dn_i] - (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i

(22)

which specifies đθ in terms of T, V, and n_i; all the coefficients involve measurable quantities.

1.7. The Gibbs Free Energy

Using by now familiar methodology, we define the Gibbs energy by the relation G = E – TS + PV. When converted to differential form we write:

dG = (T₀ – T)dS − (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i − SdT + VdP + Σ_iμ_idn_i – T₀đθ

(23)

for use under non-equilibrium conditions. To introduce the proper control variables we express the entropy function as S = S(T,P,n_i) and the volume as V = V(T,P,n_i), with corresponding differentials for dS and dV. On inserting these in Equation (25) we obtain:

dG = (T₀ – T)[(∂S/∂T)_P,nidT + (∂S/∂P)_T,nidP + Σ_i(∂S/∂n_i)_T,P,ni≠jdn_i] – (P₀ – P)[(∂V/∂T)_P,nidT + (∂V/∂P)_T,nidP + Σ_i(∂V/∂n_i)_T,P,ni≠jdn_i] + Σ_i(μ_0i – μ_i)dn_i – SdT + VdP + Σ_iμ_idn_i – T₀đθ

(24)

We next set (∂S/∂T)_P,ni = C_P/T, introduce the Maxwell relation (∂S/∂P)_T,ni = - (∂V/∂T)_P,ni and. replace the partial derivatives - (∂V/∂P)_T,ni and (∂V/∂T)_P,ni by βV and by αV respectively. Here (∂S/∂n_i)_T,P,ni≠j and (∂V/∂n_i)_T,P,ni≠j represent partial molal entropies and volumes . We then rewrite Equation (26) in the less unwieldy form:

dG = (T₀ – T)[(C_P/T)dT – αVdP + Σ_i dn_i] – (P₀ – P)[ αVdT – βVdP + Σ_i dn_i] + Σ_i(μ_0i – μ_i)dn_i – SdT + VdP + Σ_iμ_idn_i – T₀đθ

(25)

for the Gibbs function under non-equilibrium conditions. Then subtract the standard reversible form dG = – SdT + VdP + Σ_iμ_idn_i and solve for:

T₀đθ = (T₀ – T)[(C_P/T)dT – αVdP + Σ_i dn_i] – (P₀ – P)[αVdT - βVdP + Σ_i dn_i] + Σ_i(μ_0i – μ_i)dn_i

(26)

which expresses đθ in terms of T, P, and composition.

1.8. The Enthalpy

Lastly, we turn to the enthalpy H = E + PV. By the customary technique we develop the differential form dH = dE + PdV + VdP and then insert Equation (18) to obtain:

dH = (T₀ – T)dS – (P₀ – P)dV + Σ_i(μ_0i – μ_i)dn_i + TdS + VdP + Σ_iμ_idn_i – T₀ đθ

(27)

Here S, P, and composition are the applicable control variables. We therefore consider the volume first in the form V = V(P,T,n_i). We next introduce the entropy as a function of the same variables: S = S(P,T.n_i), which function we invert to read T = T(S,P,n_i). Lastly, we insert this expression into the equation of state: thus, V = V(P,T(S,P,n_i),n_i) ≡ V(S,P,n_i). Then:

dV = (∂V/∂S)_P,ni dS + (∂V/∂P)_S,nidP + (∂V/∂n_i)_S,P,ni≠jdn_i

(28)

Now we introduce Equation (30) into Equation (29) to obtain:

dH = (T₀ – T)dS − (P₀ – P)[(∂V/∂S)_P,nidS + (∂V/∂P)_S,nidP + (∂V/∂n_i)_S,P,ni≠jdn_i] + Σ_i(μ_0i – μ_i)dn_i + TdS + VdP + Σ_iμ_idn_i - T₀đθ (31)

(29)

for the infinitesimal enthalpy change under nonequilibrium conditions. Finally, subtract the standard reversible form dH = TdS + VdP + Σ_iμ_idn_i and solve for:

T₀đθ = (T₀ – T)dS − (P₀ – P)[(∂V/∂S)_P,nidS + (∂V/∂P)_S,nidP + Σi(∂V/∂n_i)_S,P,ni≠jdn_i] + Σ_i(μ_0i – μ_i)dn

(30)

which yields the deficit function in terms of S, P, and n_i.

This completes the task of specifying đθ in terms of the applicable control variables.

2. Results and Discussion

2.1. Determination of Entropy Dissipation (Deficit) Functions

We are now faced with the prospect of integrating đθ. This step has been executed in References [30,31,32,33,34,35] under a variety of assumed operating conditions. Here we summarize the methodology and the final results; for details, please consult the above- mentioned papers. We take as an example Equation (24), which we specialize to the constant volume and single component case, whereby:

đθ = đθ_T + đθ_n = (1 – T/ T₀)[(C_V/T)dT ] + [(1 – T/ T₀) + (μ₀/T₀) (1 – μ /μ₀)]dn = (1 – T/ T₀)[(C_V/T)dT ] + [ + μ₀/T₀ - /T₀]dn

(31)

where the accented terms represent differential quantities; similar expressions, with zero subscripts, apply to the reservoir. We set $\widehat{A}\equiv \mu =\widehat{E}-T\widehat{S}.$ The first task is to specify all the variables in Equation (33) via the equation of state P = P(T,V,n).

As an example of the procedure, let us adopt the Berthelot equation of state:

$$P(T,V,n)=nRT/V+n^{2}RTb/V^2-n^{2}a/V^{2}T$$

(32)

where a and b are materials-specific parameters. The energy E is obtained by integration of the caloric equation of state $(\partial E/\partial V)=T(\partial P/\partial T)-P,$

$$E(T,V,n)=3nRT/2-2n^{2}a/VT+E(T_i,V,n_i)$$

(33)

where T_i and n_i refer to the initial values of the temperature and mole numbers for the system.

Then:

$$C_V=\partial E/\partial T=3nR/2-4na/VT$$

(34)

and:

$$S(T,V,n)={\displaystyle \int (C_V/T)dT}=\frac{3}{2}nR\ln \frac{T}{T_i}-\frac{n^{2}a}{V}\left[\frac{1}{T^2}-\frac{1}{{T_i}^2}\right]+S(T_i,V,n_i)$$

(35)

The functions $\widehat{S},\widehat{E},{\mu }_0=\widehat{E}-T\widehat{S}$ for subsequent use are found by differentiation with respect to n.

On substitution in Equation (33) and integrating, one may obtain first the contribution associated with temperature variations, namely:

$${\theta }_T={\displaystyle \int (3nR/2T)dT-{\displaystyle \int (3nR/2T_0)dT+{\displaystyle \int (2n^{2}a/V{T}^3)dT-}}}{\displaystyle \int (2n^{2}a/V{T}^2{T}_0)dT}$$

(36)

The contribution involving the transfer of matter is found via the second bracketed term in Equation (33) as:

$${\theta }_n= {{\displaystyle \int }}^{\text{}}\left[\frac{3R}{ 2}\ln \frac{T}{T_i}- \frac{3RT}{2T_0}- \frac{2na}{V} \left(\frac{1}{T^2}- \frac{1}{T_i^2}- \frac{2}{T{T}_0}\right) + \frac{{\mu }_0}{T_0} – \frac{\widehat{E}\left(T_i,V,n_i\right)}{T_0} + \widehat{S}\left(T_i,V,n_i\right)\right]dn$$

(37)

In what follows we temporarily leave aside the contribution from the last three terms in Equation (39) that remain constant in the integration.

2.2. Use of Time as a Parameter

This completes the first step. The second consists in introducing time t as a parameter to simulate the path to be followed by the system in proceeding from the initial configuration i to the final configuration f. We thus need first to supply the dependence of T and n on t, and then insert these is functions into Equations (38) and (39) so as to execute the requisite integrations.

Explicitly, the authors of Reference [35] adopted three variations of T and n with t:

(i)

$T=T_i\exp (k_{T}t),T_f/T_i=\exp (k_T\tau ),n=n_i\exp (k_{n}t),n_f/n_i=\exp (k_n\tau );k_T/k_n=\ln (T_f/T_i)/\ln (n_f/n_i),V=const,$

(ii)

$T=T_i\exp (k_{T}t),T_f/T_i=\exp (k_T\tau ),n=n_i(1+k_{n}t),n_f/n_i=1+k_n\tau ,k_T/k_n=\ln (T_f/T)/(n_f/n_i-1),V=const.$

(iii)

$T=T_i(1+k_{T}t),T_f/T_i=(1+k_T\tau ),n=n_i(1+k_{n}t),n_f/n_i=1+k_n\tau ,k_T/k_n=(T_f/T_i-1)/(n_f/n_i-1),V=const.$

The choice of time variations is, of course, arbitrary, but guidance is provided by the constructal law proposed by Bejan [27] that deals with the flow architecture so as to enable successively simpler channels to develop. In the above work the choice involved functions that were analytically tractable and that served as representative examples.

We can now specify the rate of entropy production associated with the irreversible transfer of heat and material from the reservoir to the system under the specified conditions. Let us adopt condition (ii) for insertion in Equations (38) and (39). Disregarding the last three terms in Equation (39) that are constants we find that:

$${\dot{\theta }}_T=\frac{3n_{i}R}{2T_i}(1+k_{n}t)\left[e^{-k_{T}t}-\frac{T_i}{T_0}\right]+\frac{2n_i^{2}a}{V}\frac{e^{-2k_{t}t}}{T_0{T}_i^2}\left[\frac{T_0}{T_i}{e}^{-k_{T}t}-1\right]{\left(1+k_{n}t\right)}^2$$

(38)

$${\dot{\theta }}_n=\frac{3R}{2}\left[k_{T}t-\frac{T_i}{T_0}{e}^{k_{T}t}\right]-\frac{2a{n}_i}{T_0{T}_{i}V}\left(1+k_{n}t\right)\left[\frac{T_0}{T_i}(e^{-2k_{T}t}-1)-2e^{-k_{t}t}\right]$$

(39)

The above results are of intrinsic interest as they specify the time development of the entropy evolution associated with the irreversible process.

The equations may now be integrated with integration limits from initial time t_i = 0 to final time t_f = τ. After integration one encounters k_Tτ and k_nτ as parameters in the resulting functions θ_T and θ_n. These parameters are then replaced by ln (T_f /T_i) and by ln (n_{f /} n_i). Also present are functions that display the ratios k_T/k_n; these are replaced as shown in (i)–(iii). The requisite integrations are straightforward, though lengthy and very tedious, and will not be indicated here.

2.3. Results

The upshot of the above operations is the finding that while the contribution to θ_T and θ_n such as Equations (40) and (41) differ for each of the above three paths, their sum, θ_T + θ_n does not.

Thus, for all three of the above cases the integrations lead to the following results: The total entropy change due to the ideal gas law contribution is specified by:

$${\theta }_{ig}=\frac{3R}{2}{n}_f\ln \left(\frac{T_f}{T_i}\right)-\frac{3R}{2T_0}(n_f{T}_f-n_i{T}_i)$$

(40)

The contribution arising from the Berthelot corrections from ideality reads:

$$\begin{array}{l}{\theta }_{Bg}=\frac{a}{V}{n}_f^2\left(\frac{1}{T_i^2}-\frac{1}{T_f^2}\right)+\frac{2a}{V{T}_0}\left(\frac{n_f^2}{T_i^2}-\frac{n_i^2}{T_f^2}\right)\\ \end{array}$$

(41)

We finally consider the three terms in Equation (39) that have been neglected so far. Here n is the only variable quantity. The corresponding entropy change is thus specified by:

$$\begin{array}{l}{\theta }_n^0=\left[{\mu }_0/T_0-\text{\hspace{0.05em}\hspace{0.05em}}\widehat{E}(T_i,V,n_i)/T_0+\widehat{S}(T_i,V,n_i)\right](n_f-n_i)\\ =[{\mu }_0/T_0-{\mu }_i/T_i+(1-T_i/T_0)\widehat{S}(T_i,V,n_i)](n_f-n_i)\end{array}$$

(42)

where we had set $\widehat{E}(T_i,V,n_i)-T_i\widehat{S}(T_i,V,n_i)={\mu }_i(T_i,V,n_i).$ Since the material in the system and reservoir is identical, both μ and μ₀ refer to the same reference energy, commonly chosen to be the ideal gas at their respective temperatures. Thus, on setting μ = μ^*(T_i) + RT ln P_i, as well as μ₀ = μ^*(T₀) + RT₀ ln P₀, we see that:

$${\theta }_n^0=\{[\mu *(T_0)-\mu *(T_i)]/T_0+R[\ln P_0-(T_i/T_0)\ln P_i]+(1-T_i/T_0)\widehat{S}(T_i,V,n_i)\}(n_f-n_i)$$

(43)

where the requisite pressures are calculated from the Berthelot equation of state; $\mu *(T_0)$ and $\mu *(T_i)$ are found by employing relevant formulae from statistical mechanics. On summing Equations (43)–(45) we obtain a description of the QSIP for the case under study.

More generally, for a given system the above procedure allows one to specify the entropy changes associated with the transfer of heat or work for any particular time dependence of choice, in the manner documented in References [30,31,32,33,34,35]. This is of particular relevance in engineering applications.

2.4. Comments

The above is a prototype example on how to proceed. Analogous conclusions obtain when different starting assumptions are introduced. These have been investigated in several publications: [26,27,28,29,30,31]: condensed phases instead of gases; the use of pressure rather than volume as a control parameter; the introduction of other functions of time. In all cases the intermediate θ functions do depend on the chosen path, but their sum does not. This should not come as a surprise: Equation (4) shows that đθ represents the difference of two functions of state. The fact that the path independence of the total entropy change was always encountered serves as a warrant for the correctness of the approach. On the other hand, this also indicates the limitations of the QSIP approach to deal with only a restricted class of irreversible processes. In principle the procedure can be extended as stated earlier.

3. Relation to Standard Formulation of Irreversible Processes

We now draw attention to the relation of the above methodology to standard procedures for analyzing irreversible phenomena (Meixner [5]; de Groot and Mazur [6]; Prigogine [7]; Gyarmati [36]; Haase [8]; Mueller and Ruggeri [12]; Kondepudi and Prigogine [37]). As is well established, one proceeds by identifying the applicable conjugate forces and fluxes and then setting up linear phenomenological equations in which every force contributes to every flux, subject to the Onsager reciprocity conditions. The equations are then solved by introducing a variety of steady state conditions, so as to identify the various phenomenological coefficients in terms of measurable parameters. The equation of continuity then provides a method of identifying the flux associated with the transfer of entropy, as well as the entropy production within the system.

The present procedure leads to an alternative method for specifying the entropy change associated with any given QSIP path. It is nevertheless possible to link this approach to standard methodology. Toward that end we rewrite Equation (24) under constant volume conditions as:

(44)

Now take derivatives with respect to time for an infinitesimal step, to write:

(45)

Then introduce the entropy density, ϑ, where for a junction of cross section A and length l:

(46)

In the limit of a very thin junction, and on adopting the standard formulation for entropy flux J_S and particle flux, J_n, we obtain (▽ is the gradient operator):

(47)

This expression is the standard formulation for the entropy density flux in terms of the conjugate force-flux pairs. Thus, the present formulation is seen to be a variant of the ordinary treatment of irreversible thermodynamics procedure, and is therefore subject to the extremum and variational principles and to Hamilton’s principle, discussed by Gyarmati [36].

4. Conclusions

In the first part of this paper we indicated how the standard thermodynamic functions of state may be generalized so as to become applicable to a certain class of irreversible phenomena, termed QSIPs. This approach permits one to determine the contribution of such irreversible processes to the entropy of the systems and surroundings, thereby bypassing the conventional use of inequalities. Standard results, such as the Clausius relation between heat transfer and entropy change, the Gouy-Stodola theorem, or the relation between heat tranferrred reversibly vs. irreversibly, are an automatic byproduct of this approach.

The actual determination of irreversible entropy changes is carried out by specifying how the temperature, pressure or volume, and composition of the system changes with time, while anchored to a reservoir of immense size, so that its intensive variables remain constant. As usual, it is assumed that the reservoir operates reversibly. The requisite integrations of the differential forms are then performed, thus permitting a study of the time variation of entropy changes associated with different irreversible processes of the system. Characteristically for QSIPs, while entropy changes associated with heat transfer and work performance do individually depend on the chosen time dependence, the total entropy change is independent of such a choice.

Finally, it is shown by an example how the present procedure is related to the standard procedure of handling thermodynamic processes via linear phenomenological equations.