Effects Induced by the Initial Condition in the Quantum Kibble–Zurek Scaling for Changing the Symmetry-Breaking Field
Abstract
:1. Introduction
2. Kibble–Zurek Scaling and Its Generalization
3. Effects Induced by the Initial Condition in the Kibble–Zurek Scaling for Changing the Symmetry-Breaking Field
4. Model and Numerical Method
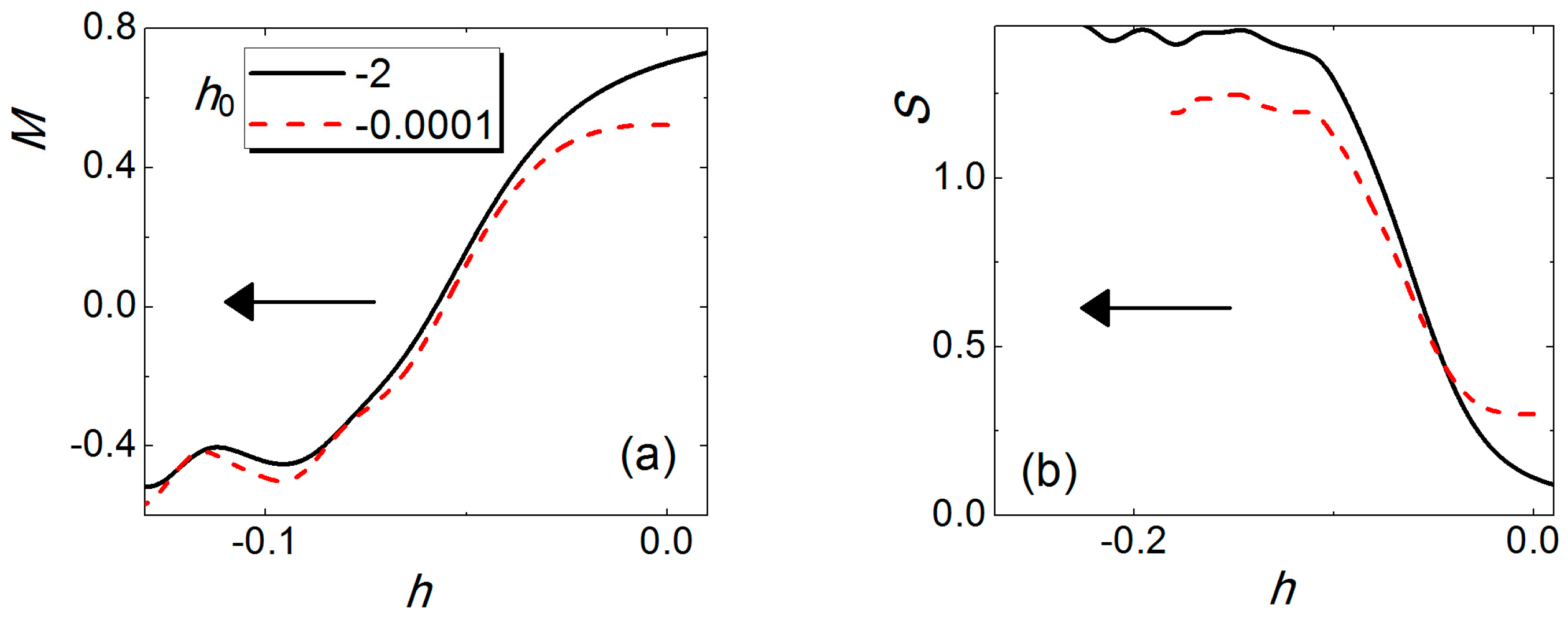
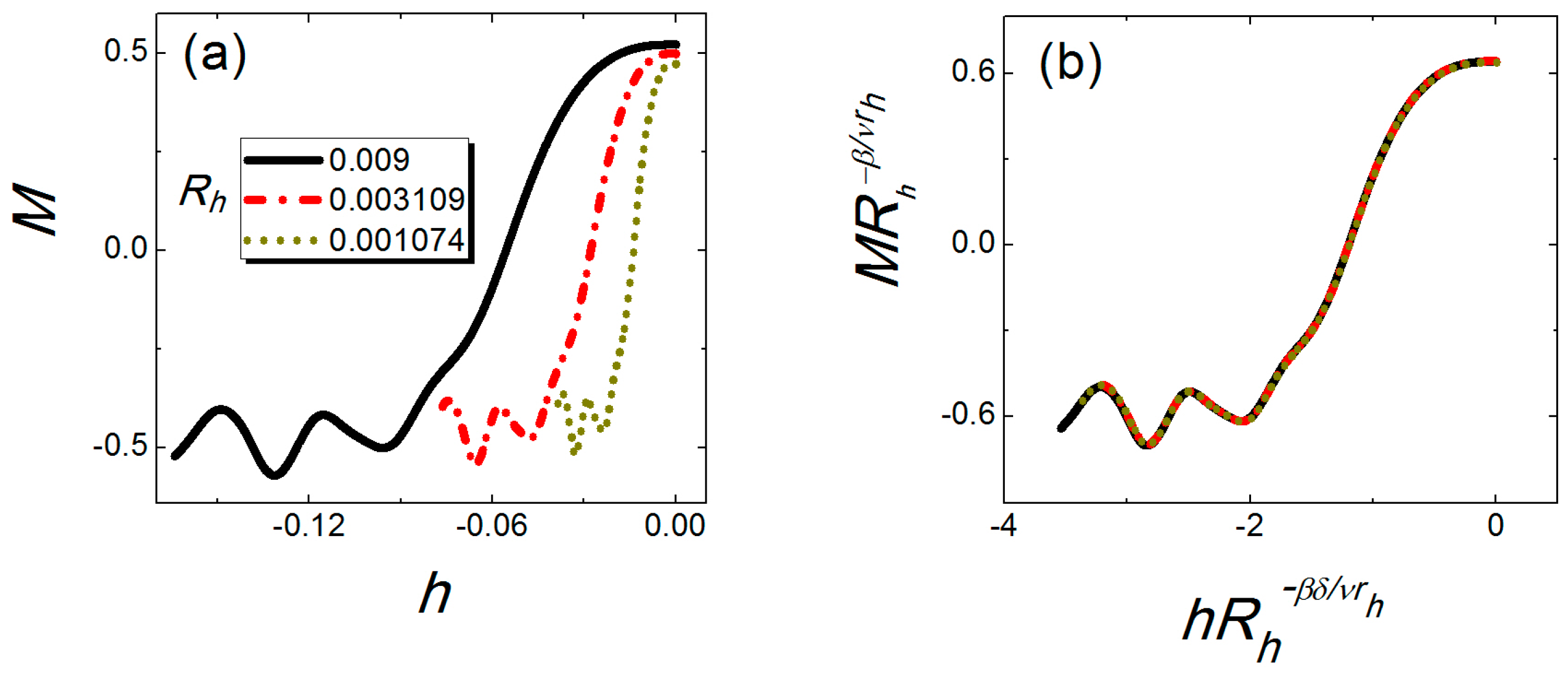
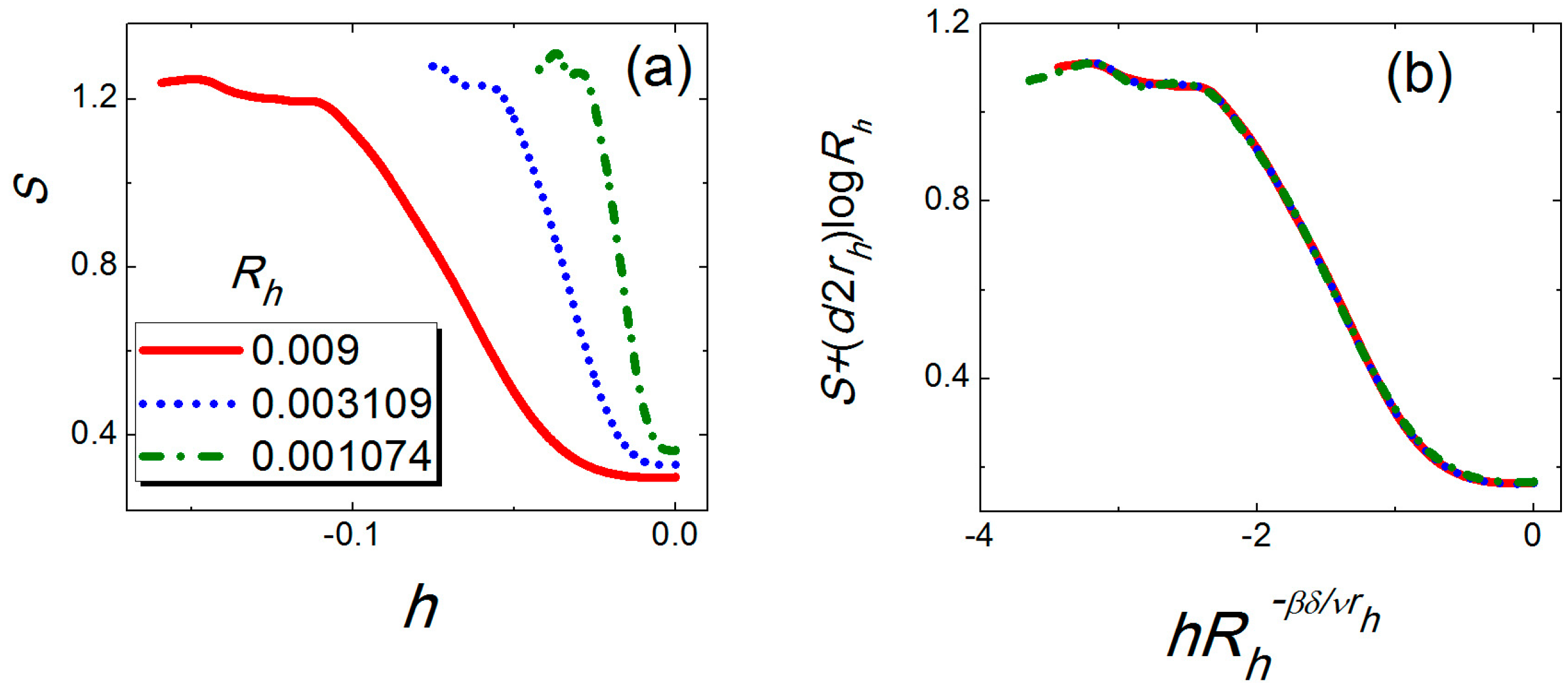
5. Numerical Results
6. Summary and Discussion
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Dziarmaga, J. Dynamics of a quantum phase transition and relaxation to a steady state. Adv. Phys. 2010, 59, 1063–1189. [Google Scholar] [CrossRef]
- Polkovnikov, A.; Sengupta, K.; Silva, A.; Vengalattore, M. Colloquium: Nonequilibrium dynamics of closed interacting quantum systems. Rev. Mod. Phys. 2011, 83, 863–883. [Google Scholar] [CrossRef]
- Dutta, A.; Aeppli, G.; Chakrabarti, B.K.; Divakaran, U.; Rosenbaum, T.F.; Sen, D. Quantum Phase Transitions in Transverse Field Spin Models: From Statistical Physics to Quantum Information, 1st ed.; Cambridge University Press: Cambridge, UK, 2015. [Google Scholar]
- Kibble, T.W.B. Topology of cosmic domains and strings. J. Phys. A Math. Gen. 1976, 9, 1387–1398. [Google Scholar] [CrossRef]
- Zurek, W.H. Cosmological experiments in superfluid helium? Nature 1985, 317, 505–508. [Google Scholar] [CrossRef]
- Zurek, W.H.; Dorner, U.; Zoller, P. Dynamics of a Quantum Phase Transition. Phys. Rev. Lett. 2005, 95, 105701. [Google Scholar] [CrossRef] [PubMed]
- Dziarmaga, J. Dynamics of a Quantum Phase Transition: Exact Solution of the Quantum Ising Model. Phys. Rev. Lett. 2005, 95, 245701. [Google Scholar] [CrossRef] [PubMed]
- Polkovnikov, A. Universal adiabatic dynamics in the vicinity of a quantum critical point. Phys. Rev. B 2005, 72, 161201. [Google Scholar] [CrossRef]
- Damski, B.; Zurek, W.H. Dynamics of a Quantum Phase Transition in a Ferromagnetic Bose-Einstein Condensate. Phys. Rev. Lett. 2007, 99, 130402. [Google Scholar] [CrossRef] [PubMed]
- Sen, D.; Sengupta, K.; Mondal, S. Defect Production in Nonlinear Quench across a Quantum Critical Point. Phys. Rev. Lett. 2008, 101, 016806. [Google Scholar] [CrossRef] [PubMed]
- Deng, S.; Ortiz, G.; Viola, L. Dynamical non-ergodic scaling in continuous finite-order quantum phase transitions. Europhys. Lett. 2008, 84, 67008. [Google Scholar] [CrossRef]
- Damski, B.; Zurek, W.H. Soliton Creation during a Bose–Einstein Condensation. Phys. Rev. Lett. 2010, 104, 160404. [Google Scholar] [CrossRef] [PubMed]
- Francuz, A.; Dziarmaga, J.; Gardas, B.; Zurek, W.H. Space and time renormalization in phase transition dynamics. Phys. Rev. B 2016, 93, 075134. [Google Scholar] [CrossRef]
- Antunes, N.D.; Gandra, P.; Rivers, R.J. Is domain formation decided before or after the transition? Phys. Rev. D 2006, 73, 125003. [Google Scholar] [CrossRef]
- Damski, B.; Zurek, W.H. Adiabatic-impulse approximation for avoided level crossings: From phase-transition dynamics to Landau-Zener evolutions and back again. Phys. Rev. A 2006, 73, 063405. [Google Scholar] [CrossRef]
- Del Campo, A.; Zurek, W.H. Universality of phase transition dynamics: Topological defects from symmetry breaking. Int. J. Mod. Phys. A 2014, 29, 1430018. [Google Scholar] [CrossRef]
- Deutschländer, S.; Dillmann, P.; Maret, G.; Keim, P. Kibble–Zurek mechanism in colloidal monolayers. Proc. Natl. Acad. Sci. USA 2015, 112, 6925–6930. [Google Scholar] [CrossRef] [PubMed]
- De Grandi, C.; Polkovnikov, A.; Sandvik, A.W. Universal nonequilibrium quantum dynamics in imaginary time. Phys. Rev. B 2011, 84, 224303. [Google Scholar] [CrossRef]
- Kolodrubetz, M.; Clark, B.K.; Huse, D.A. Nonequilibrium Dynamic Critical Scaling of the Quantum Ising Chain. Phys. Rev. Lett. 2012, 109, 015701. [Google Scholar] [CrossRef] [PubMed]
- Kolodrubetz, M.; Pekker, D.; Clark, B.K.; Sengupta, K. Nonequilibrium dynamics of bosonic Mott insulators in an electric field. Phys. Rev. B 2012, 85, 100505. [Google Scholar] [CrossRef]
- Chandran, A.; Erez, A.; Gubser, S.S.; Sondhi, S.L. Kibble–Zurek problem: Universality and the scaling limit. Phys. Rev. B 2012, 86, 064304. [Google Scholar] [CrossRef]
- Yin, S.; Qin, X.; Lee, C.; Zhong, F. Finite-time scaling of dynamic quantum criticality. arXiv 2012. [Google Scholar]
- Yin, S.; Mai, P.; Zhong, F. Nonequilibrium quantum criticality in open systems: The dissipation rate as an additional indispensable scaling variable. Phys. Rev. B 2014, 89, 094108. [Google Scholar] [CrossRef]
- Ulm, S.; Roßnagel, J.; Jacob, G.; Degünther, C.; Dawkins, S.; Poschinger, U.; Nigmatullin, R.; Retzker, A.; Plenio, M.; Schmidt-Kaler, F.; et al. Observation of the Kibble–Zurek scaling law for defect formation in ion crystals. Nat. Commun. 2013, 4, 2290. [Google Scholar] [CrossRef] [PubMed]
- Pyka, K.; Keller, J.; Partner, H.L.; Nigmatullin, R.; Burgermeister, T.; Meier, D.M.; Kuhlmann, K.; Retzker, A.; Plenio, M.B.; Zurek, W.H.; et al. Topological defect formation and spontaneous symmetry breaking in ion Coulomb crystals. Nat. Commun. 2013, 4, 2291. [Google Scholar] [CrossRef] [PubMed]
- Navon, N.; Gaunt, A.L.; Smith, R.P.; Hadzibabic, Z. Critical dynamics of spontaneous symmetry breaking in a homogeneous Bose gas. Science 2015, 347, 167–170. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Clark, L.W.; Feng, L.; Chin, C. Universal space-time scaling symmetry in the dynamics of bosons across a quantum phase transition. Science 2016, 354, 606–610. [Google Scholar] [CrossRef] [PubMed]
- Gong, S.; Zhong, F.; Huang, X.; Fan, S. Finite-time scaling via linear driving. New J. Phys. 2010, 12, 043036. [Google Scholar] [CrossRef]
- Zhong, F. Finite-time Scaling and its Applications to Continuous Phase Transitions. In Applications of Monte Carlo Method in Science and Engineering; Mordechai, S., Ed.; InTech: Rijeka, Croatia, 2011. [Google Scholar]
- Liu, C.W.; Polkovnikov, A.; Sandvik, A.W. Quantum versus Classical Annealing: Insights from Scaling Theory and Results for Spin Glasses on 3-Regular Graphs. Phys. Rev. Lett. 2015, 114, 147203. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.W.; Polkovnikov, A.; Sandvik, A.W.; Young, A.P. Universal dynamic scaling in three-dimensional Ising spin glasses. Phys. Rev. E 2015, 92, 022128. [Google Scholar] [CrossRef] [PubMed]
- Hu, Q.; Yin, S.; Zhong, F. Scaling of the entanglement spectrum in driven critical dynamics. Phys. Rev. B 2015, 91, 184109. [Google Scholar] [CrossRef]
- Huang, Y.; Yin, S.; Hu, Q.; Zhong, F. Kibble–Zurek mechanism beyond adiabaticity: Finite-time scaling with critical initial slip. Phys. Rev. B 2016, 93, 024103. [Google Scholar] [CrossRef]
- Feng, B.; Yin, S.; Zhong, F. Theory of driven nonequilibrium critical phenomena. Phys. Rev. B 2016, 94, 144103. [Google Scholar] [CrossRef]
- De Grandi, C.; Gritsev, V.; Polkovnikov, A. Quench dynamics near a quantum critical point. Phys. Rev. B 2010, 81, 012303. [Google Scholar] [CrossRef]
- De Grandi, C.; Gritsev, V.; Polkovnikov, A. Quench dynamics near a quantum critical point: Application to the sine-Gordon model. Phys. Rev. B 2010, 81, 224301. [Google Scholar] [CrossRef]
- Deng, S.; Ortiz, G.; Viola, L. Dynamical critical scaling and effective thermalization in quantum quenches: Role of the initial state. Phys. Rev. B 2011, 83, 094304. [Google Scholar] [CrossRef]
- Yin, S.; Lo, C.-Y.; Chen, P. Scaling in driven dynamics starting in the vicinity of a quantum critical point. Phys. Rev. B 2016, 94, 064302. [Google Scholar] [CrossRef]
- Sachdev, S. Quantum Phase Transitions; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Eisert, J.; Cramer, M.; Plenio, M. Colloquium: Area laws for the entanglement entropy. Rev. Mod. Phys. 2010, 82, 277–306. [Google Scholar] [CrossRef]
- Amico, L.; Fazio, R.; Osterloh, A.; Vedral, V. Entanglement in many-body systems. Rev. Mod. Phys. 2008, 80, 517–576. [Google Scholar] [CrossRef]
- Osterloh, A.; Amico, L.; Falci, G.; Fazio, R. Scaling of entanglement close to a quantum phase transition. Nature 2002, 416, 608–610. [Google Scholar] [CrossRef] [PubMed]
- Laflorencie, N. Quantum entanglement in condensed matter systems. Phys. Rep. 2016, 646, 1–59. [Google Scholar] [CrossRef]
- Calabrese, P.; Cardy, J. Evolution of entanglement entropy in one-dimensional systems. J. Stat. Mech. 2005, 2005, 04010. [Google Scholar] [CrossRef]
- Cincio, L.; Dziarmaga, J.; Rams, M.; Zurek, W. Entropy of entanglement and correlations induced by a quench: Dynamics of a quantum phase transition in the quantum Ising model. Phys. Rev. A 2007, 75, 052321. [Google Scholar] [CrossRef]
- Vidal, G. Classical Simulation of Infinite-Size Quantum Lattice Systems in One Spatial Dimension. Phys. Rev. Lett. 2007, 98, 070201. [Google Scholar] [CrossRef] [PubMed]
- Verstraete, F.; García-Ripoll, J.J.; Cirac, J.I. Matrix Product Density Operators: Simulation of Finite-Temperature and Dissipative Systems. Phys. Rev. Lett. 2004, 93, 207204. [Google Scholar] [CrossRef] [PubMed]
- Schollwöck, U. The density-matrix renormalization group in the age of matrix product states. Ann. Phys. 2011, 326, 96–192. [Google Scholar] [CrossRef]
- Del Campo, A.; Retzker, A.; Plenio, M.B. Inhomogeneous Kibble–Zurek mechanism: Vortex nucleation during Bose–Einstein condensation. New J. Phys. 2011, 13, 083022. [Google Scholar] [CrossRef]

© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhai, L.-J.; Yin, S. Effects Induced by the Initial Condition in the Quantum Kibble–Zurek Scaling for Changing the Symmetry-Breaking Field. Entropy 2016, 18, 446. https://doi.org/10.3390/e18120446
Zhai L-J, Yin S. Effects Induced by the Initial Condition in the Quantum Kibble–Zurek Scaling for Changing the Symmetry-Breaking Field. Entropy. 2016; 18(12):446. https://doi.org/10.3390/e18120446
Chicago/Turabian StyleZhai, Liang-Jun, and Shuai Yin. 2016. "Effects Induced by the Initial Condition in the Quantum Kibble–Zurek Scaling for Changing the Symmetry-Breaking Field" Entropy 18, no. 12: 446. https://doi.org/10.3390/e18120446





