Chaos on the Vallis Model for El Niño with Fractional Operators

Abstract

The Vallis model for El Niño is an important model describing a very interesting physical problem. The aim of this paper is to investigate and compare the models using both integer and non-integer order derivatives. We first studied the model with the local derivative by presenting for the first time the exact solution for equilibrium points, and then we presented the exact solutions with the numerical simulations. We further examined the model within the scope of fractional order derivatives. The fractional derivatives used here are the Caputo derivative and Caputo–Fabrizio type. Within the scope of fractional derivatives, we presented the existence and unique solutions of the model. We derive special solutions of both models with Caputo and Caputo–Fabrizio derivatives. Some numerical simulations are presented to compare the models. We obtained more chaotic behavior from the model with Caputo–Fabrizio derivative than other one with local and Caputo derivative. When compare the three models, we realized that, the Caputo derivative plays a role of low band filter when the Caputo–Fabrizio presents more information that were not revealed in the model with local derivative.

1. Introduction

Claude Shannon in 1948 established the concept of information theory. The concept has been used in many scientific fields, for instance in signal and image processing [1,2,3,4,5,6]. Recently, using the concept of fractional calculus, Machado has introduced a new formula for entropy [1,2]. Nowadays, information theory is generalized in the scope of fractional calculus and has found many novel applications in the fields of engineering and physics [1,2,3,4,5,6].

Within the class of mathematical systems of equations describing some chaotic system, we can say that the well-known Lorenz model is perhaps the most classical and exemplary problem since it is, as far we are aware, the primitive model of chaotic behavior [7,8,9,10]. This model is a modified and simplified form of the former complex model of Saltzman to portray buoyancy-driven convection patterns in the classical rectangular Rayleigh-Bernard problem used in thermal convection between two plates perpendicular to the direction of the Earth’s gravitational force. This model can also be obtained by a simple transformation of the Vallis model for El Niño given below as:

$$\{\begin{array}{l}\frac{dx\left(t\right)}{dt}=By\left(t\right)-C\left(x\left(t\right)+p\right)\\ \frac{dy\left(t\right)}{dt}=x\left(t\right)z\left(t\right)-y\left(t\right)\\ \frac{dz\left(t\right)}{dt}=-x\left(t\right)y\left(t\right)-z\left(t\right)+1\end{array}$$

(1)

The El Niño phenomenon is a devilishly irregular, anomalous, Christmas-time warming of the coastal waters off Peru and Ecuador occurring about every 3–6 years that has an enormous impact on global climate. Because of the importance of this model, more studies need to be done in order to accurately provide a more accurate model can be used to describe the mentioned physical problem [7]. Although the model has been used with great success, it is perhaps important to mention that, anomalous or irregular phenomenon cannot accurately be described with local derivative. This has been revealed in many research papers, monograms and books in the last decade [11,12,13,14,15,16,17,18,19,20]. The aim of this paper is to further observe if the model based on the fractional derivative will be more descriptive than the one with local derivative. Therefore in this paper, the comparison of models will be done using different definitions of derivatives. We shall therefore present in the section some definition of derivative with fractional order.

Definition 1. 

[17,18,19,20]. Let $f\in H^1\left(a, b\right), b>a, \alpha \in \left[0,1\right]$ then, the new Caputo derivative of fractional derivative is defined as:

$$D_t^{\alpha }\left(f\left(t\right)\right)=\frac{M\left(\alpha \right)}{1-\alpha }{\displaystyle \underset{a}{\overset{t}{\int }}}{f}^{\prime }\left(x\right)exp\left[-\alpha \frac{t-x}{1-\alpha }\right]dx$$

(2)

where $M\left(\alpha \right)$ is a normalization function such that $M\left(0\right)=M\left(1\right)=1$ [11]. Nevertheless, if the function does not belong to $H^1\left(a, b\right)$ then, the derivative can be reformulated as:

$$D_t^{\alpha }\left(f\left(t\right)\right)=\frac{\alpha M\left(\alpha \right)}{1-\alpha }{\displaystyle \underset{a}{\overset{t}{\int }}}\left(f\left(t\right)-f\left(x\right)\right)exp\left[-\alpha \frac{t-x}{1-\alpha }\right]dx$$

(3)

Definition 2. 

Let f be a function not necessary differential, let α be a real number such that 0 ≤ α ≤ 1, then the new derivative with order α is given as:

$${}_{0}D_x^{\alpha }\left\{f\left(x\right)\right\}=\frac{1}{1-\alpha }\frac{d}{dx}{\displaystyle \underset{0}{\overset{x}{\int }}}f\left(x\right)exp\left[-\alpha \frac{\left(t-x\right)}{1-\alpha }\right]dx$$

(4)

If α is zero we have the following

$${}_{0}D_x^0\left\{f\left(x\right)\right\}=\frac{d}{dx}{\displaystyle \underset{0}{\overset{x}{\int }}}f\left(x\right)dx=f\left(x\right)$$

(5)

Using the argument by Caputo and Fabrizio, we also have that when this expression also goes to 1 we recover the first derivative [17,18,19,20].

Definition 3. 

According to Caputo, the fractional derivative of a continuous and n-time differentiable function f is given as:

$$D_t^{\alpha }\left(f\left(t\right)\right)=\frac{1}{\mathrm{\Gamma }\left(n-\alpha \right)}{\displaystyle \underset{a}{\overset{t}{\int }}}{\left(t-x\right)}^{n-\alpha -1}{\left(\frac{d}{dx}\right)}^{n}f\left(x\right)dx, n-1<\alpha \le n$$

(6)

Definition 4. 

The modified Riemann–Liouville fractional derivative of a function f is given as:

$$D_t^{\alpha }\left(f\left(t\right)\right)=\frac{1}{\mathrm{\Gamma }\left(n-\alpha \right)}{\left(\frac{d}{dt}\right)}^n{\displaystyle \underset{a}{\overset{t}{\int }}}{\left(t-x\right)}^{n-\alpha -1}\left[f\left(x\right)-f\left(a\right)\right]dx, n-1<\alpha \le n$$

There are other definitions that are not mentioned here.

2. Analysis of Vallis Model with Local Derivative

In this section we present a detailed analysis system of Equation (1).

2.1. Stability Analysis of Equilibrium Points

To find the equilibrium points, we assume that the system is time-independent such that:

$$\{\begin{array}{l}0=B\overline{y}-C\left(\overline{x}+p\right)\\ 0=\overline{xz}-\overline{y }\\ 0=-\overline{xy}-\overline{z}+1\end{array}$$

(7)

The following general solutions are obtained:

$$\begin{array}{l}\overline{x}\\ =-\frac{\mathrm{p}}{3}-\frac{2^{1/3}(3\mathrm{C}(-\mathrm{B}+\mathrm{C})-{\mathrm{C}}^2{\mathrm{p}}^2)}{3\mathrm{C}{(-9{\text{BC}}^2\mathrm{p}-18{\mathrm{C}}^3\mathrm{p}-2{\mathrm{C}}^3{\mathrm{p}}^3+\sqrt{4{(3\mathrm{C}(-\mathrm{B}+\mathrm{C})-{\mathrm{C}}^2{\mathrm{p}}^2)}^3+{(-9{\text{BC}}^2\mathrm{p}-18{\mathrm{C}}^3\mathrm{p}-2{\mathrm{C}}^3{\mathrm{p}}^3)}^2})}^{1/3}}\\ +\frac{{(-9{\text{BC}}^2\mathrm{p}-18{\mathrm{C}}^3\mathrm{p}-2{\mathrm{C}}^3{\mathrm{p}}^3+\sqrt{4{(3\mathrm{C}(-\mathrm{B}+\mathrm{C})-{\mathrm{C}}^2{\mathrm{p}}^2)}^3+{(-9{\text{BC}}^2\mathrm{p}-18{\mathrm{C}}^3\mathrm{p}-2{\mathrm{C}}^3{\mathrm{p}}^3)}^2})}^{1/3}}{{32}^{1/3}\mathrm{C}}\end{array}$$

$$\begin{array}{l}\overline{y}\\ =-\frac{1}{B}(-\frac{2Cp}{3}\\ -\frac{2^{1/3}BC}{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ +\frac{2^{1/3}{C}^2}{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ -\frac{2^{1/3}{C}^2{p}^2}{3{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ -\frac{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}{{32}^{1/3}}\end{array}$$

$$\begin{array}{l}\overline{z}\\ =\frac{1}{B}(\frac{B}{3}+\frac{2C}{3}\\ -\frac{2^{2/3}{B}^{2}C}{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ +\frac{{22}^{2/3}B{C}^2}{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ -\frac{2^{2/3}{C}^3}{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ -\frac{{22}^{2/3}B{C}^2{p}^2}{3{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ +\frac{{22}^{2/3}{C}^3{p}^2}{3{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ -\frac{2^{2/3}{C}^3{p}^4}{9{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}\\ -\frac{2^{1/3}BCp}{3{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ +\frac{2^{1/3}{C}^{2}p}{3{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ -\frac{2^{1/3}{C}^2{p}^3}{9{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}\\ -\frac{p{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}}{{32}^{1/3}}\\ +\frac{1}{9}{2}^{2/3}p{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{1/3}\\ -\frac{{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3+\sqrt{4{(3C(-B+C)-C^2{p}^2)}^3+{(-9B{C}^{2}p-18C^{3}p-2C^3{p}^3)}^2})}^{2/3}}{{92}^{2/3}C})\end{array}$$

Therefore, if we chose B = 102, C = 3 and p = 0.83, we obtain the following equilibrium points:

$$\overline{x}=-6.18617,\overline{y}=-0.157534, \overline{z}=0.0254656$$

The characteristic equation associate to the above equilibrium points is given as:

$$246.868+22.8431x-11.05412-x^3=0$$

(8)

The following eigenvalues are therefore obtained:

$$x_1=-11.1102,x_2=-4.68584,x_3=4.74193$$

The above shows that the equilibrium point is unstable.

2.2. Existence of Exact Solution

We aim in this section to show that, the system (1) with initial solution has a unique set of solution. To achieve we first transform the system into integral equations by applying the antiderivative on both sides of the system to obtain:

$$\{\begin{array}{l}x\left(t\right)-x\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{By\left(l\right)-C\left(x\left(l\right)+p\right)\right\}dl\\ y\left(t\right)-y\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{x\left(l\right)z\left(l\right)-y\left(l\right)\right\}dl\\ z\left(t\right)-z\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{-x\left(l\right)y\left(l\right)-z\left(l\right)+1\right\}dl\end{array}$$

(9)

We suggest the following iterative formula from Equation (9):

$$\{\begin{array}{l}{x}_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{B{y}_{n-1}\left(l\right)-C\left(x_{n-1}\left(l\right)+p\right)\right\}dl\\ y_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{x_{n-1}\left(l\right)z_{n-1}\left(l\right)-y_{n-1}\left(l\right)\right\}dl\\ z_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{-x_{n-1}\left(l\right)y_{n-1}\left(l\right)-z_{n-1}\left(l\right)+1\right\}dl\end{array}$$

(10)

With initial components:

$$\{\begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}$$

(11)

We consider the following formula:

$$\{\begin{array}{l}{X}_n=x_n-x_{n-1}\\ Y_n=y_n-y_{n-1}\\ Z_n=z_n-z_{n-1}\end{array}$$

(12)

Thus it is easy to see that:

$$\{\begin{array}{l}{x}_n\left(t\right)={\displaystyle {\displaystyle \sum }_{j=1}^n}{X}_j\\ y_n\left(t\right)={\displaystyle {\displaystyle \sum }_{j=1}^n}{Y}_j\\ z_n\left(t\right)={\displaystyle {\displaystyle \sum }_{j=1}^n}{Z}_j\end{array}$$

(13)

Let $F\left(t\right)={\left[x\left(t\right),y\left(t\right),z\left(t\right)\right]}^T,X\left(0\right)={\left[x\left(t\right),y\left(t\right),z\left(t\right)\right]}^T$ and $K\left(t,F\left(t\right)\right)=\underset{0\le t\le T}{\text{max}}\left[\frac{dx}{dt},\frac{dy}{dt},\frac{dz}{dt}\right]$. With above definition, we can system (10) into:

$$F_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}K\left(l,F_{n-1}\left(l\right)\right)dl$$

(14)

Also:

$$D_n\left(t\right)=F_n\left(t\right)-F_{n-1}\left(t\right)$$

(15)

We now evaluate:

$$\begin{gathered} \Vert D_n\left(t\right)\Vert =\Vert F_n\left(t\right)-F_{n-1}\left(t\right)\Vert =\Vert {\displaystyle \underset{0}{\overset{t}{\int }}}\left\{K\left(l,F_{n-1}\left(l\right)\right)-K\left(l,F_{n-2}\left(l\right)\right)\right\}dl\Vert \\ \le {\displaystyle \underset{0}{\overset{t}{\int }}}\Vert \left\{K\left(l,F_{n-1}\left(l\right)\right)-K\left(l,F_{n-2}\left(l\right)\right)\right\}\Vert dl \end{gathered}$$

(16)

We shall prove that, the defined kernel has Lipschitz condition.

Theorem 1. 

The defined kernel K possesses the Lipschitz condition under the condition that, the operator F is bounded or if:

$$1+\Vert F_1\Vert +\Vert F_2\Vert <M$$

then:

$$\Vert \left\{K\left(l,F_1\left(l\right)\right)-K\left(l,F_2\left(l\right)\right)\right\}\Vert <M\Vert F_1\left(l\right)-F_2\left(l\right)\Vert$$

where $F_1\left(t\right)={\left[x_1\left(t\right),y_1\left(t\right),z_1\left(t\right)\right]}^T$ and $F_2\left(t\right)={\left[x_2\left(t\right),y_2\left(t\right),z_2\left(t\right)\right]}^T$

Proof. 

Within the scope of our study, we have that:

$$K\left(l,F_1\left(l\right)\right)=F_1^2+F_1$$

Thus:

$$\Vert \left\{K\left(l,F_1\left(l\right)\right)-K\left(l,F_2\left(l\right)\right)\right\}\Vert =\Vert F_1^2+F_1-F_2^2-F_2\Vert \le \left\{1+\Vert F_1\Vert +\Vert F_2\Vert \right\}\Vert F_1-F_2\Vert$$

Using the hypothesis, we get:

$$\Vert \left\{K\left(l,F_1\left(l\right)\right)-K\left(l,F_2\left(l\right)\right)\right\}\Vert <M\Vert F_1-F_2\Vert$$

(17)

And the requested result is obtained. □

Therefore within the boundaries of Theorem 1, we have that:

$$\Vert D_n\left(t\right)\Vert \le M{\displaystyle \underset{0}{\overset{t}{\int }}}\Vert D_{n-1}\left(l\right)\Vert dl$$

(18)

Theorem 2. 

Under the condition set in Theorem 1, the Vallis model for El Niño model has a unique set of solutions.

Proof. 

Using Equation (18), we have the following inequality, which is obtained by recursion:

$$\Vert D_n\left(t\right)\Vert \le {\left(Mt\right)}^n\text{max}\{ X{\left(0\right)}^T\}$$

Then it follows from the above that:

$$F\left(t\right)={\displaystyle \sum _{n=0}^{\infty }}{D}_n\left(t\right)$$

(19)

exists and is continuous. We now show that the above function is the solution of Equation (9), for this we let:

$$F\left(t\right)=F_n\left(t\right)-R_n\left(t\right)$$

(20)

where $R_n\left(t\right)$ is considered as a remainder term, which tends to zero when n tends to infinity. With this in mind:

$$F\left(t\right)-G\left(0\right)-{\displaystyle \underset{0}{\overset{t}{\int }}}K\left(\tau ,F\left(\tau \right)\right)d\tau =R_n\left(t\right)+{\displaystyle \underset{0}{\overset{t}{\int }}}\left\{K\left(\tau ,F\left(\tau \right)-R_n\left(t\right)\right)-K\left\{\tau ,F\left(\tau \right)\right\}\right\}d\tau$$

(21)

where $G\left(0\right)={[x\left(0\right),y\left(0\right),z\left(0\right)]}^T$.

Applying the norm on both sides and considering the Lipschitz condition of the kernel, we obtain:

$$\Vert F\left(t\right)-G\left(0\right)-{\displaystyle \underset{0}{\overset{t}{\int }}}K\left(\tau ,F\left(\tau \right)\right)d\tau \Vert \le \Vert R_n\left(t\right)\Vert +tM\Vert R_n\left(t\right)\Vert$$

Apply the limit of both sides when n tends to infinity, the right hand side produces zero then:

$$F\left(t\right)=G\left(0\right)+{\displaystyle \underset{0}{\overset{t}{\int }}}K\left(\tau ,F\left(\tau \right)\right)d\tau .$$

Thus the above is indeed the solution of system (9). The next step is to show the uniqueness of the solution. Let assume that we can find a different function $F_1\left(t\right)$ satisfying system (9). Then:

$$\Vert F\left(t\right)-F_1\left(t\right)\Vert \le \Vert R_n\left(t\right)-R_{\left(n-1\right)1}\left(t\right)\Vert +tM\Vert R_n\left(t\right)-R_{\left(n-1\right)1}\left(t\right)\Vert$$

When n tends to infinity, the right hand side tends to zero then:

$$F\left(t\right)=F_1\left(t\right)$$

This completes the proof. □

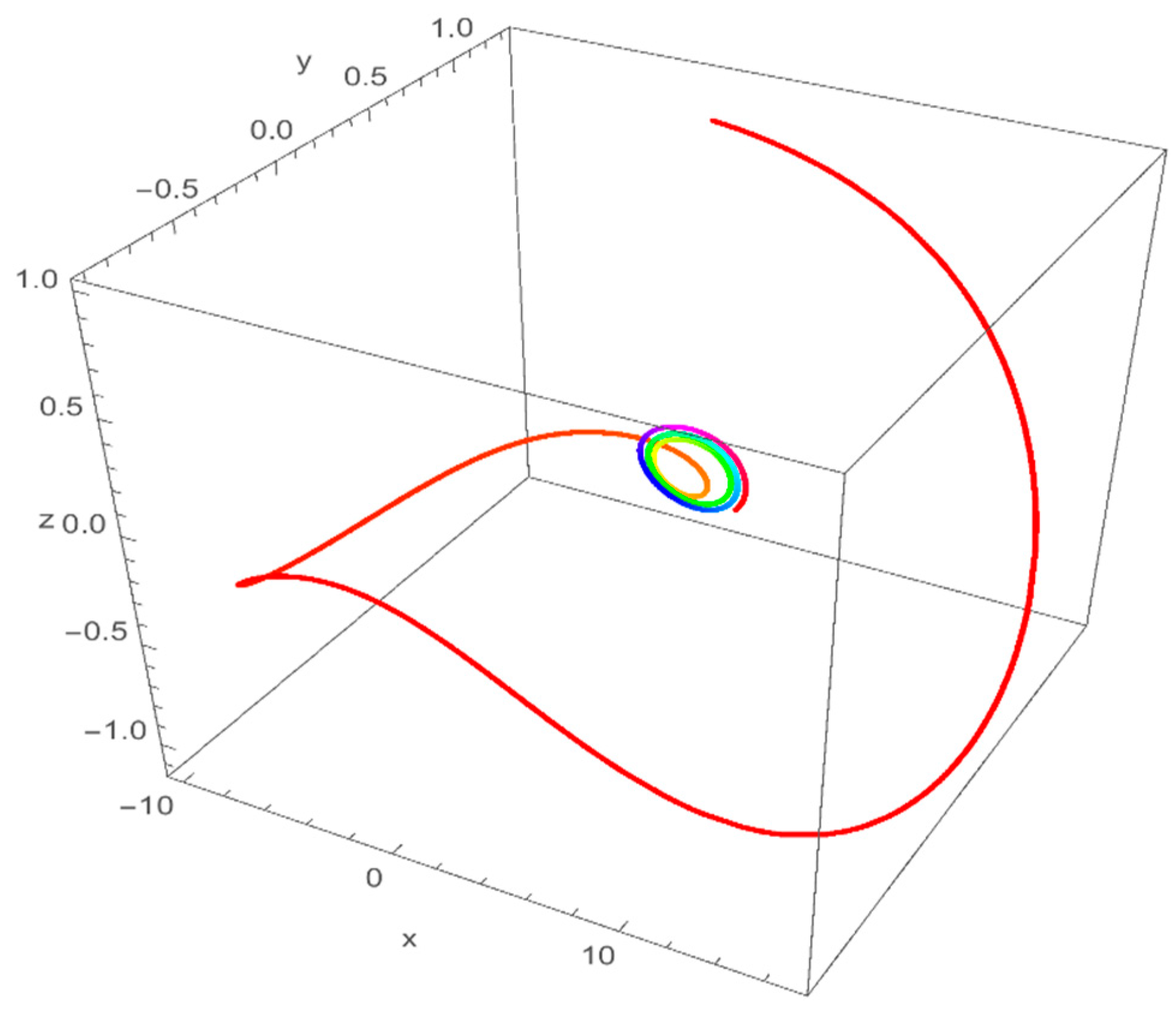
2.3. Numerical Simulations

In this section, we derive the solution of the system using some iterative technique since the system is nonlinear:

$$\{\begin{array}{l}x\left(t\right)-x\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{By\left(l\right)-C\left(x\left(l\right)+p\right)\right\}dl\\ y\left(t\right)-y\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{x\left(l\right)z\left(l\right)-y\left(l\right)\right\}dl\\ z\left(t\right)-z\left(0\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{-x\left(l\right)y\left(l\right)-z\left(l\right)+1\right\}dl\end{array}$$

We suggest the following iterative formula from equation:

$$\{\begin{array}{l}{x}_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{B{y}_{n-1}\left(l\right)-C\left(x_{n-1}\left(l\right)+p\right)\right\}dl\\ y_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{x_{n-1}\left(l\right)z_{n-1}\left(l\right)-y_{n-1}\left(l\right)\right\}dl\\ z_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{-x_{n-1}\left(l\right)y_{n-1}\left(l\right)-z_{n-1}\left(l\right)+1\right\}dl\end{array}$$

with initial components:

$$\{\begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}$$

the approximate solution can be given as:

$$\{\begin{array}{l}x\left(t\right)=\underset{n\to \infty }{\text{lim}}{x}_n\\ y\left(t\right)=\underset{n\to \infty }{\text{lim}}{y}_n\\ z\left(t\right)=\underset{n\to \infty }{\text{lim}}{z}_n\end{array}$$

(22)

To obtain the solution, we use the following Algorithm 1:

Algorithm 1.

Input $\{\begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}$ as preliminary input i-number terms in the approximation Result $\{\begin{array}{l}{x}_{ap}\left(t\right)\\ y_{ap}\left(t\right)\\ z_{ap}\left(t\right)\end{array}$ the approximate solution

Step 1: Put $\{\begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}$ and $\{\begin{array}{l}{x}_{ap}\left(t\right)=x_{ap}\left(t\right)\\ y_{ap}\left(t\right)=y_{ap}\left(t\right)\\ z_{ap}\left(t\right)=z_{ap}\left(t\right)\end{array}$

Step 2: for i = 1 to n − 1 do step 3, step 4 and step 5 $$\begin{array}{l}{x}_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{B{y}_{n-1}\left(l\right)-C\left(x_{n-1}\left(l\right)+p\right)\right\}dl\\ y_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{x_{n-1}\left(l\right)z_{n-1}\left(l\right)-y_{n-1}\left(l\right)\right\}dl\\ z_n\left(t\right)={\displaystyle \underset{0}{\overset{t}{\int }}}\left\{-x_{n-1}\left(l\right)y_{n-1}\left(l\right)-z_{n-1}\left(l\right)+1\right\}dl\end{array}$$

Step 3: compute $$\begin{array}{l}{T}_{n+1}\left(t\right)=T_n\left(t\right)+x_{ap}\left(t\right)\\ L_{n+1}\left(t\right)=L_n\left(t\right)+y_{ap}\left(t\right)\\ E_{n+1}\left(t\right)=E_n\left(t\right)+z_{ap}\left(t\right)\end{array}$$

Step 4: $$\begin{array}{l}{x}_{ap}\left(t\right)=T_{n+1}\left(t\right)+x_{ap}\left(t\right)\\ y_{ap}\left(t\right)=L_{n+1}\left(t\right)+y_{ap}\left(t\right)\\ z_{ap}\left(t\right)=E_{n+1}\left(t\right)+z_{ap}\left(t\right)\end{array}$$

Step 5: stop.

The numerical simulations are depicted in Figure 1, Figure 2, Figure 3, Figure 4, Figure 5 and Figure 6. In Figure 1 and Figure 2 we have the solution of the system 1. In Figure 3 and Figure 4, we have the 3 dimensional parametric plot of the system solution. In Figure 5 and Figure 6 we have the solution for y and z.

3. Analysis of Vallis Model with Caputo Derivative

In this section, we consider the model in system with the Caputo fractional derivative. Then system (1) becomes:

$$\{\begin{array}{l}{}_0{}^{C}D_t^{\alpha }x\left(t\right)=By\left(t\right)-C\left(x\left(t\right)+p\right)\\ {}_0{}^{C}D_t^{\alpha }y\left(t\right)=x\left(t\right)z\left(t\right)-y\left(t\right)\\ {}_0{}^{C}D_t^{\alpha }z\left(t\right)=-x\left(t\right)y\left(t\right)-z\left(t\right)+1\end{array}$$

(23)

We derive the approximate solution of the above equation using the Laplace transform operator. Thus applying it on both sides of (23), we obtain:

$$\{\begin{array}{l}\overline{x}\left(s\right)=\frac{x\left(0\right)}{s}+\frac{1}{s^{\alpha }}\mathcal{L}\left\{By\left(t\right)-C\left(x\left(t\right)+p\right)\right\}\left(s\right)\\ \overline{y}\left(s\right)=\frac{y\left(0\right)}{s}+\frac{1}{s^{\alpha }}\mathcal{L}\left\{x\left(t\right)z\left(t\right)-y\left(t\right)\right\}\left(s\right)\\ \overline{z}\left(s\right)=\frac{z\left(0\right)}{s}+\frac{1}{s^{\alpha }}\mathcal{L}\left\{-x\left(t\right)y\left(t\right)-z\left(t\right)+1\right\}\left(s\right)\end{array}$$

(24)

By application of inverse Laplace on above system, we get:

$$\{\begin{array}{l}x\left(t\right)=x\left(0\right)+{ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{By\left(t\right)-C\left(x\left(t\right)+p\right)\right\}\left(s\right)\right\}\left(t\right)\\ y\left(t\right)=y\left(0\right)+{ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{x\left(t\right)z\left(t\right)-y\left(t\right)\right\}\left(s\right)\right\}\left(t\right)\\ z\left(t\right)=z\left(0\right)+{ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{-x\left(t\right)y\left(t\right)-z\left(t\right)+1\right\}\left(s\right)\right\}\left(t\right)\end{array}$$

(25)

The following iterative formula is then proposed:

$$\begin{array}{c}\{\begin{array}{l}{x}_n\left(t\right)={ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{B{y}_{n-1}\left(t\right)-C\left(x_{n-1}\left(t\right)+p\right)\right\}\left(s\right)\right\}\left(t\right)\\ y_n\left(t\right)={ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{x_{n-1}\left(t\right)z_{n-1}\left(t\right)-y_{n-1}\left(t\right)\right\}\left(s\right)\right\}\left(t\right)\\ z_n\left(t\right)={ℒ}^{-1}\left\{\frac{1}{s^{\alpha }}ℒ\left\{-x_{n-1}\left(t\right)y_{n-1}\left(t\right)-z_{n-1}\left(t\right)+1\right\}\left(s\right)\right\}\left(t\right)\end{array}\\ \{\begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}\end{array}$$

(26)

The approximate solution is assumed to be obtain as a limit when n tends to infinity:

$$\{\begin{array}{l}x\left(t\right)=\underset{n\to \infty }{\text{lim}}{x}_n\\ y\left(t\right)=\underset{n\to \infty }{\text{lim}}{y}_n\\ z\left(t\right)=\underset{n\to \infty }{\text{lim}}{z}_n\end{array}$$

Stability Analysis of the Iteration Method

In this section, we demonstrate that the used method is stable when solving system (1). In this section, we assume the following: It is possible to find three positive constant A, W and J such that for all 0 ≤ t ≤ T ≤ ∞, $\Vert x\left(t\right)\Vert <A, \Vert y\left(t\right)\Vert <W$ and $\Vert z\left(t\right)\Vert <J$. We next consider a subset of $L_2\left(\left(a,b\right)\left(0,T\right)\right)$ defined by:

$$R=\left\{v:\left(a,b\right)\left(0,T\right)\to ℝ, \frac{1}{\mathrm{\Gamma }\left(\alpha \right)}\int {\left(t-l\right)}^{\alpha -1}v\left(l\right)u\left(l\right)dl<\infty \right\}$$

We now consider the following operator V defined as:

$$V\left(x,y,z\right)=\{\begin{array}{l}By\left(t\right)-C\left(x\left(t\right)+p\right)\\ x\left(t\right)z\left(t\right)-y\left(t\right)\\ -x\left(t\right)y\left(t\right)-z\left(t\right)+1\end{array}$$

(27)

Then:

$$\begin{array}{ll}\langle V\left(x,y,x\right)-& V\left(X,Y,Z\right), \left(x-X, y-Y,z-Z\right)\rangle \\ & = \{\begin{array}{l}\langle B\left(y\left(t\right)-Y\left(t\right)\right)-C\left(x\left(t\right)-X\left(t\right)\right),\left(x\left(t\right)-X\left(t\right)\right)\rangle \\ \langle \left(x\left(t\right)-X\left(t\right)\right)\left(z\left(t\right)-Z\left(t\right)\right)-\left(y\left(t\right)-Y\left(t\right)\right),\left(y\left(t\right)-Y\left(t\right)\right)\rangle \\ \langle –\left(x\left(t\right)-X\left(t\right)\right)\left(y\left(t\right)-Y\left(t\right)\right)-\left(z\left(t\right)-Z\left(t\right)\right),\left(z\left(t\right)-Z\left(t\right)\right)\rangle \end{array}\end{array}$$

(28)

where $x\left(t\right)\ne X\left(t\right), y\left(t\right)\ne Y\left(t\right)$ and $z\left(t\right)\ne Z\left(t\right)$ for all $0\le t\le T<\infty$.

Thus applying the absolute value on both sides, the right hand side produces:

$$\begin{array}{l}\{\begin{array}{l}B\Vert y\left(t\right)-Y\left(t\right)\Vert \Vert x\left(t\right)-X\left(t\right)\Vert +C{\Vert x\left(t\right)-X\left(t\right)\Vert }^2\\ \Vert x\left(t\right)-X\left(t\right)\Vert \Vert z\left(t\right)-Z\left(t\right)\Vert \Vert y\left(t\right)-Y\left(t\right)\Vert +{\Vert y\left(t\right)-Y\left(t\right)\Vert }^2\\ \Vert x\left(t\right)-X\left(t\right)\Vert \Vert y\left(t\right)-Y\left(t\right)\Vert \Vert z\left(t\right)-Z\left(t\right)\Vert +{\Vert z\left(t\right)-Z\left(t\right)\Vert }^2\end{array}\\ \{\begin{array}{l}\left\{B\frac{\Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert x\left(t\right)-X\left(t\right)\Vert }+C\right\}{\Vert x\left(t\right)-X\left(t\right)\Vert }^2\\ \left\{\frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert z\left(t\right)-Z\left(t\right)\Vert }{\Vert y\left(t\right)-Y\left(t\right)\Vert }+1\right\}{\Vert y\left(t\right)-Y\left(t\right)\Vert }^2\\ \left\{\frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert z\left(t\right)-Z\left(t\right)\Vert }+\right\}{\Vert z\left(t\right)-Z\left(t\right)\Vert }^2\end{array}\end{array}$$

(29)

Then:

$$\begin{array}{l}\begin{array}{ll}|\langle V\left(x,y,x\right)-& V\left(X,Y,Z\right), \left(x-X, y-Y,z-Z\right)\rangle |\\ & \le \{\begin{array}{c}\left\{B\frac{\Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert x\left(t\right)-X\left(t\right)\Vert }+C\right\}{\Vert x\left(t\right)-X\left(t\right)\Vert }^2\\ \left\{\frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert z\left(t\right)-Z\left(t\right)\Vert }{\Vert y\left(t\right)-Y\left(t\right)\Vert }+1\right\}{\Vert y\left(t\right)-Y\left(t\right)\Vert }^2\\ \left\{\frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert z\left(t\right)-Z\left(t\right)\Vert }\right\}{\Vert z\left(t\right)-Z\left(t\right)\Vert }^2\end{array}\end{array}\\ |\langle V\left(x,y,x\right)-V\left(X,Y,Z\right), \left(x-X, y-Y,z-Z\right)\rangle |<\{\begin{array}{l}M{\Vert x\left(t\right)-X\left(t\right)\Vert }^2\\ N{\Vert y\left(t\right)-Y\left(t\right)\Vert }^2\\ L{\Vert z\left(t\right)-Z\left(t\right)\Vert }^2\end{array}\end{array}$$

(30)

With:

$$B\frac{\Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert x\left(t\right)-X\left(t\right)\Vert }+C=M, \frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert z\left(t\right)-Z\left(t\right)\Vert }{\Vert y\left(t\right)-Y\left(t\right)\Vert }+1=N, L=\frac{\Vert x\left(t\right)-X\left(t\right)\Vert \Vert y\left(t\right)-Y\left(t\right)\Vert }{\Vert z\left(t\right)-Z\left(t\right)\Vert }$$

Also if we consider a given non-null vector (X, Y, Z), then using the same routine as above we obtain:

$$|\langle V\left(x,y,x\right)-V\left(X,Y,Z\right), \left(X, Y,Z\right)\rangle |<\{\begin{array}{l}M\Vert x\left(t\right)-X\left(t\right)\Vert \Vert X\left(t\right)\Vert \\ N\Vert y\left(t\right)-Y\left(t\right)\Vert \Vert Y\left(t\right)\Vert \\ L\Vert z\left(t\right)-Z\left(t\right)\Vert \Vert Z\left(t\right)\Vert \end{array}$$

(31)

From the results obtained in Equations (30) and (31), we conclude that the used iterative method is stable. Using the iterative formula in (26) we present the numerical solution in Figure 7, Figure 8 and Figure 9 for different values of α. In these simulations, we used p = 0.3, with the nitial condition z(0) = y(0) = x(0) = 1.

From the above figures and with comparison with the model with local derivative, one can see that the Caputo derivative filters all high frequencies, as the order of α tends to zero. The Caputo derivative therefore plays a role of low band filter in this model.

4. Analysis of the Vallis Model with Caputo–Fabrizio Derivative

Some moderately current outcomes as of deterministic chaos field have extensive consequence for experts of information theory. Theory of information may be applicable to representational encodings of the phase-space descriptions of physical non-linear dynamical systems so that the characterization of process in terms of its Kolmogorov–Sinai entropy.

Consequently this concept of deterministic information theory happens to deal with way for assessing the limits of compression for single finite strings, while deterministic chaos theory results further permit one to choose particularly calibrated information sources to be employed to assess the performance of specific compression algorithm.

Since any novel applied mathematical concept must be entirely underpinned by some potential applications, we are here fully here with the concept of fractional derivative with no singular kernel Some result obtained via informations theory are used to define a new family of generalized informational entropies which are indexed by a parameter clearly related to fractals, via fractional calculus, and which is quite relevant in the presence of creation of uncertainty, via defects of the observation process. The relation with Shannon’s entropy, Renyi’s entropy and Tsallis’ entropy is clarified, and it is shown that Tsallis’ generalized logarithm has a direct significance in terms of fractional calculus.

In this section we consider the model in system with Caputo–Fabrizio derivative with fractional order. Thus the model in system one becomes:

$$\{\begin{array}{l}{}_0{}^{CF}D_t^{\alpha }x\left(t\right)=By\left(t\right)-C\left(x\left(t\right)+p\right)\\ {}_0{}^{CF}D_t^{\alpha }y\left(t\right)=x\left(t\right)z\left(t\right)-y\left(t\right)\\ {}_0{}^{CF}D_t^{\alpha }z\left(t\right)=-x\left(t\right)y\left(t\right)-z\left(t\right)+1\end{array}$$

(32)

where ${}_0{}^{CF}D_t^{\alpha }$ is the Caputo–Fabrizio derivative with fractional order defined in Equation (1). To derive a special solution for the above system, we employ the Sumudu transform operator. The relation between the Sumudu transform denoted by S and the Caputo–Fabrizio derivative with fractional order was developed in [20] as:

$$S\left[f\left(t\right)\right]\left(p\right)=\frac{F\left(p\right)-pf\left(0\right)}{1-\alpha -\alpha p}$$

(33)

Therefore applying the Sumudu transform on both sides of system (32) yields:

$$\begin{array}{c}\frac{X\left(s\right)-pf\left(0\right)}{1-\alpha -\alpha s}=S\left\{By\left(t\right)-C\left(x\left(t\right)+p\right)\right\}\left(s\right)\\ \frac{Y\left(s\right)-sz\left(0\right)}{1-\alpha -\alpha s}=S\left\{x\left(t\right)z\left(t\right)-y\left(t\right)\right\}\left(s\right)\\ \frac{Z\left(s\right)-sz\left(0\right)}{1-\alpha -\alpha s}=S\left\{-x\left(t\right)y\left(t\right)-z\left(t\right)+1\right\}\left(s\right)\end{array}$$

(34)

Applying the inverse Sumudu transform on both sides, we obtain:

$$\begin{array}{c}x\left(t\right)-x\left(0\right)=S^{-1}\left\{1-\alpha -\alpha s\right\}S\left\{By\left(t\right)-C\left(x\left(t\right)+p\right)\right\}\left(p\right)\\ y\left(t\right)-y\left(0\right)=S^{-1}\left\{1-\alpha -\alpha s\right\}S\left\{x\left(t\right)z\left(t\right)-y\left(t\right)\right\}\left(p\right)\\ z\left(t\right)-z\left(0\right)=S^{-1}\left\{1-\alpha -\alpha s\right\}S\left\{-x\left(t\right)y\left(t\right)-z\left(t\right)+1\right\}\left(p\right)\end{array}$$

(35)

From the above, the following recursive method is proposed:

$$\begin{array}{c}\begin{array}{c}{x}_n\left(t\right)=x_{n-1}\left(t\right)+S^{-1}\left\{\left\{1-\alpha -\alpha s\right\}S\left\{B{y}_{n-1}\left(t\right)-C\left(x_{n-1}\left(t\right)+p\right)\right\}\left(s\right)\right\}\left(t\right)\\ y_n\left(t\right))=y_{n-1}\left(t\right)+S^{-1}\left\{\left\{1-\alpha -\alpha s\right\}S\left\{x_{n-1}\left(t\right)z_{n-1}\left(t\right)-y_{n-1}\left(t\right)\right\}\left(s\right)\right\}\left(t\right)\\ z_n\left(t\right)=z_{n-1}\left(t\right)+S^{-1}\left\{\left\{1-\alpha -\alpha s\right\}S\left\{-x_{n-1}\left(t\right)y_{n-1}\left(t\right)-z_{n-1}\left(t\right)+1\right\}\left(s\right)\right\}\left(t\right)\end{array}\\ \begin{array}{l}{x}_0\left(t\right)=x\left(0\right)\\ y_0\left(t\right)=y\left(0\right)\\ z_0\left(t\right)=z\left(0\right)\end{array}\end{array}$$

(36)

And as before the approximate solution can be obtained as:

$$\{\begin{array}{l}x\left(t\right)=\underset{n\to \infty }{\lim }{x}_n\\ y\left(t\right)=\underset{n\to \infty }{\lim }{y}_n\\ z\left(t\right)=\underset{n\to \infty }{\lim }{z}_n\end{array}$$

The stability analysis and existence of exact solutions for this model can be achieved similarly as before. However using the iteration formula suggested in (36) with initial conditions and p = 0.3, we present the numerical solution of system (32) for different values of α in Figure 10, Figure 11 and Figure 12. The initial conditions are the same as before.

Unlike the model with Caputo and local derivative, the model with Caputo–Fabrizio portrays more chaotic behavior as α is closer to 1, however when α is closer to zero we obtain approximately the chaotic behavior observed from the model with local derivative. Figure 10 and Figure 11 show a new chaotic behavior, which are not shown by the local derivative.

5. Conclusions

The Vallis model for El Niño was considered with three-different definitions of derivative in this work. We used the local derivative in one model, the Caputo derivative with power function kernel with singularity and the Caputo–Fabrizio derivative with exponential kernel, which has no singularity [21,22,23,24]. We presented the existence and uniqueness of the model with local derivative and then we derived approximate solutions via iterative method. We presented some numerical simulation and observed some chaotic behavior as p changes from zero to 0.3. We derived the solution of the model with Caputo derivative using the combination of Laplace transform and iterative formula. We presented the stability analysis of the used method together with some numerical simulations. The model with Caputo–Fabrizio was solved with Sumudu transform operator. We observed that the Caputo derivative played a role of high band filter, when the Caputo–Fabrizio shows more interesting chaotic behavior as α tends to 1.