1. Introduction
Natural convection in enclosures has received considerable attention during the last several decades due to its effect on thermal performance in many engineering applications, including the operation of solar collectors, cooling of electronic equipment, hot- and chilled-water storage tanks, ovens, furnaces, and many others. Much research work, both theoretical and experimental, has been done on the convective heat transfer processes in two-dimensional enclosures, where the common model is the square or rectangular enclosure heated horizontally or vertically. The details of the natural-convection theory in enclosures has been presented in books by Minkowycz et al. [
1], Yang [
2], Pop and Ingham [
3], Bejan [
4], and Shenoy et al. [
5], among others (see Saeid and Yaacob [
6]). It seems, however, that little work has been done for the natural convection in cavities with boundary walls having nonuniform temperatures. Several studies (Vahl Davis [
7], Ostrach [
8], Hortman et al. [
9], and Barakos et al. [
10]) considered the natural convection in a differentially heated enclosure. Fu et al. [
11] studied numerically the natural convection in an enclosure with the heated wall of the enclosure divided into two, higher- and lower-temperature regions, and the temperature of the cold wall maintained at a constant. Their results show that the local Nusselt number distribution varies drastically at the intersection of the higher and lower-temperature regions. Xia et al. [
12] studied the buoyancy-driven laminar flow in a two-dimensional square enclosure when varying sinusoidal perturbation is imposed on the hot vertical wall. These authors found that the perturbations destabilized the flow, higher amplitudes lead to lower critical Rayleigh numbers for the flow transitions. Lakhal et al. [
13] studied numerically the transient heat transfer by laminar natural convection in a square cavity partially heated from below using a finite-difference procedure. The temperature of the heating element is uniform, but its magnitude varies sinusoidally with time, oscillating about a fixed mean value. The opposite cold wall is maintained at a constant temperature, while the rest of the bottom wall and the vertical walls are adiabatic. Several researchers have studied the periodic natural convection in two-dimensional enclosures. The phenomenon of natural convection in an enclosures heated periodically from the side has been considered by Lage and Bejan [
14] and Kwak et al. [
15]. The problem of steady natural convection in a two-dimensional enclosure with sinusoidal upper wall temperature has been studied numerically by Sarris et al. [
16]. The applied sinusoidal temperature is symmetric with respect to the mid-plane of the enclosure, and the bottom and side walls are adiabatic. It should be also mentioned that Sheremet and Pop [
17,
18] numerically analyzed free convection in a porous square [
17] and in a wavy [
18] cavity under the effect of sinusoidal temperature distributions on both side walls filled with a nanofluid using the mathematical nanofluid model proposed by Buongiorno [
19].
Calculation of entropy generation is an excellent tool to obtain the energy losses inside the system. Different types of thermal systems are related to the mechanism of irreversibility which can be described with the help of entropy generation and are relevant to viscous dissipation, magnetic field, heat and mass transfer, etc. To enhance this irreversibility process, various researchers used first law of thermodynamics but they found that the obtained results are unsatisfactory (see Bejan [
20,
21,
22]). Later, many authors used the second law of thermodynamics to optimise these irreversibilities and observed that second law of thermodynamics is more effcient and reliable as compared to the first law of thermodynamics. The entropy generation was investigated for different media. For instance, Rashidi et al. [
23] investigated entropy generation over a rotating permeable disk in a nanofluid under the influence of Magnetohydrodynamics (MHD) effect. They found that the disk source is the major point that helps in entropy generation. Further, Komurgoz et al. [
24] studied the magnetic field effect on the entropy generation within an inclined porous channel. It has been shown They found that maximum entropy generation can be achieved in the absence of zero magnetic fields and zero porosity. Butt and Ali [
25] examined the influence of irreversibility on unsteady free convective hydromagnetic flow with thermal radiation through a vertical porous plate. The entropy generation in a porous cavity under the influence of the magnetic field has been considered by Mahmud and Fraser [
26] and they concluded that with the increment in the magnetic field, entropy generation increases. Finally, we mention the recently published papers by Sheremet et al. [
27,
28] and Bondareva et al. [
29] on the entropy generation through natural convection heat transfer in nanofluids inside cavities using the mathematical nanofluid model proposed by Tiwari and Das [
30].
It is worth mentioning that the basic theory of entropy generation is widely presented in a seminal work by Bejan [
20,
21,
22,
23]. The application of entropy generation is also reviewed by Oztop and Al-Salem [
31]. A numerical study on mixed convection and entropy generation for a nanofluid filled cavity is given by Nayak et al. [
32]. The entropy generation and Bejan number are evaluated to demonstrate the thermodynamic optimization of the mixed convection and they showed that the heat transfer rate increases remarkably by the addition of nanoparticles. Selimefendigil and Oztop [
33] studied the effect of the magnetic field on natural convection and entropy generation in a cavity filled by a nanofluid having different shaped obstacles inside the cavity. They observed that averaged heat transfer reduces by 21.35%, 32.85% and 34.64% for the cavity with circular, diamond and squared shaped obstacles, respectively, compared to a cavity without obstacles at
Ra = 10
6. It is also important to point out the very recently published paper by Kefayati et al. [
34] on simulation of natural convection and entropy generation of non-Newtonian nanofluid in an inclined cavity using Buongiorno’s mathematical model (Part II, entropy generation). The review paper on entropy generation in nanofluids flow by Mahian et al. [
35] should also be mentioned in this context.
Cooling is one of the most important technical challenges facing many diverse industries, including microelectronics, transportation, solid-state lighting, and manufacturing. The review paper by Manca et al. [
36] notices that the heat transfer can be enhanced by increasing the thermal conductivity of the fluid. This is possible by adding small size metallic or oxyde-metallic particles to the base fluid. When the size of added particles is in the range of nanometers, the so called nanofluid is obtained and it was shown that this fluid posses enhanced thermal properties. (see Choi [
37]). It is worth mentioning that many references on nanofluids can be found in the books by Das et al. [
38], Nield and Bejan [
39], and Shenoy et al. [
5], and in the review papers by Buongiorno [
19], Buongiorno et al. [
40] and Mahian et al. [
41], etc. Several published papers, such as those by Sivasankaran and Pan [
42], Alsabery et al. [
43,
44], and Oztop et al. [
45] have considered the natural convection in cavities filled by nanofluids where the temperature on the boundaries is non-uniform, having a sinusoidal distribution.
The main objective of the present study is to analyze the natural convection combined with entropy generation in a square cavity filled with a nanofluid with sinusoidal temperature distribution on one side wall using Buongiorno’s nanofluid model [
19]. To our best of knowledge this problem has not been considered before, so that the reported results are new and original. Periodic heating has strong implications for the glass industry, where the main objective is to increase the mixing of the glass melt. Also, the active walls may be subject to non-uniform temperature distribution due to shading or other effects in different fields such as solar energy collection and cooling of electronic components.
2. Basic Equations
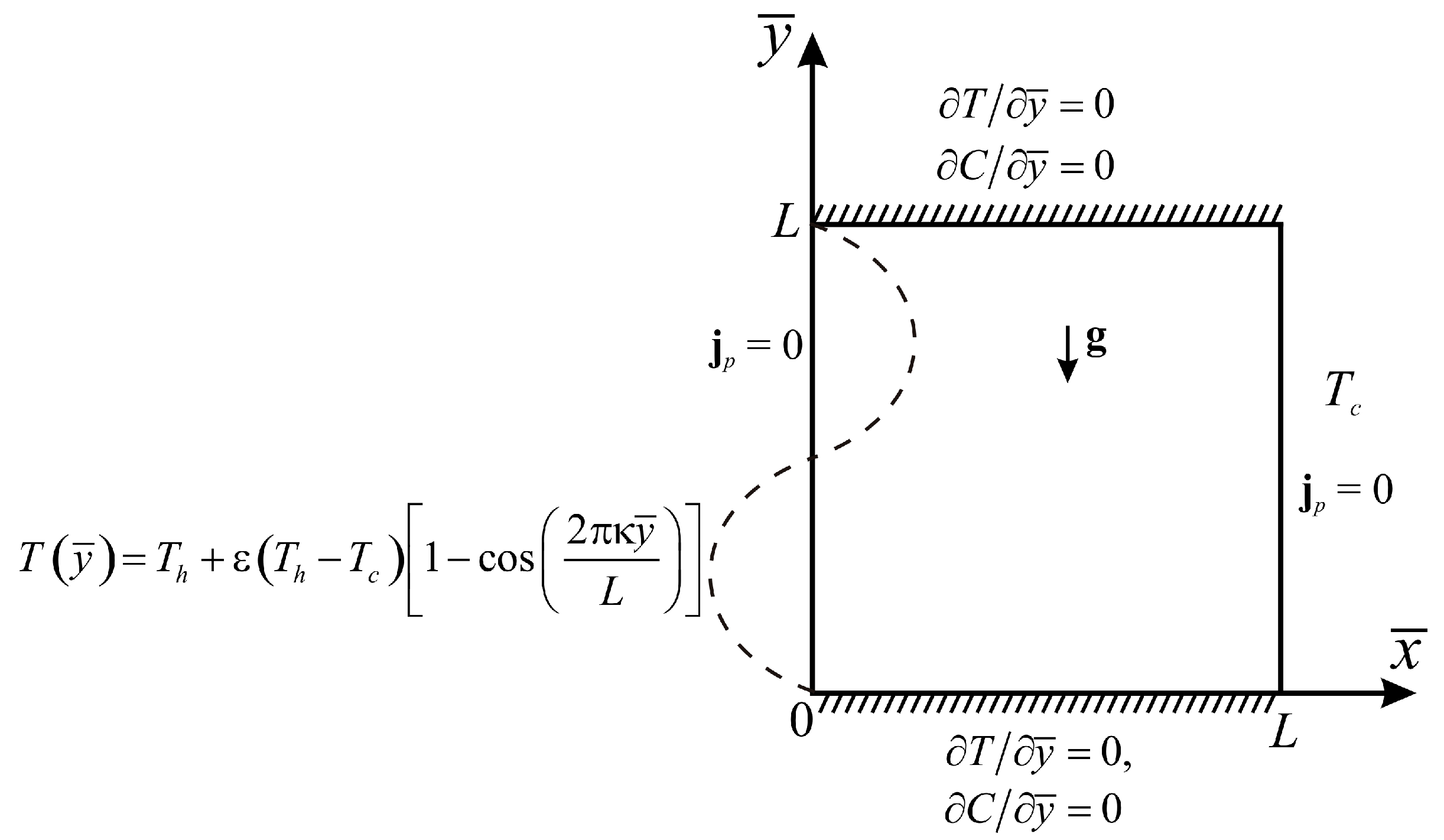
We consider the natural convective fluid flow and heat transfer within a square cavity filled with a nanofluid based on water and solid nanoparticles. A schematic geometry of the problem under consideration is shown in
Figure 1 with dimensional Cartesian coordinates, where
L is the size of the cavity. The cavity has adiabatic horizontal walls and the right vertical wall is kept at a constant temperature
Tc. The left vertical wall have variable temperature distribution according to the vertical coordinate as follows:
where
Th >
Tc,
ε is amplitude of the variable hot-wall temperature and
κ is wave number of the hot-wall temperature.
The thermophysical properties of the fluid are assumed constant, except for the density, the buoyancy force effect being described by the Boussinesq approximation. Under these assumptions, the governing equations for nanofluid can be written as follows taking into account the effects of Brownian diffusion and thermophoresis [
19]:
where
V is the velocity vector,
T is the nanofluid temperature,
C is the nanoparticle volume fraction,
t is the time,
p is the fluid pressure,
g is the gravity vector,
DB is the Brownian diffusion coefficient,
DT is the thermophoretic diffusion coefficient,
is the nanoparticles mass flux,
is the reference density of the fluid,
denote the thermal diffusivity of the nanofluid, the dynamic viscosity, nanoparticle mass density, respectively,
δ is a quantity defined by
,
is the heat capacity at constant pressure,
is heat capacity of the base fluid,
is effective heat capacity of the nanoparticle material and
β is the coefficient of thermal expansion.
Further on, we will linearize the momentum equation considering the Boussinesq approximation and dilute form of nanoparticle concentration [
17,
18]:
Governing Equations (2), (4)–(6) can be written using the stream function
and vorticity
in Cartesian coordinates
and
as
Introducing the following dimensionless variables:
where
and substituting (11) into Equations (7)–(10), we obtain:
Here ψ is the dimensionless stream function, ω is the dimensionless vorticity, x and y are the dimensionless coordinates, τ is the dimensionless time, θ is the dimensionless temperature, φ is the rescaled nanoparticles volume fraction.
Corresponding boundary conditions of these Equations (12)–(15) are given by:
The involved parameters in Equations (12)–(16) are: the Rayleigh number,
, the Prandtl number,
and the dimensionless nanoparticles mass flux,
. Further, the four parameters
Nr,
Nb,
Nt and
Le denote the buoyancy ratio parameter, the Brownian motion parameter, the thermophoresis parameter and the Lewis number, respectively, which are defined as:
The local and average Nusselt numbers along the left vertical wall can be defined as:
The definitions for local and average Sherwood numbers are similar to Nusselt numbers for left vertical wall taking into account the used boundary conditions, namely, or
The existence of some irreversible phenomena, such as, viscous dissipation and heat and mass transfer are measured by the entropy generation. The dimensional local entropy generation
is defined in the following form (see Sheremet et al. [
27,
28] and Bondareva et al. [
29]):
where
and
R is the universal gas constant.
Equation (19) includes four terms illustrating the local entropy generation due to the heat transfer (first term), the local entropy generation due to fluid friction (second term) and the local entropy generation due to mass transfer (third and fourth term).
The dimensionless local entropy generation
can be obtained as:
In Equation (20),
are the irreversibility factors. Those are defined as follows:
The integration of Equation (20) in the entire computational domain gives the dimensionless average entropy generation,
, expressed as follows:
Further, the Bejan number
Be is a parameter reflecting the importance of heat transfer irreversibility in the domain and is defined as:
The relative global dominance of heat transfer irreversibility is predicted by
(average Bejan number) which can be defined as:
3. Numerical Method and Validation
The unsteady partial differential Equations (12)–(15) with corresponding boundary conditions (16) were solved using the finite difference method (see Shenoy et al. [
5], Sheremet and Pop [
17,
18], Sheremet et al. [
27,
28]). The solution of these equations is marched in time until the steady-state flow is obtained. The convective and diffusive terms are approximated by the second-order discretizations. The transient equations were solved on the basis of Samarskii locally one-dimensional scheme. The linear discretized equations were solved by Thomas algorithm. The Poisson equation for the stream function was discretized by means of the five-point difference scheme on the basis of central differences for the second derivatives. The obtained linear discretized equation was solved by the successive over relaxation method. Optimum value of the relaxation parameter was chosen on the basis of computing experiments. The computation is finished when the residuals for the stream function get below 10
−7.
The performance of sinusoidal heating part of the model was compared with the results reported by Deng and Chang [
46], and Sivasankaran and Bhuvaneswari [
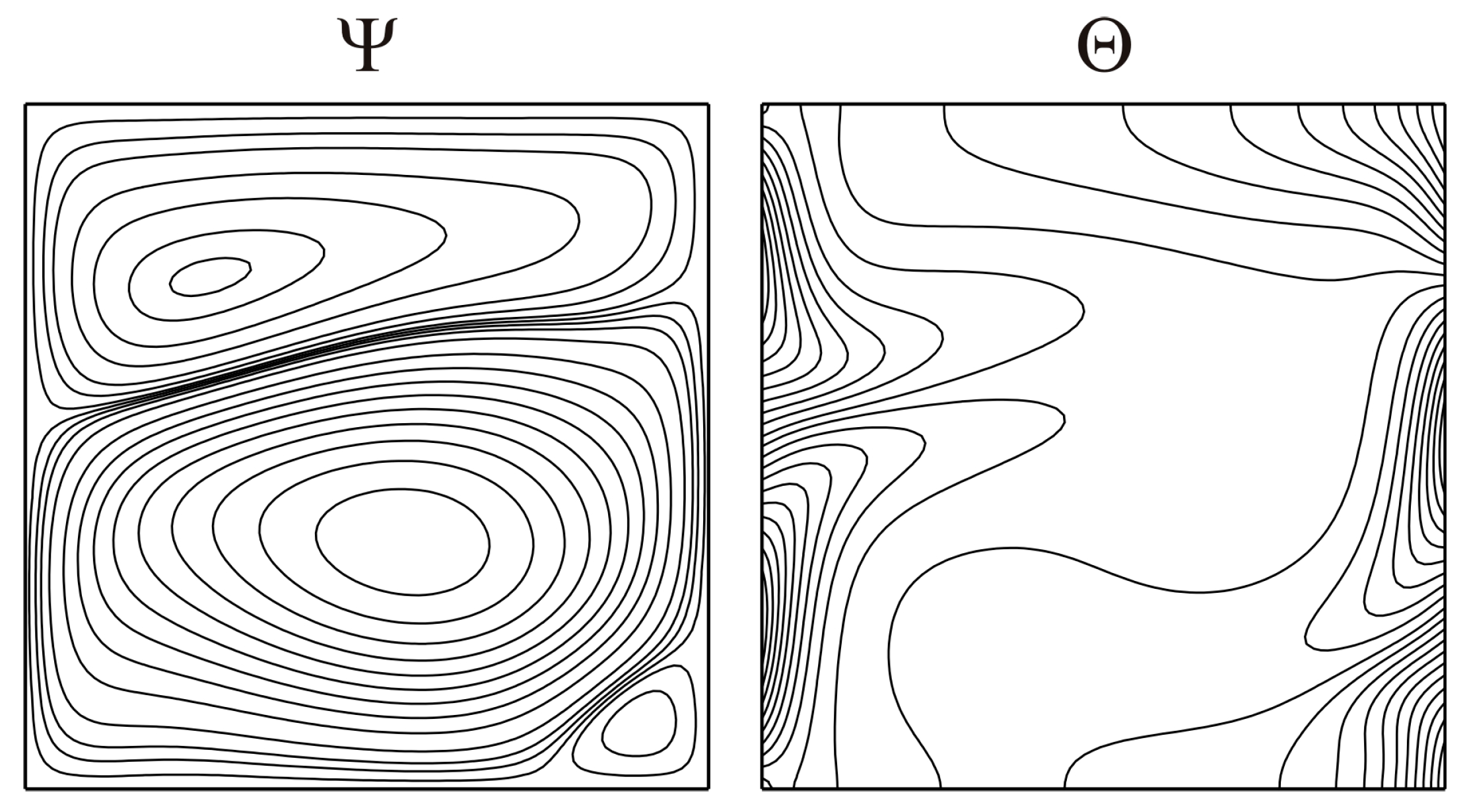
47] for the steady-state natural convection in a square cavity with sinusoidal heating at vertical walls filled with a regular fluid.
Figure 2,
Figure 3 and
Figure 4 show a good agreement between the obtained streamlines and isotherms for different Rayleigh numbers and the numerical data of Deng and Chang [
46] (see
Figure 3a,b and
Figure 11c in [
46]), and Sivasankaran and Bhuvaneswari [
47] (see
Figure 1b in [
47]). This give us the confidence that the proposed numerical scheme is correct and works efficiently.
The grid independent solution was performed by preparing the solution for steady-state natural convection in a square cavity with variable temperature distribution on left vertical wall filled with a nanofluid for
Ra = 1000,
Pr = 7.0,
Le = 1000,
Nr =
Nb =
Nt = 0.1,
ε = 1,
κ = 1,
χ1 = 0.0001,
χ2 = 0.5,
χ3 = 0.01. Values of the irreversibility factors are the same with those used in the paper by Magherbi et al. [
48]. Six cases of the uniform grid are tested: a grid of 100 × 100 points, a grid of 150 × 150 points, a grid of 200 × 200, a grid of 250 × 250 points, a grid of 300 × 300 points, and a grid of 400 × 400 points.
Table 1 shows the effect of the mesh parameters on the average Nusselt number on the hot wall. On the basis of the conducted verifications the uniform grid of 200 × 200 points has been selected for the following analysis.
4. Results and Discussion
In this section, we discuss the results obtained by numerical simulation of natural convection and entropy generation in a square cavity filled with a water based nanofluid considering a variable temperature along the left vertical wall under the effects of Brownian diffusion and thermophoresis. Analysis has been performed for the following values of the governing parameters: Rayleigh number (
Ra = 10
3–10
5), Prandtl number (
Pr = 7.0), Lewis number (
Le = 1000), buoyancy-ratio parameter (
Nr = 0.1), Brownian motion parameter (
Nb = 0.1), thermophoresis parameter (
Nt = 0.1), amplitude of the variable hot-wall temperature (
ε = 0.0–1.0), wave number of the hot-wall temperature (
κ = 0.0–5.0). Particular efforts have been focused on the effects of these parameters on the fluid flow, heat transfer and entropy generation inside the cavity. Streamlines, isotherms, nanoparticles volume fraction, entropy generation parameters as well as the average Nusselt number, fluid flow rate, average Bejan number and average entropy generation for different values of the key parameters mentioned above are illustrated in
Figure 5,
Figure 6,
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11,
Figure 12,
Figure 13 and
Figure 14.
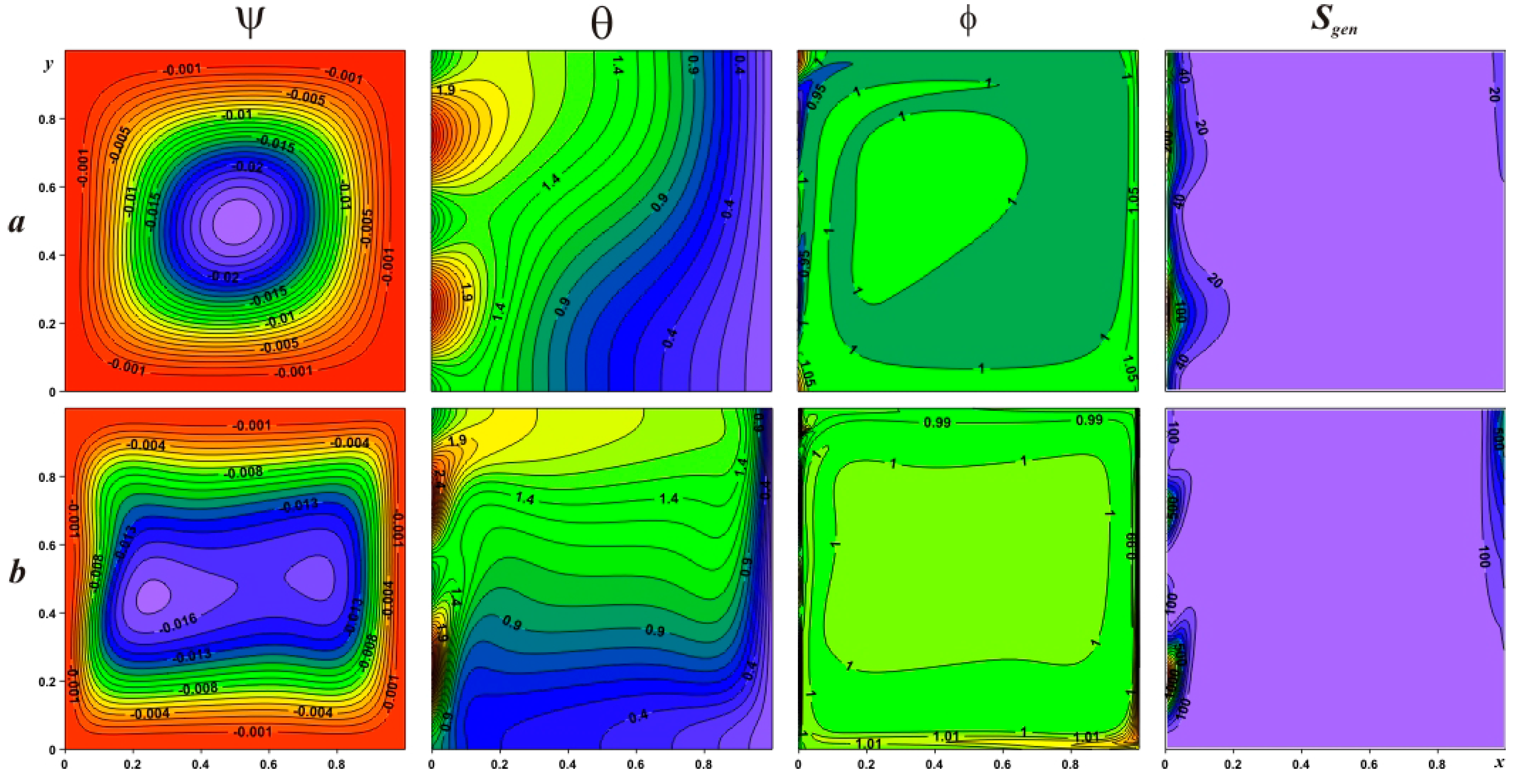
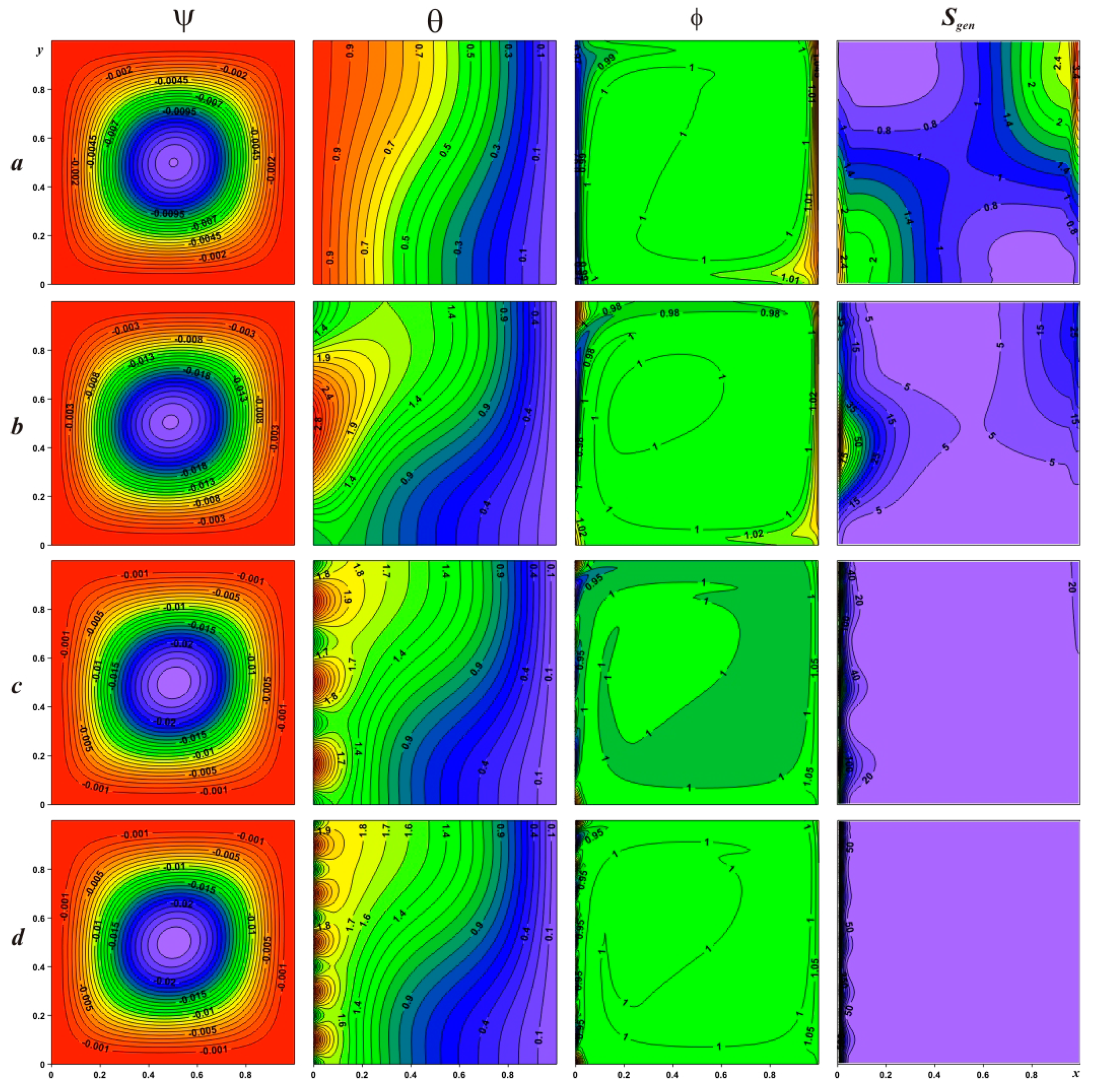
Figure 5 presents streamlines, isotherms, nanoparticles volume fraction and local entropy generation within the cavity for different values of Rayleigh number and for
ε = 1.0,
κ = 2.0. In the case of
Ra = 10
3 (
Figure 5a) one convective cell is formed inside the cavity illustrating an ascending flow near the left hot wall and descending flows close to the right cold wall. Distribution of temperature along the left wall has two local maximum points due to the value of the wave number,
κ = 2.0. At the same time, the low value of Rayleigh number shows that heat conduction dominates near the left wall, the isotherms being uniformly distributed in these zones. Domination of heat conduction reflects a significant effect of thermophoresis inside the cavity. Therefore, one can find non-uniform distribution of nanoparticles inside the cavity, where significant reduction of
φ occurs along the left hot wall and a growth of
φ occurs along the right cold wall. The distribution of local entropy generation illustrates an intensification of
Sgen along the left wall due to significant heat transfer part (high values of temperature gradient along this wall).
An increase in the Rayleigh number (
Figure 5b) leads to a formation of a convective cell with two cores similar to the case of the clear fluid (Vahl Davis [
7]) but here these cores are displaced due to the additional effects of Brownian diffusion and thermophoresis. The distribution of the temperature reflects the formation of a stratified zone in the central part of the cavity. Intensified convective flow and heat transfer illustrate non-uniform temperature distribution in heated zones along the left vertical wall. Distribution of nanoparticles is more uniform in comparison with the case of
Ra = 10
3. At the same time,
Sgen increases significantly along the vertical walls due to intensification of the convective flow and as a result a growth of temperature gradient appears.
Figure 6 shows profiles of local Nusselt number along the left vertical wall for different values of the Rayleigh number. Regardless of the Rayleigh number value, two local maxima of
Nu are along the wall in the heated zones. An increase in
Ra leads to significant growth of
Nu in these heated zones. At the same time, location of maxima goes weakly along the negative
y-coordinate. It should be noted, that bottom local maximum is a global maximum due to an interaction of hot flow from the left heated zone and cold flow from the right cooled wall.
Distributions of local parameters inside the cavity for
Ra = 10
3,
κ = 2.0 and different values of left wall temperature amplitude are presented in
Figure 7. For the considered range of
ε one can find non-significant changes in streamlines, while other parameters change significantly. High values of amplitude characterize more significant heating of the cavity due to an increase in temperature along the left vertical wall. Such heating leads to non-uniform distributions of nanoparticles inside the cavity and local entropy generation increases within the enclosure, mainly, close to the vertical walls.
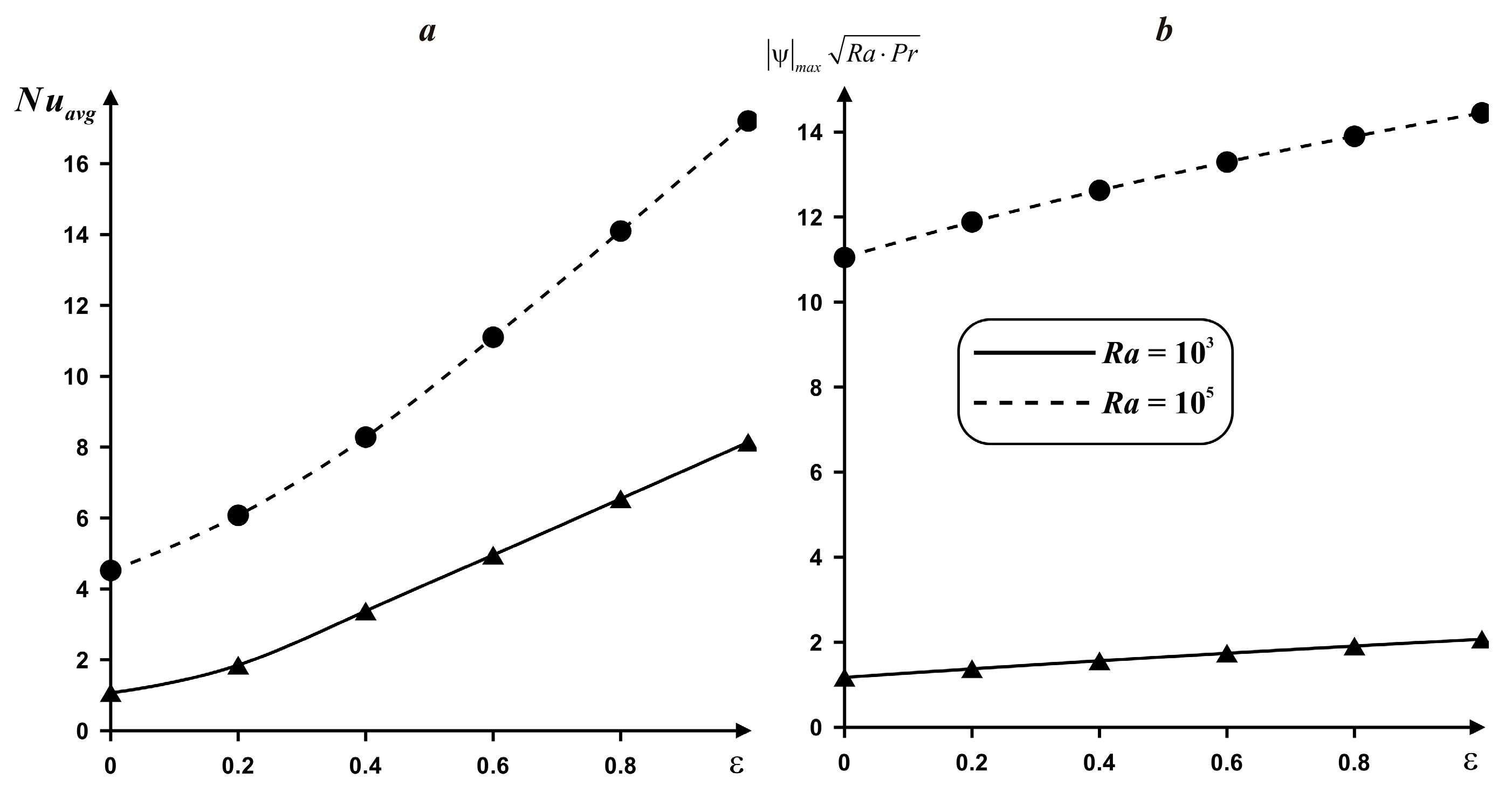
Profiles of local Nusselt number along the left vertical wall are shown in
Figure 8. An increase in
ε leads to a growth of local maxima and minima of
Nu, as a result, average Nusselt number at this wall is an increasing function of
ε (see
Figure 9a). At the same time, a rise of Rayleigh number also leads to a growth of average Nusselt number. It is worth noting that an increase in
Nuavg with
ε is more significant for high values of Rayleigh number. One can find also that fluid flow rate is an increasing function of wall temperature amplitude and Rayleigh number. Intensity of convective flow raises more significant for high values of Rayleigh number (see
Figure 9b).
The effects of
Ra and
ε on average Bejan number and average entropy generation are presented in
Figure 10. Average entropy generation is an increasing function of
Ra and
ε, while
Beavg decreases with
ε for low value of Rayleigh number (
Ra = 10
3) and increases with
ε for high value of Rayleigh number (
Ra = 10
5). Such behavior has been mentioned above, where high values of
Ra reflect more intensive convective flow and heat transfer with high values of temperature gradient. The behavior of average entropy generation is similar to average Nusselt number in the case of more significant growth for high
Ra.
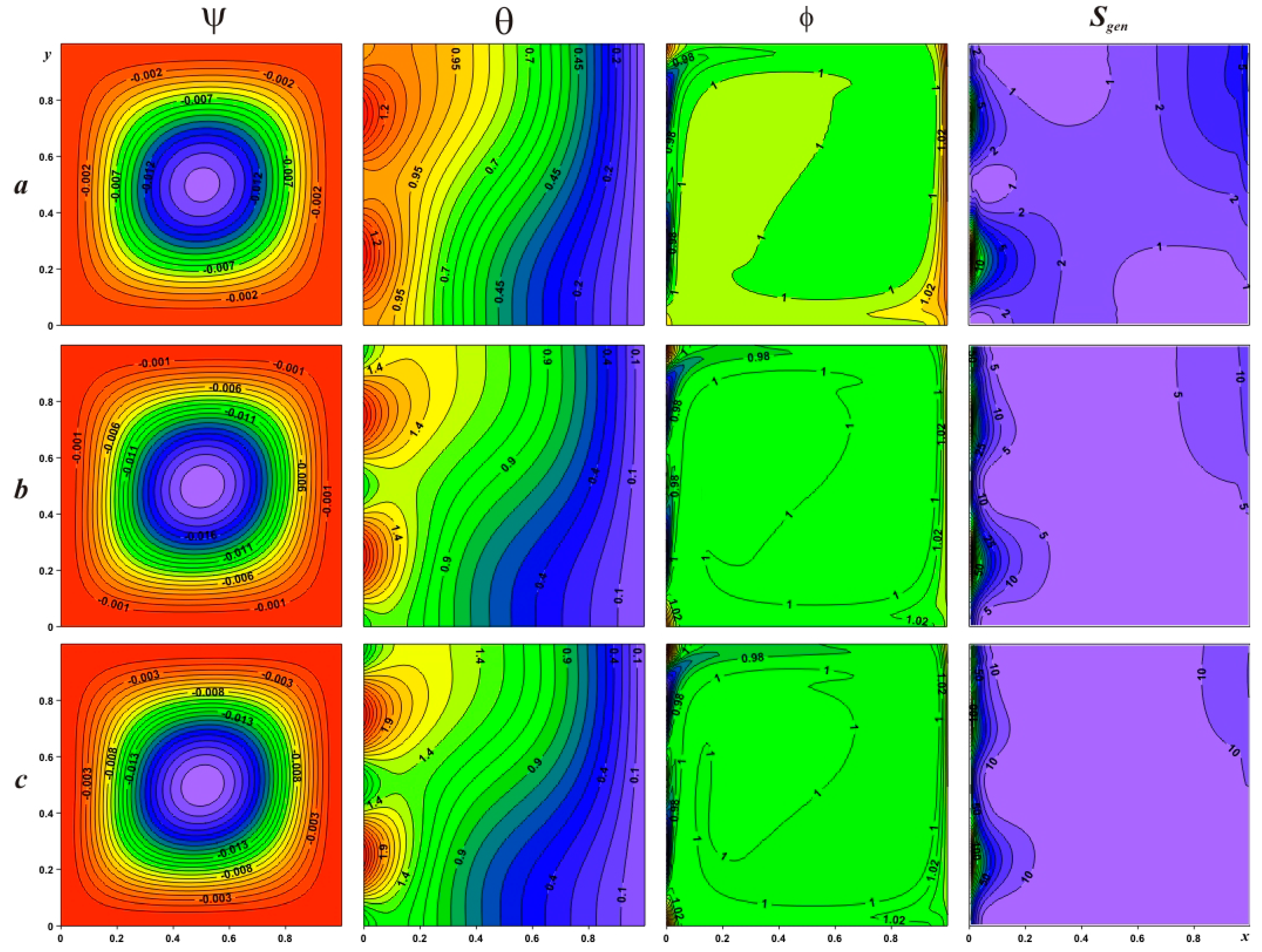
Effect of wave number on streamlines, isotherms, isoconcentrations and isolines of local entropy generation is presented in
Figure 11 for
Ra = 10
3,
ε = 1.0. It should be noted that a growth of
κ illustrates an increase in heated zones along the left vertical wall that is presented in distributions of temperature. An increase in wave number does not lead to significant modifications of streamlines, while temperature, nanoparticles volume fraction and entropy generation fields change significantly. A growth of
κ leads to more uniform heating of the left vertical wall and one can find more significant heating near this wall. Nanoparticles isoconcentrations become more uniform for high values of wave number. Local entropy generation increases also with
κ and significant growth occurs close the left vertical wall.
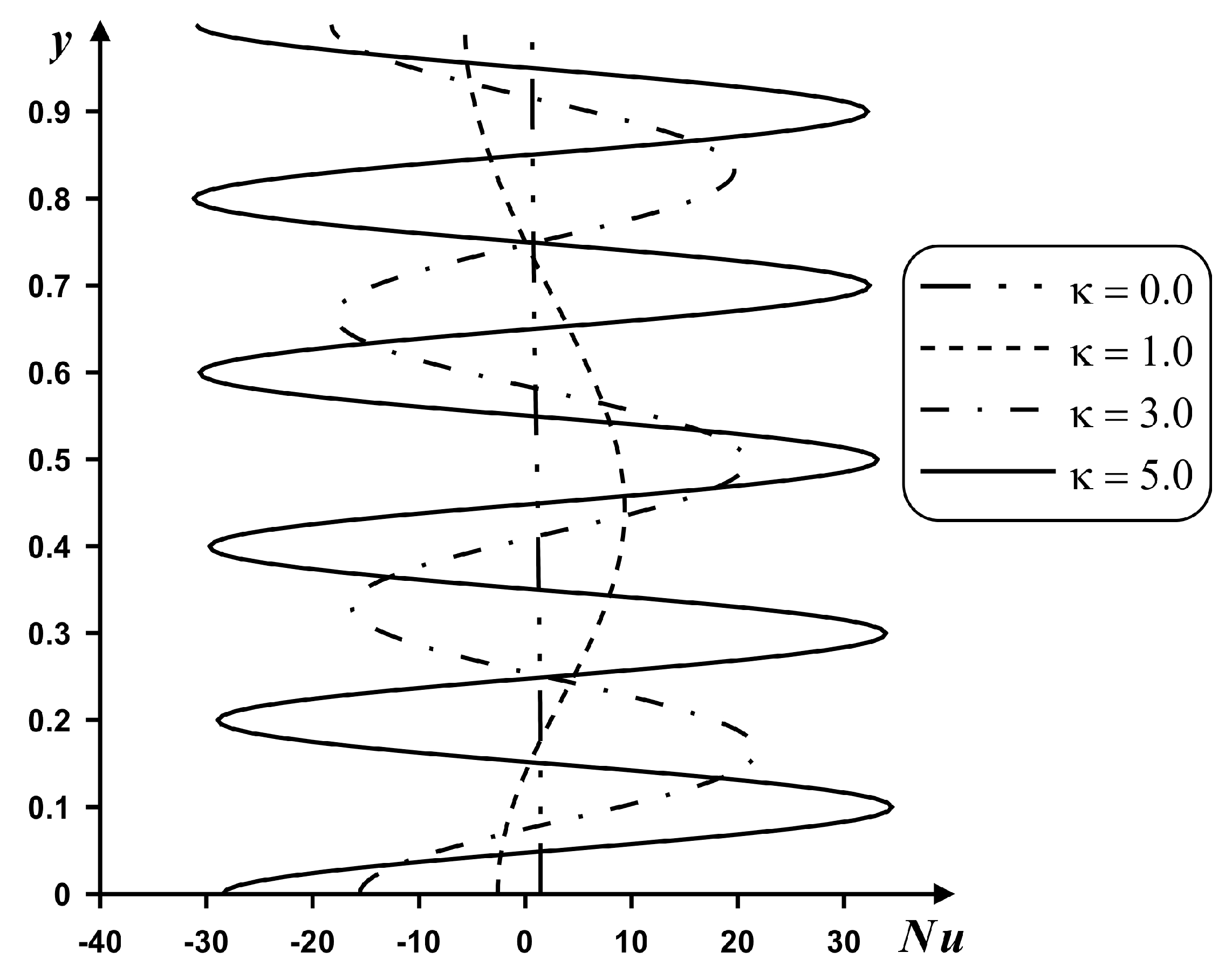
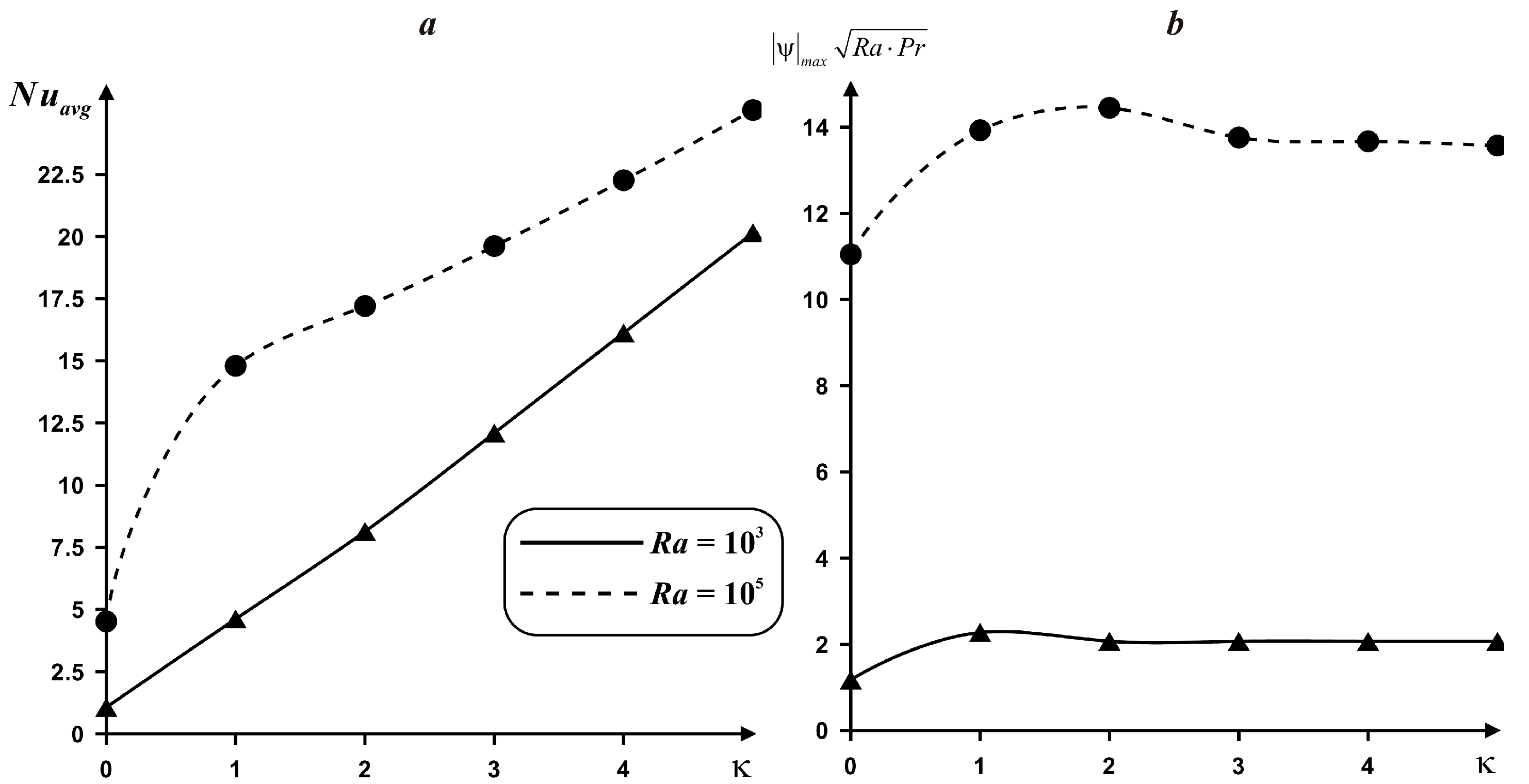
Profiles of local Nusselt number for different values of wave number are shown in
Figure 12. An increase in
κ leads not only to a rise of number of local maximum but also to a growth of amplitude of local Nusselt number. As a result, we have a significant increase in average Nusselt number at the left vertical wall (see
Figure 13a). Nanofluid flow rate increases also with
κ, but for
κ > 3 in considered range of Rayleigh number, the fluid flow rate is near constant. Local maximum in
is formed at greater value of wave number with a growth of Rayleigh number.
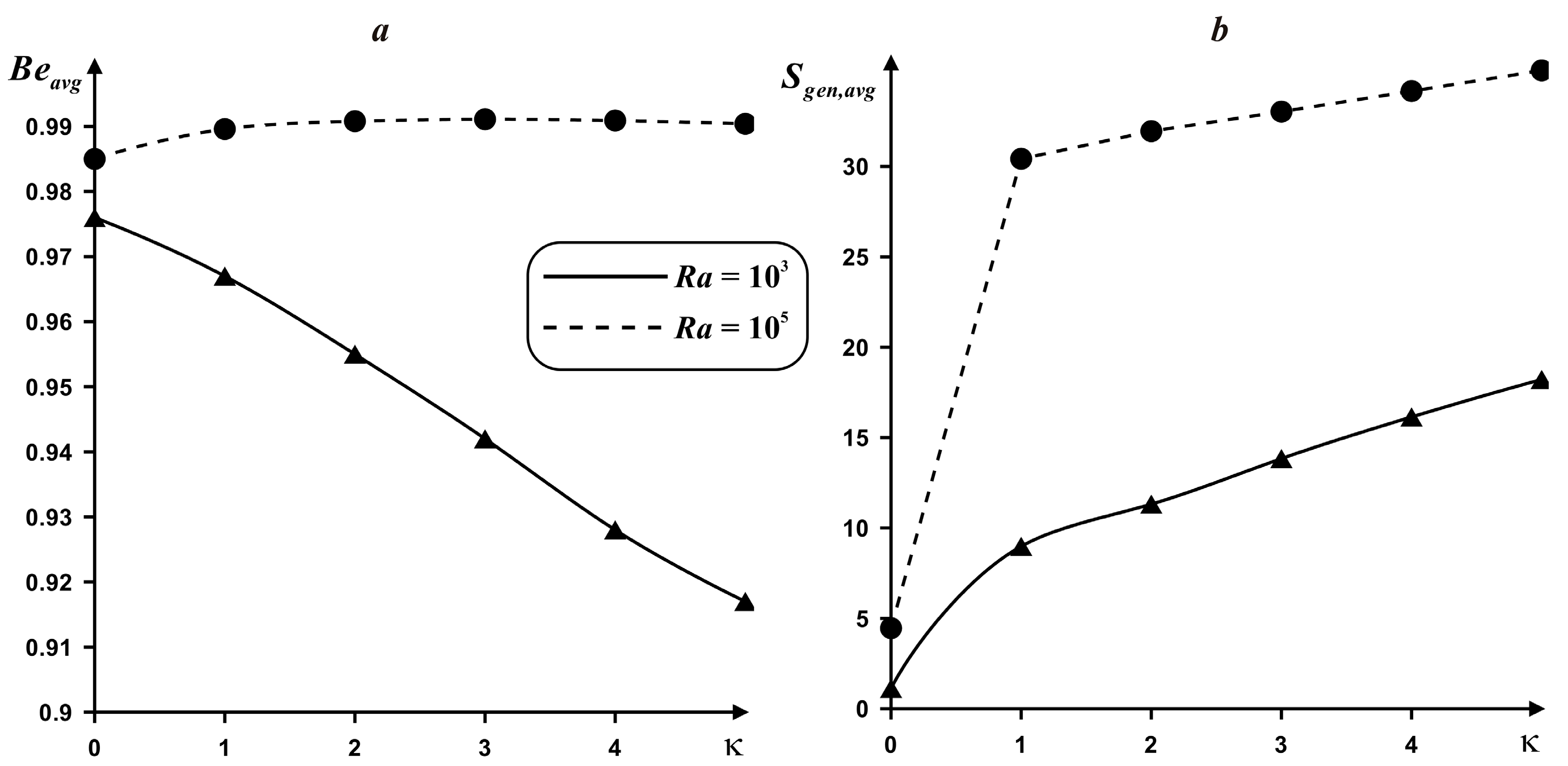
Behavior of the average Bejan number and the average entropy generation shown in
Figure 14 is similar to behavior presented in
Figure 10. It should be noted that low Rayleigh number (
Ra = 10
3) illustrates a decrease in average Bejan number with wave number due to a reduction of the heat transfer irreversibility for low intesive flow and heat transfer in comaprison with fluid friction irreversibility and mass transfer irreversibility. At the same time, the average entropy generation significantly increases with transition between constant temperature (
κ = 0) and variable temperature distribution (
κ = 1) for
Ra = 10
5 due to intesification of convective flow. Average Bejan number for high Rayleigh number characterizes a weak changes owing to significant role of heat transfer irreversibility.
Obtained results have been used to obtain the correlations for the average Nusselt number on the basis of a least-square regression technique in a wide range of governing parameters 0.2 ≤
ε ≤ 1.0, 1 ≤
κ ≤ 5 for
Ra = 10
3 and
Ra = 10
5 in the following form: