Paths of Cultural Systems
Abstract
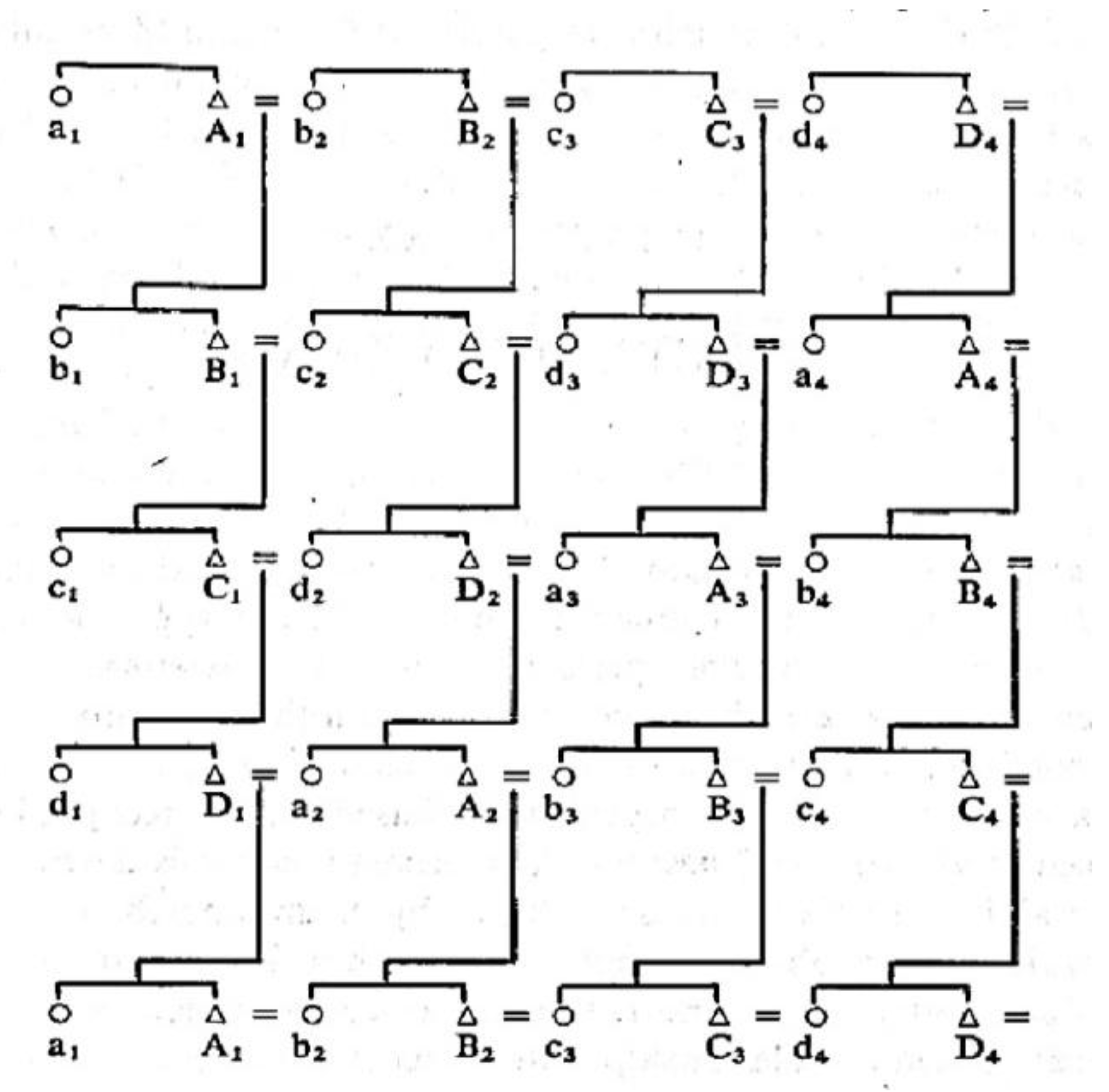
:1. Ethnographic Foundation
2. Basic Definitions
- (1)
- If there exists an a ∈ D such that a*x and a*y are defined and a*x = b and a*y = b then x = y, we call such object (D, *) a partially defined quasigroup, or pdq.
- (2)
- If (D, *) is a pdq and * is fully defined on D, then (D, *) is a (complete) quasigroup.
- (3)
- The pair L = (D, *) is a natural language with dictionary D whenever (D, *) is a pdq.
- (4)
- If L = (D, *) is a natural language, a kinship terminology is a quasigroup subset k ⊆ L.
- (1)
- A function f: X → Y is a homomorphism if f for all b, c ∈ X, f(b * c) = f(b) ° f(c).
- (2)
- If f: X → Z and g: Y → Z are homomorphisms then f and g are isotopic.
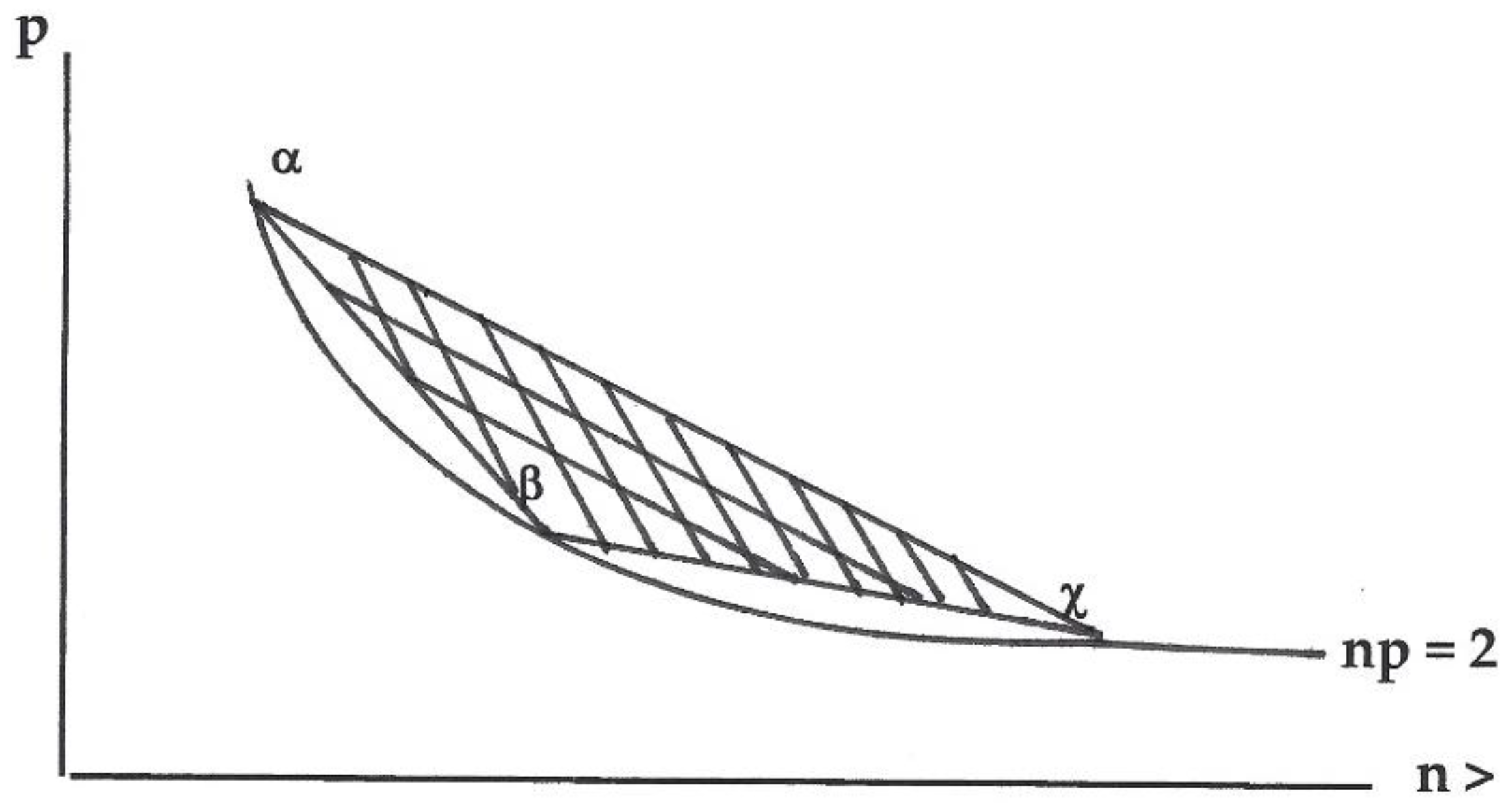
- From [2,5,6,8] and Appendix A Definition A4 each structural number s has a set of values ns and ps where nsps = 2, where ns is the average family size of a pure system of structural number s and ps is the proportion of reproducing adults of a pure system of structural number s. If history α has structural number s, then each α has modal demography (nα, pα) = (ns, ps) (see Appendix A Definition A7) where ps = 2/ns; for s ≥ 3 and sα ≠ sχ then (nα, pα) ≠ (nχ, pχ).
- Since H is finite, each non-empty set of viable histories H thus has a largest structural number smax with modal demography (nmax, pmax), and a smallest structural number smin with modal demography (nmin, pmin). Note that if nmax increases then pmax decreases (and as nmin decreases then pmin increases, since given s, nsps = 2, with 0 < p ≤ 1. Structural numbers s = 2 or 3 have identical modal demography (ns, ps) = (2, 1); all others structural numbers have distinct modal demographies see [5,8].
- The modal demography of history α with structural number sα is (nα, pα) = (ns, ps) is a set of values that represent the history α maintaining its modal demography with neither increase nor decrease in total empirical population size; it is prediction of nα and pα based on the determination that the structural number is s, and maintains the structural number s.
- n(t) = Σαvα(t)nα, α ∈ H, is the predicted average family size of Gt at t, given the vector state at t see [8]. Note that this is the average family size of the population at time t, given the vector state of each α ∈ Ht. This while the “size” of the minimal structure might be small, the size predicted by n(t) is the predicted actual size of the total population at t, not of the minimal structure; the minimal structure illustration “size” is dependent on the rules, not on the empirical size of the population.
- p(t) = Σαvα(t)pα, α ∈ H, is the predicted proportion of reproducing adults of Gt at t ascribed as married and reproducing, given the vector state of sα at t [8].
- Thus, all of the “demographics” of cultural theory discussed here are predictions on the result of maintaining or changing the vector states of t, given the SNSK determined values for each modal demography (nα, pα) at time t. Thus, [8] defineswhere r(t) ∈ R predicts an average rate of change of total population size between two generations of G, based on the vector state of structural numbers of the histories Ht ⊆ H. [8] showed that r(t) predicts changes in the probabilities v(t) imply cultural change is adiabatic.er(t) = 1/2n(t)p(t)
- Let H be a finite non-empty set of viable histories, and α, χ ∈ H. Using vα(t) = 1 − vχ(t), nα = 2/pα and nχ = 2/pχ, then Equation (1) becomeswhere:er(t) = 1 + (nαχ – 2)vα(t) + (2 – nαχ)vα(t)2is a constant determined by the values of nα and nχ; note nαχ = nχα.nαχ := (nα2 + nχ2)/(nαnχ)
3. Paths of Descent Sequences
- 1.
- r(t) ≥ 0, and r(t) = 0 if all structural numbers have the same modal demography or if all have structural numbers 2 or 3.
- 2.
- If α and χ are distinct structural numbers and at least one has structural number >3, then r(t) > 0 and r(t) has a maximum at v(t) = (0.5, 0.5).
- 3.
- Given any finite non-empty set H of two or more viable histories, there is a unique maximum r(t), given by (ii).
4. Pictures of States on Descent Sequences
- 1.
- (nt|:= (nα, …, nχ) be a row vector;
- 2.
- |pt) := (pα,…, …, pχ) be a column vector;
- 3.
- for all α, χ∈ H, arranging the sum of the inner product (nt|pt) as a square matrix then for all α, χ∈ H, H(t): = [nαpχ] is a demographic picture (analogous to a Heisenberg picture in physics) at t;
- 4.
- the square matrix we get by arranging the products of v(t)v(t)T as V(t) := [vαχ(t)] is a probability picture (analogous to a Schroedinger picture in physics) of the vector state of a descent sequence at t;
- 5.
- for ε ≥ 0 let V(Δ(t)) := V(t + ε) − V(t) = [vαχ(t + ε) − vαχ(t)] := [Δαχ(t)]. (Notice that −1 ≤ Δαχ(t) ≤ 1).We note [25] for our analogy of terminology.
5. Comments on Demographic Pictures
- The result nα = nχ occurs if structural numbers sα, sχ are <4 or whenever sα = sχ; so r(t) = 0. Otherwise, then Lemma 2 implies Lemma 1, which says that er(t) ≠ 1, and thus r(t) > 0. This occurs since nαpχ does not equal nχpα; thus from Lemma 1 and [8] the off-diagonal elements of ½H(t) implies adiabatic change in r(t).
- In discussions in physics, when nαpχ ≠ nχpα some claim that the resulting r(t) is “not commutative”. In physics, the “non-commutative” result actually means switching which experiment is taken, then comparing their results; in physics when changing the order of the products it also means changing the experiment; but this comparison of the two results also creates an equation that looks like our Equations (1), (2), (7) or (8). However, in physics reversing the experiment causes different measurements, which causes the physical uncertainty between the two results. In contrast, the seemingly “non-commuting” values in culture theory exist because the equation for computing r(t) requires computing both “directions” of the modal demography of histories in H (similar to comparing both directions of the physics model), and if any two (or more) of those have histories of distinct structural numbers (at least one >3), so that one or more nαχ > 2 (see Equation (3)), then nχpα ≠ nαpχ. Culture theory thus predicts adiabatic demographic change, not uncertainty, from a mechanism similar to that which causes uncertainty in physics.
6. Comments on Probability Pictures
- Let 2Ht = {α, χ} ⊆ Ht be a two-history subset of Ht. Let R(t) = ½[rij(t)] be a projection, let r1(t), r2(t), and r3(t) be real numbers such that r1(t)2 + r2(t)2 + r3(t)2 = 1, such that 0 ≤ r1(t) < 1, 0 ≤ r2(t) < 1, and such that vα(t) = ½r1(t) = ½(1 + r3(t)). Then R(t) is the status of Gt.
- A unit circle C is meant a set of points (x, y) in the plane R2 which satisfy the equation x2 + y2 = 1.
- (1)
- trR(t) = 1;
- (2)
- vχ(t) = ½(1 − r3(t));
- (3)
- the vector state v(t) of 2Ht is given by the main diagonal of R(t);
- (4)
- r(t) is a maximum when r3 = 0.
7. Discussion
Conflicts of Interest
Appendix A. Mathematical Background from Previous Papers
- (i)
- We call a pair (n, k) an assignment.
- (ii)
- A set of assignments is a selection denoted by A with subsets A ⊆ A. To specify more detail of the membership of a set A we may also use subscripts or a square bracket notation [n, k] with subscripts as required.
- (iii)
- [n, k] := {(n, k)|given a positive integer n, (n, k) where 1 ≤ k ≤ n}.
- (iv)
- [n, k]j := {(n, k)|given a finite positive integer j, (n, k) where 1 ≤ n ≤ j and for each n, 1 ≤ k ≤ n}.
- (v)
- [n, k]j,i := {(n, k)|given finite integers i, j where i ≥ j, (n, k) for 1 ≤ n ≤ j and 1 ≤ k ≤ i}.
- (vi)
- Pj := P([n, k]j) denotes the set of subsets of [n, k]j.
- (vii)
- If (n1, k1), (n2, k2) are assignments such that n1 ≠ n2 or k1 ≠ k2, then (n1, k1) and (n2, k2) are distinct assignments.
- (viii)
- If A is a set of assignments, is a unary relation on A ⊆ A such that A’ := A\A.If (n, k) is an assignment, then
- (ix)
- n := n/k is the average family size of (n, k).
- (x)
- p := 2/n is the reproductive ratio of (n, k).
- (i)
- S(n, k) is a Stirling Number of the Second Kind, whereis the number of ways to partition a set of n distinct elements into k nonempty subsets. S(n, k) computes the number of ways to achieve an assignment (n, k) for n individuals in a generation partitioned into k = βt = μt−1 ≥ sα non-empty subsets [J] which we call families. Then:
- (ii)
- S[n, k] := {S(n, k)|for given n, S(n, k), k = 1, …, n} is called a distribution.
- (iii)
- Given a distribution S[n, k], then [n, k] := {(n, k)| for given n, k = 1, …, n} is the underlying selection of S[n, k].
- (iv)
- n↑ := {j | given n}.
- (v)
- n↑s := {n↑ | j = s, for a given s > 0}.
- (vi)
- S[n, n↑s] := {S(n, n↑)|given s, n↑ ∈ n↑s}.
- (vii)
- Ns := n|S(n, n↑) = max(S[n, n↑s]).
- (viii)
- A[s] := ∪ [n, k] for n such that n↑ ∈ n↑s and for each such n, 1 ≤ k ≤ n, called the minimal collection of s.
- (ix)
- AM := ∪ A[s], given a positive integer M, for structural numbers 1 < s ≤ M.
- (x)
- ms := (Ns, s) is the modal assignment for s.
- (xi)
- As := {ms | s ∈ S} is the set of modal assignments for s ∈ S.
- (xii)
- ns := Ns/s is the modal average family size for s.
- (xiii)
- ps := 2/ns is the modal reproductive ratio for s.
- (xiv)
- (ns, ps) is the modal demography of s.
References
- Ballonoff, P. Structural statistics: Models relating demography and social structure with applications to Apache and Hopi. Soc. Biol. 1973, 10, 421–426. [Google Scholar] [CrossRef]
- Ballonoff, P. Theory of Lineage Organizations. Am. Anthropol. 1983, 85, 79–91. [Google Scholar] [CrossRef]
- Ballonoff, P. Mathematical Demography of Social Systems. In Progress in Cybernetics and Systems Research; Trappl, R., Pichler, F.R., Eds.; Hemisphere Publishing: Washington, DC, USA, 1982; Volume 10, pp. 101–112. [Google Scholar]
- Ballonoff, P. Mathematical Demography of Social Systems, II. In Cybernetics and Systems Research; Trappl, R., Ed.; North Holland Publishing Co: Amsterdam, The Netherlands, 1982; pp. 555–560. [Google Scholar]
- Ballonoff, P. MV-Algebra for Cultural Rules. Int. J. Theor. Phys. 2008, 47, 223–235. [Google Scholar] [CrossRef]
- Ballonoff, P. Some Properties of Transforms in Culture Theory. Int. J. Theor. Phys. 2010, 49, 2998–3004. [Google Scholar] [CrossRef]
- Ballonoff, P. Cross-Comment on Terminologies and Natural Languages. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2012; Volume 3, pp. 1–15. [Google Scholar]
- Ballonoff, P. Manuals of Cultural Systems. Int. J. Theor. Phys. 2014, 53, 3613–3627. [Google Scholar] [CrossRef]
- Levi-Strauss, C. Elementary Structures of Kinship; Beacon Press: Boston, MA, USA, 1969. [Google Scholar]
- Radcliffe-Brown, A.R. A Natural Science of Society; Free Press: Glencoe, UK, 1948. [Google Scholar]
- Special Issue on Australian Systems. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2013; Volume 5, Available online: http://mathematicalanthropology.org/toc.html (accessed on 18 December 2017).
- Dravidian Kinship Analysis. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2010; Volume 3, Available online: http://mathematicalanthropology.org/toc.html (accessed on 18 December 2017).
- White, H.C.; Coleman, J. An Anatomy of Kinship; Prentice Hall: Englewood Cliffs, NJ, USA, 1963. [Google Scholar]
- Chiara, D.M.; Guintini, R.; Greechie, R. Reasoning in Quantum Theory, Sharp and Unsharp Quantum Logics; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2004. [Google Scholar]
- Hirshleifer, J. Economics from a biological viewpoint. J. Law Econ. 1977, 20, 1–52. [Google Scholar] [CrossRef]
- Denham, W. Kinship, Marriage and Age in Aboriginal Australia. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2012; Volume 4, pp. 1–79. [Google Scholar]
- Cargal, J.M. An Analysis of the Marriage Structure of the Murngin Tribe of Australia. Behav. Sci. 1983, 23, 157–168. [Google Scholar] [CrossRef]
- Greechie, R.; Ottenheimer, M. An Introduction to a Mathematical Approach to the Study of Kinship. In Genealogical Mathematics; Ballonoff, P., Ed.; Mouton: Paris, France, 1974; pp. 63–84. [Google Scholar]
- Gottscheiner, A. On some classes of kinship systems, I: Abelian systems. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2008. [Google Scholar]
- Gottscheiner, A. On some classes of kinship systems, II: Non-Abelian systems. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2008. [Google Scholar]
- Kronenfeld, D. (Ed.) A New System for the Formal Analysis of Kinship; University Press of America: Lanham, MD, USA, 2000. [Google Scholar]
- Barbosa de Almeida, M.W. On the Structure of Dravidian Relationship Systems. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2010; Volume 3, pp. 1–43. [Google Scholar]
- Barbosa de Almeida, M.W. Comment on Vaz’ “Relatives, Molecules and Particles”. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2014; Volume 6, pp. 1–9. [Google Scholar]
- Hildon, P.; Peterson, J.; Stiger, J. On Partitions, Surjections and Stirling Numbers. In Bulletin of the Belgian Mathematical Society; Belgian Mathematical Society: Brussels, Belgium, 1994; Volume 1, pp. 713–735. [Google Scholar]
- Foulis, D.J. Effects, Observables, States and Symmetries in Physics. Found. Phys. 2007, 37, 1421–1446. [Google Scholar] [CrossRef]
- Jordan, T.F.; Jagannathan, K. Quantum Mechanics in Simple Matrix Form; Dover Publications: Mineola, NY, USA, 1986. [Google Scholar]
- Beltrametti, E.; Cassinelli, G. The Logic of Quantum Mechanics; Addisson-Wessley: London, UK, 1981. [Google Scholar]
- Beim Graben, P. Comment on Gil’s “What are the best hierarchical organisations for the success of a common endeavor”. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2016; Volume 9, pp. 1–4. [Google Scholar]
- Gil, L. What are the best hierarchical organizations for the success of a common endeavor? In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, 2016; Volume 9, pp. 1–24. [Google Scholar]
- Blutner, R.; Beim Graben, P. Dynamic Semantics and the Geometry of Meaning. Available online: https://pdfs.semanticscholar.org/a4ce/22edc62ff93305dde18d70edaad96fc220d3.pdf (accessed on 21 December 2017).
- Wang, Z.; Busemeyer, J.R. A Quantum Question Order Model Supported by Empirical Texts of an A Priori and Precise Prediction. Top. Cogn. Sci. 2013, 5, 689–710. [Google Scholar] [PubMed]
- Cargal, J.M. Reflections on the Algebraic Representations of Kinship Structure. In Mathematical Anthropology and Cultural Theory; 1and1.com: Philadelphia, PA, USA, 2017; Volume 11, pp. 1–5. [Google Scholar]
- Leaf, M. Personal communication, 2015.
- Wright, S. Evolution and the Genetics of Populations. In The Theory of Gene Frequencies; University of Chicago Press: Chicago, IL, USA, 1969. [Google Scholar]
© 2017 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ballonoff, P. Paths of Cultural Systems. Entropy 2018, 20, 8. https://doi.org/10.3390/e20010008
Ballonoff P. Paths of Cultural Systems. Entropy. 2018; 20(1):8. https://doi.org/10.3390/e20010008
Chicago/Turabian StyleBallonoff, Paul. 2018. "Paths of Cultural Systems" Entropy 20, no. 1: 8. https://doi.org/10.3390/e20010008




