On the Estimation of the Remainder Term in Møller-Plesset MP2 Theory from Limited Configuration Interaction
Abstract
:1 Introduction
2 The remainder term in MP2 theory
3 Estimation of the remainder term from limited configuration interaction

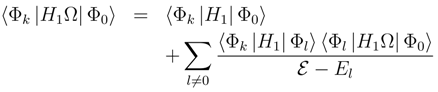

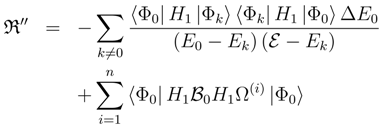
4 Discussion
Acknowledgements
References
- Møller, C.; Plesset, M.S. Phys. Rev. 1934, 46, 618. [CrossRef] [Green Version]
- Moncrieff, D.; Wilson, S. J. Phys. B: At. Mol. Opt. Phys. 1999, 32, 5379.
- Quiney, H.M.; Moncrieff, D.; Wilson, S. Quantum Systems in Chemistry and Physics. Basic Problems and Model Systems; Hernandez-Laguna, A., Maruani, J., McWeeny, R., Wilson, S., Eds.; Kluwer Academic: Dordrecht, 2000; p. 127. [Google Scholar]
- Wilson, S. Specialists Periodical Reports, Chemical Modelling: Applications and Theory 2000, 1, 364.
- Wilson, S.; Hubač, I. Molec. Phys. 2001, 99, 1813.
- Löwdin, P.O. J. Chem. Phys. 1951, 19, 1396.
- Löwdin, P.O. J. Molec. Spectros. 1963, 10, 12.
- Löwdin, P.O. J. Molec. Spectros. 1964, 13, 326.
- Löwdin, P.O. J. Math. Phys. 1962, 3, 969.
- Löwdin, P.O. J. Math. Phys. 1962, 3, 1171.
- Löwdin, P.O. J. Molec. Spectros. 1964, 14, 112.
- Löwdin, P.O. J. Molec. Spectros. 1964, 14, 119.
- Löwdin, P.O. J. Molec. Spectros. 1964, 14, 131.
- Löwdin, P.O. J. Math. Phys. 1965, 6, 1341.
- Löwdin, P.O. Phys. Rev. A 1965, 139, 357.
- Löwdin, P.O. Perturbation Theory and its Application to Quantum Mechanics; Wilcox, C.H., Ed.; John Wiley and Sons: New York, 1966. [Google Scholar]
- Löwdin, P.O. Intern. J. Quantum Chem. 1968, 2, 867.
- Löwdin, P.O.; Goscinski, O. Intern. J. Quantum Chem. 1971, 5, 685.
- Hubač, I.; Wilson, S. Adv. Quantum Chem. 2001, 39, 210.
- Lennard-Jones, J.E. Proc. Roy. Soc. Lond. 1930, A129, 598.
- Brillouin, L. J. Physique 1932, 7, 373.
- Wigner, E.P. Math. naturw. Anz. ungar. Akad. Wiss. 1935, 53, 475.
- Hubač, I.; Wilson, S. J. Phys. B: At. Mol. Opt. Phys. 2000, 33, 365.
- Lippmann, B.P.; Schwinger, J. Phys. Rev. 1950, 79, 469.
- Eden, R.J.; Francis, N.C. Phys. Rev. 1955, 97, 1366.
- Lindgren, I. J. Phys. B: At. Mol. Phys. 1974, 7, 2441.
- Lindgren, I. Int. J. Quant. Chem. Symp. 1978, 12, 33.
- Hubač, I.; Mach, P.; Wilson, S. J. Phys. B: At. Mol. Opt. Phys. 2000, 33, 4735.
- Hubač, I.; Mach, P.; Wilson, S. Molec. Phys. 2001, 100, 859.
- Hubač, I.; Mach, P.; Wilson, S. Adv. Quantum Chem. 2001, 39, 225.
© 2002 by MDPI, Basel, Switzerland. Reproduction for noncommercial purposes permitted.
Share and Cite
Hubac, I.; Wilson, S. On the Estimation of the Remainder Term in Møller-Plesset MP2 Theory from Limited Configuration Interaction. Int. J. Mol. Sci. 2002, 3, 570-578. https://doi.org/10.3390/i3050570
Hubac I, Wilson S. On the Estimation of the Remainder Term in Møller-Plesset MP2 Theory from Limited Configuration Interaction. International Journal of Molecular Sciences. 2002; 3(5):570-578. https://doi.org/10.3390/i3050570
Chicago/Turabian StyleHubac, I., and S. Wilson. 2002. "On the Estimation of the Remainder Term in Møller-Plesset MP2 Theory from Limited Configuration Interaction" International Journal of Molecular Sciences 3, no. 5: 570-578. https://doi.org/10.3390/i3050570



