Partial Cooperative Equilibria: Existence and Characterization
Abstract
:1. Introduction
2. Preliminaries
3. Network Formation with Consent
- (i)
- Every Nash equilibrium of Γ induces the empty network.
- (ii)
- For each group such that , there exists a partial cooperative Cournot-Nash equilibrium of that results in the formation of an efficient nonempty network.
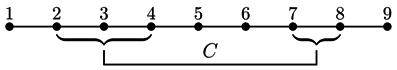
4. Axiomatization of the Partial Cooperative Cournot-Nash Equilibrium
4.1. More Definitions
4.2. Axioms
4.3. Characterization
5. Existence in Supermodular Games
5.1. Supermodular Games
- (i)
- f is supermodular in w for each fixed θ, i.e., for any fixed , and for any w and in W, we have
- (ii)
- f satisfies increasing differences in .
- is a sublattice of some Euclidean space;
- is supermodular on for each ;
- has increasing differences in .
5.2. The Partial Cooperative Stackelberg Equilibrium
- (a)
- X is finite.
- (b)
- is finite and, for all , is monotone increasing (or monotone decreasing) in , i.e., implies (or
5.3. The Partial Cooperative Cournot-Nash Equilibrium
6. Conclusions
Acknowledgments
References
- Mallozzi, L.; Tijs, S. Conflict and Cooperation in Symmetric Potential Games. Int. Game Theor. Rev. 2008a, 10, 245–256. [Google Scholar] [CrossRef]
- Mallozzi, L.; Tijs, S. Partial Cooperation and Non-Signatories Multiple Decision. AUCO Czech Econ. Rev. 2008b, 2, 21–27. [Google Scholar]
- Mallozzi, L.; Tijs, S. Stackelberg vs. Nash assumption in IEA Models. J. Opt. Appl. (Forthcoming).
- Ray, D.; Vohra, R. Equilibrium Binding Agreements. J. Econ. Theory 1997, 73, 30–78. [Google Scholar] [CrossRef]
- Ray, D. A Game Theoretic Perspective of Coalition Formation; Oxford University Press: New York, NY, USA, 2007. [Google Scholar]
- Chakrabarti, S.; Gilles, R.P.; Lazarova, E. Partial Cooperation in Symmetric Games; School of Management and Economics, Queen’s University Belfast: Belfast, UK, 2009; (Working Paper). [Google Scholar]
- Chander, P.; Tulkens, H. A Core of an Economy with Multilateral Environmental Externalities. Int. J. Game Theory 1997, 26, 379–401. [Google Scholar] [CrossRef]
- Deneckere, R.; Davidson, C. Incentives to Form Coalitions with Bertrand Competition. RAND J. Econ. 1985, 16, 473–486. [Google Scholar] [CrossRef]
- Duggan, J. Non-Cooperative Games among Groups; Department of Political Science and Department of Economics, University of Rochester: Rochester, NY, USA, 2001; (Working Paper). [Google Scholar]
- Salant, S.W.; Switzer, S.; Reynolds, R.J. Losses from Horizontal Merger: The Effects of Exogenous Change in the Industry Structure on Cournot-Nash Equilibrium. Quart. J. Econ. 1983, 98, 184–199. [Google Scholar] [CrossRef]
- Beaudry, P.; Cahuc, P.; Kempf, H. Is it Harmful to Allow Partial Cooperation. Scand. J. Econ. 2000, 102, 1–21. [Google Scholar] [CrossRef]
- Carraro, C; Siniscalco, D. The International Dimension of Environmental Policy. Eur. Econ. Rev. 1992, 36, 379–387. [Google Scholar]
- Carraro, C.; Siniscalco, D. Strategies for International Protection of the Environment. J. Public Econ. 1993, 52, 309–328. [Google Scholar] [CrossRef]
- Funaki, Y.; Yamato, T. The Core of an Economy with a Common Pool Resource: A Partition Function Form Approach. Int. J. Game Theory 1999, 28, 157–171. [Google Scholar] [CrossRef]
- Barrett, S. Self-Enforcing International Environmental Agreements. Oxford Econ. Pap. 1994, 46, 804–878. [Google Scholar]
- D’Aspremont, C.; Jacquemin, A.; Gabszewicz, J.J.; Weymark, J.A. On the Stability of Collusive Price Leadership. Can. J. Econ. 1983, 16, 17–25. [Google Scholar]
- Diamantoudi, E.; Sartzetakis, E.S. Stable International Environmental Agreements: An Analytical Approach. J. Public Econ. Theory 2006, 8, 247–263. [Google Scholar] [CrossRef]
- Peleg, B.; Tijs, S. The Consistency Principle for Games in Strategic Form. Int. J. Game Theory 1996, 25, 13–34. [Google Scholar] [CrossRef]
- Jackson, M.O.; Wilkie, S. Endogenous Games and Mechanisms: Side Payments Among Players. Rev. Econ. Stud. 2005, 72, 543–566. [Google Scholar] [CrossRef]
- Kalai, A.D.; Kalai, E. Engineering Cooperation in Two-Player strategic Games. Microsoft Research and Kellogg School of Management, Northwestern University: Evanston, IL, USA, 2010; (Working Paper). [Google Scholar]
- Topkis, D.M. Equilibrium Points in Nonzero-sum Submodular Games. SIAM J. Contr. Optimizat. 1979, 17, 773–787. [Google Scholar] [CrossRef]
- Milgrom, P.; Roberts, J. Rationalizability, Learning and Equilibrium in Games with Strategic Complementarities. Econometrica 1990, 58, 1255–1277. [Google Scholar] [CrossRef]
- Vives, X. Nash Equilibrium with Strategic Complementarities. J. Math. Econ. 1990, 19, 305–329. [Google Scholar] [CrossRef]
- Zhou, L. The Set of Nash Equilibria of a Supermodular Game is a Complete Lattice. Game. Econ. Behav. 1994, 7, 295–300. [Google Scholar] [CrossRef]
- Nash, J.F. Equilibrium Points in N-person Games. Proc. Nat. Acad. Sci. 1950, 36, 48–49. [Google Scholar] [CrossRef] [PubMed]
- Myerson, R.B. Game Theory: Analysis of Conflict; Harvard University Press: Cambridge, MA, USA, 1991. [Google Scholar]
- Jackson, M.O.; Wolinsky, A. A Strategic Model of Social and Economic Networks. J. Econ. Theory 1996, 71, 44–74. [Google Scholar] [CrossRef]
- Béal, S.; Quérou, N. Bounded Rationality in Repeated Network Formation. Math. Soc. Sci. 2007, 54, 71–89. [Google Scholar] [CrossRef]
- Sundaram, R.K. A First Course in Optimization Theory; Cambridge University Press: New York, NY, USA, 1996. [Google Scholar]
- Berge, C. Topological Spaces: Including a Treatment of Multi-Valued Functions, Vector Spaces and Convexity, 1963; reprinted by Dover Publication, Inc.: Mineola, NY, USA, 1997. [Google Scholar]
- Moore, J.C. Mathematical Methods for Economic Theory 2; Springer-Verlag: Heidelberg, Germany, 1999. [Google Scholar]
Appendix
Proof of Proposition 1
Proof of Lemma 1
- 1.See also Ray and Vohra [4].
- 2.Note that .
- 3.For completeness, note that the group can simultaneously delete and add links between its members. However any such change in the network configuration cannot improve the value of the network for the group.
© 2010 by the authors; licensee MDPI, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Béal, S.; Chakrabarti, S.; Ghintran, A.; Solal, P. Partial Cooperative Equilibria: Existence and Characterization. Games 2010, 1, 338-356. https://doi.org/10.3390/g1030338
Béal S, Chakrabarti S, Ghintran A, Solal P. Partial Cooperative Equilibria: Existence and Characterization. Games. 2010; 1(3):338-356. https://doi.org/10.3390/g1030338
Chicago/Turabian StyleBéal, Sylvain, Subhadip Chakrabarti, Amandine Ghintran, and Philippe Solal. 2010. "Partial Cooperative Equilibria: Existence and Characterization" Games 1, no. 3: 338-356. https://doi.org/10.3390/g1030338



