Optimization Design of Electromagnetic Actuator Applied as Fast Tool Servo
Abstract
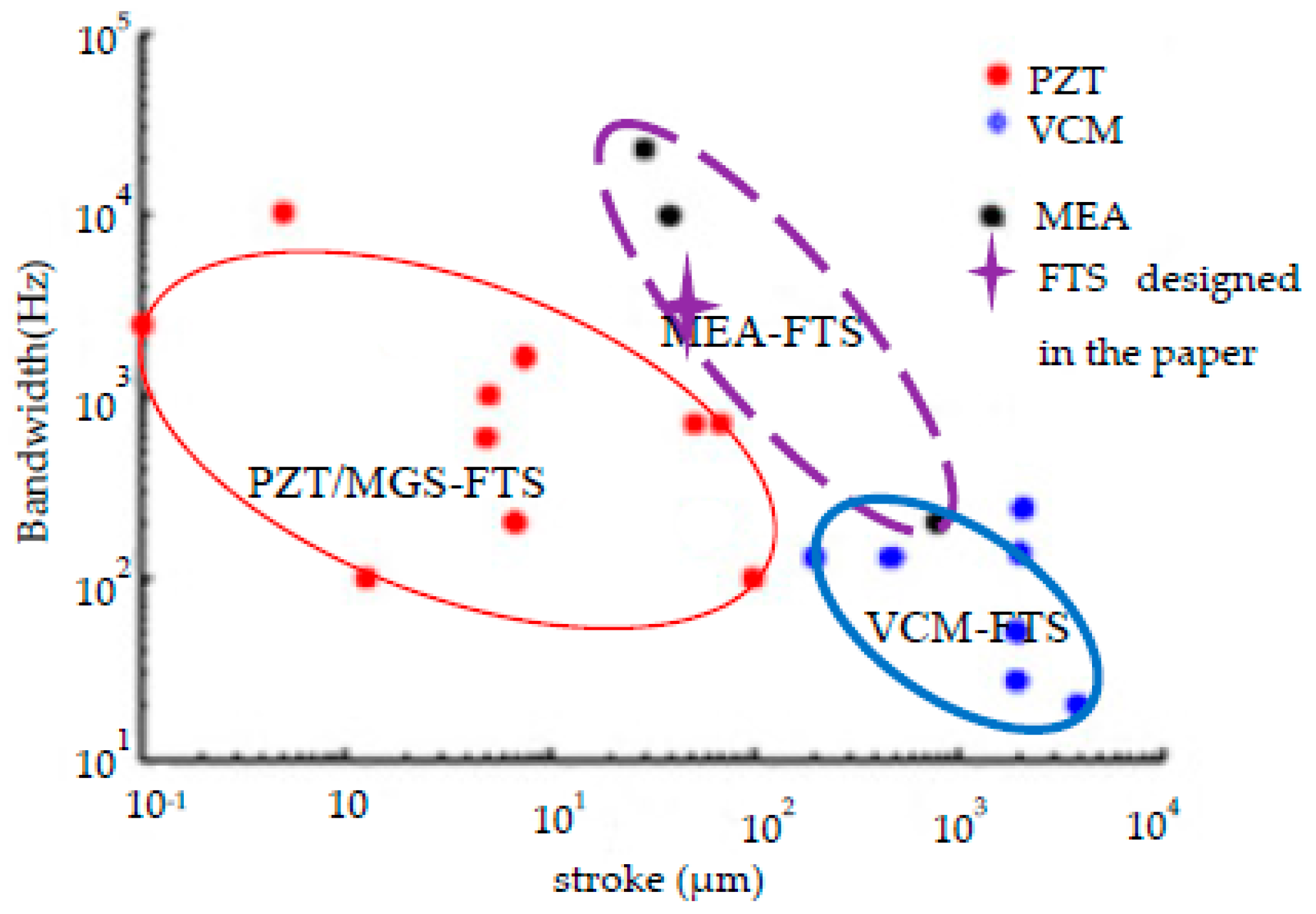
:1. Introduction
2. Materials and Methods
2.1. Construction of MEA-FTS Model
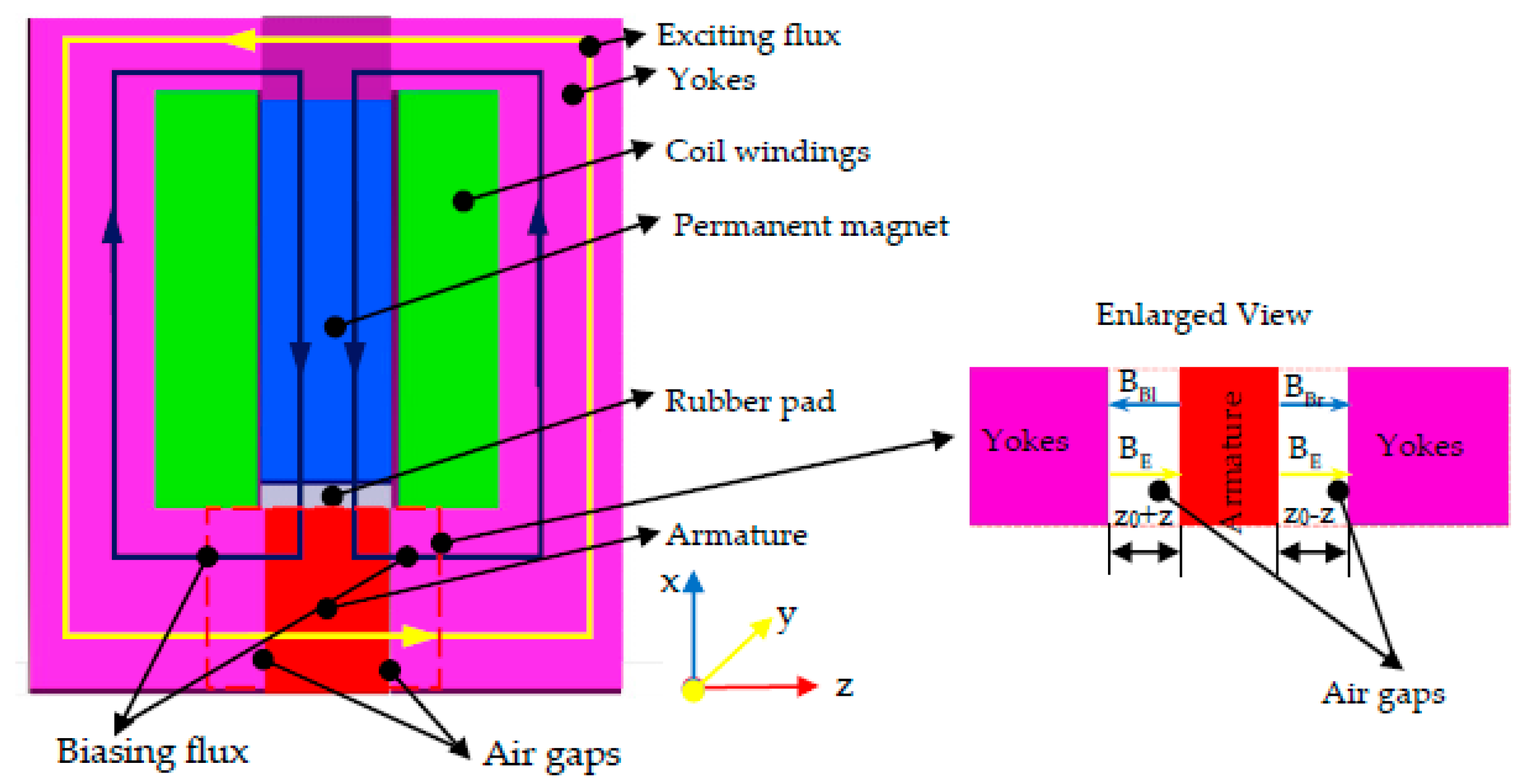
2.1.1. Electromagnetic Driving Model
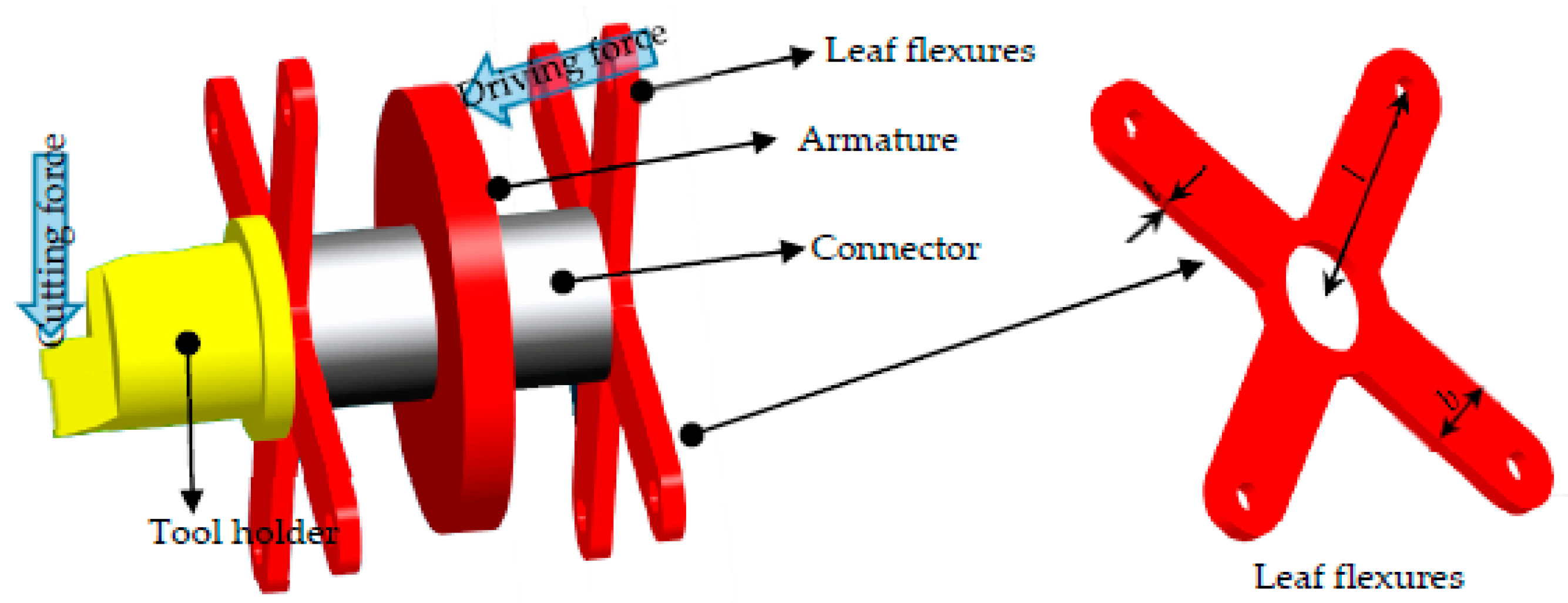
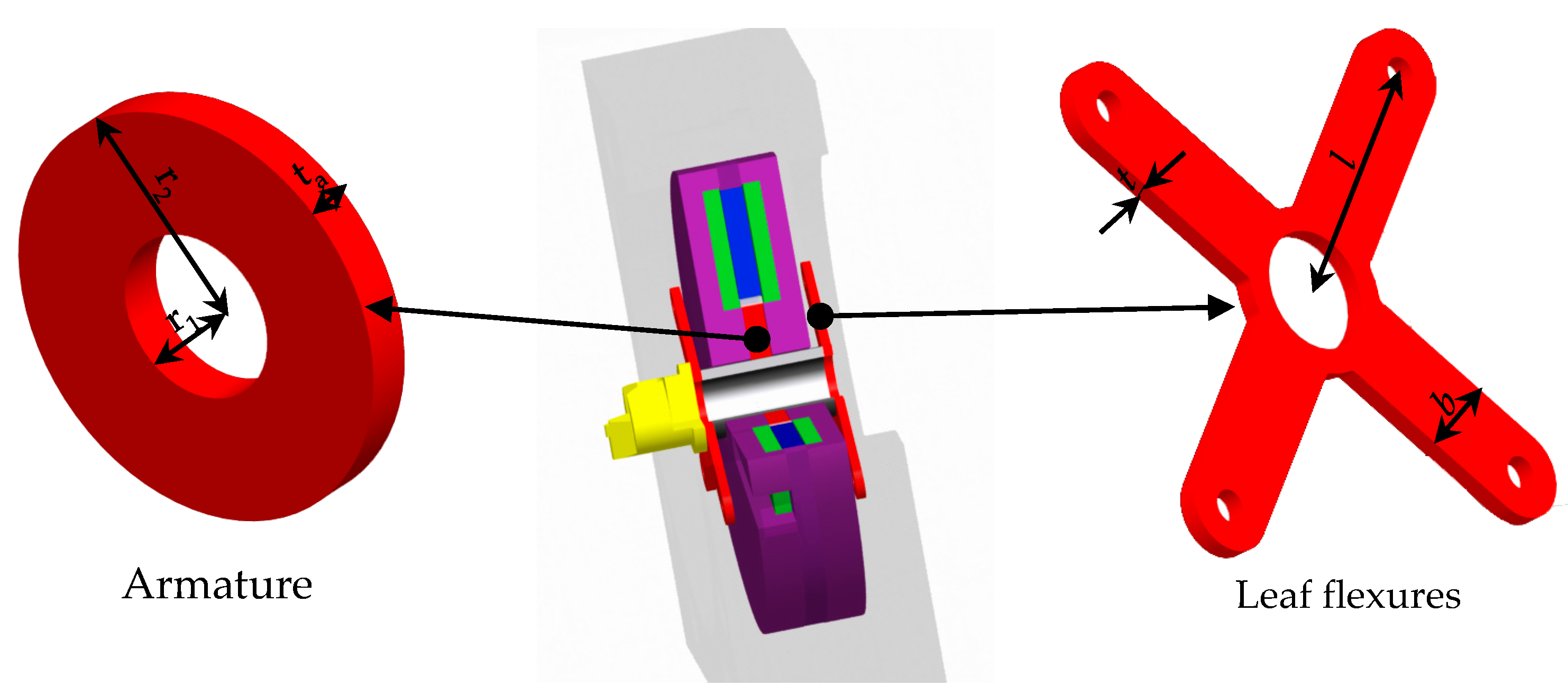
2.1.2. Mechanic Model
2.1.3. Driving Response Model
2.1.4. Construction and Implementation of Optimization Model
Objective Functions of the Problem
Constraints of the Problem
Construction of Optimization Model
2.2. Implementation of the Problem
2.2.1. Reformulation of the Problem
2.2.2. Solution of the Problem
3. Results and Discussion
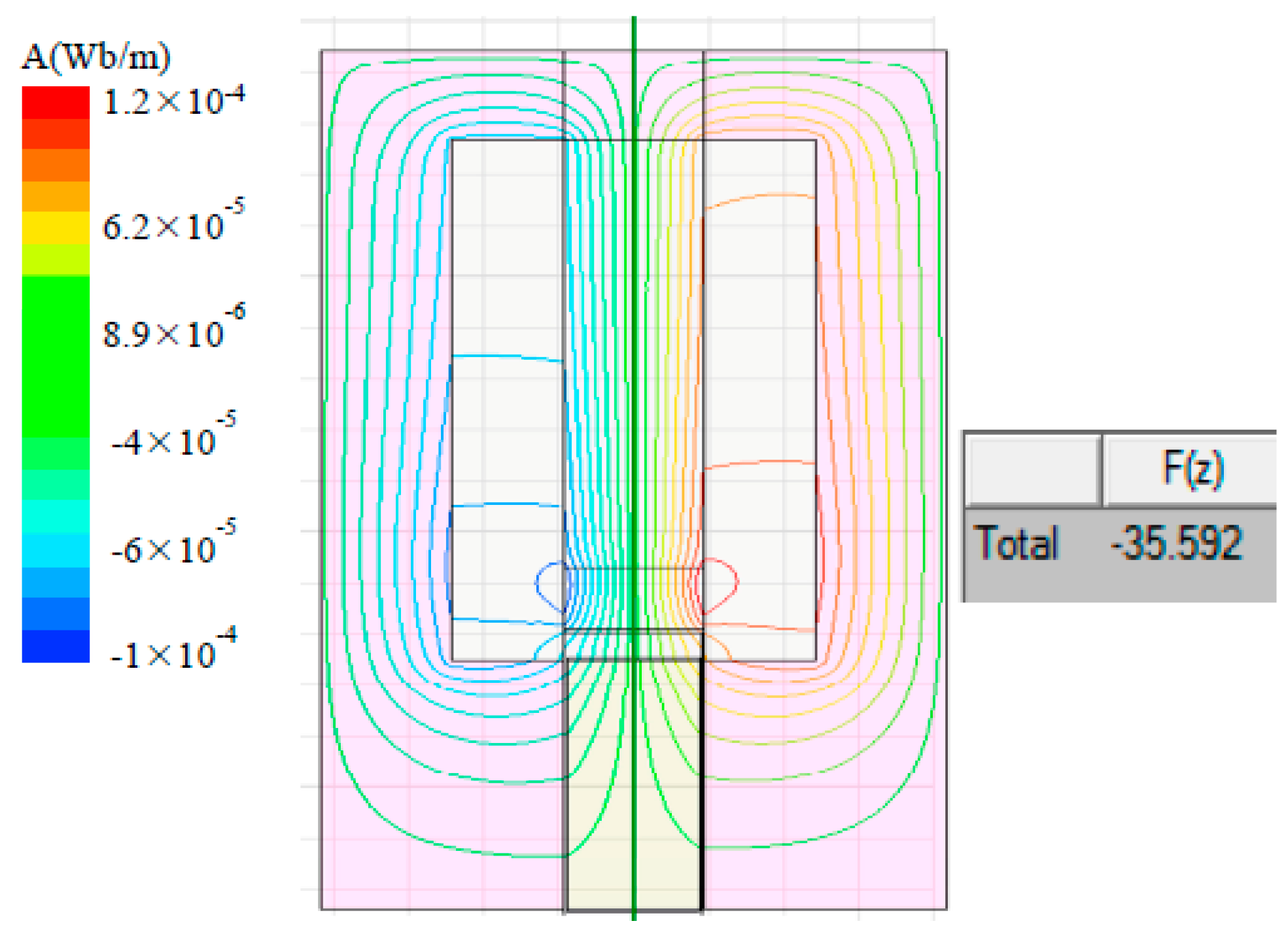
3.1. Finite Element Analysis Validation
3.1.1. Finite Element Analysis for Electromagnetic Field
3.1.2. Finite Element Analysis for Mechanical Structure
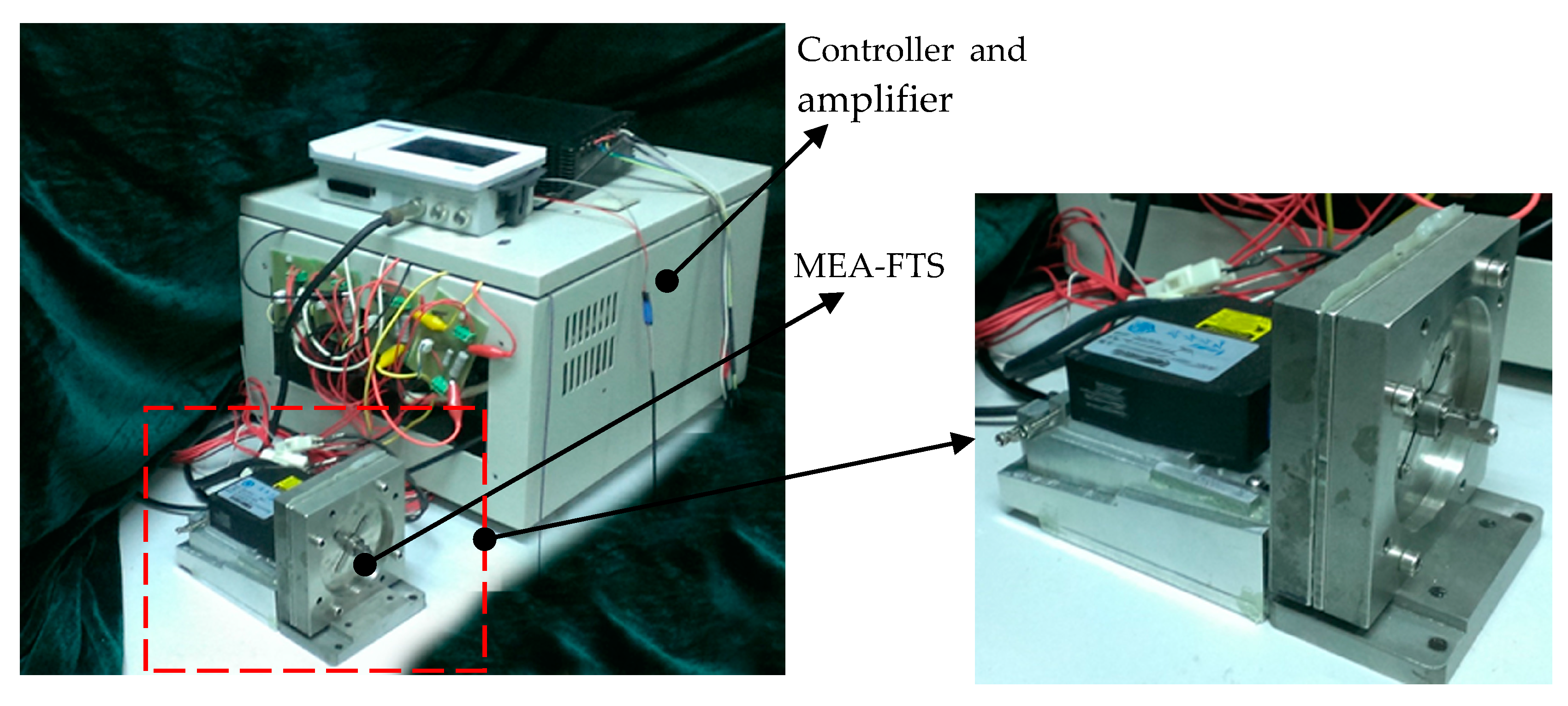
3.2. Experiment Tests
3.2.1. Experiment of Displacement
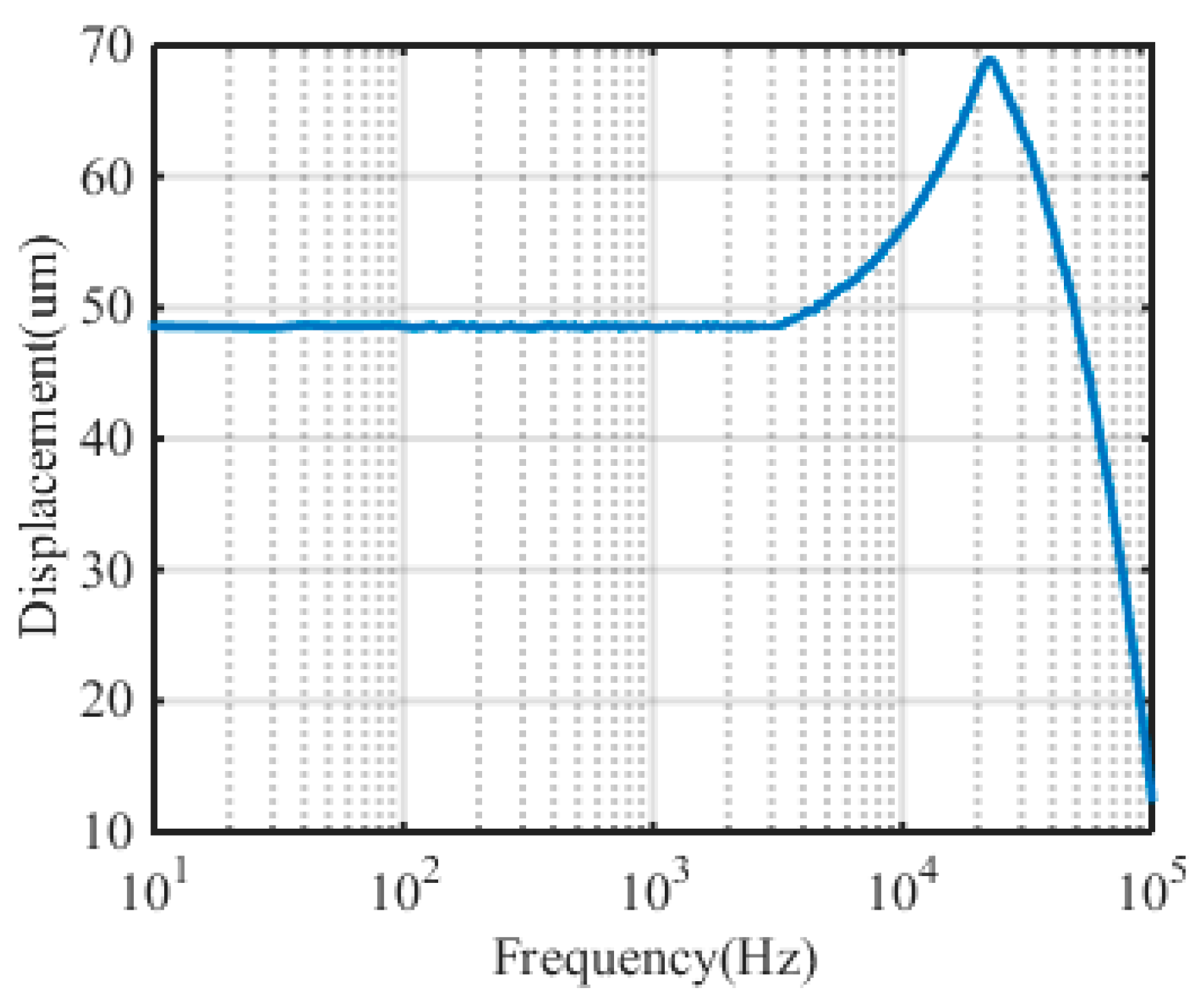
3.2.2. Experiment of Frequency Response
3.2.3. Experiment of Dynamic Response
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Chapman, G. Ultra-Precision Machining Systems; an Enabling Technology for Perfect Surfaces; Moore Nanotechnology Systems: Keene, NH, USA, 2004; Volume 1, pp. 1–9. [Google Scholar]
- Drive Technique Comparisons 2005. Available online: http://www.pecncsu.edu (accessed on 8 October 2011).
- Kirk, R.; Roblee, J. Freeform Machining with Precitech Servo Tool Options; Precitech Ultra Precision Technology: Berwyn, PA, USA, 2005; Volume 25, pp. 1–10. [Google Scholar]
- Physik Instrumente. Fast Tool Servo Precision Machining Piezo Actuator. Available online: http://www.pi-usa.us (accessed on 8 October 2013).
- Ma, H.Q.; Tian, J.; Hu, D.J. Development of a fast tool servo in noncircular turning and its control. Mech. Syst. Signal Process. 2013, 41, 705–713. [Google Scholar] [CrossRef]
- Kang, D.; Kim, K.; Kim, D.; Shin, J.; Gweon, D.-G.; Jeong, J. Optimal design of high precision XY-scanner with nanometer-level resolution and millimeter-level working range. Mechatronics 2009, 19, 562–570. [Google Scholar] [CrossRef]
- Zhou, X.; Hu, L. An improved adaptive feedforward cancellation for tool trajectory tracking in diamond turning of freeform optics. In Proceedings of the 2010 International Conference on Mechanic Automation and Control Engineering, Wuhan, China, 26–28 June 2010; pp. 3590–3593. [Google Scholar]
- Gutierrez, H.M.; Ro, P.I. Magnetic servo levitation by sliding-mode control of nonaffine systems with algebraic input invertibility. IEEE Trans. Ind. Electron. 2005, 52, 1449–1455. [Google Scholar] [CrossRef]
- Lu, X.D.; Trumper, D.L. Ultrafast tool servos for diamond turning. CIRP Ann. Manuf. Technol. 2005, 54, 383–388. [Google Scholar] [CrossRef]
- Zhu, Z.W.; Zhou, X.Q.; Liu, Z.W.; Wang, R.Q.; Zhu, L. Development of a piezoelectrically actuated two-degree-of-freedom fast tool servo with decoupled motions for micro-/nanomachining. Precis. Eng. 2014, 38, 809–820. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, S.; Huo, D.; Cheng, K. Performance analysis and optimal design of fast tool servo used for machining microstructured surfaces. Process. Inst. Mech. Eng. Part C 2008, 222, 1541–1546. [Google Scholar] [CrossRef]
- Zhu, Z.W.; Zhou, X.Q.; Liu, Q.; Zhao, S.X. Multi-objective optimum design of fast tool servo based on improved differential evolution algorithm. J. Mech. Sci. Technol. 2011, 25, 3141–3149. [Google Scholar] [CrossRef]
- Zhuang, C.; Xu, M.; Xiong, Z. Multi-objective topology optimization of compliant mechanism for fast tool servo. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Wollongong, Australia, 9–12 July 2013. [Google Scholar]
- Rakuff, S.; Cuttino, J.F. Design and testing of a long-range, precision fast tool servo system for diamond turning. Precis. Eng. 2009, 33, 18–25. [Google Scholar] [CrossRef]
- Lu, X. Electromagnetically-Driven Ultra-Fast Tool Servos for Diamond Turning. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2005. [Google Scholar]
- Wu, D.; Xie, X.D.; Zhou, S.Y. Design of a normal stress electromagnetic fast linear actuator. IEEE Trans. Magn. 2010, 46, 1007–1014. [Google Scholar]
- Fang, F.Z.; Nie, Y.H.; Zhang, X.D. Design of fast tool servo system based on magnetic field analysis. Nanotechnol. Precis. Eng. 2011, 9, 539–544. [Google Scholar]
- Lee, W. Prediction of microcutting force variation in ultra-precision machining. Precis. Eng. 1990, 12, 25–28. [Google Scholar] [CrossRef]
- Smith, S.T. Flexures: Elements of Elastic Mechanisms; Gordon and Breach Science Publishers: Perth, Australia, 2000. [Google Scholar]
- Nie, Y.H.; Fang, F.Z.; Zhang, X.D. System design of Maxwell force driving fast tool servos based on model analysis. Int. J. Adv. Manuf. Technol. 2014, 72, 25–32. [Google Scholar] [CrossRef]
- Franklin, G.F.; David, J.P.; Abbas, E.-N. Feedback Control of Dynamics Systems; Pretince Hall Inc.: Upper Saddle River, NJ, USA, 2006. [Google Scholar]
- Schittkowski, K.; Zillober, C.; Zotemantel, R. Numerical comparison of nonlinear programming algorithms for structural optimization. Struct. Multidiscip. Optim. 1994, 7, 1–19. [Google Scholar] [CrossRef]
- Grace, A.C.W. Computer-Aided Control System Design Using Optimization Techniques. Ph.D. Thesis, University of Wales, Bangor, Gwynedd, UK, 1989. [Google Scholar]
- Francisco, F.; Fischer, A.; Kanzow, C. On the accurate identification of active constraints. SIAM J. Optim. 1998, 9, 14–32. [Google Scholar]
- Fletcher, R.; Leyffer, S.; Ralph, D.; Scholtes, S. Local convergence of SQP methods for mathematical programs with equilibrium constraints. SIAM J. Optim. 2006, 17, 259–286. [Google Scholar] [CrossRef]
- Luo, Z. The Study of Sequential Quadratic Programming (SQP) Algorithms. Ph.D. Thesis, Guilin University of Electronic Technology, Guilin, China, 2008. [Google Scholar]
- Hu, Q. Research on Sequential Quadratics Programming Method for Solving Constrained Optimization. Ph.D. Thesis, Hunan University, Hunan, China, 2008. [Google Scholar]





| f* (Hz) | δ* (μm) | f (Hz) | δ (μm) | t (mm) | b (mm) | l (mm) | r1 (mm) | r2 (mm) |
|---|---|---|---|---|---|---|---|---|
| target bandwidth | target stroke | optimized bandwidth | optimized stroke | optimized thickness of flexure | optimized width of flexure | optimized length of flexure | optimized outer radius of armature | optimized inner radius of armature |
| 500 | 250 | 786.9 | 127.6 | 1.4 | 9.8 | 17.8 | 3.5 | 14.9 |
| 1000 | 100 | 999.4 | 99.4 | 1.1 | 4.5 | 10 | 3.8 | 15 |
| 2000 | 50 | 1.969 k | 49.2 | 1.4 | 5.4 | 10 | 5 | 15 |
| 3000 | 30 | 3.057 k | 30.57 | 1.5 | 6.1 | 10 | 5 | 15 |
| 3500 | 25 | 3.584 k | 25.6 | 1.6 | 6.4 | 10 | 5 | 15 |
| 5000 | 18 | 4.925 k | 17.73 | 1.7 | 7 | 10 | 5 | 15 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nie, Y.; Du, Y.; Xu, Z. Optimization Design of Electromagnetic Actuator Applied as Fast Tool Servo. Actuators 2017, 6, 25. https://doi.org/10.3390/act6030025
Nie Y, Du Y, Xu Z. Optimization Design of Electromagnetic Actuator Applied as Fast Tool Servo. Actuators. 2017; 6(3):25. https://doi.org/10.3390/act6030025
Chicago/Turabian StyleNie, Yahui, Yinfei Du, and Zhuo Xu. 2017. "Optimization Design of Electromagnetic Actuator Applied as Fast Tool Servo" Actuators 6, no. 3: 25. https://doi.org/10.3390/act6030025




