Natural Convection Flow of Fractional Nanofluids Over an Isothermal Vertical Plate with Thermal Radiation
Abstract
:1. Introduction
2. Statement of the Problem
3. Solution of the Problem
3.1. Determination of the Temperature Field
3.2. Calculation of the Velocity Field
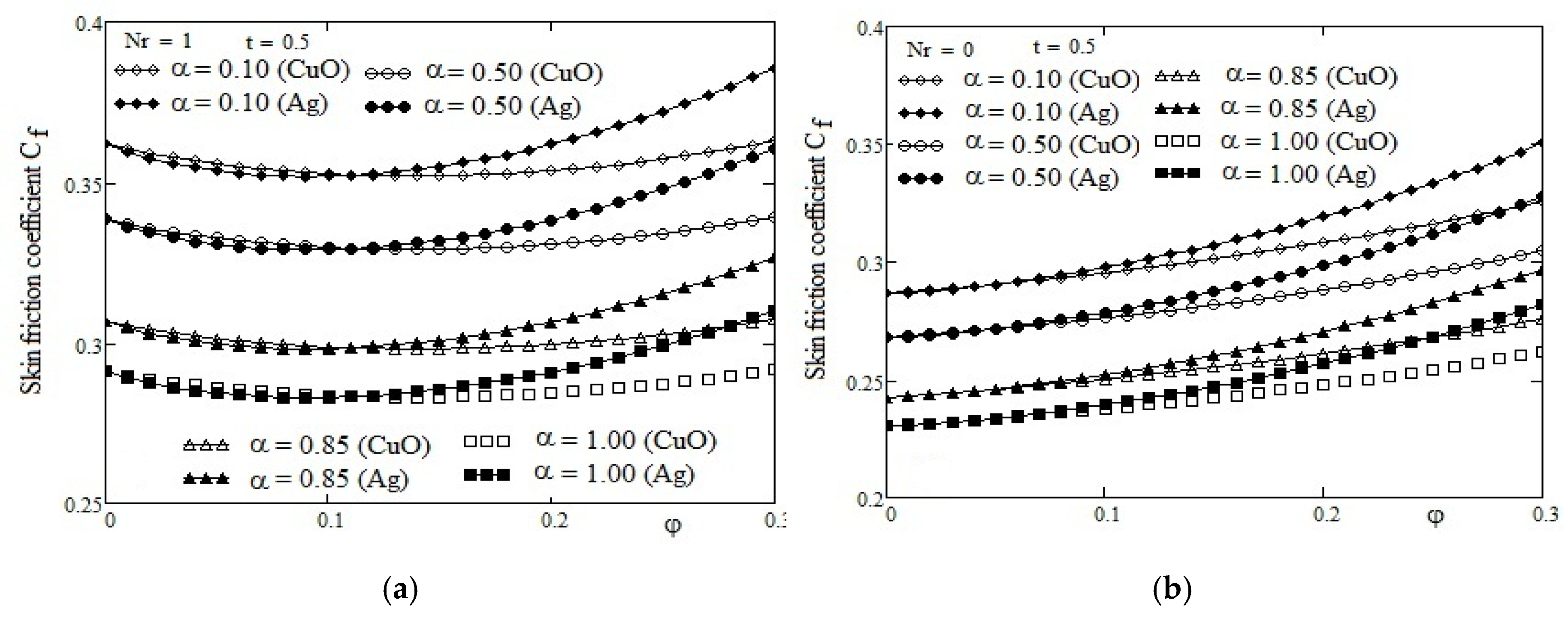
3.3. Nusselt Number and Skin Friction
4. Validation
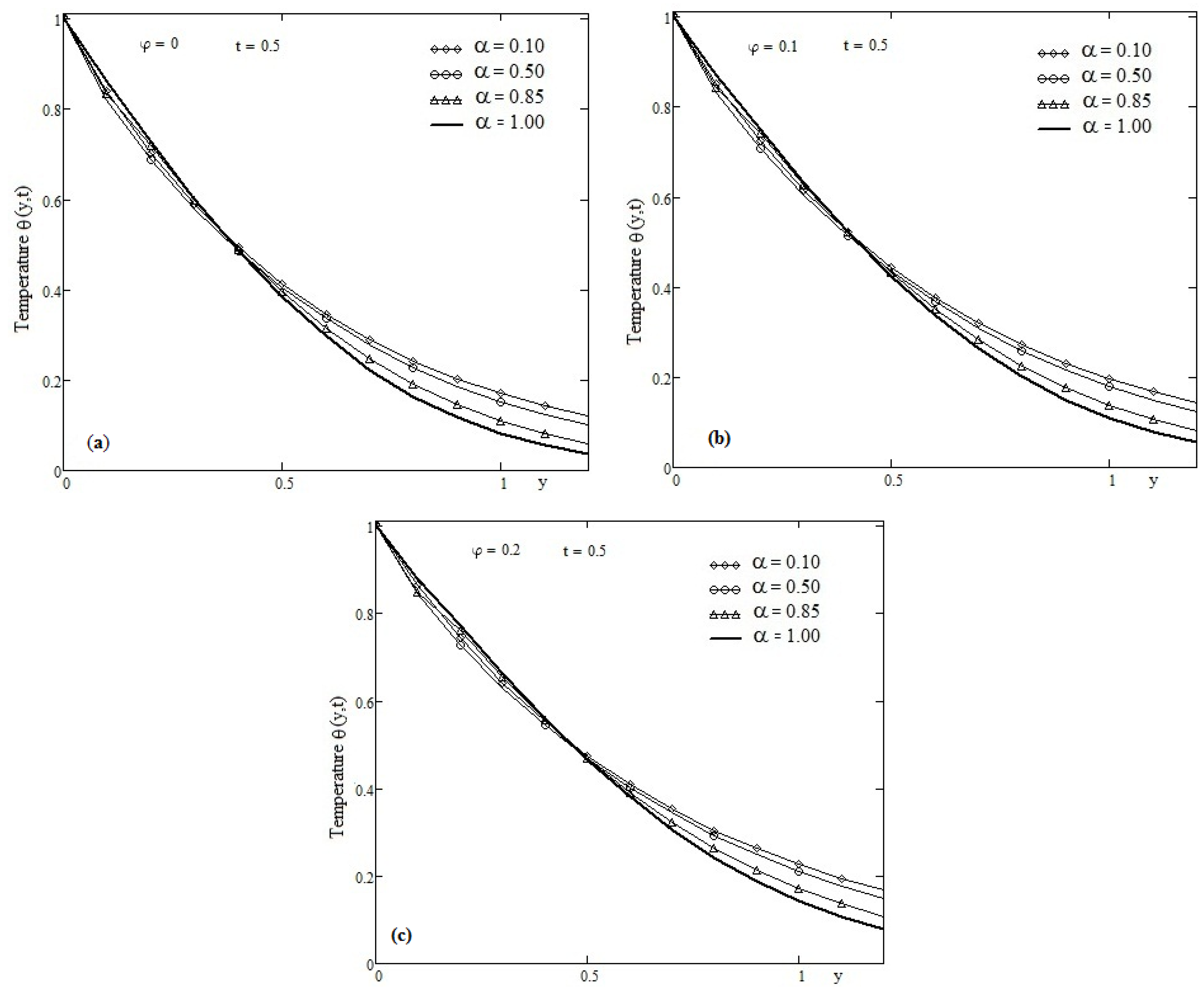
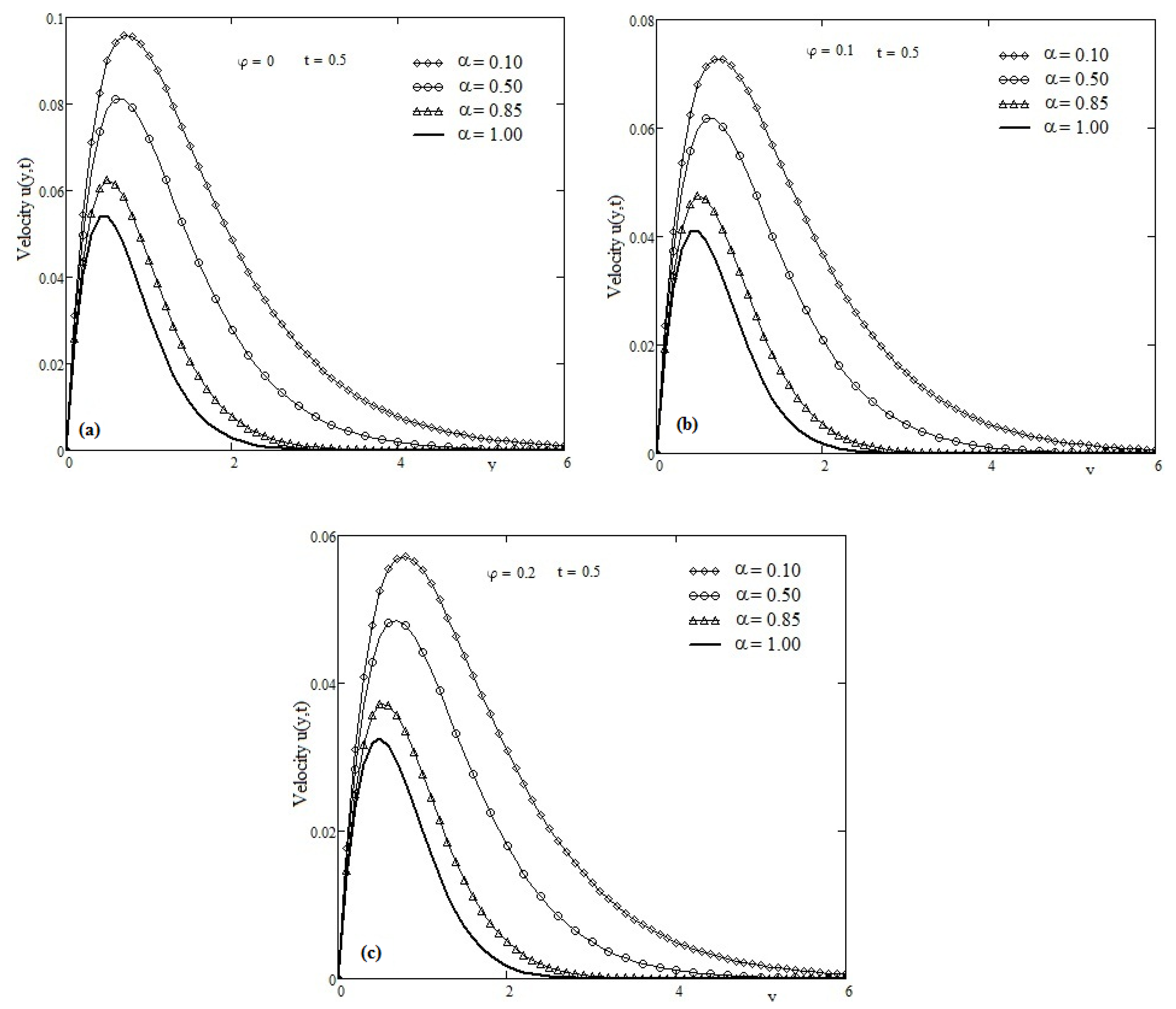
5. Numerical Results and Discussion
6. Conclusions
Acknowledgments
Conflicts of Interest
Appendix A
References
- Raptis, A. Unsteady free convection flow through a porous medium. Int. J. Eng. Sci. 1983, 21, 345–348. [Google Scholar] [CrossRef]
- Kim, S.J.; Vafai, K. Analysis of natural convection about a vertical plate embedded in a porous medium. Int. J. Heat Mass Transfer 1989, 32, 665–677. [Google Scholar] [CrossRef]
- Narasimha, K.R.; Pop, I. Transient free convection in a fluid saturated porous media with temperature dependent viscosity. Int. Commun. Heat Mass Transf. 1994, 21, 573–581. [Google Scholar]
- Ghosh, S.K.; Beg, O.A. Theoretical analysis of radiative effects on transient free convection heat transfer past a hot vertical surface in porous media. Nonlinear Anal. Model. Control 2008, 13, 419–432. [Google Scholar]
- Mondal, S.; Haroun, N.A.H.; Sibanda, P. The effects of thermal radiation on an unsteady MHD axisymmetric stagnation-point flow over a shrinking sheet in presence of temperature dependent thermal conductivity with Navier slip. PLoS ONE 2015, 10, e0138355. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Zheng, L.; Zhang, X.; Chen, G. MHD flow and radiation heat transfer of nanofluids in porous media with variable surface heat flux and chemical reaction. Appl. Math. Model. 2015, 39, 165–181. [Google Scholar] [CrossRef]
- Abd Elazem, N.Y.; Ebaid, A.; Aly, H.E. Radiation effect of MHD on Cu-water and Ag-water nanofluids flow over a stretching sheet: Numerical study. J. Appl. Computat. Math. 2015, 4. [Google Scholar] [CrossRef]
- Bianco, V.; Manca, O.; Nardini, S.; Vafai, K. Heat Transfer Enhancement with Nanofluids; CRC Press: London, UK, 2015. [Google Scholar]
- Choi, S.U.S. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the ASME International Mechanical Engineering Congress and Exposition 66, San Francisco, CA, USA, 12–17 November 1995; pp. 99–105.
- Khan, W.A.; Aziz, A. Natural convection flow of a nanofluid over a vertical plate with uniform surface heat flux. Int. J. Therm. Sci. 2011, 50, 1207–1214. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Exact analytical solutions for heat and mass transfer of MHD slip flow in nanofluids. Chem. Eng. Sci. 2012, 84, 182–187. [Google Scholar] [CrossRef]
- Bachok, N.; Ishak, A.; Pop, I. Flow and heat transfer characteristics on a moving plate in a nanofluid. Int. J. Heat Mass Transf. 2012, 55, 642–648. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M.; Pop, I. Heat and mass transfer of unsteady natural convection flow of some nanofluids past a vertical infinite flat plate with radiation effect. Int. J. Heat Mass Transf. 2013, 59, 167–171. [Google Scholar] [CrossRef]
- Mohankrishna, P.; Sugunamma, V.; Sandeep, N. Radiation and magnetic field effects on unsteady natural convection flow of a nanofluid past an infinite vertical plate with heat source. Chem. Process. Eng. Res. 2014, 25, 39–52. [Google Scholar]
- Ellahi, R. The effects of MHD and temperature dependent viscosity on the flow of non-Newtonian nanofluid in a pipe: Analytical solutions. Appl. Math. Model. 2013, 37, 1451–1457. [Google Scholar] [CrossRef]
- Rizwan, U.H.; Khan, Z.H.; Hussain, S.T.; Hammouch, Z. Flow and heat transfer analysis of water and ethylene glycol based Cu nanoparticles between two parallel disks with suction/injection effects. J. Mol. Liq. 2016, 221, 298–304. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Zaigham, Q.M.; Ellahi, R. Influence of induced magnetic field on free convection of nanofluid considering Koo-Kleinstreuer-Li (KKL) correlation. Appl. Sci. 2016, 324, 1–13. [Google Scholar] [CrossRef]
- Sekrani, G.; Poncet, S. Further investigation on laminar forced convection of nanofluid flows in a uniformly heated pipe using direct numerical simulations. Appl. Sci. 2016, 332, 1–24. [Google Scholar] [CrossRef]
- Rizwan, U.H.; Prabhakar, B.; Bandari, S. Impact of inclined Lorentz forces on Tangent hyperbolic nanofluid flow with zero normal flux of nanoparticles at the stretching sheet. Neural Comput. Appl. 2016. [Google Scholar] [CrossRef]
- Ibrahim, W.; Ul Haq, R. Magnetohydrodynamic (MHD) stagnation point flow of nanofluid past a stretching sheet with convective boundary condition. J. Braz. Soc. Mech. Sci. Eng. 2016, 38, 1155–1164. [Google Scholar] [CrossRef]
- Waqar, A.; Khan, C.R.; Rizwan, U.H. Heat Transfer Analysis of MHD Water Functionalized Carbon Nanotube Flow over a Static/Moving Wedge. J. Nanomater. 2015, 16, 934367. [Google Scholar] [CrossRef]
- Khan, W.A.; Khan, Z.H.; Haq, R.U. Flow and heat transfer of ferrofluids over a flat plate with uniform heat flux. Eur. Phys. J. Plus 2015, 130. [Google Scholar] [CrossRef]
- Rizwan, U.H.; Rajotia, D.; Noor, N.F.M. Thermophysical effects of water driven copper nanoparticles on MHD axisymmetric permeable shrinking sheet: Dual-nature study. Eur. Phys. J. 2016, 39. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ellahi, R. Electrohydrodynamic nanofluid hydrothermal treatment in an enclosure with sinusoidal upper wall. Appl. Sci. 2015, 5, 294–306. [Google Scholar] [CrossRef]
- Rahman, S.U.; Ellahi, R.; Nadeem, S.; Zia, Q.M. Simultaneous effects of nanoparticles and slip on Jeffrey fluid through tapered artery with mild stenosis. J. Mol. Liq. 2016, 218, 484–493. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ellahi, R. Three dimensional mesoscopic simulation of magnetic field effect on natural convection of nanofluid. Int. J. Heat Mass Transf. 2015, 89, 799–808. [Google Scholar] [CrossRef]
- Akbar, N.S.; Raza, M.; Ellahi, R. Copper oxide nanoparticles analysis with water as base fluid for peristaltic flow in permeable tube with heat transfer. Comput. Meth. Prog. Biomed. 2016, 130, 22–30. [Google Scholar] [CrossRef] [PubMed]
- Oztop, H.F.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer argument in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Kakac, S.; Pramuanjaroenkij, A. Review of convective heat transfer enhancement with nanofluids. Int. J. Heat Mass Transf. 2009, 52, 3187–3196. [Google Scholar] [CrossRef]
- Povstenko, Y. Linear Fractional Diffusion-Wave Equation for Scientists and Engineers; Springer: Basel, Switzerland, 2015. [Google Scholar]
- Stankovic, B. On the function of E. M. Wright. Publ. I’Inst. Math. 1970, 10, 113–124. [Google Scholar]
- Cao, Z.; Zhao, J.; Wang, Z.; Liu, F.; Zheng, L. MHD flow and heat transfer of fractional Maxwell viscoelastic nanofluid over a moving plate. J. Mol. Liq. 2016, 222, 1121–1127. [Google Scholar] [CrossRef]
- Pan, M.; Zheng, L.; Liu, F.; Zhang, X. Modeling heat transport in nanofluids with stagnation point flow using fractional calculus. Appl. Math. Model. 2016, 40, 8974–8984. [Google Scholar] [CrossRef]

| Basic Fluid/Nanoparticles | (Kg/m3) | Cp(J/Kg K) | k (W/m·K) | β × 105 (1/K) |
|---|---|---|---|---|
| Pure water | 997.1 | 4179 | 0.613 | 21 |
| Copper oxide (CuO) | 6320 | 531.8 | 76.5 | 1.80 |
| Silver (Ag) | 10,500 | 235 | 429 | 1.89 |
| CuO | Ag | |||||||
|---|---|---|---|---|---|---|---|---|
| = 0.1 | = 0.5 | = 0.85 | = 1 | = 0.1 | = 0.5 | = 0.85 | = 1 | |
| 0 | 1 | 1 | 1 | 1 | 1 | 1 | 1 | 1 |
| 0.1 | 0.86341 | 0.84348 | 0.84816 | 0.87689 | 0.86675 | 0.84694 | 0.85013 | 0.87864 |
| 0.2 | 0.74363 | 0.72536 | 0.75925 | 0.77086 | 0.74937 | 0.73083 | 0.76448 | 0.7768 |
| 0.3 | 0.64057 | 0.62803 | 0.65475 | 0.6609 | 0.648 | 0.63504 | 0.66273 | 0.6691 |
| 0.4 | 0.55187 | 0.54341 | 0.55664 | 0.55868 | 0.56041 | 0.55166 | 0.56628 | 0.56886 |
| 0.5 | 0.47551 | 0.46851 | 0.46887 | 0.46476 | 0.48472 | 0.47766 | 0.47961 | 0.47635 |
| 0.6 | 0.40976 | 0.40218 | 0.39111 | 0.38035 | 0.4193 | 0.4119 | 0.4025 | 0.39275 |
| 0.7 | 0.35313 | 0.34379 | 0.32302 | 0.30608 | 0.36274 | 0.35376 | 0.33461 | 0.31872 |
| 0.8 | 0.30436 | 0.29277 | 0.26415 | 0.24213 | 0.31383 | 0.30272 | 0.27556 | 0.25448 |
| 0.9 | 0.26234 | 0.2485 | 0.21388 | 0.18821 | 0.27154 | 0.25821 | 0.22481 | 0.19985 |
| 1 | 0.22613 | 0.2103 | 0.17148 | 0.14371 | 0.23496 | 0.2196 | 0.1817 | 0.15432 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fetecau, C.; Vieru, D.; Azhar, W.A. Natural Convection Flow of Fractional Nanofluids Over an Isothermal Vertical Plate with Thermal Radiation. Appl. Sci. 2017, 7, 247. https://doi.org/10.3390/app7030247
Fetecau C, Vieru D, Azhar WA. Natural Convection Flow of Fractional Nanofluids Over an Isothermal Vertical Plate with Thermal Radiation. Applied Sciences. 2017; 7(3):247. https://doi.org/10.3390/app7030247
Chicago/Turabian StyleFetecau, Constantin, Dumitru Vieru, and Waqas Ali Azhar. 2017. "Natural Convection Flow of Fractional Nanofluids Over an Isothermal Vertical Plate with Thermal Radiation" Applied Sciences 7, no. 3: 247. https://doi.org/10.3390/app7030247





