Application of the Wave Propagation Approach to Sandwich Structures: Vibro-Acoustic Properties of Aluminum Honeycomb Materials
Abstract
:Featured Application
Abstract
1. Introduction
2. Theory
2.1. Sound Propagation through a Partition
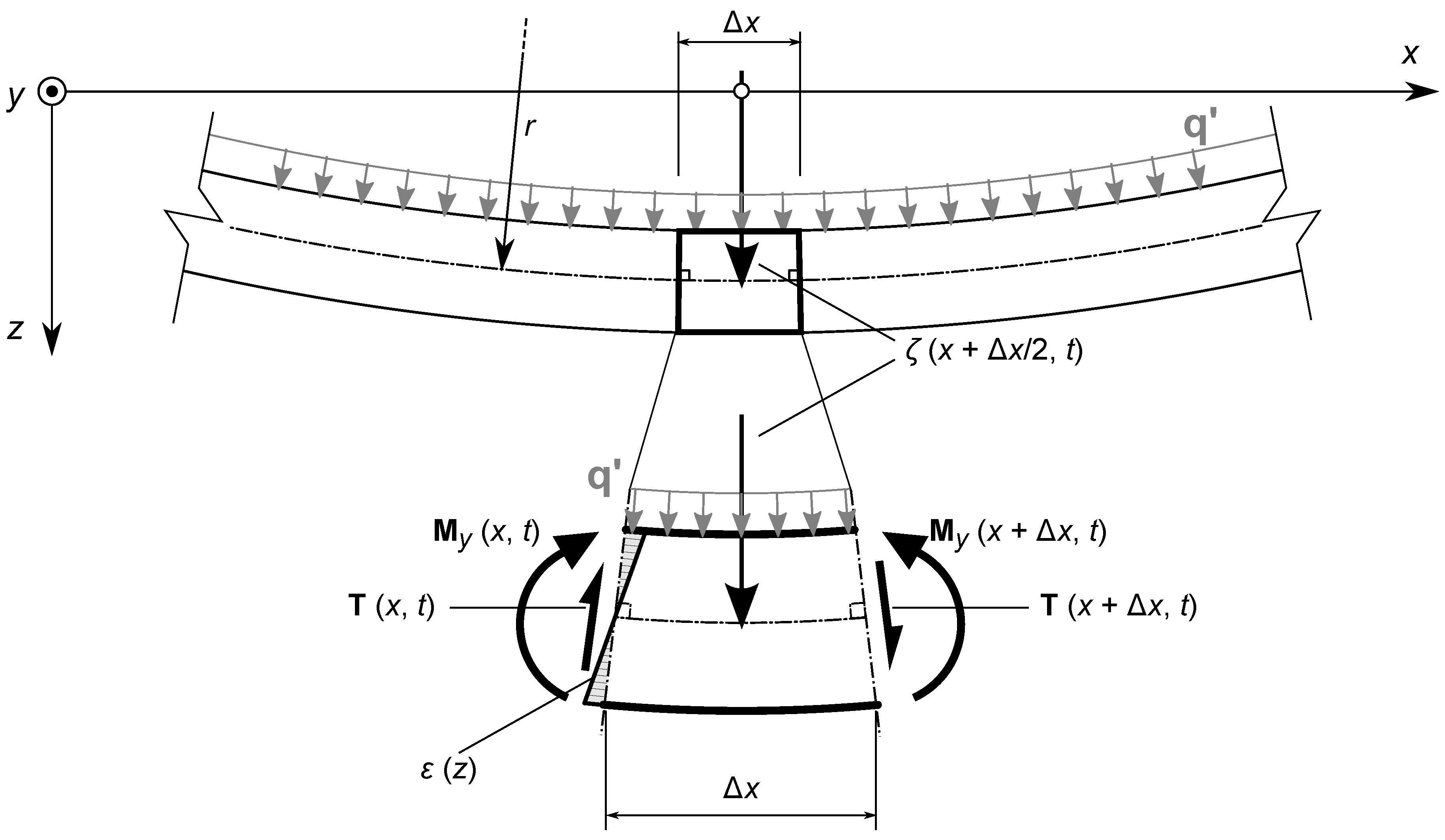
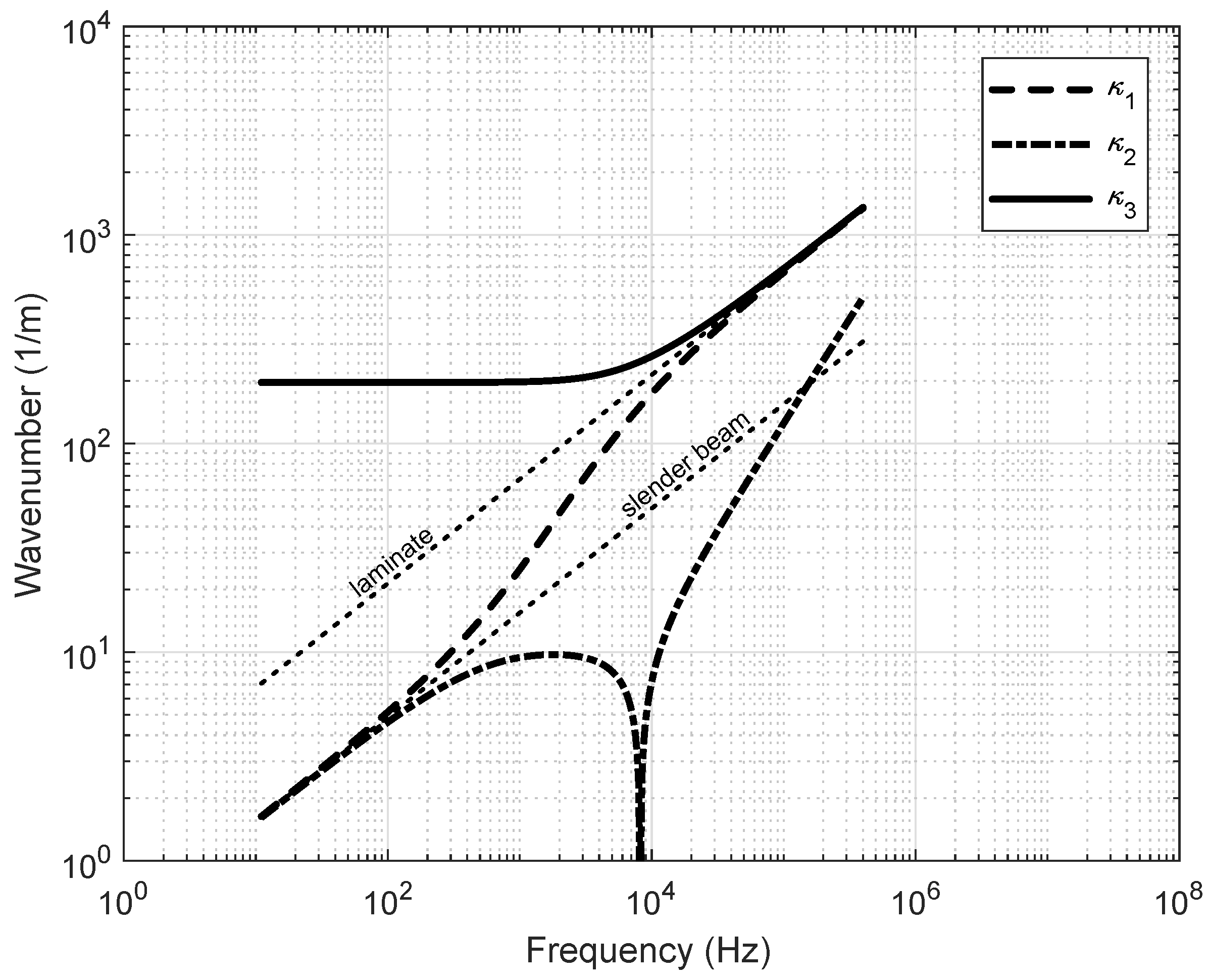
2.2. Propagation of Bending Waves
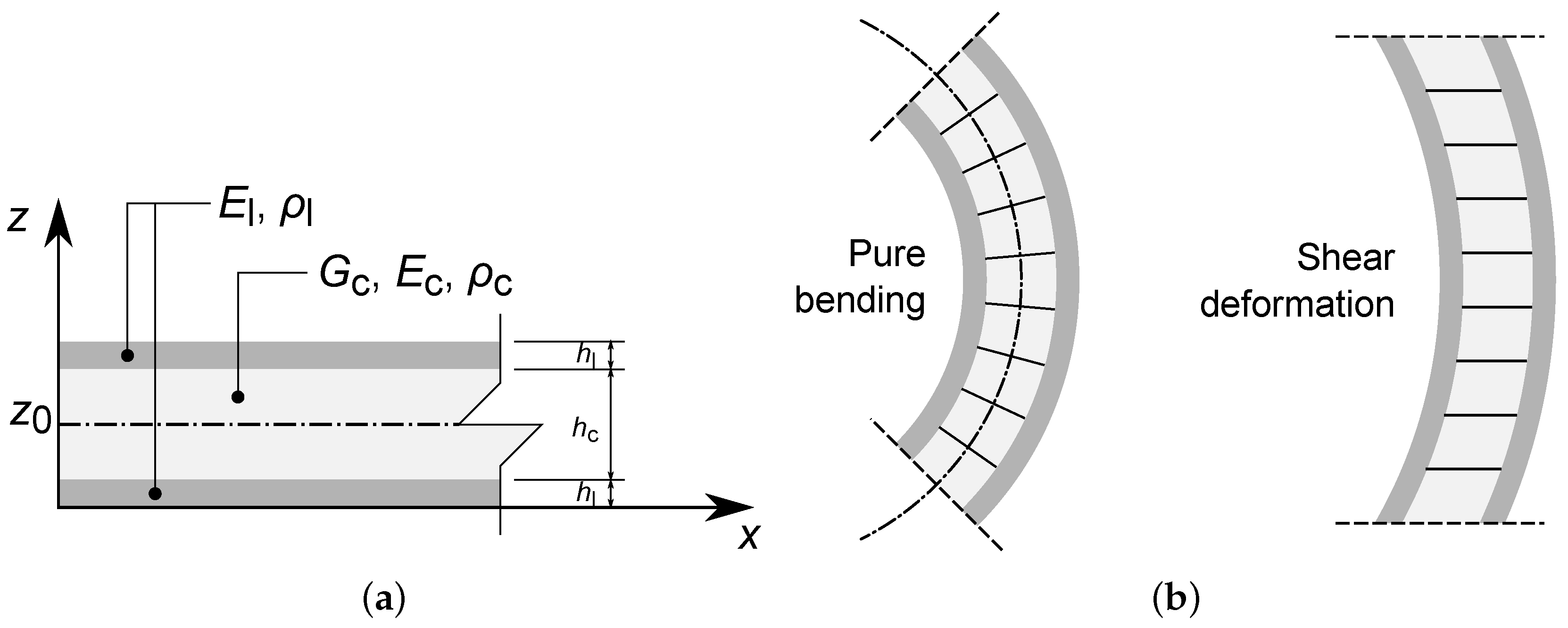
2.3. From Homogeneous to Sandwich Partitions
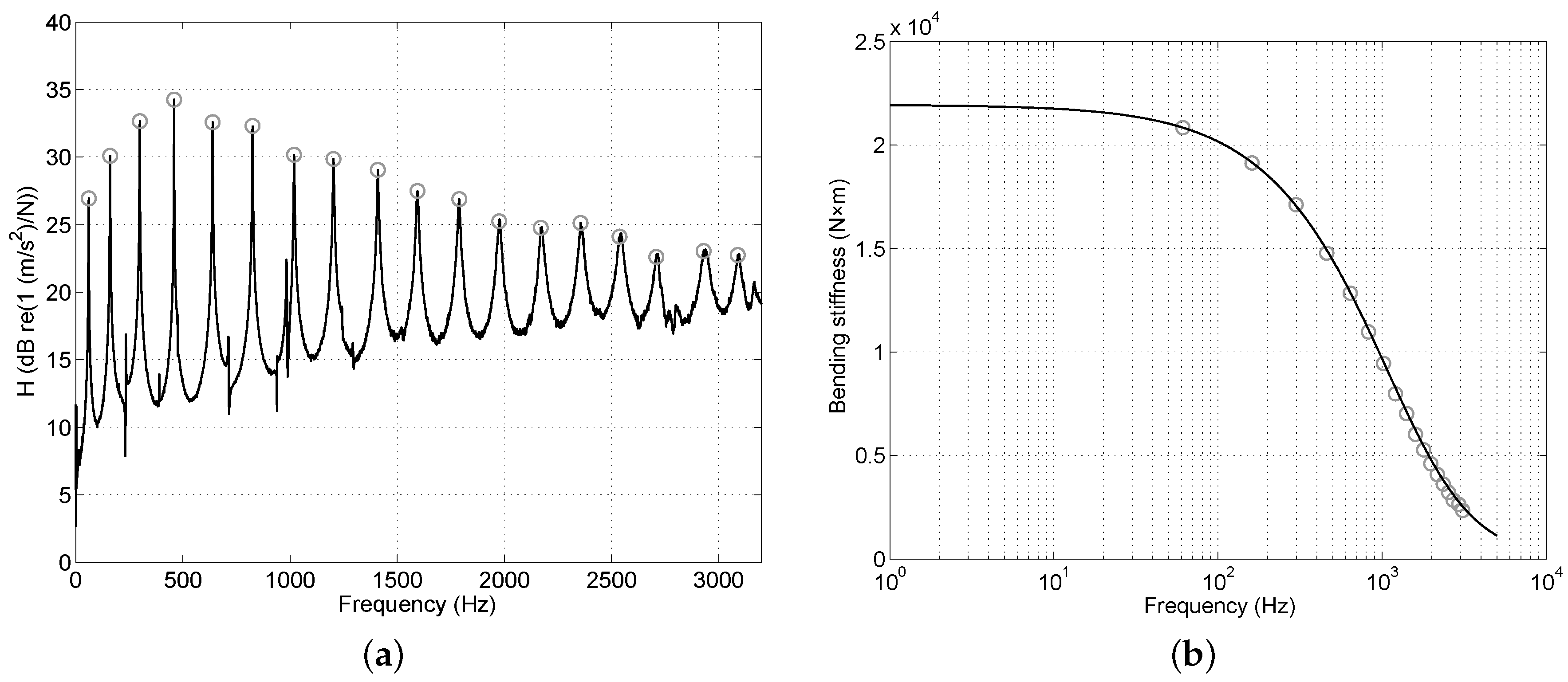
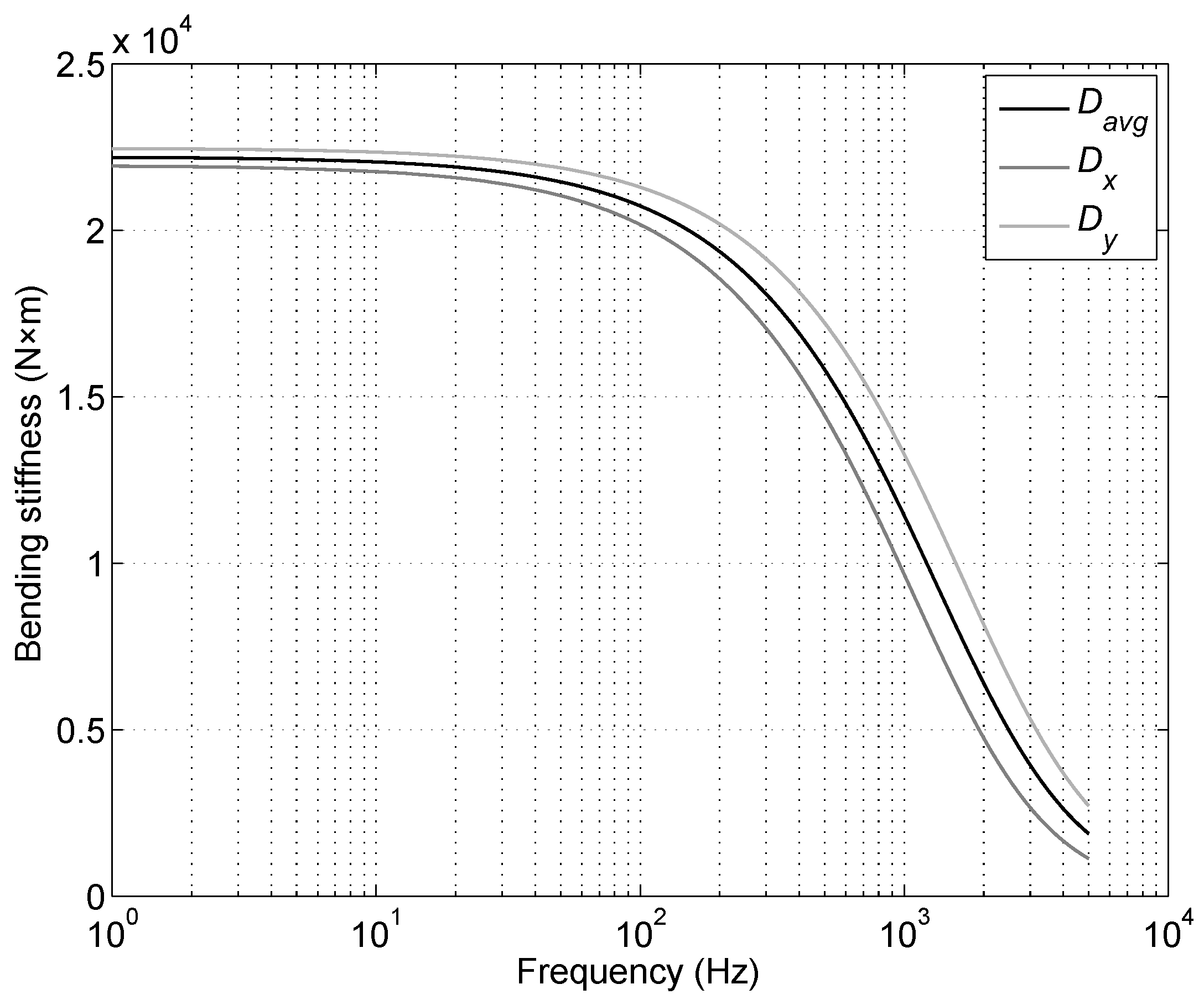
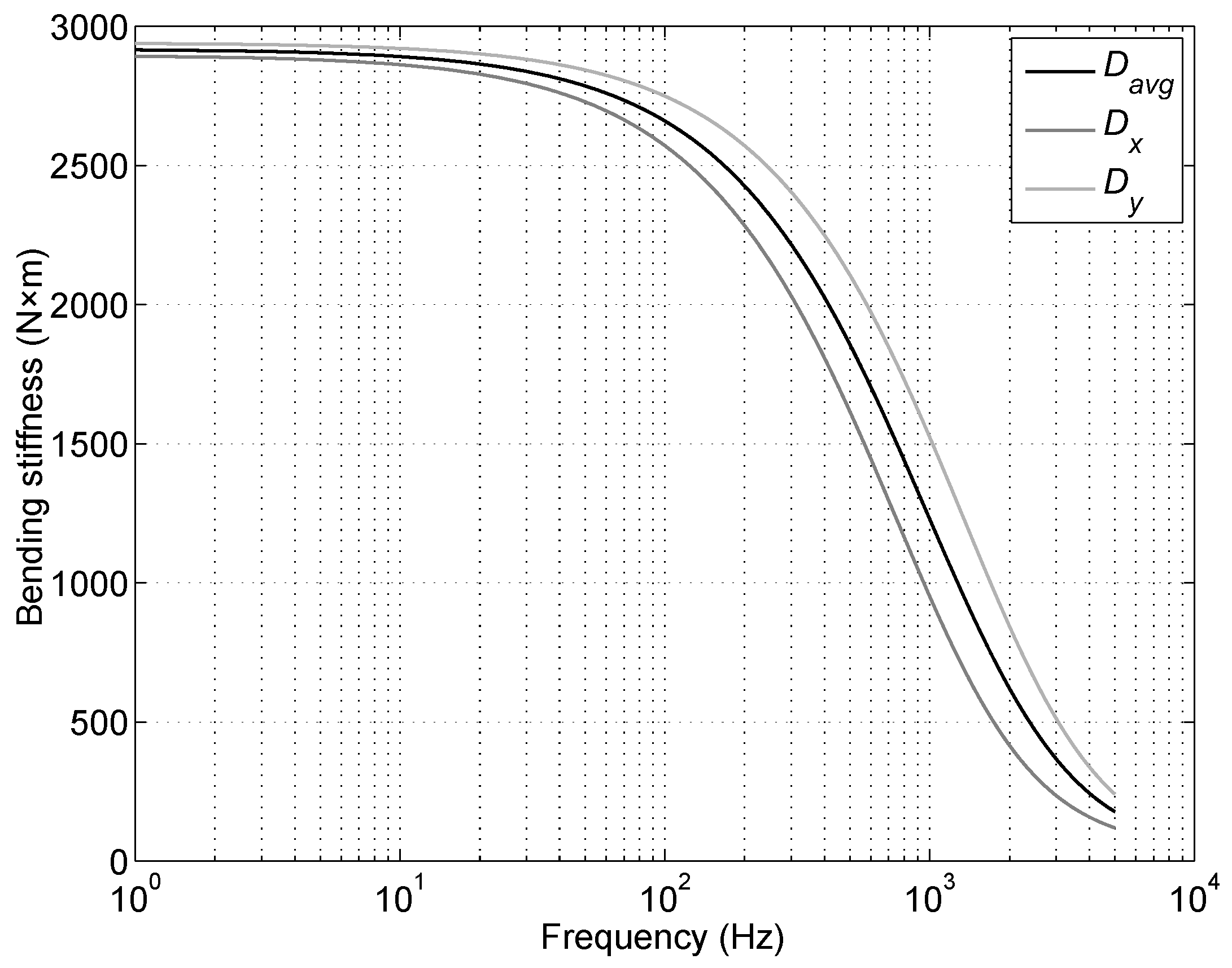
2.4. Determination of the Apparent Bending Stiffness
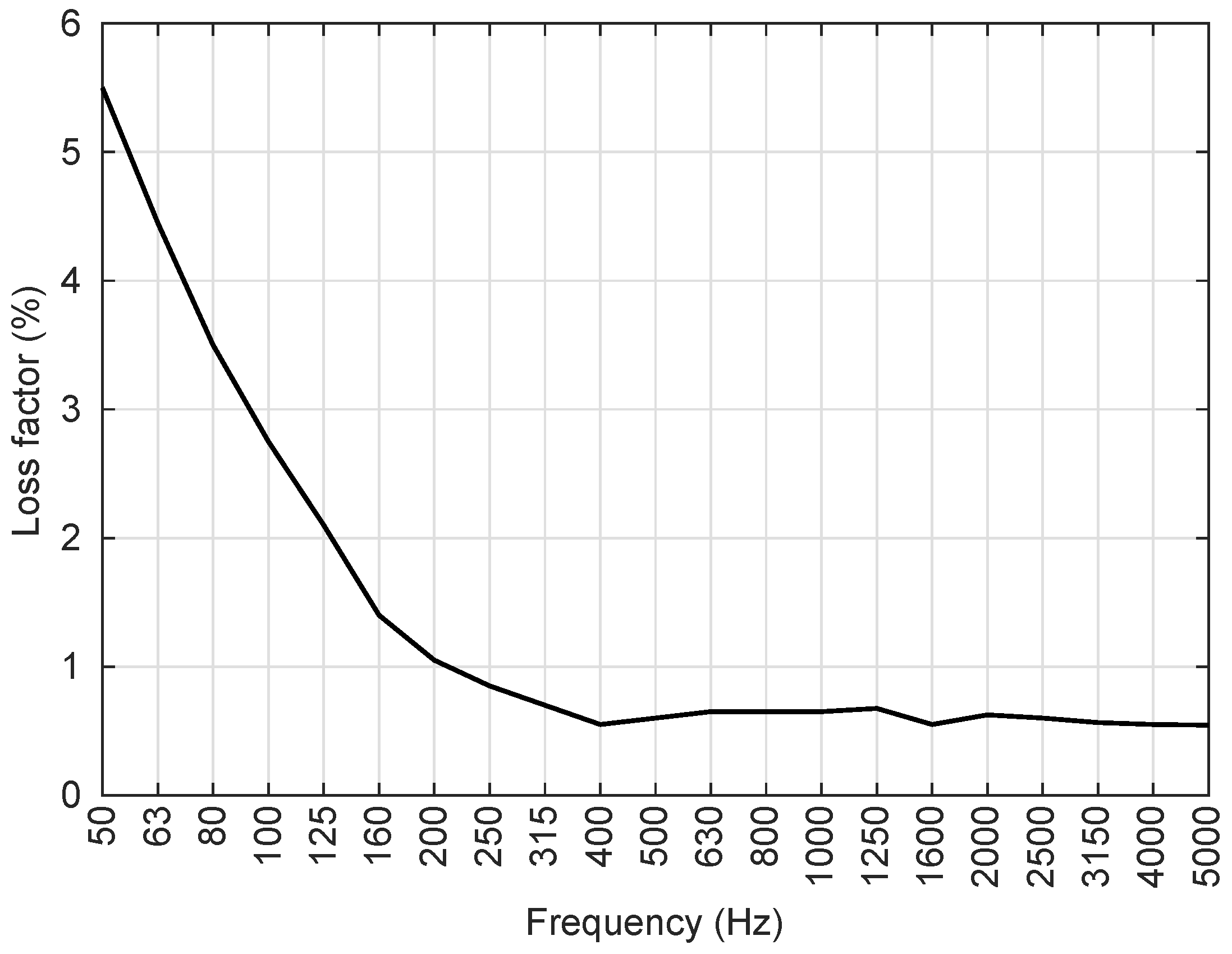
2.5. Losses in Solid Structures
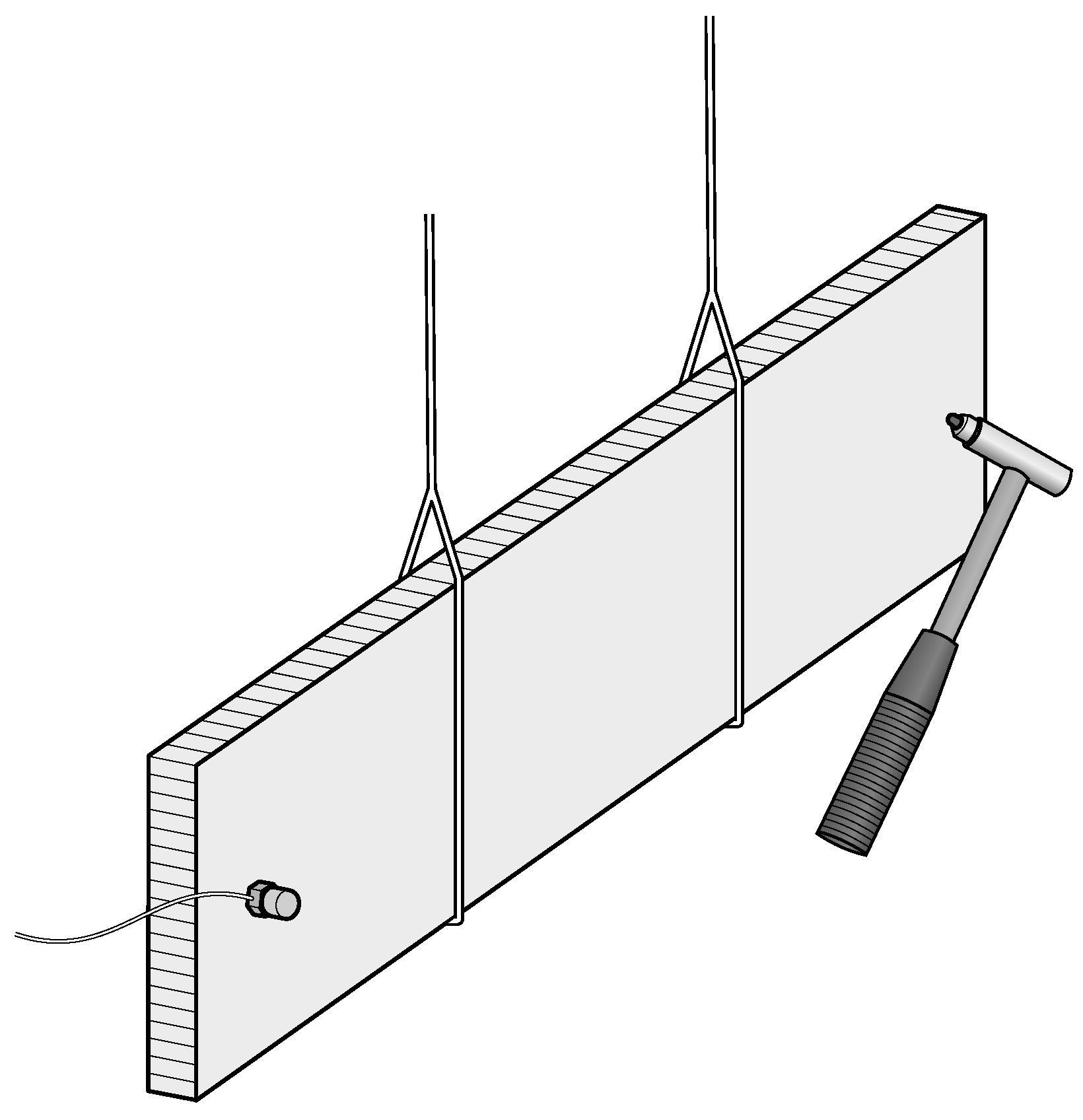
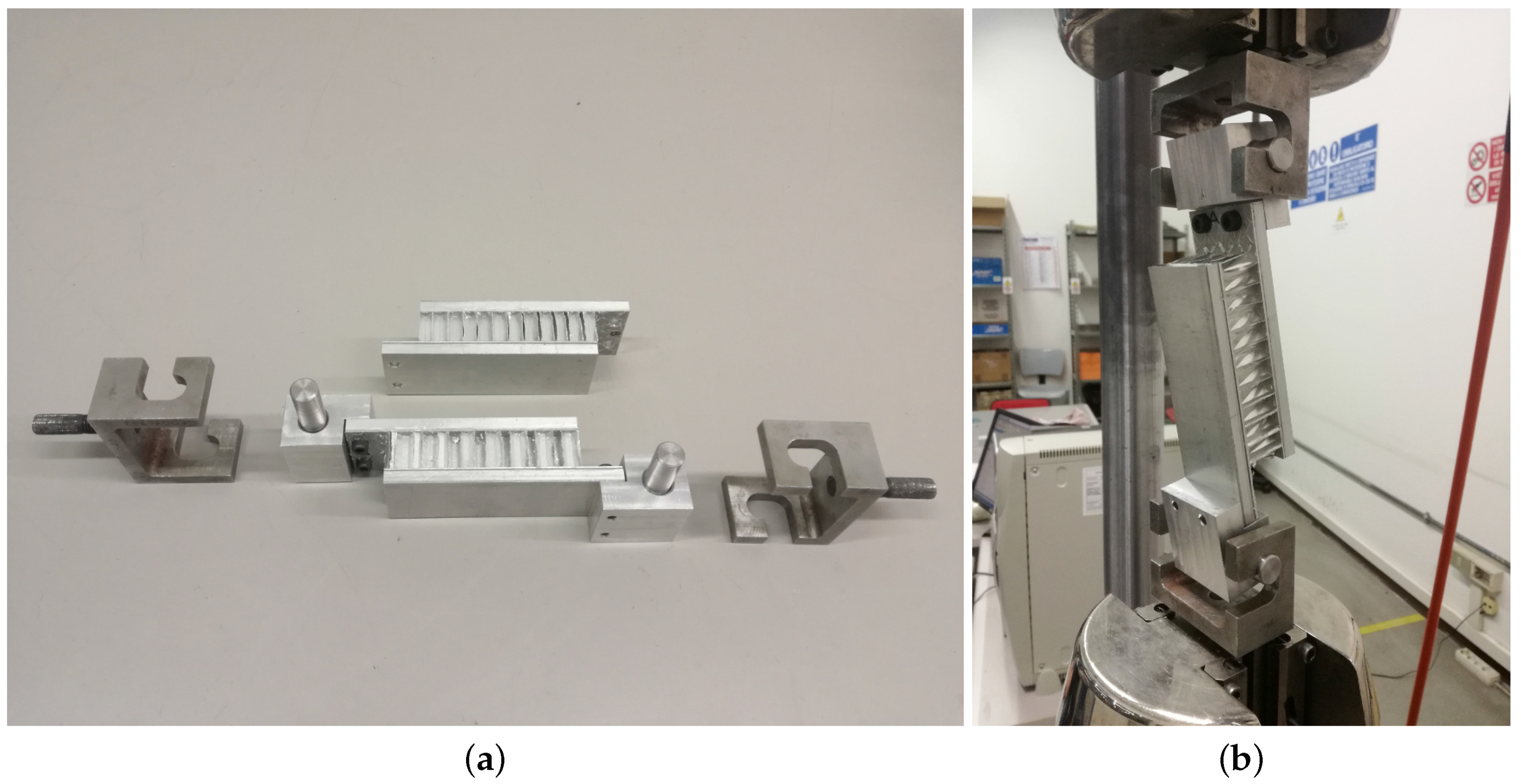
3. Materials and Methods
4. Results and Discussion
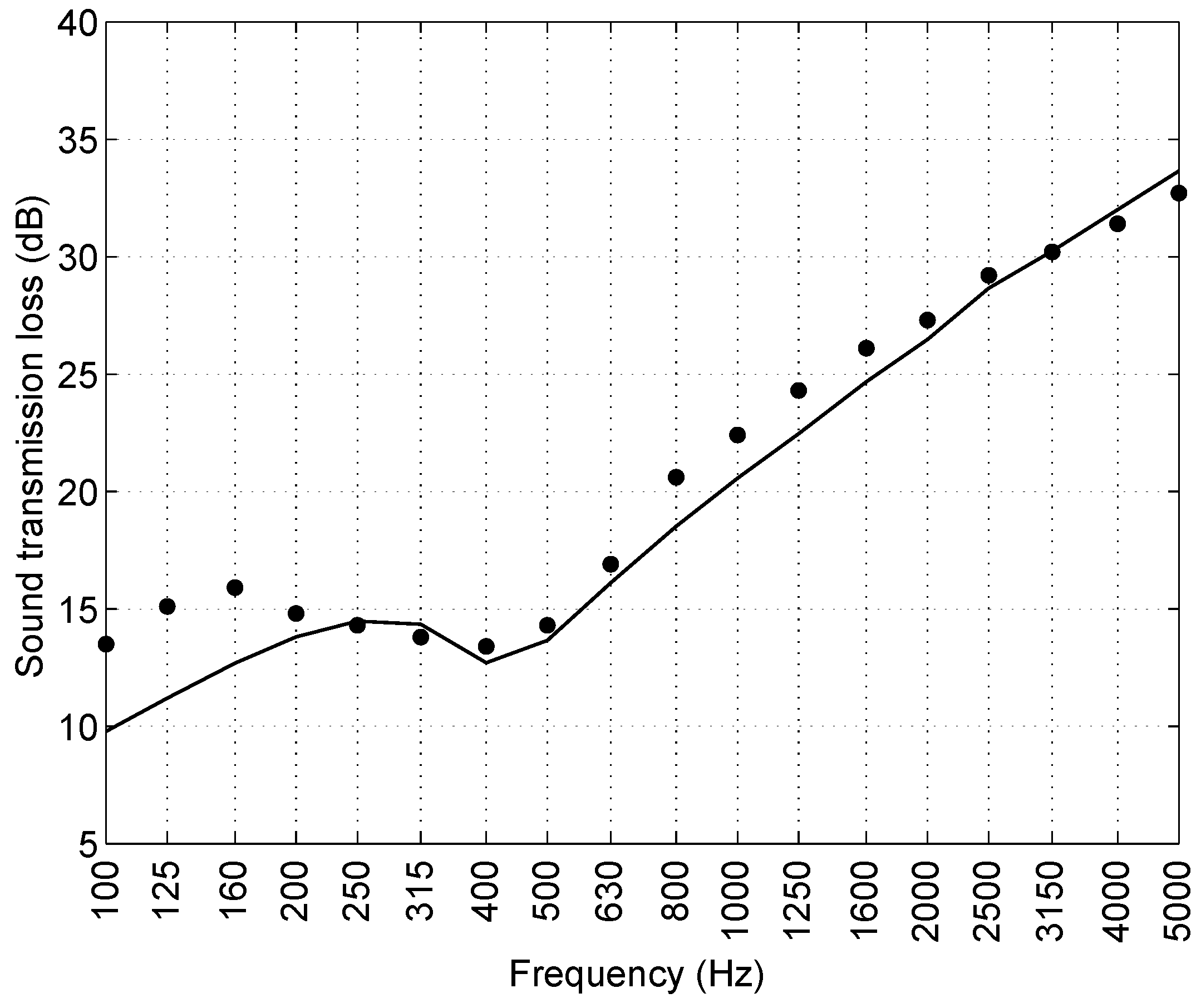
4.1. Sound Transmission Loss
4.2. Shear Modulus
- a different sample aspect ratio (5:1) with respect to the standard prescriptions (12:1), an inevitable choice given the characteristics of the test bench;
- the presence of sandwich laminates and adhesive layers, which could not be removed without damaging the core;
- the use of aluminum clamps and plates, whereas the standard requires steel components, because of the technological limits of the available machining equipment.
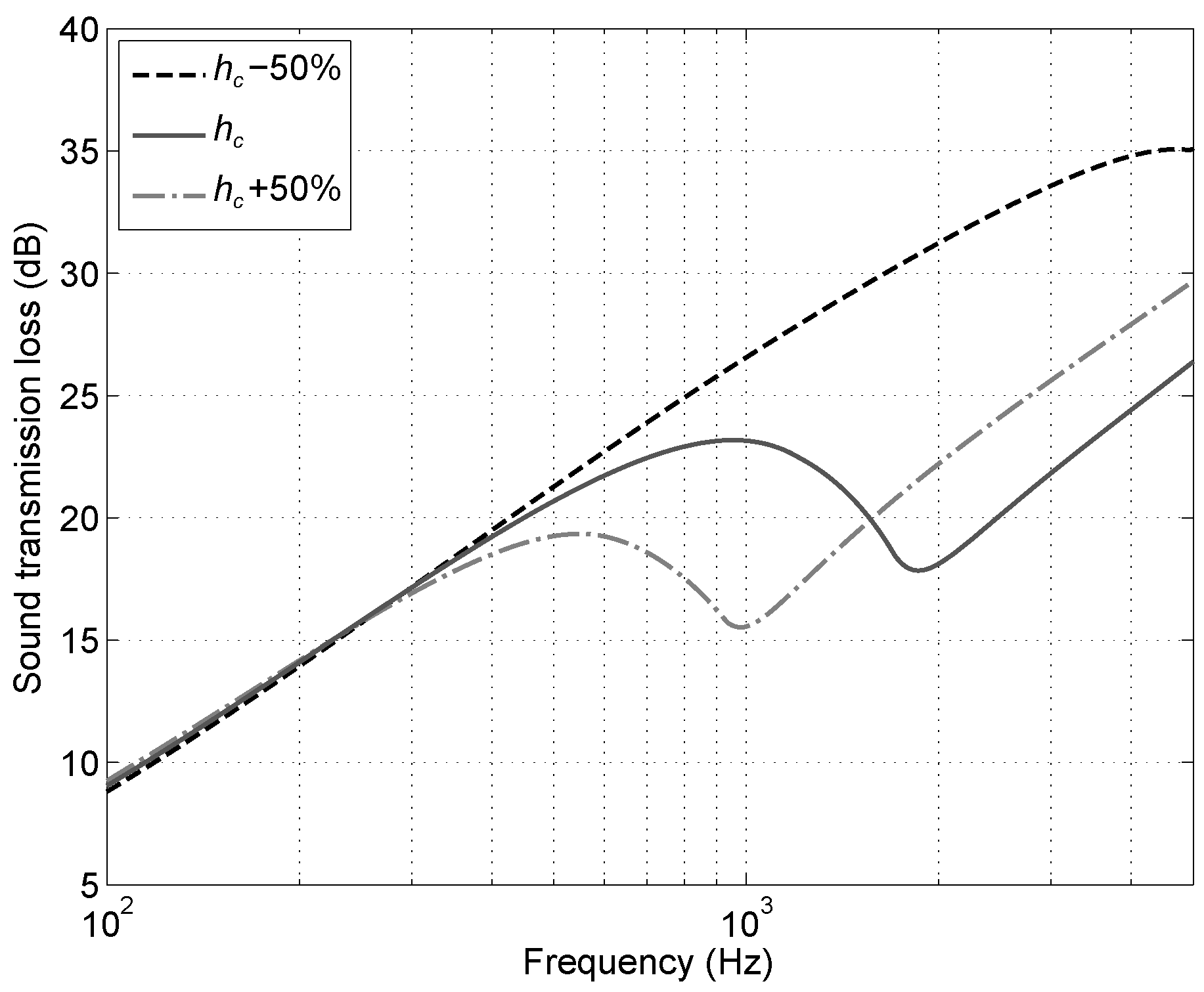
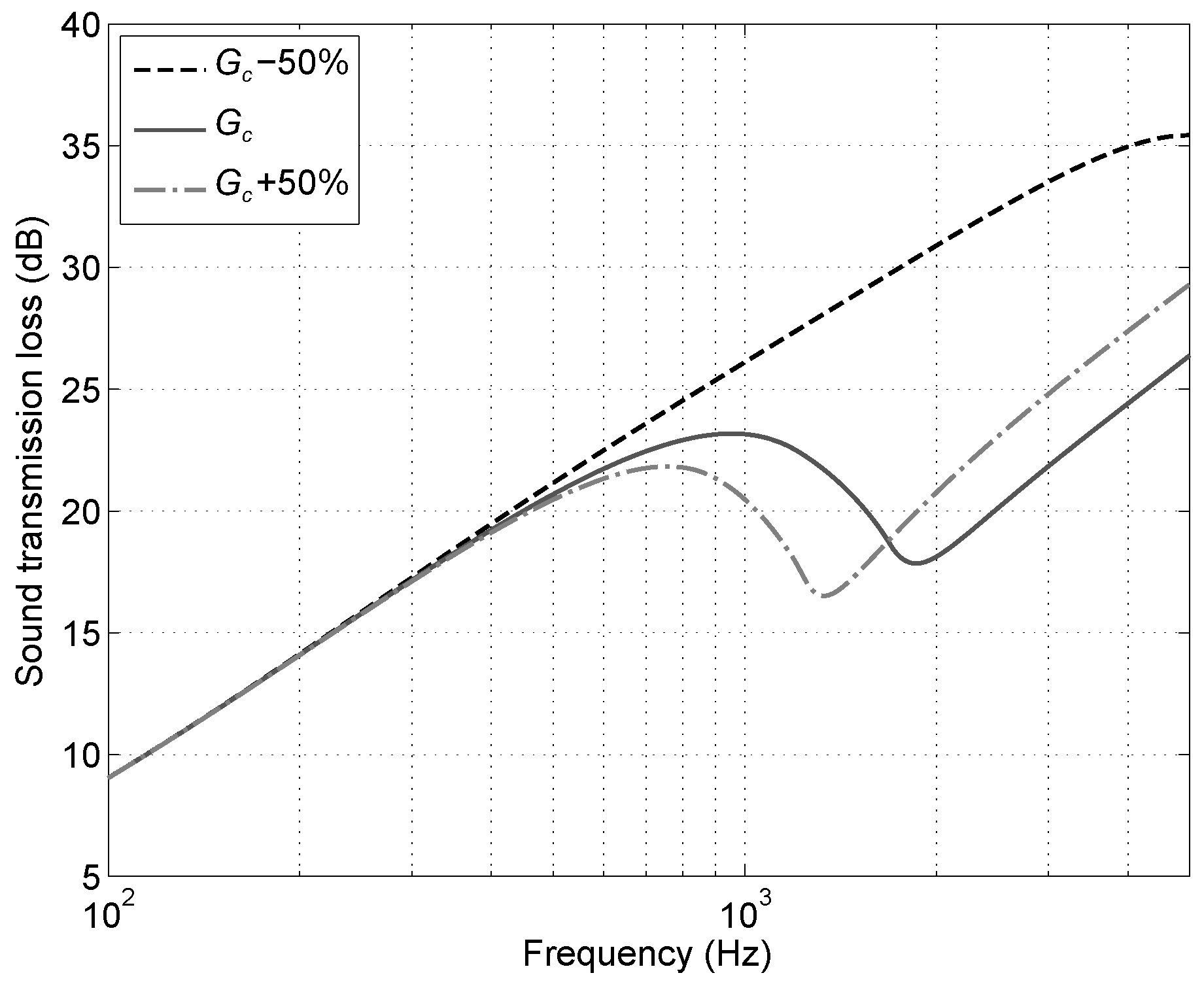
4.3. Parametric Study
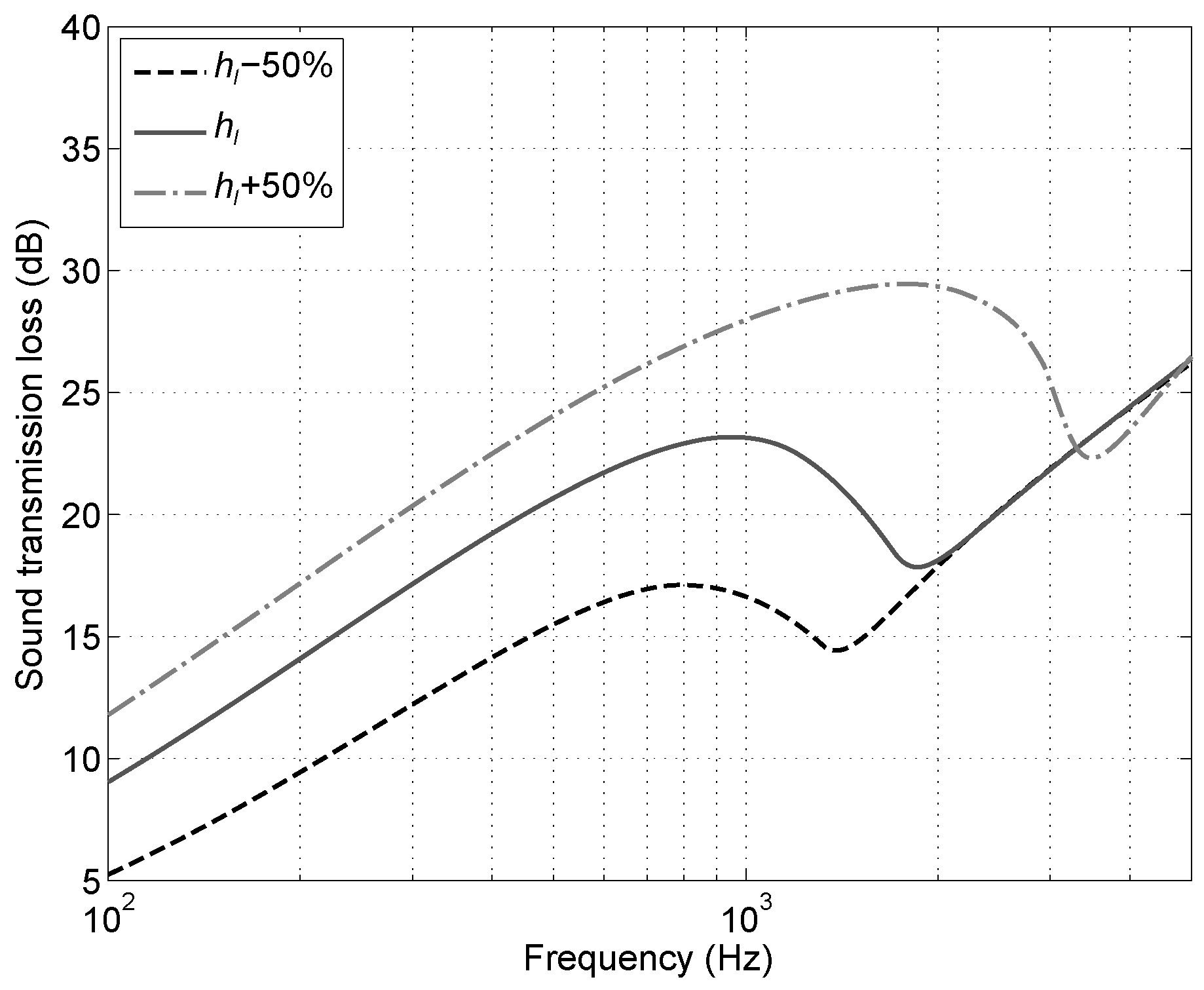
- Figure 17: Increasing the laminate thickness increases the stiffness of the structure and its surface mass. As a result, the sound transmission loss below the critical frequency, following the mass law, increases, while the coincidence region moves to higher frequencies. No significant difference can be observed above due to the identical loss factor applied.
- Figure 18: An increase in the core thickness does not change the mass significantly since it is lightweight. Therefore, the mass-dominated region below does not change, either. The coincidence region moves to lower or higher frequencies depending on , since .
- Figure 19: Similar considerations to changing the core thickness also apply to changing the core shear modulus. The latter approach can be an interesting alternative in the case of weight or size constraints.
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- U.S. Department of Transportation; Federal Aviation Administration. Advanced Composite Materials. In Aviation Maintenance Technician Handbook-Airframe, 2012 ed.; Aviation Supplies and Academics, Inc.: Newcastle, WA, USA, 2012; Volume 1. [Google Scholar]
- Kumar, S.; Feng, L.; Orrenius, U. Predicting the sound transmission loss of honeycomb panels using the wave propagation approach. Acta Acust. United Acust. 2011, 97, 869–876. [Google Scholar] [CrossRef]
- Crupi, V.; Epasto, G.; Guglielmino, E. Comparison of aluminum sandwiches for lightweight ship structures: Honeycomb vs. foam. Mar. Struct. 2013, 30, 74–96. [Google Scholar] [CrossRef]
- Kee, P.; Thayamballi, A.; Sung, K. Strength characteristics of aluminum honeycomb sandwich panels. Thin-Walled Struct. 1999, 35, 205–231. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, F. Analysis for equivalent in-plane elastic parameters of the honeycomb core. Appl. Mech. Mater. 2014, 441, 84–90. [Google Scholar] [CrossRef]
- Che, L.; Xu, G.D.; Zeng, T.; Cheng, S.; Zhou, X.W.; Yang, S.C. Compressive and shear characteristics of an octahedral stitched sandwich composite. Compos. Struct. 2014, 112, 179–187. [Google Scholar] [CrossRef]
- Petrone, G.; D’Alessandro, V.; Franco, F.; De Rosa, S. Numerical and experimental investigations on the acoustic power radiated by Aluminium Foam Sandwich panels. Compos. Struct. 2014, 118, 170–177. [Google Scholar] [CrossRef]
- Xu, X.; Jiang, Y.; Pueh, L. Multi-objective optimal design of sandwich panels using a genetic algorithm. Eng. Optim. 2017, 49, 1665–1684. [Google Scholar] [CrossRef]
- Xu, X.M.; Jiang, Y.P.; Lee, H.P.; Chen, N. Sound insulation performance optimization of lightweight sandwich panels. J. Vibroeng. 2016, 18, 2574–2586. [Google Scholar] [CrossRef]
- Griese, D.; Summers, J.; Thompson, L. The effect of honeycomb core geometry on the sound transmission performance of sandwich panels. J. Vib. Acoust. Trans. ASME 2015, 137. [Google Scholar] [CrossRef]
- Grünewald, J.; Parlevliet, P.; Altstädt, V. Manufacturing of thermoplastic composite sandwich structures: A review of literature. J. Thermoplast. Compos. Mater. 2017, 30, 437–464. [Google Scholar] [CrossRef]
- Krzyzak, A.; Mazur, M.; Gajewski, M.; Drozd, K.; Komorek, A.; Przybyłek, P. Sandwich Structured Composites for Aeronautics: Methods of Manufacturing Affecting Some Mechanical Properties. Int. J. Aerosp. Eng. 2016, 2016. [Google Scholar] [CrossRef]
- Pourvais, Y.; Asgari, P.; Moradi, A.; Rahmani, O. Experimental and finite element analysis of higher order behavior of sandwich beams using digital projection moiré. Polym. Test. 2014, 38, 7–17. [Google Scholar] [CrossRef]
- Li, P.; Liu, S.; Lu, Z. Experimental study on the performance of polyurethane-steel sandwich structure under debris flow. Appl. Sci. Switz. 2017, 7, 1018. [Google Scholar] [CrossRef]
- Piana, E.; Marchesini, A.; Nilsson, A. Evaluation of different methods to predict the transmission loss of sandwich panels. In Proceedings of the 20th International Congress on Sound and Vibration, Bangkok, Thailand, 7–11 July 2013; International Institute of Acoustics and Vibrations: Auburn, AL, USA, 2013; Volume 4, pp. 3552–3559. [Google Scholar]
- Visentin, C.; Prodi, N.; Bonfiglio, P.; Pompoli, R. On the prediction of transmission loss of honeycomb panels: Experimental measurements and numerical simulations. In Proceedings of the 7th Forum Acusticum, FA 2014, Krakow, Poland, 7–12 September 2014; European Acoustics Association: Madrid, Spain, 2014; Volume 2014-January, pp. 1–6. [Google Scholar]
- Piana, E.; Marchesini, A. How to lower the noise level in the owner’s cabin of a yacht through the improvement of bulkhead and floor. In Proceedings of the 21st International Congress on Sound and Vibration, Beijing, China, 13–17 July 2014; International Institute of Acoustics and Vibrations: Auburn, AL, USA, 2014; Volume 5, pp. 3692–3699. [Google Scholar]
- Davy, J.; Cowan, A.; Pearse, J.; Latimer, M. Predicting the sound insulation of lightweight sandwich panels. Build. Acoust. 2013, 20, 177–192. [Google Scholar] [CrossRef]
- Scamoni, F.; Piana, E.; Scrosati, C. Experimental evaluation of the sound absorption and insulation of an innovative coating through different testing methods. Build. Acoust. 2017, 24, 173–191. [Google Scholar] [CrossRef]
- Cremer, L.; Heckl, M.; Petersson, B.A.T. Structural Vibrations and Sound Radiation at Audio Frequencies, 3rd ed.; Springer: Berlin, Germany; New York, NY, USA, 2005. [Google Scholar]
- Cremer, L. Theorie der Schalldämmung dünner Wände bei schrägem Einfall. Akustische Zeitschrift 1942, 7, 81–104. [Google Scholar]
- Sharp, B.H. Prediction Methods for the Sound Transmission of Building Elements. Noise Control Eng. J. 1978, 11, 53–63. [Google Scholar] [CrossRef]
- D’Alessandro, V.; Petrone, G.; Franco, F.; De Rosa, S. A review of the vibroacoustics of sandwich panels: Models and experiments. J. Sandw. Struct. Mater. 2013, 15, 541–582. [Google Scholar] [CrossRef]
- Nilsson, A.; Liu, B. Vibro-Acoustics: 2, 2nd ed.; Springer: Berlin, Germany, 2015. [Google Scholar]
- Nilsson, A. Wave propagation in and sound transmission through sandwich plates. J. Sound Vib. 1990, 138, 73–94. [Google Scholar] [CrossRef]
- Nilsson, E.; Nilsson, A. Prediction and measurement of some dynamic properties of sandwich structures with honeycomb and foam cores. J. Sound Vib. 2002, 251, 409–430. [Google Scholar] [CrossRef]
- Backström, D.; Nilsson, A. Modelling the vibration of sandwich beams using frequency-dependent parameters. J. Sound Vib. 2007, 300, 589–611. [Google Scholar] [CrossRef]
- Blevins, R.D. Formulas for Natural Frequency and Mode Shape; Van Nostrand Reinhold Co.: New York, NY, USA, 1979. [Google Scholar]
- Piana, E.; Milani, P.; Granzotto, N. Simple method to determine the transmission loss of gypsum panels. In Proceedings of the 21st International Congress on Sound and Vibration, Beijing, China, 13–17 July 2014; International Institute of Acoustics and Vibrations: Auburn, AL, USA, 2014; Volume 5, pp. 3700–3706. [Google Scholar]
- Piana, E. A method for determining the sound reduction index of precast panels based on point mobility measurements. Appl. Acoust. 2016, 110, 72–80. [Google Scholar] [CrossRef]
- Piana, E.; Petrogalli, C.; Solazzi, L. Dynamic and acoustic properties of a joisted floor. In Proceedings of the 6th International Conference on Simulation and Modeling Methodologies, Technologies and Applications, Lisbon, Portugal, 29–31 July 2016; Obaidat, M.S., Merkuryev, Y., Oren, T., Eds.; SciTePress: Setúbal, Portugal, 2016; pp. 277–282. [Google Scholar]
- Hopkins, C. Sound Insulation, 1st ed.; Butterworth-Heinemann: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Maidanik, G. Response of Ribbed Panels to Reverberant Acoustic Fields. J. Acoust. Soc. Am. 1962, 34, 809–826. [Google Scholar] [CrossRef]
- Leppington, F.; Broadbent, E.; Heron, K. The acoustic radiation efficiency of rectangular panels. Proc. R. Soc. Lon. Ser. A Math. Phys. Sci. 1982, 382, 245–271. [Google Scholar] [CrossRef]
- Bies, D.A.; Hansen, C.H. Engineering Noise Control: Theory and Practice, 4th ed.; CRC Press: London, UK; New York, NY, USA, 2009. [Google Scholar]
- International Organization for Standardization. ISO 10140-2:2010–Acoustics–Laboratory Measurement Of Sound Insulation of Building Elements–Part 2: Measurement of Airborne Sound Insulation; ISO: Geneva, Switzerland, 2010. [Google Scholar]
- Farina, A. AURORA Plug-ins. Available online: http://pcfarina.eng.unipr.it/Aurora_XP/index.htm (accessed on 25 November 2017).
- American Society for Testing and Materials. ASTM C273/C273M-16–Standard Test Method for Shear Properties of Sandwich Core Materials; ASTM: West Conshohocken, PA, USA, 2016. [Google Scholar]




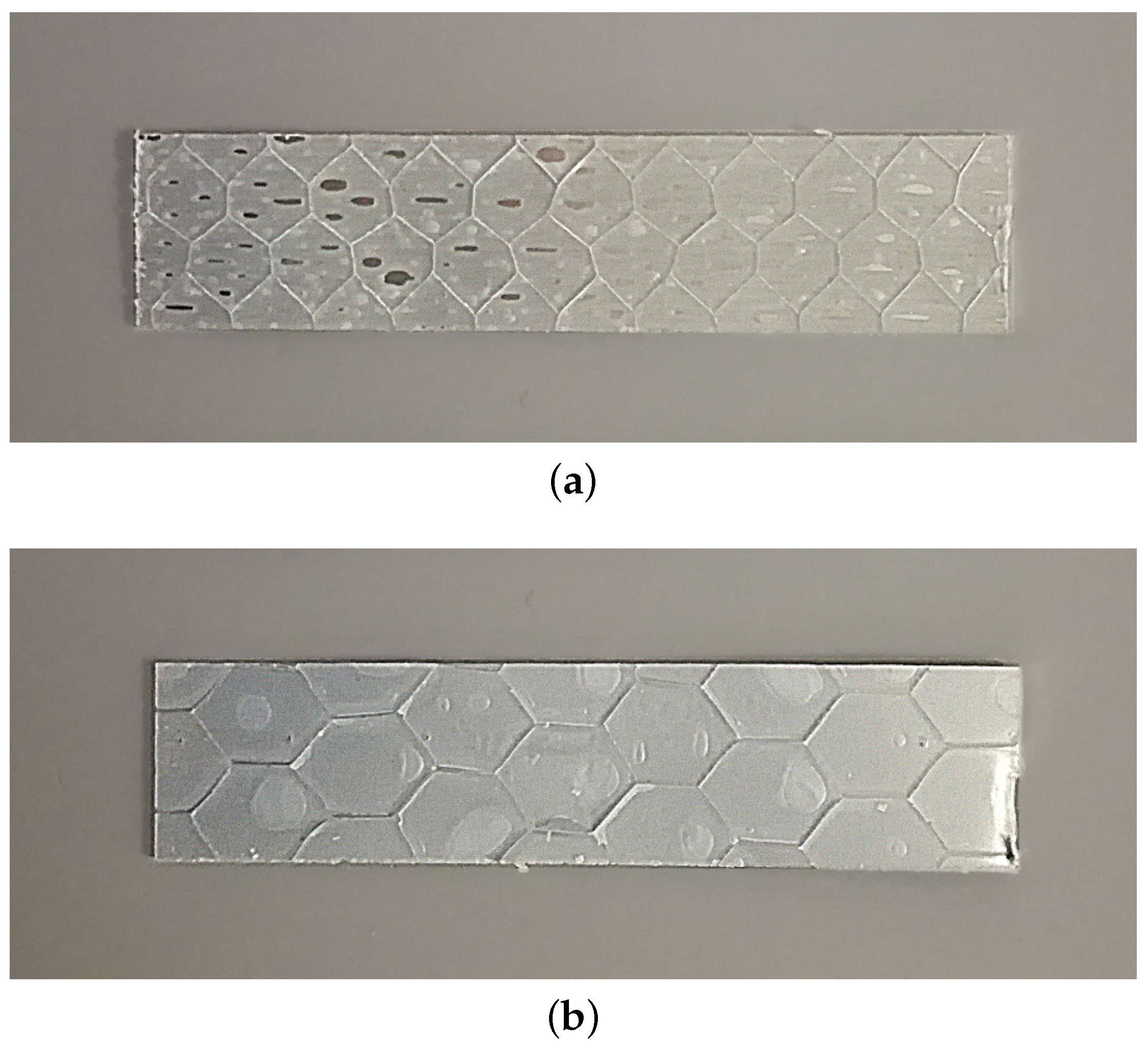

| n | 1 | 2 | 3 | 4 | ≥5 |
|---|---|---|---|---|---|
| 4.73 | 7.85 | 11.0 | 14.14 |
| Characteristics | Symbol | Units | x-Direction | y-Direction |
|---|---|---|---|---|
| Length | L | m | 1.803 | 0.993 |
| Core thickness | m | 0.024 | ||
| Laminate thickness | m | 0.001 | ||
| Width | b | m | 0.050 | |
| Mass per unit area | kg m | 6.72 | ||
| Young’s modulus of the laminates | GPa | 72 | ||
| Cell size | - | in | 3/8 | |
| Core density | kg m | 46 | ||
| Manufacturer Model | Euro-Composites ECM 9.6-41 | Alcore PAA-CORE™ 5052 | Alucoat AluNID |
|---|---|---|---|
| Cell size | 3/8 | 3/8 | 3/8 |
| (kg m) | 41 | 48 | 40 |
| G (x-Direction) (MPa) | 227 | 207 | 214 |
| G (y-Direction) (MPa) | 98 | 103 | 107 |
| Estimated | Product Datasheets (Average) | |
|---|---|---|
| (MPa) | 189 | 216 |
| (MPa) | 119 | 103 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piana, E.A.; Petrogalli, C.; Paderno, D.; Carlsson, U. Application of the Wave Propagation Approach to Sandwich Structures: Vibro-Acoustic Properties of Aluminum Honeycomb Materials. Appl. Sci. 2018, 8, 45. https://doi.org/10.3390/app8010045
Piana EA, Petrogalli C, Paderno D, Carlsson U. Application of the Wave Propagation Approach to Sandwich Structures: Vibro-Acoustic Properties of Aluminum Honeycomb Materials. Applied Sciences. 2018; 8(1):45. https://doi.org/10.3390/app8010045
Chicago/Turabian StylePiana, Edoardo Alessio, Candida Petrogalli, Diego Paderno, and Ulf Carlsson. 2018. "Application of the Wave Propagation Approach to Sandwich Structures: Vibro-Acoustic Properties of Aluminum Honeycomb Materials" Applied Sciences 8, no. 1: 45. https://doi.org/10.3390/app8010045






