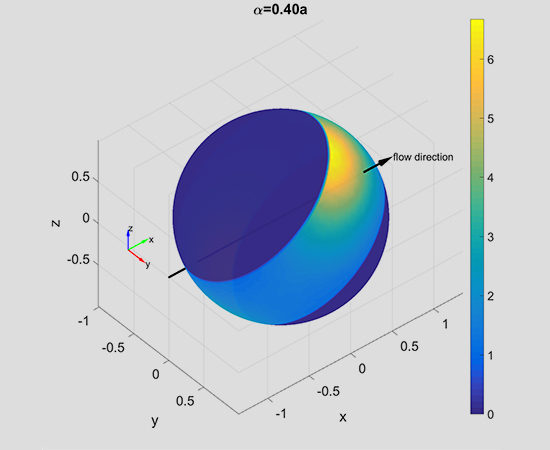
The Effect of Weak Confinement on the Orientation of Nanorods under Shear Flows
Abstract
:1. Introduction
2. Numerical Method
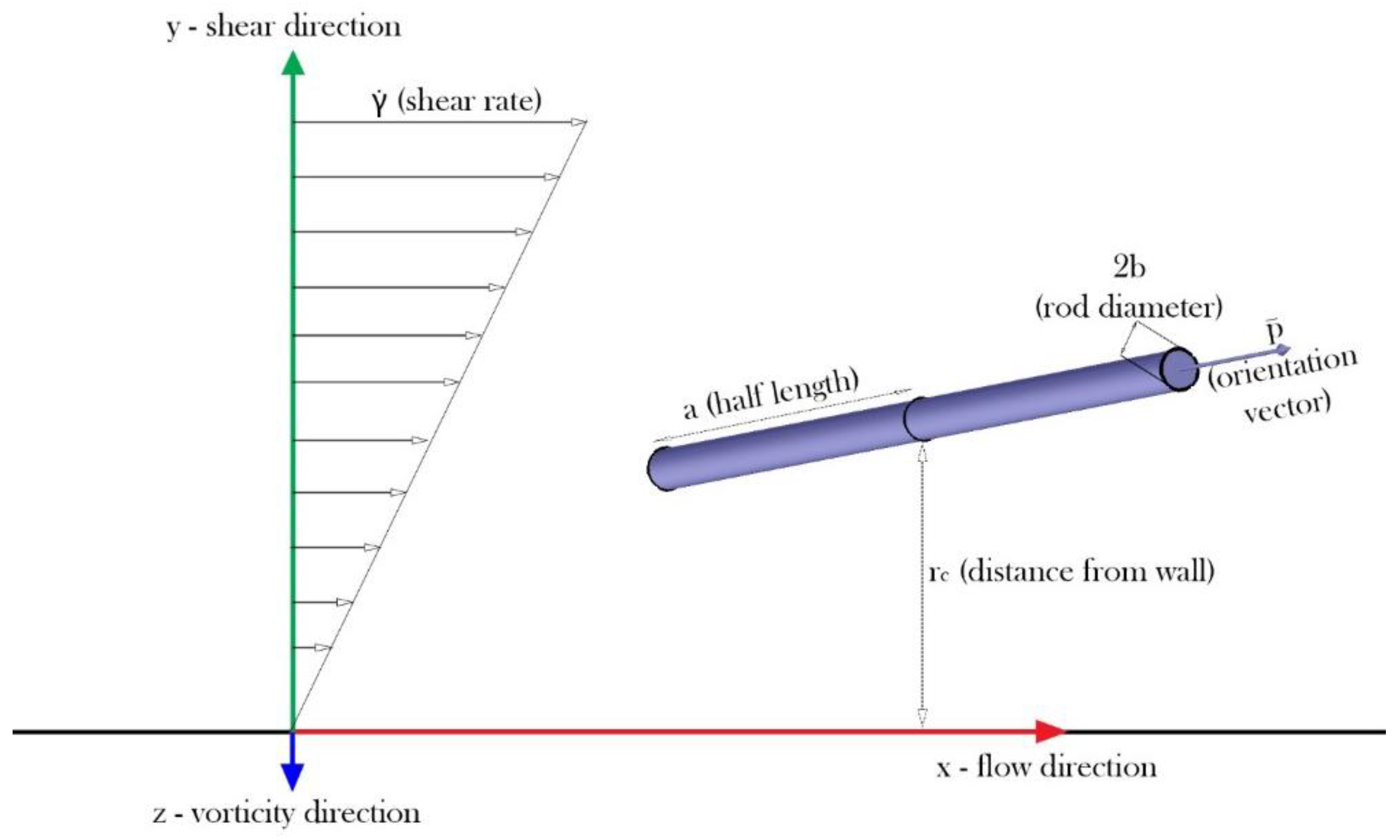
2.1. Definitions of the Variables for a Rod Configuration
2.2. Simulation Approach and Assumptions
2.3. Initial Configuration
- (1)
- px, py and pz are assigned a random number between −1 and 1.
- (2)
- If |p| > 1, repeat step (1). Otherwise, normalize px, py and pz with |p|.
- (3)
- If the normalized py is not between −α/a and +α/a, repeat steps (1) and (2) until py is correctly constrained (−α/a ≤ py ≤ +α/a).
2.4. Equation of Motion
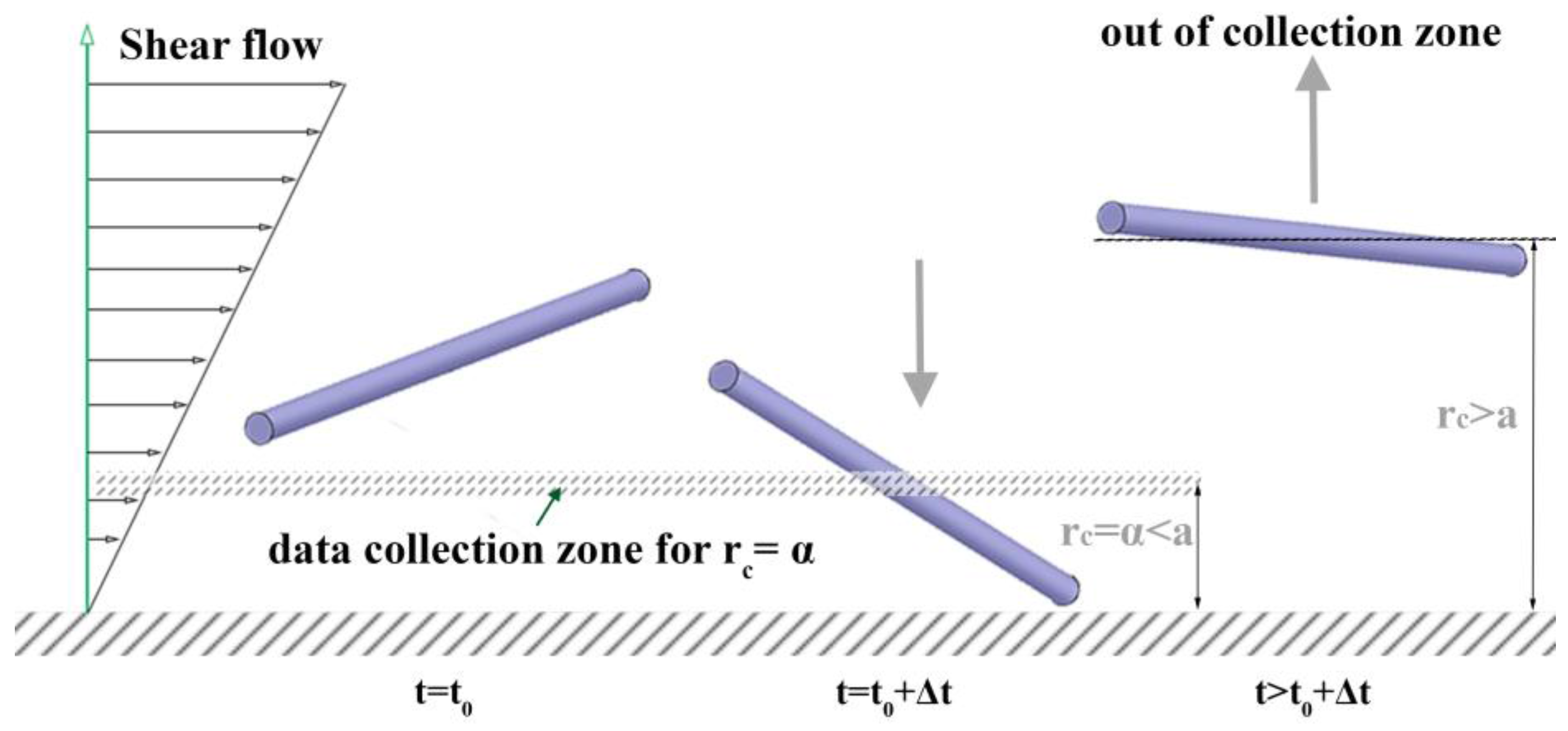
2.5. Sampling Data during Dynamic Simulation
2.6. Orientation Distribution
2.7. Average Orientation Moments Calculation
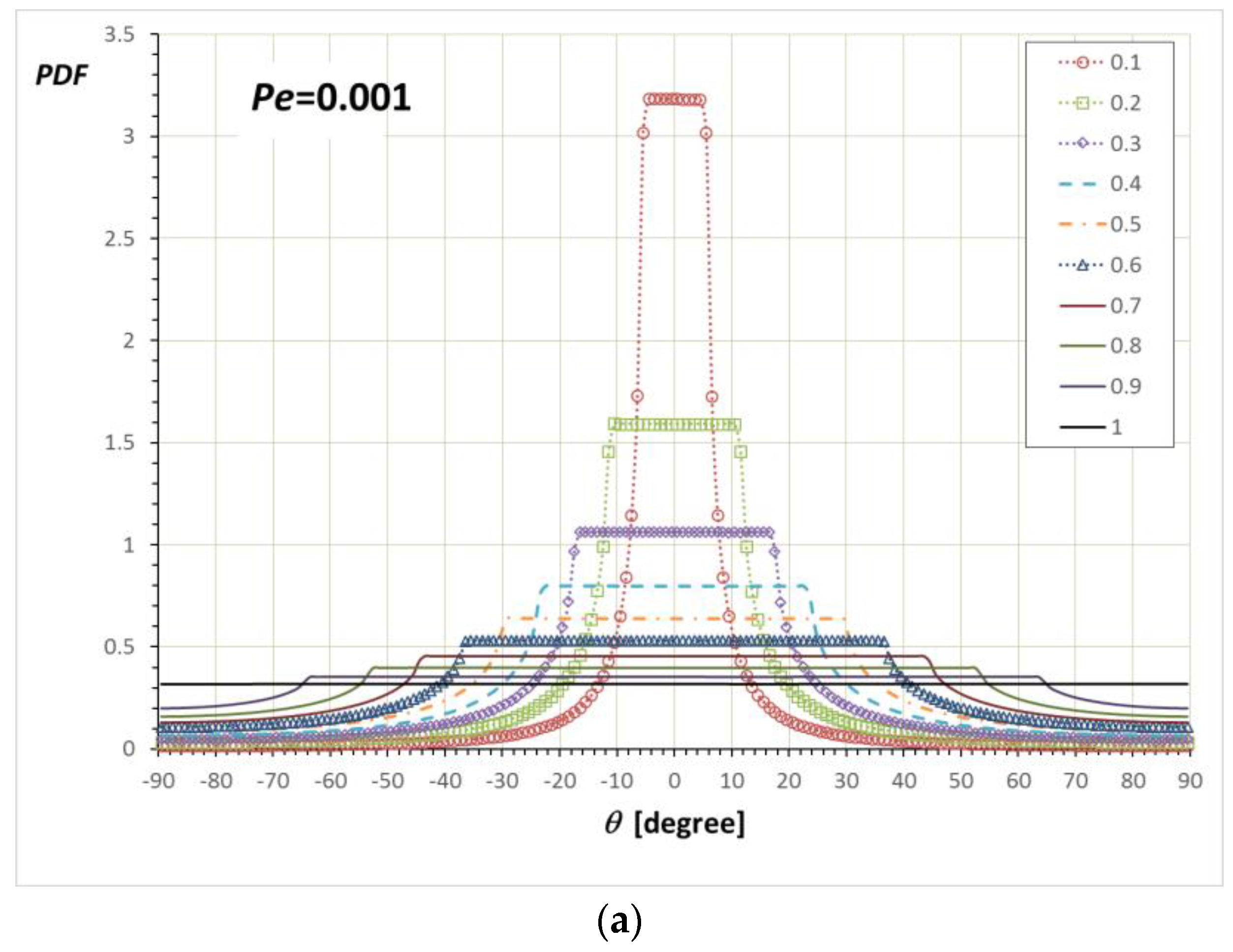
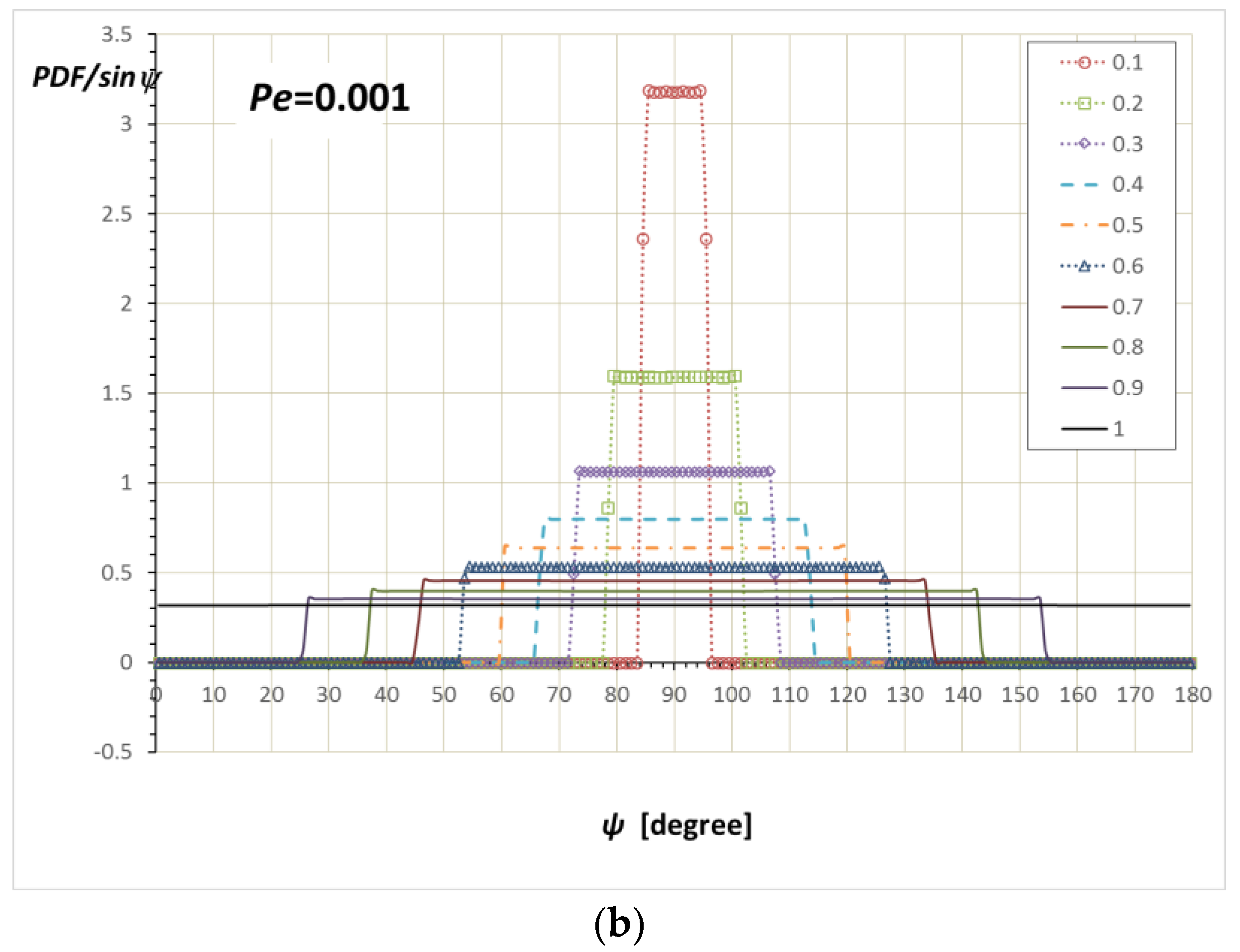
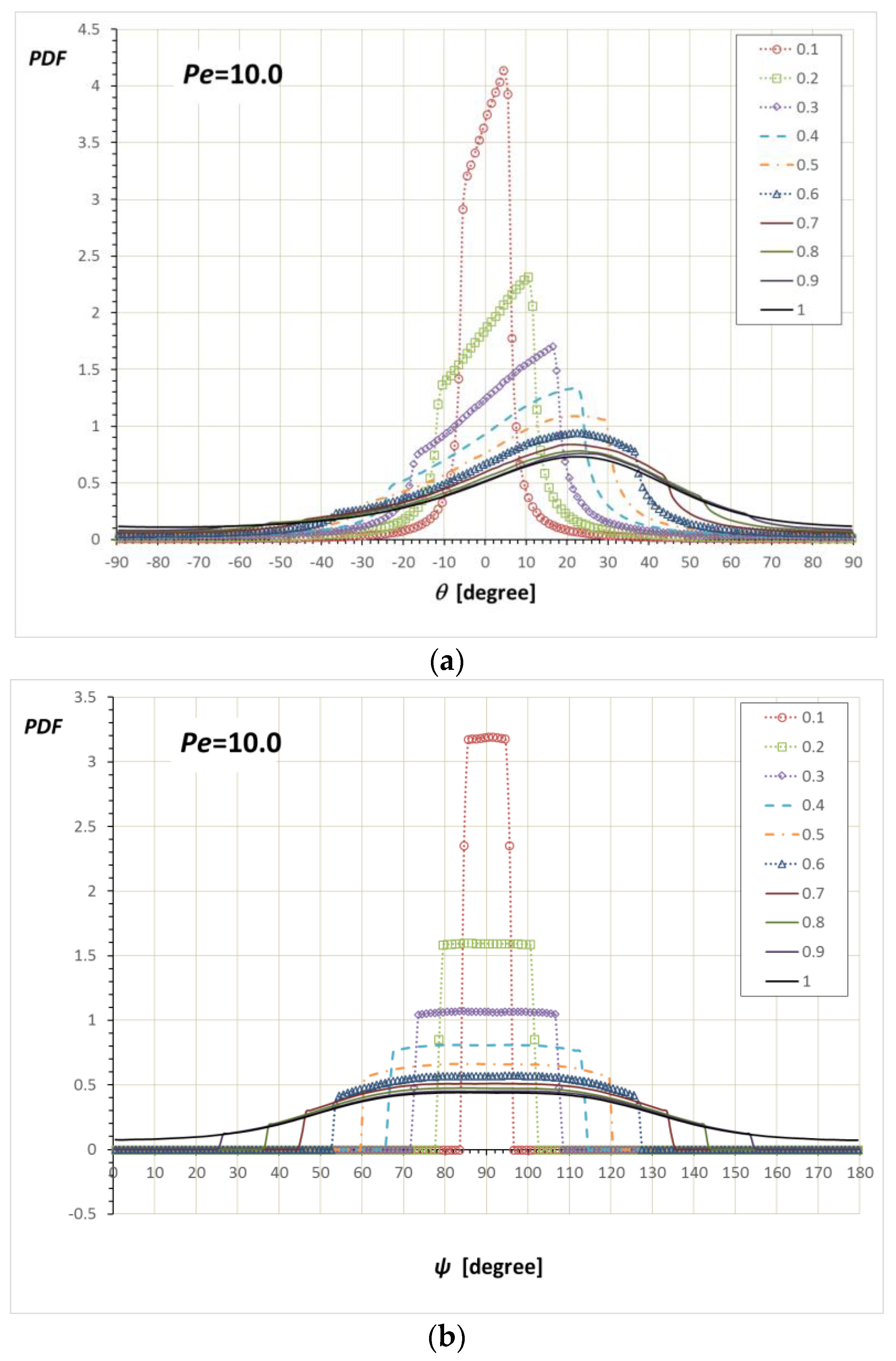
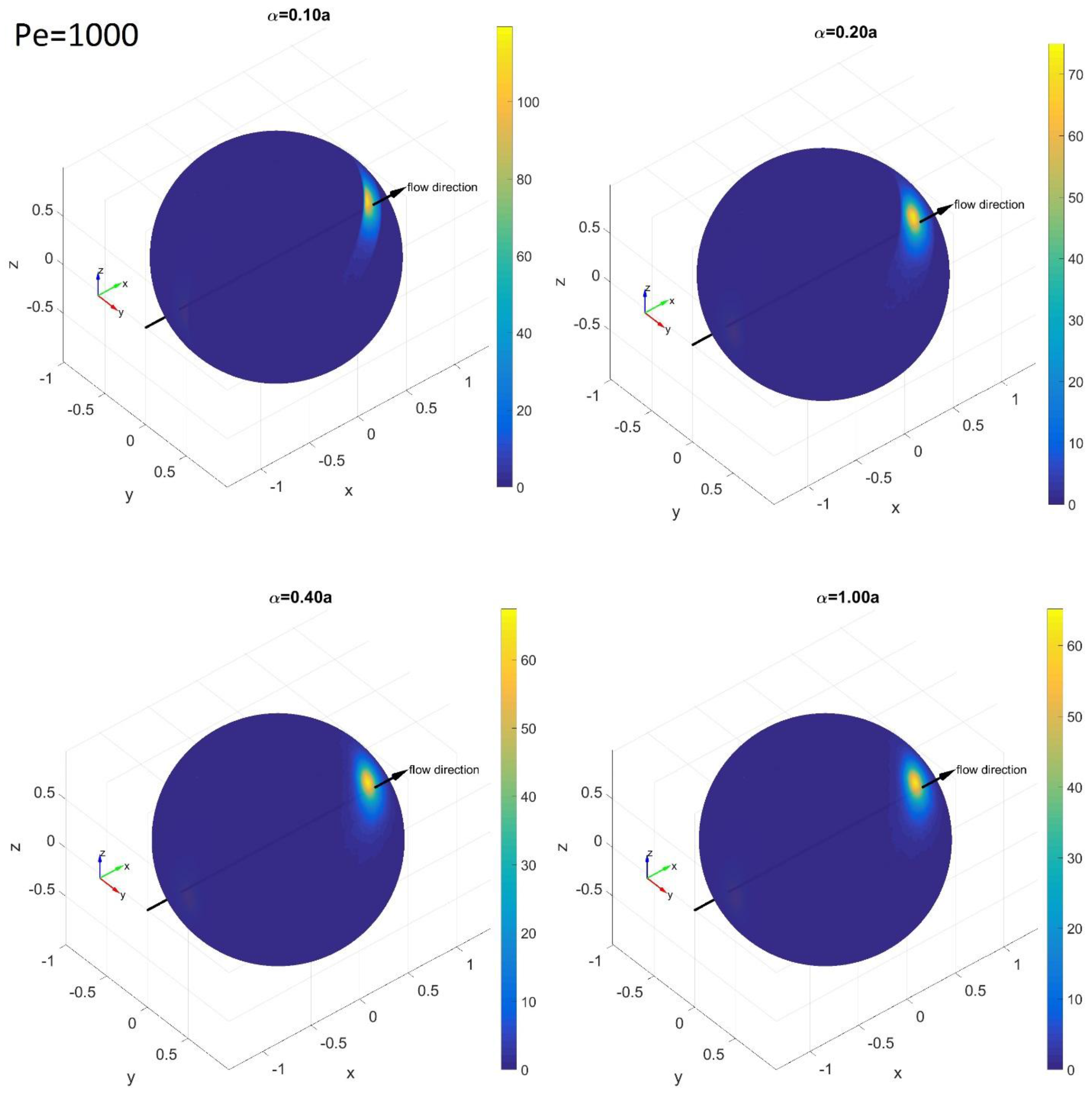
3. Results and Discussion
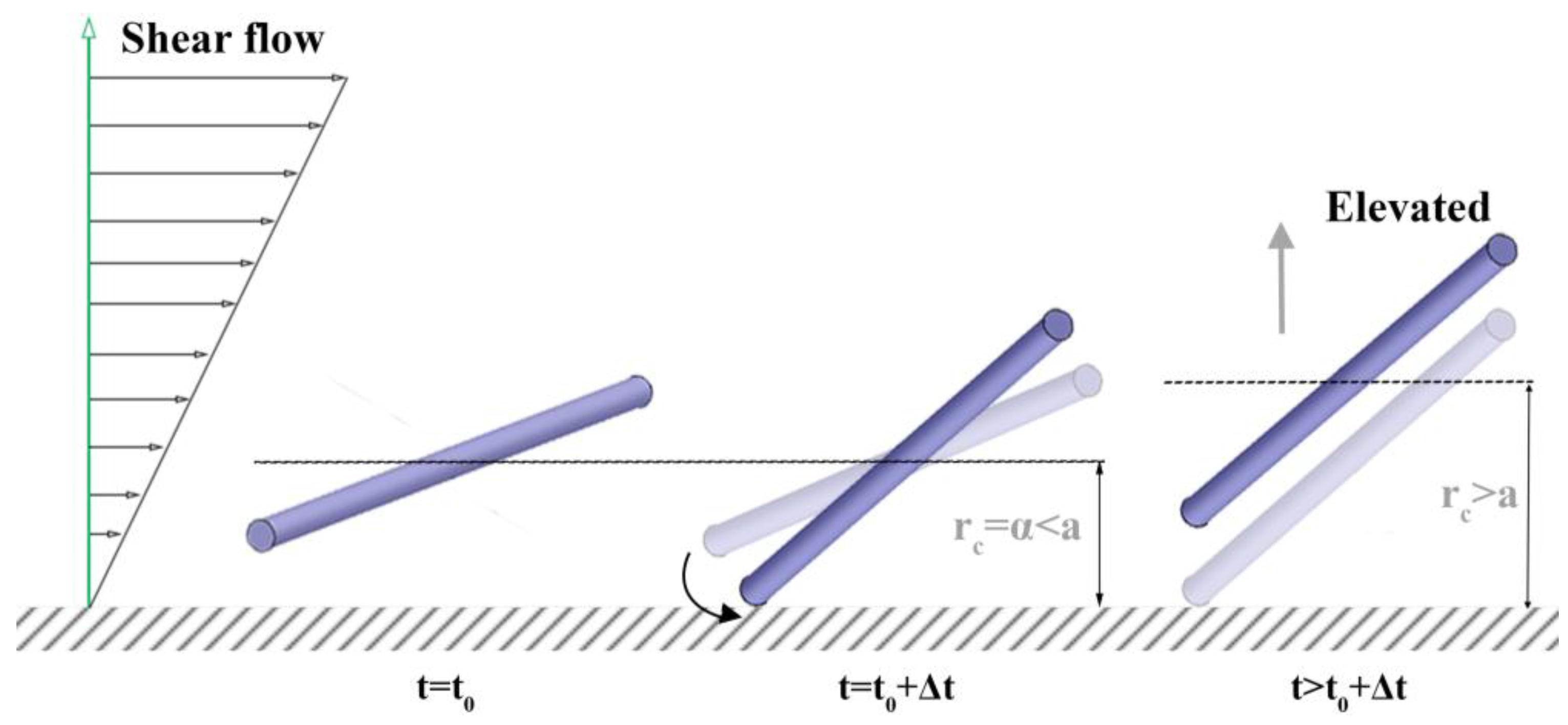
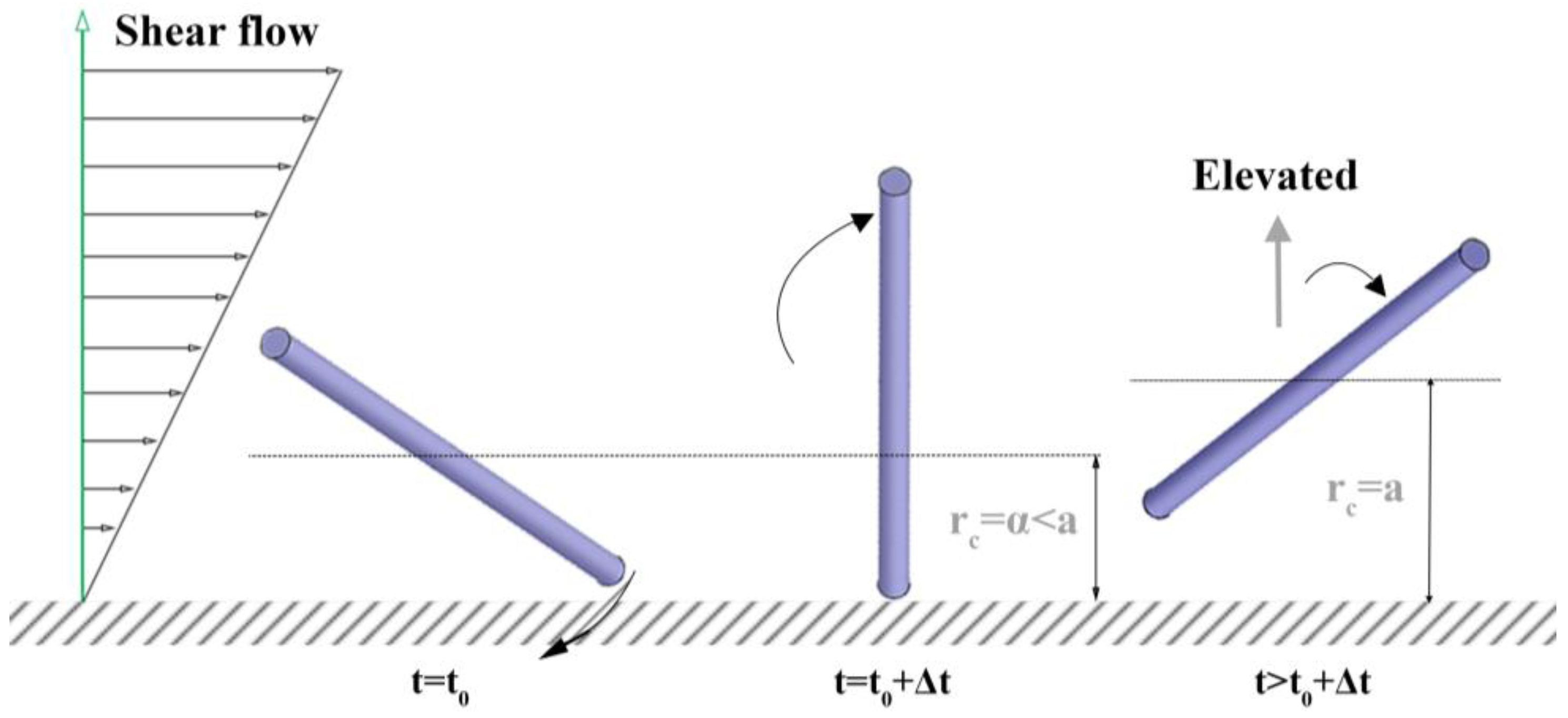
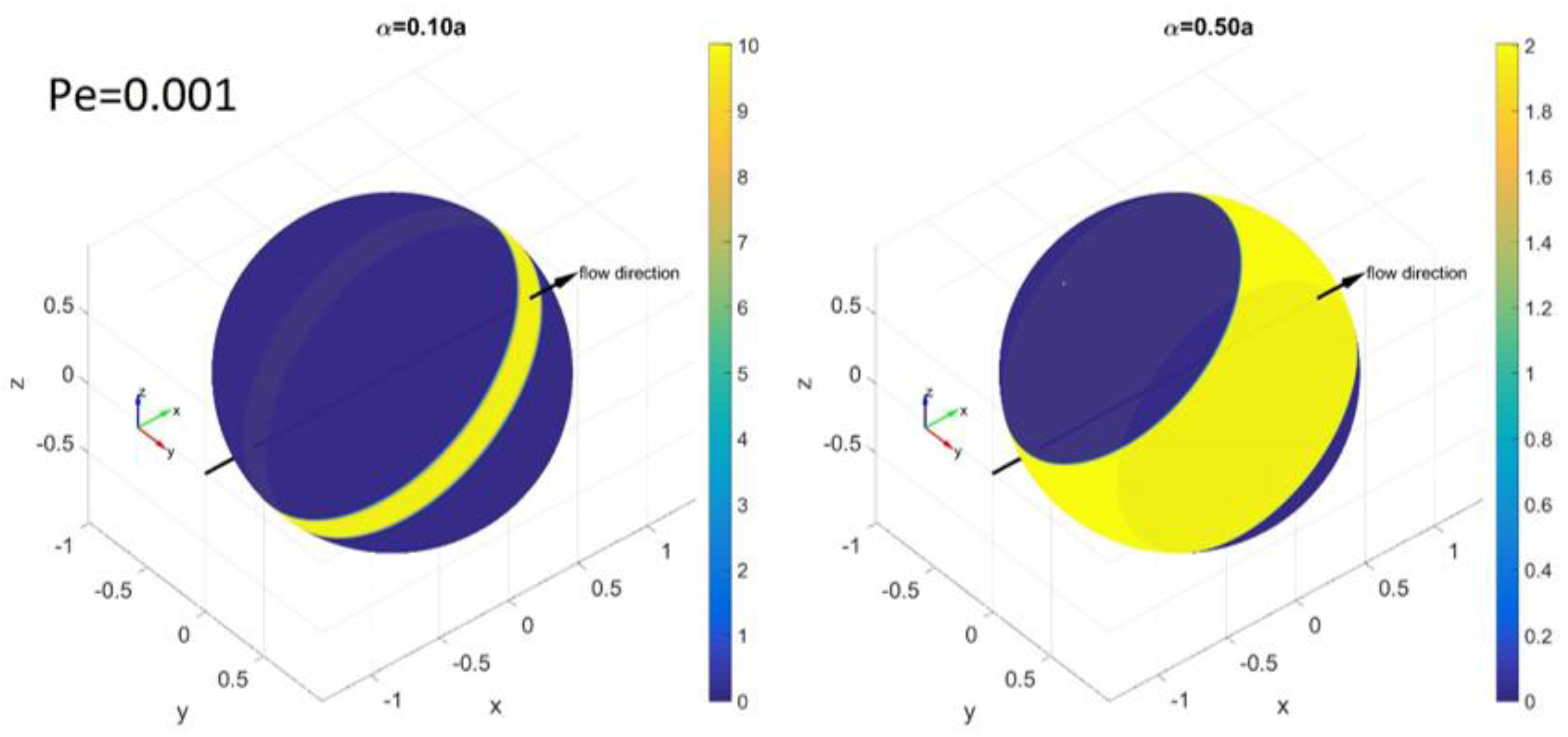
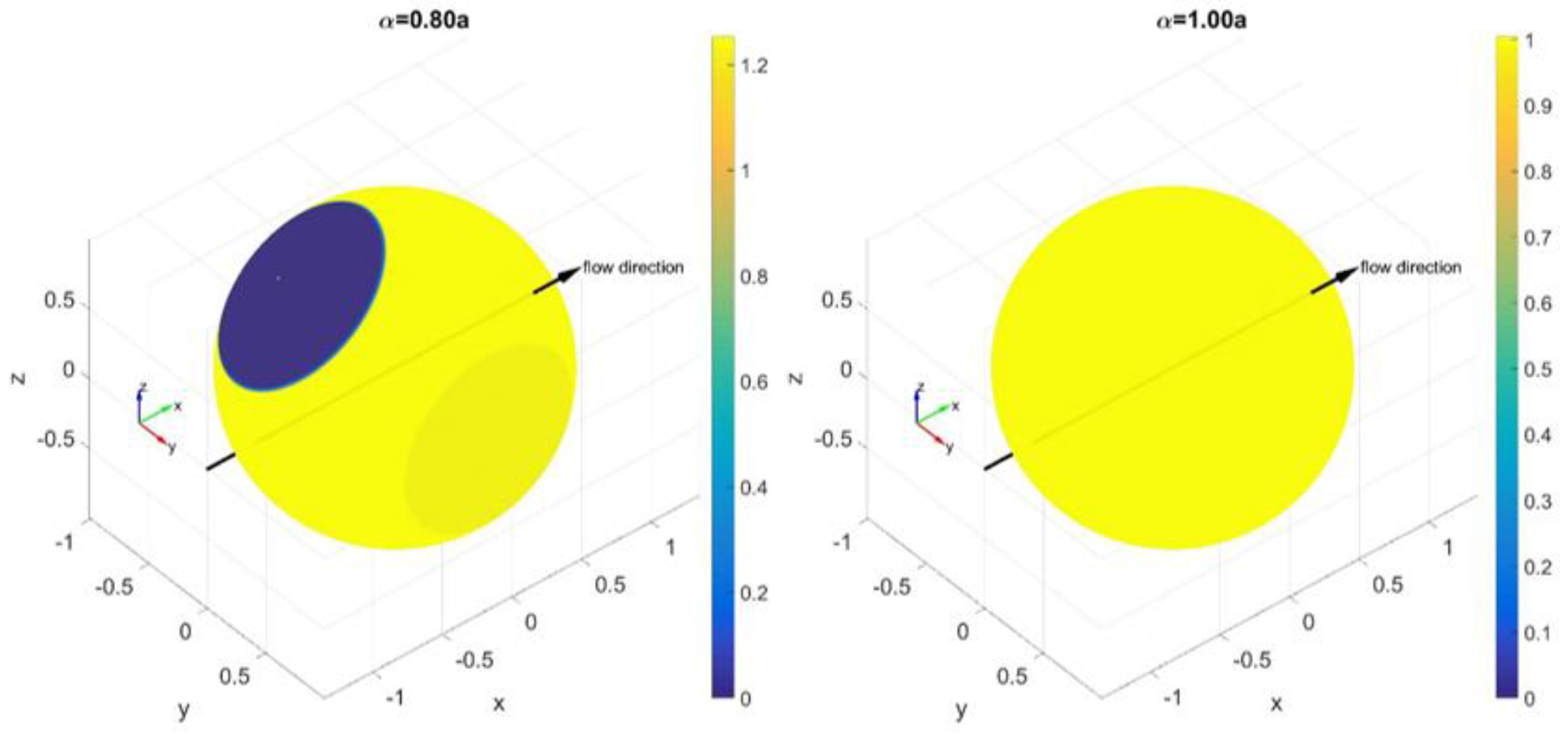
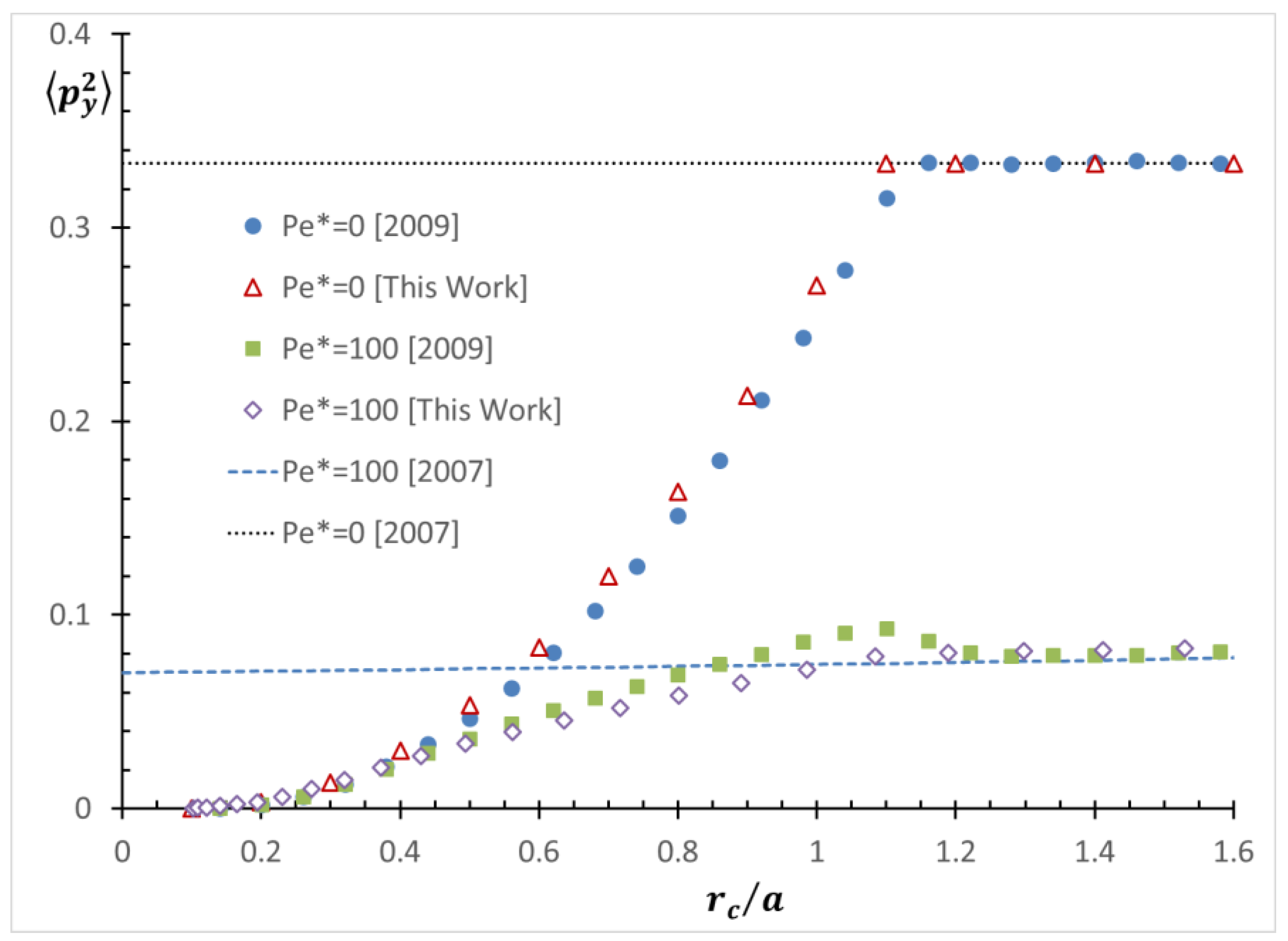
3.1. Orientation Distribution near a Wall
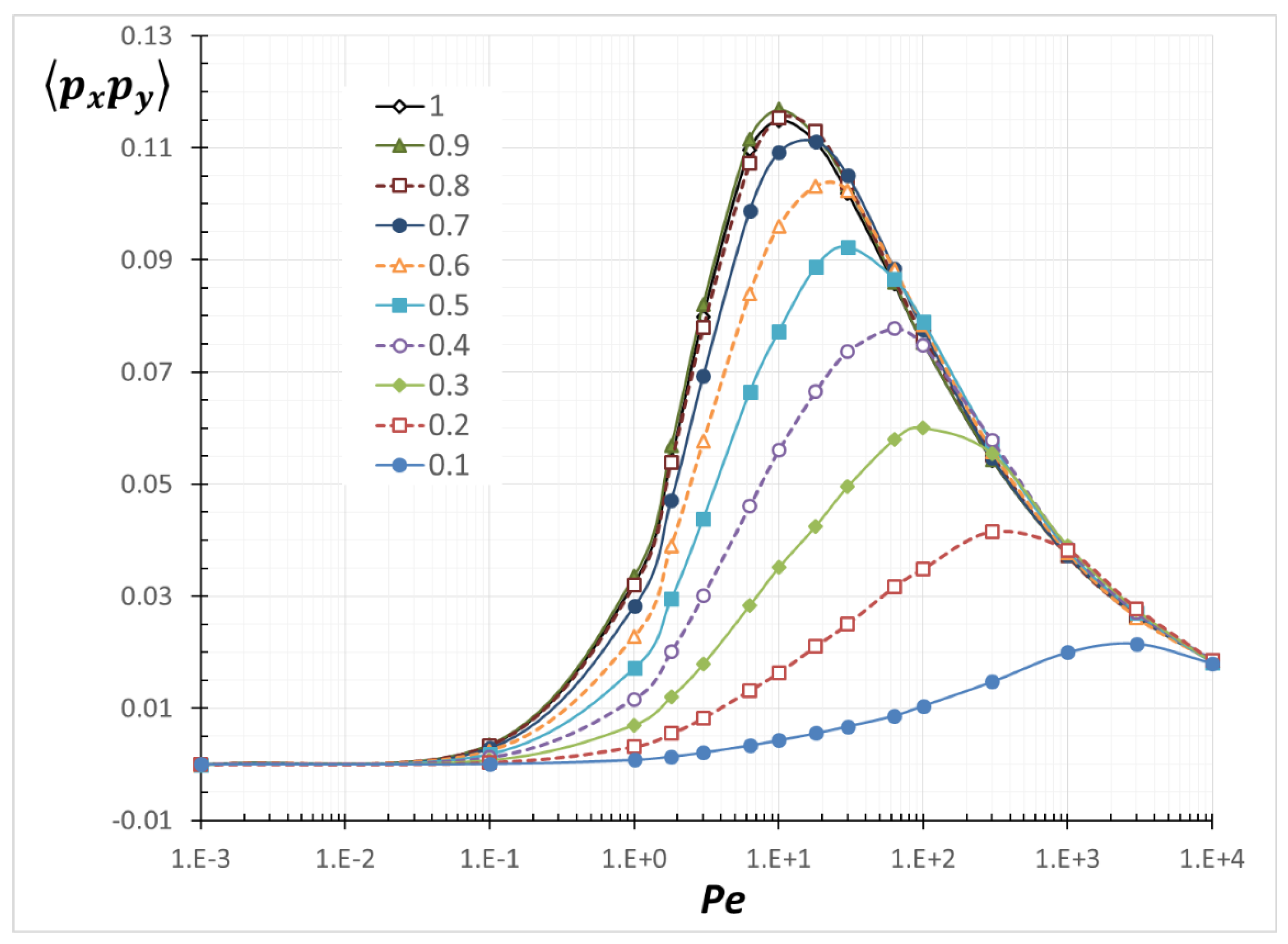
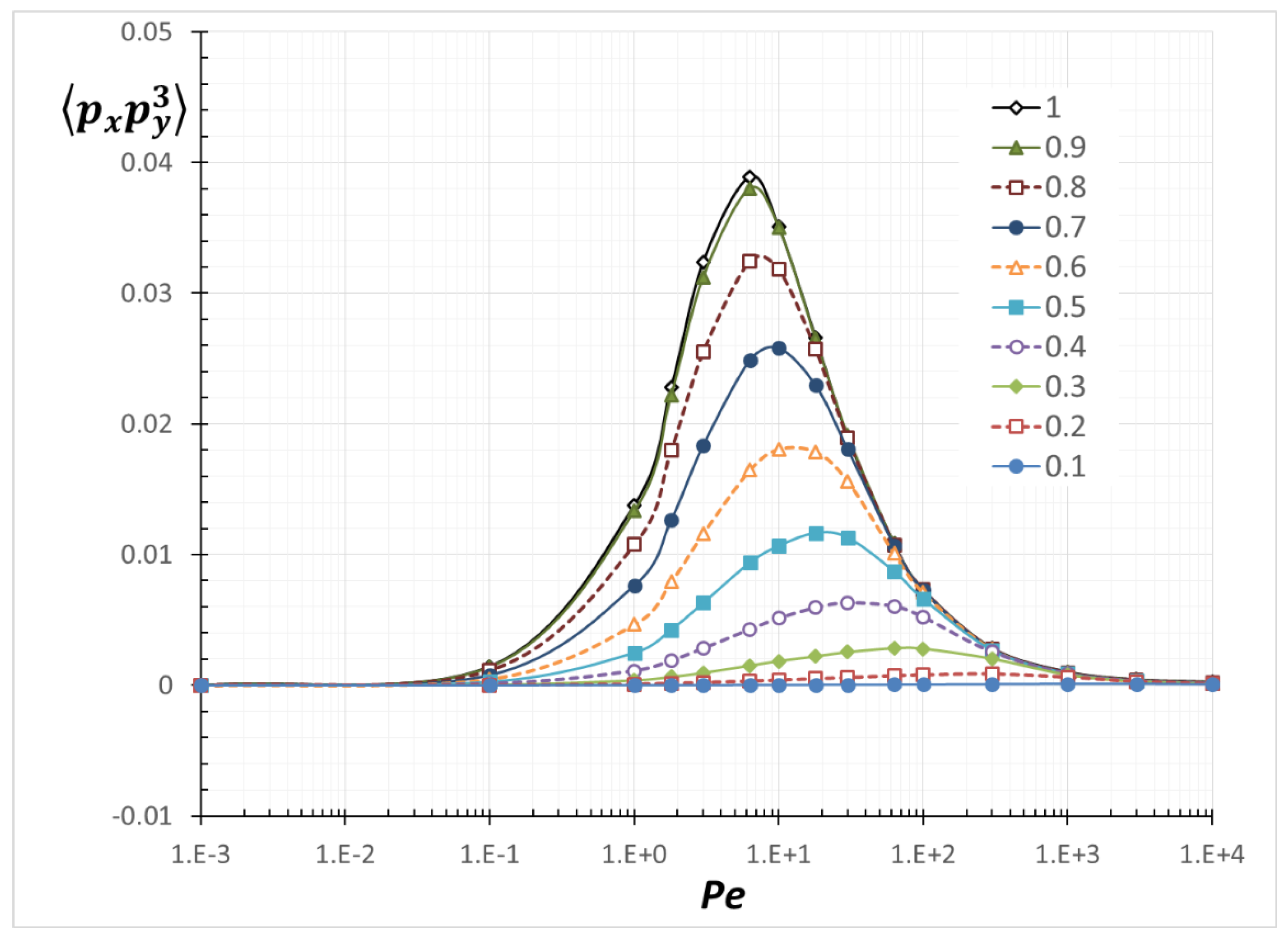
3.2. Average Orientation Moments near a Wall
3.3. Application to Improving a Shear-Induced Migration Theory
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| Notation | |
| a | the half-length of the long principal axis of a rod |
| b | the half-length of the short principal axis of a rod |
| d | the length of the short principal axis (diameter or thickness) of a rod |
| DR | Rotational diffusivity of a rod |
| I | identity matrix |
| kB | Boltzmann constant |
| L | the length of the long principal axis of a rod |
| m | index of the sample time |
| M(n) | the total number of sampled orientation data for the n-th particle. |
| n | index of a particle |
| N | the number of particles in each set of simulation |
| rc | the rod center-of-mass position |
| p | rod orientation vector with a magnitude of unity |
| pi | the i-direction component of p |
| probability distribution function (normalized so that its integration gives 1) | |
| Pe | rotational Peclet number |
| Pe* | rotational Peclet number averaged over cross section for a pressure driven flow |
| Pe(y) | local rotational Peclet number at a cross sectional position y in a pressure driven flow |
| t | dimensionless time |
| tm,n | the m-th sampling time for the n-th particle |
| Brownian torque | |
| T | Absolute temperature of the flow |
| w | random vector with zero mean and variance of 1 |
| a unit vector in the x-direction | |
| x | flow direction in the Cartesian coordinate system |
| y | shear direction in the Cartesian coordinate system |
| z | vorticity direction in the Cartesian coordinate system |
| Greek Letters | |
| shear rate | |
| α | wall confinement (distance from the wall surface to the rod center-of-mass position) |
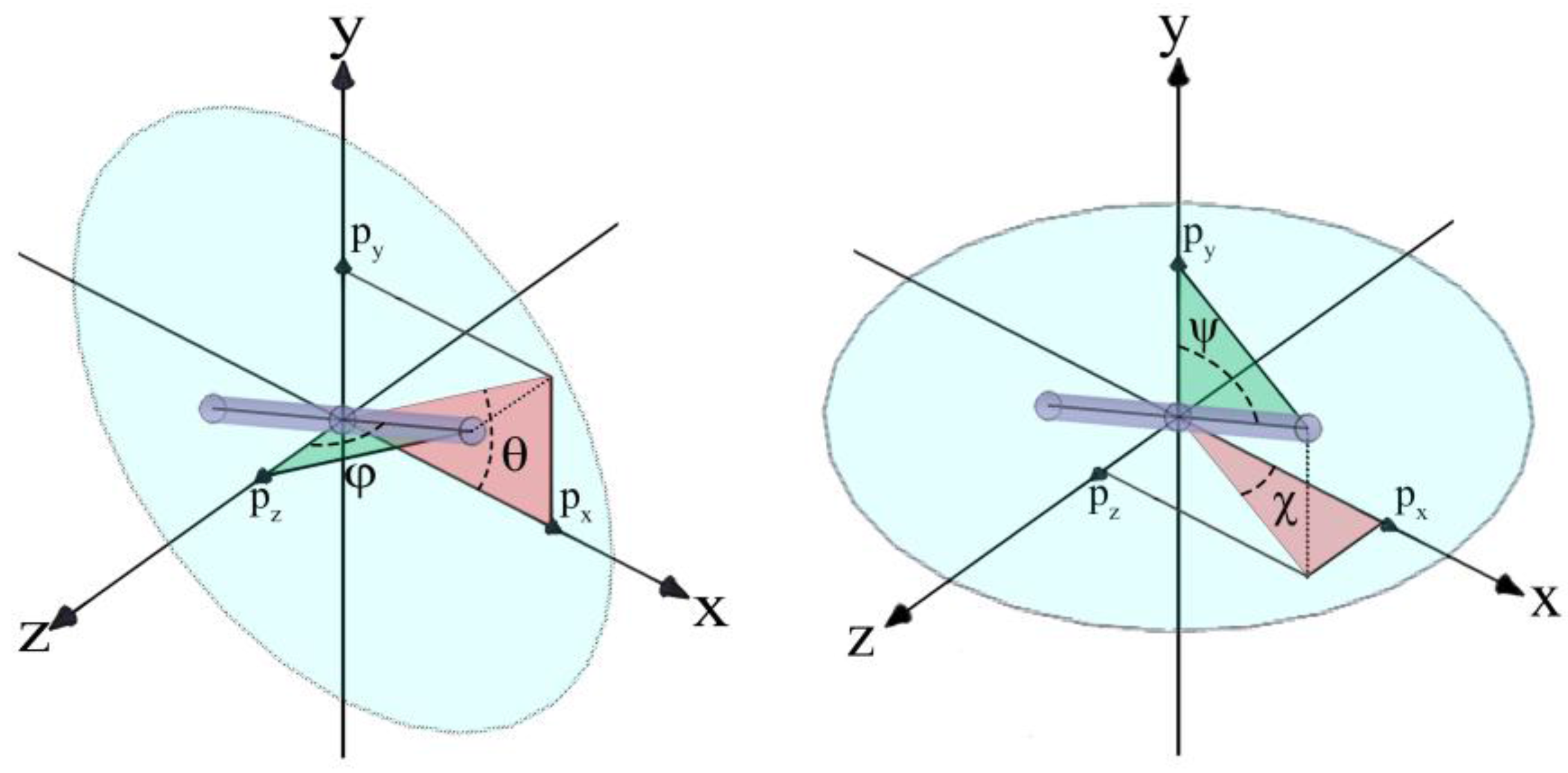
| θ | the angle between a rod’s principal axis and the flow direction on the xy-plane |
| ϕ | the angle between a rod’s principal axis and the vorticity direction (z) |
| χ | the angle between a rod’s principal axis and the flow direction on the xz-plane |
| ψ | the angle between a rod’s principal axis and the shear direction (y) |
| μ | solvent viscosity |
References
- Agarwal, U.; Dutta, A.; Mashelkar, R. Migration of macromolecules under flow: The physical origin and engineering implications. Chem. Eng. Sci. 1994, 49, 1693–1717. [Google Scholar] [CrossRef]
- Petrie, C.J. The rheology of fibre suspensions. J. NonNewton. Fluid Mech. 1999, 87, 369–402. [Google Scholar] [CrossRef]
- Sharma, V.; Park, K.; Srinivasarao, M. Colloidal dispersion of gold nanorods: Historical background, optical properties, seed-mediated synthesis, shape separation and self-assembly. Mater. Sci. Eng. R 2009, 65, 1–38. [Google Scholar] [CrossRef]
- Lee, G.; Cho, Y.-S.; Park, S.; Yi, G.-R. Synthesis and assembly of anisotropic nanoparticles. Korean J. Chem. Eng. 2011, 28, 1641–1650. [Google Scholar] [CrossRef]
- Berthet, H. Single and Collective Fiber Dynamics in Confined Microflows. Ph.D. Thesis, Université Pierre et Marie Curie-Paris VI, Paris, France, 2012. [Google Scholar]
- Barua, S.; Mitragotri, S. Synergistic targeting of cell membrane, cytoplasm, and nucleus of cancer cells using rod-shaped nanoparticles. ACS Nano 2013, 7, 9558–9570. [Google Scholar] [CrossRef] [PubMed]
- Monjezi, S.; Bhedani, B.; Palaniappan, M.B.; Jones, J.D.; Park, J. Computational studies of DNA separations in micro-fabricated devices: Review of general approaches and recent applications. Adv. Chem. Eng. Sci. 2017, 7, 362–393. [Google Scholar] [CrossRef]
- Hjerrild, N.E.; Taylor, R.A. Boosting solar energy conversion with nanofluids. Phys. Today 2017, 70, 40–45. [Google Scholar] [CrossRef]
- Schimpf, M.E.; Caldwell, K.; Giddings, J.C. Field-Flow Fractionation Handbook; John Wiley & Sons: Hoboken, NJ, USA, 2000. [Google Scholar]
- Gigault, J.; Cho, T.J.; MacCuspie, R.I.; Hackley, V.A. Gold nanorod separation and characterization by asymmetric-flow field flow fractionation with UV–Vis detection. Anal. Bioanal. Chem. 2013, 405, 1191–1202. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, T.M.; Liu, J.; Hackley, V.A. Fractionation and characterization of high aspect ratio gold nanorods using asymmetric-flow field flow fractionation and single particle inductively coupled plasma mass spectrometry. Chromatography 2015, 2, 422–435. [Google Scholar] [CrossRef]
- Alfi, M.; Park, J. Theoretical analysis of the local orientation effect and the lift-hyperlayer mode of rodlike particles in field-flow fractionation. J. Sep. Sci. 2014, 37, 876–883. [Google Scholar] [CrossRef] [PubMed]
- Park, J.; Mittal, A. An improved model for the steric-entropic effect on the retention of rod-like particles in field-flow fractionation: Discussion of aspect ratio-based separation. Chromatography 2015, 2, 472–487. [Google Scholar] [CrossRef]
- Jeffery, G.B. The motion of ellipsoidal particles immersed in a viscous fluid. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 1922, 102, 161–179. [Google Scholar] [CrossRef]
- Sundararajakumar, R.; Koch, D.L. Structure and properties of sheared fiber suspensions with mechanical contacts. J. NonNewton. Fluid Mech. 1997, 73, 205–239. [Google Scholar] [CrossRef]
- Stover, C.A.; Koch, D.L.; Cohen, C. Observations of fibre orientation in simple shear flow of semi-dilute suspensions. J. Fluid Mech. 1992, 238, 277–296. [Google Scholar] [CrossRef]
- Rahnama, M.; Koch, D.L.; Shaqfeh, E.S. The effect of hydrodynamic interactions on the orientation distribution in a fiber suspension subject to simple shear flow. Phys. Fluids 1995, 7, 487–506. [Google Scholar] [CrossRef]
- Leal, L.; Hinch, E. The effect of weak brownian rotations on particles in shear flow. J. Fluid Mech. 1971, 46, 685–703. [Google Scholar] [CrossRef]
- Einarsson, J.; Candelier, F.; Lundell, F.; Angilella, J.; Mehlig, B. Rotation of a spheroid in a simple shear at small reynolds number. Phys. Fluids 2015, 27, 063301. [Google Scholar] [CrossRef]
- Boeder, P. Über strömungsdoppelbrechung. Zeitschrift für Physik A Hadrons Nuclei 1932, 75, 258–281. [Google Scholar]
- Hijazi, A.; Zoaeter, M. Brownian dynamics simulations for rod-like particles in dilute flowing solution. Eur. Polym. J. 2002, 38, 2207–2211. [Google Scholar] [CrossRef]
- Chen, S.B.; Jiang, L. Orientation distribution in a dilute suspension of fibers subject to simple shear flow. Phys. Fluids 1999, 11, 2878–2890. [Google Scholar] [CrossRef]
- Asokan, K.; Ramamohan, T.; Kumaran, V. A novel approach to computing the orientation moments of spheroids in simple shear flow at arbitrary peclet number. Phys. Fluids 2002, 14, 75–84. [Google Scholar] [CrossRef]
- Park, J.; Butler, J.E. Inhomogeneous distribution of a rigid fibre undergoing rectilinear flow between parallel walls at high peclet numbers. J. Fluid Mech. 2009, 630, 267–298. [Google Scholar] [CrossRef]
- Park, J.; Bricker, J.M.; Butler, J.E. Cross-stream migration in dilute solutions of rigid polymers undergoing rectilinear flow near a wall. Phys. Rev. E 2007, 76, 040801. [Google Scholar] [CrossRef] [PubMed]
- Park, J. Dynamics of Suspensions of Rodlike Polymers with Hydrodynamic Interactions; University of Florida: Gainesville, FL, USA, 2009. [Google Scholar]
- Park, J.; Butler, J.E. Analysis of the migration of rigid polymers and nanorods in a rotating viscometric flow. Macromolecules 2010, 43, 2535–2543. [Google Scholar] [CrossRef]
- Hijazi, A.; Khater, A. Brownian dynamics simulations of rigid rod-like macromolecular particles flowing in bounded channels. Comput. Mater. Sci. 2001, 22, 279–290. [Google Scholar] [CrossRef]
- Hijazi, A.; Khater, A. Simulations of distribution functions for rod-like macromolecules in linear flow near solid surfaces. Comput. Mater. Sci. 2001, 20, 213–227. [Google Scholar] [CrossRef]
- Schiek, R.L.; Shaqfeh, E.S. A nonlocal theory for stress in bound, Brownian suspensions of slender, rigid fibres. J. Fluid Mech. 1995, 296, 271–324. [Google Scholar] [CrossRef]
- Schiek, R.L.; Shaqfeh, E.S. Cross-streamline migration of slender Brownian fibres in plane Poiseuille flow. J. Fluid Mech. 1997, 332, 23–39. [Google Scholar] [CrossRef]
- Nitsche, L.C.; Hinch, E. Shear-induced lateral migration of Brownian rigid rods in parabolic channel flow. J. Fluid Mech. 1997, 332, 1–21. [Google Scholar] [CrossRef]
- Stover, C.A.; Cohen, C. The motion of rodlike particles in the pressure-driven flow between two flat plates. Rheol. Acta 1990, 29, 192–203. [Google Scholar] [CrossRef]
- Holm, R.; Söderberg, D. Shear influence on fibre orientation. Rheol. Acta 2007, 46, 721–729. [Google Scholar] [CrossRef]
- Kaya, T.; Koser, H. Characterization of hydrodynamic surface interactions of Escherichia coli cell bodies in shear flow. Phys. Rev. Lett. 2009, 103, 138103. [Google Scholar] [CrossRef] [PubMed]
- Batchelor, G. Slender-body theory for particles of arbitrary cross-section in stokes flow. J. Fluid Mech. 1970, 44, 419–440. [Google Scholar] [CrossRef]
- Doi, M.; Edwards, S.F. The Theory of Polymer Dynamics; Oxford University Press: Oxford, UK, 1988. [Google Scholar]
- Larson, R.G. The Structure and Rheology of Complex Fluids; Oxford University Press: Oxford, UK, 1999. [Google Scholar]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids; Oxford University Press: Oxford, UK, 1989. [Google Scholar]
- Cobb, P.D.; Butler, J.E. Simulations of concentrated suspensions of rigid fibers: Relationship between short-time diffusivities and the long-time rotational diffusion. J. Chem. Phys. 2005, 123, 054908. [Google Scholar] [CrossRef] [PubMed]
- Vidal-Meza, M.P.; Zhou, R.; Barua, S.; Wang, C.; Park, J. Simulation of interstitial nanoparticle flow for development of tumor-on-a-chip device. In Proceedings of the 2016 COMSOL Conference, Boston, MA, USA, 5–7 October 2016. [Google Scholar]




© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Monjezi, S.; Jones, J.D.; Nelson, A.K.; Park, J. The Effect of Weak Confinement on the Orientation of Nanorods under Shear Flows. Nanomaterials 2018, 8, 130. https://doi.org/10.3390/nano8030130
Monjezi S, Jones JD, Nelson AK, Park J. The Effect of Weak Confinement on the Orientation of Nanorods under Shear Flows. Nanomaterials. 2018; 8(3):130. https://doi.org/10.3390/nano8030130
Chicago/Turabian StyleMonjezi, Saman, James D. Jones, Alyssa K. Nelson, and Joontaek Park. 2018. "The Effect of Weak Confinement on the Orientation of Nanorods under Shear Flows" Nanomaterials 8, no. 3: 130. https://doi.org/10.3390/nano8030130