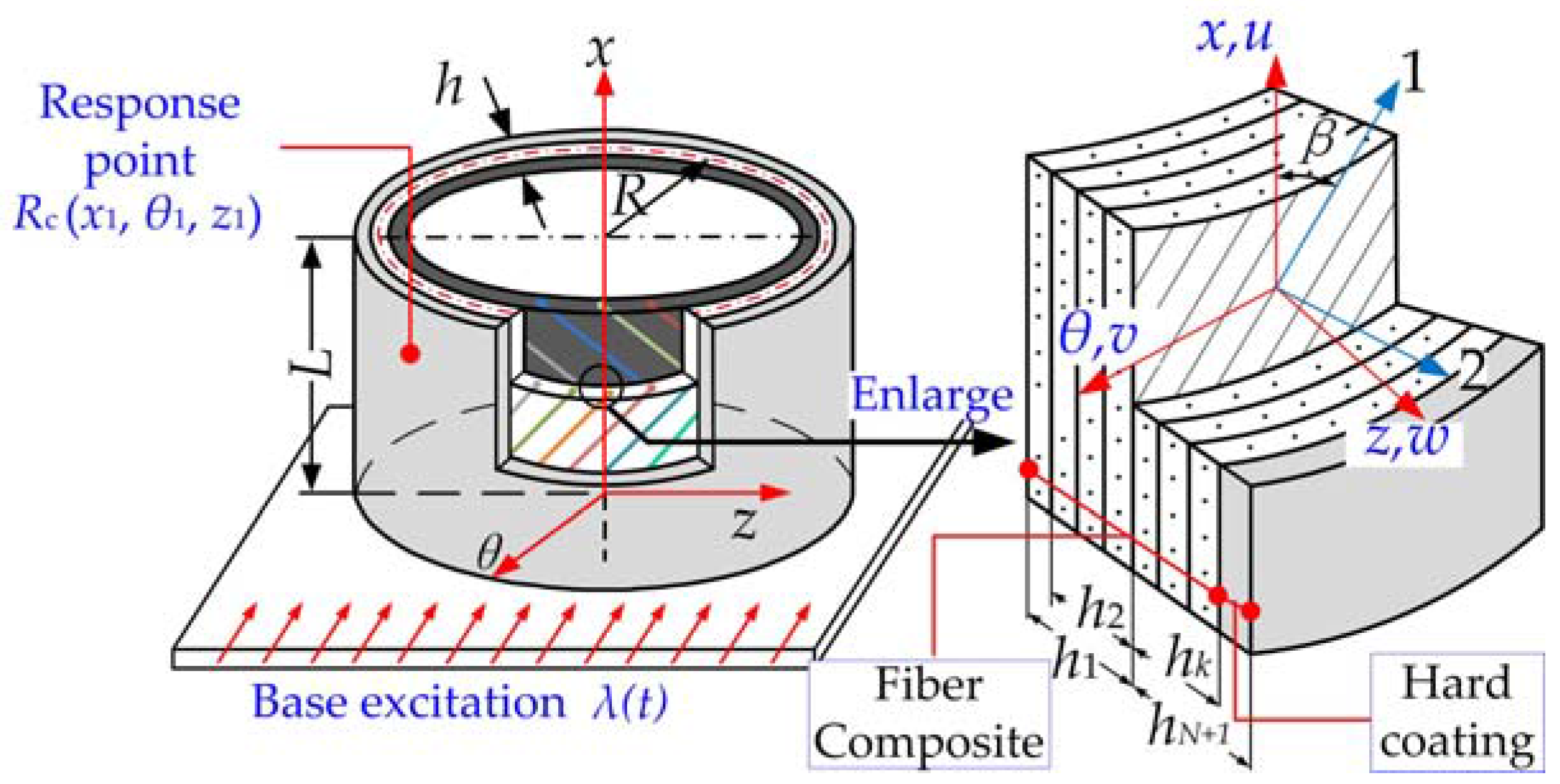
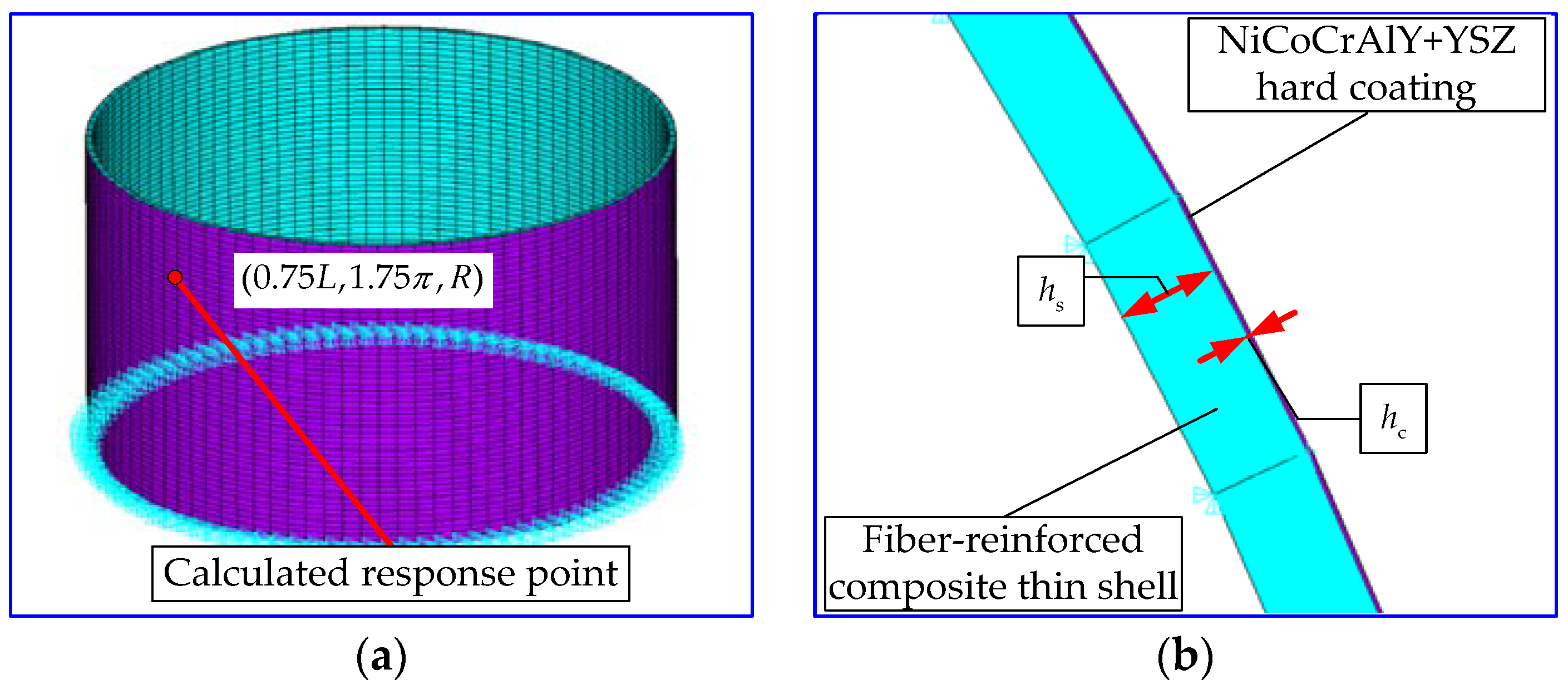
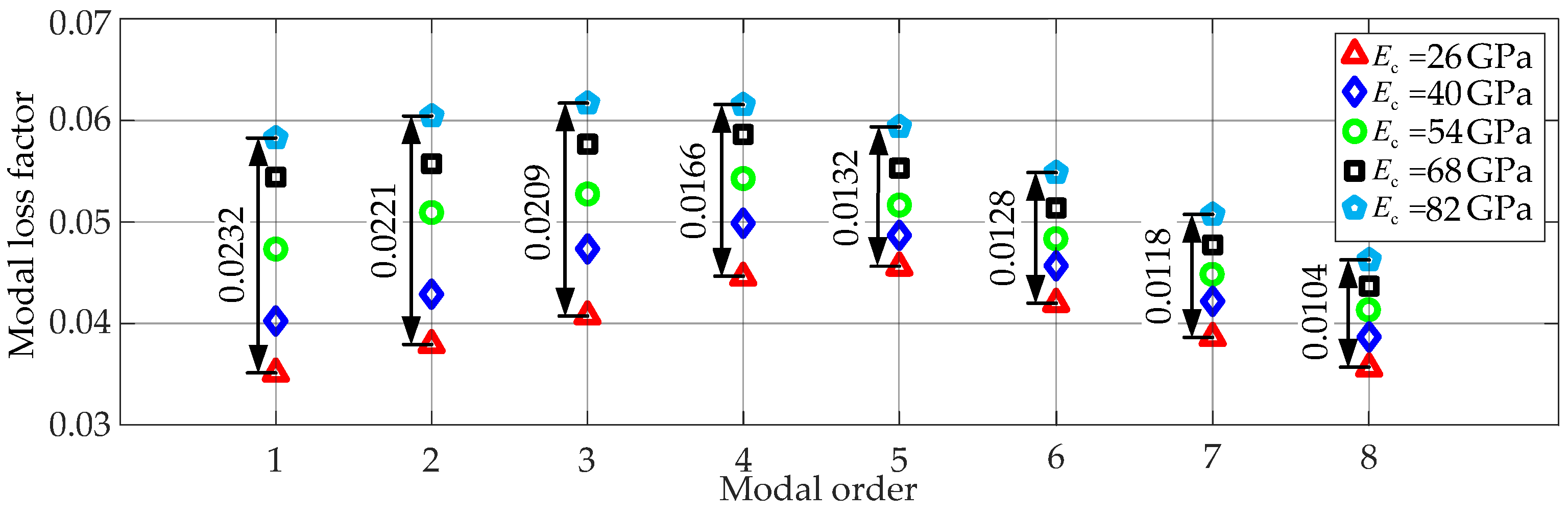
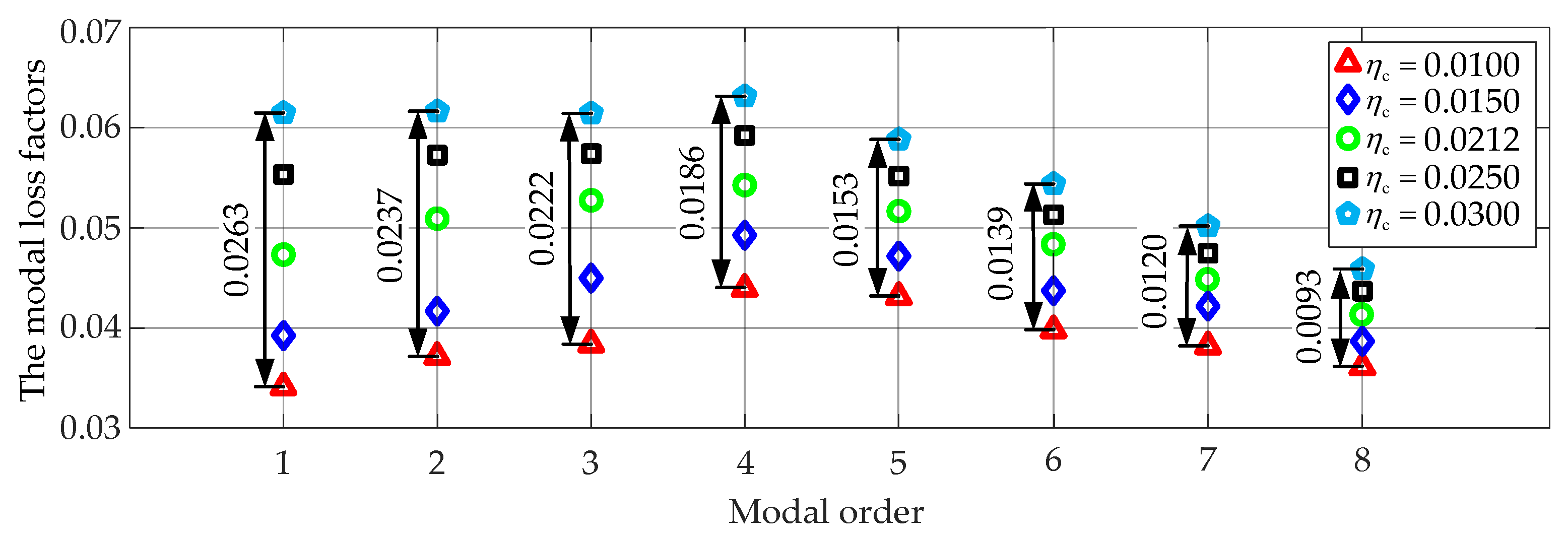
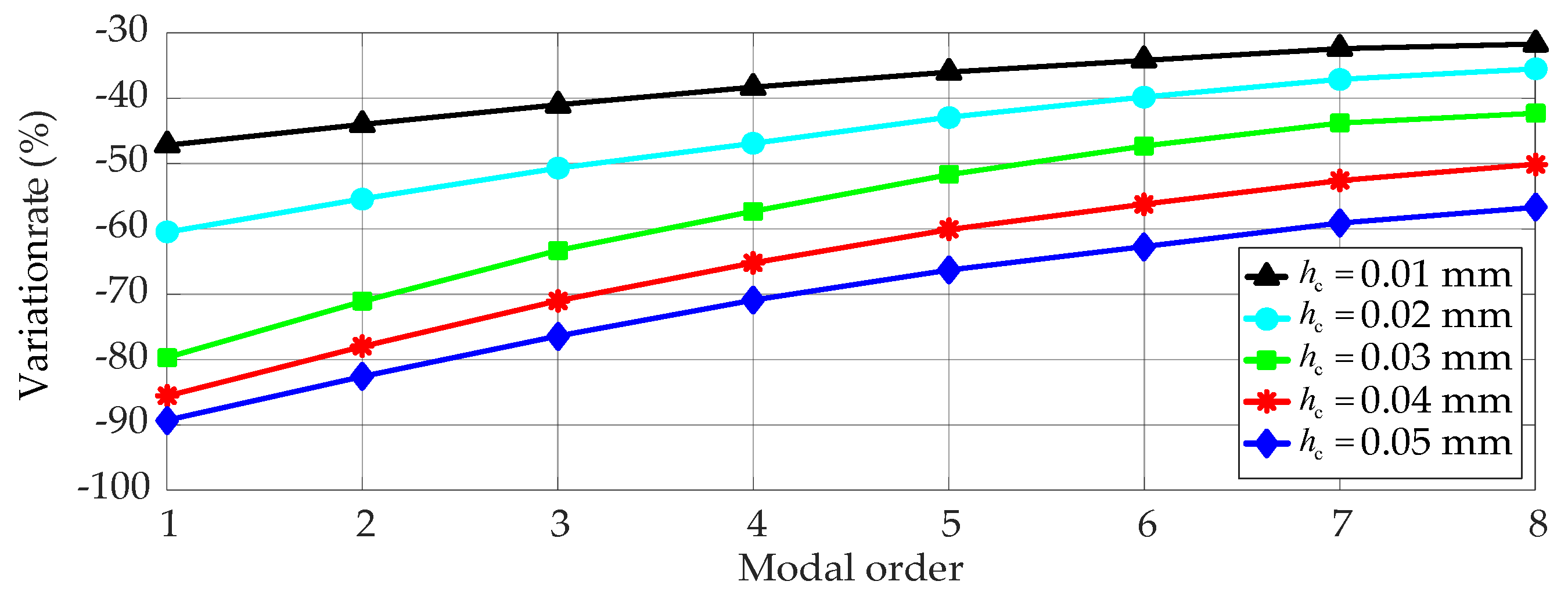
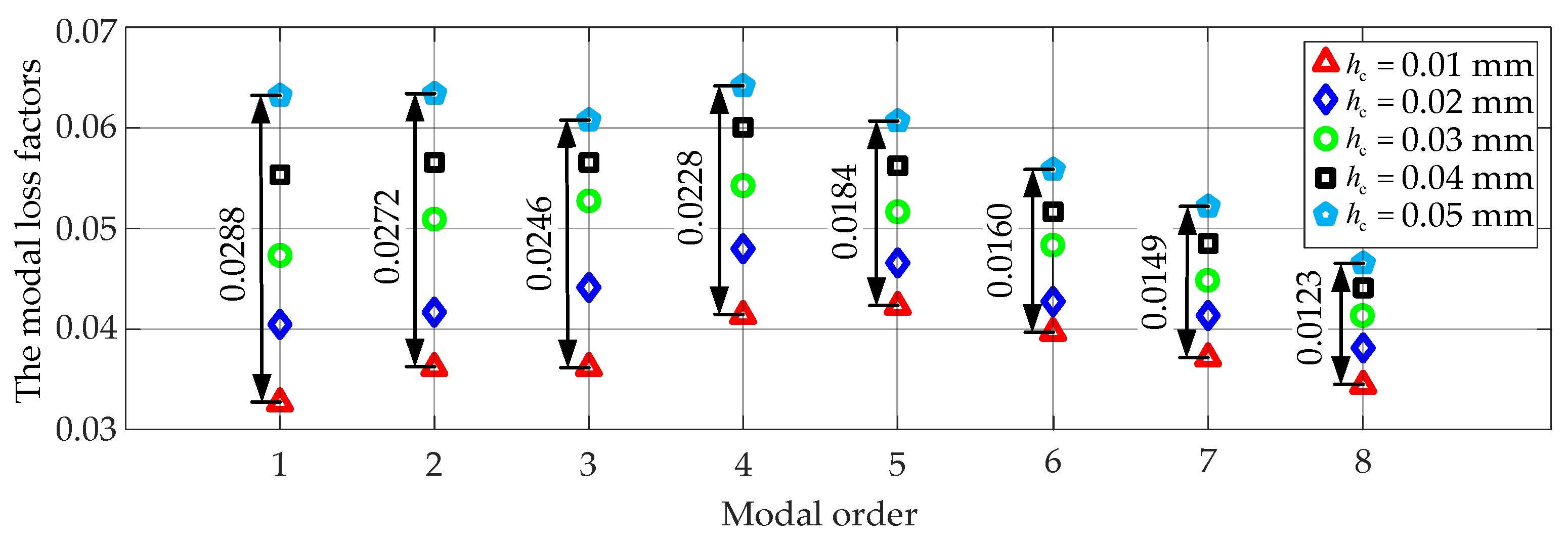
Due to the high strength to weight ratio and other superior performances, the fiber-reinforced composite thin shell has been increasingly used in a variety of engineering fields, such as composite casings in aircraft engines, pressure resistant composite shells in submarines, and high temperature resistant composite shells in rocket motor cases. As fiber-reinforced composite thin shell (FCTS) often works in complex environments, such as ones with vibration, noise, high pressure, and high-speed impact, some problems easily result such as high-level vibration amplitude, fatigue failure, fiber delamination, destructive damage, etc., which could lead to some disastrous accidents [
1,
2,
3,
4]. Therefore, it is of great scientific significance to study how to reduce the vibration of FCTS.
The hard coating is a kind of coating material manufactured using metals, ceramics, and mixtures, which is used as a surface treatment for anti-friction, anti-erosion, vibration reduction, and other engineering application fields [
5,
6,
7,
8]. In recent years, due to continuously reduced costs and more mature technologies, it has drawn increasing academic and engineering attention. If the hard coating material can be deposited on the surface of FCTS, then, through the internal frictions of coated particles and interface frictions of the coating and FCTS, the vibration energy of such composite shell structures can be transformed into heat energy, and the goal of reducing vibration of FCTS can be achieved.
At present, great efforts have been made to study vibration characteristics of thin-walled structures with hard coating, such as beams, plates, and shells, including the corresponding modeling and analysis techniques, vibration reduction design, evaluation methods, etc. For example, Green and Patsias [
9] proposed a friction model to calculate the response of a coated beam, and the variation of damping effectiveness for several vibration modes and a range of amplitudes were calculated. The results showed this model can capture the behaviors of the test piece well, after comparing with experimental results. Patsias et al. [
10] developed an experimental procedure that could accurately control the vibration amplitude, excitation frequency, and temperature so as to extract the damping parameter and elastic modulus of hard coating. Masti and Sainsbury [
11] established the corresponding formulas with finite element method and adaptive shell elements to study the vibration damping characteristics of the cylindrical shell that was partially deposited with viscoelastic coating. Ivancic, Blackwell and Palazotto [
12,
13] measured the natural characteristics of a titanium plate coated with magnesium aluminate spinel. They found that compared with the uncoated specimen, the frequency response of the hard coating composite plate was not symmetric about the resonance frequency, and the resonance frequency decreased with the increase of the applied force amplitude, which indicated the nonlinear stiffness characteristics, also called the “strain softening”. Sofiyev and Kuruoglu [
14,
15] conducted numerical analysis of torsional vibration and bucking of cylindrical and conical shells with functionally graded (FG) coating resting on Pasternak elastic foundations, and the basic equations were derived by Donnell’s theory and solved by the Galerkin method. Song et al. [
16] presented a strategy to solve the high-order vibration of the rotating thin shell based on the transfer matrix method, and the damping effects of hard coating on the natural characteristics were illustrated. Zhang and Li [
17] presented an analysis procedure, where the generalized differential quadrature (GDQ) method was applied to investigate the free vibration of the functionally graded conical shells with a variety of boundary conditions. Najafov et al. [
18] investigated the effect of thickness and material composition of the functionally graded coatings on the frequency-amplitude relationships of a truncated conical shell. Chen et al. [
19] analyzed the damping effect of the hard coating on the blades based on the constitutive model of the complex modulus. Dey and Mukhopadhyay et al. [
20] used a surrogate model (D-optimal design) approach to study the stochastic natural frequencies of laminated composite conical shells. The effects of twist angle, ply orientation, and material properties on the uncertainty quantification of the natural frequency was also considered. Sun et al. [
21,
22] considered the elastic constraints and the strain dependence to analyze the free vibration behavior of hard coating cylindrical metal shell and beam structures using the Rayleigh-Ritz method, and the correctness of the created models were verified by the comparison between the calculation and experiment. Naskar and Mukhopadhyay et al. [
23] presented a novel concept of stochastic representative volume element (SRVE) to study the natural frequencies of thin-walled laminated composite beams with spatially varying matrix cracking damage in a multi-scale framework through a stochastic approach. Zhang et al. [
24] proposed a four-node composite cylindrical shell model to solve the nonlinear vibration problems efficiently by combining the Love’s first approximation theory with finite element method, and the influence of the strain dependence of the coating material on the complex stiffness matrix was considered.
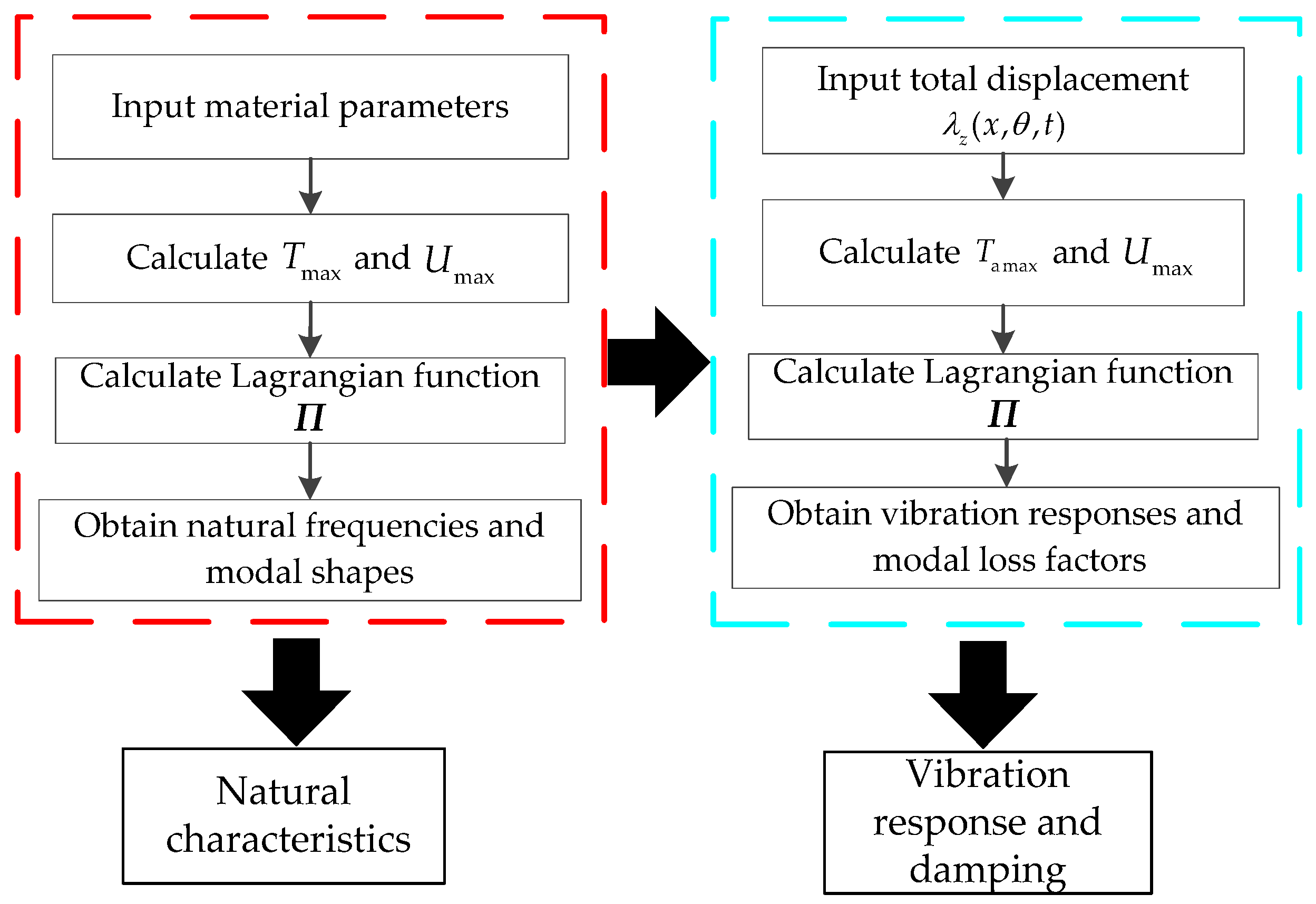
From the above literature reviews, it is clear that the vibration modeling techniques are still rare for fiber-reinforced composite shell structures coated with hard coating material, and the influences of hard coating on vibration characteristics of FCTS are not well researched. Therefore, this research tries to establish an appropriate theoretical model to study the influence of the elastic modulus, loss factor, and thickness of hard coating on the vibration characteristics of the composite shell. The paper is organized as follows. Firstly, the theoretical model of the hard coating FCTS is established by using Hamilton’s principle in conjunction with the classical laminated shell theory, Love’s first approximation theory, the Rayleigh-Ritz method, and strain energy method; the natural frequencies, modal shapes, vibration responses, and modal loss factors of the hard coating composite shell can be obtained in
Section 2. Then, the corresponding analysis procedure is summarized in
Section 3, and a case study is carried out to verify the developed theoretical model in
Section 4. Finally, in
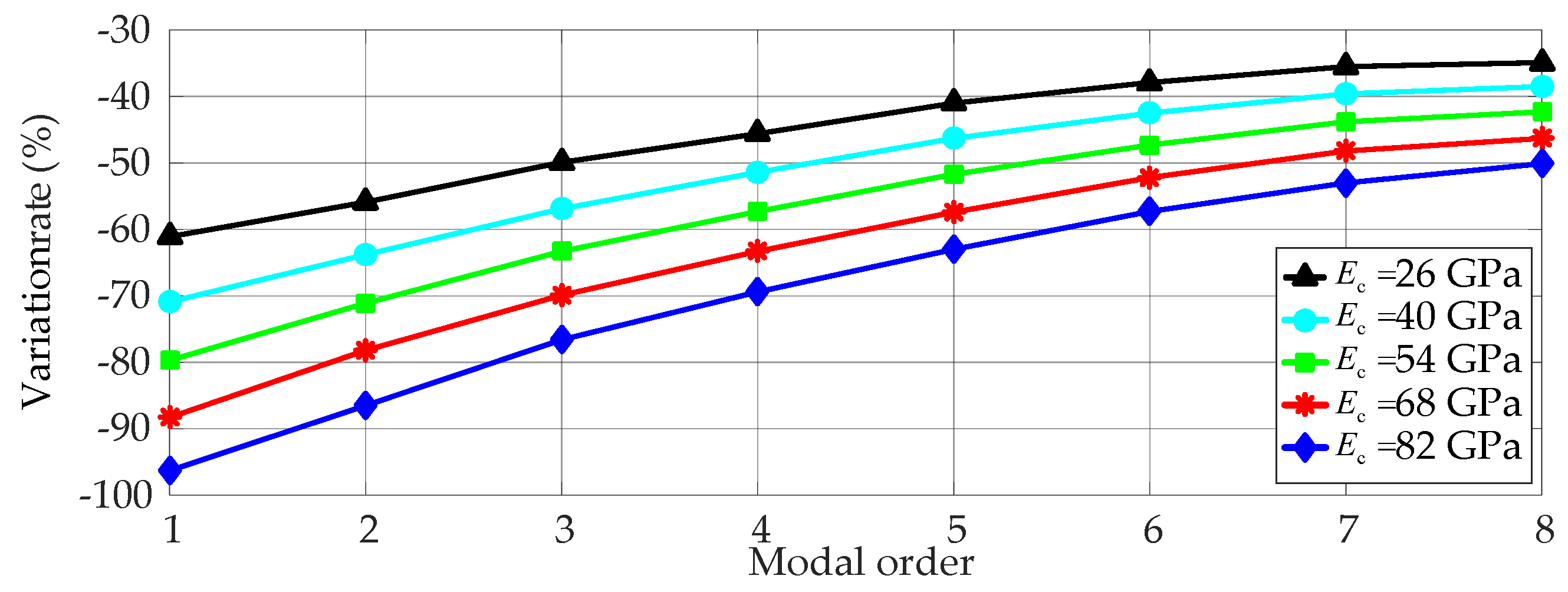
Section 5, the influence of hard coating parameters on the vibration behavior of the composite shell is discussed and some conclusions are drawn in
Section 6. This research can provide a dynamic modeling service for FCTS with hard coating and also provide an important reference for vibration reduction design of hard coating applied on the composite shell structures.