Electrodynamics of s-Wave Superconductors Using First-Order Formalism

Abstract

In this paper we give a derivation of a system of equations which generalize the London brothers and Ginzburg–Landau systems of equations, to describe the electrodynamics of s-wave superconductors. First, we consider a relativistically covariant theory in terms of gauge four-vector electromagnetic potential and scalar complex field. We use the first-order formalism to obtain the supplemented Maxwell equations for gauge-invariant electric, magnetic, four-vector fields and the modulus of the superconducting order parameter. The new four-vector field appears in some of the equations as a gauge-invariant super-current, and in other ones, while gauge invariant, as a four-vector electromagnetic potential. This dual contribution of the new four-vector field is the basis of the electrodynamics of superconductors. We focus on the system of equations with time-independent fields. The qualitative analysis shows that the applied magnetic field suppresses the superconductivity, while the applied electric field impacts oppositely, supporting it. Secondly, we consider time-dependent non-relativistic Ginzburg–Landau theory.

1. Introduction

The earliest study of the electrodynamics of s-wave superconductors is attributed to the London brothers [1]. They supplemented the Maxwell system of equations with a set of equations to explain the electrodynamics of superconductors, and more particularly, the Meissner–Ochsenfeld effect. The generalized Maxwell–London equations are discussed in [2] without account for the spontaneous breakdown of the charge symmetry. The quantum-mechanical foundation of these equations was discussed in phenomenological Ginzburg–Landau theory [3].

There is also an attempt to explain the Meissner–Ochsenfeld effect in a purely classical way [4] and superconductivity as a limiting phenomena [5]. In [6], a microscopic justification is given that a superconductor may have an electric field in its interior. The phenomenon is considered as a consequence of hole superconductivity [7] (see also [8]).

The main purpose of the present paper is to give a derivation of a system of equations which generalize the London brothers’ and Ginzburg–Landau systems of equations. In the Londons’ theory, the amplitude of the order parameter is not included, which does not permit the different impact of the applied electric and magnetic fields to be obtained. The Ginzburg–Landau theory does not consider the electric field.

The Londons’ system of equations is relativistic covariant [1]. We want to generalize this system, and this is why we first consider a relativistically covariant theory in terms of gauge four-vector electromagnetic potentials and scalar complex field.

The results of the relativistic theory are very important guidance for the non-relativistic one, while the non-relativistic theory has more options.

We focus on the system of equations with time-independent fields. The qualitative analysis shows that the applied magnetic field suppresses the superconductivity, while the applied electric field impacts oppositely, supporting it.

The system of equations is derived from a relativistically non-covariant theory, and shows that the effect of the applied electric field depends on the direction of the field.

The paper is organized as follows: In Section 2, we derive the system of equations to describe the electrodynamics of s-wave superconductors from relativistically covariant theory of superconductivity. For the case when density of Cooper pairs is a constant, the system of equations reduces to the London brothers’ equations. The system of equations derived in the present paper includes an equation for the density of Cooper pairs which shows the different impacts of applied electric and magnetic fields on superconductivity. In Section 3, we use the same technique of calculations to consider time-dependent relativistically non-covariant Ginzburg–Landau theory. The main results are reported and commented in Section 4.

2. Relativistically Covariant Theory of Superconductivity

We begin with a well-known field-theory action [9] for a relativistically covariant theory of superconductivity

$$\begin{array}{ccc}\hfill S& =& \int d^{4}x\left[-\frac{1}{4}\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)\left({\partial }^{\lambda }{A}^{\nu }-{\partial }^{\nu }{A}^{\lambda }\right)\right.\hfill \\ & +& \left({\partial }_{\lambda }-i{e}^{*}{A}_{\lambda }\right){\psi }^{*}\left({\partial }^{\lambda }+i{e}^{*}{A}^{\lambda }\right)\psi \hfill \\ & +& \left.\alpha {\psi }^{*}\psi -\frac{g}{2}{\left({\psi }^{*}\psi \right)}^2\right]\hfill \end{array}$$

(1)

written in terms of gauge four-vector electromagnetic potential “A” and complex scalar field “$\psi$”, the superconducting order parameter. The parameter

$$\alpha ={\alpha }_0(T_c-T),$$

(2)

where T is the temperature and $T_c$ is the critical temperature, is positive when the system is a superconductor. We use the standard notations for relativistically covariant systems: $x=(x^0,x^1,x^2,x^3)=(x_0,-x_1,-x_2,-x_3)=(\upsilon t,x,y,z)$, ${\upsilon }^{-2}=\mu \epsilon$, where $\mu$ is the magnetic permeability and $\epsilon$ is the electric permittivity of the superconductor. We assume that these parameters do not change their values when the system undergoes normal-to-superconductor transition. The action (1) is invariant under the gauge transformations

$$\begin{array}{ccc}\hfill {\psi }^{\prime }\left(x\right)& =& exp\left[i{e}^{*}\varphi \left(x\right)\right]\psi \hfill \\ \hfill A_{\nu }^{\prime }& =& A_{\nu }-{\partial }_{\nu }\varphi \left(x\right),\hfill \end{array}$$

(3)

where $\varphi \left(x\right)$ is a real function.

We represent the order parameter $\psi \left(x\right)$ in the form

$$\psi \left(x\right)=\rho \left(x\right)exp\left[i{e}^{*}\theta \left(x\right)\right],$$

(4)

where $\rho \left(x\right)=\left|\psi \right(x\left)\right|$ is a gauge invariant, and the gauge transformation of $\theta \left(x\right)$ is

$${\theta }^{\prime }\left(x\right)=\theta \left(x\right)+\varphi \left(x\right).$$

(5)

The action (1), rewritten in terms of $\rho$ and $\theta$, adopts the form

$$\begin{array}{ccc}\hfill S& =& \int d^{4}x\left[-\frac{1}{4}\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)\left({\partial }^{\lambda }{A}^{\nu }-{\partial }^{\nu }{A}^{\lambda }\right)\right.\hfill \\ & +& e^{*2}{\rho }^2\left({\partial }_{\lambda }\theta +A_{\lambda }\right)\left({\partial }^{\lambda }\theta +A^{\lambda }\right)\hfill \\ & +& \left.{\partial }_{\lambda }\rho {\partial }^{\lambda }\rho +\alpha {\rho }^2-\frac{g}{2}{\rho }^4\right].\hfill \end{array}$$

(6)

It is convenient to use the action in the first-order formalism

$$\begin{array}{ccc}\hfill S& =& \int d^{4}x\left\{-\frac{1}{2}\left[\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)F^{\lambda \nu }-\frac{1}{2}{F}_{\lambda \nu }{F}^{\lambda \nu }\right]\right.\hfill \\ & +& 2e^{*2}{\rho }^2\left[\left({\partial }_{\lambda }\theta +A_{\lambda }\right)Q^{\lambda }-\frac{1}{2}{Q}_{\lambda }{Q}^{\lambda }\right]\hfill \\ & +& \left.{\partial }_{\lambda }\rho {\partial }^{\lambda }\rho +\alpha {\rho }^2-\frac{g}{2}{\rho }^4\right\},\hfill \end{array}$$

(7)

where gauge potential $A^{\lambda }$, phase $\theta$, gauge invariant antisymmetric field $F^{\lambda \nu }=-F^{\nu \lambda }$, gauge invariant four-vector field $Q^{\lambda }$ and gauge invariant scalar field $\rho$ are assumed to be independent degrees of freedom in the theory.

To derive the system of Maxwell equations for s-wave superconductors, we vary the action (7) with respect to $F^{\lambda \nu }$, $Q^{\lambda }$, $A^{\lambda }$, $\theta$, and $\rho$. The resulting system of equations reads:

$$\begin{array}{c}{F}_{\lambda \nu }=\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)\hfill \end{array}$$

(8)

$$\begin{array}{c}{Q}_{\lambda }={\partial }_{\lambda }\theta +A_{\lambda }\hfill \end{array}$$

(9)

$$\begin{array}{c}{\partial }_{\lambda }{F}^{\lambda \nu }+2e^{*2}{\rho }^2{Q}^{\nu }=0\hfill \end{array}$$

(10)

$$\begin{array}{c}{\partial }_{\lambda }\left({\rho }^2{Q}^{\lambda }\right)=0\hfill \end{array}$$

(11)

$$\begin{array}{c}{\partial }_{\lambda }{\partial }^{\lambda }\rho -\alpha \rho +g{\rho }^3=2e^{*2}\rho \left[\left({\partial }_{\lambda }\theta +A_{\lambda }\right)Q^{\lambda }-\frac{1}{2}{Q}_{\lambda }{Q}^{\lambda }\right]\hfill \end{array}$$

(12)

If we set in Equations (10)–(12) the expressions for $F_{\lambda \nu }$ and $Q^{\lambda }$ from Equations (8) and (9), we obtain the equations of motion following from the action (6). This means that theories with actions (6) and (7) are equivalent.

Alternatively, one eliminates the gauge fields $A_{\lambda }$ and $\theta$ from Equations (8)–(12) to obtain the system of equations for the gauge invariant fields $F^{\lambda \nu }$, $Q^{\lambda }$ and $\rho$:

$$\begin{array}{c}{\partial }_{\lambda }{F}_{\nu \delta }+{\partial }_{\nu }{F}_{\delta \lambda }+{\partial }_{\delta }{F}_{\lambda \nu }=0\hfill \end{array}$$

(13)

$$\begin{array}{c}{\partial }_{\lambda }{F}^{\lambda \nu }+2e^{*2}{\rho }^2{Q}^{\nu }=0\hfill \end{array}$$

(14)

$$\begin{array}{c}{\partial }_{\lambda }\left({\rho }^2{Q}^{\lambda }\right)=0\hfill \end{array}$$

(15)

$$\begin{array}{c}{\partial }_{\lambda }{Q}_{\nu }-{\partial }_{\nu }{Q}_{\lambda }=F_{\lambda \nu }\hfill \end{array}$$

(16)

$$\begin{array}{c}{\partial }_{\lambda }{\partial }^{\lambda }\rho -\alpha \rho +g{\rho }^3-e^{*2}\rho Q_{\lambda }{Q}^{\lambda }=0.\hfill \end{array}$$

(17)

Equation (13) follows from Equation (8), while Equation (16) from Equation (9).

Straightforward calculations show that Equation (15) can be obtained from Equation (14) and Equation (13) from Equation (16). The system of independent equations is:

$$\begin{array}{c}{\partial }_{\lambda }{F}^{\lambda \nu }+2e^{*2}{\rho }^2{Q}^{\nu }=0\hfill \end{array}$$

(18)

$$\begin{array}{c}{\partial }_{\lambda }{Q}_{\nu }-{\partial }_{\nu }{Q}_{\lambda }=F_{\lambda \nu }\hfill \end{array}$$

(19)

$$\begin{array}{c}{\partial }_{\lambda }{\partial }^{\lambda }\rho -\alpha \rho +g{\rho }^3-e^{*2}\rho Q_{\lambda }{Q}^{\lambda }=0.\hfill \end{array}$$

(20)

We construct the antisymmetric tensor $F_{\lambda \nu }$ by means of the electric $\mathbf{E}$ and magnetic $\mathbf{B}$ fields in a standard way: $(F_{01},F_{02},F_{03})=\mathbf{E}/\upsilon$, $(F_{32},F_{13},F_{21})=\mathbf{B}$ and $(Q^0,Q^1,Q^2,Q^3)=(Q/\upsilon ,\mathbf{Q})$. In terms of $\mathbf{E},\mathbf{B},\mathbf{Q}$, and Q, the system of equations which describes the electrodynamics of s-wave superconductors is:

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{B}=\mu \epsilon \frac{\partial \mathbf{E}}{\partial t}-2e^{*2}{\rho }^2\mathbf{Q}\hfill \end{array}$$

(21)

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{Q}=\mathbf{B}\hfill \end{array}$$

(22)

$$\begin{array}{c}\overrightarrow{\nabla }·\mathbf{E}=-2e^{*2}{\rho }^{2}Q\hfill \end{array}$$

(23)

$$\begin{array}{c}\overrightarrow{\nabla }Q+\frac{\partial \mathbf{Q}}{\partial t}=-\mathbf{E}\hfill \end{array}$$

(24)

$$\begin{array}{c}\mu \epsilon \frac{{\partial }^2\rho }{\partial t^2}-\Delta \rho -\alpha \rho +g{\rho }^3-e^{*2}\rho \left[\mu \epsilon Q^2-{\mathbf{Q}}^2\right]=0.\hfill \end{array}$$

(25)

It is important to stress that the gauge-invariant vector $\mathbf{Q}$ and scalar Q fields take part in Equations (22) and (24) as a magnetic vector and electric scalar potentials, while in Equation (21) $(-2e^{*2}{\rho }^2\mathbf{Q})$ is a supercurrent and in Equation (23) $(-2e^{*2}{\rho }^{2}Q)$ is a density of superconducting quasi-particles. This dual contribution of the new fields is the basis of the electrodynamics of superconductors.

We focus on the system of equations with time-independent fields:

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{B}=-2e^{*2}{\rho }^2\mathbf{Q}\hfill \end{array}$$

(26)

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{Q}=\mathbf{B}\hfill \end{array}$$

(27)

$$\begin{array}{c}\overrightarrow{\nabla }·\mathbf{E}=-2e^{*2}{\rho }^{2}Q\hfill \end{array}$$

(28)

$$\begin{array}{c}\overrightarrow{\nabla }Q=-\mathbf{E}\hfill \end{array}$$

(29)

$$\begin{array}{c}\Delta \rho +\alpha \rho -g{\rho }^3+e^{*2}\rho \left[\mu \epsilon Q^2-{\mathbf{Q}}^2\right]=0.\hfill \end{array}$$

(30)

To compare our result with the London equations [1], we assume that deep inside the superconductor $\mathbf{Q}$ and Q are zero, while $\rho ={\rho }_0$ is a constant determined from the equation $\alpha {\rho }_0-g{\rho }_0^3=0$, which follows from Equation (30). The gauge transformation (5) of the phase of the order parameter $\theta$ implies that one can impose the gauge fixing condition $\theta =0$. In that case, the gauge-invariant vector is equal to the vector potential $\mathbf{Q}=\mathbf{A}$, the gauge invariant scalar field is equal to the scalar potential $Q=A^0$, and the system of Equations (26)–(29) adopts the form

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{B}=-2e^{*2}{\rho }_0^2\mathbf{A}\hfill \end{array}$$

(31)

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{A}=\mathbf{B}\hfill \end{array}$$

(32)

$$\begin{array}{c}\overrightarrow{\nabla }·\mathbf{E}=-2e^{*2}{\rho }_0^2{A}^0\hfill \end{array}$$

(33)

$$\begin{array}{c}\overrightarrow{\nabla }{A}^0=-\mathbf{E}.\hfill \end{array}$$

(34)

With the London brothers’ postulates in mind

$$\begin{array}{c}\mathbf{J}/c=-2e^{*2}{\rho }_0^2\mathbf{A}\hfill \end{array}$$

(35)

$$\begin{array}{c}\rho =-2e^{*2}{\rho }_0^2{A}^0,\hfill \end{array}$$

(36)

we arrived at London equations [1] .

Taking the curl of (31), using Equation (32) and the identity $\overrightarrow{\nabla }·\mathbf{B}=0$, which follows from this equation, we obtain

$$\Delta \mathbf{B}=\frac{1}{{\lambda }_L^2}\mathbf{B},$$

(37)

where

$${\lambda }_L=\sqrt{g/(2e^{*2}\alpha )}.$$

(38)

Taking the divergence of (34) and using the Equation (33) we obtain

$$\Delta \mathbf{E}=\frac{1}{{\lambda }_L^2}\mathbf{E}.$$

(39)

Equations (37) and (39) imply that an electric field penetrates a distance ${\lambda }_L$ as a magnetic field does [10].

This approximation is very rough and does not account for the last term in Equation (30), which is responsible for the different impact of applied electric and magnetic fields on the superconductivity. If we apply magnetic field ($\mathbf{E}=0,Q=0$), the qualitative analysis of Equation (30) shows that the magnetic vector potential effectively decreases the $\alpha$ parameter, $\alpha \to \alpha -e^{*2}<{\mathbf{Q}}^2>$, where $<{\mathbf{Q}}^2>$ is some average value. Therefore, the Ginzburg–Landau coherence length increases (see Appendix A), which means that applied magnetic field destroys superconductivity. On the other hand, when the electric field is applied ($\mathbf{B}=0,\mathbf{Q}=0)$, the electric scalar potential effectively increases the $\alpha$ parameter $\alpha \to \alpha +e^{*2}\mu \epsilon <Q^2>$. 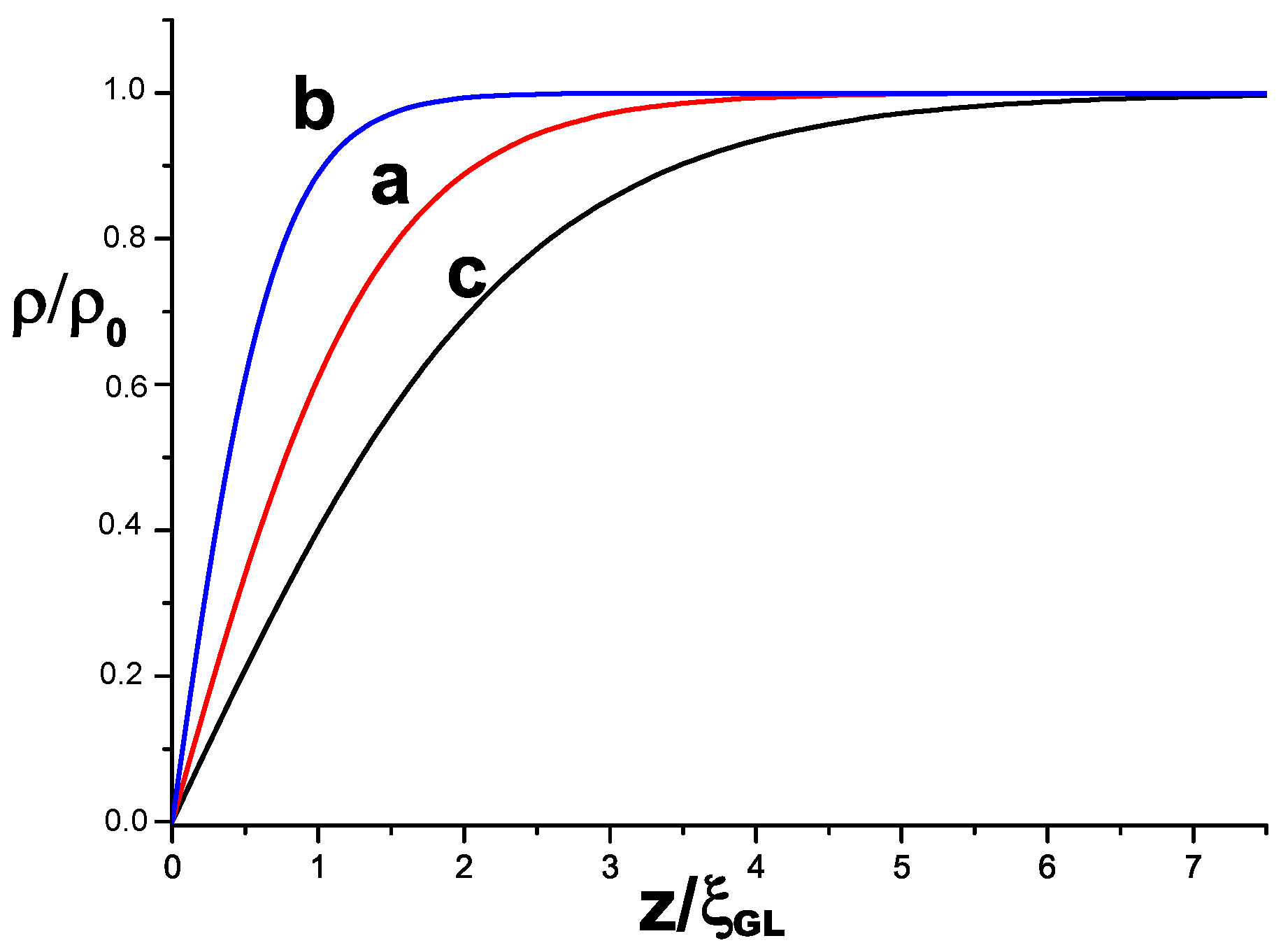Hence, the Ginzburg–Landau coherence length decreases. This qualitative analysis permits us to formulate the hypotheses that by applying electric field at very low temperature one increases the critical magnetic field. This result is experimentally testable.

3. Time-Dependent Ginzburg–Landau Theory

A number of authors have discussed the non-relativistic time-dependent generalization of the Ginzburg–Landau theory [11,12,13,14,15,16]. We investigate a model with field-theory action [13,15,16]

$$\begin{array}{ccc}\hfill S& =& \int d^{4}x\left[-\frac{1}{4}\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)\left({\partial }^{\lambda }{A}^{\nu }-{\partial }^{\nu }{A}^{\lambda }\right)\right.\hfill \\ & +& \frac{1}{D}{\psi }^{*}\left(i{\partial }_t-e^{*}\phi \right)\psi \hfill \\ & -& \frac{1}{2m^{*}}\left({\partial }_k-i{e}^{*}{A}_k\right){\psi }^{*}\left({\partial }_k+i{e}^{*}{A}_k\right)\psi \hfill \\ & +& \left.\alpha {\psi }^{*}\psi -\frac{g}{2}{\left({\psi }^{*}\psi \right)}^2\right],\hfill \end{array}$$

(40)

where $\phi =\upsilon A_0$ is the electric scalar potential, with gauge transformation (3) ${\phi }^{\prime }=\phi -{\partial }_t\varphi$, D is the normal-state diffusion constant [16], and ($e^{*},m^{*}$) are effective charge and mass of superconducting quasi-particles. The index k runs $k=x,y,z$.

We follow the same procedure to derive the system of equations which describe the electrodynamics of s-wave superconductors. We represent the order parameter $\psi$ by means of modulus and phase (4), and write the field-theory action, in the first-order formalism, in the form

$$\begin{array}{ccc}\hfill S& =& \int d^{4}x\left\{-\frac{1}{2}\left[\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)F^{\lambda \nu }-\frac{1}{2}{F}_{\lambda \nu }{F}^{\lambda \nu }\right]\right.\hfill \\ & -& \frac{e^{*}}{D}{\rho }^2\left(\phi +{\partial }_t\theta \right)\hfill \\ & -& \frac{e^{*2}}{m^{*}}{\rho }^2\left[\left({\partial }_k\theta +A_k\right)Q_k-\frac{1}{2}{Q}_k{Q}_k\right]\hfill \\ & -& \left.\frac{1}{2m^{*}}{\partial }_k\rho {\partial }_k\rho +\alpha {\rho }^2-\frac{g}{2}{\rho }^4\right\}.\hfill \end{array}$$

(41)

It is important to stress that $Q_k$ is a three-component vector field and $k=x,y,z$. Using a variational principle, we obtain the system of equations

$$\begin{array}{c}{F}_{\lambda \nu }=\left({\partial }_{\lambda }{A}_{\nu }-{\partial }_{\nu }{A}_{\lambda }\right)\hfill \end{array}$$

(42)

$$\begin{array}{c}{Q}_k={\partial }_k\theta +A_k\hfill \end{array}$$

(43)

$$\begin{array}{c}{\partial }_{\lambda }{F^k}_{\lambda }+\frac{e^{*2}}{m^{*}}{\rho }^2{Q}_k=0\hfill \end{array}$$

(44)

$$\begin{array}{c}{\partial }_k{F}_{0k}-\frac{\upsilon e^{*}}{D}{\rho }^2=0\hfill \end{array}$$

(45)

$$\begin{array}{c}{\partial }_t{\rho }^2+\frac{D}{m^{*}}{\partial }_k\left({\rho }^2{Q}_k\right)=0\hfill \end{array}$$

(46)

$$\begin{array}{c}\frac{1}{2m*}\Delta \rho +\alpha \rho -g{\rho }^3-\frac{e^{*}}{D}\rho \left(\phi +{\partial }_t\theta \right)\hfill \\ =\frac{e^{*2}}{m^{*}}\rho \left[\left({\partial }_k\theta +A_k\right)Q_k-\frac{1}{2}{Q}_k{Q}_k\right].\hfill \end{array}$$

(47)

We supplement the system of Equations (42)–(47) with Equation (48):

$$Q={\partial }_t\theta +\phi ,$$

(48)

which is a definition of the new gauge-invariant field Q. In the same way, starting from the system of Equations (42)–(48), we arrive at the Maxwell equations for superconductors in a non-relativistic theory.

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{B}=\mu \epsilon \frac{\partial \mathbf{E}}{\partial t}-\frac{e^{*2}}{m^{*}}{\rho }^2\mathbf{Q}\hfill \end{array}$$

(49)

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{Q}=\mathbf{B}\hfill \end{array}$$

(50)

$$\begin{array}{c}\overrightarrow{\nabla }·\mathbf{E}=\frac{\mu \epsilon e^{*}}{D}{\rho }^2\hfill \end{array}$$

(51)

$$\begin{array}{c}\overrightarrow{\nabla }Q+\frac{\partial \mathbf{Q}}{\partial t}=-\mathbf{E}\hfill \end{array}$$

(52)

$$\begin{array}{c}\frac{1}{2m^{*}}\Delta \rho +\alpha \rho -g{\rho }^3-\frac{e^{*}}{D}\rho Q-\frac{e^{*}}{2m^{*}}\rho {\mathbf{Q}}^2=0.\hfill \end{array}$$

(53)

The system of equations for time-independent fields is:

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{B}=-\frac{e^{*2}}{m^{*}}{\rho }^2\mathbf{Q}\hfill \end{array}$$

(54)

$$\begin{array}{c}\overrightarrow{\nabla }\times \mathbf{Q}=\mathbf{B}\hfill \end{array}$$

(55)

$$\begin{array}{c}\overrightarrow{\nabla }·\mathbf{E}=\frac{\mu \epsilon e^{*}}{D}{\rho }^2\hfill \end{array}$$

(56)

$$\begin{array}{c}\overrightarrow{\nabla }Q=-\mathbf{E}\hfill \end{array}$$

(57)

$$\begin{array}{c}\frac{1}{2m^{*}}\Delta \rho +\alpha \rho -g{\rho }^3-\frac{e^{*}}{D}\rho Q-\frac{e^{*}}{2m^{*}}\rho {\mathbf{Q}}^2=0.\hfill \end{array}$$

(58)

There are two important differences between Equations in relativistic theory (26)–(30) and Equations in non-relativistic one (54)–(58). In contrast to Equation (28), in Equation (56) there is no dependence on gauge-invariant scalar field Q. The last Equation (58) depends on the electric potential Q linearly, which makes the impact of the applied electric field on superconductivity quite nontrivial.

4. Summary

The electrodynamics of superconductors is based on the fundamental phenomenon in physics-spontaneous breakdown of $U\left(1\right)$ gauge symmetry. The system of equations depends on the mechanism of symmetry breaking. The equations in the present paper describe the electrodynamics of s-wave superconductors, while the equations for d-wave or p-wave superconductors look in a different way. The equations are different if the superconductor is a metal or insulator in normal state. If the material is metal above the critical temperature, we have to account for the Ohm law, and we have to supplement Equation (26) with a term proportional to the electric field $\mathbf{J}=\sigma \mathbf{E}$. The conductivity $\sigma$ depends on the density of conducting electrons, and is responsible for the screening of the electric field [17]. The density of normal quasiparticles decreases at low temperature; at very low temperature $\sigma$ is zero, there is no quasiparticle current, and the system of Equations (26)–(30) is appropriate to describe the electrodynamics of s-wave superconductors. On the other hand, there are materials which possess superconductor–insulator transition. For these systems, Equations (26)–(30) describe the properties of the material well when an electric field is applied, even near the critical temperature. Therefore, we can apply an electric field near the critical superconductor–insulator transition temperature to study the critical behavior of this type of superconductor. One of the examples is sulfur hydride [18]. It is a superconductor at very high pressure and temperature. Temperature dependence of the resistance, measured at different pressures, shows that the material undergoes a superconductor-insulator transition below 129 GPa.

The shape of the sample is very important. In the present paper, we study systems with half space ($z>0$) occupied by the superconductor. We assume that deep inside the superconductor the electric and magnetic fields approach zero and hence the fields $\mathbf{Q}$ and Q are zero, while $\rho ={\rho }_0$ is a constant. In this way, we obtain London brothers equations and Equations (37) and (39), which imply that an electric field penetrates as a magnetic field does. This approximation fails when the geometry of the system is with finite size—slab geometry. This is because the electric and magnetic fields are finite within a finite-size sample. Therefore, the vector and scalar fields $\mathbf{Q}$ and Q are not zero, and one cannot neglect them in the last term of Equation (30), and cannot set the density of Cooper pairs $\rho$ equal to constant. Due to this, Equations (37) and (39) cannot be considered even as a rough approximations. They are not a correct description of the electrodynamics of finite-size samples. This makes the electrodynamics of superconductors with finite sizes more difficult to investigate.

The possibility that a superconductor may have an electric field in its interior has been discussed in other theoretical frameworks [1,6,10]. The equations in these papers discuss the charge and electric field distribution in superconductors. They result from assumptions [1] and phenomenological considerations. This is in stark contrast to the present paper, where the Maxwell equations for s-type superconductors are obtained from first principles. In References [1,6,10], authors consider a system of equations for electric field, magnetic field, scalar electric potential and vector magnetic potential. They consider the density of Cooper pairs equal to constant included in the London penetration depth ${\lambda }_L$ (38). While the resulting Equation (37) explains the Meissner–Ochsenfeld effect, Equations (37) and (39) do not match the physical reality.

In the present paper, a system of equations based on strong mathematical fundament is derived. They describe the electrodynamics of all s-wave superconductors at low temperature, where there are no normal quasiparticles and electric field can penetrate, and at all temperatures below the critical one when the normal state of the superconductor is insulator.

When the model is relativistically covariant, we obtained that the applied electric field supports the superconductivity. When the model is non-relativistic, the impact of the applied electric field on superconductivity is more complicated.

The equations in relativistic theory (26)–(30) are invariant under the discrete transformation $\mathbf{B}\to -\mathbf{B}$, $\mathbf{Q}\to -\mathbf{Q}$, and independently under the transformation $\mathbf{E}\to -\mathbf{E}$, $Q\to -Q$. In contrast, the system of equations in non-relativistic theory (54)–(58) are invariant under the discrete transformation of magnetic field $\mathbf{B}$ and gauge-invariant field $\mathbf{Q}$, but they are not invariant under the discrete transformation of electric field $\mathbf{E}$ and gauge-invariant field Q. This means that the effects of the applied electric fields ${\mathbf{E}}_0$ and $-{\mathbf{E}}_0$ on superconductivity are different.

It is important to underline that the fields Q and $\mathbf{Q}$ are gauge-invariant. This is why they should be measurable, as the electric and magnetic fields are measurable. The role of these fields is fundamental in superconductivity, but is not investigated.