On the Binding Energy of Atoms in Crystals of Noble Gases and Metals and the Speed of Sound
Abstract
:1. Introduction
2. Theory
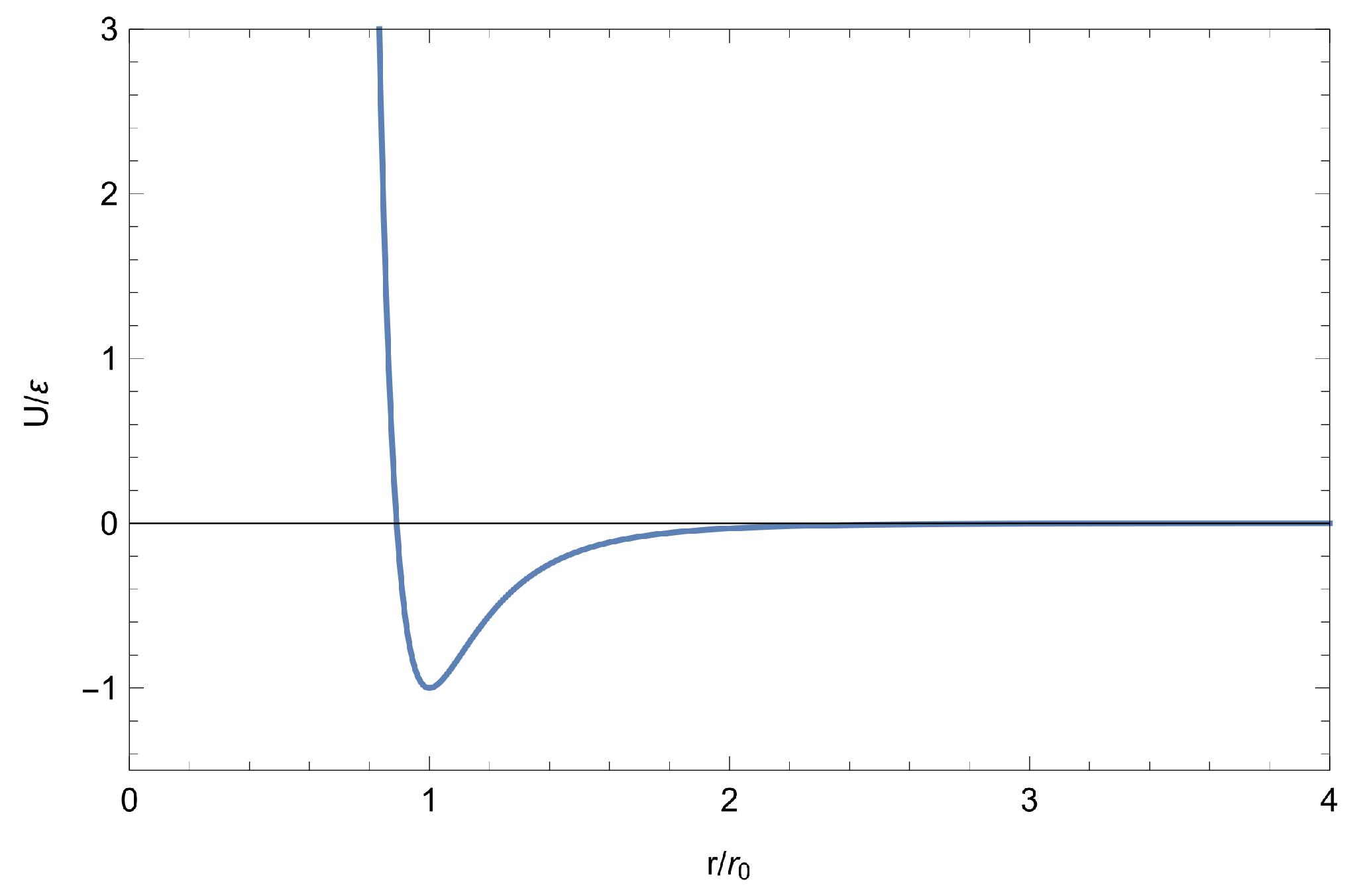
2.1. Preliminary Observations
2.2. Young’s Modulus from Mie and Lennard-Jones Potential
2.3. Relationship between Binding Energy and Speed of Sound
2.4. Binding Energy in a Crystal and the Associated Mass Defect
3. Values of Binding Energy for Several Elements
3.1. Values of Binding Energy Using the 12-6 Lennard-Jones Potential
3.2. Values of Binding Energy Using the Mie Potential
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
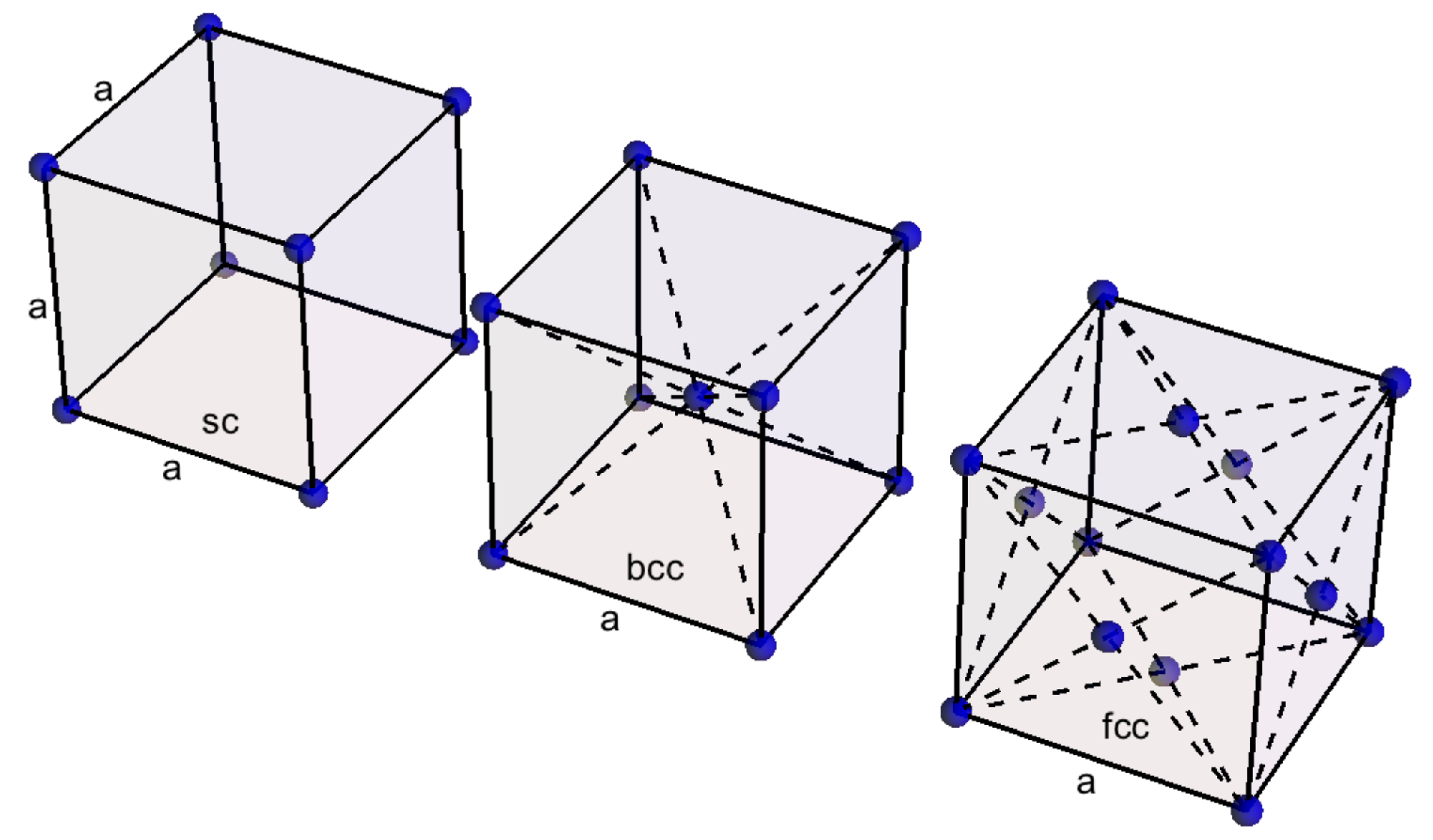
Appendix A. About Anisotropy in Crystals
Appendix B. Case of Face Centered Cubic Structure
Appendix C. Determination of Fürth’s Mie Potential Parameters
| Element | (kcal/mol) |
|---|---|
| Al | 67.60 |
| Au | 90.70 |
| Pb | 47.00 |
| Ni | 98.00 |
| Pt | 124.7 |
| Ag | 69.40 |
| Cu | 81.70 |
| Fe | 96.50 |
| Mo | 156.0 |
| W | 203.9 |
References
- Müsera, M.H.; Sukhomlinov, S.V.; Pastewka, L. Interatomic potentials: Achievements and challenges. Adv. Phys. X 2023, 8, 2093129. [Google Scholar] [CrossRef]
- Kshirsagar, S.; Mandadapu, K.K.; Papadopoulos, P. Classical molecular dynamics simulations of crystal lattices with truncated Taylor series-based interatomic potentials. Comput. Mater. Sci. 2016, 120, 127–134. [Google Scholar] [CrossRef]
- Hafskjold, B.; Travis, K.P.; Hass, A.B.; Hammer, M.; Aasen, A.; Wilhelmsen, Ø. Thermodynamic properties of the 3D Lennard-Jones/spline model. Mol. Phys. 2019, 117, 3754–3769. [Google Scholar] [CrossRef]
- Magomedov, M.N. The Correlation of the Parameters of Interatomic Interaction in Crystals with the Position of Atom in the Periodic Table. High Temp. 2008, 46, 484–494. [Google Scholar] [CrossRef]
- Blazhynska, M.M.; Kyrychenko, A.; Kalugin, O.N. Molecular dynamics simulation of the size-dependent morphological stability of cubic shape silver nanoparticles. Mol. Simul. 2018, 44, 981–991. [Google Scholar] [CrossRef]
- Ramezani-Dakhel, H.; Ruan, L.; Huang, Y.; Heinz, H. Molecular Mechanism of Specific Recognition of Cubic Pt Nanocrystals by Peptides and of the Concentration-Dependent Formation from Seed Crystals. Adv. Funct. Mater. 2015, 25, 1374–1384. [Google Scholar] [CrossRef]
- Lutsko, J.F.; Schoonen, C. Classical density-functional theory applied to the solid state. Phys. Rev. E 2020, 102, 062136. [Google Scholar] [CrossRef] [PubMed]
- Ferrante, J.; Smith, J.R.; Rose, J.H. Diatomic Molecules and Metallic Adhesion, Cohesion, and Chemisorption: A Single Binding-Energy Relation. Phys. Rev. Lett. 1986, 50, 1385. [Google Scholar] [CrossRef]
- Stacey, W.M. Nuclear Reactor Physics, 2nd ed.; Wiley-VCH Verlag GmbH & Co., KGaA: Weinheim, Germany, 2007. [Google Scholar]
- Chai, Y.; Gao, X.; Liang, Y.; Wang, J.; Hu, W.; Wang, Y. Improvement and prediction on high temperature melting characteristics of coal ash. High Temp. Mater. Process. 2023, 42, 20220039. [Google Scholar] [CrossRef]
- Trachenko, K.; Monserrat, B.; Pickard, C.J.; Brazhkin, V.V. Speed of sound from fundamental physical constants. Sci. Adv. 2020, 6, eabc8662. [Google Scholar] [CrossRef]
- Erokhin, K.M.; Kalashnikov, N.P. Relationships of Macroscopic Characteristics of a Solid with the Binding Energy of an Ion in a Metal Lattice. Phys. Solid State 2021, 63, 973–977. [Google Scholar] [CrossRef]
- Vos, F.L.J.; Aalberts, D.P.; van Saarloos, W. Simple method for calculating the speed of sound in tight-binding models: Application to the Su-Schrieffer-Heeger model. Phys. Rev. B 1996, 53, R5986. [Google Scholar] [CrossRef]
- Khrapak, S.A. Sound Velocities of Lennard-Jones Systems Near the Liquid-Solid Phase Transition. Molecules 2020, 25, 3498. [Google Scholar] [CrossRef] [PubMed]
- Kinsler, L.E.; Frey, A.R.; Coppens, A.B.; Sanders, J.V. Fundamentals of Acoustics, 4th ed.; John Wiley & Sons: Hoboken, NJ, USA, 1999. [Google Scholar]
- Lung, C.W.; March, N.H. Mechanical Properties of Metals: Atomistic and Fractal Continuum Approaches; World Scientific Pub. Co., Inc.: Singapore, 1999. [Google Scholar]
- Roylance, D. Mechanics of Materials; John Wiley & Sons: Hoboken, NJ, USA, 1996. [Google Scholar]
- Kittel, C. Introduction to Solid State Physics; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Stone, A. The Theory of Intermolecular Forces; Oxford University Press: Oxford, UK, 2013. [Google Scholar]
- Magomedov, M.N. The Calculation of the Parameters of the Mie-Lennard-Jones Potential. High Temp. 2006, 44, 513–529. [Google Scholar] [CrossRef]
- Horton, G.K. Ideal Rare-Gas Crystals. Am. J. Phys. 1968, 36, 93–119. [Google Scholar] [CrossRef]
- Fürth, R. On the equation of state for solids. Proc. R. Soc. A 1994, 183, 87–110. [Google Scholar]
- Heinz, H.; Vaia, R.A.; Farmer, B.L.; Naik, R.R. Accurate Simulation of Surfaces and Interfaces of Face-Centered Cubic Metals Using 12-6 and 9-6 Lennard-Jones Potentials. J. Phys. Chem. C 2008, 112, 17281–17290. [Google Scholar] [CrossRef]
- Aleksandrov, I.V.; Goncharov, A.F.; Zisman, A.N.; Stishov, S.M. Diamond at high pressures: Raman scattering of light, equation of state, and high-pressure scale. Sov. Phys. JETP 1987, 66, 384–390. [Google Scholar]
- Niebel, K.F.; Venables, J.A. An explanation of the crystal structure of the rare gas solids. Proc. R. Soc. Lond. A 1974, 336, 365–377. [Google Scholar]
- Hakim, T.M.; Glyde, H.R. Dynamics of solid neon monolayers. Phys. Rev. B 1988, 37, 984. [Google Scholar] [CrossRef]
- Lawrence, D.J.; Neale, F.E. Longitudinal wave velocity in solid argon. Proc. Phys. Soc. 1965, 85, 1261. [Google Scholar] [CrossRef]
- Peter, H.; Korpiun, P.; Lüscher, E. Measurement of the longitudinal sound velocity in solid krypton at 4.2 °K, 77 °K and 90 °K. Phys. Lett. A 1968, 26, 207. [Google Scholar] [CrossRef]
- Weigel, P.L.R.; Hansen, E.V.; Dolinski, M.J. Development and Characterization of Solid Noble Bolometers. In Proceedings of the 2019 Meeting of the Division of Particles and Fields of the American Physical Society, DPF 2019, Boston, MA, USA, 29 July–2 August 2019. [Google Scholar]
- Lide, D.R. (Ed.) CRC Handbook of Chemistry and Physics; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Samsonov, G.V. (Ed.) Handbook of the Physicochemical Properties of the Elements; IFI/Plenum: New York, NY, USA; Washington, DC, USA, 1968. [Google Scholar]
- Kanhaiya, K.; Kim, S.; Im, W.; Heinz, H. Accurate simulation of surfaces and interfaces of ten FCC metals and steel using Lennard-Jones potentials. NPJ Comput. Mater. 2021, 7, 17. [Google Scholar] [CrossRef]
- Jacobson, D.W.; Thompson, G.B. Revisting Lennard Jones, Morse, and N-M potentials for metals. Comput. Mater. Sci. 2022, 205, 111206. [Google Scholar] [CrossRef]
- Kube, C.M. Elastic anisotropy of crystals. AIP Adv. 2016, 6, 095209. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. Theory of Elasticity, 2nd ed.; Pergamon Press: Oxford, UK, 1970. [Google Scholar]
- Ziegenhain, G.; Urbassek, H.M.; Hartmaier, A. Influence of crystal anisotropy on elastic deformation and onset of plasticity in nanoindentation: A simulational study. J. Appl. Phys. 2010, 107, 061807. [Google Scholar] [CrossRef]
| Atomic Weight | Crystal Structure | Speed of Sound (m/s) | Reference | |
|---|---|---|---|---|
| Ne | 20.149 | fcc | 1290 | [26] |
| Ar | 39.948 | fcc | 1630 | [27] |
| Kr | 83.798 | fcc | 1335 | [28] |
| Xe | 131.29 | fcc | 1150 | [29] |
| A1 | 26.981 | fcc | 6420 | [30] |
| Au | 196.97 | fcc | 3240 | [30] |
| Pb | 207.20 | fcc | 2160 | [30] |
| Ni | 58.693 | fcc | 6040 | [30] |
| Pt | 195.08 | fcc | 3260 | [30] |
| Ag | 107.87 | fcc | 3650 | [30] |
| Cu | 63.546 | fcc | 4760 | [30] |
| Fe | 55.845 | bcc | 5950 | [30] |
| Mo | 95.940 | bcc | 6250 | [30] |
| W | 183.84 | bcc | 5220 | [30] |
| Ce | 140.12 | fcc | 2100 | [31] |
| Yb | 173.04 | fcc | 1590 | [31] |
| Th | 232.04 | fcc | 2490 | [31] |
| This Work | Horton [21] | |
|---|---|---|
| Ne | 0.00483 | 0.00450 |
| Ar | 0.01528 | 0.01473 |
| Kr | 0.02150 | 0.02028 |
| Xe | 0.02500 | 0.02859 |
| This Work | Heinz et al. [23] | Jacobson and Thompson [33] | |
|---|---|---|---|
| Al | 0.1601 | 0.1743 | 0.1983 |
| Au | 0.2977 | 0.2294 | 0.2231 |
| Pb | 0.1392 | 0.1270 | 0.1188 |
| Ni | 0.3083 | 0.2450 | 0.2608 |
| Pt | 0.2985 | 0.3382 | 0.3427 |
| Ag | 0.2069 | 0.1977 | 0.1722 |
| Cu | 0.2073 | 0.2046 | 0.2050 |
| Fe | 0.2846 | 0.2601 * | 0.2625 |
| Mo | 0.5395 | - | - |
| W | 0.7211 | - | 0.5377 |
| This Work | Kanhaiya et al. [32] | |
|---|---|---|
| Ce | 0.0890 | 0.2766 |
| Yb | 0.0630 | 0.1175 |
| Th | 0.2071 | 0.3672 |
| This Work | Magomedov [20] | n | m | |
|---|---|---|---|---|
| Ne | 0.0028 | 0.0045 | 5.83 | 21.39 |
| Ar | 0.0100 | 0.0150 | 6.62 | 16.69 |
| Kr | 0.0148 | 0.0205 | 6.56 | 15.92 |
| Xe | 0.0173 | 0.0285 | 6.73 | 15.42 |
| This Work | Magomedov [20] | n | m | |
|---|---|---|---|---|
| Al | 0.4239 | 0.5714 | 2.49 | 10.92 |
| Au | 0.7027 | 0.6387 | 1.96 | 15.56 |
| Pb | 0.3100 | 0.3399 | 2.27 | 14.24 |
| Ni | 0.8149 | 0.7506 | 3.56 | 7.65 |
| Pt | 0.6372 | 0.9795 | 2.53 | 13.33 |
| Ag | 0.4673 | 0.4944 | 3.08 | 10.35 |
| Cu | 0.5884 | 0.5895 | 3.03 | 8.37 |
| Fe | 0.8975 | 1.0838 | 3.54 | 6.45 |
| Mo | 2.3635 | 1.7042 | 2.14 | 7.68 |
| W | 1.7695 | 2.2068 | 3.42 | 8.58 |
| This Work | Magomedov [20] | n | m | |
|---|---|---|---|---|
| Ce | 0.5390 | 0.7334 | 2.45 | 4.85 |
| Yb | 0.2374 | 0.2682 | 3.61 | 5.29 |
| Th | 0.8161 | 0.9970 | 3.02 | 6.05 |
| This Work | Fürth [22] | n | m | |
|---|---|---|---|---|
| Al | 0.4117 | 0.4886 | 4 | 7 |
| Au | 0.4871 | 0.6556 | 5.5 | 8 |
| Pb | 0.2783 | 0.3397 | 3 | 12 |
| Ni | 0.7926 | 0.7083 | 4 | 7 |
| Pt | 0.4884 | 0.9013 | 5.5 | 8 |
| Ag | 0.4729 | 0.5016 | 4.5 | 7 |
| Cu | 0.5330 | 0.5905 | 4 | 7 |
| Fe | 0.7319 | 1.0462 | 4 | 7 |
| Mo | 1.1099 | 1.6913 | 5 | 7 |
| W | 1.4835 | 2.2107 | 5 | 7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dall’Osso, A. On the Binding Energy of Atoms in Crystals of Noble Gases and Metals and the Speed of Sound. Crystals 2024, 14, 852. https://doi.org/10.3390/cryst14100852
Dall’Osso A. On the Binding Energy of Atoms in Crystals of Noble Gases and Metals and the Speed of Sound. Crystals. 2024; 14(10):852. https://doi.org/10.3390/cryst14100852
Chicago/Turabian StyleDall’Osso, Aldo. 2024. "On the Binding Energy of Atoms in Crystals of Noble Gases and Metals and the Speed of Sound" Crystals 14, no. 10: 852. https://doi.org/10.3390/cryst14100852






