Nonlinear Seismic Response of Tunnel Structures under Traveling Wave Excitation
Abstract
:1. Introduction
2. Seismic Wave Reflection Theory
2.1. Plane Wave Reflection Theory
2.2. Seismic Wave Reflection Discussion
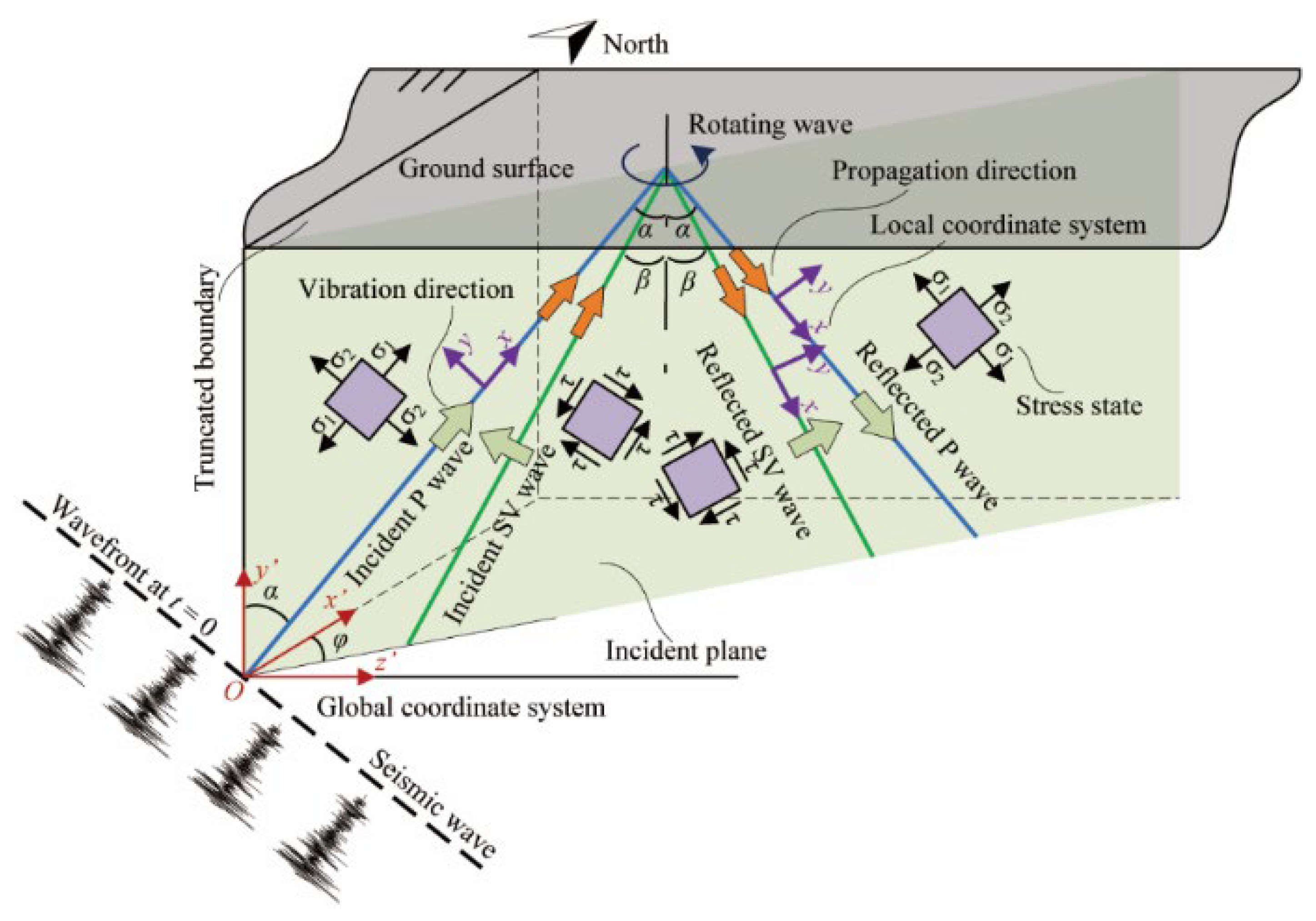
3. Theory of Seismic Wave Incidence
3.1. Matrix Methods for Coordinate Transformations
- (1)
- Acceleration, velocity, displacement:
- (2)
- Stress and strain
- (3)
- Equivalent Nodal Load
3.2. Extension to 3D Modeling
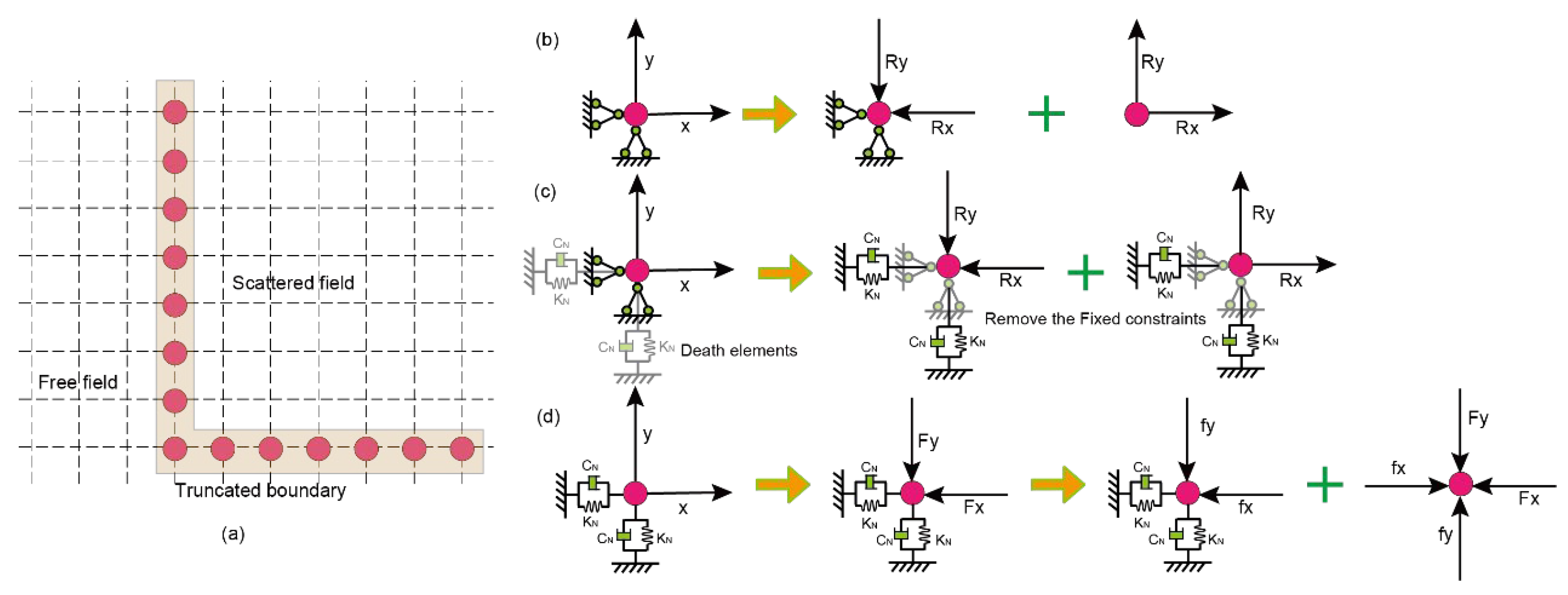
3.3. Transformation of Static and Dynamic Boundary Conditions
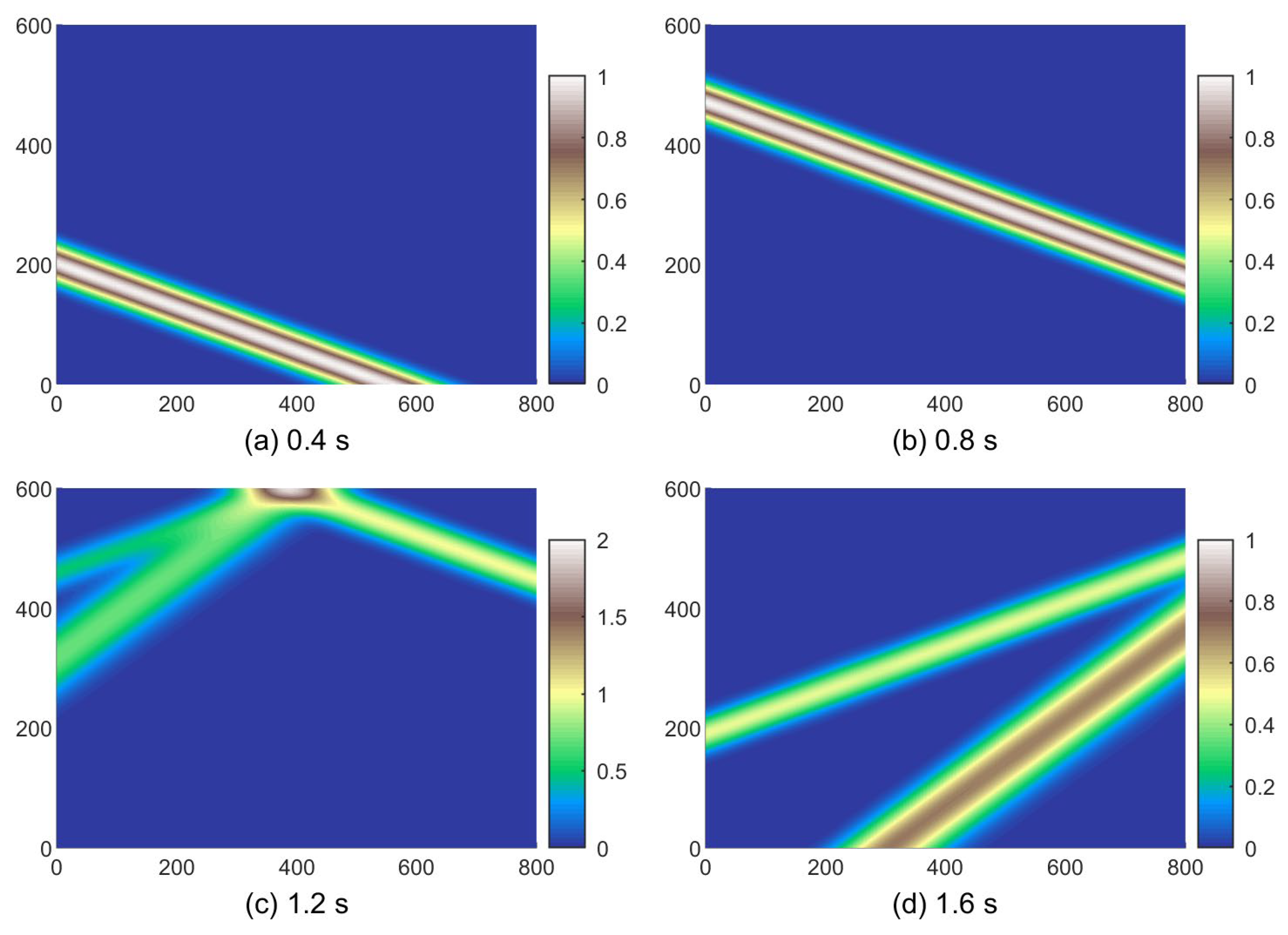
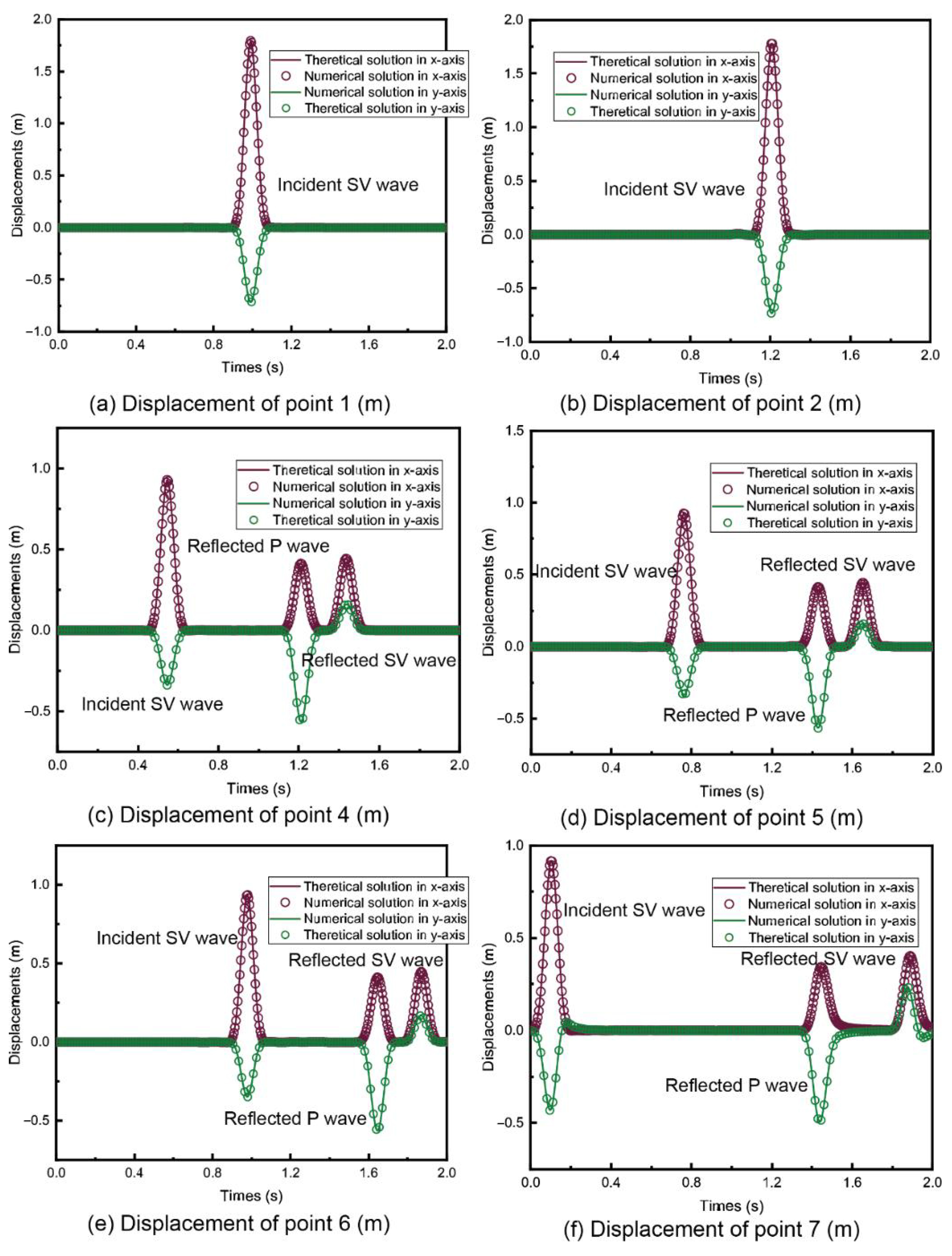
4. Example Validation
5. Numerical Simulation of Tunnels
5.1. Numerical Modeling and Parameterization
5.2. Analysis of Calculation Results
- (1)
- Spatial coherence factor
- (2)
- Initial support moment
- (3)
- Lining Fourier spectrum
- (4)
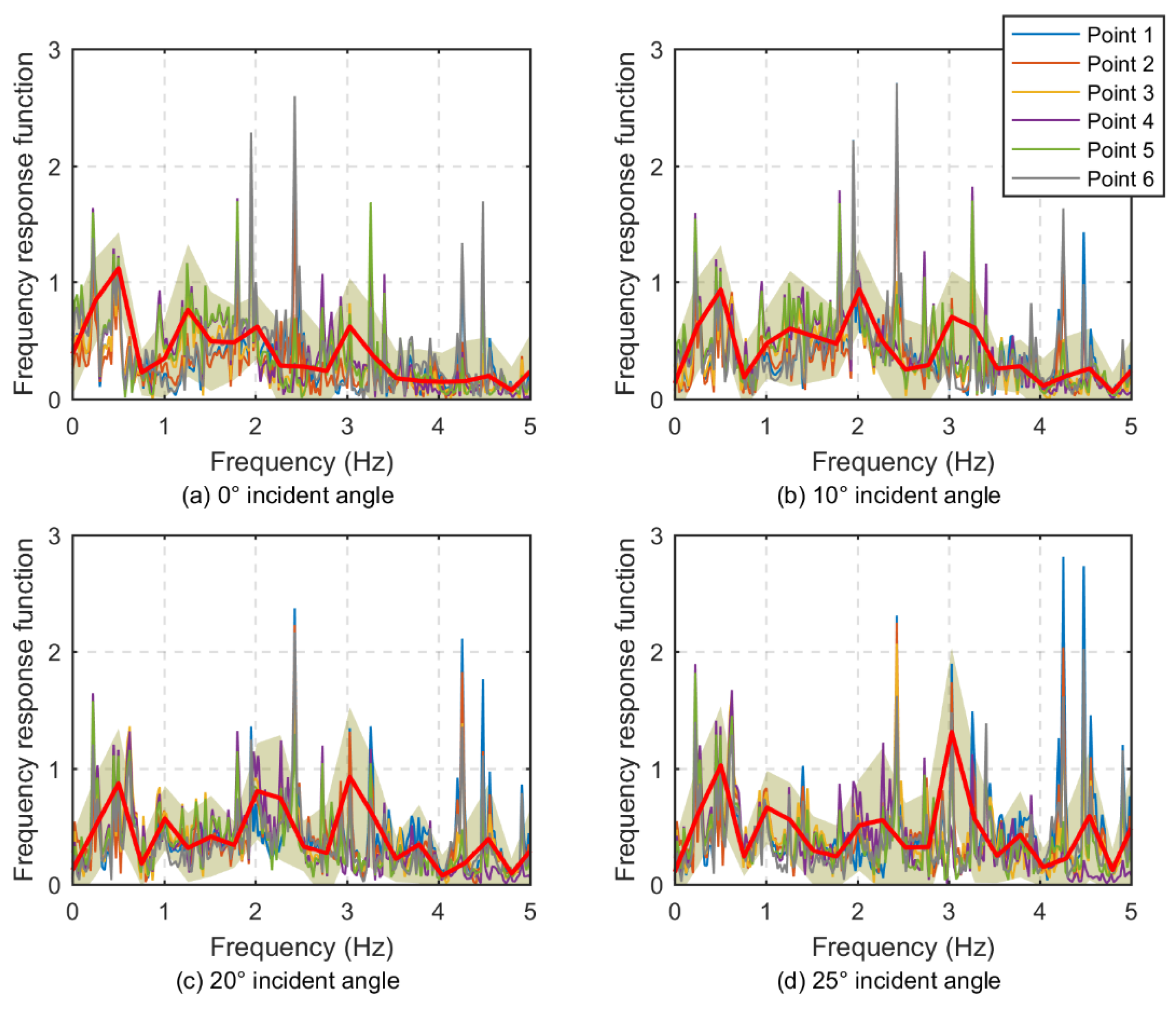
- Lining frequency response function
- (5)
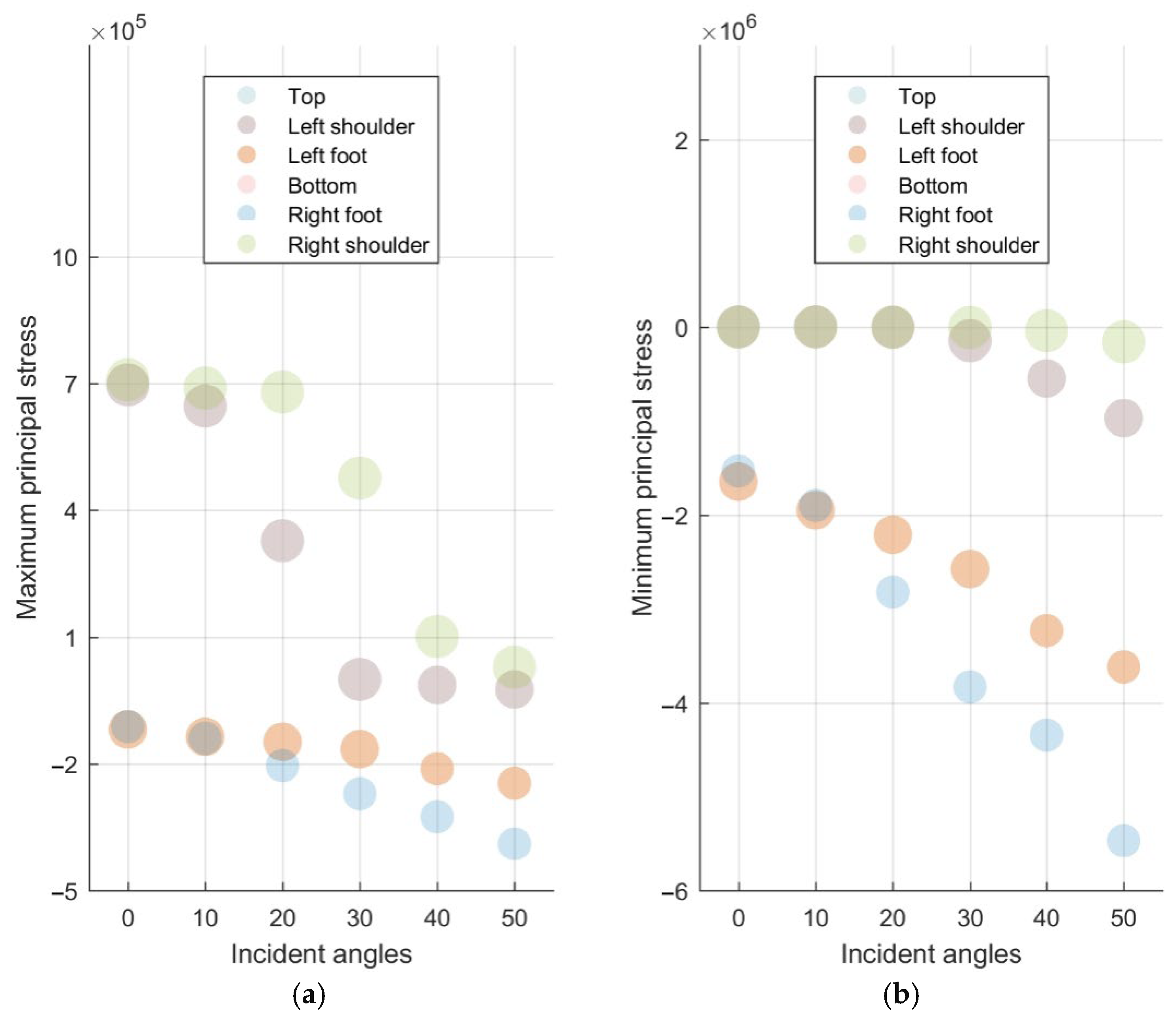
- Lining principal stresses
6. Conclusions
- (1)
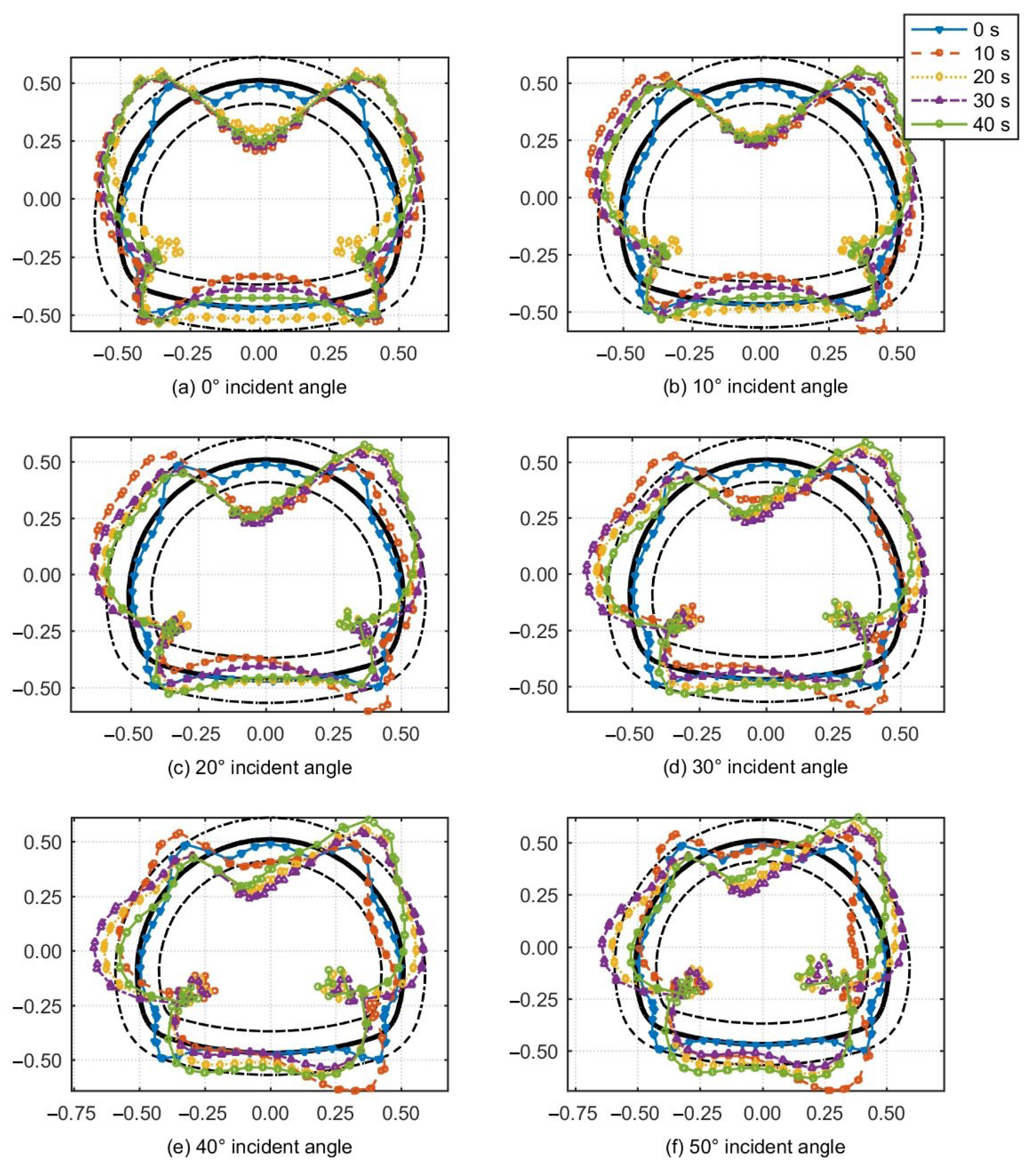
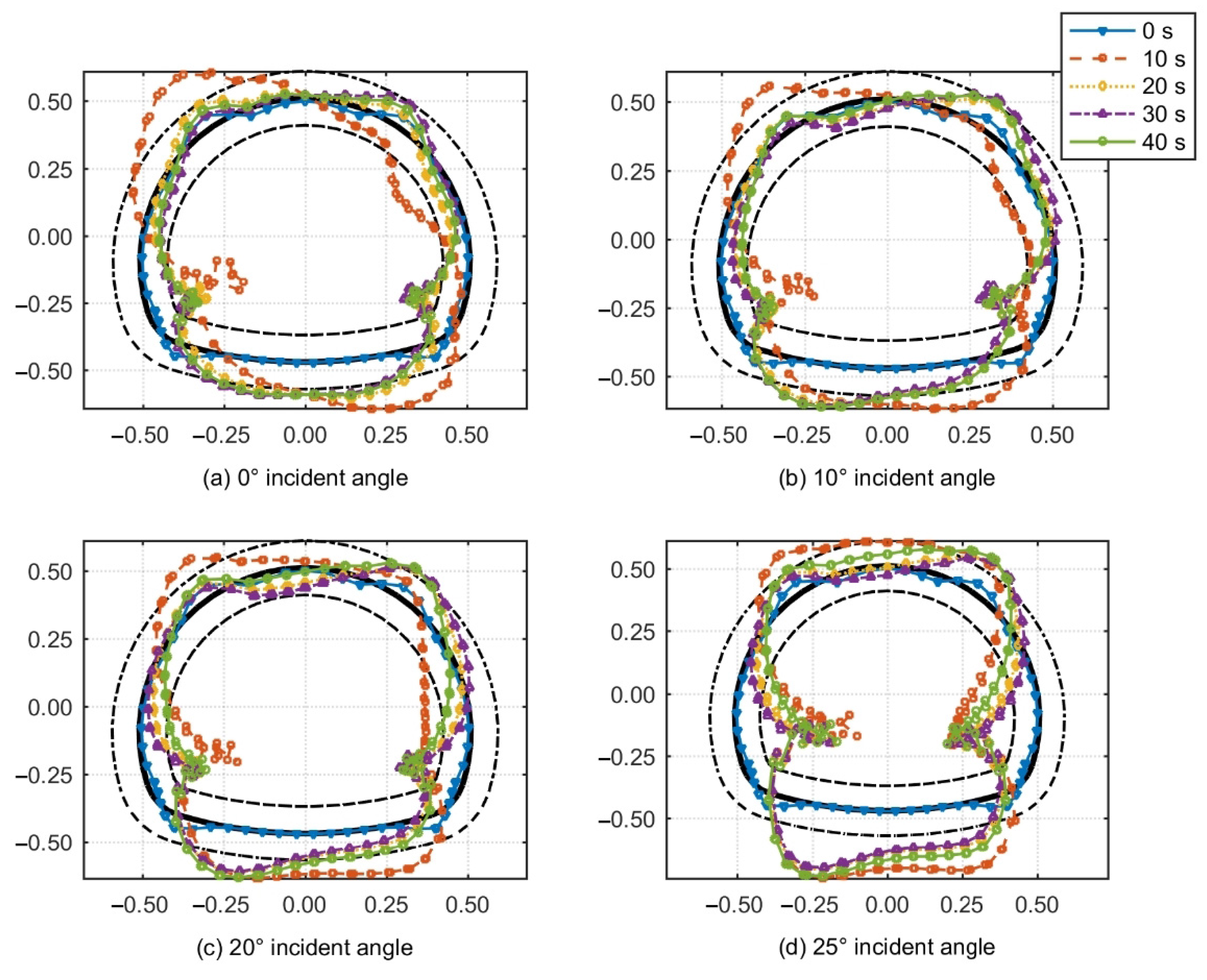
- The oblique incidence method effectively captures the traveling wave effect, whereas the attenuation and coherence effects are partially dependent on the random excitation method. In the analysis of P wave and SV wave incidence, the “rotation effect” due to oblique incidence must be accounted for, as it significantly influences the stress and deformation of the tunnel structure, exhibiting distinct rotational characteristics for different wave types.
- (2)
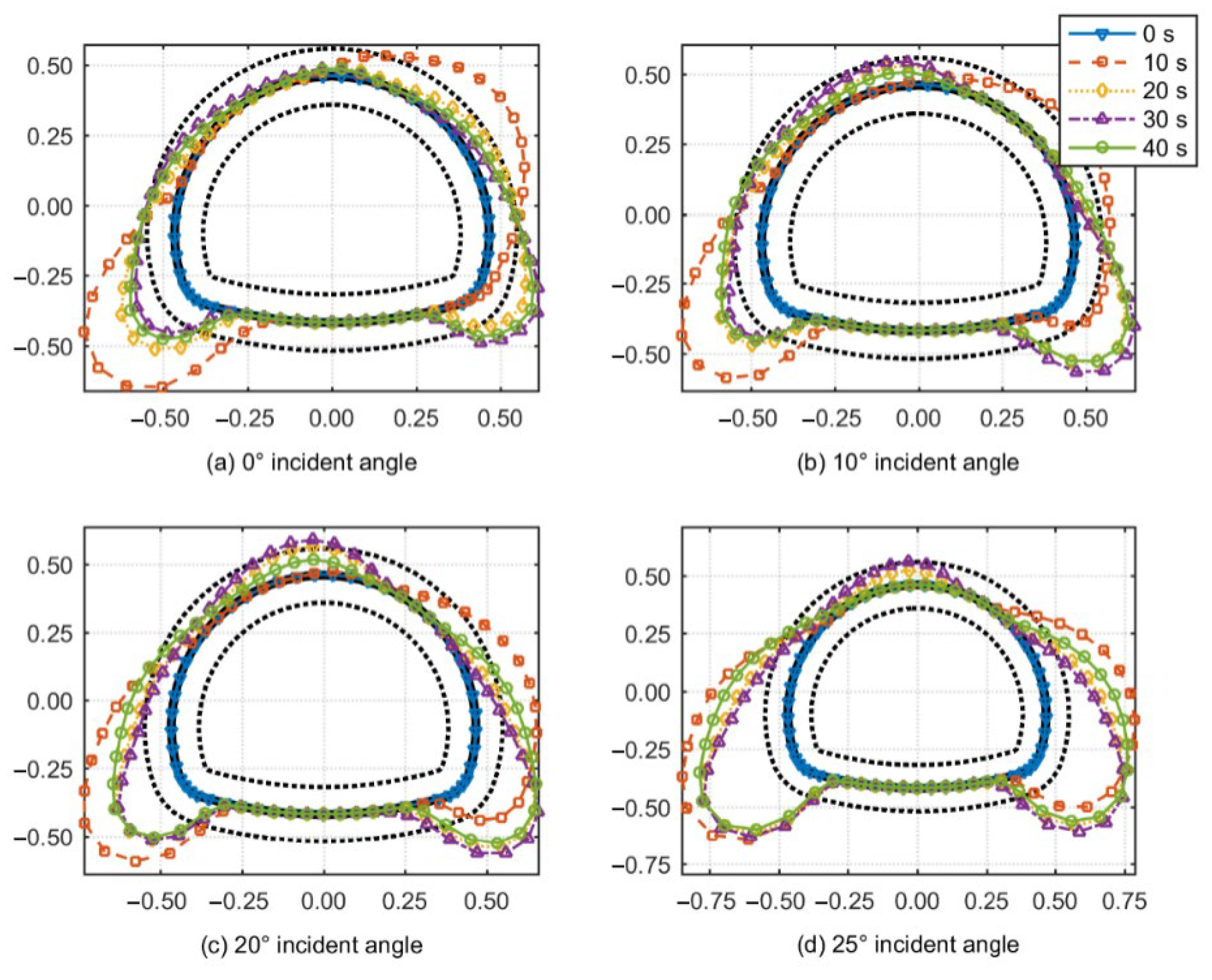
- During P wave incidence, particular attention should be given to the structural safety of the vault and the haunch due to the impact of extension waves. As the oblique incidence angle increases, the structural forces and deformations rotate to a certain extent, with a marked increase in relevant values, posing a potential threat to the stability of the tunnel structure.
- (3)
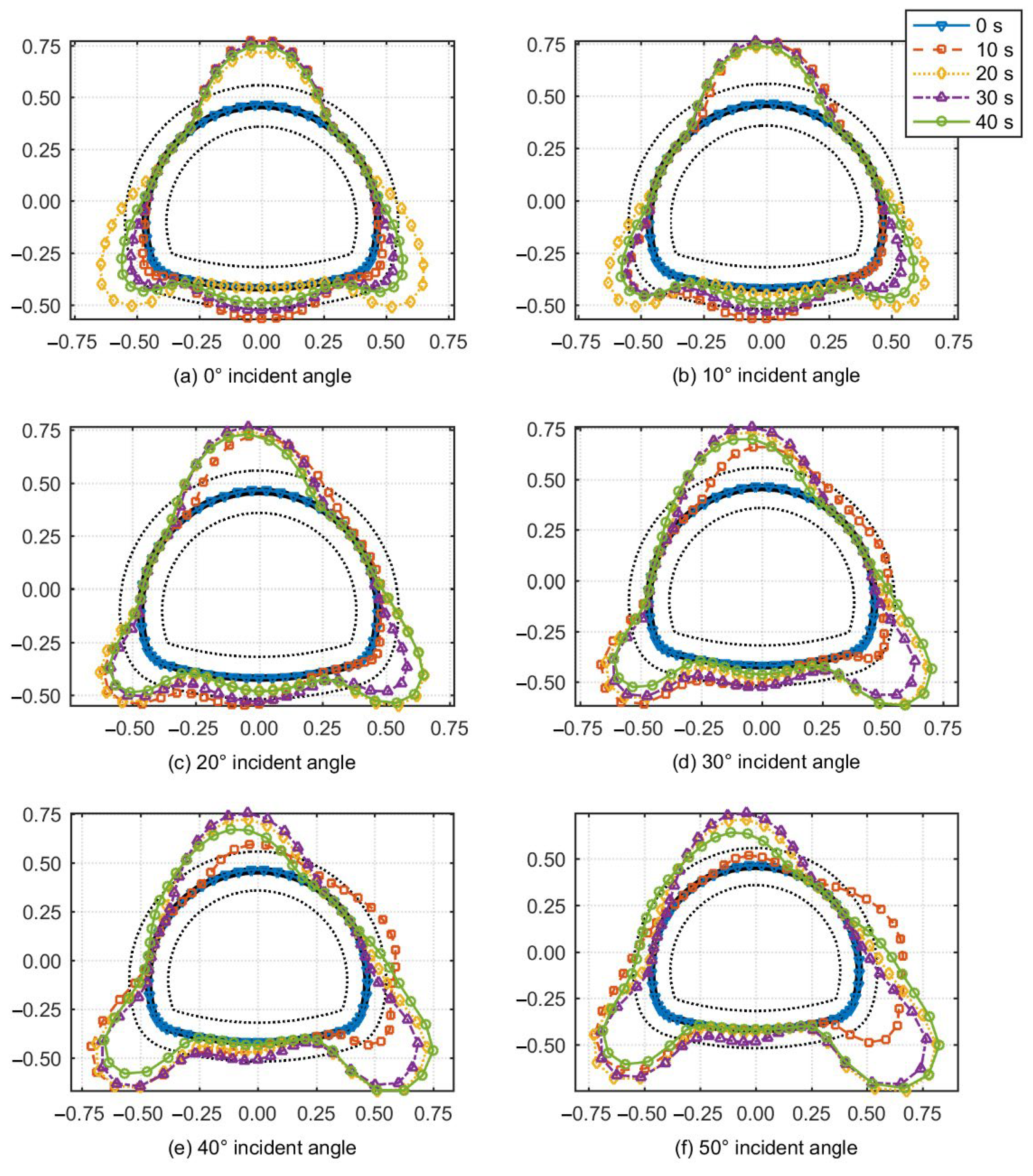
- Under the shear action of SV waves, the tunnel structure may undergo ovaling deformation, rendering the arch shoulders and side walls more susceptible to damage. As the angle of oblique incidence rises, the hazardous areas are shifted to the crown and inverted arch by the “rotation effect,” although the changes in the relevant values are not pronounced.
- (4)
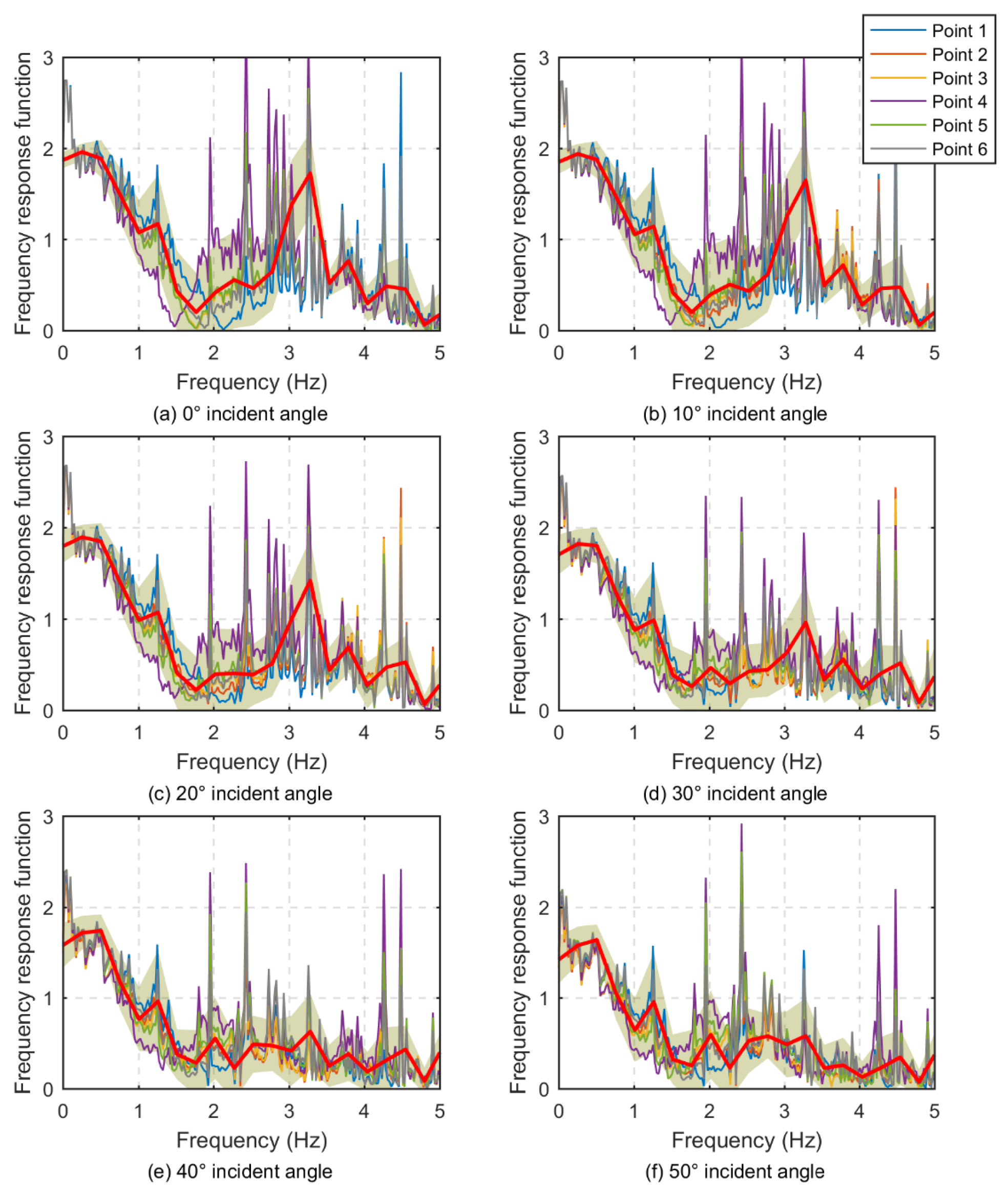
- Compared to tunnels with a circular cross-section, the low-frequency amplification of seismic waves in the surrounding rock and the variability of the frequency response function across different lining sections are more pronounced. Notably, the dominant frequency characteristics are very significant during P wave incidence; with the increase in the incidence angle, the attenuation of the seismic wave signal becomes more apparent. In contrast, the SV wave exhibits a more uniform behavior. These insights are of paramount importance for enhancing the understanding and refinement of the seismic design of tunnels and underground structures.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Reflection Characteristics of SV Incident Free Surfaces
Appendix B. SV Incident Equivalent Nodal Load Calculations
References
- Du, X.L.; Li, Y.; Xu, C.S.; Lu, D.C.; Xu, Z.G.; Jin, L. Review on damage causes and disaster mechanism of Daikai subway station during 1995 Osaka-Kobe Earthquake. Chin. J. Geotech. Eng. 2018, 40, 223–236. [Google Scholar]
- Yan, K.; Zhang, J.; Wang, Z.; Liao, W.; Wu, Z. Seismic responses of deep buried pipeline under non-uniform excitations from large scale shaking table test. Soil Dyn. Earthq. Eng. 2018, 113, 180–192. [Google Scholar]
- Wang, W.; Wang, T.; Su, J.; Lin, C.; Seng, C.; Huang, T. Assessment of damage in mountain tunnels due to the Taiwan Chi-Chi Earthquake. Tunn. Undergr. Space Technol. 2001, 16, 133–150. [Google Scholar]
- Wang, T.; Kwok, O.A.; Jeng, F. Seismic response of tunnels revealed in two decades following the 1999 Chi-Chi earthquake (Mw 7.6) in Taiwan: A review. Eng. Geol. 2021, 287, 106090. [Google Scholar]
- Wang, W.L.; Su, Z.J.; Lin, J.H.; Cheng, J.R.; Wang, T.D.; Wang, C.H. Discussion on damaged extent of mountainous tunnels due to earthquake, Taiwan. Mod. Tunn. Technol. 2001, 02, 52–60. [Google Scholar]
- St John, C.M.; Zahrah, T.F. Aseismic design of underground structures. Tunn. Undergr. Space Technol. 1987, 2, 165–197. [Google Scholar]
- Penzien, J. Seismically induced racking of tunnel linings. Earthq. Eng. Struct. Dyn. 2000, 29, 683–691. [Google Scholar]
- Zhu, J.; Li, X.J.; Liang, J.W. Seismic responses of underground tunnels subjected to obliquely incident seismic waves by 2.5D FE-BE coupling method. Chin. J. Geotech. Eng. 2022, 44, 1846–1854. [Google Scholar]
- Huang, W.Z.; He, C.; Xu, G.Y.; Li, B. Seismic response of a submarine immersed tunnel under oblique incidence of SV waves. Tunnel Constr. 2024, 44, 724. [Google Scholar]
- Hashash, Y.M.A.; Tseng, W.S.; Krimotat, A. Seismic Soil-Structure Interaction Analysis for Immersed Tube Tunnels Retrofit. In Geotechnical Earthquake Engineering and Soil Dynamics III. Geotech. Spec. Publ. 1998, 75, 1380–1391. [Google Scholar]
- Hwang, J.H.; Lu, C.C. Seismic capacity assessment of old Sanyi railway tunnels. Tunn. Undergr. Space Technol. 2007, 22, 433–449. [Google Scholar]
- Yu, H.B.; Yang, Y.S.; Yuan, Y.; Duan, K.P.; Gu, Q. A comparison between vibration and wave methods in seismic analysis of underground structures. Earthq. Eng. J. 2019, 41, 845–852. [Google Scholar]
- Ding, Z.D.; Chen, Y.S.; Zi, H. Study on artificial boundary and ground motion input method in tunnel seismic response. Earthq. Eng. Eng. Dyn. 2022, 42, 52–61. [Google Scholar]
- Yan, L.; Haider, A.; Li, P.; Song, E. A numerical study on the transverse seismic response of lined circular tunnels under obliquely incident asynchronous P and SV waves. Tunn. Undergr. Space Technol. 2020, 97, 103235. [Google Scholar]
- Zhu, S.; Li, W.H.; Lee, V.W.; Zhao, C. Analytical solution of seismic response of an undersea cavity under incident P1-wave. Rock Soil Mech. 2021, 42, 93–103. [Google Scholar]
- Zhu, J.; Li, X.J.; Liang, J.W.; Bin, Z. Effects of a tunnel on site ground motion for 3D obliquely incident seismic waves. China Civil Eng. J. 2020, 53, 318–324. [Google Scholar]
- Du, X.L.; Xu, Z.G.; Xu, C.S.; Li, Y.; Jiang, J.W. Time-history analysis method for soil-underground structure system based on equivalent linear method. Chin. J. Geotech. Eng. 2018, 40, 2155–2163. [Google Scholar]
- Liu, J.B.; Bao, X.; Tan, H.; Wang, D.Y.; Li, S.T. Seismic wave input method for soil-structure dynamic interaction analysis based on internal substructure. China Civil Eng. J. 2020, 53, 1–8. [Google Scholar]
- Zhang, D.D.; Liu, Y.; Xiong, F.; Mei, Z. Seismic response analysis of rock tunnel near-portals under oblique incidence of P-wave and SV-wave. Shock Vib. 2022, 41, 278–286. [Google Scholar]
- Song, Z.; Wang, F.; Li, Y.; Liu, Y. Nonlinear seismic responses of the powerhouse of a hydropower station under near-fault plane P-wave oblique incidence. Eng. Struct. 2019, 199, 109613. [Google Scholar]
- Chen, D.; Pan, Z.; Zhao, Y. Seismic damage characteristics of high arch dams under oblique incidence of SV waves. Eng. Fail. Anal. 2023, 152, 107445. [Google Scholar]
- Huang, J.-Q.; Du, X.-L.; Zhao, M.; Zhao, X. Impact of incident angles of earthquake shear (S) waves on 3-D non-linear seismic responses of long lined tunnels. Eng. Geol. 2017, 222, 168–185. [Google Scholar]
- Zhang, W.; Seylabi, E.E.; Taciroglu, E. An ABAQUS toolbox for soil-structure interaction analysis. Comput. Geotech. 2019, 114, 103143. [Google Scholar]
- Kontoe, S.; Zdravkovic, L.; Potts, D.M.; Menkiti, C.O. Case study on seismic tunnel response. Can. Geotech. J. 2008, 45, 1743–1764. [Google Scholar]
- Aki, K.; Richards, P.G. Quantitative Seismology: Theory and Methods; University Science Books: Sausalito, CA, USA, 1980; pp. 204–356. [Google Scholar]
- Babich, V.; Kiselev, A. Elastic Waves; Taylor and Francis: Washington DC, USA, 2018; pp. 1–65. [Google Scholar]
- Pujol, J. Elastic Wave Propagation and Generation in Seismology; Cambridge University Press: Cambridge, UK, 2003; pp. 112–193. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Theory of Elasticity, 3rd ed.; Pergamon Press: Oxford, UK, 1986; pp. 21–87. [Google Scholar]
- Ma, S.J.; Chi, M.J.; Chen, X.L.; Chen, S.; Chen, H.J.; Xing, H.J. Research on the static-dynamic boundary switch and its rationality verification method. Acta Seismol. Sinica 2024, 46, 157–171. [Google Scholar]
- Liu, J.; Wang, Z.; Du, X.; Du, Y.-X. Three dimensional viscous-spring artificial boundaries in time domain for wave motion problems. Eng. Mech. 2005, 22, 46–51. [Google Scholar]
- Gao, F.; Zhao, Z.B. Study on transformation method for artificial boundaries in static-dynamic analysis of underground structure. J. Vib. Shock 2011, 30, 165–170. [Google Scholar]
- Su, W.; Qiu, Y.-X.; Xu, Y.-J.; Wang, J.-T. A scheme for switching boundary condition types in the integral static-dynamic analysis of soil-structures in Abaqus. Soil Dyn. Earthq. Eng. 2021, 141, 106458. [Google Scholar]
- Zhou, Y.Q.; Sheng, Q.; Li, N.N.; Fu, X.D. Preliminary study on time-space effect of the dynamic response of long tunnel under non-uniform ground motion. Rock Soil Mech. 2021, 11, 2287–2297. [Google Scholar]
- Hashash, Y.M.A.; Hook, J.J.; Schmidt, B.; Yao, I.C. Seismic design and analysis of underground structures. Tunn. Undergr. Space Technol. 2001, 16, 247–293. [Google Scholar]
- Shen, Y.; Gao, B.; Yang, X.; Tao, S. Seismic damage mechanism and dynamic deformation characteristic analysis of mountain tunnel after Wenchuan earthquake. Eng. Geol. 2014, 180, 85–98. [Google Scholar]





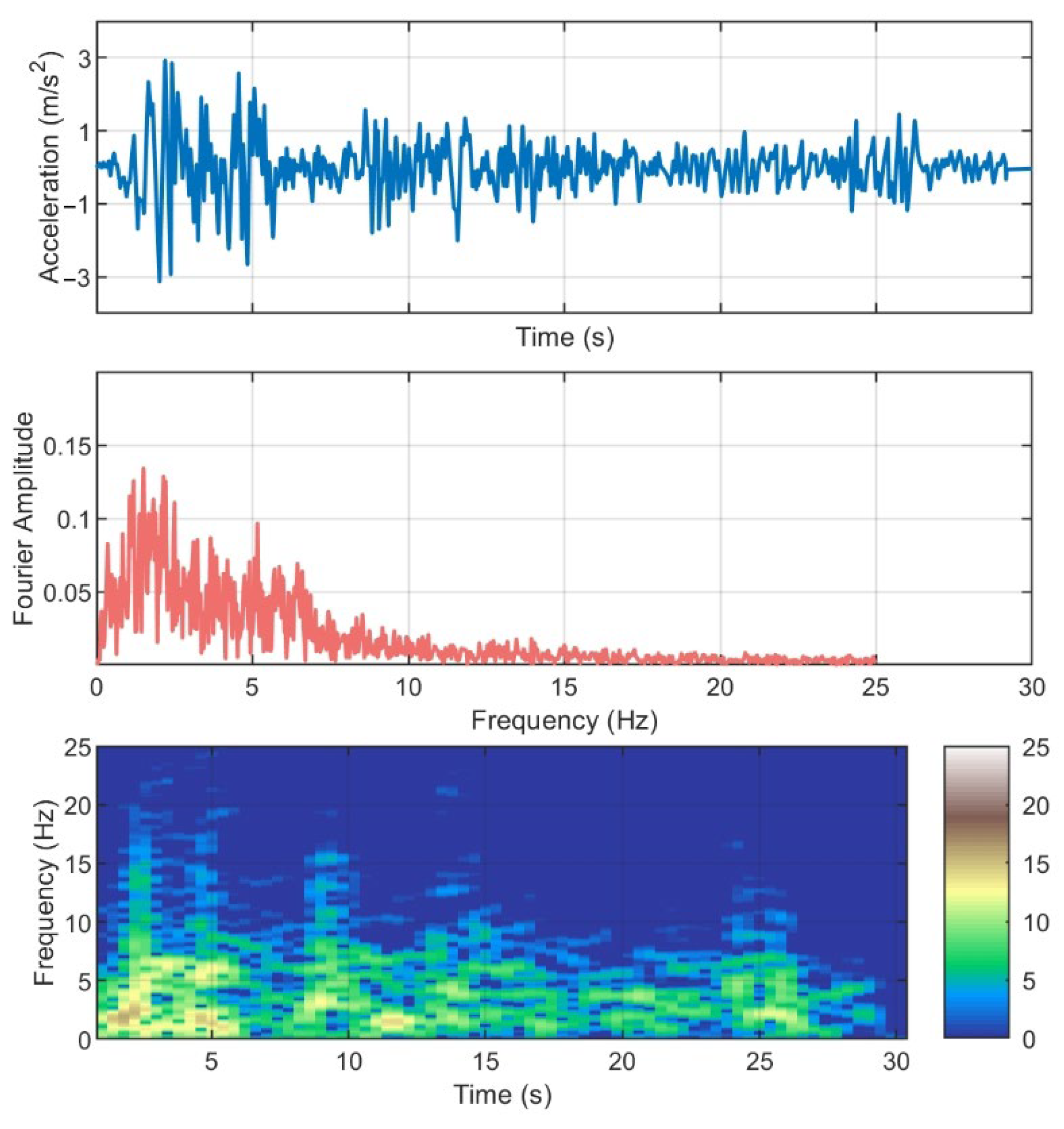


| Monitoring Station | Max Accel. (m/s)2 | Largest Peak Accel. (m/s)2 | Freq. (Hz) | 2nd Peak Accel. (m/s)2 | Freq. (Hz) | 3rd Peak Accel. (m/s)2 | Freq. (Hz) |
|---|---|---|---|---|---|---|---|
| Excitation acceleration | 1.826 | 0.113 | 1.175 | 0.089 | 1.500 | 0.088 | 2.150 |
| Vault | 1.826 | 0.137 | 1.177 | 0.127 | 0.852 | 0.126 | 0.351 |
| Left arch shoulder | 1.926 | 0.125 | 0.351 | 0.119 | 0.852 | 0.117 | 0.376 |
| Left arch footing | 1.971 | 0.124 | 0.351 | 0.116 | 0.376 | 0.115 | 0.852 |
| Inverted arch | 2.598 | 0.127 | 2.154 | 0.122 | 0.351 | 0.113 | 0.376 |
| Right arch shoulder | 1.984 | 0.124 | 0.351 | 0.116 | 0.376 | 0.114 | 0.852 |
| Right arch footing | 1.927 | 0.125 | 0.351 | 0.119 | 0.852 | 0.117 | 0.376 |
| Monitoring Station | Max Accel. (m/s)2 | Largest Peak Accel. (m/s)2 | Freq. (Hz) | 2nd Peak Accel. (m/s)2 | Freq. (Hz) | 3rd Peak Accel. (m/s)2 | Freq. (Hz) |
|---|---|---|---|---|---|---|---|
| Excitation acceleration | 1.826 | 0.113 | 1.175 | 0.089 | 1.500 | 0.088 | 2.150 |
| Vault | 0.987 | 0.044 | 1.779 | 0.042 | 2.079 | 0.042 | 2.204 |
| Left arch shoulder | 0.830 | 0.036 | 1.779 | 0.033 | 1.578 | 0.031 | 1.503 |
| Left arch footing | 1.056 | 0.054 | 1.503 | 0.048 | 1.779 | 0.047 | 1.177 |
| Inverted arch | 1.337 | 0.072 | 1.503 | 0.063 | 1.177 | 0.063 | 1.779 |
| Right arch shoulder | 1.372 | 0.074 | 1.503 | 0.069 | 1.779 | 0.060 | 1.578 |
| Right arch footing | 1.219 | 0.060 | 2.204 | 0.059 | 2.154 | 0.059 | 1.779 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suo, X.; Liu, L.; Qiao, D.; Xiang, Z.; Zhou, Y. Nonlinear Seismic Response of Tunnel Structures under Traveling Wave Excitation. Buildings 2024, 14, 2940. https://doi.org/10.3390/buildings14092940
Suo X, Liu L, Qiao D, Xiang Z, Zhou Y. Nonlinear Seismic Response of Tunnel Structures under Traveling Wave Excitation. Buildings. 2024; 14(9):2940. https://doi.org/10.3390/buildings14092940
Chicago/Turabian StyleSuo, Xiaoqing, Lilong Liu, Dan Qiao, Zhengsong Xiang, and Yuanfu Zhou. 2024. "Nonlinear Seismic Response of Tunnel Structures under Traveling Wave Excitation" Buildings 14, no. 9: 2940. https://doi.org/10.3390/buildings14092940





