Radii of Starlikeness of Ratios of Analytic Functions with Fixed Second Coefficients

Abstract

We introduce three classes of analytic functions with fixed second coefficients that are defined using the class $\mathcal{P}$ of analytic functions with positive real parts. The objective of this paper is to determine the radii such that the three classes are contained in various subclasses of starlike functions. The radii estimated in the present investigation are better than the radii obtained earlier. Furthermore, connections with previously known results are shown.

1. Introduction

Let $\Delta =\{z\in \mathbb{C}:|z|<1\}$ denote the open unit disc in $\mathbb{C}$ and the class $\mathcal{A}$ be defined as the collection of all analytic functions in $\Delta$ satisfying $f\left(0\right)=1$ and $f^{\prime }\left(0\right)=1$. The class $\mathcal{S}$ is defined to be a collection of univalent functions in class $\mathcal{A}$. The well-known Bieberbach theorem states that, for a univalent function $f\left(z\right)=z+a_2{z}^2+\dots$, the bound on the second coefficient, that is, $|a_2|\le 2$ plays an important role in the study of univalent function theory. This bound has attracted the interest of many mathematicians, which led to the investigation of the class $A_b$ consisting of the functions of the form $f\left(z\right)=z+a_2{z}^2+\dots$, $|a_2|=b$ for a fixed b with $0\le b\le 1$. For $n\in \mathbb{N}$ and $0\le b\le 1$, let ${\mathcal{A}}_{nb}$ be the class of analytic functions of the form $f\left(z\right)=z+nb{z}^2+\dots$ for $z\in \Delta$ such that ${\mathcal{A}}_b:={\mathcal{A}}_{1b}$.

The study of class $A_b$ was initiated as early as 1920 by Gronwall [1]. He determined the growth and distortion estimates for the class of univalent functions with fixed second coefficients. In 2011, Ali et al. [2] obtained various results for the class of functions with fixed second coefficients by applying the theory of second-order differential subordination. Later, Lee et al. [3] investigated certain applications of differential subordination for such functions. Kumar et al. [4] determined the best possible estimates on the initial coefficients of Ma-Minda type univalent functions; see also [5,6]. Ali et al. [7] obtained sharp radii of starlikeness for certain classes of functions with fixed second coefficients. A survey on functions with a fixed initial coefficient can be found in [8].

Let $\mathcal{P}\left(\alpha \right)$ denote the class of analytic functions $p\left(z\right)=1+b_{1}z+b_2{z}^2+\dots$ satisfying the condition $Re\left\{p\right(z\left)\right\}>\alpha$ for some $\alpha$ ($0\le \alpha <1$) and for all $z\in \Delta$. Recall that $\mathcal{P}=\mathcal{P}\left(0\right)$ is a well-known class of Carathéodory functions having a positive real part. It is well known that $|b_1|\le 2(1-\alpha )$; see, for example, [9]. For any two subclasses M and N of family $\mathcal{A}$ of all analytic functions of the form $f\left(z\right)=z+a_2{z}^2+\dots (z\in \Delta )$, the N-radius for the class M, denoted by $R_N\left(M\right),$ is the largest number $\rho \in (0,1)$ such that $r^{-1}f\left(rz\right)\in N,$ for all $f\in M$ and for $0<r<\rho .$ In [10,11,12], MacGregor found the radius of starlikeness for the class of functions f satisfying one of the conditions $Re\left(f\right(z)/z)>1/2$, $Re\left(f\right(z)/g(z\left)\right)>0$ and $|f^{\prime }\left(z\right)/g^{\prime }\left(z\right)-1|>0$ for some univalent function g. Recently, Anand et al. [13] determined various radii results for the class of functions f with fixed second coefficients and satisfying the conditions $Re\left(f\right(z)/g(z\left)\right)>0$, where either $g\left(z\right)=1+z$ or ${(1+z)}^2$. In recent years, several authors have studied radius problems involving ratios between functions belonging to two classes where one of them belong to some particular subclass of $\mathcal{A}$; for example, see [12,14,15,16,17,18]. Motivated by these studies and by making use of the classes ${\mathcal{A}}_{6b}$, ${\mathcal{A}}_{4c}$ and $\mathcal{P}$, we define the following classes:

$$H_{b,c}^1=\{f\in {\mathcal{A}}_{6b}:\frac{f}{g}\in \mathcal{P}\mathrm{and}\frac{g}{zp}\in \mathcal{P}\mathrm{where}g\in {\mathcal{A}}_{4c},p\in \mathcal{P}\}$$

$$H_{b,c}^2=\{f\in {\mathcal{A}}_{5b}:\frac{f}{g}\in \mathcal{P}\mathrm{and}\frac{g}{zp}\in \mathcal{P}(1/2)\mathrm{where}g\in {\mathcal{A}}_{3c},p\in \mathcal{P}\}$$

and

$$H_b^3=\{f\in {\mathcal{A}}_{4b}:\frac{f}{zp}\in \mathcal{P}\mathrm{where}p\in \mathcal{P}\}$$

where $b\in [0,1]$ and $c\in [0,1]$. By choosing suitable functions $p\left(z\right)$ in the class $\mathcal{P}$ and letting $b=1$ and $c=1$, we may obtain several well-known classes as special cases of our three classes; for example:

• For $p\left(z\right)=1/{(1+z)}^2$, Anand et al. [13] determined some sharp radius constants for $H_b^3$.
• For $p\left(z\right)=(1-z)/(1+z)$, the classes $H_{1,1}^1,H_{1,1}^2$ and $H_1^3$ yield the classes studied by Lecko et al. [14].
• Letting $p\left(z\right)=1/(1-z^2),1+z/2,1/(1-z^2)$ and $1/(1+z)$ in $H_{1,1}^1$ and $H_1^3$, we obtain the classes of functions studied in [19,20,21,22], respectively, for which various radius problems have been studied.
• Ali et al. [23] obtained certain a radius of starlikeness for the classes $H_{1,1}^1$ and $H_{1,1}^2$ with $p\left(z\right)=1$.

In Section 2, we obtain discs centred at 1 that contain the images of the unit disc $\Delta$ under the mapping $z{f}^{\prime }\left(z\right)/f\left(z\right)$ where f belongs to each of the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$. Using the results of Section 2, we then determine extensions of the radii estimates in [14] along with improved radii constants for functions in the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ to belong to several subclasses of $\mathcal{A}$, such as starlike functions of order $\alpha$, starlike functions associated with the lemniscate of Bernoulli, thereverse lemniscate, the sine function, the exponential function, the cardioid, the lune, the nephroid, a particular rational function, the modified sigmoid function and parabolic starlike functions.

2. Analysis and Mapping of ${\mathit{zf}}^{\prime }\left(\mathit{z}\right)/\mathit{f}\left(\mathit{z}\right)$ for ${\mathit{H}}_{\mathit{b},\mathit{c}}^{\mathbf{1}}$, ${\mathit{H}}_{\mathit{b},\mathit{c}}^{\mathbf{2}}$ and ${\mathit{H}}_{\mathit{b}}^{\mathbf{3}}$

In this section, we investigate extremal functions for all three classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$, which demonstrate the fact that the classes are non empty. Furthermore, we obtain discs centred at 1, containing the images of the disc $\Delta$ under the mapping $z{f}^{\prime }/f$, where f belongs to each of these classes. We begin by stating the following lemmas by McCarty:

Lemma 1 

([24]). Let $b\in [0,1]$ and $0\le \alpha <1$. If $p\in {\mathcal{P}}_b\left(\alpha \right)$, then, for $\left|z\right|=r<1$,

$$\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|\le \frac{2(1-\alpha )r}{1-r^2}\frac{b{r}^2+2r+b}{(1-2\alpha )r^2+2b(1-\alpha )r+1}.$$

Lemma 2 

([25]). Let $b\in [0,1]$ and $0\le \alpha <1$. If $p\in {\mathcal{P}}_b\left(\alpha \right)$, then, for $\left|z\right|=r<1$,

$$Re\left(\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right)\ge \left\{\begin{array}{c}\frac{-2(1-\alpha )r}{1+2\alpha br+(2\alpha -1)r^2}\frac{b{r}^2+2r+b}{r^2+2br+1}\mathrm{if}{R}_{\alpha }\le R_b\hfill \\ \frac{2\sqrt{\alpha C_1}-C_1-\alpha }{1-\alpha }\mathrm{if}{R}_{\alpha }\ge R_b\hfill \end{array}\right.,$$

where $R_b=C_b-D_b$, $R_{\alpha }=\sqrt{\alpha C_1}$ and $r=\left|z\right|<1$;

$$C_b=\frac{{(1+br)}^2-(2\alpha -1){(b+r)}^2{r}^2}{(1+2br+r^2)(1-r^2)}and{D}_b=\frac{2(1-\alpha )(b+r)(1+br)r}{(1+2br+r^2)(1-r^2)}$$

(1)

Lemma 3. 

Let $d=|6b-4c|\le 2$, $s=|4c-q|\le 2$, $b\in [0,1]$ and $c\in [0,1]$. If $f\in H_{b,c}^1$, then, for $\left|z\right|=r<1$,

$$\begin{gathered} \left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r[(d{r}^2+4r+d)(r^2+sr+1)(r^2+qr+1)+(s{r}^2+4r+s) \\ (r^2+dr+1)(r^2+qr+1)+(q{r}^2+4r+q)(r^2+dr+1)(r^2+sr+1)]}{(1-r^2)(r^2+dr+1)(r^2+sr+1)(r^2+qr+1)}. \end{gathered}$$

Furthermore, the class $H_{b,c}^1$ is non-empty.

Proof. 

Let the functions f and g whose Taylor series expansions are given by $f\left(z\right)=z+f_1{z}^2+\dots$ and $g\left(z\right)=z+g_1{z}^2+\dots$ be such that $Re\{f/g\}>0$ and $Re\{g/(zp\left)\right\}>0$, where $p\in \mathcal{P}$ is represented by $p\left(z\right)=1+qz+\dots$. Now, consider, $g/\left(zp\right)=1+(g_1-q)z+\dots$, where $|g_1-q|\le 2$ and $\left|q\right|\le 2$, which gives $|g_1|\le 4.$ Furthermore, $f/g=1+(f_1-g_1)z+\dots ,$ where $|f_1-g_1|\le 2$, and hence $|f_1|\le 6$. Thus, for $b,c\in [0,1]$, we consider the class involving the functions f and g with fixed second coefficients whose Taylor series expansions are given by $f\left(z\right)=z+6b{z}^2+\dots$ and $g\left(z\right)=z+4c{z}^2+\dots$ such that $f\in {\mathcal{A}}_{6b}$ and $g\in {\mathcal{A}}_{4c}$. If the function $f\in$$H_{b,c}^1$, then there exists an element $g\in {\mathcal{A}}_{4c}$ and $p\in \mathcal{P}$ such that $f/g\in \mathcal{P}$ and $g/\left(zp\right)\in \mathcal{P}$. Define

$$h\left(z\right)=\frac{f\left(z\right)}{g\left(z\right)}=1+(6b-4c)z+\dots \mathrm{and}k\left(z\right)=\frac{g\left(z\right)}{zp\left(z\right)}=1+(4c-q)z+\dots ,$$

where $|6b-4c|\le 2$ and $|4c-q|\le 2$. Therefore, we observe that $h\in {\mathcal{P}}_{(6b-4c)/2}$, $k\in {\mathcal{P}}_{(4c-q)/2}$, $p\in {\mathcal{P}}_{q/2}$, and f can be expressed as $f\left(z\right)=zp\left(z\right)h\left(z\right)k\left(z\right)$. Then, a calculation shows that

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|+\left|\frac{z{k}^{\prime }\left(z\right)}{k\left(z\right)}\right|+\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|.$$

(2)

For $d=|6b-4c|\le 2$, $s=|4c-q|\le 2$ and $\alpha =0$, using Lemma 1, we obtain

$$\left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(d{r}^2+4r+d)}{(r^2+dr+1)},\left|\frac{z{k}^{\prime }\left(z\right)}{k\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(s{r}^2+4r+s)}{(r^2+sr+1)},$$

(3)

and

$$\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(q{r}^2+4r+q)}{(r^2+qr+1)}.$$

(4)

By (2), (3) and (4), it follows that

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r[(d{r}^2+4r+d)(r^2+sr+1)(r^2+qr+1)+(s{r}^2+4r+s)(r^2+dr+1)(r^2+qr+1)+(q{r}^2+4r+q)(r^2+dr+1)(r^2+sr+1)]}{(1-r^2)(r^2+dr+1)(r^2+sr+1)(r^2+qr+1)}.$$

(5)

Define the functions $f_1$, $g_1$ and $p_1:\Delta \to \mathbb{C}$ by

$$f_1\left(z\right)=\frac{z(1-qz+z^2)(1-(4c-q)z+z^2)(1-(6b-4c)z+z^2)}{{(1-z^2)}^3},$$

(6)

$$g_1\left(z\right)=\frac{z(1-qz+z^2)(1-(4c-q)z+z^2)}{{(1-z^2)}^2},\mathrm{and}{p}_1\left(z\right)=\frac{(1-qz+z^2)}{(1-z^2)},$$

(7)

$$\mathrm{where}|6b-4c|\le 2,|4c-q|\le 2\mathrm{and}\left|q\right|\le 2.$$

By (6) and (7), we have

$$\frac{f_1\left(z\right)}{g_1\left(z\right)}=\frac{(1-(6b-4c)z+z^2)}{(1-z^2)}=\frac{1+w_1\left(z\right)}{1-w_1\left(z\right)},$$

$$\frac{g_1\left(z\right)}{z{p}_1\left(z\right)}=\frac{(1-(4c-q)z+z^2)}{(1-z^2)}=\frac{1+w_2\left(z\right)}{1-w_2\left(z\right)}\mathrm{and}{p}_1\left(z\right)=\frac{(1-qz+z^2)}{(1-z^2)}=\frac{1+w_3\left(z\right)}{1-w_3\left(z\right)},$$

where

$$w_1\left(z\right)=\frac{z(z-(6b-4c)/2)}{(1-z(6b-4c)/2)},w_2\left(z\right)=\frac{z(z-(4c-q)/2)}{(1-z(4c-q)/2)}\mathrm{and}{w}_3\left(z\right)=\frac{z(z-q/2)}{(1-zq/2)},$$

which are analytic functions satisfying the conditions of the Schwarz lemma in $\Delta$; hence, $Re(f_1/g_1)>0$, $Re(g_1/\left(z{p}_1\right))>0$, and $Re\left(p_1\right)>0$. Thus, $f_1/g_1\in {\mathcal{P}}_{(6b-4c)/2}$, $g_1/\left(z{p}_1\right)\in {\mathcal{P}}_{(4c-q)/2}$ and $p_1\in {\mathcal{P}}_{(q/2)}$. Thus, the function $f_1\in H_{b,c}^1$, and the class $H_{b,c}^1$ is non-empty. The functions

$$F_1\left(z\right)=\frac{z{(1-z^2)}^3}{(1-qz+z^2)(1-(4c-q)z+z^2)(1-(6b-4c)z+z^2)}$$

(8)

and $f_1$ are extreme functions for the class $H_{b,c}^1$ provided $q\le 2,c\ge q/4$ and $b\ge 2c/3.$ □

Lemma 4. 

Let $m=|5b-3c|\le 2$, $n=|3c-q|\le 1$, $b\in [0,1]$ and $c\in [0,1]$. If $f\in H_{b,c}^2$, then, for $\left|z\right|=r<1$,

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right).$$

Furthermore, the class $H_{b,c}^2$ is non-empty.

Proof. 

Let f and g be functions given by $f\left(z\right)=z+f_1{z}^2+\dots$ and $g\left(z\right)=z+g_1{z}^2+\dots$ such that $Re\{f/g\}>0$ and $Re\{g/(zp\left)\right\}>1/2$, where $p\in \mathcal{P}$ is represented by $p\left(z\right)=1+qz+\dots$. Now, consider $g/zp=1+(g_1-q)z+\dots$, where $|g_1-q|\le 1$ (Lemma 2, pg 33 et al. [26]) and $\left|q\right|\le 2$, which gives $|g_1|\le 3.$ Furthermore, $f/g=1+(f_1-g_1)z+\dots$, where $|f_1-g_1|\le 2$, and hence $|f_1|\le 5$. Therefore, we consider the class involving the functions f and g with fixed second coefficients whose Taylor series expansions are given by $f\left(z\right)=z+5b{z}^2+\dots$ and $g\left(z\right)=z+3c{z}^2+\dots$ where $b\in [0,1]$ and $c\in [0,1]$ such that $f\in {\mathcal{A}}_{5b}$ and $g\in {\mathcal{A}}_{3c}$. If the function $f\in$$H_{b,c}^2$, then there exists an element $g\in {\mathcal{A}}_{3c}$ and $p\in \mathcal{P}$ such that $f/g\in \mathcal{P}$ and $g/\left(zp\right)\in \mathcal{P}(1/2).$ Define

$$h\left(z\right)=\frac{f\left(z\right)}{g\left(z\right)}=1+(5b-3c)z+\dots$$

and

$$k\left(z\right)=\frac{g\left(z\right)}{zp\left(z\right)}=1+(3c-q)z+\dots .$$

It is easy to see that $h\in {\mathcal{P}}_{(5b-3c)/2}$, $k\in {\mathcal{P}}_{(3c-q)}$, $p\in P_{q/2}$, and $f\left(z\right)=zp\left(z\right)h\left(z\right)k\left(z\right)$. Then, a calculation shows that

$$\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}=1+\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}+\frac{z{k}^{\prime }\left(z\right)}{k\left(z\right)}+\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}$$

(9)

so that

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|+\left|\frac{z{k}^{\prime }\left(z\right)}{k\left(z\right)}\right|+\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|.$$

(10)

Let $m=|5b-3c|\le 2$, $n=|3c-q|\le 1$ and $\alpha =0$. Using Lemma 1 for the functions $h,p$ and k, we have

$$\left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(m{r}^2+4r+m)}{(r^2+mr+1)},\left|\frac{z{k}^{\prime }\left(z\right)}{k\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(n{r}^2+2r+n)}{(nr+1)},$$

(11)

and

$$\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(q{r}^2+4r+q)}{(r^2+qr+1)}.$$

(12)

Inequality (10) together with (11) and (12) gives

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right).$$

(13)

Define the functions $f_2$, $g_2$$:\Delta \to \mathbb{C}$ by

$$f_2\left(z\right)=\frac{z(1-qz+z^2)(1-(3c-q)z)(1-(5b-3c)z+z^2)}{{(1-z^2)}^3},$$

(14)

and

$$g_2\left(z\right)=\frac{z(1-qz+z^2)(1-(3c-q)z)}{{(1-z^2)}^2},\mathrm{where}|5b-3c|\le 2\mathrm{and}|3c-q|\le 1.$$

(15)

It follows from (7), (14) and (15) that

$$\frac{f_2\left(z\right)}{g_2\left(z\right)}=\frac{(1-(5b-3c)z+z^2)}{(1-z^2)}=\frac{1+w_4\left(z\right)}{1-w_4\left(z\right)},$$

and

$$\frac{g_2\left(z\right)}{z{p}_1\left(z\right)}=\frac{(1-(3c-q\left)z\right)}{(1-z^2)}=\frac{1+w_5\left(z\right)}{1-w_5\left(z\right)},$$

where

$$w_4\left(z\right)=\frac{z(z-(5b-3c)/2)}{(1-z(5b-3c)/2)}\mathrm{and}{w}_5\left(z\right)=\frac{z(z-(3c-q\left)\right)}{(2-(3c-q)z-z^2)}$$

are Schwarz functions in the unit disc $\Delta$, and hence $Re(f_2/g_2)>0$, $Re(g_2/\left(z{p}_1\right))>1/2$ and $Re\left(p_1\right)>0$ (as shown for class $H_{b,c}^1$). Thus, $f_2/g_2\in {\mathcal{P}}_{(5b-3c)/2}$, $g_2/\left(z{p}_1\right)\in {\mathcal{P}}_{(3c-q)}$, and $p_1\in {\mathcal{P}}_{(q/2)}$. Hence, $f_2\in H_{b,c}^2$, and the class $H_{b,c}^2$ is non-empty. Furthermore, the function $f_2$ is an extreme function for the class $H_{b,c}^2$ provided $q\le 2$, $c\ge q/3$ and $b\ge 3c/5.$ □

Lemma 5. 

Let $l=|4b-q|\le 2,\left|q\right|\le 2$ and $b\in [0,1]$. If $f\in H_b^3$, then, for $\left|z\right|=r<1$,

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r[(l{r}^2+4r+l)(q{r}^2+4r+q)+(q{r}^2+4r+q)(r^2+lr+1)]}{(1-r^2)(r^2+lr+1)(r^2+qr+1)}.$$

Furthermore, the class $H_b^3$ is non-empty.

Proof. 

Let the functions f and g whose Taylor series expansions are given by $f\left(z\right)=z+f_1{z}^2+..$ be such that $Re\{f/(zp\left)\right\}>0$, where $p\in \mathcal{P}$ is represented by $p\left(z\right)=1+qz+\dots$. Now, consider $f/\left(zp\right)=1+(f_1-q)z+\dots$, where $|f_1-q|\le 2$ and $\left|q\right|\le 2$, which gives $|f_1|\le 4.$ Therefore, we consider the function f with a fixed second coefficient whose Taylor series expansion is given by $f\left(z\right)=z+4b{z}^2+\dots$ where $b\in [0,1]$ such that $f\in {\mathcal{A}}_{4b}$. If the function $f\in$$H_b^3$, then there exists $p\in \mathcal{P}$ such that $f/\left(zp\right)\in \mathcal{P}$. Define the function

$$h\left(z\right)=\frac{f}{zp}\left(z\right)=1+(4b-q)z+\dots$$

so that $h\in {\mathcal{P}}_{(4b-q)/2}$, $p\in {\mathcal{P}}_{q/2}$ and f can be expressed as $f\left(z\right)=zp\left(z\right)h\left(z\right)$. Then, a calculation shows that

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|+\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|.$$

(16)

From Lemma 1, for $l=|4b-q|\le 2,\left|q\right|\le 2$ and $\alpha =0$, we obtain

$$\left|\frac{z{h}^{\prime }\left(z\right)}{h\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(l{r}^2+4r+l)}{(r^2+lr+1)}\mathrm{and}\left|\frac{z{p}^{\prime }\left(z\right)}{p\left(z\right)}\right|\le \frac{r}{(1-r^2)}\frac{(q{r}^2+4r+q)}{(r^2+qr+1)}.$$

(17)

Using (16) and (17), it is easy to show that

$$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r[(l{r}^2+4r+l)(q{r}^2+4r+q)+(q{r}^2+4r+q)(r^2+lr+1)]}{(1-r^2)(r^2+lr+1)(r^2+qr+1)}.$$

(18)

Define the functions $f_3:\Delta \to \mathbb{C}$ by

$$f_3\left(z\right)=\frac{z(1-qz+z^2)(1-(4b-q)z+z^2)}{{(1-z^2)}^2},|4b-q|\le 2.$$

(19)

Then, (17) together with (7) gives

$$\frac{f_3\left(z\right)}{z{p}_1\left(z\right)}=\frac{(1-(4b-q)z+z^2)}{(1-z^2)}=\frac{1+w_6\left(z\right)}{1-w_6\left(z\right)},\mathrm{where}{w}_6\left(z\right)=\frac{z(z-(4b-q)/2)}{(1-z(4b-q)/2)},$$

which is an analytic function satisfying the conditions of the Schwarz lemma in $\Delta$, and hence $Re(f_3/\left(z{p}_1\right))>0$ and $Re\left(p_1\right)>0$ (shown above in class $H_{b,c}^1$). Thus, $f_3/\left(z{p}_1\right)\in {\mathcal{P}}_{(6b-q)/2}$ and $p_1\in {\mathcal{P}}_{(q/2)}$. Thus, the function $f_3\in H_b^3$, and the class $H_b^3$ is non-empty. Furthermore, the functions given by

$$F_3\left(z\right)=\frac{z{(1-z^2)}^2}{(1-qz+z^2)(1-(4b-q)z+z^2)},|4b-q|\le 2$$

(20)

and $f_3$ are extreme functions for the class $H_b^3$ provided $q\le 2$ and $b\ge q/4.$ □

3. Radius of Starlikeness

Using the information in the previous sections, we now investigate several radius problems associated with the functions in the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$. In particular, we determine sharp estimates of the radii constants $R_N\left(H_{b,c}^1\right)$, $R_N\left(H_{b,c}^2\right)$ and $R_N\left(H_b^3\right)$, where N is one of the classes of starlike functions mentioned in Section 1 that can be obtained from the Ma and Minda [10] class ${\mathcal{S}}^{*}\left(\psi \right)$ given by

$${\mathcal{S}}^{*}\left(\psi \right)=\{f\in \mathcal{A}:z{f}^{\prime }\left(z\right)/f\left(z\right)\prec \psi \left(z\right)\}.$$

Here, ≺ is the usual notation for subordination, and $\psi$ is an analytic and univalent function with a positive real part in $\Delta$ with $\psi \left(0\right)=1$. ${\psi }^{\prime }\left(0\right)>0$ and $\psi$ maps $\Delta$ onto a region that is starlike with respect to 1 and symmetric with respect to the real axis. Recently, Anand et al. [27] obtained results for a class of analytic functions defined using the function $\psi$. Throughout this section, we assume that $d=|6b-4c|\le 2$, $s=|4c-q|\le 2$, $m=|5b-3c|\le 2$, $n=|3c-q|\le 1$, and $l=|4b-q|\le 2$.

For $0\le \alpha \le 1$, the class ${\mathcal{S}}^{*}\left(\alpha \right)={\mathcal{S}}^{*}[1-2\alpha ,-1]$ = $\{f\in \mathcal{A}:Re(z{f}^{\prime }\left(z\right)/f\left(z\right))>\alpha \}$ is the class of starlike functions of order $\alpha$. In our first main theorem, we determine sharp estimates of the radii constants $R_{{\mathcal{S}}^{*}\left(\alpha \right)}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}^{*}\left(\alpha \right)}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}^{*}\left(\alpha \right)}\left(H_b^3\right)$.

Theorem 1. 

The sharp ${\mathcal{S}}^{*}\left(\alpha \right)$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}^{*}\left(\alpha \right)$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=\alpha -1+\alpha (d+s+q)r+(10+2\alpha +ds+\alpha ds+(1+\alpha )(d+s)q)r^2+((10+\alpha )(s+q)+d(10+\alpha +(2+\alpha )sq))r^3+8(3+sq+d(s+q))r^4+(-(-12+\alpha )(s+q)-d(-12+\alpha +(-4+\alpha )sq))r^5+(14+3sq+3d(s+q)-\alpha (2+sq+d(s+q)))r^6-(-2+\alpha )(d+s+q)r^7+(1-\alpha )r^8$.

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}^{*}\left(\alpha \right)$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$$x_2\left(r\right)=-\frac{4}{1-r^2}-\frac{1}{1+nr}+\frac{2+2nr}{1+2nr+r^2}+\frac{2+mr}{1+r(m+r)}+\frac{2+qr}{1+r(q+r)}-\alpha .$$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}^{*}\left(\alpha \right)$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=\alpha -1+\alpha (l+q)r+(7+\alpha +(1+\alpha )lq)r^2+6(l+q)r^3+(9+3lq-\alpha (1+lq))r^4-(-2+\alpha )(l+q)r^5+(1-\alpha )r^6$.

Proof. 

The radii estimates for their respective classes are found as follows:

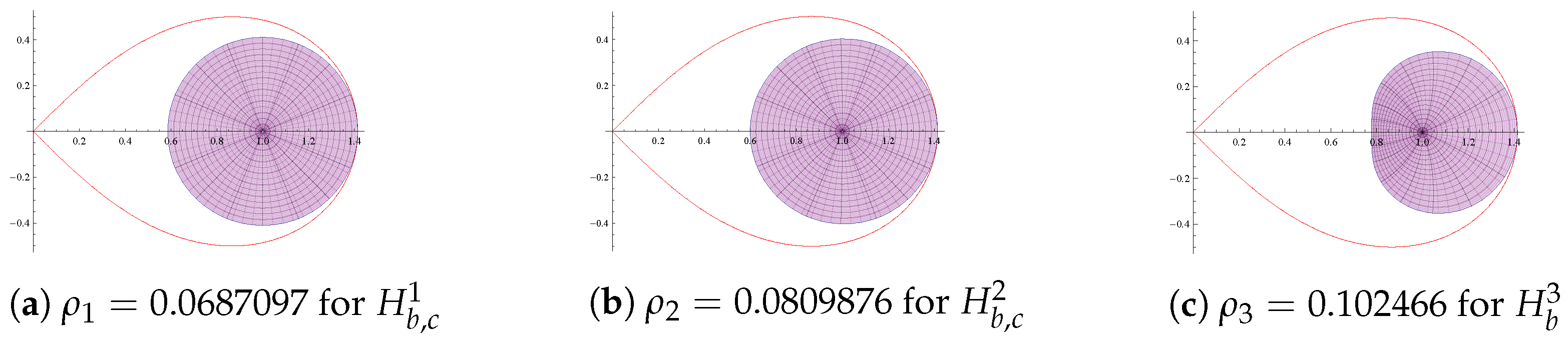
• Note that $x_1\left(0\right)=(\alpha -1)<0$ and $x_1\left(1\right)=6(2+d)(2+e)(2+q)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. For $f\in H_{b,c}^1$, using (5), we have

  $$\left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right),$$

  (21)

  which yields

  $$\begin{array}{cc}\hfill Re\left(\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}\right)& \ge 1-\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\hfill \\ \hfill & \ge \alpha \hfill \end{array}$$

  (22)

  whenever $x_1\left(r\right)\le 0$. This shows that $Re(z{f}^{\prime }\left(z\right)/f\left(z\right))\ge \alpha$ for $\left|z\right|=r\le {\rho }_1$.
  For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (22), the function $F_1\left(z\right)$, defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the equality

  $$\begin{array}{cc}\hfill Re\left(\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}\right)& =1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\hfill \\ \hfill & =\alpha .\hfill \end{array}$$
  This proves that the radius is sharp.
• A calculation shows that $x_2\left(0\right)=1-\alpha >0$ and $x_2(1/3)=-(-60(-5+(-12+n)n)+6(135+n(148+21n))q+3m(9(30+17q)+n(296+42n+156q+27nq))+2(10+3m)(3+n)(5+3n)(10+3q)\alpha )/\left(2(10+3m)(3+n)(5+3n)(10+3q)\right)<0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1/3)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1/3)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. An easy calculation shows that, for $0<r<1/3$,

  $$C_b-D_b-\sqrt{C_1/2}=\frac{-1+4r^2+2b^2{r}^2+8b{r}^3+r^4+2b^2{r}^4}{2(-1+r)(1+r){\left(1+2br+r^2\right)}^2}>0,$$

  (23)

  where $C_b$ and $D_b$ are given by (1). From (9) and (11) and using Lemma 2 together with (23), we have

  $$\begin{array}{cc}\hfill & Re\left(\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}\right)\hfill \\ \hfill & \ge 1-\left(\frac{r}{1-r^2}\right)\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)-\frac{r\left(n+2r+n{r}^2\right)}{(1+nr)\left(1+2nr+r^2\right)}\ge \alpha \hfill \end{array}$$

  (24)

  whenever $x_2\left(r\right)\le 0$. Thus, $Re(z{f}^{\prime }\left(z\right)/f\left(z\right))\ge \alpha$ for $\left|z\right|=r\le {\rho }_2$. To prove the sharpness, consider the function $F_2,G_2:\Delta \to \mathbb{C}$ defined by

  $$F_2\left(z\right)=\frac{z(1+3cz-qz){(1-z^2)}^2}{(1+5bz-3cz+z^2)(1+6cz-2qz+z^2)(1+qz+z^2)}$$

  and

  $$G_2\left(z\right)=z\frac{1-z^2}{(1+qz+z^2)(1+3cz-qz)},$$

  where $|5b-3c|\le 2$ and $|3c-q|\le 1.$ Note that, for $c=(1+q)/3$,

  $$\frac{F_2\left(z\right)}{G_2\left(z\right)}=\frac{(1-z^2)}{1-(1-5b+q)z+z^2}=\frac{1+w_1\left(z\right)}{1-w_1\left(z\right)}$$

  and

  $$\frac{G_2\left(z\right)}{z{p}_1\left(z\right)}=\frac{1}{1+3cz-qz}=\frac{1+w_2\left(z\right)}{1-w_2\left(z\right)},$$

  where

  $$w_1\left(z\right)=\frac{(1-5b+q-2z)z}{2+(-1+5b-q)z}\mathrm{and}{w}_2\left(z\right)=\frac{(q-3c)z}{2+(3c-q)z}$$

  are Schwarz functions; hence, $Re(F_2/G_2)>0$, $Re(G_2/\left(z{p}_1\right))>0$, and $Re\left(p_1\right)>0$. For $5b-3c\ge 0$, $3c-q=1$ and $z={\rho }_2$, it follows from (24) that

  $$\begin{array}{cc}\hfill & Re\left(\frac{z{F}_2^{\prime }\left(z\right)}{F_2\left(z\right)}\right)\hfill \\ & =1-\left(\frac{{\rho }_2}{1-{\rho }_2^2}\right)\left(\frac{m{\rho }_2^2+4{\rho }_2+m}{{\rho }_2^2+m{\rho }_2+1}+\frac{q{\rho }_2^2+4{\rho }_2+q}{{\rho }_2^2+q{\rho }_2+1}\right)-\frac{{\rho }_2\left(n+2{\rho }_2+n{\rho }_2^2\right)}{(1+n{\rho }_2)\left(1+2n{\rho }_2+{\rho }_2^2\right)}\hfill \\ \hfill & =\alpha .\hfill \end{array}$$
• It is easy to see that $x_3\left(0\right)=\alpha -1<0$ and $x_3\left(1\right)=4(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. From (18), it follows that, for any $f\in H_b^3$,

  $$\begin{array}{cc}\hfill Re\left(\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}\right)& \ge 1-\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\ge \alpha \hfill \end{array}$$

  (25)

  whenever $x_3\left(r\right)\le 0$. This proves that $Re(z{f}^{\prime }\left(z\right)/f\left(z\right))\ge \alpha$ for $\left|z\right|=r\le {\rho }_3$. The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (25) that

  $$\begin{array}{cc}\hfill Re\left(\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right)& =1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)=\alpha .\hfill \end{array}$$

□

Remark 1. 

Figure 1 represents that the ${\mathcal{S}}^{*}\left(\frac{1}{2}\right)$ radii estimated for all three classes are sharp.

Remark 2. 

For $b=1$, $c=1$ and $q=2$, Theorem 1 yields the corresponding result determined in (Theorem 1, p. 6, [14]).

Placing $\alpha =0$ in Theorem 1, we obtain the radius of starlikeness for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$.

Corollary 1. 

The sharp ${\mathcal{S}}^{*}:={\mathcal{S}}^{*}\left(0\right)$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=-1+(10+qs+d(q+s))r^2+2(5(q+s)+d(5+qs))r^3+8(3+qs+d(q+s))r^4+4(3(q+s)+d(3+qs))r^5+(14+3qs+3d(q+s))r^6+2(d+q+s)r^7+r^8.$

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$$x_2\left(r\right)=-\frac{4}{1-r^2}-\frac{1}{1+nr}+\frac{2+2nr}{1+2nr+r^2}+\frac{2+mr}{1+r(m+r)}+\frac{2+qr}{1+r(q+r)}.$$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=-1+(7+lq)r^2+6(l+q)r^3+(9+3lq)r^4+2(l+q)r^5+r^6$.

The class ${\mathcal{S}}_L^{*}$=${\mathcal{S}}^{*}\left(\sqrt{1+z}\right)$ is another class that can be obtained from the Ma-Minda class. It represents the collection of functions in the class $\mathcal{A}$ whose $z{f}^{\prime }\left(z\right)/f\left(z\right)$ lies in the region bounded by the lemniscate of Bernoulli $|w^2-1|=1.$ Various studies on ${\mathcal{S}}_L^{*}$ can be seen in [28,29]. In the following result, we obtain the sharp radii constants $R_{{\mathcal{S}}_L^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_L^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_L^{*}}\left(H_b^3\right)$.

Theorem 2. 

The sharp ${\mathcal{S}}_L^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_L^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=(1-\sqrt{2})-(-2+\sqrt{2})(d+s+q)r+(-2(-7+\sqrt{2})-(-3+\sqrt{2})(sq+d(s+q)))r^2+(-(-12+\sqrt{2})(s+q)-d(-12+\sqrt{2}+(-4+\sqrt{2})sq))r^3+8(3+sq+d(s+q))r^4+((10+\sqrt{2})(s+q)+d(10+\sqrt{2}+(2+\sqrt{2})sq))r^5+(2(5+\sqrt{2})+(1+\sqrt{2})(sq+d(s+q)))r^6+\sqrt{2}(d+s+q)r^7+(-1+\sqrt{2})r^8$.

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}_L^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=(1-\sqrt{2})-(-2+\sqrt{2})(m+n+q)r+(11-\sqrt{2}-(-3+\sqrt{2})(nq+m(n+q)))r^2+(8(m+q)-n(-12+\sqrt{2}+(-4+\sqrt{2})mq))r^3+(11+\sqrt{2}+(3+\sqrt{2})mq+8n(m+q))r^4+((10+\sqrt{2})n+(2+\sqrt{2})q+(2+\sqrt{2})m(1+nq))r^5+(1+\sqrt{2})(1+n(m+q))r^6+\sqrt{2}n{r}^7$.

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_L^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=(1-\sqrt{2})-(-2+\sqrt{2})(l+q)r+(9-\sqrt{2}-(-3+\sqrt{2})lq)r^2+6(l+q)r^3+(7+\sqrt{2}+(1+\sqrt{2})lq)r^4+\sqrt{2}(l+q)r^5+(-1+\sqrt{2})r^6$.

Proof. 

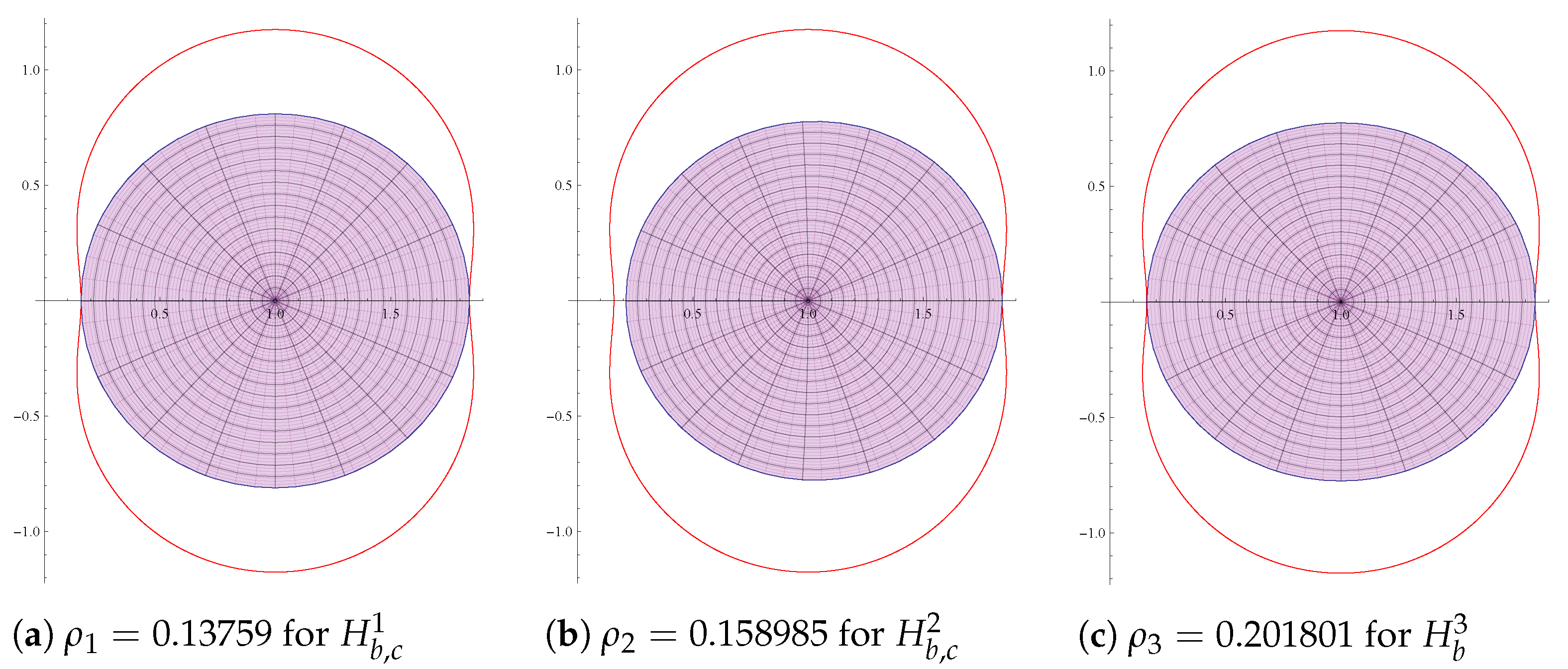
• Note that $x_1\left(0\right)=(1-\sqrt{2})<0$ and $x_1\left(1\right)=6(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. Ali et al. [30] (Lemma 2.2) proved that, for $2\sqrt{2}/3<C<\sqrt{2},$

  $$\left\{w\in \mathbb{C}:|w-C|<\sqrt{2}-C\right\}\subset \left\{w\in \mathbb{C}:|w^2-1|<1\right\}.$$

  (26)
  In view of (26) and the fact that the centre of the disc in (21) is 1, $f\in {\mathcal{S}}_L^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \sqrt{2}-1,$$

  (27)

  which is equivalent to $f\in {\mathcal{S}}_L^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_L^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (27), the function $f_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (6) at $z=-{\rho }_1$ satisfies the following equality

  $$\begin{array}{cc}\hfill & \left|{\left(\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}\right)}^2-1\right|\hfill \\ \hfill & =\left|{\left(1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right)}^2-1\right|=1.\hfill \end{array}$$
  Thus, the radius is sharp.
• A calculation shows that $x_2\left(0\right)=1-\sqrt{2}<0$ and $x_2\left(1\right)=6(2+m)(1+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$, and $f\in H_{b,c}^2$. As the centre of the disc in (13) is 1, by (26), $f\in {\mathcal{S}}_L^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \sqrt{2}-1,$$

  (28)

  which is equivalent to $f\in {\mathcal{S}}_L^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_L^{*}$ for $\left|z\right|=r\le {\rho }_2$. To prove the sharpness, consider the function $f_2$ defined in (14). For $u=5b-3c\ge 0$, $v=3c-q\ge 0$ and $z=-{\rho }_2$, it follows from (28) that

  $$\begin{array}{cc}\hfill & \left|{\left(\frac{z{f}_2^{\prime }\left(z\right)}{f_2\left(z\right)}\right)}^2-1\right|\hfill \\ \hfill & =\left|{\left(1-\frac{{\rho }_2}{(1-{\rho }_2^2)}\left(\frac{u{\rho }_2^2+4{\rho }_2+u}{{\rho }_2^2+u{\rho }_2+1}+\frac{v{\rho }_2^2+2{\rho }_2+v}{v{\rho }_2+1}+\frac{q{\rho }_2^2+4{\rho }_2+q}{{\rho }_2^2+q{\rho }_2+1}\right)\right)}^2-1\right|=1.\hfill \end{array}$$
• It is easy to see that $x_3\left(0\right)=1-\sqrt{2}<0$ and $x_3\left(1\right)=4(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. From (16) and (17) it follows that, for any $f\in H_b^3$

  $$\begin{array}{c}\hfill \left|\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}-1\right|\le \frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right).\end{array}$$

  (29)
  As the centre of the disc in (29) is 1, by (26), $f\in {\mathcal{S}}_L^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \sqrt{2}-1,$$

  (30)

  which is equivalent to $f\in {\mathcal{S}}_L^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3)$. This proves that $f\in {\mathcal{S}}_L^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $f_3$ defined for the class $H_b^3$ in (19). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (30) that

  $$\begin{array}{cc}\hfill & \left|{\left(\frac{z{f}_3^{\prime }\left(z\right)}{f_3\left(z\right)}\right)}^2-1\right|=\left|{\left(\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right)}^2-1\right|=1.\hfill \end{array}$$

□

Remark 3. 

Figure 2 represents extreme ${\mathcal{S}}_L^{*}$ radii estimated for all three classes.

Remark 4. 

For $b=1$, $c=1$ and $q=2$, Theorem 2 yields the corresponding result determined in (Theorem 2, p. 8, [14]).

For ${\varphi }_{PAR}=1+\frac{2}{{\pi }^2}{\left(log\left({\displaystyle \frac{1+\sqrt{z}}{1-\sqrt{z}}}\right)\right)}^2$, the class ${\mathcal{S}}_p^{*}:={\mathcal{S}}^{*}\left({\varphi }_{PAR}\right)$ is the class of parabolic starlike functions. A function $f\in {\mathcal{S}}_p^{*}$ provided $z{f}^{\prime }\left(z\right)/f\left(z\right)$ lies in the parabolic region given by $\{w\in \mathbb{C}:Re(w)>|w-1\left|\right\}$. For further reading, refer to [11,31,32,33]. The following theorem gives the sharp radii constants $R_{{\mathcal{S}}_p^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_p^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_p^{*}}\left(H_b^3\right)$.

Theorem 3. 

The sharp ${\mathcal{S}}_p^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_p^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=-1+(d+s+q)r+(22+3sq+3d(s+q))r^2+(21(s+q)+d(21+5sq))r^3+16(3+sq+d(s+q))r^4+(23(s+q)+d(23+7sq))r^5+(26+5sq+5d(s+q))r^6+3(d+s+q)r^7+r^8.$

2. 

For the class $H_{b,c}^2$, ${\mathcal{S}}_p^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=-1+(m+q)r+(18+4mn+3mq+4nq)r^2+(17m+36n+17q+8mnq)r^3+(40+28mn+12mq+28nq)r^4+(19m+40n+19q+12mnq)r^5+(22+8mn+5mq+8nq)r^6+(3m+4n+3q)r^7+r^8.$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_p^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=-1+(l+q)r+3(5+lq)r^2+12(l+q)r^3+(17+5lq)r^4+3(l+q)r^5+r^6.$

Proof. 

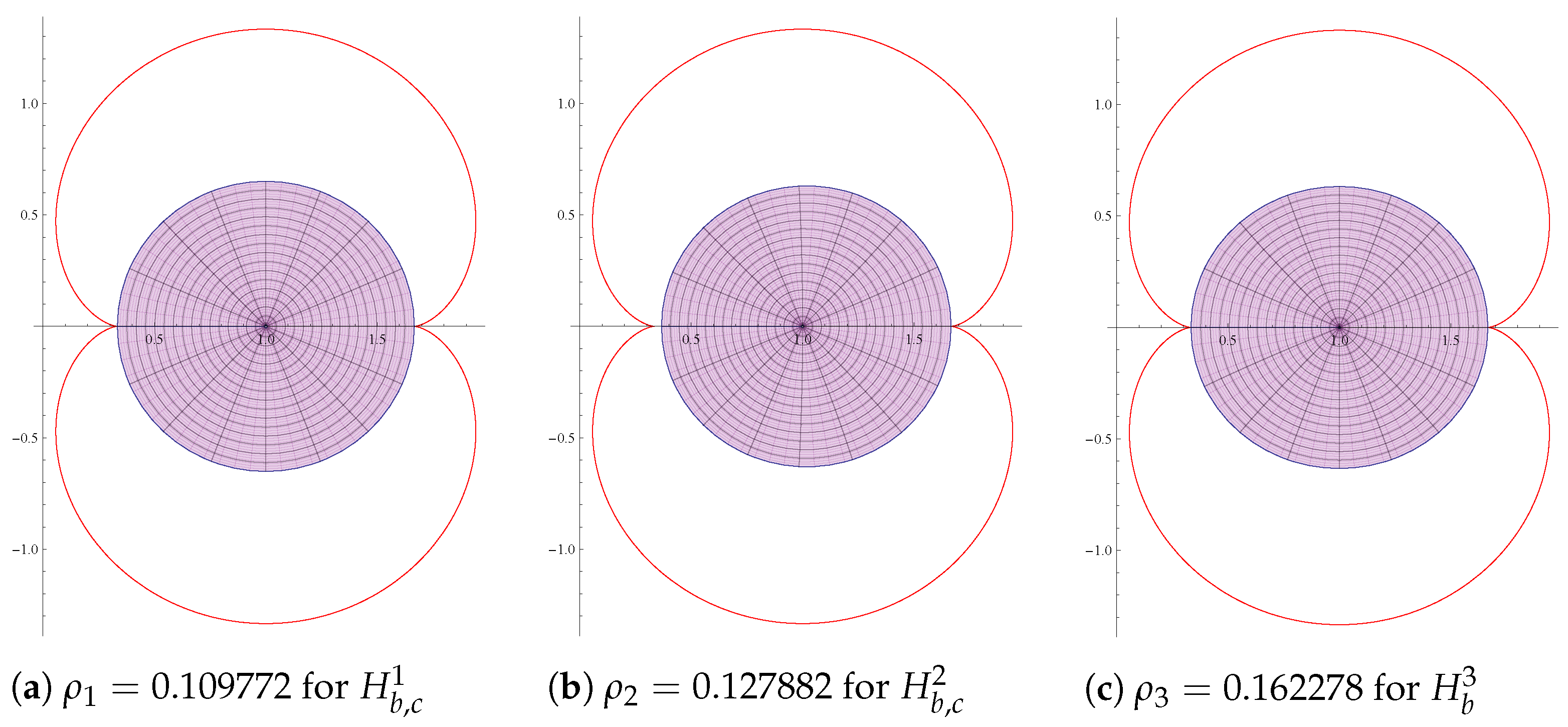
• Note that $x_1\left(0\right)=-1<0$ and $x_1\left(1\right)=12(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. Shanmughan and Ravichandran (p. 321, [34]) proved, for $1/2<C<3/2,$ that

  $$\{w\in \mathbb{C}:|w-C|<C-1/2\}\subset \{w\in \mathbb{C}:Re(w)>|w-1\left|\right\}.$$

  (31)
  As the centre of the disc in (21) is 1, by (31), $f\in {\mathcal{S}}_p^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{1}{2},$$

  (32)

  which is equivalent to $f\in {\mathcal{S}}_p^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this, $f\in {\mathcal{S}}_p^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (32), the function $F_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{cc}\hfill Re\left(\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}\right)& =\left|1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right|\hfill \\ \hfill & =\left|\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}-1\right|.\hfill \end{array}$$
  This proves that the radius is sharp.
• A calculation shows that $x_2\left(0\right)=-1<0$ and

  $$x_2\left(\frac{1}{3}\right)=\frac{4\left(9m\right(190+89q+n(146+63q))+2(1250+855q+3n(410+219q)\left)\right)}{6561}$$
  , which is greater than 0. By the Intermediate Value Theorem, there exists a root $r\in (0,1/3)$ of the equation $x_2\left(r\right)$. Let ${\rho }_2\in (0,1/3)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. From (9) and (11) and using Lemma 2 together with (23), we have

  $$\begin{array}{cc}\hfill & Re\left(\frac{z{f}^{\prime }\left(z\right)}{f\left(z\right)}\right)\hfill \\ \hfill & \ge 1-\left(\frac{r}{1-r^2}\right)\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)-\frac{r\left(n+2r+n{r}^2\right)}{(1+nr)\left(1+2nr+r^2\right)}\hfill \\ \hfill & \ge \frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\ge \left|\frac{z{f}_2^{\prime }\left(z\right)}{f_2\left(z\right)}-1\right|\hfill \end{array}$$

  whenever $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_p^{*}$ for $\left|z\right|=r\le {\rho }_2$.
• It is easy to see that $x_3\left(0\right)=1<0$ and $x_3\left(1\right)=8(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. In view of (31) and the fact that the centre of the disc in (29) is 1, $f\in {\mathcal{S}}_p^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{1}{2},$$

  (33)

  which is equivalent to $f\in {\mathcal{S}}_p^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3)$. This proves that $f\in {\mathcal{S}}_p^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (33) that

  $$Re\left(\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right)=\left|1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right|=\left|\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}-1\right|.$$

□

Remark 5. 

Placing $b=1$, $c=1$ and $q=2$ in Theorem 3, we obtain the result (Theorem 3, p. 9, [14]) with the part$\left(ii\right)$ having an improved radius ($=0.0990195>0.0972$).

In 2015, the class of starlike functions associated with the exponential function as ${\mathcal{S}}_e^{*}={\mathcal{S}}^{*}\left(e^z\right)$ was introduced by Mendiratta et al. [35]. It satisfies the condition $|log(z{f}^{\prime }\left(z\right)/f\left(z\right)\left)\right|$$<1$. Our next theorem gives the sharp radii constants $R_{{\mathcal{S}}_e^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_e^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_e^{*}}\left(H_b^3\right)$.

Theorem 4. 

The ${\mathcal{S}}_e^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_e^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=(1-e)+(d+q+s)r+(2+10e+dq+deq+(1+e)(d+q)s)r^2+(q+s+10e(q+s)+d(1+qs+2e(5+qs)))r^3+8e(3+qs+d(q+s))r^4+((-1+12e)(q+s)+d(-1-qs+4e(3+qs)))r^5+(-2+14e-dq+3deq+(-1+3e)(d+q)s)r^6+(-1+2e)(d+q+s)r^7+(-1+e)r^8$.

2. 

For the class $H_{b,c}^2$, ${\mathcal{S}}_e^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=(1-e)+(m+n+q)r+(1+9e+(1+e)(nq+m(n+q)))r^2+(8e(m+q)+n(1+mq+2e(5+mq)))r^3+(-1-mq+e(13+8mn+5mq+8nq))r^4+(-n-q+4e(3n+q)+m(-1+4e)(1+nq))r^5+(-1+3e)(1+n(m+q))r^6+n(-1+2e)r^7.$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_e^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=(1-e)+(l+q)r+(1+7e+(1+e)lq)r^2+6e(l+q)r^3+(-1+9e+(-1+3e)lq)r^4+(-1+2e)(l+q)r^5+(-1+e)r^6$.

Proof. 

• Note that $x_1\left(0\right)=1-e<0$ and $x_1\left(1\right)=6e(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. Mendiratta et al. [35] proved, for $e^{-1}\le C\le (e+e^{-1})/2)$, that

  $$\{w\in \mathbb{C}:|w-C|<C-e^{-1}\}\subset \{w\in \mathbb{C}:|log\left(w\right)|<1\}.$$

  (34)
  As the centre of the disc in (21) is 1, by (34), $f\in {\mathcal{S}}_e^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 1-\frac{1}{e},$$

  (35)

  which is equivalent to $f\in {\mathcal{S}}_e^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this, $f\in {\mathcal{S}}_e^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (35), the function $F_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the following equality,

  $$\begin{array}{cc}\hfill & \left|log\left(\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}\right)\right|\hfill \\ \hfill & =\left|log\left(1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right)\right|\hfill \\ \hfill & =\left|log\left(\frac{1}{e}\right)\right|=1.\hfill \end{array}$$
  Thus, the radius is sharp.
• A calculation shows that $x_2\left(0\right)=1-e<0$ and $x_2\left(1\right)=6e(2+m)(1+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (34) and the fact that the centre of the disc in (13) is 1, $f\in {\mathcal{S}}_e^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 1-\frac{1}{e},$$

  which is equivalent to $f\in {\mathcal{S}}_e^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_e^{*}$ for $\left|z\right|=r\le {\rho }_2$.
• It is easy to see that $x_3\left(0\right)=1-e<0$ and $x_3\left(1\right)=4e(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (34), $f\in {\mathcal{S}}_e^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 1-\frac{1}{e},$$

  (36)

  which is equivalent to $f\in {\mathcal{S}}_e^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3)$. This proves that $f\in {\mathcal{S}}_e^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (36) that

  $$\begin{array}{cc}\hfill \left|log\left(\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right)\right|& =\left|log\left(1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right)\right|\hfill \\ \hfill & =\left|log\left(\frac{1}{e}\right)\right|=1.\hfill \end{array}$$

□

Remark 6. 

For $b=1$, $c=1$ and $q=2$, Theorem 4 reduces to the result (Theorem 4, p. 10, [14]).

The class ${\mathcal{S}}_c^{*}={\mathcal{S}}^{*}(1+(4/3)z+(2/3)z^2)$ is the class of starlike functions $f\in \mathcal{A}$ such that $z{f}^{\prime }\left(z\right)/f\left(z\right)$ lies in the region bounded by the cardioid ${\mathsf{\Omega }}_c=\{u+iv:{(9u^2+9v^2-18u+5)}^2-16(9u^2+9v^2-6u+1)=0\}.$ Sharma et al. [36] studied various properties of the class ${\mathcal{S}}_c^{*}$. The following theorem determines the sharp radii constants $R_{{\mathcal{S}}_c^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_c^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_c^{*}}\left(H_b^3\right)$.

Theorem 5. 

The ${\mathcal{S}}_c^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_c^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=-2+(d+q+s)r+4(8+qs+d(q+s))r^2+(31(q+s)+d(31+7qs))r^3+24(3+qs+d(q+s))r^4+(35(q+s)+d(35+11qs))r^5+8(5+qs+d(q+s))r^6+5(d+q+s)r^7+2r^8$.

2. 

For the class $H_{b,c}^2$, the ${\mathcal{S}}_c^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=-2+(m+n+q)r+4(7+nq+m(n+q))r^2+(31n+24q+m(24+7nq))r^3+(38+24mn+24nq+14mq)r^4+(35n+11q+11m(1+nq))r^5+8(1+n(m+q))r^6+5n{r}^7$.

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_c^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=-2+(l+q)r+(22+4lq)r^2+18(l+q)r^3+(26+8lq)r^4+5(l+q)r^5+2r^6$.

Proof. 

• Note that $x_1\left(0\right)=-2<0$ and $x_1\left(1\right)=18(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. Sharma et al. [36] proved that, for $1/3<C\le 5/3$,

  $$\{w\in \mathbb{C}:|w-C|<(3C-1)/3\}\subseteq {\mathsf{\Omega }}_c.$$

  (37)
  As the centre of the disc in (21) is 1, by (37), $f\in {\mathcal{S}}_c^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  (38)

  which is equivalent to $f\in {\mathcal{S}}_c^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_c^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (38), the function $F_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{c}\hfill \left|\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}\right|=\left|1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right|=\frac{1}{3},\end{array}$$

  which belongs to boundary of the region ${\mathsf{\Omega }}_c$. Thus, the radius is sharp.
• A calculation shows that $x_2\left(0\right)=-2<0$ and $x_2\left(1\right)=18(2+m)(1+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (37) and the fact that the centre of the disc in (13) is 1, $f\in {\mathcal{S}}_c^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  which is equivalent to $f\in {\mathcal{S}}_c^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_c^{*}$ for $\left|z\right|=r\le {\rho }_2$.
• It is easy to see that $x_3\left(0\right)=-2<0$ and $x_3\left(1\right)=12(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. In view of the fact that the centre of the disc in (29) is 1, by (37), $f\in {\mathcal{S}}_c^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  (39)

  which is equivalent to $f\in {\mathcal{S}}_e^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3)$. This proves that $f\in {\mathcal{S}}_c^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (39) that

  $$\begin{array}{cc}\hfill \left|\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right|& =\left|1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right|=\frac{1}{3}.\hfill \end{array}$$

□

Remark 7. 

Placing $b=1$, $c=1$ and $q=2$ in Theorem 5, we obtain the result (Theorem 5, p. 11, [14]).

In 2019, Cho et al. [37] considered the class of starlike functions ${\mathcal{S}}_{sin}^{*}=\{f\in \mathcal{A}:z{f}^{\prime }\left(z\right)/f\left(z\right)\prec 1+sin\left(z\right):=q_0\left(z\right)\}$ associated with the sine function. Note that ${\mathcal{S}}_{sin}^{*}={\mathcal{S}}^{*}(1+sin\left(z\right))$. In next theorem, we determine sharp estimates of radii constants $R_{{\mathcal{S}}_{sin}^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_{sin}^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_{sin}^{*}}\left(H_b^3\right)$.

Theorem 6. 

The sharp ${\mathcal{S}}_{sin}^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_{sin}^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=-sin\left(1\right)-(d+q+s)(-1+sin\left(1\right))r-2(-6+sin\left(1\right)-(qs+d(q+s))(sin\left(1\right)-2))r^2+(11(q+s)+d(11+3qs)-(d+q+s+dqs)sin\left(1\right))r^3+8(3+qs+d(q+s))r^4+((q+s)(11+sin\left(1\right))+d(11+sin\left(1\right)+qs(3+sin\left(1\right))))r^5+((qs+d(q+s))(2+sin\left(1\right))+2(6+sin\left(1\right)))r^6+(d+q+s)(1+sin\left(1\right))r^7+sin\left(1\right)r^8$.

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}_{sin}^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=-sin\left(1\right)-(m+n+q)(sin\left(1\right)-1)r+(10-(nq+m(n+q))(-2+sin\left(1\right))-sin\left(1\right))r^2+(8m+11n+8q+3mnq-n(1+mq)sin\left(1\right))r^3+(12+8nq+sin\left(1\right)+m(8n+q(4+sin\left(1\right))))r^4+(q(3+sin\left(1\right))+m(1+nq)(3+sin\left(1\right))+n(11+sin\left(1\right)))r^5+(1+n(m+q))(2+sin\left(1\right))r^6+n(1+sin\left(1\right))r^7$.

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_{sin}^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=-sin\left(1\right)-(l+q)(sin\left(1\right)-1)r+(8-lq(-2+sin\left(1\right))-sin\left(1\right))r^2+6(l+q)r^3+(8+sin\left(1\right)+lq(2+sin\left(1\right)))r^4+(l+q)(1+sin\left(1\right))r^5+sin\left(1\right)r^6$.

Proof. 

• Note that $x_1\left(0\right)=-sin\left(1\right)<0$ and $x_1\left(1\right)=6(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. For $|C-1|\le sin\left(1\right),$ Cho et al. [37] established the following inclusion property,

  $$\{w\in \mathbb{C}:|w-C|<sin\left(1\right)-|C-1\left|\right\}\subseteq {\mathsf{\Omega }}_s,$$

  (40)

  where ${\mathsf{\Omega }}_s:=q_0(\Delta )$ is the image of the unit disc $\Delta$ under the mappings $q_0\left(z\right)=1+sin\left(z\right)$. As the centre of the disc in (21) is 1, by (40), $f\in {\mathcal{S}}_{sin}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le sin\left(1\right),$$

  (41)

  which is equivalent to $f\in {\mathcal{S}}_{sin}^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_{sin}^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (41), the function $f_1\left(z\right)$ defined for class $H_{b,c}^1$ in (6) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{cc}\hfill \left|\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}\right|& =\left|1+\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right|\hfill \\ \hfill & =1+sin1=q_0\left(1\right),\hfill \end{array}$$

  which belongs to the boundary of region ${\mathsf{\Omega }}_s$. This proves the radius is sharp.
• A calculation shows that $x_2\left(0\right)=-sin\left(1\right)<0$ and $x_2\left(1\right)=6(2+m)(1+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (40) and the fact that centre of the disc in (13) is 1, $f\in {\mathcal{S}}_{sin}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le sin\left(1\right),$$

  (42)

  which is equivalent to $f\in {\mathcal{S}}_{sin}^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_{sin}^{*}$ for $\left|z\right|=r\le {\rho }_2$. To prove sharpness, consider the function $f_2$ defined in (14). For $u=5b-3c\ge 0$, $v=3c-q\ge 0$ and $z:=-{\rho }_2$, it follows from (42) that

  $$\begin{array}{cc}\hfill \left|\frac{z{f}_2^{\prime }\left(z\right)}{f_2\left(z\right)}\right|& =\left|1+\frac{{\rho }_2}{(1-{\rho }_2^2)}\left(\frac{u{\rho }_2^2+4{\rho }_2+u}{{\rho }_2^2+u{\rho }_2+1}+\frac{v{\rho }_2^2+2{\rho }_2+v}{v{\rho }_2+1}+\frac{q{\rho }_2^2+4{\rho }_2+q}{{\rho }_2^2+q{\rho }_2+1}\right)\right|\hfill \\ \hfill & =1+sin\left(1\right),\hfill \end{array}$$

  which illustrates the sharpness.
• It is easy to see that $x_3\left(0\right)=-sin\left(1\right)<0$ and $x_3\left(1\right)=4(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (40), $f\in {\mathcal{S}}_{sin}^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le sin\left(1\right),$$

  (43)

  which is equivalent to $f\in {\mathcal{S}}_{sin}^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3).$ This proves that $f\in {\mathcal{S}}_{sin}^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for function $f_3$ defined for the class $H_b^3$ in (19). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (43) that

  $$\begin{array}{c}\hfill \left|\frac{z{f}_3^{\prime }\left(z\right)}{f_3\left(z\right)}\right|=\left|1+\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right|=1+sin\left(1\right).\end{array}$$

□

Remark 8. 

Figure 3 represents sharp ${\mathcal{S}}_{sin}^{*}$ radii estimated for all three classes.

Remark 9. 

Substituting $b=1$, $c=1$ and $q=2$ in Theorem 6, we obtain the result (Theorem 6, p. 13, [14]).

In 2015, Raina and Sokól [38] introduced the class ${\mathcal{S}}_{☾}^{*}={\mathcal{S}}^{*}(z+\sqrt{1+z^2})$. Geometrically, a function $f\in {\mathcal{S}}_{☾}^{*}$ if and only if $z{f}^{\prime }\left(z\right)/f\left(z\right)$ lies in the region bounded by the lune shaped region $\{w\in \mathbb{C}:|w^2-1|<2|w\left|\right\}.$

Theorem 7. 

The ${\mathcal{S}}_{☾}^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_{☾}^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=\sqrt{2}-2+(\sqrt{2}-1)(d+q+s)r+(2(4+\sqrt{2})+\sqrt{2}(qs+d(q+s)))r^2+((9+\sqrt{2})(q+s)+d(9+\sqrt{2}+(1+\sqrt{2})qs))r^3+8(3+qs+d(q+s))r^4+(-(-13+\sqrt{2})(q+s)-d(-13+\sqrt{2}+(-5+\sqrt{2})qs))r^5+(-2(-8+\sqrt{2})-(-4+\sqrt{2})(qs+d(q+s)))r^6-(-3+\sqrt{2})(d+q+s)r^7+(2-\sqrt{2})r^8$.

2. 

For the class $H_{b,c}^2$, the ${\mathcal{S}}_{☾}^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=\sqrt{2}-2+(\sqrt{2}-1)(m+n+q)r+(8+\sqrt{2}+\sqrt{2}(nq+m(n+q)))r^2+(8(m+q)+n(9+\sqrt{2}+(1+\sqrt{2})mq))r^3+(14-\sqrt{2}+6mq-\sqrt{2}mq+8n(m+q))r^4+(-(-5+\sqrt{2})(m+q)-n(-13+\sqrt{2}+(-5+\sqrt{2})mq))r^5-(-4+\sqrt{2})(1+n(m+q))r^6-(\sqrt{2}-3)n{r}^7$.

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_{☾}^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=\sqrt{2}-2+(\sqrt{2}-1)(l+q)r+(6+\sqrt{2}+\sqrt{2}lq)r^2+6(l+q)r^3+(10-\sqrt{2}-(-4+\sqrt{2})lq)r^4-(-3+\sqrt{2})(l+q)r^5+(2-\sqrt{2})r^6$.

Proof. 

• Note that $x_1\left(0\right)=\sqrt{2}-2<0$ and $x_1\left(1\right)=6(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. Gandhi and Ravichandran [39] (Lemma 2.1) proved that, for $\sqrt{2}-1<C\le \sqrt{2}+1$,

  $$\{w\in \mathbb{C}:|w-C|<1-|\sqrt{2}-C\left|\right\}\subseteq \{w\in \mathbb{C}:|w^2-1|<2|w\left|\right\}.$$

  (44)
  As the centre of the disc in (21) is 1, by (44), $f\in {\mathcal{S}}_{☾}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 2-\sqrt{2},$$

  (45)

  which is equivalent to $f\in {\mathcal{S}}_{☾}^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_{☾}^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (45), the function $F_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{cc}\hfill & \left|{\left(\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}\right)}^2-1\right|\hfill \\ \hfill & =\left|{\left(1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right)}^2-1\right|\hfill \\ \hfill & =|1-{(2-\sqrt{2})}^2-1|=2(1-\sqrt{2})=2\left|\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}\right|.\hfill \end{array}$$
  This proves the sharpness.
• A calculation shows that $x_2\left(0\right)=\sqrt{2}-2<0$ and $x_2\left(1\right)=6(2+m)(1+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (44) and the fact centre of the disc in (13) is 1, $f\in {\mathcal{S}}_{☾}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 2-\sqrt{2},$$

  which is equivalent to $f\in {\mathcal{S}}_{☾}^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_{☾}^{*}$ for $\left|z\right|=r\le {\rho }_2$.
• It is easy to see that $x_3\left(0\right)=\sqrt{2}-2<0$ and $x_3\left(1\right)=4(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (44), $f\in {\mathcal{S}}_{☾}^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 2-\sqrt{2},$$

  (46)

  which is equivalent to $f\in {\mathcal{S}}_{☾}^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3).$ This proves that $f\in {\mathcal{S}}_{☾}^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (46) that

  $$\begin{array}{cc}\hfill \left|{\left(\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right)}^2-1\right|& =\left|{\left(1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right)}^2-1\right|\hfill \\ & =2\left|\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right|.\hfill \end{array}$$

□

Remark 10. 

For $b=1$, $c=1$ and $q=2$, Theorem 7 yields the result (Theorem 7, p. 14, [14]).

Kumar et al. [40] introduced the class of starlike functions, defined by ${\mathcal{S}}_R^{*}={\mathcal{S}}^{*}\left(\psi \left(z\right)\right)$, consisting of functions associated with a rational function $\psi \left(z\right)=1+z(k+z)/\left(k\right(k-z\left)\right)$, where $k=\sqrt{2}+1.$ The following theorem yields the radii constants $R_{{\mathcal{S}}_R^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_R^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_R^{*}}\left(H_b^3\right)$.

Theorem 8. 

The ${\mathcal{S}}_R^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_R^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=(2\sqrt{2}-3)+2(\sqrt{2}-1)(d+q+s)r+(6+4\sqrt{2}+(2\sqrt{2}-1)(qs+d(q+s)))r^2+2((4+\sqrt{2})(q+s)+d(4+\sqrt{2}+\sqrt{2}qs))r^3+8(3+qs+d(q+s))r^4-2((-7+\sqrt{2})(q+s)+d(-7+\sqrt{2}+(-3+\sqrt{2})qs))r^5+(18-4\sqrt{2}+(5-2\sqrt{2})(qs+d(q+s)))r^6-2\left((-2+\sqrt{2})(d+q+s)\right)r^7+(3-2\sqrt{2})r^8$.

2. 

For the class $H_{b,c}^2$, the ${\mathcal{S}}_R^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=(2\sqrt{2}-3)+2(\sqrt{2}-1)(m+n+q)r+(7+2\sqrt{2}+(2\sqrt{2}-1)(nq+m(n+q)))r^2+(8(m+q)+2n(4+\sqrt{2}+\sqrt{2}mq))r^3+(15-2\sqrt{2}+7mq-2\sqrt{2}mq+8n(m+q))r^4-2((-7+\sqrt{2})n+(-3+\sqrt{2})q+(-3+\sqrt{2})m(1+nq))r^5+(5-2\sqrt{2})(1+n(m+q))r^6-2\left((-2+\sqrt{2})n\right)r^7$.

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_R^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=(2\sqrt{2}-3)+2(\sqrt{2}-1)(l+q)r+(5+2\sqrt{2}+(-1+2\sqrt{2})lq)r^2+6(l+q)r^3+(11-2\sqrt{2}+(5-2\sqrt{2})lq)r^4-2\left((-2+\sqrt{2})(l+q)\right)r^5+(3-2\sqrt{2})r^6.$

Proof. 

• Note that $x_1\left(0\right)=2\sqrt{2}-3<0$ and $x_1\left(1\right)=6(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. For $2(\sqrt{2}-1)<C\le \sqrt{2},$ Kumar et al. [40] proved that

  $$\{w\in \mathbb{C}:|w-C|<C-2(\sqrt{2}-1)\}\subseteq \psi (\Delta ).$$

  (47)
  As the centre of the disc in (21) is 1, by (47) $f\in {\mathcal{S}}_R^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 3-2\sqrt{2},$$

  (48)

  which is equivalent to $f\in {\mathcal{S}}_R^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_R^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (48), the function $F_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (8) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{cc}\hfill \left|\frac{z{F}_1^{\prime }\left(z\right)}{F_1\left(z\right)}\right|& =\left|1-\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right|\hfill \\ \hfill & =2(\sqrt{2}-1)=\psi (-1).\hfill \end{array}$$
  This proves the sharpness.
• A calculation shows that $x_2\left(0\right)=2\sqrt{2}-3<0$ and $x_2\left(1\right)=6(2+m)(l+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (47) and the fact that the centre of the disc in (13) is 1, $f\in {\mathcal{S}}_R^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 3-2\sqrt{2},$$

  which is equivalent to $f\in {\mathcal{S}}_R^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_R^{*}$ for $\left|z\right|=r\le {\rho }_2$.
• It is easy to see that $x_3\left(0\right)=2\sqrt{2}-3<0$ and $x_3\left(1\right)=4(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (47), $f\in {\mathcal{S}}_R^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le 3-2\sqrt{2},$$

  (49)

  which is equivalent to $f\in {\mathcal{S}}_R^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3).$ This proves that $f\in {\mathcal{S}}_R^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (49) that

  $$\begin{array}{cc}\hfill \left|\frac{z{F}_3^{\prime }\left(z\right)}{F_3\left(z\right)}\right|& =\left|1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right|=\psi (-1).\hfill \end{array}$$

□

Remark 11. 

Substituting $b=1$, $c=1$ and $q=2$ in Theorem 8, we obtain the result (Theorem 8, p. 15, [14]).

In 2020, Wani and Swaminathan [41] (Lemma 2.2) introduced the class ${\mathcal{S}}_{Ne}^{*}={\mathcal{S}}^{*}(1+z-(z^3/3))$ consisting of functions associated with a nephroid. Thus, as per definition, a function $f\in {\mathcal{S}}_{Ne}^{*}$ if and only if $z{f}^{\prime }/f$ maps the open unit disc $\Delta$ onto the interior of a two cusped kidney shaped curve ${\mathsf{\Omega }}_{Ne}:=\{u+iv:{({(u-1)}^2+v^2-4/9)}^3-4v^2/3<0\}.$ In next theorem, we find the sharp radii constants $R_{{\mathcal{S}}_{Ne}^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_{Ne}^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_{Ne}^{*}}\left(H_b^3\right)$.

Theorem 9. 

The sharp ${\mathcal{S}}_{Ne}^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_{Ne}^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=-2+(d+q+s)r+4(8+qs+d(q+s))r^2+(31(q+s)+d(31+7qs))r^3+24(3+qs+d(q+s))r^4+(35(q+s)+d(35+11qs))r^5+8(5+qs+d(q+s))r^6+5(d+q+s)r^7+2r^8$.

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}_{Ne}^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=-2+(m+n+q)r+4(7+nq+m(n+q))r^2+(31n+24q+m(24+7nq))r^3+(38+24mn+14mq+24nq)r^4+(35n+11q+11m(1+nq))r^5+8(1+n(m+q))r^6+5n{r}^7.$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_{Ne}^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=-2+(2+l)r+(22+8l)r^2+18(2+l)r^3+(26+16l)r^4+5(2+l)r^5+2r^6$.

Proof. 

• Note that $x_1\left(0\right)=-2<0$ and $x_1\left(1\right)=18(2+d)(2+q)(2+s)>0$; thus, in view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. For $1\le C<5/3,$ Wani and Swaminathan [41] (Lemma 2.2) had proved that

  $$\{w\in \mathbb{C}:|w-C|<5/3-C\}\subseteq {\mathsf{\Omega }}_{Ne}.$$

  (50)
  As the centre of the disc in (21) is 1, by (50), $f\in {\mathcal{S}}_{Ne}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  (51)

  which is equivalent to $f\in {\mathcal{S}}_{Ne}^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_{Ne}^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (51), the function $f_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (6) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{cc}\hfill \left|\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}\right|& =\left|1+\frac{{\rho }_1}{(1-{\rho }_1^2)}\left(\frac{u{\rho }_1^2+4{\rho }_1+u}{{\rho }_1^2+u{\rho }_1+1}+\frac{v{\rho }_1^2+4{\rho }_1+v}{{\rho }_1^2+v{\rho }_1+1}+\frac{q{\rho }_1^2+4{\rho }_1+q}{{\rho }_1^2+q{\rho }_1+1}\right)\right|\hfill \\ \hfill & =\frac{5}{3},\hfill \end{array}$$

  which belongs to the boundary of the region ${\mathsf{\Omega }}_{Ne}$. This proves sharpness.
• A calculation shows that $x_2\left(0\right)=-2<0$ and $x_2\left(1\right)=18(2+m)(l+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (50) and the fact that centre of the disc in (13) is 1, $f\in {\mathcal{S}}_{Ne}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  (52)

  which is equivalent to $f\in {\mathcal{S}}_{Ne}^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_{Ne}^{*}$ for $\left|z\right|=r\le {\rho }_2$. To prove the sharpness, consider the function $f_2$ defined in (14). For $u=5b-3c\ge 0$, $v=3c-q\ge 0$ and $z=-{\rho }_2$, it follows from (52) that

  $$\begin{array}{cc}\hfill \left|\frac{z{f}_2^{\prime }\left(z\right)}{f_2\left(z\right)}\right|& =\left|1+\frac{{\rho }_2}{(1-{\rho }_2^2)}\left(\frac{u{\rho }_2^2+4{\rho }_2+u}{{\rho }_2^2+u{\rho }_2+1}+\frac{v{\rho }_2^2+2{\rho }_2+v}{v{\rho }_2+1}+\frac{q{\rho }_2^2+4{\rho }_2+q}{{\rho }_2^2+q{\rho }_2+1}\right)\right|\hfill \\ \hfill & =\frac{5}{3},\hfill \end{array}$$

  which illustrates the sharpness.
• It is easy to see that $x_3\left(0\right)=-2<0$ and $x_3\left(1\right)=12(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (50), $f\in {\mathcal{S}}_{Ne}^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{2}{3},$$

  (53)

  which is equivalent to $f\in {\mathcal{S}}_{Ne}^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3).$ This proves that $f\in {\mathcal{S}}_{Ne}^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  The result is sharp for the function $f_3$ defined for the class $H_b^3$ in (19). At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, it follows from (53) that

  $$\begin{array}{cc}\hfill \left|\frac{z{f}_3^{\prime }\left(z\right)}{f_3\left(z\right)}\right|& =\left|1-\frac{{\rho }_3}{1-{\rho }_3^2}\left(\frac{u{\rho }_3^2+4{\rho }_3+u}{{\rho }_3^2+u{\rho }_3+1}+\frac{q{\rho }_3^2+4{\rho }_3+q}{{\rho }_3^2+q{\rho }_3+1}\right)\right|=\frac{5}{3}.\hfill \end{array}$$

□

Remark 12. 

Figure 4 represents sharp ${\mathcal{S}}_{Ne}^{*}$ radii estimated for all three classes.

Remark 13. 

For $b=1$, $c=1$ and $q=2$, Theorem 9 reduces to the corresponding results in (Theorem 10, p. 18, [14]).

In 2020, the class ${\mathcal{S}}_{SG}^{*}={\mathcal{S}}^{*}(2/(1+e^{-z}))$ that maps the open unit disc $\Delta$ onto a domain ${\Delta }_{SG}:=\{w\in \mathbb{C}:|log(w/(2-w))|<1\}$ was introduced by Goel and Kumar [42]. Some results for the class ${\mathcal{S}}_{SG}^{*}$ can be seen in [43]. The following theorem gives the sharp radii constants $R_{{\mathcal{S}}_{SG}^{*}}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}_{SG}^{*}}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}_{SG}^{*}}\left(H_b^3\right)$.

Theorem 10. 

The sharp ${\mathcal{S}}_{SG}^{*}$ radii for the classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$ are as follows:

1. 

For the class $H_{b,c}^1$, the sharp ${\mathcal{S}}_{SG}^{*}$ radius ${\rho }_1\in (0,1)$ is the smallest root of the equation $x_1\left(r\right)=0$, where

$x_1\left(r\right)=(1-e)+2(d+q+s)r+(2(7+5e)+(3+e)(qs+d(q+s)))r^2+(2(6+5e)(q+s)+2d(6+5e+(2+e)qs))r^3+8(1+e)(3+qs+d(q+s))r^4+2((5+6e)(q+s)+d(5+qs+2e(3+qs)))r^5+(2(5+7e)+(1+3e)(qs+d(q+s)))r^6+2e(d+q+s)r^7+(-1+e)r^8.$

2. 

For the class $H_{b,c}^2$, the sharp ${\mathcal{S}}_{SG}^{*}$ radius ${\rho }_2\in (0,1)$ is the smallest root of the equation $x_2\left(r\right)=0$, where

$x_2\left(r\right)=(1-e)+2(m+n+q)r+(11+9e+(3+e)(nq+m(n+q)))r^2+2(4(1+e)m+(6+5e)n+4(1+e)q+(2+e)mnq)r^3+(11+8mn+3mq+8nq+e(13+8mn+5mq+8nq))r^4+2((5+6e)n+q+2eq+(1+2e)m(1+nq))r^5+(1+3e)(1+n(m+q))r^6+2en{r}^7.$

3. 

For the class $H_b^3$, the sharp ${\mathcal{S}}_{SG}^{*}$ radius ${\rho }_3\in (0,1)$ is the smallest root of the equation $x_3\left(r\right)=0$, where

$x_3\left(r\right)=(1-e)+2(l+q)r+(9+7e+(3+e)lq)r^2+6(1+e)(l+q)r^3+(7+lq+3e(3+lq))r^4+2e(l+q)r^5+(-1+e)r^6.$

Proof. 

• Note that $x_1\left(0\right)=1-e<0$ and $x_1\left(1\right)=6(1+e)(2+d)(2+q)(2+s)>0$. In view of the Intermediate Value Theorem, there exists a root of the equation $x_1\left(r\right)=0$ in the interval $(0,1)$. Let $r={\rho }_1\in (0,1)$ be the smallest root of the equation $x_1\left(r\right)=0$. For $2/(1+e)<C<2e/(1+e),$ Goel and Kumar [42] proved the following inclusion property,

  $$\{w\in \mathbb{C}:|w-C|<r_{SG}\}\subset {\Delta }_{SG},\mathrm{where}{r}_{SG}=\left(\frac{e-1}{e+1}\right)-|C-1|.$$

  (54)
  As the centre of the disc in (21) is 1, by (54), $f\in {\mathcal{S}}_{SG}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{d{r}^2+4r+d}{r^2+dr+1}+\frac{s{r}^2+4r+s}{r^2+sr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{e-1}{e+1},$$

  (55)

  which is equivalent to $f\in {\mathcal{S}}_{SG}^{*}$ if $x_1\left(r\right)\le 0$. Since $x_1\left(0\right)<0$ and ${\rho }_1$ is the smallest root of the equation $x_1\left(r\right)=0$, $x_1\left(r\right)$ is an increasing function on $(0,{\rho }_1)$. In view of this $f\in {\mathcal{S}}_{SG}^{*}$ for $\left|z\right|=r\le {\rho }_1$. For $u=6b-4c\ge 0$, $v=4c-q\ge 0$, using (55), the function $f_1\left(z\right)$ defined for the class $H_{b,c}^1$ in (6) at $z=-{\rho }_1$, satisfies the following equality

  $$\begin{array}{c}\hfill \left|log\left(\frac{\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}}{2-\frac{z{f}_1^{\prime }\left(z\right)}{f_1\left(z\right)}}\right)\right|=\left|log\left(\frac{1+\frac{e-1}{e+1}}{2-\left(1+\frac{e-1}{e+1}\right)}\right)\right|=\left|log\left(e\right)\right|=1.\end{array}$$

  (56)
  It follows that the radius is sharp.
• A calculation shows that $x_2\left(0\right)=1-e<0$ and $x_2\left(1\right)=6(1+e)(2+m)(l+n)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_2\left(r\right)=0$. Let ${\rho }_2\in (0,1)$ be the smallest root of the equation $x_2\left(r\right)=0$ and $f\in H_{b,c}^2$. In view of (54) and the fact that the centre of the disc in (13) is 1, $f\in {\mathcal{S}}_{SG}^{*}$ if

  $$\frac{r}{(1-r^2)}\left(\frac{m{r}^2+4r+m}{r^2+mr+1}+\frac{n{r}^2+2r+n}{nr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{e-1}{e+1},$$

  (57)

  which is equivalent to $f\in {\mathcal{S}}_{SG}^{*}$ if $x_2\left(r\right)\le 0$. Since $x_2\left(0\right)<0$ and ${\rho }_2$ is the smallest root of the equation $x_2\left(r\right)=0$, $x_2\left(r\right)$ is an increasing function on $(0,{\rho }_2)$. Thus, $f\in {\mathcal{S}}_{SG}^{*}$ for $\left|z\right|=r\le {\rho }_2$. To prove the sharpness, consider the function $f_2$ defined in (14). For $u=5b-3c\ge 0$, $v=3c-q\ge 0$ and $z=-{\rho }_2$, the similar calculations as in (56) together with (57) proves that the result is sharp.
• It is easy to see that $x_3\left(0\right)=1-e<0$ and $x_3\left(1\right)=4(1+e)(2+l)(2+q)>0$. By the Intermediate Value Theorem, there exists a root $r\in (0,1)$ of the equation $x_3\left(r\right)=0$. Let ${\rho }_3\in (0,1)$ be the smallest root of the equation $x_3\left(r\right)=0$. Since the centre of the disc in (29) is 1, by (54), $f\in {\mathcal{S}}_{SG}^{*}$ if

  $$\frac{r}{1-r^2}\left(\frac{l{r}^2+4r+l}{r^2+lr+1}+\frac{q{r}^2+4r+q}{r^2+qr+1}\right)\le \frac{e-1}{1+e},$$

  which is equivalent to $f\in {\mathcal{S}}_{SG}^{*}$ if $x_3\left(r\right)\le 0$. Since $x_3\left(0\right)<0$ and ${\rho }_3$ is the smallest root of the equation $x_3\left(r\right)=0$, $x_3\left(r\right)$ is an increasing function on $(0,{\rho }_3).$ This proves that $f\in {\mathcal{S}}_{SG}^{*}$ for $\left|z\right|=r\le {\rho }_3$.
  At $z=-{\rho }_3$ and for $u=4b-q\ge 0$, a calculation as in part(i) shows that the result is sharp for the function $F_3$ defined for the class $H_b^3$ in (20)

□

Remark 14. 

Figure 5 represents sharp ${\mathcal{S}}_{SG}^{*}$ radii estimated for all three classes.

Remark 15. 

Placing $b=1$, $c=1$ and $q=2$ in Theorem 10, we obtain the result (Theorem 11, p. 19, [14]).

4. Conclusions

The well-known classes as particular cases can be obtained from the newly defined classes $H_{b,c}^1$, $H_{b,c}^2$ and $H_b^3$. In Section 3, we found the sharp radii constants $R_N\left(H_{b,c}^1\right)$, $R_N\left(H_{b,c}^2\right)$ and $R_N\left(H_b^3\right)$, where N is any one of the subclasses, as mentioned in Section 1, of Ma-Minda class ${\mathcal{S}}^{*}\left(\psi \right)$. However, it is challenging to investigate the following open problem: Find sharp estimates of radii constants $R_{{\mathcal{S}}^{*}\left(\psi \right)}\left(H_{b,c}^1\right)$, $R_{{\mathcal{S}}^{*}\left(\psi \right)}\left(H_{b,c}^2\right)$ and $R_{{\mathcal{S}}^{*}\left(\psi \right)}\left(H_{b,c}^3\right)$.

Remark 16. 

If this open problem is solved, then Theorem 1 to Theorem 10 may become special cases of this new theorem.