On Extended Beta Function and Related Inequalities

Abstract

In this article, we consider a unified generalized version of extended Euler’s Beta function’s integral form a involving Macdonald function in the kernel. Moreover, we establish functional upper and lower bounds for this extended Beta function. Here, we consider the most general case of the four-parameter Macdonald function $K_{\nu +{\displaystyle \frac{1}{2}}}\left(p{t}^{-\lambda }+q{(1-t)}^{-\mu }\right)$ when $\lambda \ne \mu$ in the argument of the kernel. We prove related bounding inequalities, simultaneously complementing the recent results by Parmar and Pogány in which the extended Beta function case $\lambda =\mu$ is resolved. The main mathematical tools are integral representations and fixed-point iterations that are used for obtaining the stationary points of the argument of the Macdonald kernel function $K_{\nu +{\displaystyle \frac{1}{2}}}$.

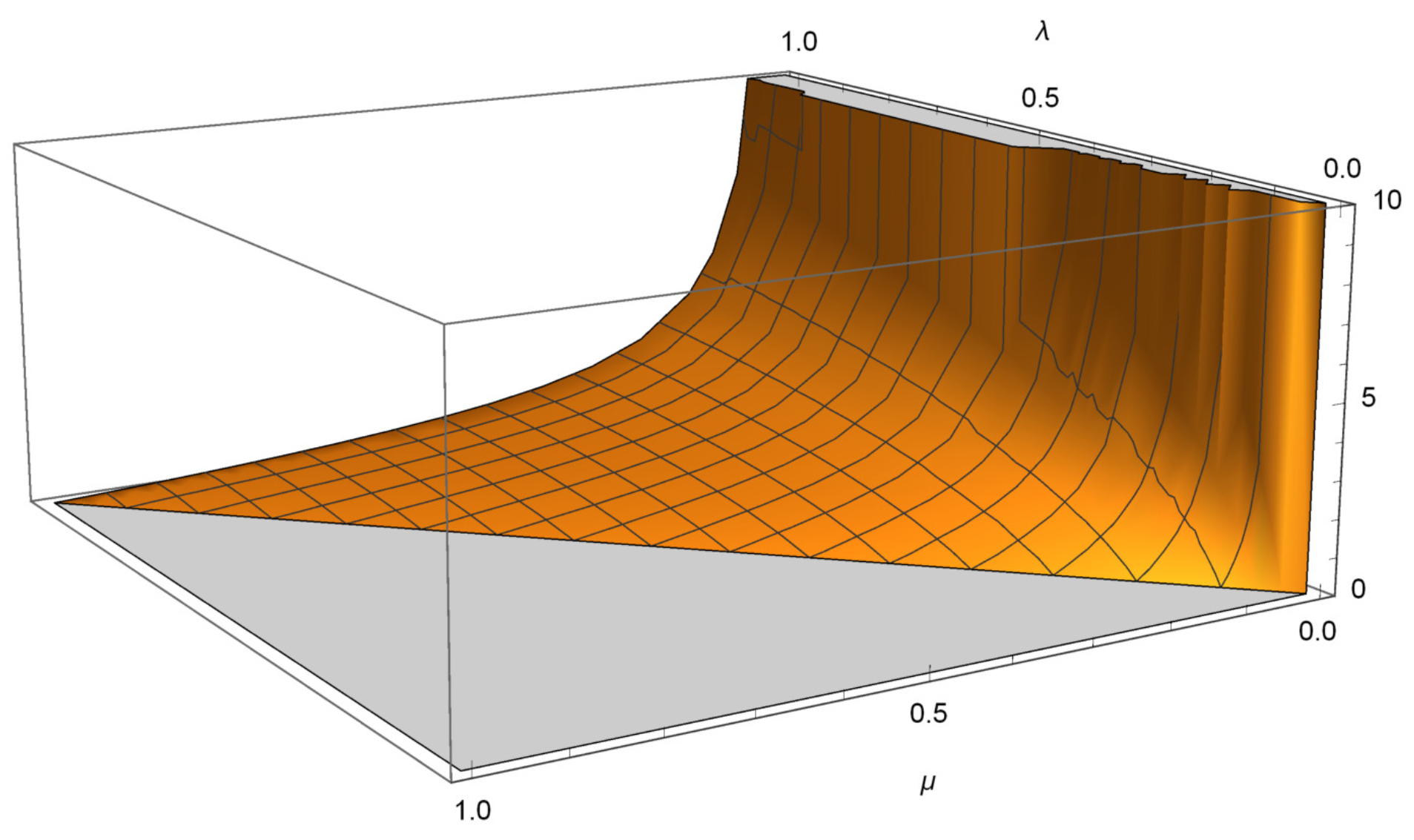
1. Introduction and Preliminaries

The Euler function of the first kind (Beta function, in other words) reads [1]:

$$\mathrm{B}(a,b)={\int }_0^1{t}^{a-1}{(1-t)}^{b-1}\mathrm{d}t,a\wedge b>0,$$

(1)

which has been considered as the base function for several extensions involving the exponential, Kummer hypergeometric function, the Macdonald function (modified Bessel function of the second kind) and/or the Mittag–Leffler function, etc., of a suitable argument (see, for instance, [2,3,4,5,6,7,8,9,10,11,12,13] and the references therein). The extensions are obtained with the following procedure: the Beta function transform maps a suitable input function $h\left(t\right)$ into a multiparameter integral [14]

$$B_h(a,b):=B(a,b)\left[h\right]={\int }_0^1{t}^{a-1}{(1-t)}^{b-1}h\left(t\right)\mathrm{d}t,a\wedge b>0,$$

The goal is to obtain and discuss mathematical properties of the output function. When the input function is of an exponential type and asymptomatically vanishing at one or both endpoints of the integration interval, an evolution of the generalizations can be summarized, as follows. The p-extended Beta ${\mathrm{B}}_p(a,b)$ was introduced by Chaudhry et al. in [6] using $h\left(t\right)=exp\{-{\displaystyle \frac{p}{t(1-t)}}\}$. Next, employing $h_{{\theta }_1}\left(t\right)=exp\{-{\displaystyle \frac{p}{t^m{(1-t)}^m}}\}$, Lee et al. [11] introduced the ${\mathrm{B}}_{m,p}(a,b)$ generalized Beta function, whilst the $(p,q)$-extended Beta defined by Choi et al. appears in [8], exploiting $h_{{\theta }_2}\left(t\right)=exp\{-{\displaystyle \frac{p}{t}}-{\displaystyle \frac{q}{1-t}}\}$. The Kummer-extended Beta function consists of $h_{{\theta }_3}\left(t\right)={}_{1}F_1\left(\alpha ;\beta ;-{\displaystyle \frac{b}{t^{\rho }{(1-t)}^{\lambda }}}\right)$ (see [15]), where [16] (p. 322, Equation (13).2.2)

$${}_{1}F_1(\alpha ;\beta ;z)=\sum _{n\ge 0}{\displaystyle \frac{{\left(a\right)}_n}{{\left(b\right)}_n}}{\displaystyle \frac{z^n}{n!}},$$

stands for the confluent hypergeometric function (or Kummer function), while the kernel $h_{{\theta }_4}\left(t\right)=\sqrt{{\displaystyle \frac{2p}{\pi t(1-t)}}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p}{t(1-t)}}\right)$ defines the Beta transform in [17] by Parmar et al. We recall that the MacDonald function (or modified Bessel function of the second kind) of the order $\mu$ [16] (p. 251, Equation (10).27.4) is given by

$$K_{\mu }\left(z\right)={\displaystyle \frac{\pi }{2}}{\displaystyle \frac{I_{-\mu }\left(z\right)-I_{\mu }\left(z\right)}{sin\left(\pi \mu \right)}},I_{\mu }\left(z\right)=\sum _{n\ge 0}{\displaystyle \frac{{\left(\frac{z}{2}\right)}^{2n+\mu }}{\Gamma (\mu +1+n)n!}},$$

where $I_{\mu }$ is the modified Bessel function of the first kind (see [16] (p. 249, Equation (10).25.2)), quoting that $I_{\mu }\left(x\right)$ is real when $\mu \in \mathbb{R}$ and $arg\left(z\right)=0$.

Finally, recently, Pogány and Parmar applied

$$h_{{\theta }_5}\left(t\right)=\sqrt{{\displaystyle \frac{2}{\pi }}\left({\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\lambda }}}\right)}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\lambda }}}\right)$$

to introduce the extended Beta function in their article [18].

Our main goal is to consider the extended Beta function with not necessarily equal parameters $\lambda$ and $\mu$, that is, the Beta integral with the input kernel

$$h_{\varphi }\left(t\right)=\sqrt{{\displaystyle \frac{2}{\pi }}\left({\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}\right)}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}\right),$$

(2)

which indicates the following definition, extending some findings of [18].

In what follows, we conventionally write $x\wedge y=min\{x,y\}$ and $x\vee y=max\{x,y\}$.

Definition 1.

The extended Beta function built with the Macdonald function reads

$$\begin{array}{cc}\hfill {\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)& ={\int }_0^1{t}^{a-1}{(1-t)}^{b-1}{h}_{\varphi }\left(t\right)\mathrm{d}t,\nu \in \mathbb{R},\hfill \end{array}$$

(3)

where $\varphi =(p,q,\lambda ,\mu )$ satisfies the constraints $\lambda \wedge \mu >0;\Re \left(p\right)\wedge \Re \left(q\right)>0$ and $(a-{\displaystyle \frac{\lambda }{2}})\wedge (b-{\displaystyle \frac{\mu }{2}})>0$.

2. Bounds for Extended Beta and Consequences

In this section, we expose our first main result about the functional upper bound for ${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }$ with some related consequences. Since we are mainly interested in the case $\lambda \ne \mu$, we refer to Theorem 2 in [18] for $\lambda =\mu >0.$

Theorem 1.

Let $(p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^4,\nu \in \mathbb{R},$ and $(a-{\displaystyle \frac{\lambda }{2}})\wedge (b-{\displaystyle \frac{\mu }{2}})>0$. Then, we have

$$\begin{array}{cc}\hfill {\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)& \le \sqrt{{\displaystyle \frac{2}{\pi }}}\sqrt{p{(1-t^{*})}^{\mu }+q{\left(t^{*}\right)}^{\lambda }}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p}{t_{*}^{\lambda }}}+{\displaystyle \frac{q}{{(1-t_{*})}^{\mu }}}\right)\mathrm{B}\left(a-{\displaystyle \frac{\lambda }{2}},b-{\displaystyle \frac{\mu }{2}}\right),\hfill \end{array}$$

where $t^{*}$ is given as follows:

(i) 

if $0<\mu <\lambda <1,$ and ${\displaystyle \frac{q}{p}<\frac{\mu }{\lambda }{\left(\frac{1-\lambda }{1-\mu }\right)}^{1-\lambda }}$, then $t^{*}=\underset{n\to \infty }{lim}{t}_n$, where

$$t_{n+1}={\left({\displaystyle \frac{q\lambda }{p\mu }}{(1-t_n)}^{1-\mu }\right)}^{{\displaystyle \frac{1}{1-\lambda }}},t_0\in (0,1);$$

(4)

(ii) 

if $\mu =1,\lambda \in (0,1),$ and ${\displaystyle \frac{q}{p}<\frac{4\lambda }{{(1+\lambda )}^2}}$, then $t^{*}={\left({\displaystyle \frac{\lambda q}{p}}\right)}^{{\displaystyle \frac{1}{1-\lambda }}};$

(iii) 

if $\lambda =1,\mu \in (0,1),$ and ${\displaystyle \mu <\frac{q}{p}<\frac{1}{\mu };}$

and in all of the above cases, $t_{*}$ is given by $t_{*}=\underset{n\to \infty }{lim}{\tilde{t}}_n$, where

$${\tilde{t}}_{n+1}=1-{\left({\displaystyle \frac{q\mu }{p\lambda }}{\left({\tilde{t}}_n\right)}^{1+\lambda }\right)}^{{\displaystyle \frac{1}{1+\mu }}},{\tilde{t}}_0\in (0,1);$$

(5)

(iv) 

if $0<\lambda <\mu <1,$ and ${\displaystyle \frac{q}{p}>\frac{\mu }{\lambda }{\left(\frac{1-\lambda }{1-\mu }\right)}^{1-\mu },}$ then $t^{*}=\underset{n\to \infty }{lim}{t}_n$, where

$$t_{n+1}=1-{\left({\displaystyle \frac{p\mu }{q\lambda }}{t}_n^{1-\lambda }\right)}^{{\displaystyle \frac{1}{1-\mu }}},t_0\in (0,1);$$

and $t_{*}$ is given by $t_{*}=\underset{n\to \infty }{lim}{\tilde{t}}_n$, where

$${\tilde{t}}_{n+1}={\left({\displaystyle \frac{p\lambda }{q\mu }}{\left(1-{\tilde{t}}_n\right)}^{1+\mu }\right)}^{{\displaystyle \frac{1}{1+\lambda }}},{\tilde{t}}_0\in (0,1).$$

Proof. 

As both functions in the integrand of (3) are positive in the declared range of parameters and the Macdonald function $K_{\mu }\in \mathrm{C}\left({\mathbb{R}}_{+}\right)$ is monotonically decreasing, we obtain

$$\begin{array}{cc}\hfill {\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)& \le \sqrt{{\displaystyle \frac{2}{\pi }}}\underset{0\le t\le 1}{max}\sqrt{p{(1-t)}^{\mu }+q{t}^{\lambda }}\underset{0<t<1}{sup}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}\right){\int }_0^1{t}^{a-{\displaystyle \frac{\lambda }{2}}-1}{(1-t)}^{b-{\displaystyle \frac{\mu }{2}}-1}\mathrm{d}t\hfill \\ \hfill & \le \sqrt{{\displaystyle \frac{2}{\pi }}}\sqrt{\underset{0\le t\le 1}{max}\{p{(1-t)}^{\mu }+q{t}^{\lambda }\}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(\underset{0<t<1}{inf}\left\{{\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}\right\}\right)·\mathrm{B}\left(a-{\displaystyle \frac{\lambda }{2}},b-{\displaystyle \frac{\mu }{2}}\right).\hfill \end{array}$$

We first consider $f\left(t\right):=p{(1-t)}^{\mu }+q{t}^{\lambda }\in {\mathrm{C}}^{\infty }(0,1),$ and find its stationary point $t^{*}\in (0,1)$ by solving the equation $f^{\prime }\left(t\right)=0,$ i.e.,

$$-\mu p{(1-t)}^{\mu -1}+\lambda q{t}^{\lambda -1}=0.$$

(6)

The positive-to-negative and monotone decreasing behavior of $f^{\prime }\left(t\right)$ through its unique zero $t^{*}$ will give the global maximum $f\left(t^{*}\right)$. Indeed,

$$f^{\prime }(0+)=\underset{t\to 0+}{lim}\left[q\lambda t^{\lambda -1}-p\mu {(1-t)}^{\mu -1}\right]=-p\mu +q\lambda \underset{t\to 0+}{lim}{t}^{\lambda -1}=\left\{\begin{array}{cc}-p\mu ,\hfill & \lambda >1,\hfill \\ -p\mu +q,\hfill & \lambda =1,\hfill \\ \infty ,\hfill & \lambda \in (0,1),\hfill \end{array}\right.$$

and

$$f^{\prime }(1-)=\underset{t\to 1-}{lim}\left[q\lambda t^{\lambda -1}-p\mu {(1-t)}^{\mu -1}\right]=q\lambda -p\mu \underset{t\to 1-}{lim}{(1-t)}^{\mu -1}=\left\{\begin{array}{cc}q\lambda ,\hfill & \mu >1,\hfill \\ q\lambda -p,\hfill & \mu =1,\hfill \\ -\infty ,\hfill & \mu \in (0,1).\hfill \end{array}\right.$$

Thus, $f^{\prime }(0+)f^{\prime }(1-)<0$ if (i), (ii), or (iii) holds. In fact, when $\mu =1$ and $\lambda \in (0,1)$, we need only $p>q\lambda ,$ i.e., ${\displaystyle \frac{q}{p}}<{\displaystyle \frac{1}{\lambda }}$. This follows from (ii), since ${\displaystyle \frac{4\lambda }{{(1+\lambda )}^2}}<{\displaystyle \frac{1}{\lambda }}$ for $\lambda \in (0,1).$ Similarly, when $\lambda =1$ and $\mu \in (0,1)$, we need only $q>p\mu$ (the left-hand side inequality of (iii)). The stronger conditions given in (ii) and (iii) will appear in the analysis of the iterative procedure (5) in the second part of the proof.

In all three cases under consideration, we have

$$f^{″}\left(t\right)=\mu (\mu -1)p{(1-t)}^{\mu -2}+\lambda (\lambda -1)t^{\lambda -2}<0,t\in (0,1),$$

so that there exists a unique $t^{*}\in (0,1)$ for which $f^{\prime }\left(t^{*}\right)$ vanishes, which implies that $\underset{0\le t\le 1}{max}f\left(t\right)=f\left(t^{*}\right)$.

Let the assumptions in (i) hold. In order to calculate $t^{*}$, we use the fixed-point iteration procedure and transform (6) into $t={\phi }_f\left(t\right)$, which gives the iteration function

$${\phi }_f\left(t\right)={\left({\displaystyle \frac{q\lambda }{p\mu }}\right)}^{{\displaystyle \frac{1}{1-\lambda }}}{(1-t)}^{{\displaystyle \frac{1-\mu }{1-\lambda }}},$$

constructed so that its modulus of the first derivative is sub-unimodular, as follows:

$$|{\phi }_f^{\prime }\left(t\right)|={\left({\displaystyle \frac{q\lambda }{p\mu }}\right)}^{{\displaystyle \frac{1}{1-\lambda }}}{\displaystyle \frac{1-\mu }{1-\lambda }}{(1-t)}^{{\displaystyle \frac{\lambda -\mu }{1-\lambda }}}<1,t\in (0,1).$$

With the conditions given in (i), it follows that

$$|{\phi }_f^{\prime }\left(t\right)|\le {\left({\displaystyle \frac{q\lambda }{p\mu }}\right)}^{{\displaystyle \frac{1}{1-\lambda }}}{\displaystyle \frac{1-\mu }{1-\lambda }}<1.$$

Then, the iteration process (4) converges to

$$t^{*}={\left({\displaystyle \frac{\lambda q}{\mu p}}\right)}^{{\displaystyle \frac{1}{1-\lambda }}}\underset{n\to \infty }{lim}{(1-t_n)}^{{\displaystyle \frac{1-\mu }{1-\lambda }}},$$

and consequently

$$\underset{0\le t\le 1}{max}f\left(t\right)=f\left(t^{*}\right)=p{(1-t^{*})}^{\mu }+q{\left(t^{*}\right)}^{\lambda }.$$

In cases (ii) and (iii), $t^{*}$ is calculated directly from (6).

Next, we consider the procedure for finding $t_{*}$. We denote the argument function of the Macdonald function by

$$g\left(t\right)={\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}.$$

The stationary point $t_{*}$ is the solution of $g^{\prime }\left(t\right)=-p\lambda t^{-\lambda -1}+q\mu {(1-t)}^{-\mu -1}=0$ with respect to $t\in (0,1)$. As $g\in {\mathrm{C}}^1(0,1)$ and obviously $g^{\prime }(0+)g^{\prime }(1-)<0$ for $\theta \in {\mathbb{R}}_{+}^4$, considering

$$g^{″}\left(t\right)=p\lambda (\lambda +1)t^{-\lambda -2}+q\mu (\mu +1){(1-t)}^{-\mu -2}>0,t\in (0,1),$$

we conclude that $g\left(t_{*}\right)$ is the global minimum at the stationary point $t_{*}$. To find the stationary point, we use the related iterative function

$${\phi }_g\left(t\right)=1-{\left({\displaystyle \frac{q\mu }{p\lambda }}{t}^{1+\lambda }\right)}^{{\displaystyle \frac{1}{1+\mu }}},t\in (0,1),$$

for which

$$|{\phi }_g^{\prime }\left(t\right)|={\left({\displaystyle \frac{q\mu }{p\lambda }}{t}^{\lambda -\mu }\right)}^{{\displaystyle \frac{1}{1+\mu }}}{\displaystyle \frac{1+\lambda }{1+\mu }}<1,t\in (0,1),$$

holds if $\mu \le \lambda$ and

$${\left({\displaystyle \frac{q\mu }{p\lambda }}\right)}^{{\displaystyle \frac{1}{1+\mu }}}{\displaystyle \frac{1+\lambda }{1+\mu }}<1.$$

(7)

Condition (7) is equivalent to ${\displaystyle \frac{q}{p}<\frac{\lambda }{\mu }{\left(\frac{1+\mu }{1+\lambda }\right)}^{1+\mu }}$ which is satisfied when (i) holds, since

$${\displaystyle \frac{\mu }{\lambda }}{\left({\displaystyle \frac{1-\lambda }{1-\mu }}\right)}^{1-\lambda }\le {\displaystyle \frac{\lambda }{\mu }}{\left({\displaystyle \frac{1+\mu }{1+\lambda }}\right)}^{1+\mu },0<\mu \le \lambda <1,$$

See Figure 1. In cases (ii) and (iii), inequality (7) is obviously satisfied. Therefore, the iterative sequence (5) gives

$$t_{*}=1-{\left({\displaystyle \frac{q\mu }{p\lambda }}\right)}^{{\displaystyle \frac{1}{1+\mu }}}\underset{n\to \infty }{lim}{\tilde{t}}_n^{{\displaystyle \frac{1+\lambda }{1+\mu }}},$$

for which

$$\underset{t\in (0,1)}{min}g\left(t\right)=g\left(t_{*}\right)={\displaystyle \frac{p}{t_{*}^{\lambda }}}+{\displaystyle \frac{q}{{(1-t_{*})}^{\mu }}}.$$

The case (iv) can be proved in a similar way as (i), so we omit the proof. □

Remark 1.

For $\lambda =\mu$, Equation (6) reduces to $-p{(1-t)}^{\lambda -1}+q{t}^{\lambda -1}=0$, which has explicit solution $t_0={\left[1+{\left(\frac{q}{p}\right)}^{{\displaystyle \frac{1}{\lambda -1}}}\right]}^{-1}$; compare this with [18] (p. 5). However, iteration (6) cannot be solved explicitly; therefore, the iteration method is applied as above.

Now, we present another fashion functional upper bound result.

Theorem 2.

Let $(p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^4$, whilst $\nu \in \mathbb{R}$. Then,

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\le 2\sqrt{{\displaystyle \frac{p\vee q}{\pi 2^{\lambda \wedge \mu }}}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(2^{1+\lambda \wedge \mu }·p\wedge q\right)\mathrm{B}\left(a-{\displaystyle \frac{1}{2}}(\lambda \vee \mu ),b-{\displaystyle \frac{1}{2}}(\lambda \vee \mu )\right),$$

(8)

provided $\lambda \vee \mu <2(a\wedge b)$.

Proof. 

We start with the integral definition (3), as follows:

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)={\int }_0^1{t}^{a-1}{(1-t)}^{b-1}{h}_{\varphi }\left(t\right)\mathrm{d}t,$$

in which we increase the input function $h_{\varphi }\left(t\right)$ by letting $\lambda \le \mu$, $p\le q$ and the fact that $K_{\mu }\left(x\right)\searrow$ in x, letting us obtain

$$\begin{array}{cc}\hfill h_{\varphi }\left(t\right)& \le \sqrt{{\displaystyle \frac{2q}{\pi }}}{t}^{-{\displaystyle \frac{\mu }{2}}}{(1-t)}^{-{\displaystyle \frac{\mu }{2}}}\sqrt{\underset{0<t<1}{max}\left(t^{\lambda }+{(1-t)}^{\lambda }\right)}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(p(t^{-\lambda }+{(1-t)}^{-\lambda })\right)\hfill \\ \hfill & \le \sqrt{{\displaystyle \frac{q}{\pi }}}{2}^{1-{\displaystyle \frac{\lambda }{2}}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(2^{1+\lambda }p\right)t^{-{\displaystyle \frac{\mu }{2}}}{(1-t)}^{-{\displaystyle \frac{\mu }{2}}},\hfill \end{array}$$

(9)

since $4t(1-t)\le 1$ inside the unit interval in the argument of the Macdonald function term, we apply the A–G inequalities.

The counterpart estimate, which has the same form, follows when we replace $\lambda \leftrightarrow \mu$ and $p\leftrightarrow q$ in the above bound. Finally, inserting this estimate into (3), we arrive at the statement (8). □

The lower bound result upon the extended Beta function follows. Let us introduce the functions class

$${\mathrm{L}}_{\mathrm{B}}^1[0,1]=\left\{{k\left(t\right):\parallel k\parallel }_{1,\mathrm{B}}={\int }_0^1\left|k\left(t\right)\right|t^{a-1}{(1-t)}^{b-1}\mathrm{d}t<\infty ;\Re \left(a\right)\wedge \Re \left(b\right)>0\right\}.$$

Theorem 3.

For all $(p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^4$ and $\nu \in \mathbb{R}$, we have

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\ge C_{\lambda ,\mu }\parallel K_{\nu +{\displaystyle \frac{1}{2}}}\left({\displaystyle \frac{p\vee q}{t^{\lambda \vee \mu }}}+{\displaystyle \frac{p\vee q}{{(1-t)}^{\lambda \vee \mu }}}\right){\parallel }_{1,\mathrm{B}},a\wedge b>0.$$

(10)

Here, we have the constant

$$C_{\lambda ,\mu }=\sqrt{(2/\pi ){\left(p^{{\displaystyle \frac{1}{\lambda \wedge \mu +1}}}+q^{{\displaystyle \frac{1}{\lambda \wedge \mu +1}}}\right)}^{\lambda \wedge \mu +1}}.$$

Proof. 

Assume $\lambda \le \mu$. Recalling the extended Beta from (2) results in

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)=\sqrt{{\displaystyle \frac{2}{\pi }}}{\int }_0^1{t}^{a-1}{(1-t)}^{b-1}\sqrt{g_{\varphi }\left(t\right)}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\varphi }\left(t\right)\right)\mathrm{d}t,$$

where we see that

$$g_{\varphi }\left(t\right)={\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\mu }}}\ge {\displaystyle \frac{p}{t^{\lambda }}}+{\displaystyle \frac{q}{{(1-t)}^{\lambda }}}:=g_{\lambda }\left(t\right)\ge \underset{t\in [0,1]}{min}{g}_{\lambda }\left(t\right)=g_{\lambda }\left(t_s\right),$$

where $t_s$ stands for the stationary point of $g_{\lambda }\left(t\right)$. It readily follows that

$$t_s={\displaystyle \frac{p^{\frac{1}{\lambda +1}}}{p^{\frac{1}{\lambda +1}}+q^{\frac{1}{\lambda +1}}}};g_{\lambda }\left(t_s\right)={\left(p^{{\displaystyle \frac{1}{\lambda +1}}}+q^{{\displaystyle \frac{1}{\lambda +1}}}\right)}^{\lambda +1}.$$

Hence,

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\ge \sqrt{{\displaystyle \frac{2}{\pi }}}\sqrt{g_{\lambda }\left(t_s\right)}{\int }_0^1{t}^{a-1}{(1-t)}^{b-1}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\mu }\left(t\right)\right)\mathrm{d}t.$$

Indeed, this estimate follows since $K_{\nu +{\displaystyle \frac{1}{2}}}\left(u\right)\searrow$ when $u>0$ and $g_{\varphi }\left(t\right)\le g_{\mu }\left(t\right)=p{t}^{-\mu }+q{(1-t)}^{-\mu }$.

To complete the proof of the lower bound (10), it is enough to show that $K_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\mu }\left(t\right)\right)\in {\mathrm{L}}_{\mathrm{B}}^1[0,1]$. Indeed, due to the behavior of the measure $t^{a-1}{(1-t)}^{b-1}\mathrm{d}t$, and the fact that $K_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\varphi }\left(t\right)\right)\in \mathrm{C}(0,1)$ is bounded, together with $\underset{t\to 0+,1-}{lim}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\varphi }\left(t\right)\right)=0$, it follows that $\parallel K_{\nu +{\displaystyle \frac{1}{2}}}\left(g_{\varphi }\left(t\right)\right){\parallel }_{1,\mathrm{B}}$ is finite.

The proof for $\lambda >\mu$ follows immediately by replacing the roles of $\lambda$ and $\mu$ above. □

Finally, another fashion inequality is formulated.

Theorem 4.

Let $(p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^4;\nu \in ,R$ and $(a-|c\left|\right)\wedge (b-|d\left|\right)\}>0$ with $a\wedge b>0$ and $c,d\in \mathbb{R}$. Then, we have

$${\left[{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\right]}^2\le {\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a+c,b+d){\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a-c,b-d).$$

(11)

Here, the equality occurs for $c=d=0$.

Proof. 

Letting $c,d>0$, which obviously preserves the generality, consider the difference

$$\Delta ={\left[{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\right]}^2-{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a+c,b+d){\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a-c,b-d),$$

By firstly rewriting the above into an integral form by permuting the variables t and s in the integrand of the second (product) expression in $\Delta$, we can obtain

$$\begin{array}{cc}\hfill {\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a+c,b+d){\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a-c,b-d)& ={\displaystyle \frac{1}{2}}{\int }_0^1{\int }_0^1{\left(ts\right)}^{a-1}{\left[(1-t)(1-s)\right]}^{b-1}[{\left({\displaystyle \frac{t}{s}}\right)}^c{\left({\displaystyle \frac{1-t}{1-s}}\right)}^d\hfill \\ \hfill & +{\left({\displaystyle \frac{s}{t}}\right)}^c{\left({\displaystyle \frac{1-s}{1-t}}\right)}^d]h_{\varphi }\left(t\right)h_{\varphi }\left(s\right)\mathrm{d}t\mathrm{d}s.\hfill \end{array}$$

Thus,

$$\Delta ={\displaystyle \frac{1}{2}}{\int }_0^1{\int }_0^1{\displaystyle \frac{{\left(ts\right)}^{a-1}}{{\left[(1-t)(1-s)\right]}^{-b+1}}}\left[2-{\left({\displaystyle \frac{t}{s}}\right)}^c{\left({\displaystyle \frac{1-t}{1-s}}\right)}^d-{\left({\displaystyle \frac{s}{t}}\right)}^c{\left({\displaystyle \frac{1-s}{1-t}}\right)}^d\right]h_{\varphi }\left(t\right)h_{\varphi }\left(s\right)\mathrm{d}t\mathrm{d}s\le 0,$$

since the obvious inequality $x+x^{-1}\ge 2,x>0$, which proves (11). □

3. Discussion and Further Remarks

A.

It is worth mentioning that ${}_{1}F_1\left(\alpha ;\alpha ;-z\right)={\mathrm{e}}^{-z}=E_1(-z)$, where the Mittag–Leffler function [19]

$$E_{\alpha }\left(z\right)=\sum _{n\ge 0}{\displaystyle \frac{z^n}{\Gamma (\alpha n+1)}},\Re \left(\alpha \right)>0,$$

whilst the Kummer-extended Beta function appears in [13] (p. 350, Equation (1.13)). However, we skip the Mittag–Leffler extension cases in this article, referring to the recent exhaustive publication [20] and the relevant references therein. Publications [21,22,23] also contain certain further information about this topic.

B.

Beta function unification involving products of two Kummer confluent hypergeometric functions, Appell hypergeometric functions of two variables, and their ’exotic’ combinations with exponentials are also considered in references [13,21,24,25].

We point out certain results by Grinshpan [26,27,28] in which he considered the Beta function transform with $h_G\left(t\right)$ built by a modulus square of integral of the following form [26] (p. 724–5, Theorem A):

$$h_G\left(t\right)=|{\int }_0^1{t}^{\alpha -1}{(1-t)}^{\beta -1}\varphi \left(\tau t\right)\psi \left(\tau (1-t)\right)\mathrm{d}t{|}^2;\alpha ,\beta >0,$$

Also see [27] (p. 188, Theorem B). Here, the integrands are built by means of continuous complex-valued functions $\varphi \left(t\right),\psi \left(t\right),t\in [0,1]$; for this transform, the author obtained a set of elegant inequalities. Moreover, he reported on the equality analysis for derived inequalities [28] (p. 188, Theorem B), which involves the values $\varphi \left(0\right),\psi \left(0\right)$.

Alternatively, our considerations involve the Macdonald kernel function $K_{\nu +{\displaystyle \frac{1}{2}}}\left(p{t}^{-\lambda }+q{(1-t)}^{-\mu }\right)$ for which the endpoints of the interval $[0,1]$ are singularities.

C.

In the proof of the Theorem 2, we can apply the obvious estimate $t(t-1)\le 4t(t-1)\le 1,t\in [0,1]$ during the minimization of the argument of the Macdonald function in (9), which results in

$$h_{\varphi }\left(t\right)\le \sqrt{{\displaystyle \frac{q}{\pi }}}{2}^{1-{\displaystyle \frac{\lambda }{2}}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(2p\right)t^{-{\displaystyle \frac{\mu }{2}}}{(1-t)}^{-{\displaystyle \frac{\mu }{2}}},\lambda \le \mu ;p\le q,$$

Therefore, this does not change the conditions of Theorem 2. This elegant observation gives the simpler upper bound

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\le 2\sqrt{{\displaystyle \frac{p\vee q}{\pi 2^{\lambda \wedge \mu }}}}{K}_{\nu +{\displaystyle \frac{1}{2}}}\left(2(p\wedge q)\right)\mathrm{B}\left(a-{\displaystyle \frac{1}{2}}(\lambda \vee \mu ),b-{\displaystyle \frac{1}{2}}(\lambda \vee \mu )\right),\lambda \vee \mu <2(a\wedge b).$$

D.

Regarding the lower bound result, by setting $\eta =\nu +\frac{1}{2}$ in Ismail’s result [29] (p. 354, Equation (1.4)) (also consult [30] (p. 718, Theorem 1. (f)))

$$x^{\eta }{K}_{\eta }\left(x\right){\mathrm{e}}^x>2^{\eta -1}\Gamma \left(\eta \right),x>0;\eta >\frac{1}{2},$$

we conclude

$$K_{\nu +{\displaystyle \frac{1}{2}}}\left(x\right)>2^{\nu -{\displaystyle \frac{1}{2}}}\Gamma \left(\nu +\frac{1}{2}\right)x^{-(\nu +{\displaystyle \frac{1}{2}})}{\mathrm{e}}^{-x},x>0;\nu >0.$$

Hence, Relation (10) of Theorem 3 becomes

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)\ge C_{\lambda ,\mu }^0\parallel {\left({\displaystyle \frac{p\vee q}{t^{\lambda \vee \mu }}}+{\displaystyle \frac{p\vee q}{{(1-t)}^{\lambda \vee \mu }}}\right)}^{-\nu -{\displaystyle \frac{1}{2}}}exp\left\{-{\displaystyle \frac{p\vee q}{t^{\lambda \vee \mu }}}-{\displaystyle \frac{p\vee q}{{(1-t)}^{\lambda \vee \mu }}}\right\}{\parallel }_{1,\mathrm{B}},\nu >0,$$

(12)

associated with the constant

$$C_{\lambda ,\mu }^0={\displaystyle \frac{2^{\nu }}{\sqrt{\pi }}}\Gamma \left(\nu +\frac{1}{2}\right)\sqrt{{\left(p^{{\displaystyle \frac{1}{\lambda \wedge \mu +1}}}+q^{{\displaystyle \frac{1}{\lambda \wedge \mu +1}}}\right)}^{\lambda \wedge \mu +1}}.$$

Thus, Inequality (12) holds for all $(p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^4$, since the norm is obviously finite.

E.

Concerning the probabilistic application of the extended Beta function and the related moment and Turánian inequalities, we mention the generalization of the distribution pioneered in [18] (p. 8 et seq., Section 4).

As a probabilistic use of ${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)$, we define a random variable (rv) $\xi$, for example, we define it on a standard probability space ($\Omega ,\mathcal{F},\mathsf{P}$). This rv is distributed according to the so-called ${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }$-extended Beta distribution, whose probability density function is given by

$$f_{\Theta }\left(x\right)={\displaystyle \frac{x^{a-1}{(1-x)}^{b-1}}{{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)}}{h}_{\varphi }\left(x\right)·{\mathsf{1}}_{(0,1)}\left(x\right),$$

where $h_{\varphi }\left(x\right)$ is accurate in (2), and $\Theta =(a,b,p,q,\lambda ,\mu )\in {\mathbb{R}}_{+}^6$; therefore, $a>\frac{\lambda }{2}>0$, $b>\frac{\mu }{2}>0$ and $\nu \in \mathbb{R}$ (see (3)). The cumulative distribution function associated with $f_{\Theta }\left(x\right)$, bearing in mind Definition 1, becomes

$$F_{\Theta }\left(x\right)={\int }_{-\infty }^x{f}_{\Theta }\left(t\right)\mathrm{d}t=\left\{\begin{array}{cc}0\hfill & x\le 0\hfill \\ {\displaystyle \frac{{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b;x)}{{\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)}}\hfill & 0<x\le 1\hfill \\ 1\hfill & x>1\hfill \end{array}\right.,$$

where the incomplete extended Beta function

$${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b;x)={\int }_0^x{t}^{a-1}{(1-t)}^{b-1}{h}_{\varphi }\left(t\right)\mathrm{d}t,x>0$$

takes place. Now, it is obvious that we should transfer the results of Section 4 from [18] to the extended Beta case.

4. Conclusions

The evolution of the Beta transform functions is presented in Section 1, pointing out the previous research related to the exponential and Macdonald-type kernels, whose arguments have singularities at the endpoints 0 and 1 of Euler’s Beta integral integration domain $[0,1]$. Links are given to Mittag–Leffler-type kernels in [21,22,23], whilst the double-integral-type kernels, exploited in Grishpan’s articles, are briefly discussed in Part $\mathsf{B}$ of the discussion Section 3. The difference between our considerations and the latter is that in [26,27,28], the input functions in the integrand are piecewise continuous-, or continuous complex-valued. With this work, we supplement the Macdonald- type kernel extensions of the Beta integrals.

The main results are given in Section 2: Theorem 1 provides an upper bound for the newly introduced Beta function with four parameters in the Macdonald-type kernel function, emphasizing the case $\lambda \ne \mu$ (consult Definition 1). To prove these results, we used the fixed-point iteration method. Theorem 2 provides another fashion upper bound, intervening with the A–G inequalities in the integrand of the input-extended Beta integral. Theorem 3 provides a lower bound for the extended Beta function ${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b)$. Finally, Theorem 4 presents a Turán-type inequality with respect the initial Euler parameters $a,b$ in (1).

Parts $\mathsf{A}$–$\mathsf{E}$ in the Discussion and Further Remarks section contain additional explanations, links to similar problems in the literature, some open questions, and an interpretation of the newly defined extended Beta function in probability. More precisely, in Part $\mathsf{E}$ of Section 3, the probability density function $f_{\Theta }$ generates the associated cumulative distribution function $F_{\Theta }$, which turns out to be the normalized incomplete extended Beta function ${\mathrm{B}}_{p,q,\nu }^{\lambda ,\mu }(a,b;x)$. However, the study of mathematical properties of such functions will be the subject of a future research study.