Interweaving the Principle of Least Potential Energy in School and Introductory University Physics Courses
Abstract
:1. Introduction
2. Description of the Principle of Least Potential Energy
3. Examples Demonstrating the Use of the Least Potential Energy Principle
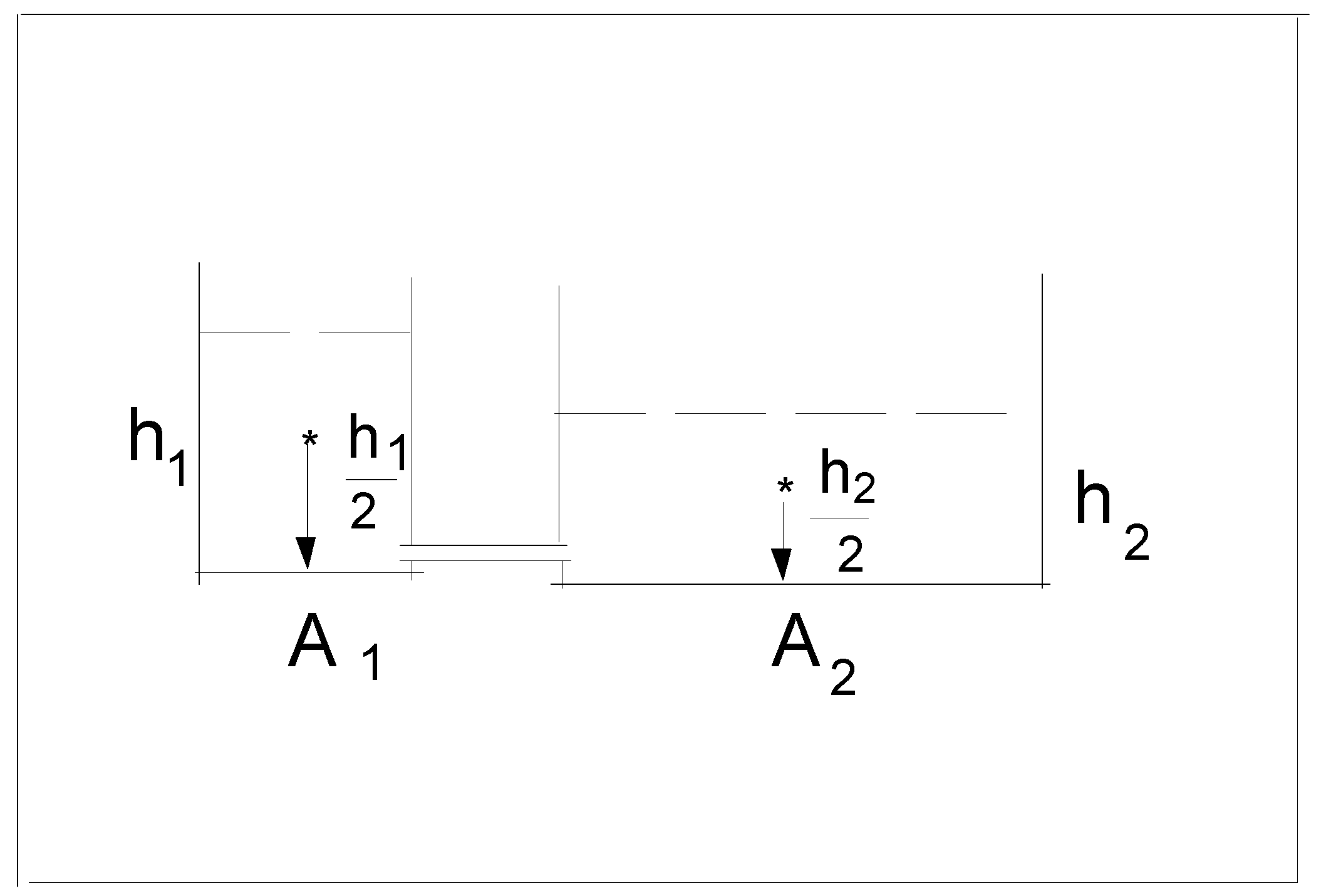
3.1. Example a: Fluid Static in a Piston of Small Cross-Sectional Area
3.1.1. Solution Based on Newton’s Laws
3.1.2. Solution Based on the Least Potential Energy Principle
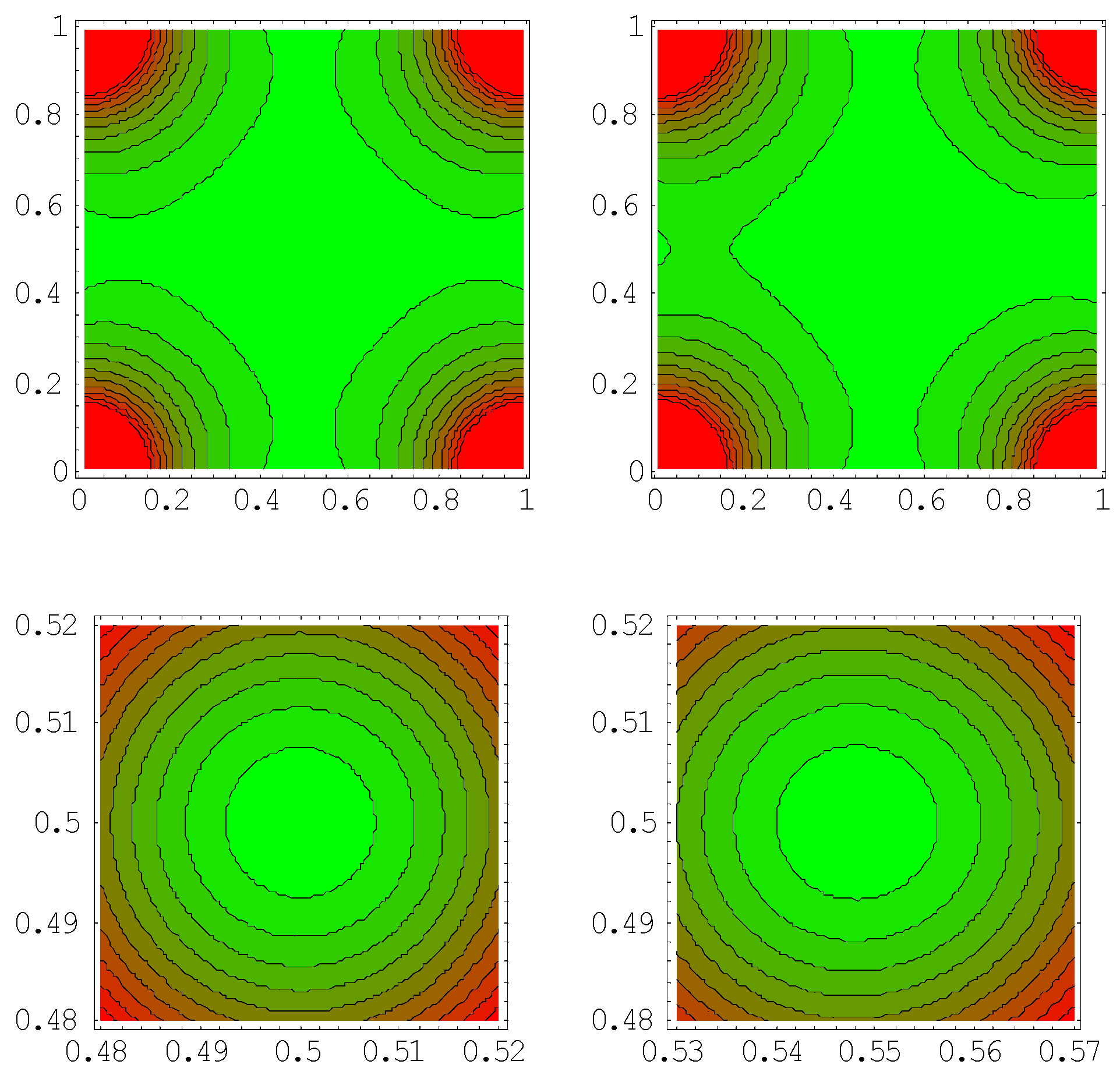
3.2. Example b: A System of Point Charges
3.2.1. Solution Based on Newton’s Laws
3.2.2. Solution Based on the Least Potential Energy Principle
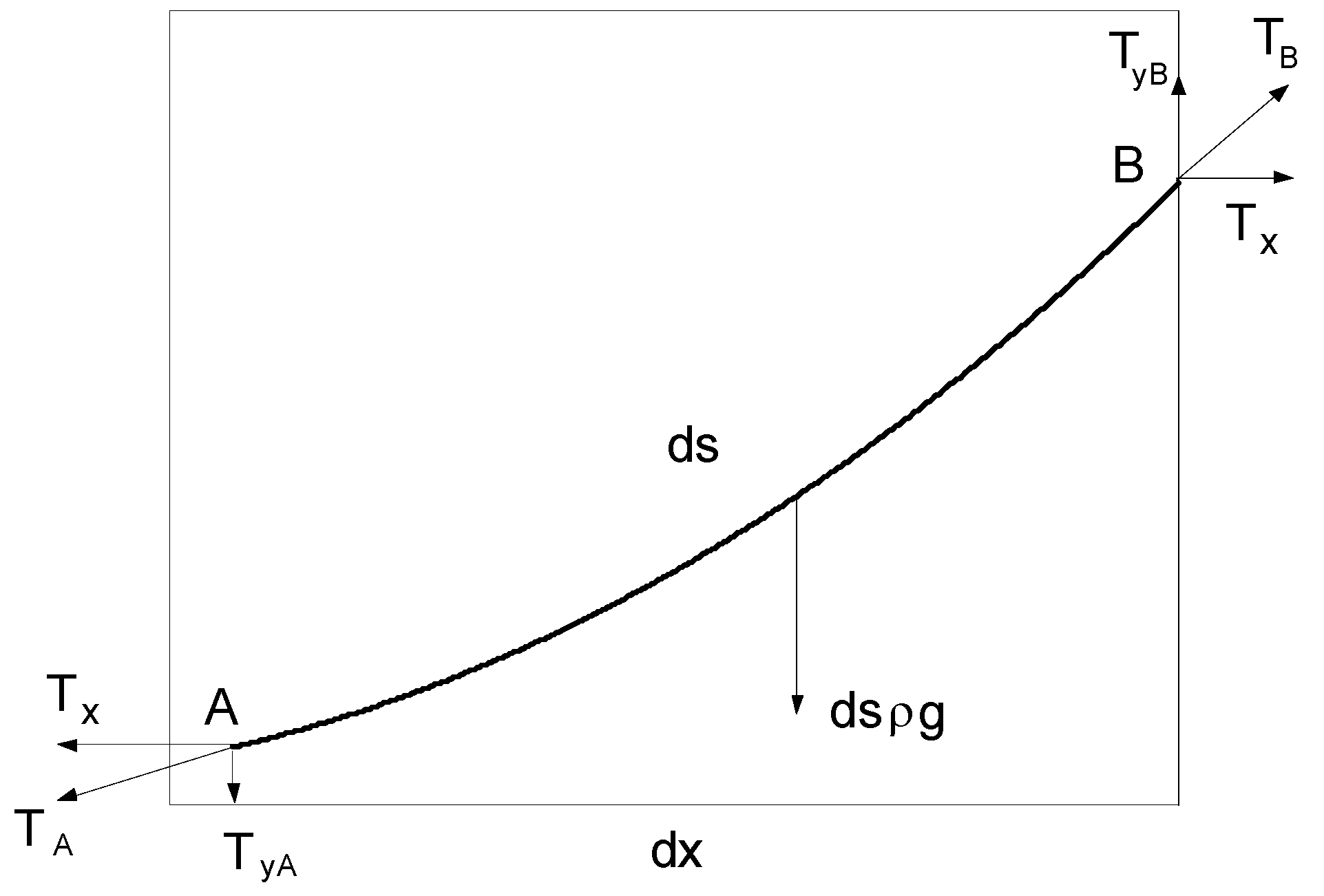
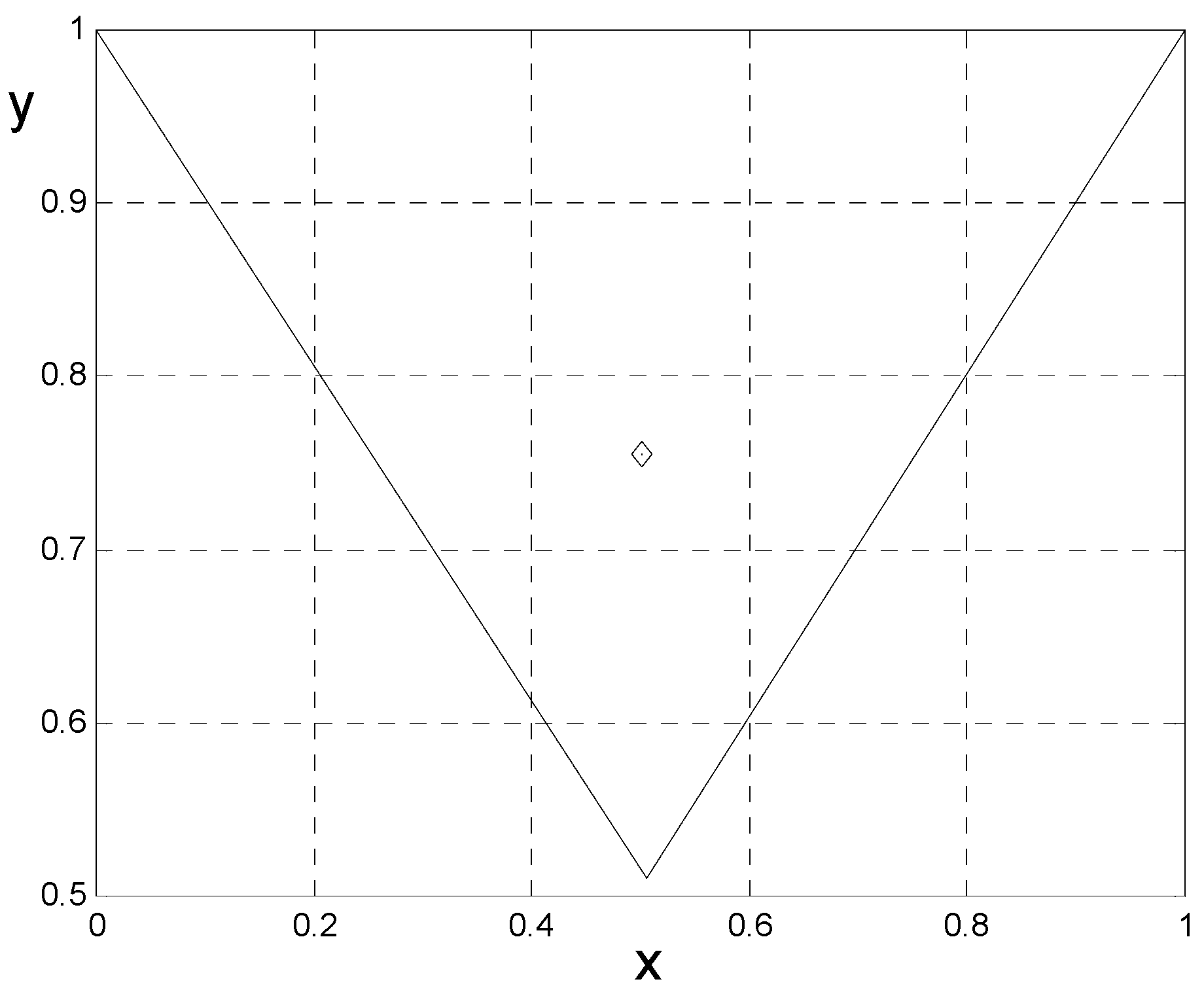
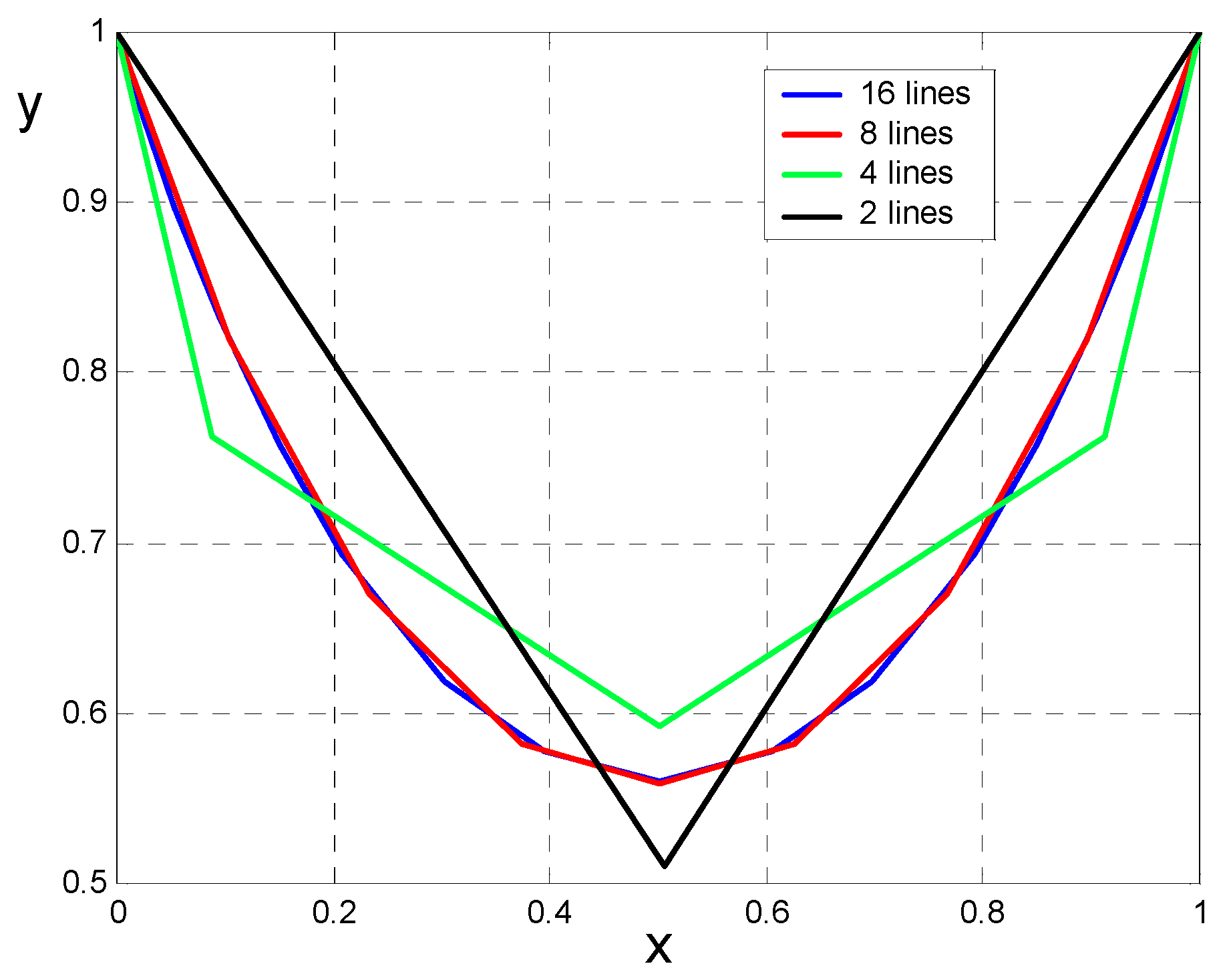
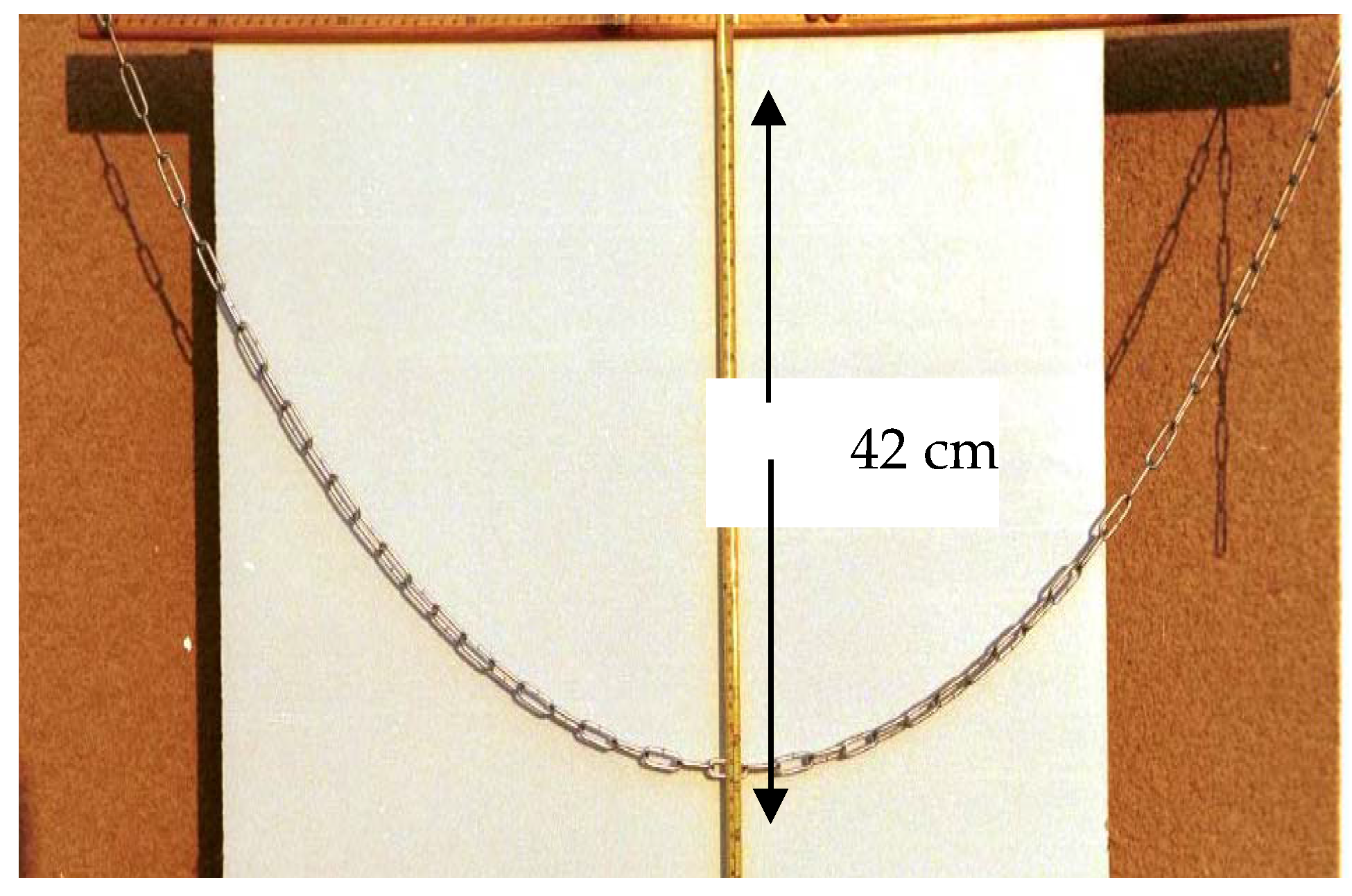
3.3. Example c: The Catenary Problem
3.3.1. Solution Based on Newton’s Laws
3.3.2. Solution Based on the Least Potential Energy Principle
4. Conclusions
Author Contributions
Conflicts of Interest
References
- Hilderbrandt, S.; Tromba, A. The Persimmons Universe; Springer: New York, NY, USA, 1996; p. 241. [Google Scholar]
- Duit, R. Should energy be illustrated as something quasi-material? Int. J. Sci. Educ. 1987, 9, 139–145. [Google Scholar] [CrossRef]
- Lemons, D.S. Perfect Form; Princeton University Press: Princeton, NJ, USA, 1997. [Google Scholar]
- Hanc, J.; Tuleja, S.; Hancova, M.; Derives, M. Simple derivation of Newtonian mechanics from the principle of least action. Am. J. Phys. 2003, 71, 386–391. [Google Scholar] [CrossRef]
- Glass, B.; Temkin, O.; Straus, V. Forerunners of Darwin; Johns Hopkins University Press: Baltimore, MD, USA, 1959. [Google Scholar]
- Hilderbrandt, S.; Tromba, A. The Persimmons Universe; Springer: New York, NY, USA, 1996. [Google Scholar]
- Meriam, J.L.; Kraige, L.G. Engineering Mechanics, Statics; John Wiley & Sons, Inc.: New York, NY, USA, 2002; p. 287. [Google Scholar]
- Dai, C.; Renner, B.; Doyle, D.S. Orogin of metastable knot in single flexible chain. Phys. Rev. Lett. 2015, 114, 1–5. [Google Scholar] [CrossRef] [PubMed]
- Antman, S.S. The equations for large vibrations of strings. Amer. Math. Mon. 1980, 87, 359–370. [Google Scholar] [CrossRef]
- Bailey, H. Motion of a hanging chain after the free end is given an initial velocity. Amer. J. Phys. 2000, 68, 764–767. [Google Scholar] [CrossRef]
- Belmonte, A.; Shelley, M.J.; Eldakar, S.T.; Wiggins, C.H. Dynamic patterns and self-knotting of a driven hanging chain. Phys. Rev. Lett. 2001, 87, 114301. [Google Scholar] [CrossRef] [PubMed]
- Feynman, R.P.; Leighton, R.B.; Sands, M.; Lindsay, R.B. The Feynman Lectures on Physics; Addison-Wesley: Boston, MA, USA, 1964; Chapter 19; pp. 344–456. [Google Scholar]
- Wolfram, S. The Mathematica Book, 3rd ed.; Wolfram Media/Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Mareno, A.; English, L. The stability of the catenary shapes for a hanging cable of unspecified length. Eur. J. Phys. 2008, 30, 97. [Google Scholar] [CrossRef]
- Fallis, M.C. Hanging shapes of nonuniform cables. Am. J. Phys. 1997, 65, 117–122. [Google Scholar] [CrossRef]
- Denzler, J.; Hinz, A.M. Catenaria Vera—The True Catenary. Expo. Math. 1999, 17, 117–142. [Google Scholar]
- De Sapio, V.; Khatib, O.; Delp, S. Least action principles and their application to constrained and task-level problems in robotics and biomechanics. Multibody Syst. Dyn. 2008, 19, 303–322. [Google Scholar] [CrossRef]
- Principle Of Least Action Interactive. Available online: http://www.eftaylor.com/software/ActionApplets/LeastAction.html (accessed on 12 March 2003).
- Taylor, E.F. A call for action. Am. J. Phys. 2003, 71, 423. [Google Scholar] [CrossRef]
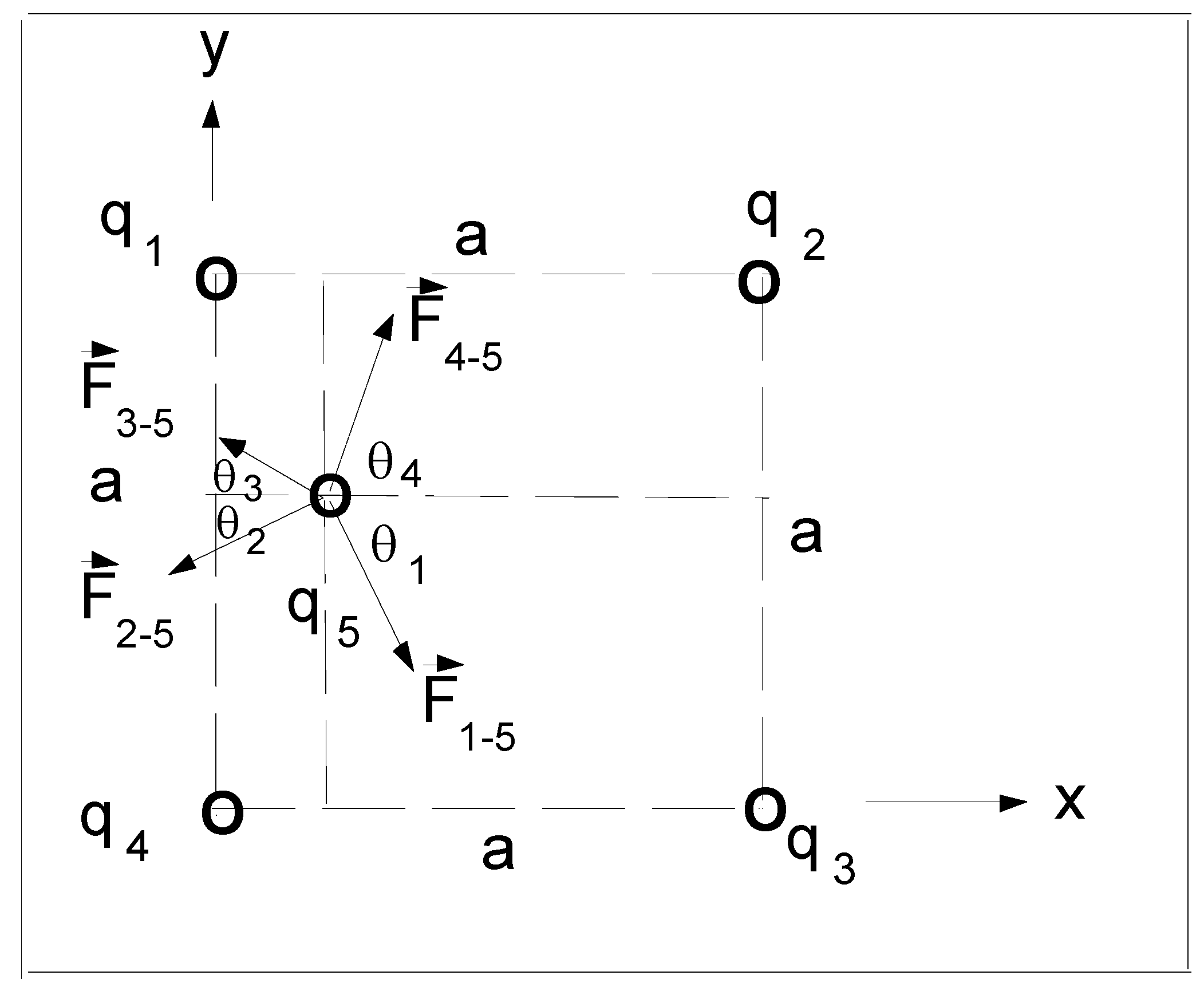






© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ben-Abu, Y.; Eshach, H.; Yizhaq, H. Interweaving the Principle of Least Potential Energy in School and Introductory University Physics Courses. Symmetry 2017, 9, 45. https://doi.org/10.3390/sym9030045
Ben-Abu Y, Eshach H, Yizhaq H. Interweaving the Principle of Least Potential Energy in School and Introductory University Physics Courses. Symmetry. 2017; 9(3):45. https://doi.org/10.3390/sym9030045
Chicago/Turabian StyleBen-Abu, Yuval, Haim Eshach, and Hezi Yizhaq. 2017. "Interweaving the Principle of Least Potential Energy in School and Introductory University Physics Courses" Symmetry 9, no. 3: 45. https://doi.org/10.3390/sym9030045




