Temperature Resistance of Mo3Si: Phase Stability, Microhardness, and Creep Properties
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
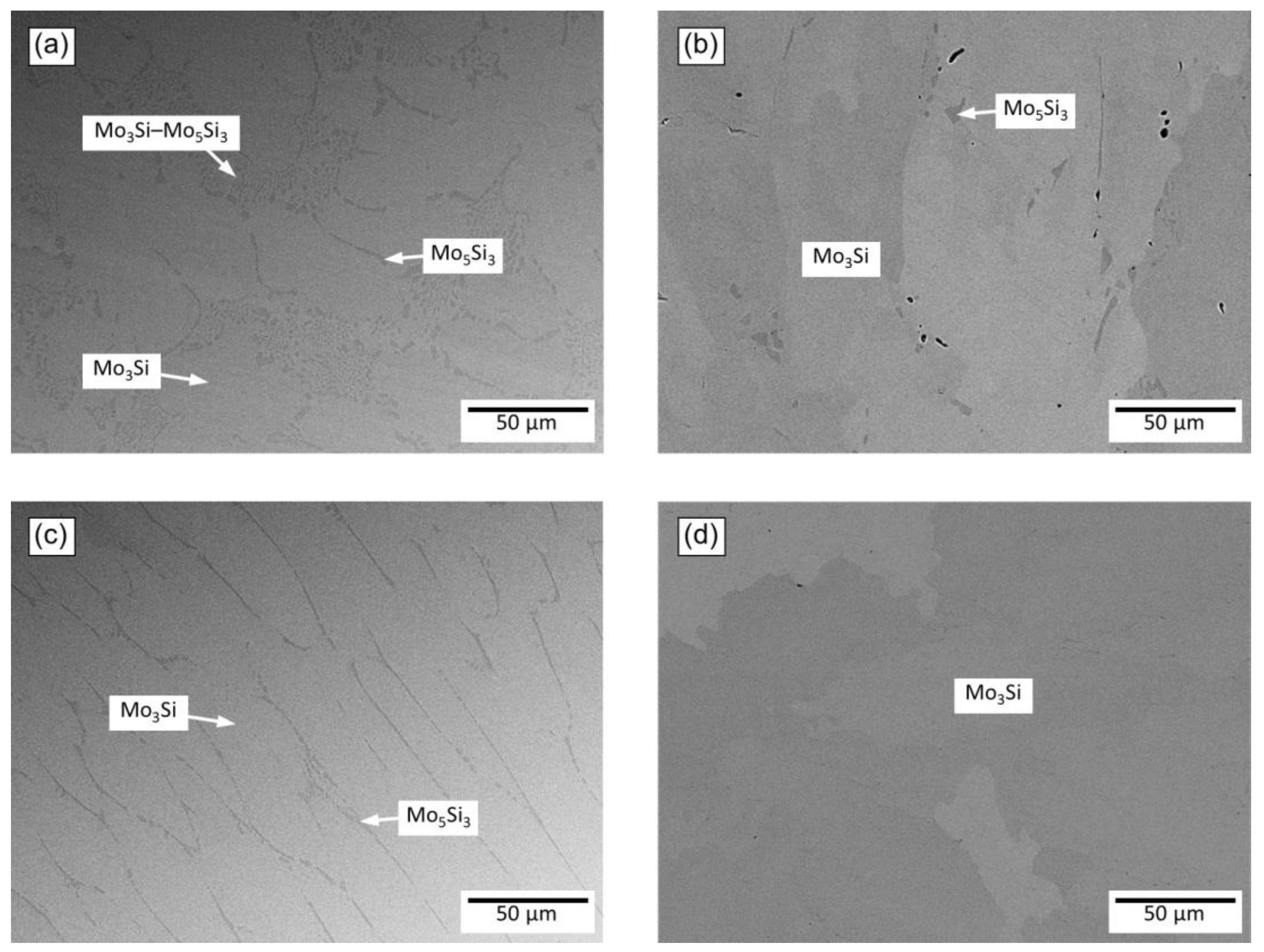
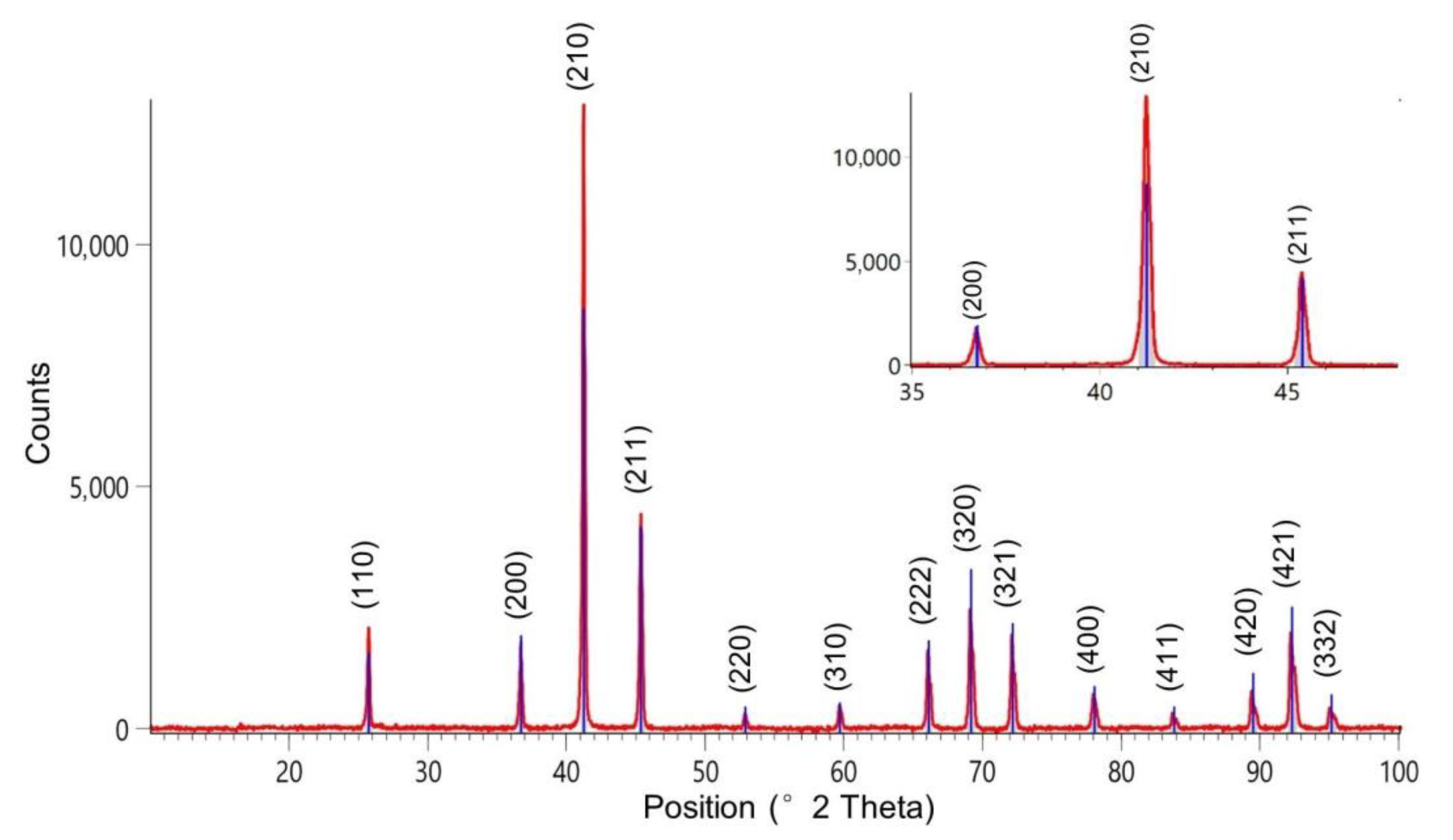
3.1. Microstructure
3.2. Microhardness
3.3. Constant Load Compressive Creep Tests
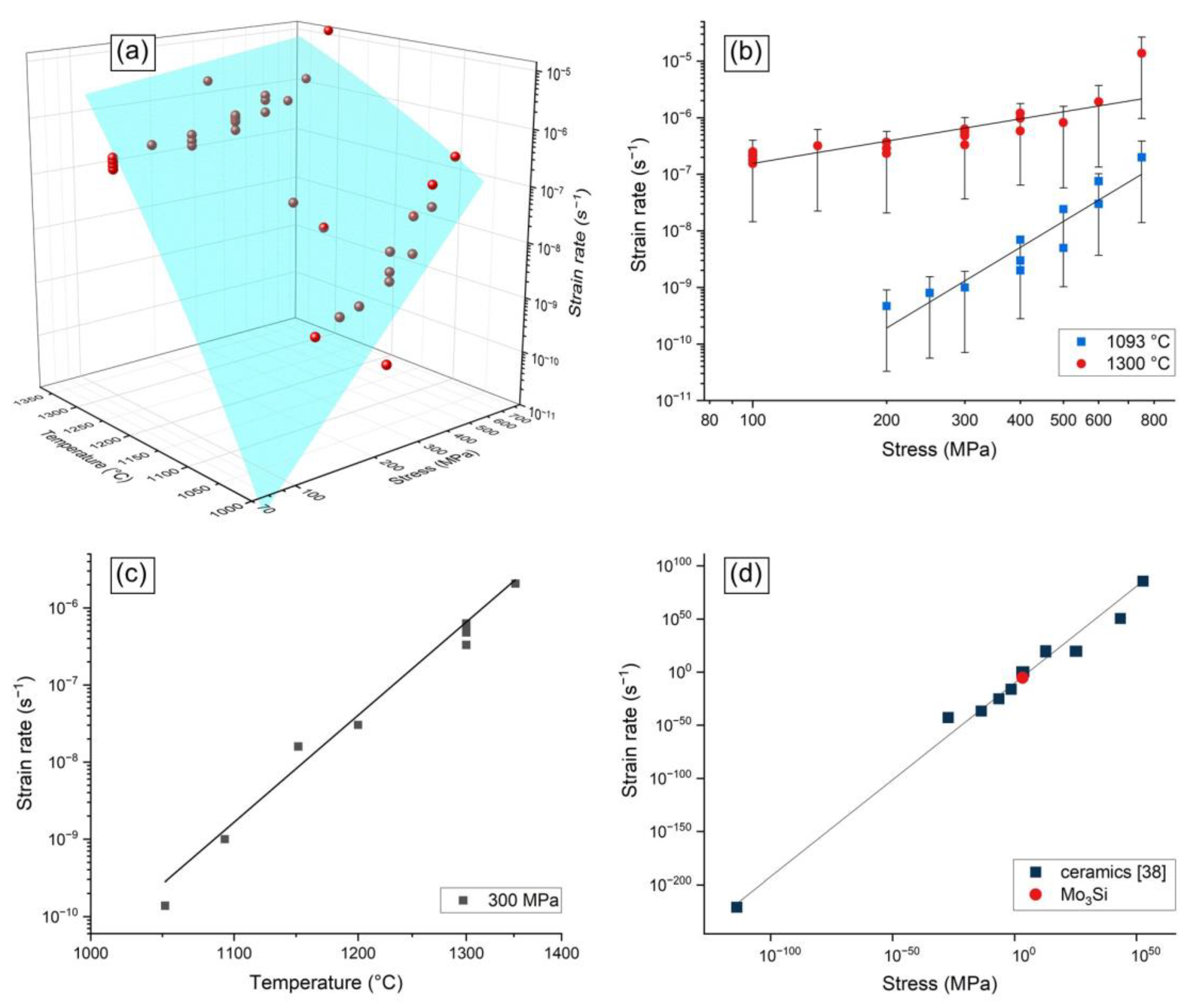
3.3.1. Experimental Results
3.3.2. Regression with Power Law with Constant Creep Exponent
3.3.3. Regression with Stress Range Dependent Constitutive Model
3.3.4. Regression with Power Law Using Temperature-Dependent Creep Exponent
4. Discussion on Creep Mechanism
- Regressions with (i) power law with constant creep exponent and with (ii) power law with temperature-dependent creep exponent
- regression with stress range dependent constitutive model.
4.1. Regressions with (i) Power Law with Constant Creep Exponent in Section 3.3.2 and with (ii) Power Law with Temperature-Dependent Creep Exponent in Section 3.3.4
4.2. Regression with Stress Range Dependent Constitutive Model in Section 3.3.3
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature and Abbreviations List
| Abbreviations | |
| BSE | backscattered electrons |
| EDM | electrical discharge machining |
| EDX | energy dispersive X-Ray |
| SE | secondary electrons |
| SEM | scanning electron microscope |
| XRD | X-ray diffraction |
| Latin Letters | |
| A | material constant |
| a | material constant |
| B | material constant |
| b | material constant |
| c | material constant |
| d | material constant |
| E | Young modulus |
| n | material constant |
| Q | activation energy for the mechanism involved in the deformation process |
| Qa | activation energy of linear creep |
| Qb | activation energy of power law creep |
| R | gas constant |
| R-squared values | |
| T | test temperature |
| Tm | melting temperature |
| Greek Letters | |
| minimum creep rate | |
| material constant | |
| εp | pivot point creep rate |
| σ | stress |
| σ0 | material constant |
| σp | pivot point stress |
References
- Lemberg, J.A.; Ritchie, R.O. Mo-Si-B Alloys for Ultrahigh-Temperature Structural Applications. Adv. Mater. 2012, 24, 3445–3480. [Google Scholar] [CrossRef]
- Dimiduk, D.M.; Perepezko, J.H. Mo-Si-B Alloys: Developing a Revolutionary Turbine-Engine Material. MRS Bull. 2003, 28, 639–645. [Google Scholar] [CrossRef]
- Mitra, R. 5-Molybdenum silicide-based composites. In Intermetallic Matrix Composites; Mitra, R., Ed.; Woodhead Publishing: Cambridge, UK, 2018; pp. 95–146. ISBN 978-0-85709-346-2. [Google Scholar]
- Rosales, I.; Schneibel, J.H. Stoichiometry and Mechanical Properties of Mo3Si. Intermetallics 2000, 8, 885–889. [Google Scholar] [CrossRef]
- Zhang, L.; Pan, K.; Lin, J. Fracture Toughness and Fracture Mechanisms in Mo5SiB2 at Ambient to Elevated Temperatures. Intermetallics 2013, 38, 49–54. [Google Scholar] [CrossRef]
- Liu, C.T.; Schneibel, J.H.; Heatherly, L. Processing, Microstructure, and Properties of Multiphase Mo Silicide Alloys. MRS Online Proc. Libr. OPL 1998, 552. [Google Scholar] [CrossRef] [Green Version]
- Schneibel, J.H.; Liu, C.T.; Easton, D.S.; Carmichael, C.A. Microstructure and Mechanical Properties of Mo–Mo3Si–Mo5SiB2 Silicides. Mater. Sci. Eng. A 1999, 261, 78–83. [Google Scholar] [CrossRef]
- Schneibel, J.H.; Kramer, M.J.; Ünal, Ö.; Wright, R.N. Processing and Mechanical Properties of a Molybdenum Silicide with the Composition Mo–12Si–8.5B (at %). Intermetallics 2001, 9, 25–31. [Google Scholar] [CrossRef]
- Schneibel, J.H.; Kramer, M.J.; Easton, D.S. A Mo–Si–B Intermetallic Alloy with a Continuous α-Mo Matrix. Scr. Mater. 2002, 46, 217–221. [Google Scholar] [CrossRef]
- Choe, H.; Schneibel, J.H.; Ritchie, R.O. On the Fracture and Fatigue Properties of Mo-Mo3Si-Mo5SiB2 Refractory Intermetallic Alloys at Ambient to Elevated Temperatures (25 °C to 1300 °C). Metall. Mater. Trans. A 2003, 34, 225–239. [Google Scholar] [CrossRef]
- Kruzic, J.J.; Schneibel, J.H.; Ritchie, R.O. Fracture and Fatigue Resistance of Mo–Si–B Alloys for Ultrahigh-Temperature Structural Applications. Scr. Mater. 2004, 50, 459–464. [Google Scholar] [CrossRef] [Green Version]
- Schneibel, J.H.; Tortorelli, P.F.; Ritchie, R.O.; Kruzic, J.J. Optimization of Mo-Si-B Intermetallic Alloys. Metall. Mater. Trans. A 2005, 36, 525–531. [Google Scholar] [CrossRef]
- Mitra, R.; Srivastava, A.K.; Eswara Prasad, N.; Kumari, S. Microstructure and Mechanical Behaviour of Reaction Hot Pressed Multiphase Mo–Si–B and Mo–Si–B–Al Intermetallic Alloys. Intermetallics 2006, 14, 1461–1471. [Google Scholar] [CrossRef]
- Choe, H.; Chen, D.; Schneibel, J.H.; Ritchie, R.O. Ambient to High Temperature Fracture Toughness and Fatigue-Crack Propagation Behavior in a Mo–12Si–8.5B (at %) Intermetallic. Intermetallics 2001, 9, 319–329. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Jackson, M.R.; Gigliotti, M.F.X. Niobium Silicide High Temperature In Situ Composites. In Intermetallic Compounds—Principles and Practice; John Wiley & Sons, Ltd.: London, UK, 2002; pp. 541–560. ISBN 978-0-470-84585-1. [Google Scholar]
- Schneibel, J.H.; Lin, H.T. Creep Properties of Molybdenum Silicide Intermetallics Containing Boron. Mater. High Temp. 2002, 19, 25–28. [Google Scholar] [CrossRef]
- Hasemann, G.; Kaplunenko, D.; Bogomol, I.; Krüger, M. Near-Eutectic Ternary Mo-Si-B Alloys: Microstructures and Creep Properties. JOM 2016, 68, 2847–2853. [Google Scholar] [CrossRef]
- Jain, P.; Kumar, K.S. Tensile Creep of Mo–Si–B Alloys. Acta Mater. 2010, 58, 2124–2142. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Jackson, M.R.; Subramanian, P.R.; Zhao, J.-C. A Review of Very-High-Temperature Nb-Silicide-Based Composites. Metall. Mater. Trans. A 2003, 34, 2043–2052. [Google Scholar] [CrossRef]
- Bewlay, B.P.; Jackson, M.R.; Zhao, J.-C.; Subramanian, P.R.; Mendiratta, M.G.; Lewandowski, J.J. Ultrahigh-Temperature Nb-Silicide-Based Composites. MRS Bull. 2003, 28, 646–653. [Google Scholar] [CrossRef]
- Schneibel, J.H. Beyond Nickel-Base Superalloys. In Processing and Fabrication of Advanced Materials XIII, Proceedings of the Conference Organized by National University of Singapore [NUS], Singapore Institute of Manufacturing Technology, Co-Sponsored by American Society for Materials International (ASM Int.) (The Materials Information Society, USA), Singapore, 6–8 December 2004; Gupta, M., Srivatsan, T.S., Lim, C.Y.H., Varin, R.A., Eds.; Stallion Press: Singapore, 2005; Volume 2, pp. 563–574. ISBN 981-05-3000-5. [Google Scholar]
- Kauss, O.; Tsybenko, H.; Naumenko, K.; Hütter, S.; Krüger, M. Structural Analysis of Gas Turbine Blades Made of Mo-Si-B under Transient Thermo-Mechanical Loads. Comput. Mater. Sci. 2019, 165, 129–136. [Google Scholar] [CrossRef]
- Kauss, O.; Naumenko, K.; Hasemann, G.; Krüger, M. Structural Analysis of Gas Turbine Blades Made of Mo-Si-B Under Stationary Thermo-Mechanical Loads. In Advances in Mechanics of High-Temperature Materials; Naumenko, K.D., Krüger, M., Eds.; Advanced Structured Materials; Springer: Cham, Switzerland, 2020; Volume 117, pp. 79–91. ISBN 978-3-030-23868-1. [Google Scholar]
- Krüger, M.; Kauss, O.; Naumenko, K.; Burmeister, C.; Wessel, E.; Schmelzer, J. The Potential of Mechanical Alloying to Improve the Strength and Ductility of Mo–9Si–8B–1Zr Alloys—Experiments and Simulation. Intermetallics 2019, 113, 106558. [Google Scholar] [CrossRef]
- Gokhale, A.B.; Abbaschian, G.J. The Mo-Si (Molybdenum-Silicon) System. J. Phase Equilibria 1991, 12, 493–498. [Google Scholar] [CrossRef]
- Gnesin, I.; Gnesin, B. Composition of the Mo-Mo3Si Alloys Obtained via Various Methods. Int. J. Refract. Met. Hard Mater. 2020, 88, 105188. [Google Scholar] [CrossRef]
- Bolbut, V.; Bogomol, I.; Loboda, P.; Krüger, M. Microstructure and Mechanical Properties of a Directionally Solidified Mo-12Hf-24B Alloy. J. Alloys Compd. 2018, 735, 2324–2330. [Google Scholar] [CrossRef]
- Bolbut, V.; Bogomol, I.; Bauer, C.; Krüger, M. Gerichtet erstarrte Mo-Zr-B-Legierungen. Mater. Werkst. 2017, 48, 1113–1124. [Google Scholar] [CrossRef]
- Ha, S.-H.; Yoshimi, K.; Maruyama, K.; Tu, R.; Goto, T. Compositional Regions of Single Phases at 1800 °C in Mo-Rich Mo–Si–B Ternary System. Mater. Sci. Eng. A 2012, 552, 179–188. [Google Scholar] [CrossRef]
- Frost, H.J.; Ashby, M.F. Deformation-Mechanism Maps: The Plasticity and Creep of Metals and Ceramics; Pergamon Press: Oxford, UK, 1982; ISBN 978-0-08-029338-7. [Google Scholar]
- Hasemann, G.; Bogomol, I.; Schliephake, D.; Loboda, P.I.; Krüger, M. Microstructure and Creep Properties of a Near-Eutectic Directionally Solidified Multiphase Mo–Si–B Alloy. Intermetallics 2014, 48, 28–33. [Google Scholar] [CrossRef]
- Hayashi, T.; Ito, K.; Ihara, K.; Fujikura, M.; Yamaguchi, M. Creep of Single Crystalline and Polycrystalline T2 Phase in the Mo–Si–B System. Intermetallics 2004, 12, 699–704. [Google Scholar] [CrossRef]
- Hasemann, G. Microstructure and Properties of Near-Eutectic Mo-Si-B Alloys for High Temperature Applications. Ph.D. Thesis, Otto-von-Guericke-Universität, Magdeburg, Germany, 2017. [Google Scholar]
- Schneibel, J.H. High Temperature Strength of Mo–Mo3Si–Mo5SiB2 Molybdenum Silicides. Intermetallics 2003, 11, 625–632. [Google Scholar] [CrossRef]
- Naumenko, K.; Altenbach, H. Foundations of Engineering Mechanics. In Modeling of Creep for Structural Analysis; Springer: Berlin/Heidelberg, Germany, 2007; ISBN 978-3-540-70834-6. [Google Scholar]
- Penny, R.K.; Marriott, D.L. Design for Creep, 2nd ed.; Springer: Dordrecht, The Netherlands, 1995; ISBN 978-0-412-59040-5. [Google Scholar]
- Naumenko, K.; Altenbach, H.; Gorash, Y. Creep Analysis with a Stress Range Dependent Constitutive Model. Arch. Appl. Mech. 2009, 79, 619–630. [Google Scholar] [CrossRef]
- Iost, A. The Correlation between the Power-Law Coefficients in Creep: The Temperature Dependence. J. Mater. Sci. 1998, 33, 3201–3206. [Google Scholar] [CrossRef]
- Prasad, S.; Paul, A. Growth Mechanism of Phases by Interdiffusion and Atomic Mechanism of Diffusion in the Molybdenum–Silicon System. Intermetallics 2011, 19, 1191–1200. [Google Scholar] [CrossRef]
- Maier, H.J.; Niendorf, T.; Bürgel, R. Handbuch Hochtemperatur-Werkstofftechnik: Grundlagen, Werkstoffbeanspruchungen, Hochtemperaturlegierungen Und-Beschichtungen; Springer Vieweg: Berlin, Germany; Springer Fachmedien Wiesbaden GmbH: Wiesbaden, Germany, 2019; ISBN 978-3-658-25314-1. [Google Scholar]
- Murty, K.L.; Gollapudi, S.; Ramaswamy, K.; Mathew, M.D.; Charit, I. Creep deformation of materials in light water reactors (LWRs). In Materials Ageing and Degradation in Light Water Reactors; Elsevier: Paris, France, 2013; pp. 81–148. ISBN 978-0-85709-239-7. [Google Scholar]
- Sherby, O.D.; Burke, P.M. Mechanical Behavior of Crystalline Solids at Elevated Temperature. Prog. Mater. Sci. 1968, 13, 323–390. [Google Scholar] [CrossRef]
- Pharr, G.M. Some Observations on the Relation between Dislocation Substructure and Power Law Breakdown in Creep. Scr. Metall. 1981, 15, 713–717. [Google Scholar] [CrossRef]
- Poirier, J.P. Is Power-Law Creep Diffusion-Controlled? Acta Metall. 1978, 26, 629–637. [Google Scholar] [CrossRef]








| T | n | ||
|---|---|---|---|
| 1093 °C | 4.6 | 5.08 × 10−21 | 0.878 |
| 1300 °C | 1.3 | 3.85 × 10−10 | 0.722 |
| n | |||||
|---|---|---|---|---|---|
| 7 | 2.68 × 1013 | 2129 | 590 | 19 | 0.981 |
| 13 | 1.77 × 1013 | 2364 | 577 | 17 | 0.998 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kauss, O.; Obert, S.; Bogomol, I.; Wablat, T.; Siemensmeyer, N.; Naumenko, K.; Krüger, M. Temperature Resistance of Mo3Si: Phase Stability, Microhardness, and Creep Properties. Metals 2021, 11, 564. https://doi.org/10.3390/met11040564
Kauss O, Obert S, Bogomol I, Wablat T, Siemensmeyer N, Naumenko K, Krüger M. Temperature Resistance of Mo3Si: Phase Stability, Microhardness, and Creep Properties. Metals. 2021; 11(4):564. https://doi.org/10.3390/met11040564
Chicago/Turabian StyleKauss, Olha, Susanne Obert, Iurii Bogomol, Thomas Wablat, Nils Siemensmeyer, Konstantin Naumenko, and Manja Krüger. 2021. "Temperature Resistance of Mo3Si: Phase Stability, Microhardness, and Creep Properties" Metals 11, no. 4: 564. https://doi.org/10.3390/met11040564








