Evaluating the Response Time of an Optical Gas Sensor Based on Gasochromic Nanostructures
Abstract
:1. Introduction
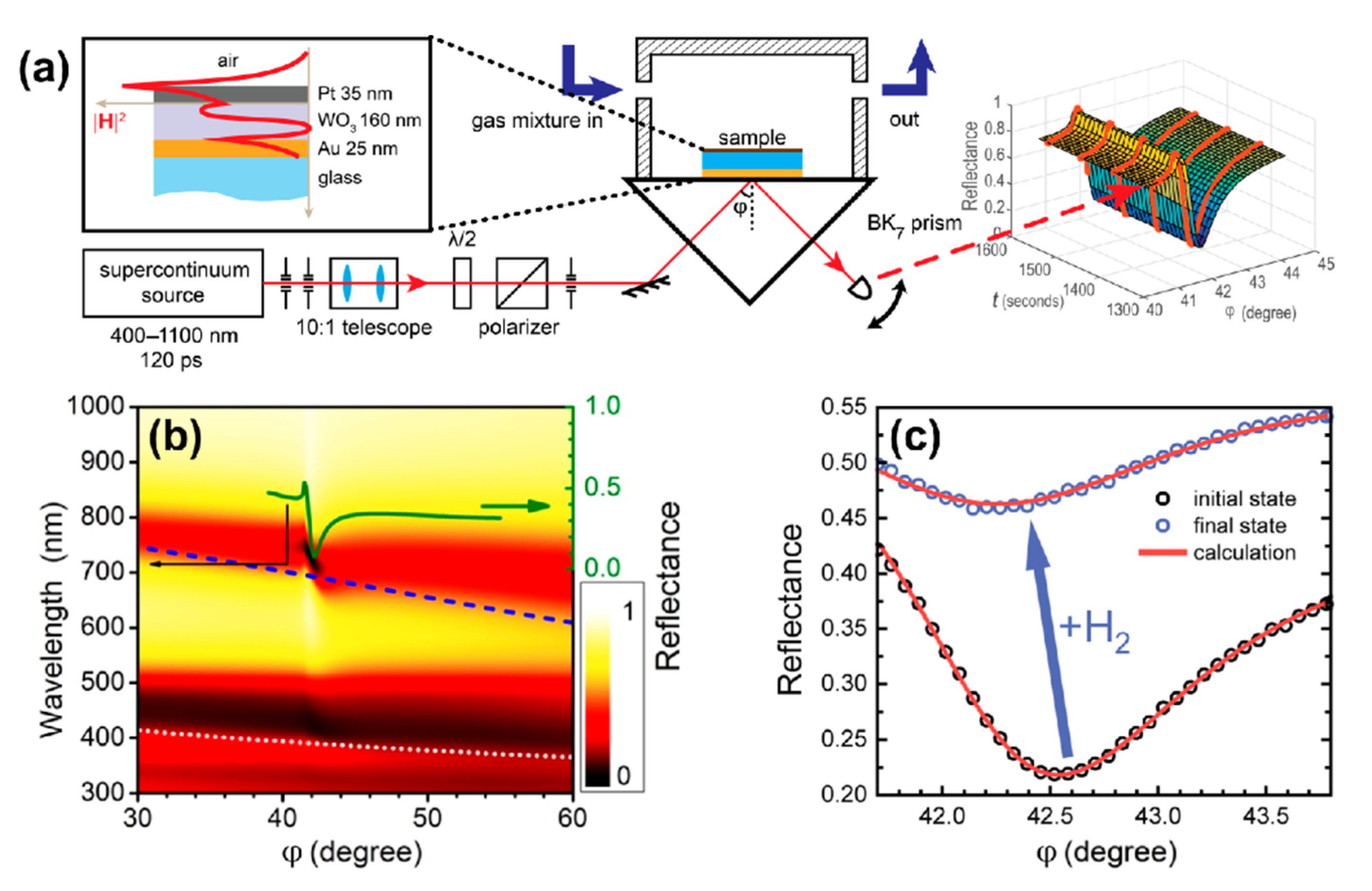
2. Experimental and Calculation Details
3. Sensing Element Response in Case of Monochromatic Light
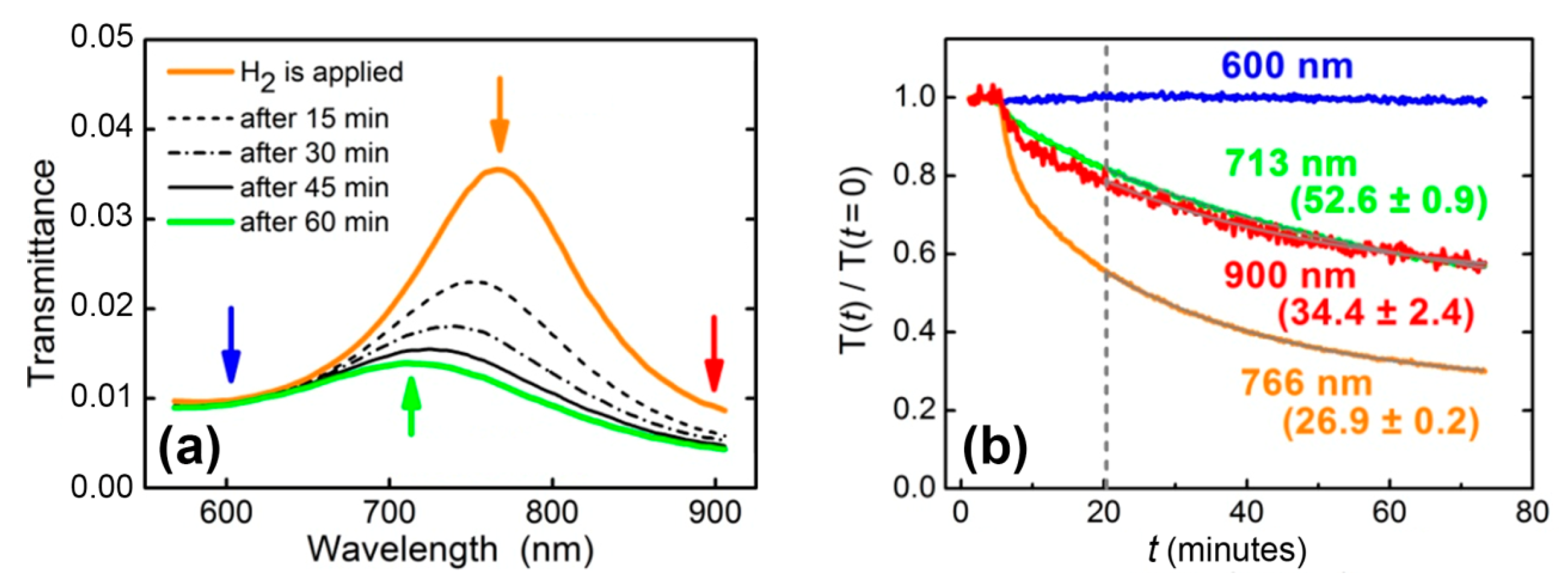
4. Influence of Spectral Features on Sensing Element Response Times
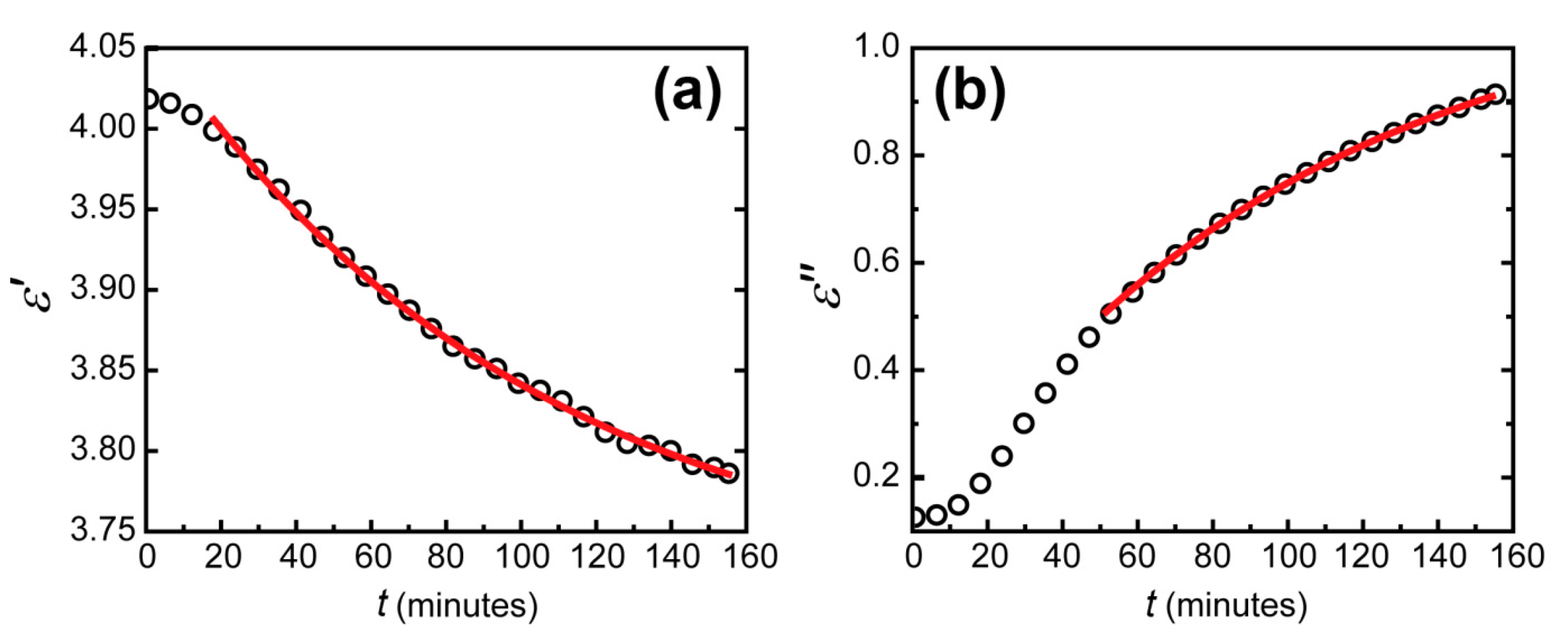
5. Time-Dependent Refractive Index of Tungsten Trioxide Interacting with Hydrogen
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, X.; Cheng, S.; Liu, H.; Hu, S.; Zhang, D.; Ning, H. A survey on gas sensing technology. Sensors 2012, 12, 9635–9665. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, S.; Jiang, C.; Wei, S.-H. Gas sensing in 2D materials. Appl. Phys. Rev. 2017, 4, 021304. [Google Scholar] [CrossRef]
- Hodgkinson, J.; Tatam, R.P. Optical gas sensing: A review. Meas. Sci. Technol. 2012, 24, 012004. [Google Scholar] [CrossRef] [Green Version]
- Kohl, C.-D.; Wagner, T. Gas Sensing Fundamentals; Springer: Heidelberg, Germany, 2014; Volume 15. [Google Scholar]
- Hübner, M.; Simion, C.; Haensch, A.; Barsan, N.; Weimar, U. CO sensing mechanism with WO3 based gas sensors. Sens. Actuators B Chem. 2010, 151, 103–106. [Google Scholar] [CrossRef]
- Aleixandre, M.; Gerboles, M. Review of small commercial sensors for indicative monitoring of ambient gas. Chem. Eng. Trans 2012, 30. [Google Scholar] [CrossRef]
- Wusiman, M.; Taghipour, F. Methods and mechanisms of gas sensor selectivity. Crit. Rev. Solid State Mater. Sci. 2021, 1–20. [Google Scholar] [CrossRef]
- Krivetskiy, V.; Malkov, I.; Garshev, A.; Mordvinova, N.; Lebedev, O.; Dolenko, S.; Efitorov, A.; Grigoriev, T.; Rumyantseva, M.; Gaskov, A. Chemically modified nanocrystalline SnO2-based materials for nitrogen-containing gases detection using gas sensor array. J. Alloy. Compd. 2017, 691, 514–523. [Google Scholar] [CrossRef]
- Krivetskiy, V.; Efitorov, A.; Arkhipenko, A.; Vladimirova, S.; Rumyantseva, M.; Dolenko, S.; Gaskov, A. Selective detection of individual gases and CO/H2 mixture at low concentrations in air by single semiconductor metal oxide sensors working in dynamic temperature mode. Sens. Actuators B Chem. 2018, 254, 502–513. [Google Scholar] [CrossRef]
- Shi, J.; Zhu, Y.; Zhang, X.; Baeyens, W.R.; García-Campaña, A.M. Recent developments in nanomaterial optical sensors. Trends Anal. Chem. 2004, 23, 351–360. [Google Scholar] [CrossRef]
- Tittl, A.; Giessen, H.; Liu, N. Plasmonic gas and chemical sensing. Nanophotonics 2014, 3, 157–180. [Google Scholar] [CrossRef] [Green Version]
- Degler, D.; Weimar, U.; Barsan, N. Current understanding of the fundamental mechanisms of doped and loaded semiconducting metal-oxide-based gas sensing materials. ACS Sens. 2019, 4, 2228–2249. [Google Scholar] [CrossRef] [PubMed]
- Seiyama, T.; Kato, A.; Fujiishi, K.; Nagatani, M. A new detector for gaseous components using semiconductive thin films. Anal. Chem. 1962, 34, 1502–1503. [Google Scholar] [CrossRef]
- Miller, D.R.; Akbar, S.A.; Morris, P.A. Nanoscale metal oxide-based heterojunctions for gas sensing: A review. Sens. Actuators B Chem. 2014, 204, 250–272. [Google Scholar] [CrossRef]
- Reyes, L.; Hoel, A.; Saukko, S.; Heszler, P.; Lantto, V.; Granqvist, C.G. Gas sensor response of pure and activated WO3 nanoparticle films made by advanced reactive gas deposition. Sens. Actuators B Chem. 2006, 117, 128–134. [Google Scholar] [CrossRef]
- Mirzaei, A.; Kim, J.-H.; Kim, H.W.; Kim, S.S. Gasochromic WO3 nanostructures for the detection of hydrogen gas: An overview. Appl. Sci. 2019, 9, 1775. [Google Scholar] [CrossRef] [Green Version]
- Sekimoto, S.; Nakagawa, H.; Okazaki, S.; Fukuda, K.; Asakura, S.; Shigemori, T.; Takahashi, S. A fiber-optic evanescent-wave hydrogen gas sensor using palladium-supported tungsten oxide. Sens. Actuators B Chem. 2000, 66, 142–145. [Google Scholar] [CrossRef]
- Benson, D.K.; Tracy, C.E.; Hishmeh, G.A.; Ciszek, P.E.; Lee, S.-H.; Haberman, D. Low-cost fiber optic hydrogen gas detector using guided-wave surface-plasmon resonance in chemochromic thin films. In Proceedings of the Photonics East Symposium, Boston, MA, USA, 1–5 November 1998; pp. 185–202. [Google Scholar]
- Watanabe, T.; Okazaki, S.; Nakagawa, H.; Murata, K.; Fukuda, K. A fiber-optic hydrogen gas sensor with low propagation loss. Sens. Actuators B Chem. 2010, 145, 781–787. [Google Scholar] [CrossRef]
- Matsuura, S.; Yamasaku, N.; Nishijima, Y.; Okazaki, S.; Arakawa, T. Characteristics of highly sensitive hydrogen sensor based on Pt-WO3/Si microring resonator. Sensors 2020, 20, 96. [Google Scholar] [CrossRef] [Green Version]
- Çoban, Ö.; Gür, E.; Tüzemen, S. Platinum activated WO3 optical hydrogen sensors. Mater. Today Proc. 2021, 46, 6913–6915. [Google Scholar] [CrossRef]
- Shen, Y.; She, X.; Jin, C. Mechanically reconfigurable Pd nanogroove array: An ultrasensitive optical hydrogen detector. ACS Photonics 2018, 5, 1334–1342. [Google Scholar] [CrossRef]
- She, X.; Shen, Y.; Wang, J.; Jin, C. Pd films on soft substrates: A visual, high-contrast and low-cost optical hydrogen sensor. Light Sci. Appl. 2019, 8, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Granqvist, C.G. Handbook of Inorganic Electrochromic Materials; Elsevier: Amsterdam, The Netherlands, 1995. [Google Scholar]
- Ndaya, C.C.; Javahiraly, N.; Brioude, A. Recent advances in palladium nanoparticles-based hydrogen sensors for leak detection. Sensors 2019, 19, 4478. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ghosh, R.; Baker, M.B.; Lopez, R. Optical properties and aging of gasochromic WO3. Thin Solid Films 2010, 518, 2247–2249. [Google Scholar] [CrossRef]
- Ito, K.; Ohgami, T. Hydrogen detection based on coloration of anodic tungsten oxide film. Appl. Phys. Lett. 1992, 60, 938–940. [Google Scholar] [CrossRef]
- Niklasson, G.A.; Granqvist, C.G. Electrochromics for smart windows: Thin films of tungsten oxide and nickel oxide, and devices based on these. J. Mater. Chem. 2007, 17, 127–156. [Google Scholar] [CrossRef] [Green Version]
- Yamaguchi, Y.; Imamura, S.; Ito, S.; Nishio, K.; Fujimoto, K. Influence of oxygen gas concentration on hydrogen sensing of Pt/WO3 thin film prepared by sol–gel process. Sens. Actuators B Chem. 2015, 216, 394–401. [Google Scholar] [CrossRef]
- Amrehn, S.; Wu, X.; Wagner, T. Tungsten oxide photonic crystals as optical transducer for gas sensing. ACS Sens. 2018, 3, 191–199. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Georg, A.; Graf, W.; Neumann, R.; Wittwer, V. Mechanism of the gasochromic coloration of porous WO3 films. Solid State Ion. 2000, 127, 319–328. [Google Scholar] [CrossRef]
- Valyukh, I.; Green, S.; Arwin, H.; Niklasson, G.A.; Wäckelgård, E.; Granqvist, C.-G. Spectroscopic ellipsometry characterization of electrochromic tungsten oxide and nickel oxide thin films made by sputter deposition. Sol. Energy Mater. Sol. Cells 2010, 94, 724–732. [Google Scholar] [CrossRef] [Green Version]
- Kulikova, D.P.; Dobronosova, A.A.; Kornienko, V.V.; Nechepurenko, I.A.; Baburin, A.S.; Sergeev, E.V.; Lotkov, E.S.; Rodionov, I.A.; Baryshev, A.V.; Dorofeenko, A.V. Optical properties of tungsten trioxide, palladium, and platinum thin films for functional nanostructures engineering. Opt. Express 2020, 28, 32049–32060. [Google Scholar] [CrossRef]
- Özkan, E.; Tepehan, F. Optical and structural characteristics of sol–gel-deposited tungsten oxide and vanadium-doped tungsten oxide films. Sol. Energy Mater. Sol. Cells 2001, 68, 265–277. [Google Scholar] [CrossRef]
- Saygin-Hinczewski, D.; Hinczewski, M.; Sorar, I.; Tepehan, F.Z.; Tepehan, G.G. Modeling the optical properties of WO3 and WO3–SiO2 thin films. Sol. Energy Mater. Sol. Cells 2008, 92, 821–829. [Google Scholar] [CrossRef]
- Von Rottkay, K.; Rubin, M.; Wen, S.-J. Optical indices of electrochromic tungsten oxide. Thin Solid Film. 1997, 306, 10–16. [Google Scholar] [CrossRef]
- Davazoglou, D.; Donnadieu, A. Optical oscillator strengths and quantum mechanics matrix elements of WO3 polycrystalline thin films. J. Non-Cryst. Solids 1994, 169, 64–71. [Google Scholar] [CrossRef]
- Charles, C.; Martin, N.; Devel, M.; Ollitrault, J.; Billard, A. Correlation between structural and optical properties of WO3 thin films sputter deposited by glancing angle deposition. Thin Solid Film. 2013, 534, 275–281. [Google Scholar] [CrossRef] [Green Version]
- Schweiger, D.; Georg, A.; Graf, W.; Wittwer, V. Examination of the kinetics and performance of a catalytically switching (gasochromic) device. Sol. Energy Mater. Sol. Cells 1998, 54, 99–108. [Google Scholar] [CrossRef]
- Vinogradov, A.; Dorofeenko, A.; Pukhov, A.; Lisyansky, A. Exciting surface plasmon polaritons in the Kretschmann configuration by a light beam. Phys. Rev. B 2018, 97, 235407. [Google Scholar] [CrossRef] [Green Version]
- Kornienko, V.V.; Nechepurenko, I.A.; Tananaev, P.N.; Chubchev, E.D.; Baburin, A.S.; Echeistov, V.V.; Zverev, A.V.; Novoselov, I.I.; Kruglov, I.A.; Rodionov, I.A. Machine learning for optical gas sensing: A leaky-mode humidity sensor as example. IEEE Sens. J. 2020, 20, 6954–6963. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nechepurenko, I.A.; Kulikova, D.P.; Kornienko, V.V.; Afanasiev, K.N.; Shekoyan, L.A.; Baryshev, A.V.; Dorofeenko, A.V. Evaluating the Response Time of an Optical Gas Sensor Based on Gasochromic Nanostructures. Sensors 2021, 21, 8472. https://doi.org/10.3390/s21248472
Nechepurenko IA, Kulikova DP, Kornienko VV, Afanasiev KN, Shekoyan LA, Baryshev AV, Dorofeenko AV. Evaluating the Response Time of an Optical Gas Sensor Based on Gasochromic Nanostructures. Sensors. 2021; 21(24):8472. https://doi.org/10.3390/s21248472
Chicago/Turabian StyleNechepurenko, Igor A., Daria P. Kulikova, Vladimir V. Kornienko, Konstantin N. Afanasiev, Landzhik A. Shekoyan, Alexander V. Baryshev, and Alexander V. Dorofeenko. 2021. "Evaluating the Response Time of an Optical Gas Sensor Based on Gasochromic Nanostructures" Sensors 21, no. 24: 8472. https://doi.org/10.3390/s21248472
APA StyleNechepurenko, I. A., Kulikova, D. P., Kornienko, V. V., Afanasiev, K. N., Shekoyan, L. A., Baryshev, A. V., & Dorofeenko, A. V. (2021). Evaluating the Response Time of an Optical Gas Sensor Based on Gasochromic Nanostructures. Sensors, 21(24), 8472. https://doi.org/10.3390/s21248472






