Overview of Fiber Optic Sensor Technologies for Strain/Temperature Sensing Applications in Composite Materials
Abstract
:1. Introduction
2. Composite Materials and Demand for the SHM in Composite Material Structures

3. Composite Materials with Embedded Fiber Sensors: Fabrication Methods
3.1. Fabrication Method of Composite Samples Embedded with FOS by Hand Layup and Pre-Preg Layup Methods
3.2. Rotating Filament Wound Pressure Cylinder with FOS

3.3. Composite Panels Embedded with FOS
3.4. Braided Composites Embedded with FOS
3.5. FOS Stitched Carbon Fibre Preforms for Advanced Composite Structures
4. Composite Material Degradation Associated with Embedding of Fiber Sensors
5. Types of Fiber Optic Sensors for Strain/Temperature Measurements in Composite Materials
5.1. Fiber Bragg Grating Sensor for Composite Materials

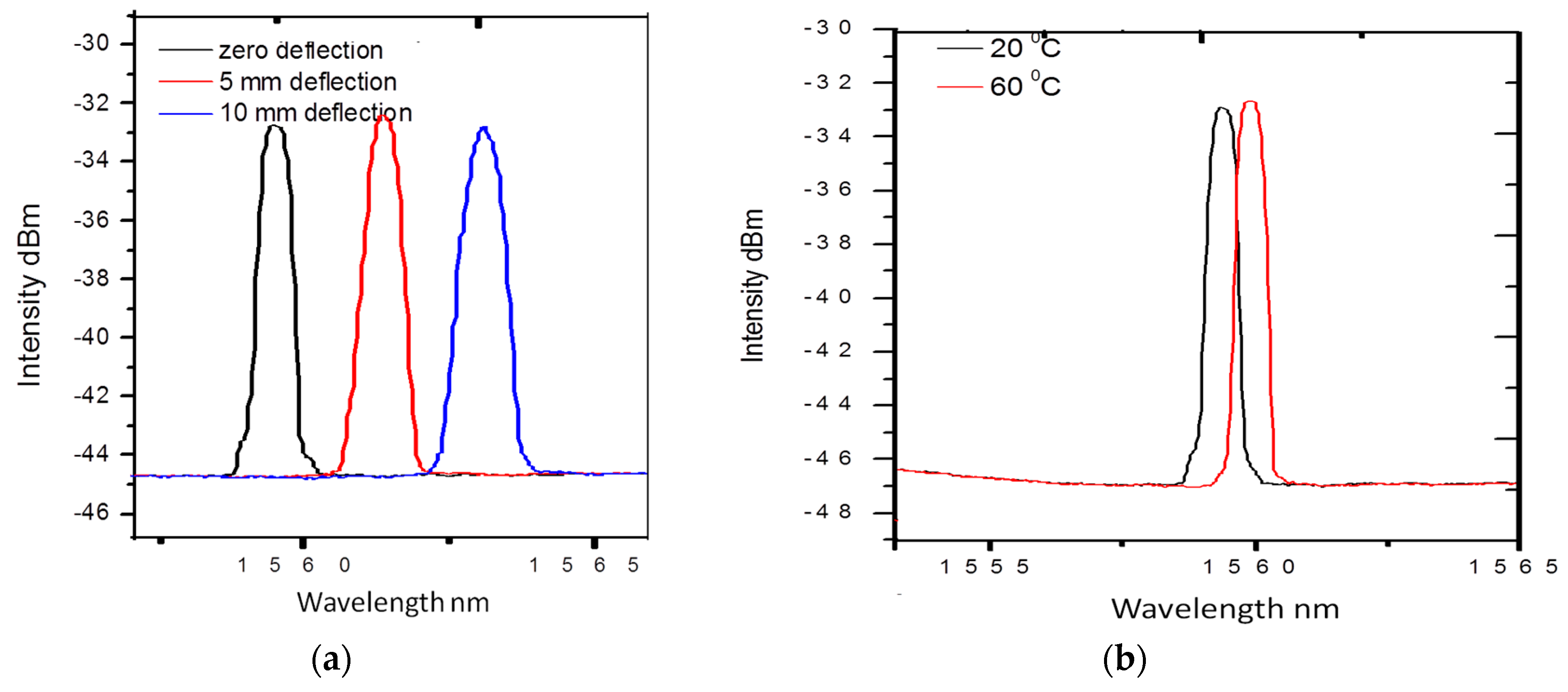
5.1.1. FBG Written in Micro-Structured Fibers as a Sensor for Composite Materials
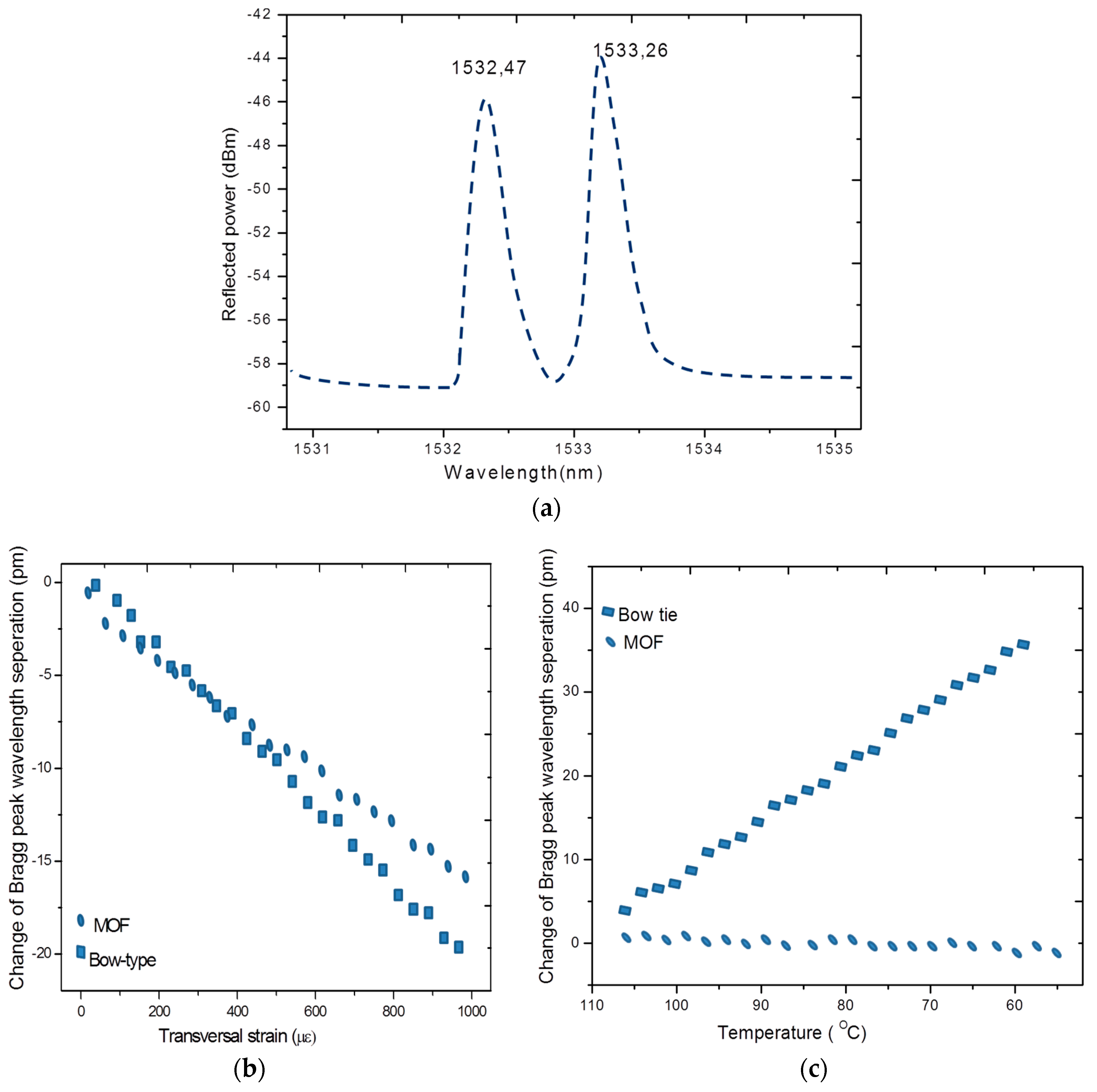
5.1.2. Phase-Shifted FBGs for Composite Materials

5.1.3. Polymer FBG Sensor for Composite Materials


5.2. Interferometric Fiber Sensors for Composite Materials




5.3. Optical Fiber Polarimetric Sensors for Composite Materials

5.4. Fiber Optic Micro Bend Sensors
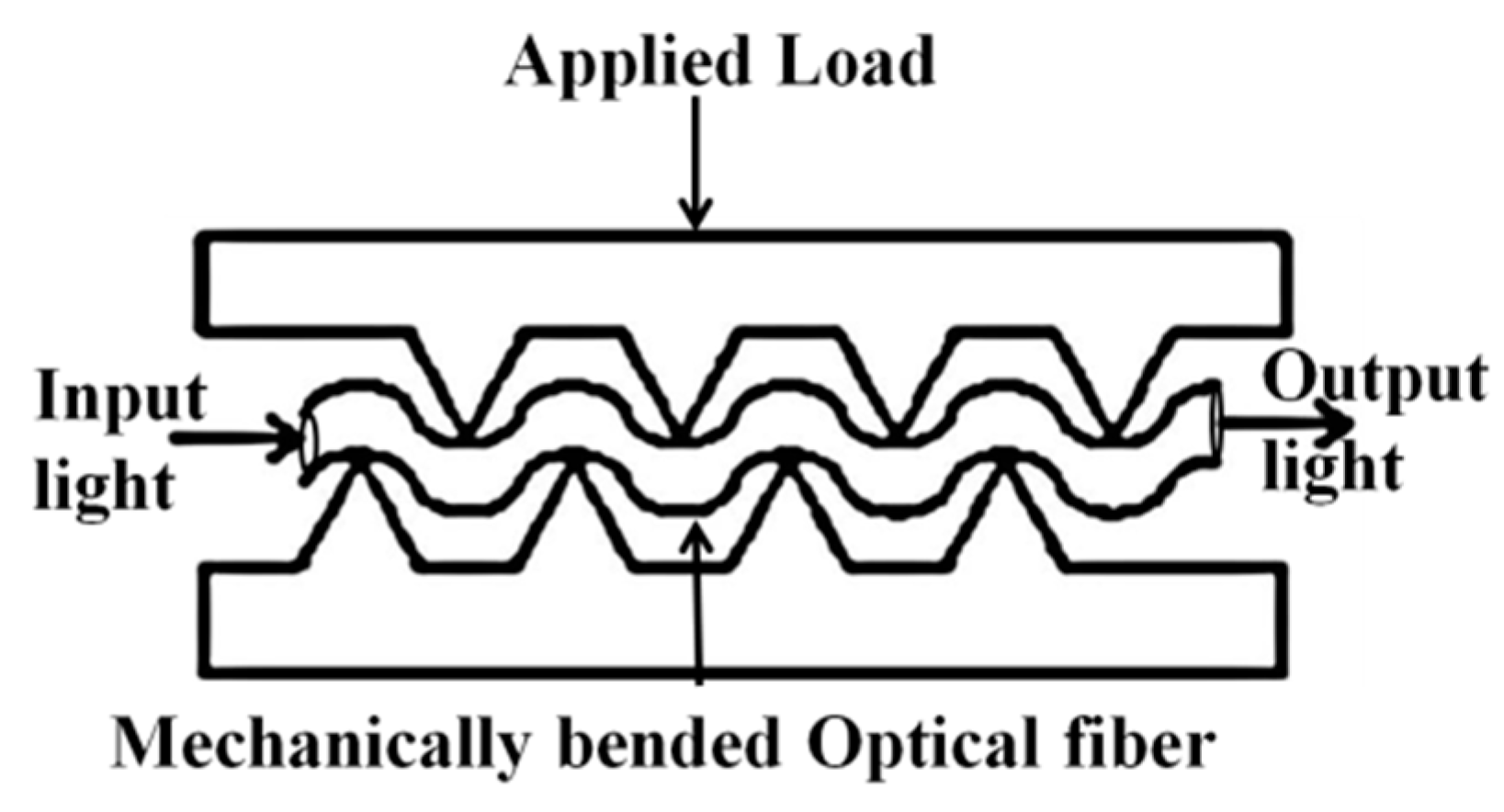
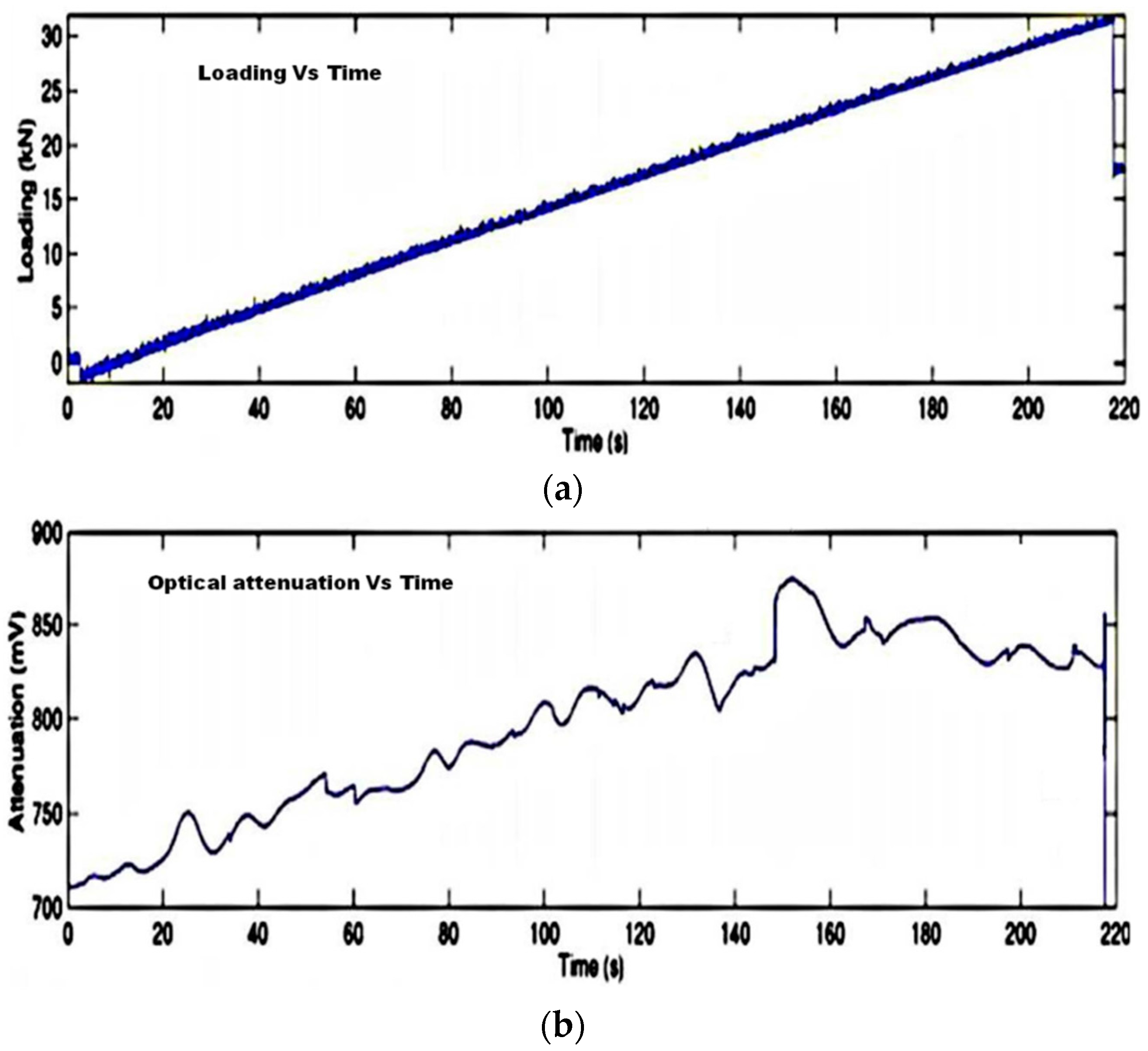
5.5. Distributed Fiber Optic Sensors
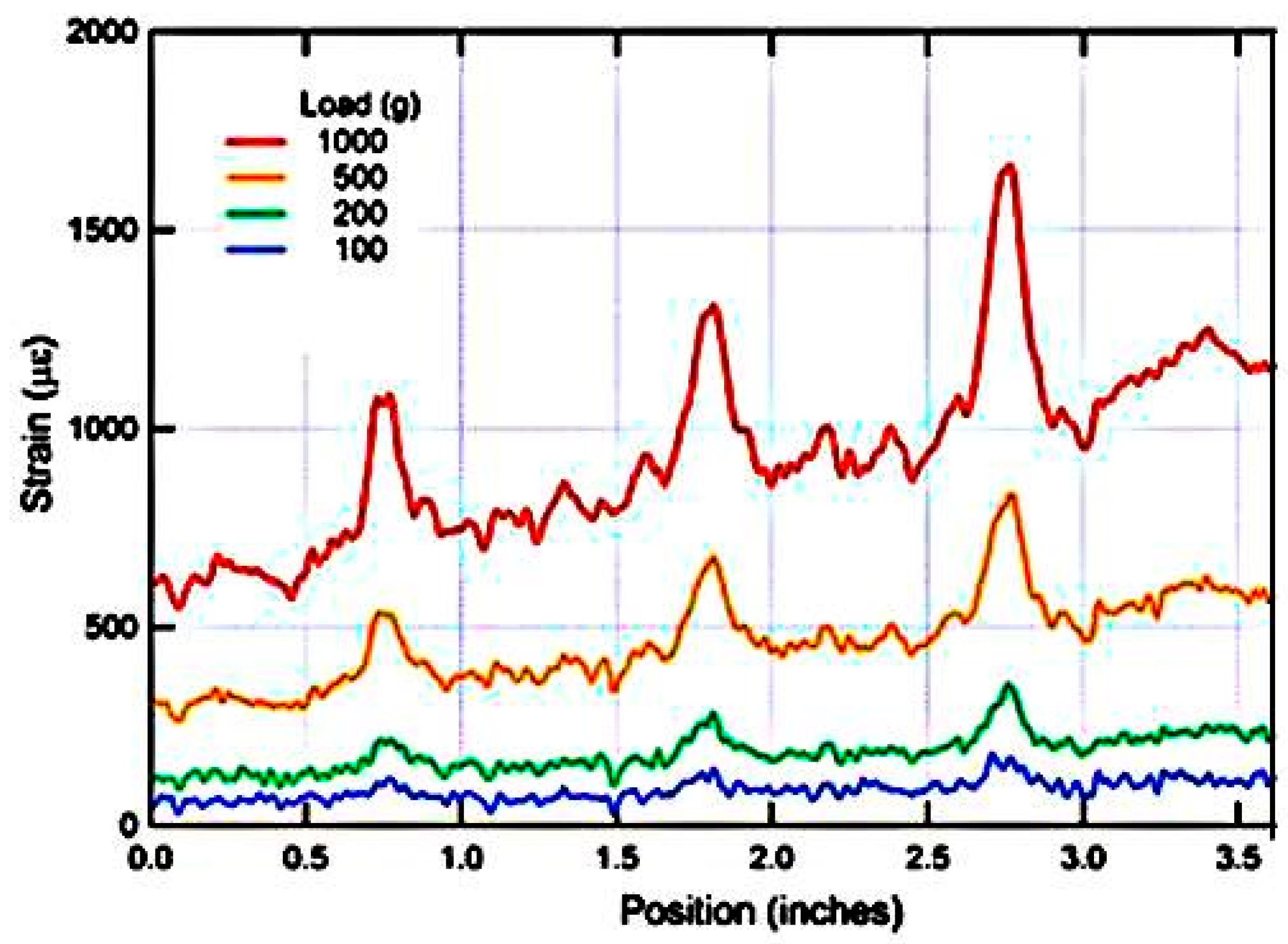
5.6. Hybrid Sensing Approaches for Simultaneous Strain and Temperature Measurements in Composite Materials
6. Recent Trends, Issues and Future Challenges of the FOS Technology
| FOS Technology | Advantages | Disadvantages | Remarks | Main Applications |
|---|---|---|---|---|
| Standard FBGs | Most accepted technology, allows for point measurements of strain and temperature | Temperature and strain cross sensitivity issues | Typical strain sensitivity ~1.2 pm/µε and typical temperature sensitivity ~11.6 pm/°C | Strain, temperature, vibration, cure process, localized damage, etc. |
| FBGs written in MOF | Can discriminate both axial and transverse strain components of composite material with insignificant temperature sensitivity | FBGs written in bow-tie fibers have temperature and strain cross sensitivity. But FBGs written in MOF have lower strain sensitivity compared to FBGs written in bow-tie fibers. | The cross-sensitivity issue can be resolved by using FBGs written in low temperature sensitive MOFs | Multi directional strain sensing, localized damage, etc. |
| Interferometric fiber optic sensors | Possesses higher temperature and strain sensitivities and are flexible in terms of size | Temperature and strain cross sensitivity issue, and brittle sensor | The cross-sensitivity issue can be resolved by using low temperature sensitive MOFs | Strain, temperature, vibration, cure process, localized damage, etc. |
| Polarimetric sensors | Sensitivity can be tuned by choosing different optical fiber types and sensor lengths | Difficult to measure strain/temperature at localized points, provide information averaged over the sensor’s length | The cross-sensitivity issue can be resolved by using low temperature sensitive HB-PM-PCF | Strain, temperature, vibration, cure process, etc. |
| Fiber optic micro bend sensors | Can measure continuous strain profile in a composite material using single optical fiber | Low accuracy | Output signal is strongly attenuated by any mechanical wave propagating in the composite material | Delamination and damage detection |
| Distributed sensors | Can measure continuous strain/temperature profile in a composite material using single optical fiber | For better resolution require the use of spectral demodulation techniques that are expensive and bulky | Appropriate sensing technology can be selected based on the application and its requirements | Strain, temperature, delamination, damage detection |
| Hybrid sensors | Two or more FOS operate in a combined manner to eliminate the disadvantages of individual FOSs providing accurate and independent strain/temperature information | Since two or more sensors are employed complicated interrogation methods are needed | Capable of discriminating between strain, temperature and thermal strain | Strain, thermal strain, temperature, vibration, cure process, damage point, etc. |


7. Conclusions
Acknowledgments
Conflicts of Interest
References
- Garg, D.P.; Zikry, M.A.; Anderson, G.L.; Gros, X.E. Current and potential future research activities in adaptive structures: An ARO perspective. Smart Mater. Struct. 2001, 10, 610–623. [Google Scholar] [CrossRef]
- Balageas, D. Introduction to structural health monitoring. In Structural Health Monitoring; ISTE: London, UK, 2006; pp. 16–43. [Google Scholar]
- Cai, J.; Qiu, L.; Yuan, S.; Shi, L.; Liu, P.; Liang, D. Structural Health Monitoring for Composite Materials. In Composites and Their Applications; InTech: Rijeka, Croatia, 2012. [Google Scholar]
- Zhu, Y.K.; Gui, Y.T.; Rong, S.L.; Hong, Z. A review of optical NDT technologies. Sensors 2011, 11, 7773–7798. [Google Scholar] [CrossRef] [PubMed]
- Gros, X.E. Current and Future Trends in Non-Destructive testing of Composite Materials. Ann. Chim. Sci. Mater. 2000, 25, 539–544. [Google Scholar] [CrossRef]
- Ye, X.W.; Su, Y.H.; Han, J.P. Structural health monitoring of civil infrastructure using optical fiber sensing technology: A comprehensive review. The Sci. World J. 2014, 2014. [Google Scholar] [CrossRef] [PubMed]
- Méndez, A.; Csipkes, A. Overview of fiber optic sensors for NDT applications. In Nondestructive Testing of Materials and Structures; Springer Netherlands: Dordrecht, The Netherlands, 2013; pp. 179–184. [Google Scholar]
- Chung, D.D.L. Composite Materials: Science and Applications, 2nd ed.; Springer-Verlag London: London, UK, 2010. [Google Scholar]
- Hull, D.; Clyne, T.W. An Introduction to Composite Materials; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Kaw, A.K. Mechanics of Composite Materials; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Bunsell, A.R.; Jacques, R. Fundamentals of Fibre Reinforced Composite Materials; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Fernando, G. Sensors and Composites: Requirements, Opportunities and Complexity. In Presented at the 1st International Workshop on Embedded Optical Sensors for Composite Materials, Ghent, Belgium, 7–8 October 2013.
- Kaufmann, M. Composite Materials: State-of-the-art and Future Challenges. In Presented at the 1st International Workshop on Embedded Optical Sensors for Composite Materials, Ghent, Belgium, 7–8 October 2013.
- Elmar, W.; Bernhard, J. Composites Market Report 2014. Available online: http://www.eucia.eu/userfiles/files/20141008_market_report_grpcrp.pdf (accessed on 13 January 2016).
- Ramakrishnan, M.; Rajan, G.; Semenova, Y.; Boczkowska, A.; Domański, A.; Wolinski, T.; Farrell, G. Measurement of thermal elongation induced strain of a composite material using a polarization maintaining photonic crystal fiber sensor. Sens. Actuators A Phys. 2013, 190, 44–51. [Google Scholar] [CrossRef]
- Ramakrishnan, M.; Rajan, G.; Semenova, Y.; Lesiak, P.; Domanski, A.; Wolinski, T.; Boczkowska, A.; Farrell, G. The influence of thermal expansion of a composite material on embedded polarimetric sensors. Smart Mater. Struct. 2011, 20. [Google Scholar] [CrossRef]
- Lesiak, P.; Szeląg, M.; Budaszewski, D.; Plaga, R.; Mileńko, K.; Rajan, G.; Semenova, Y.; Farrell, G.; Boczkowska, A.; Domański, A.; et al. Influence of lamination process on optical fiber sensors embedded in composite material. Measurement 2012, 45, 2275–2280. [Google Scholar] [CrossRef]
- Degrieck, J.; Wim, D.W.; Patricia, V. Monitoring of fibre reinforced composites with embedded optical fibre Bragg sensors, with application to filament wound pressure vessels. NDT&E Int. 2001, 34, 289–296. [Google Scholar]
- Hernández-Moreno, H.; Collombet, F.; Douchin, B.; Choqueuse, D.; Davies, P.; Velázquez, J.G. Entire life time monitoring of filament wound composite cylinders using Bragg grating sensors: I. Adapted tooling and instrumented specimen. Appl. Compos. Mater. 2009, 16, 173–182. [Google Scholar] [CrossRef]
- Keulen, C.; Rocha, B.; Yildiz, M.; Suleman, A. Embedded fiber optic sensors for monitoring processing, quality and structural health of resin transfer molded components. J. Phys. Conf. Ser. 2011, 305. [Google Scholar] [CrossRef]
- Bektaş, G.; Boz, T.; Keulen, C.J.; Yıldız, M.; Öztürk, C.; Menceloğlu, Y.Z.; Suleman, A. Fiber Bragg grating and etched optic sensors for flow and cure monitoring of resin transfer molded composite structures. In Proceedings of the 18th International Conference on Composite Materials (ICCM18), Jeju Island, Korea, 21–26 August 2011.
- Tuccillo, F.; Antonucci, V.; Calabro, A.M.; Giordano, M.; Nicolais, L. Practical and theoretic analysis of resin flow in vacuum assisted resin transfer molding processes. In Macromolecular Symposia; WILEY-VCH Verlag: Campania, Italy, 2005; Volume 228, pp. 201–218. [Google Scholar]
- Yuan, S.; Huang, R.; Rao, Y. Internal strain measurement in 3D braided composites using co-braided optical fiber sensors. J. Mater. Sci. Technol. 2004, 20, 199–202. [Google Scholar]
- Antonucci, V.; Esposito, M.; Ricciardi, M.R.; Raffone, M.; Zarrelli, M.; Giordano, M. Permeability characterization of stitched carbon fiber preforms by fiber optic sensors. Express Polym. Lett. 2011, 5, 1075–1084. [Google Scholar] [CrossRef]
- Jensen, D.W.; Pascual, J. Degradation of graphite/bismaleimide laminates with multiple embedded fiber-optic sensors. Proc. SPIE 1990, 1370. [Google Scholar] [CrossRef]
- Lee, D.C.; Lee, J.J.; Yun, S.J. The mechanical characteristics of smart composite structures with embedded optical fiber sensors. Compos. Struct. 1995, 32, 39–50. [Google Scholar] [CrossRef]
- Güemes, J.; Perez, J.S. Fiber Optics Sensors. In New Trends in Structural Health Monitoring; Springer: Vienna, Austria, 2013; pp. 265–316. [Google Scholar]
- Luycks, G.; Voet, E.; Lammens, N.; Degrieck, J. Strain Measurements of Composite Laminates with Embedded Fibre Bragg Gratings: Criticism and Opportunities for Research. Sensors 2011, 11, 384–408. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Błażejewski, W.; Gąsior, P.; Kaleta, J. Application of Optical Fiber Sensors to Measuring the Mechanical Properties of Composite Materials and Structures. In Advances in Composite Materials. Ecodesign and Analysis; InTech: Rijeka, Croatia, 2011. [Google Scholar]
- Emmanwori, L.; Shivakumar, K.N. Structural performance of composite laminates with embedded fiber optic sensor under tension and compression loads. In Proceedings of the 43rd Annual Conference of American Institute of Aeronautics and Astronautics, Denver, CO, USA, 22–25 April 2002.
- Ciang, C.C.; Jung, R.L.; Hyung, J.B. Structural health monitoring for a wind turbine system: A review of damage detection methods. Meas. Sci. Technol. 2008, 19. [Google Scholar] [CrossRef]
- Zhou, G.; Sim, L.M. Damage detection and assessment in fibre-reinforced composite structures with embedded fibre optic sensors-review. Smart Mater. Struct. 2002, 11. [Google Scholar] [CrossRef]
- Di Sante, R. Fibre Optic Sensors for Structural Health Monitoring of Aircraft Composite Structures: Recent Advances and Applications. Sensors 2015, 15, 18666–18713. [Google Scholar] [CrossRef] [PubMed]
- Minakuchi, S. From Material Characterization to Product Quality Control: Applicability of Fibre-Optic Sensors to Composites Process Monitoring. In Presented at the 1st International Workshop on Embedded Optical Sensors for Composite Materials, Ghent, Belgium, 7–8 October 2013.
- Du, W.; Tao, X.M.; Tam, H.Y.; Choy, C.L. Fundamentals and applications of optical fiber Bragg grating sensors to textile structural composites. Compos. Struct. 1998, 42, 217–229. [Google Scholar] [CrossRef]
- Di Sante, R.; Donati, L.; Troiani, E.; Proli, P. Reliability and accuracy of embedded fiber Bragg grating sensors for strain monitoring in advanced composite structures. Met. Mater. Int. 2014, 20, 537–543. [Google Scholar] [CrossRef]
- Lau, K.T. Structural health monitoring for smart composites using embedded FBG sensor technology. Mater. Sci. Technol. 2014, 30, 1642–1654. [Google Scholar] [CrossRef]
- Rao, Y.J. Recent progress in fiber-optic extrinsic Fabry-Perot interferometric sensors. Opt. Fiber Technol. 2006, 12, 227–237. [Google Scholar] [CrossRef]
- Murukeshan, V.M.; Chan, P.Y.; Ong, L.S.; Asundi., A. On-line health monitoring of smart composite structures using fiber polarimetric sensor. Smart Mater. Struct. 1999, 8. [Google Scholar] [CrossRef]
- Rippert, L.; Jean-Michel, P.; Martine, W.; Sabine, V.H. Fibre optic sensor for continuous health monitoring in CFRP composite materials. Proc. SPIE 2002, 4693. [Google Scholar] [CrossRef]
- Murayama, H.; Kazuro, K.; Hiroshi, N.; Akiyoshi, S.; Kiyoshi, U. Application of fiber-optic distributed sensors to health monitoring for full-scale composite structures. J. Intell. Mater. Syst. Struct. 2003, 14, 3–13. [Google Scholar] [CrossRef]
- López-Higuera, J.M.; Rodriguez Cobo, L.; Quintela Incera, A.; Cobo, A. Fiber optic sensors in structural health monitoring. J. Lightwave Technol. 2011, 29, 587–608. [Google Scholar] [CrossRef]
- Gerard, F.F. Fibre optic sensor systems for monitoring composite structures. In Porceedings of the RP Asia 2005 Conference, Bangkok, Thailand, 25–26 August 2005.
- Montanini, R.; d’Acquisto, L. Simultaneous measurement of temperature and strain in glass fiber/epoxy composites by embedded fiber optic sensors: I. Cure monitoring. Smart Mater. Struct. 2007, 16. [Google Scholar] [CrossRef]
- Glisic, B.; Inaudi, D. Fibre Optic Methods for Structural Health Monitoring, 1st ed.; John Wiley & Sons, Ltd.: Southern Gate, Chichester, UK, 2007. [Google Scholar]
- Hill, K.O.; Meltz, G. Fiber Bragg grating technology fundamentals and overview. J. Lightwave Technol. 1997, 15, 1263–1276. [Google Scholar] [CrossRef]
- Raman, K. Fiber Bragg Gratings; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Yashiro, S.; Okabe, T.; Toyama, N.; Takeda, N. Monitoring damage in holed laminates using embedded chirped FBG sensors. Int. J. Solids Struct. 2007, 44, 603–613. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, H.; Liu, B.; Miao, Y. Tilted fiber Bragg gratings: Principle and sensing applications. Photonic Sens. 2011, 1, 6–30. [Google Scholar] [CrossRef]
- Zhao, Y.; Yanbiao, L. Discrimination methods and demodulation techniques for fiber Bragg grating sensors. Opt. Lasers Eng. 2004, 41, 1–18. [Google Scholar] [CrossRef]
- Xu, M.G.; Archambault, J.L.; Reekie, L.; Dakin, J.P. Discrimination between strain and temperature effects using dual-wavelength fibre grating sensors. Electron. Lett. 1994, 30, 1085–1087. [Google Scholar] [CrossRef]
- James, S.W.; Dockney, M.L.; Tatam, R.P. Simultaneous independent temperature and strain measurement using in-fibre Bragg grating sensors. Electron. Lett. 1996, 32, 1133–1134. [Google Scholar] [CrossRef]
- Samer, K.B.; Sun, T.; Grattan, K.T.V. Simultaneous measurement of temperature and strain with long period grating pairs using low resolution detection. Sens. Actuators 2008, 144, 83–89. [Google Scholar]
- Oliveira, R.D.; Ramos, C.A.; Marques, A.T. Health monitoring of composite structures by embedded FBG and interferometric Fabry-Perot sensors. Comput. Struct. 2008, 86, 340–346. [Google Scholar] [CrossRef]
- Guan, B.O.; Tam, H.Y.; Tao, X.M.; Dong, X.Y. Simultaneous strain and temperature measurement using a superstructure fiber Bragg grating. IEEE Photonics Technol. Lett. 2000, 12, 675–677. [Google Scholar] [CrossRef]
- Rajan, G.; Ramakrishnan, M.; Lesiak, P.; Semenova, Y.; Wolinski, T.; Boczkowska, A.; Farrell, G. Composite materials with embedded photonic crystal fiber interferometric sensors. Sens. Actuators A Phys. 2012, 182, 57–67. [Google Scholar] [CrossRef]
- Kreuzer, M. Strain Measurement with Fiber Bragg Grating Sensors; HBM: Darmstadt, Germany, 2006. [Google Scholar]
- Sonnenfeld, C.; Sulejmani, S.; Geernaert, T.; Eve, S.; Lammens, N.; Luyckx, G.; Eli, V.; Thienpont, H. Microstructured optical fiber sensors embedded in a laminate composite for smart material applications. Sensors 2011, 11, 2566–2579. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guemes, J.A.; Menendez, J.M. Response of Bragg grating fiber-optic sensors when embedded in composite laminates. Compos. Sci. Technol. 2002, 62, 959–966. [Google Scholar] [CrossRef]
- Luyckx, G.; Voet, E.; Geernaert, T.; Chah, K.; Nasilowski, T.; De Waele, W.; van Paepegem, W.; Becker, M.; Bartelt, H.; Urbanczyk, W.; et al. Response of FBGs in microstructured and bow tie fibers embedded in laminated composite. IEEE Photonics Technol. Lett. 2009, 21, 1290–1292. [Google Scholar] [CrossRef]
- Azmi, A.I.; Deep, S.; Wenjuan, S.; John, C.; Gang, D.P. Performance enhancement of vibration sensing employing multiple phase-shifted fiber Bragg grating. J. Lightwave Technol. 2011, 29, 3453–3460. [Google Scholar] [CrossRef]
- Webb, D.J. Polymer optical fibre Bragg gratings. OSA Tech. Dig. 2012. [Google Scholar] [CrossRef]
- Kalli, K.; Dobb, H.L.; Webb, D.J.; Carroll, K.; Themistos, C.; Komodromos, M.; Peng, G.-D.; Fang, Q.; Boyd, I.W. Development of an electrically tuneable Bragg grating filter in polymer optical fibre operating at 1.55 µm. Meas. Sci. Technol. 2007, 17, 3155–3164. [Google Scholar] [CrossRef]
- Zhang, W.; Webb, D.J.; Peng, G.-D. Investigation in to time response of polymer fibre Bragg grating based humidity sensors. J. Lightwave Technol. 2012, 30, 1090–1096. [Google Scholar] [CrossRef]
- Rajan, G.; Ramakrishnan, M.; Semeonva, Y.; Ambikairajah, E.; Farrell, G.; Peng, G.D. Experimental study and analysis of a polymer fibre Bragg grating embedded in a composite material. J. Lightwave Technol. 2014, 32, 1726–1733. [Google Scholar] [CrossRef]
- Lee, B.H.; Kim, Y.H.; Park, K.S.; Eom, J.B.; Kim, M.J.; Rho, B.S.; Choi, H.Y. Interferometric fiber optic sensors. Sensors 2012, 12, 2467–2486. [Google Scholar] [CrossRef] [PubMed]
- Udd, E.; Spillman, W.B. Fiber Optic Sensors: An Introduction for Engineers and Scientists, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Liu, T.; Wu, M.; Rao, Y.; Jackson, D.A.; Fernando, G.F. A multiplexed optical fibre-based extrinsic Fabry-Perot sensor system for in-situ strain monitoring in composites. Smart Mater. Struct. 1998, 7. [Google Scholar] [CrossRef]
- Leng, J.S.; Asundi, A. Structural health monitoring of smart composite materials by using EFPI and FBG sensors. Sens. Actuators A Phys. 2003, 103, 330–340. [Google Scholar] [CrossRef]
- Fernando, G.F.; Liu, T.; Crosby, P.; Doyle, C.A.; Martin, D.B.; Ralph, B.; Badcock, R. A multi-purpose optical fibre sensor design for fibre reinforced composite materials. Meas. Sci. Technol. 1997, 8. [Google Scholar] [CrossRef]
- Woliński, T.R. I Polarimetric optical fibers and sensors. Prog. Opt. 2000, 40, 1–75. [Google Scholar]
- Domanski, A.W.; Tomasz, R.W.; Wojtek, J.B. Polarimetric fiber optic sensors: State of the art and future. Proc. SPIE 1994, 2341. [Google Scholar] [CrossRef]
- Woliński, T.R.; Lesiak, P.; Domański, A.W. Polarimetric optical fiber sensors of a new generation for industrial applications. Tech. Sci. 2008, 56, 125–132. [Google Scholar]
- Ma, J.; Asundi, A. Structural health monitoring using a fiber optic polarimetric sensor and a fiber optic curvature sensor-static and dynamic test. Smart Mater. Struct. 2001, 10, 181–188. [Google Scholar] [CrossRef]
- Hogg, W.D.; Roderick, D.T. Polarimetric fibre optic structural strain sensor characterisation. Proc. SPIE 1990, 1170, 542–550. [Google Scholar]
- Domański, A.W.; Lesiak, P.; Karolina, M.; Boczkowska, A.; Budaszewski, D.; Ertman, S.; Woliński, T.R. Temperature-insensitive fiber optic deformation sensor embedded in composite material. Photonics Lett. Pol. 2009, 1. [Google Scholar] [CrossRef]
- Kim, D.H.; Kang, J.U. Analysis of temperature-dependent birefringence of a polarization-maintaining photonic crystal fiber. Opt. Eng. 2007, 46. [Google Scholar] [CrossRef]
- Murukeshan, V.M.; Chan, P.Y.; Ong, L.S.; Asundi, A. Effects of different parameters on the performance of a fiber polarimetric sensor for smart structure applications. Sens. Actuators A Phys. 2000, 80, 249–255. [Google Scholar] [CrossRef]
- Berthold, J.W. Historical review of microbend fiber-optic sensors. J. Lightwave Technol. 1995, 13, 1193–1199. [Google Scholar] [CrossRef]
- Pandey, N.K.; Yadav, B.C. Embedded fibre optic microbend sensor for measurement of high pressure and crack detection. Sens. Actuators A Phys. 2006, 128, 33–36. [Google Scholar] [CrossRef]
- Wevers, M.; Rippert, L.; Papy, J.M.; van Huffel, S. Processing of transient signals from damage in composite materials monitored with embedded intensity-modulated fiber optic sensors. NDT&E Int. 2006, 39, 229–235. [Google Scholar]
- Adachi, S. Distributed optical fiber sensors and their applications. In Proceedings of the SICE Annual Conference, Tokyo, Japan, 20–22 August 2008.
- Niklès, M. Fibre optic distributed scattering sensing system: Perspectives and challenges for high performance applications. Proc. SPIE 2007, 6619. [Google Scholar] [CrossRef]
- Grattan, K.T.V.; Sun, T. Fiber optic sensor technology: An overview. Sens. Actuators A Phys. 2000, 82, 40–61. [Google Scholar] [CrossRef]
- Kersey, A.D. A review of recent developments in fiber optic sensor technology. Opt. Fiber Technol. 1996, 2, 291–317. [Google Scholar] [CrossRef]
- Bao, X.; Liang, C. Recent progress in Brillouin scattering based fiber sensors. Sensors 2011, 11, 4152–4187. [Google Scholar] [CrossRef] [PubMed]
- Hartog, A.H. Progress in Distributed Fiber Optic Temperature Sensing. Environmental and Industrial Sensing. Proc. SPIE 2002, 4578. [Google Scholar] [CrossRef]
- Culshaw, B. Optical fiber sensor technologies: Opportunities and-perhaps-pitfalls. J. Lightwave Technol. 2004, 22. [Google Scholar] [CrossRef]
- Ravet, F. Distributed Brillouin Sensor Application to Structural Failure Detection. In New Developments in Sensing Technology for Structural Health Monitoring; Springer Berlin Heidelberg: Berlin, Germany, 2011; pp. 93–136. [Google Scholar]
- Bernini, R.; Minardo, A.; Zeni, L. Distributed Sensing at centimetre-scale spatial resolution by BOFDA: Measurements and signal processing. IEEE Photonics J. 2012, 4, 48–56. [Google Scholar] [CrossRef]
- Zeng, X.; Bao, X.; Chia, Y.C.; Theodore, W.B.; Anthony, W.B.; Michael, D.D.; Graham, F.; Alexander, L.K.; Anastasis, V.G. Strain measurement in a concrete beam by use of the Brillouin-scattering-based distributed fiber sensor with single-mode fibers embedded in glass fiber reinforced polymer rods and bonded to steel reinforcing bars. Appl. Opt. 2002, 41, 5105–5114. [Google Scholar] [CrossRef] [PubMed]
- Patrick, H.J.; Williams, G.M.; Kersey, A.D.; Pedrazzani, J.R. Hybrid fibre Bragg grating/long period grating sensor for strain/temperature discrimination. IEEE Photonics Technol. Lett. 1996, 8, 1223–1225. [Google Scholar] [CrossRef]
- Dong, B.; Hao, J.; Liaw, C.; Lin, B.; Tjin, S.C. Simultaneous strain and temperature measurement using a compact photonic crystal fiber inter-modal interferometer and a fiber Bragg grating. Appl. Opt. 2010, 49, 6232–6235. [Google Scholar] [CrossRef] [PubMed]
- Andre, R.M.; Marques, M.B.; Roy, P.; Frazao, O. Fiber Loop Mirror Using a Small Core Micro-structured Fiber for Strain and Temperature Discrimination. IEEE Photonics Technol. Lett. 2010, 22, 1120–1122. [Google Scholar] [CrossRef]
- Frazão, O.; Carvalho, J.P.; Ferreira, L.A.; Araújo, F.M.; Santos, J.L. Discrimination of strain and temperature using Bragg gratings in microstructured and standard optical fibres. Meas. Sci. Technol. 2005, 16. [Google Scholar] [CrossRef]
- Rajan, G.; Ramakrishnan, M.; Semenova, Y.; Karolina, M.; Lesiak, P.; Domanski, A.W.; Wolinski, T.R.; Farrell, G. A Photonic Crystal Fiber and Fiber Bragg Grating-Based Hybrid Fiber-Optic Sensor System. Sens. J. 2012, 12, 39–43. [Google Scholar] [CrossRef]
- Kang, H.K.; Park, J.W.; Ryu, C.Y.; Hong, C.S.; Kim, C.G. Development of fibre optic ingress/egress methods for smart composite structures. Smart Mater. Struct. 2000, 9. [Google Scholar] [CrossRef]
- Green, A.K.; Zaidman, M.; Shafir, E.; Tur, M.; Gali, S. Infrastructure development for incorporating fibre-optic sensors in composite materials. Smart Mater. Struct. 2000, 9. [Google Scholar] [CrossRef]
- Simon, W.R.; William, R.P. Apparatus for Ingress and Egress of Fiber Optic Sensor Leads from the Surface of Composite Parts and a Method for the Manufacture Thereof. U.S. Patent 6,173,090, 9 January 2001. [Google Scholar]
- Kinet, D.; Mégret, P.; Goossen, K.W.; Qiu, L.; Heider, D.; Caucheteur, C. Fiber Bragg Grating Sensors toward Structural Health Monitoring in Composite Materials: Challenges and Solutions. Sensors 2014, 14, 7394–7419. [Google Scholar] [CrossRef] [PubMed]
- Qiu, L.; Goossen, K.W.; Heider, D.; Wetzel, E.D. Nonpigtail optical coupling to embedded fiber Bragg grating sensors. Opt. Eng. 2010, 49. [Google Scholar] [CrossRef]
- Minakuchi, S.; Umehara, T.; Takagaki, K.; Ito, Y.; Takeda, N. Life cycle monitoring and advanced quality assurance of L-shaped composite corner part using embedded fiber-optic sensor. Compos. Part A Appl. Sci. Manuf. 2013, 48, 153–161. [Google Scholar] [CrossRef]
- Chojetzki, C.; Rothhardt, M.; Ommer, J.; Unger, S.; Schuster, K.; Mueller, H.R. High-reflectivity draw-tower fiber Bragg gratings—Arrays and single gratings of type II. Opt. Eng. 2005, 44. [Google Scholar] [CrossRef]
- Palmer, D.D.; Engelbart, R.W.; Vaccaro, C.M. Future Directions Relative to NDE of Composite Structures. SAE Tech. Pap. 2004. [Google Scholar] [CrossRef]
- Van, H.; Bram, G.L.; Erwin, B.; Jeroen, M.; Sandeep, K.; Oliver, M.; David, J.W.; Kate, S.; Peter, V.D.; Geert, V.S. Ultra small integrated optical fiber sensing system. Sensors 2012, 12, 12052–12069. [Google Scholar] [CrossRef] [Green Version]
- Missinne, J.; Geert, V.S.; Bram, V.H.; Kristof, V.C.; Tim, V.G.; Peter, D.; Jan, V.; Peter, V.D. An array waveguide sensor for artificial optical skins. Proc. SPIE 2009, 7221. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramakrishnan, M.; Rajan, G.; Semenova, Y.; Farrell, G. Overview of Fiber Optic Sensor Technologies for Strain/Temperature Sensing Applications in Composite Materials. Sensors 2016, 16, 99. https://doi.org/10.3390/s16010099
Ramakrishnan M, Rajan G, Semenova Y, Farrell G. Overview of Fiber Optic Sensor Technologies for Strain/Temperature Sensing Applications in Composite Materials. Sensors. 2016; 16(1):99. https://doi.org/10.3390/s16010099
Chicago/Turabian StyleRamakrishnan, Manjusha, Ginu Rajan, Yuliya Semenova, and Gerald Farrell. 2016. "Overview of Fiber Optic Sensor Technologies for Strain/Temperature Sensing Applications in Composite Materials" Sensors 16, no. 1: 99. https://doi.org/10.3390/s16010099
APA StyleRamakrishnan, M., Rajan, G., Semenova, Y., & Farrell, G. (2016). Overview of Fiber Optic Sensor Technologies for Strain/Temperature Sensing Applications in Composite Materials. Sensors, 16(1), 99. https://doi.org/10.3390/s16010099








