Planar Microstrip Ring Resonators for Microwave-Based Gas Sensing: Design Aspects and Initial Transducers for Humidity and Ammonia Sensing
Abstract
1. Introduction
2. Principle of Planar Microwave Transducers
3. Sensor Design
3.1. Microstrip Ring Resonator Design
3.2. Simulation Studies
3.3. Influence of the Coupling Gap
3.4. Miniaturization
3.5. Final Microwave Gas-Sensing Device
4. Experiment and Methods
5. Results and Discussion
5.1. Initial Tests: Proof of Concept
- Sensor outside the glass (i.e., in air) without zeolite layer.
- Sensor inside the glass (ammonia-in-air ambience) without zeolite layer.
- Sensor outside the glass (i.e., in air) with zeolite layer.
- Sensor inside the glass (ammonia-in-air ambience) with zeolite layer.
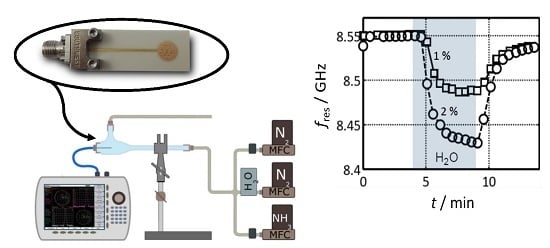
5.2. Tests under Defined Gas Exposure
6. Conclusions and Outlook
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A


References
- Comini, E.; Faglia, G.; Sberveglieri, G. Solid State Gas Sensing; Springer International Publishing AG: Heidelberg, Germany, 2009. [Google Scholar]
- Bănică, F.-G. Chemical Sensors and Biosensors: Fundamentals and Applications; John Wiley & Sons, Ltd.: Chichester, UK, 2012; pp. 246–257. [Google Scholar] [CrossRef]
- Yamazoe, N.; Shimanoe, K. Fundamentals of Semiconductor Gas Sensors. In Semiconductor Gas Sensors; Jaaniso, R., Tan, O.K., Eds.; Woodhead Publishing Ltd.: Cambridge, UK, 2013; pp. 3–34. [Google Scholar]
- Barsan, N.; Koziej, D.; Weimar, U. Metal Oxide-Based Gas Sensor Research: How to? Sens. Actuators B Chem. 2007, 121, 18–35. [Google Scholar] [CrossRef]
- Zarifi, M.H.; Shariaty, P.; Hashisho, Z.; Daneshmand, M. A Non-Contact Microwave Sensor for Monitoring the Interaction of Zeolite 13X with CO2 and CH4 in Gaseous Streams. Sens. Actuators B Chem. 2017, 238, 1240–1247. [Google Scholar] [CrossRef]
- Zarifi, M.H.; Sohrabi, A.; Shaibani, P.M.; Daneshmand, M.; Thundat, T. Detection of Volatile Organic Compounds Using Microwave Sensors. IEEE Sens. J. 2015, 15, 248–254. [Google Scholar] [CrossRef]
- Rossignol, J.; Barochi, G.; de Fonseca, B.; Brunet, J.; Bouvet, M.; Pauly, A.; Markey, L. Microwave-based gas sensor with phthalocyanine film at room temperature. Sens. Actuators B Chem. 2013, 189, 213–216. [Google Scholar] [CrossRef]
- Bailly, G.; Harrabi, A.; Rossignol, J.; Stuerga, D.; Pribetich, P. Microwave Gas Sensing with a Microstrip Interdigital Capacitor: Detection of NH3 with TiO2 nanoparticles. Sens. Actuators B Chem. 2016, 236, 554–564. [Google Scholar] [CrossRef]
- Bailly, G.; Rossignol, J.; de Fonseca, B.; Pribetich, P.; Stuerga, D. Microwave Gas Sensing with Hematite: Shape Effect on Ammonia Detection Using Pseudocubic, Rhombohedral, and Spindlelike Particles. ACS Sens. 2016, 1, 656–662. [Google Scholar] [CrossRef]
- Chen, L.F.; Ong, C.K.; Neo, C.P.; Varadan, V.V.; Varadan, V.K. Microwave Electronics: Measurement and Materials Characterization; John Wiley & Sons, Ltd.: Chichester, UK, 2004. [Google Scholar]
- Yeow, Y.; Abbas, Z.; Khalid, K. Application of Microwave Moisture Sensor for Determination of Oil Palm Fruit Ripeness. Meas. Sci. Rev. 2010, 10, 714. [Google Scholar] [CrossRef]
- Yogi, R.A.; Parolia, R.S.; Karekar, R.N.; Aiyer, R.C. Microwave Microstrip Ring Resonator as a Paper Moisture Sensor: Study with Different Grammage. Meas. Sci. Technol. 2002, 13, 1558–1562. [Google Scholar] [CrossRef]
- Sarabandi, K.; Li, E.S. Microstrip Ring Resonator for Soil Moisture Measurements. IEEE Trans. Geosci. Remote Sens. 1997, 35, 1223–1231. [Google Scholar] [CrossRef]
- Abegaonkar, M.P.; Karekar, R.N.; Aiyer, R.C. A Microwave Microstrip Ring Resonator as a Moisture Sensor for Biomaterials: Application to Wheat Grains. Meas. Sci. Technol. 1999, 10, 195–200. [Google Scholar] [CrossRef]
- Schwerthoeffer, U.; Weigel, R.; Kissinger, D. A Highly Sensitive Glucose Biosensor Based on a Microstrip Ring Resonator. In Proceedings of the IEEE MTT-S International Microwave Workshop Series on RF and Wireless Technologies for Biomedical and Healthcare Applications (IMWS-BIO), Singapore, 9–11 December 2013; pp. 1–3. [Google Scholar] [CrossRef]
- Zarifi, M.H.; Thundat, T.; Daneshmand, M. High resolution microwave microstrip resonator for sensing applications. Sens. Actuators A Phys. 2015, 233, 224–230. [Google Scholar] [CrossRef]
- Yi, F.-Y.; Chen, D.; Wu, M.-K.; Han, L.; Jiang, H.-L. Chemical Sensors Based on Metal–Organic Frameworks. ChemPlusChem 2016, 81, 675–690. [Google Scholar] [CrossRef]
- Marr, I.; Reiß, S.; Hagen, G.; Moos, R. Planar Zeolite film-Based Potentiometric Gas Sensors Manufactured by a Combined Thick-film and Electroplating Technique. Sensors 2011, 11, 7736–7748. [Google Scholar] [CrossRef] [PubMed]
- Chen, P.; Schönebaum, S.; Simons, T.; Rauch, D.; Dietrich, M.; Moos, R.; Simon, U. Correlating the Integral Sensing Properties of Zeolites with Molecular Processes by Combining Broadband Impedance and DRIFT Spectroscopy—A New Approach for Bridging the Scales. Sensors 2015, 15, 28915–28941. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Wang, J.; Long, Y. Zeolite-based Materials for Gas Sensors. Sensors 2006, 6, 1751–1764. [Google Scholar] [CrossRef]
- Bahoumina, P.; Hallil, H.; Lachaud, J.L.; Rebiere, D.; Dejous, C.; Abdelghani, A.; Frigui, K.; Bila, S.; Baillargeat, D.; Zhang, Q.; et al. Chemical Gas Sensor Based on a Novel Capacitive Microwave Flexible Transducer and Composite Polymer Carbon Nanomaterials. In Proceedings of the Symposium on Design, Test, Integration and Packaging of MEMS/MOEMS (DTIP), Cannes, France, 1–4 April 2017. [Google Scholar] [CrossRef]
- Zarifi, M.H.; Gholidoustb, A.; Abdolrazzaghic, M.; Shariatyb, P.; Hashisho, Z.; Daneshmand, M. Sensitivity Enhancement in Planar Microwave Active-Resonator Using Metal Organic Framework for CO2 Detection. Sens. Actuators B Chem. 2017, in press. [Google Scholar] [CrossRef]
- Bailly, G.; Harrabi, A.; Rossignol, J.; Michel, M.; Stuerga, D.; Pribetich, P. Microstrip Spiral Resonator For Microwave-Based Gas Sensing. IEEE Sens. Lett. 2017, 1. [Google Scholar] [CrossRef]
- Piekarz, I.; Socki, J.; Wincza, K.; Gruszczynski, S. Microwave Sensors for Dielectric Sample Measurement Based on Coupled-Line Section. IEEE Trans. Microw. Theory Tech. 2017, 65, 1615–1631. [Google Scholar] [CrossRef]
- Yakhlef, Y.; Benhabiles, M.T.; Benkhaoua, L.; Riabi, M.L. Compact Miniature Sensors Based on Tapered Lines Coupled Metamaterial Resonators. In Proceedings of the First IEEE MTT-S International Microwave Bio Conference (IMBIOC), Gothenburg, Sweden, 15–17 May 2017; pp. 1–4. [Google Scholar]
- Denoual, M.; Pouliquen, M.; Jorel, C.; Radu, C.; Robbes, D.; Harnois, M.; de Sagazan, O.; Grand, J.; Awala, H.; Mintova, S.; et al. Zeolite-Based Thermal Mass Gas Sensor with Self-Identification Algorithm. In Proceedings of the Symposium on Design, Test, Integration and Packaging of MEMS/MOEMS (DTIP), Gothenburg, Sweden, 19–21 September 2017; pp. 1–4. [Google Scholar]
- Reinecke, T.; Walter, J.-G.; Kobelt, T.; Ahrens, A.; Scheper, T.; Zimmermann, S. Biosensor Based on a Split-Ring Resonator. In Proceedings of the SENSOR 2017, Nuremberg, Germany, 30 May–1 June 2017; pp. 78–83. [Google Scholar] [CrossRef]
- Rydosz, A.; Maciak, E.; Wincza, K.; Gruszczynski, S. Microwave-Based Sensors with Phthalocyanine Films for Acetone, Ethanol and Methanol Detection. Sens. Actuators B Chem. 2016, 237, 876–886. [Google Scholar] [CrossRef]
- Kröcher, O.; Devadas, M.; Elsener, M.; Wokaun, A.; Söger, N.; Pfeifer, M.; Demel, Y.; Mussmann, L. Investigation of the Selective Catalytic Reduction of NO by NH3 on Fe-ZSM5 Monolith Catalysts. Appl. Catal. B Environ. 2006, 66, 208–216. [Google Scholar] [CrossRef]
- Reiß, S.; Schönauer, D.; Hagen, G.; Fischerauer, G.; Moos, R. Monitoring the Ammonia Loading of Zeolite-Based Ammonia SCR Catalysts by a Microwave Method. Chem. Eng. Technol. 2011, 34, 791–796. [Google Scholar] [CrossRef]
- Pozar, D.M. Microwave Engineering, 4th ed.; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2012. [Google Scholar]
- Dietrich, M.; Rauch, D.; Porch, A.; Moos, R. A Laboratory Test Setup for in Situ Measurements of the Dielectric Properties of Catalyst Powder Samples under Reaction Conditions by Microwave Cavity Perturbation: Set up and Initial Tests. Sensors 2014, 14, 16856–16868. [Google Scholar] [CrossRef] [PubMed]
- Heine, C.; Girgsdies, F.; Truschke, A.; Schlögl, R.; Eichelbaum, M. The Model Oxidation Catalyst α-V2O5: Insights from Contactless in Situ Microwave Permittivity and Conductivity Measurements. Appl. Phys. A 2013, 112, 289–296. [Google Scholar] [CrossRef]
- Beulertz, G.; Votsmeier, M.; Moos, R. Effect of Propene, Propane, and Methane on Conversion and Oxidation State of Three-Way Catalysts: A Microwave Cavity Perturbation Study. Appl. Catal. B 2015, 165, 369–377. [Google Scholar] [CrossRef]
- Dietrich, M.; Rauch, D.; Simon, U.; Porch, A.; Moos, R. Ammonia Storage Studies on H-ZSM-5 Zeolites by Microwave Cavity Perturbation: Correlation of Dielectric Properties with Ammonia Storage. J. Sens. Sens. Syst. 2015, 4, 263–269. [Google Scholar] [CrossRef]
- Feulner, M.; Seufert, F.; Müller, A.; Hagen, G.; Moos, R. Influencing Parameters on the Microwave-Based Soot Load Determination of Diesel Particulate Filters. Top. Catal. 2017, 60, 374–380. [Google Scholar] [CrossRef]
- Moos, R. Microwave-Based Catalyst State Diagnosis—State of the Art and Future Perspectives. SAE Int. J. Engines 2015, 8, 1240–1245. [Google Scholar] [CrossRef]
- Hoffmann, R.K. Integrierte Mikrowellenschaltungen; Springer: Berlin/Heidelberg, Germany, 1983. [Google Scholar]
- Dietrich, M.; Steiner, C.; Hagen, G.; Moos, R. Radio-Frequency-Based Urea Dosing Control for Diesel Engines with Ammonia SCR Catalysts. SAE Int. J. Engines 2017, 10, 1638–1645. [Google Scholar] [CrossRef]
- Rauch, D.; Dietrich, M.; Simons, T.; Simon, U.; Porch, A.; Moos, R. Microwave Cavity Perturbation Studies on H-form and Cu Ion-Exchanged SCR Catalyst Materials: Correlation of Ammonia Storage and Dielectric Properties. Top. Catal. 2017, 60, 243–249. [Google Scholar] [CrossRef]
- Simon, U.; Flesch, U.; Maunz, W.; Müller, R.; Plog, C. The Effect of NH3 on the Ionic Conductivity of Dehydrated Zeolites Na Beta and H Beta. Microporous Mesoporous Mater. 1998, 21, 111–116. [Google Scholar] [CrossRef]
- Anderson, P.A.; Armstrong, A.R.; Porch, A.; Edwards, P.P.; Woodall, L.J. Structure and Electronic Properties of Potassium-Loaded Zeolite L. J. Phys. Chem. B 1997, 101, 9892–9900. [Google Scholar] [CrossRef]
- Kraus, M.; Kopinke, F.-D.; Roland, U. Influence of Moisture Content and Temperature on the Dielectric Permittivity of Zeolite NaY. Phys. Chem. Chem. Phys. 2011, 13, 4119–4125. [Google Scholar] [CrossRef] [PubMed]
- Niwa, M.; Iwamoto, M.; Segawa, K. Temperature-Programmed Desorption of Ammonia on Zeolites. Influence of the Experimental Conditions on the Acidity Measurement. BCSJ 1986, 59, 3735–3739. [Google Scholar] [CrossRef]
- Marr, I.; Groß, A.; Moos, R. Overview on Conductometric Solid-State Gas Dosimeters. J. Sens. Sens. Syst. 2014, 3, 29–46. [Google Scholar] [CrossRef]











| /GHz | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| R/mm | 29.2 | 14.6 | 9.72 | 7.28 | 5.81 | 4.84 | 4.14 | 3.62 | 3.21 | 2.88 |
| Step | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| ε’ | 3 + j0 | 3.2 + j0.1 | 3.4 + j0.2 | 3.6 + j0.3 | 3.8 + j0.4 | 4 + j0.5 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bogner, A.; Steiner, C.; Walter, S.; Kita, J.; Hagen, G.; Moos, R. Planar Microstrip Ring Resonators for Microwave-Based Gas Sensing: Design Aspects and Initial Transducers for Humidity and Ammonia Sensing. Sensors 2017, 17, 2422. https://doi.org/10.3390/s17102422
Bogner A, Steiner C, Walter S, Kita J, Hagen G, Moos R. Planar Microstrip Ring Resonators for Microwave-Based Gas Sensing: Design Aspects and Initial Transducers for Humidity and Ammonia Sensing. Sensors. 2017; 17(10):2422. https://doi.org/10.3390/s17102422
Chicago/Turabian StyleBogner, Andreas, Carsten Steiner, Stefanie Walter, Jaroslaw Kita, Gunter Hagen, and Ralf Moos. 2017. "Planar Microstrip Ring Resonators for Microwave-Based Gas Sensing: Design Aspects and Initial Transducers for Humidity and Ammonia Sensing" Sensors 17, no. 10: 2422. https://doi.org/10.3390/s17102422
APA StyleBogner, A., Steiner, C., Walter, S., Kita, J., Hagen, G., & Moos, R. (2017). Planar Microstrip Ring Resonators for Microwave-Based Gas Sensing: Design Aspects and Initial Transducers for Humidity and Ammonia Sensing. Sensors, 17(10), 2422. https://doi.org/10.3390/s17102422