An Architecture Providing Depolarization Ratio Capability for a Multi-Wavelength Raman Lidar: Implementation and First Measurements
Abstract
:1. Introduction
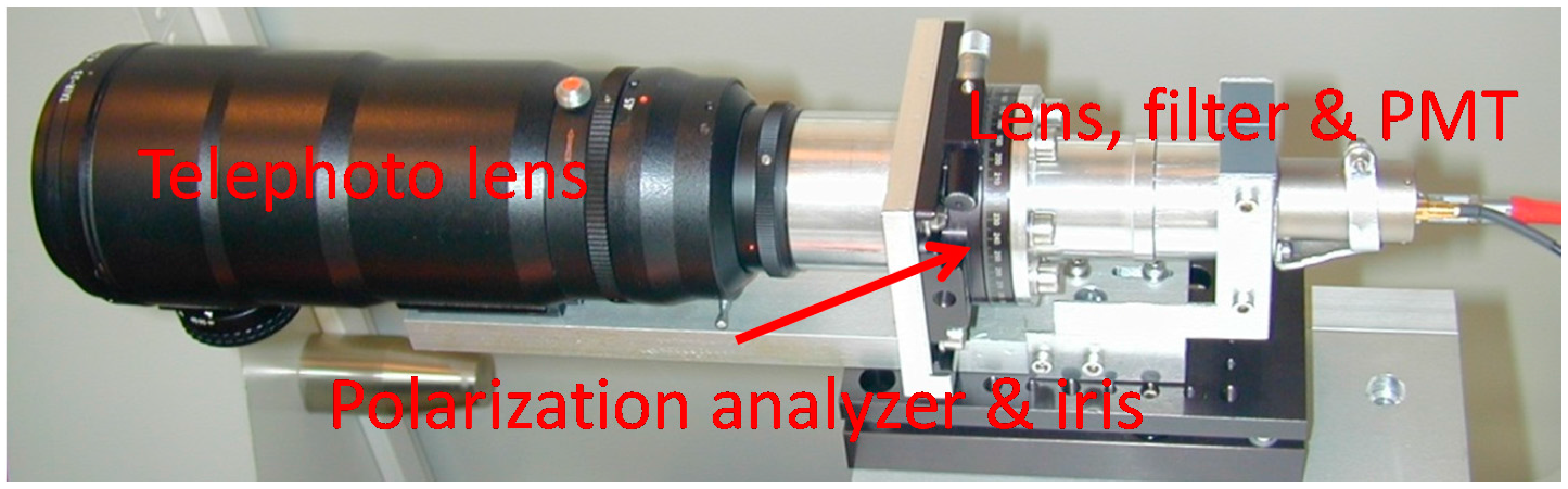
2. System Architecture
3. Theory of Operation
4. Calibrations
5. Depolarization Ratio Measurements
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Error Estimation
- is the average value of the signal detected by the total power channel, as a function of range,
- is the average value of the signal detected by the depolarization channel (with the polarizer oriented 90° from the transmitted beam polarization,
- is the standard deviation of the signal detected by the total power channel.
- is the standard deviation of the signal detected by the depolarization channel.
References
- Müller, D.; Ansmann, A.; Mattis, I.; Tesche, M.; Wandinger, U.; Althausen, D.; Pisani, G. Aerosol-type-dependent lidar ratios observed with Raman lidar. J. Geophys. Res. 2007, 112, D16202. [Google Scholar] [CrossRef]
- Angstrom, B.A.; Eppley, T. The parameters of atmospheric turbidity. Tellus 1964, 16, 64–75. [Google Scholar] [CrossRef]
- Schotland, R.M.; Sassen, K.; Stone, R. Observations by Lidar of Linear Depolarization Ratios for Hydrometeors. J. Appl. Meteorol. 1971, 10, 1011–1017. [Google Scholar] [CrossRef]
- Pal, S.R.; Carswell, A.I. Polarization properties of lidar backscattering from clouds. Appl. Opt. 1973, 12, 1530–1535. [Google Scholar] [CrossRef] [PubMed]
- Winker, D.M.; Osborn, M.T. Airborne lidar observations of the Pinatubo volcanic plume. Geophys. Res. Lett. 1992, 19, 167–170. [Google Scholar] [CrossRef]
- Murayama, T.; Müller, D.; Wada, K.; Shimizu, A.; Sekiguchi, M.; Tsukamoto, T. Characterization of Asian dust and Siberian smoke with multi-wavelength Raman lidar over Tokyo, Japan in Spring 2003. Geophys. Res. Lett. 2004, 31. [Google Scholar] [CrossRef]
- Tafuro, A.M.; Barnaba, F.; De Tomasi, F.; Perrone, M.R.; Gobbi, G.P. Saharan dust particle properties over the central Mediterranean. Atmos. Res. 2006, 81, 67–93. [Google Scholar] [CrossRef]
- Tesche, M.; Ansmann, A.; Müller, D.; Althausen, D.; Mattis, I.N.A.; Heese, B.; Freudenthaler, V.; Wiegner, M.; Esselborn, M.; Pisani, G.; et al. Vertical profiling of Saharan dust with Raman lidars and airborne HSRL in southern Morocco during SAMUM. Tellus Ser. B Chem. Phys. Meteorol. 2009, 61, 144–164. [Google Scholar] [CrossRef] [Green Version]
- Groß, S.; Gasteiger, J.; Freudenthaler, V.; Wiegner, M.; Geiß, A.; Schladitz, A.; Toledano, C.; Kandler, K.; Tesche, M.; Ansmann, A.; et al. Characterization of the planetary boundary layer during SAMUM-2 by means of lidar measurements. Tellus Ser. B Chem. Phys. Meteorol. 2011, 63, 695–705. [Google Scholar] [CrossRef]
- Groß, S.; Tesche, M.; Freudenthaler, V.; Toledano, C.; Wiegner, M.; Ansmann, A.; Althausen, D.; Seefeldner, M. Characterization of Saharan dust, marine aerosols and mixtures of biomass-burning aerosols and dust by means of multi-wavelength depolarization and Raman lidar measurements during SAMUM 2. Tellus Ser. B Chem. Phys. Meteorol. 2011, 63, 706–724. [Google Scholar] [CrossRef]
- Bravo-Aranda, J.A.; de Arruda Moreira, G.; Navas-Guzmán, F.; Granados-Muñoz, M.J.; Guerrero-Rascado, J.L.; Pozo-Vázquez, D.; Arbizu-Barrena, C.; Reyes, F.J.O.; Mallet, M.; Arboledas, L.A. A new methodology for PBL height estimations based on lidar depolarization measurements: Analysis and comparison against MWR and WRF model-based results. Atmos. Chem. Phys. 2017, 17, 6839–6851. [Google Scholar] [CrossRef]
- Wandinger, U.; Ansmann, A.; Mattis, I.; Müller, D.; Pappalardo, G. Calipso and beyond: Long-term ground-based support of space-borne aerosols and cloud lidar missions. In Proceedings of the 24th International Laser Radar Conference, Boulder, CO, USA, 23–27 June 2008; pp. 715–718. [Google Scholar]
- Burton, S.P.; Ferrare, R.A.; Hostetler, C.A.; Hair, J.W.; Rogers, R.R.; Obland, M.D.; Butler, C.F.; Cook, A.L.; Harper, D.B.; Froyd, K.D. Aerosol classification using airborne High Spectral Resolution Lidar measurements-methodology and examples. Atmos. Meas. Tech. 2012, 5, 73–98. [Google Scholar] [CrossRef] [Green Version]
- Burton, S.P.; Hair, J.W.; Kahnert, M.; Ferrare, R.A.; Hostetler, C.A.; Cook, A.L.; Harper, D.B.; Berkoff, T.A.; Seaman, S.T.; Collins, J.E.; et al. Observations of the spectral dependence of linear particle depolarization ratio of aerosols using NASA Langley airborne High Spectral Resolution Lidar. Atmos. Chem. Phys. 2015, 15, 13453–13473. [Google Scholar] [CrossRef]
- Olmo, F.J.; Quirantes, A.; Lara, V.; Lyamani, H.; Alados-Arboledas, L. Aerosol optical properties assessed by an inversion method using the solar principal plane for non-spherical particles. J. Quant. Spectrosc. Radiat. Transf. 2008, 109, 1504–1516. [Google Scholar] [CrossRef]
- Veselovskii, I.; Goloub, P.; Podvin, T.; Bovchaliuk, V.; Derimian, Y.; Augustin, P.; Fourmentin, M.; Tanre, D.; Korenskiy, M.; Whiteman, D.N.; et al. Retrieval of optical and physical properties of African dust from multiwavelength Raman lidar measurements during the SHADOW campaign in Senegal. Atmos. Chem. Phys. 2016, 16, 7013–7028. [Google Scholar] [CrossRef]
- Müller, D.; Veselovskii, I.; Kolgotin, A.; Tesche, M.; Ansmann, A.; Dubovik, O. Vertical profiles of pure dust and mixed smoke–dust plumes inferred from inversion of multiwavelength Raman/polarization lidar data and comparison to AERONET retrievals and in situ observations. Appl. Opt. 2013, 52, 3178–3202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chaikovsky, A.; Dubovik, O.; Holben, B.; Bril, A.; Goloub, P.; Tanré, D.; Pappalardo, G.; Wandinger, U.; Chaikovskaya, L.; Denisov, S.; et al. Lidar-Radiometer Inversion Code (LIRIC) for the retrieval of vertical aerosol properties from combined lidar/radiometer data: Development and distribution in EARLINET. Atmos. Meas. Tech. 2016, 9, 1181–1205. [Google Scholar] [CrossRef] [Green Version]
- Rodríguez-Gómez, A.; Sicard, M.; Muñoz-Porcar, C.; Barragán, R.; Comerón, A.; Rocadenbosch, F.; Vidal, E. Depolarization channel for barcelona lidar. Implementation and preliminary measurements. In Proceedings of the 28th International Laser Radar Conference, Bucharest, Romania, 25–30 June 2017; pp. 1–4. [Google Scholar]
- Kumar, D.; Rocadenbosch Burillo, F.; Sicard, M.; Comerón Tejero, A.; Muñoz, C.; Lange, D.; Tomás Martínez, S.; Gregorio, E. Six-channel polychromator design and implementation for the UPC elastic/Raman LIDAR. In Proceedings of the SPIE International Symposium—Remote Sensensing, Prague, Czech Republic, 19–20 September 2011; Volume 8182, pp. 81820W-1–81820W-10. [Google Scholar] [CrossRef] [Green Version]
- Althausen, D.; Müller, D.; Ansmann, A.; Wandinger, U.; Hube, H.; Clauder, E.; Zörner, S. Scanning 6-Wavelength 11-Channel Aerosol Lidar. J. Atmos. Ocean. Technol. 2000, 17, 1469–1482. [Google Scholar] [CrossRef]
- Freudenthaler, V.; Esselborn, M.; Wiegner, M.; Heese, B.; Tesche, M.; Ansmann, A.; Müller, D.; Althausen, D.; Wirth, M.; Fix, A.; et al. Depolarization ratio profiling at several wavelengths in pure Saharan dust during SAMUM 2006. Tellus Ser. B Chem. Phys. Meteorol. 2009, 61, 165–179. [Google Scholar] [CrossRef] [Green Version]
- De Tomasi, F.; Perrone, M.R. Multiwavelengths lidar to detect atmospheric aerosol properties. IET Sci. Meas. Technol. 2014, 8, 143–149. [Google Scholar] [CrossRef]
- Engelmann, R.; Kanitz, T.; Baars, H.; Heese, B.; Althausen, D.; Skupin, A.; Wandinger, U.; Komppula, M.; Stachlewska, I.S.; Amiridis, V.; et al. The automated multiwavelength Raman polarization and water-vapor lidar PollyXT: The neXT generation. Atmos. Meas. Tech. 2016, 9, 1767–1784. [Google Scholar] [CrossRef]
- Freudenthaler, V. About the effects of polarising optics on lidar signals and the Δ90 calibration. Atmos. Meas. Tech. 2016, 9, 4181–4255. [Google Scholar] [CrossRef]
- Esselborn, M.; Wirth, M.; Fix, A.; Weinzierl, B.; Rasp, K.; Tesche, M.; Petzold, A. Spatial distribution and optical properties of Saharan dust observed by airborne high spectral resolution lidar during SAMUM 2006. Tellus Ser. B Chem. Phys. Meteorol. 2009, 61, 131–143. [Google Scholar] [CrossRef] [Green Version]
- Lukacs, M.; Bhadra, D. Brilliant & Brilliant B User’s Manual; Quantel: Les Ulis, France, 2003; p. 157. [Google Scholar] [CrossRef]
- Wandinger, U. Introduction to Lidar. In Lidar; Weitkamp, C., Ed.; Springer: New York, NY, USA, 2005; pp. 1–18. [Google Scholar] [CrossRef]
- Gong, W.; Mao, F.; Li, J. OFLID: Simple method of overlap factor calculation with laser intensity distribution for biaxial lidar. Opt. Commun. 2011, 284, 2966–2971. [Google Scholar] [CrossRef]
- Mao, F.; Gong, W.; Li, J. Geometrical form factor calculation using Monte Carlo integration for lidar. Opt. Laser Technol. 2012, 44, 907–912. [Google Scholar] [CrossRef]
- Halldórsson, T.; Langerholc, J. Geometrical form factors for the lidar function. Appl. Opt. 1978, 17, 240–244. [Google Scholar] [CrossRef] [PubMed]
- Stelmaszczyk, K.; Dell’Aglio, M.; Chudzyński, S.; Stacewicz, T.; Wöste, L. Analytical function for lidar geometrical compression form-factor calculations. Appl. Opt. 2005, 44, 1323–1331. [Google Scholar] [CrossRef] [PubMed]
- Comeron, A.; Sicard, M.; Kumar, D.; Rocadenbosch, F. Use of a field lens for improving the overlap function of a lidar system employing an optical fiber in the receiver assembly. Appl. Opt. 2011, 50, 5538–5544. [Google Scholar] [CrossRef] [PubMed]
- Kumar, D.; Rocadenbosch, F. Determination of the overlap factor and its enhancement for medium-size tropospheric lidar systems: A ray-tracing approach. J. Appl. Remote Sens. 2013, 7, 1–15. [Google Scholar] [CrossRef]
- Kokkalis, P. Using paraxial approximation to describe the optical setup of a typical EARLINET lidar system. Atmos. Meas. Tech. 2017, 10, 3103–3115. [Google Scholar] [CrossRef]
- Vidal, E. Disseny D’un Canal de Despolarització a 532 nm per al Lidar d’EARLINET de la UPC. BarcelonaTech (2013). Available online: http://hdl.handle.net/2099.1/18273 (accessed on 15 July 2017).
- Licel. Transient Recorder Overview. Available online: http://licel.com/transient_overview.html (accessed on 5 July 2017).
- Sassen, K. Polarization in Lidar. In Lidar; Weitkamp, C., Ed.; Springer: New York, NY, USA, 2005; pp. 19–42. [Google Scholar] [CrossRef]
- Comerón, A.; Sicard, M.; Vidal, E.; Barragán, R.; Muñoz, C.; Rodríguez, A.; Tiana-Alsina, J.; Rocadenbosch, F.; García-Vizcaíno, D. Concept Design of a Multiwavelength Aerosol Lidar System with Mitigated Diattenuation Effects and Depolarization-Measurement Capability. In Proceedings of the 27th International Laser Radar Conference, New York, NY, USA, 5–10 July 2015; EPJ Web of Conferences. Volume 119. Article Number 23003. [Google Scholar] [CrossRef]
- Licel. Licel PM Module. Available online: http://licel.com/DET-HV.htm (accessed on 5 July 2017).
- Klett, J.D. Stable analytical inversion solution for processing lidar returns. Appl Opt. 1981, 20, 211–220. [Google Scholar] [CrossRef] [PubMed]
- Fernald, F.G. Analysis of atmospheric lidar observations: Some comments. Appl. Opt. 1984, 23, 652–653. [Google Scholar] [CrossRef] [PubMed]
- Ansmann, A.; Riebesell, M.; Weitkamp, C. Measurement of atmospheric aerosol extinction profiles with a Raman lidar. Opt. Lett. 1990, 15, 746–748. [Google Scholar] [CrossRef] [PubMed]
- Ansmann, A.; Wandinger, U.; Riebesell, M.; Weitkamp, C.; Michaelis, W. Independent measurement of extinction and backscatter profiles in cirrus clouds by using a combined Raman elastic-backscatter lidar. Appl. Opt. 1992, 31, 7113–7131. [Google Scholar] [CrossRef] [PubMed]
- Behrendt, A.; Nakamura, T. Calculation of the calibration constant of polarization lidar and its dependency on atmospheric temperature. Opt. Express 2002, 10, 805–817. [Google Scholar] [CrossRef] [PubMed]
- Belegante, L.; Bravo-Aranda, J.A.; Freudenthaler, V.; Nicolae, D.; Nemuc, A.; Alados-Arboledas, L.; Amodeo, A.; Pappalardo, G.; D’Amico, G.; Engelmann, R.; et al. Experimental assessment of the lidar polarizing sensitivity. Atmos. Meas. Tech. Discuss. 2016, 1–44. [Google Scholar] [CrossRef]
- Reba, M.M. Data Processing and Inversion Interfacing the UPC Elastic-Raman LIDAR System. Ph.D. Thesis, Universitat Politècnica de Catalunya, Barcelona, Spain, 2010. [Google Scholar]
- SigmaSpace. Micro Pulse Lidar Type 4, Instruction Manual; SigmaSpace Corporation: Lanham, MD, USA, 2012. [Google Scholar]
- Sicard, M.; Izquierdo, R.; Alarcón, M.; Belmonte, J.; Comerón, A.; Baldasano, J.M. Near-surface and columnar measurements with a micro pulse lidar of atmospheric pollen in Barcelona, Spain. Atmos. Chem. Phys. 2016, 16, 6805–6821. [Google Scholar] [CrossRef] [Green Version]
- Belmonte, J. Aerobiology of Barcelona. Historical and Current Data. Available online: http://lap.uab.cat/aerobiologia/en/historical/barcelona (accessed on 25 June 2017).
- Costa, M.J.; Guerrero-Rascado, J.; Sicard, M.; Gómez-Amo, J.L.; Ortíz-Amezcua, P.; Bortoli, D.; Comerón, A.; Marcos, C.; Bedoya, A.E.; Muñoz-Porcar, C.; et al. Main features of an outstanding desert dust transport over Iberia. In Proceedings of the 5th Iberian Meeting on Aerosol Science and Technology (RICTA), Barcelona, Spain, 3–6 July 2017. [Google Scholar]
- Groß, S.; Esselborn, M.; Weinzierl, B.; Wirth, M.; Fix, A.; Petzold, A. Aerosol classification by airborne high spectral resolution lidar observations. Atmos. Chem. Phys. 2013, 13, 2487–2505. [Google Scholar] [CrossRef] [Green Version]
- Sassen, K.; Hsueh, C. Contrail properties derived from high-resolution lidar studies during SUCCESS Geophys. Res. Lett. 1998, 25, 1165–1168. [Google Scholar] [CrossRef]
- Goodman, L.A. On the Exact Variance of Products. J. Am. Stat. Assoc. 1960, 55, 708–713. [Google Scholar] [CrossRef]
- Ku, H.H. Notes on the use of propagation of error formulas. J. Res. Natl. Bur. Stand. Sect. C Eng. Instrum. 1966, 70C, 263. [Google Scholar] [CrossRef]










| Parameter | Value |
|---|---|
| d4 | 138.9 mm (estimated) |
| d5 | 1 mm |
| d6 | 39.4 mm |
| d7 | 5 mm |
| d8 | 23 mm |
| Telephoto lens focal length | 300 mm |
| Eye-piece lens focal length | 38 mm |
| Field-of-view stop iris diameter | 1 mm |
| Interference filter | BARR 532-0.5 nm (custom made) |
| Center wavelength | 531.9 nm |
| Spectral width | 0.5 nm |
| Thickness | 11 mm |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rodríguez-Gómez, A.; Sicard, M.; Granados-Muñoz, M.-J.; Ben Chahed, E.; Muñoz-Porcar, C.; Barragán, R.; Comerón, A.; Rocadenbosch, F.; Vidal, E. An Architecture Providing Depolarization Ratio Capability for a Multi-Wavelength Raman Lidar: Implementation and First Measurements. Sensors 2017, 17, 2957. https://doi.org/10.3390/s17122957
Rodríguez-Gómez A, Sicard M, Granados-Muñoz M-J, Ben Chahed E, Muñoz-Porcar C, Barragán R, Comerón A, Rocadenbosch F, Vidal E. An Architecture Providing Depolarization Ratio Capability for a Multi-Wavelength Raman Lidar: Implementation and First Measurements. Sensors. 2017; 17(12):2957. https://doi.org/10.3390/s17122957
Chicago/Turabian StyleRodríguez-Gómez, Alejandro, Michaël Sicard, María-José Granados-Muñoz, Enis Ben Chahed, Constantino Muñoz-Porcar, Rubén Barragán, Adolfo Comerón, Francesc Rocadenbosch, and Eric Vidal. 2017. "An Architecture Providing Depolarization Ratio Capability for a Multi-Wavelength Raman Lidar: Implementation and First Measurements" Sensors 17, no. 12: 2957. https://doi.org/10.3390/s17122957
APA StyleRodríguez-Gómez, A., Sicard, M., Granados-Muñoz, M.-J., Ben Chahed, E., Muñoz-Porcar, C., Barragán, R., Comerón, A., Rocadenbosch, F., & Vidal, E. (2017). An Architecture Providing Depolarization Ratio Capability for a Multi-Wavelength Raman Lidar: Implementation and First Measurements. Sensors, 17(12), 2957. https://doi.org/10.3390/s17122957







