An Underwater Time Reversal Communication Method Using Symbol-Based Doppler Compensation with a Single Sound Pressure Sensor
Abstract
:1. Introduction
2. Theory of TRM-SBDC UWA Communication
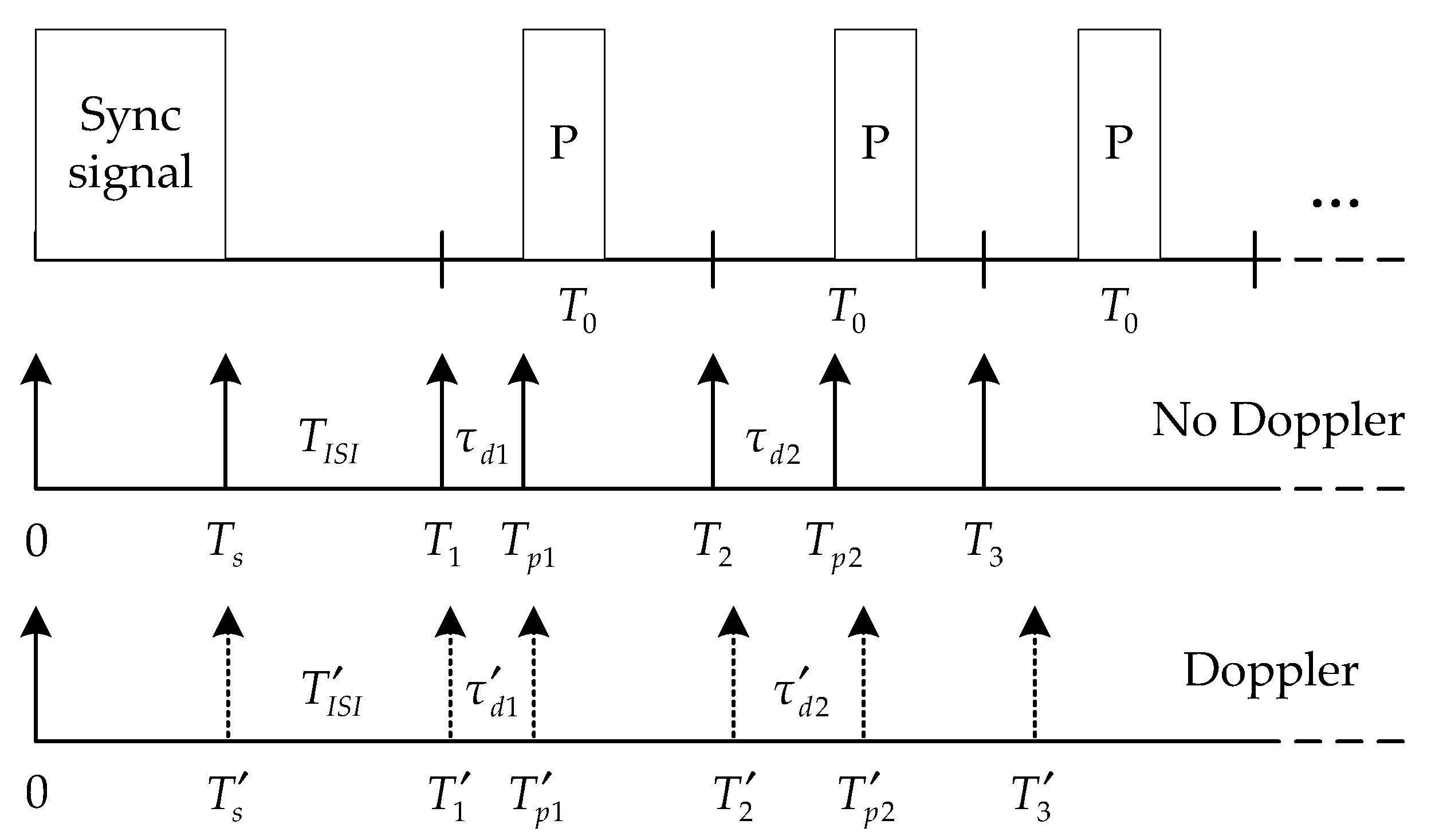
2.1. The Process Chain of the Communication System
2.2. Principles of PDS
2.3. Principles of Single Element TRM
2.4. Principles of Copy-Correlation
2.5. Principles of SBDC
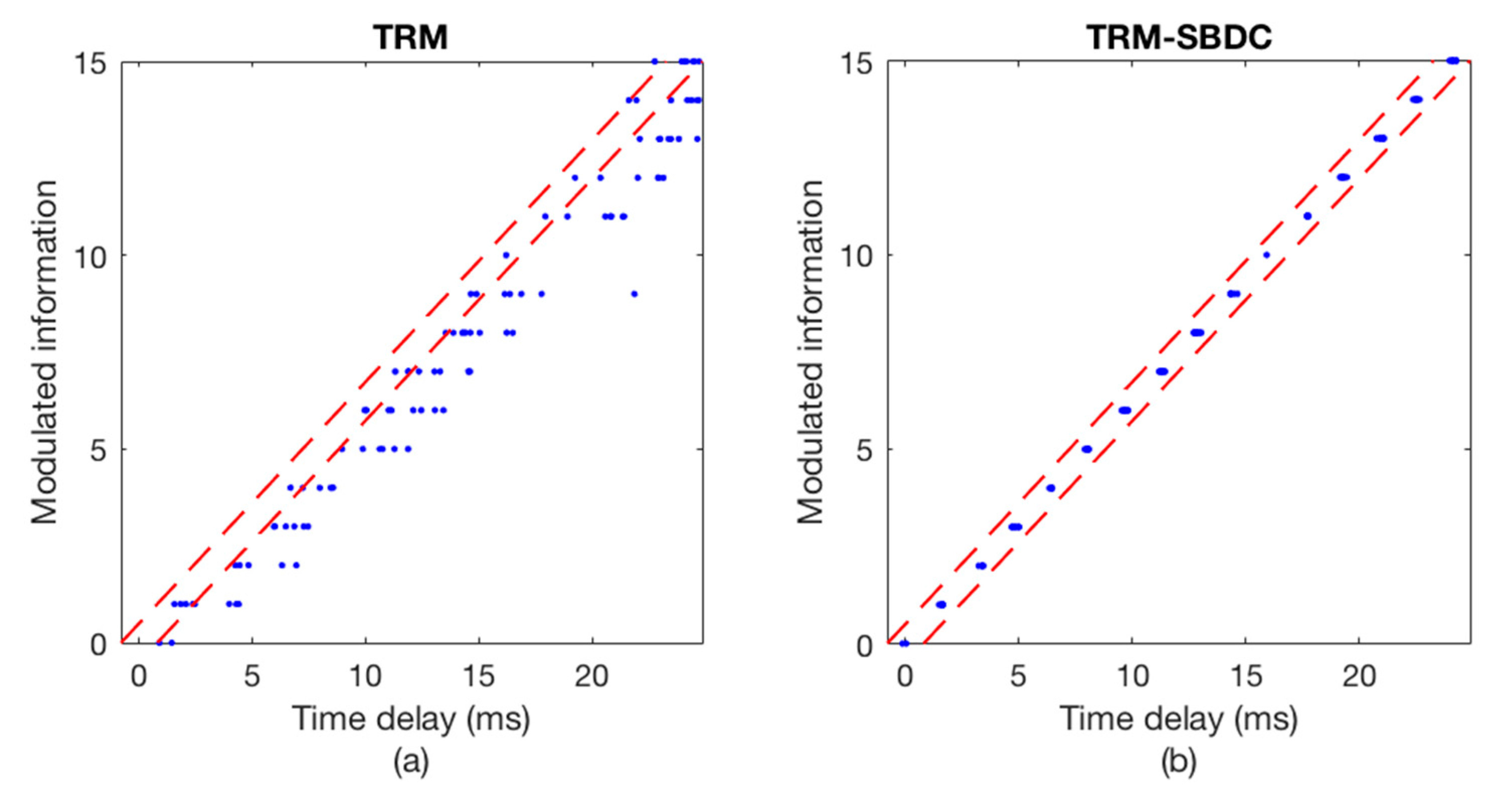
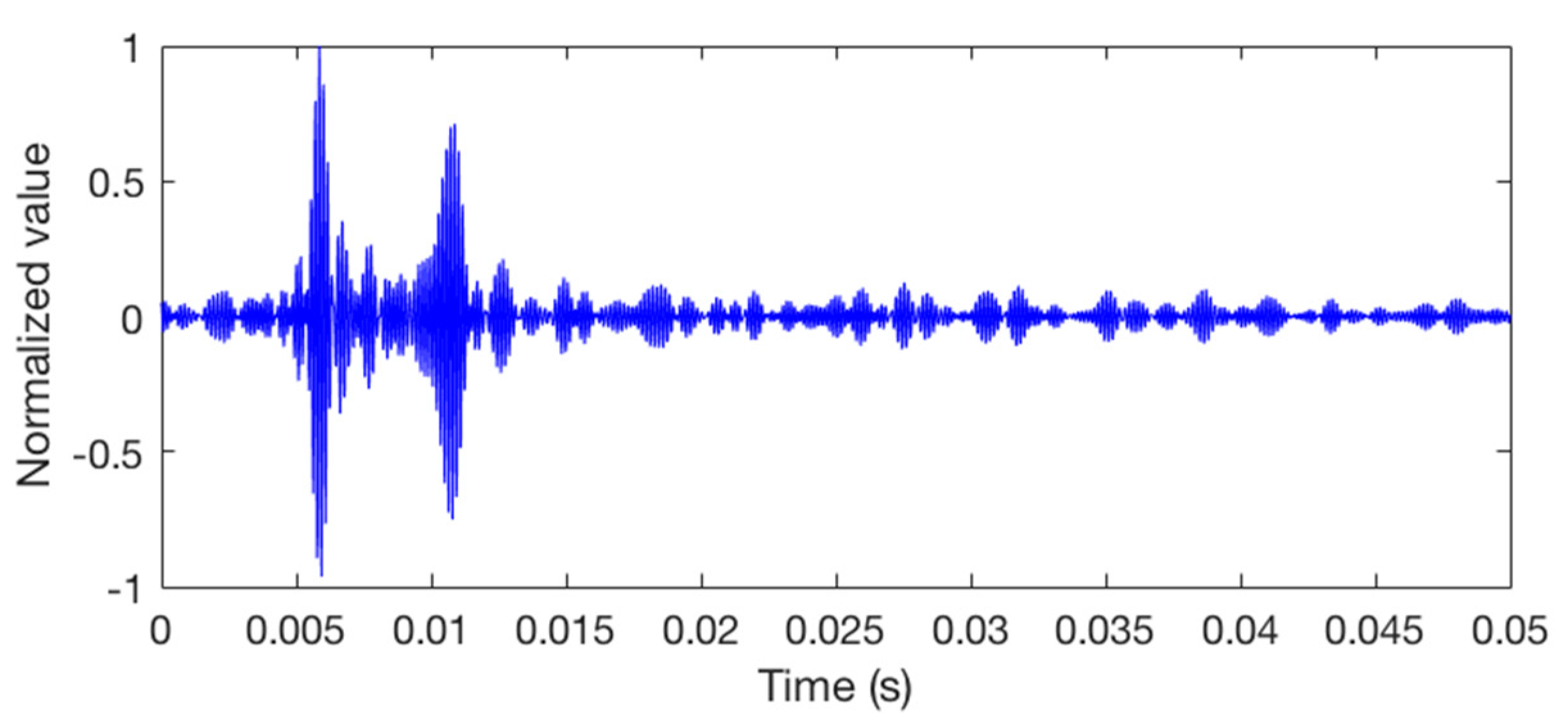
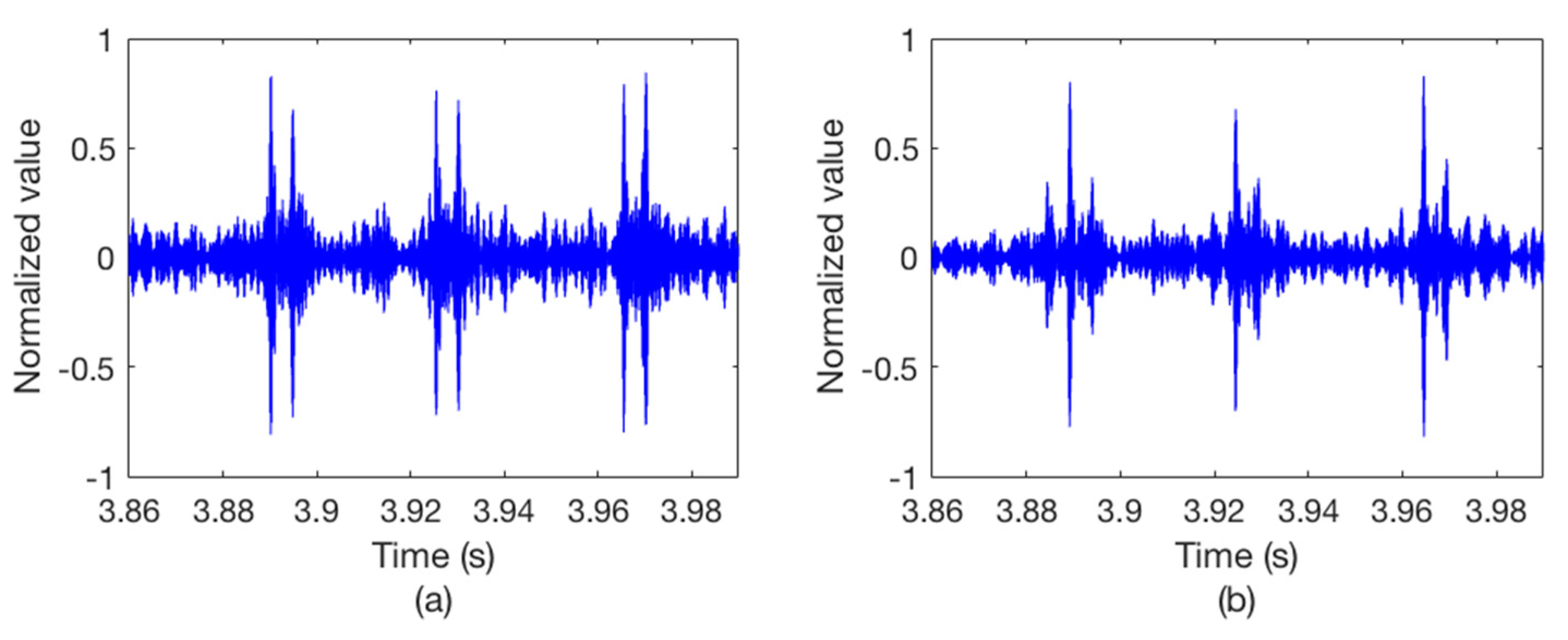
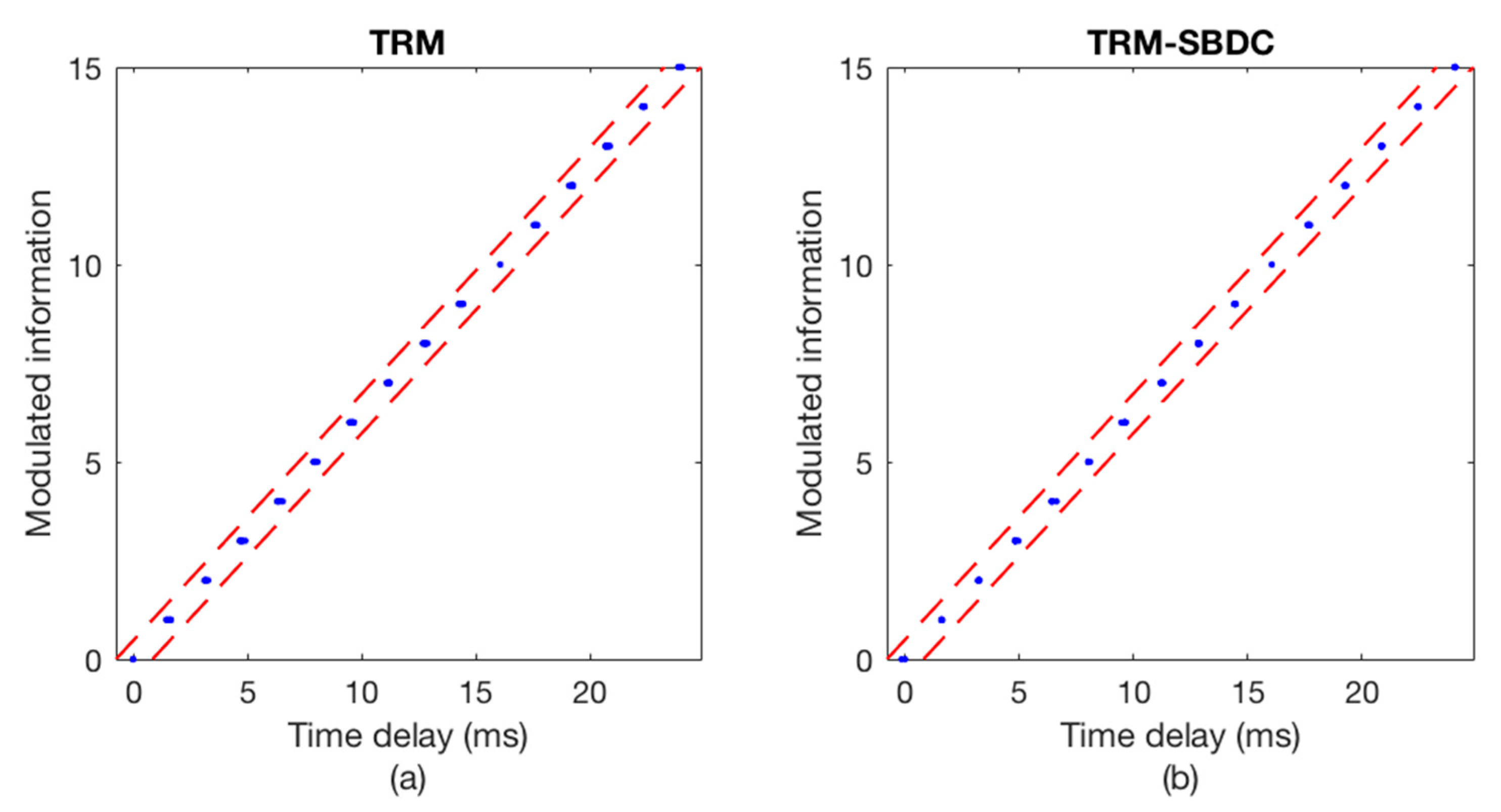
3. Simulations
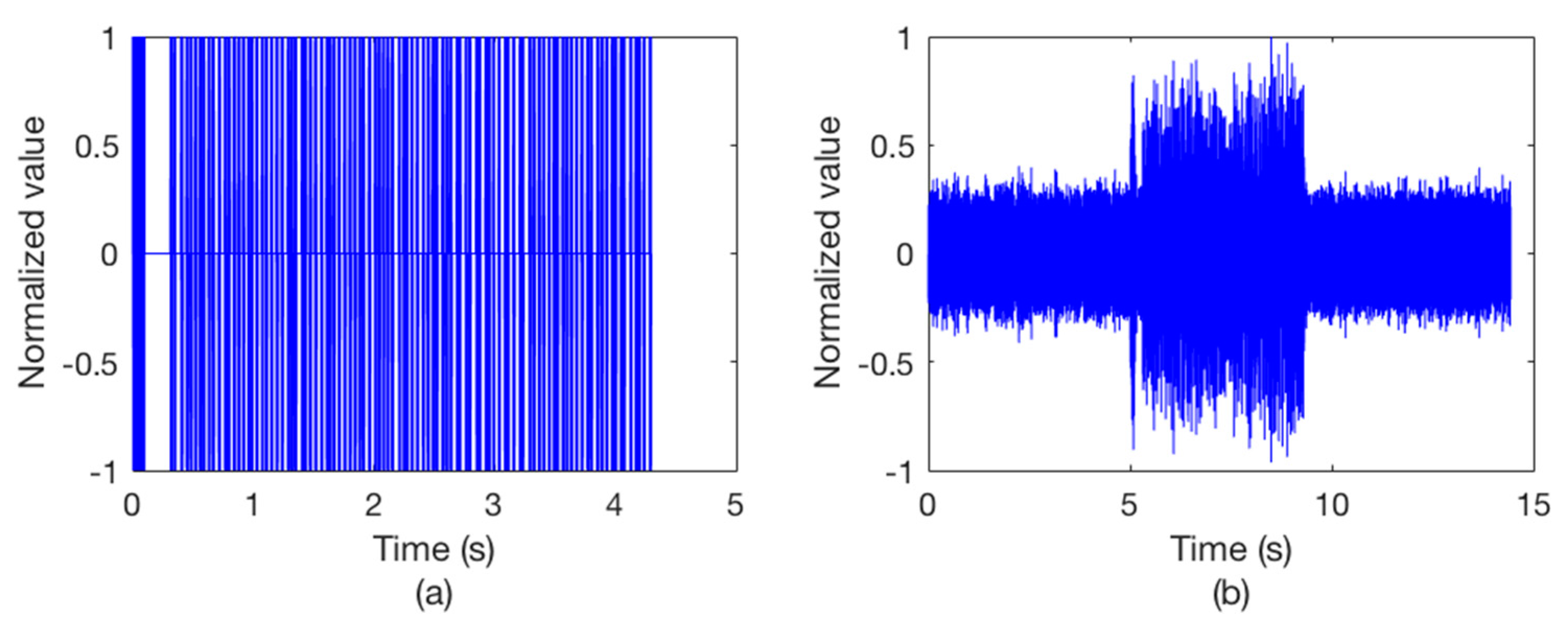
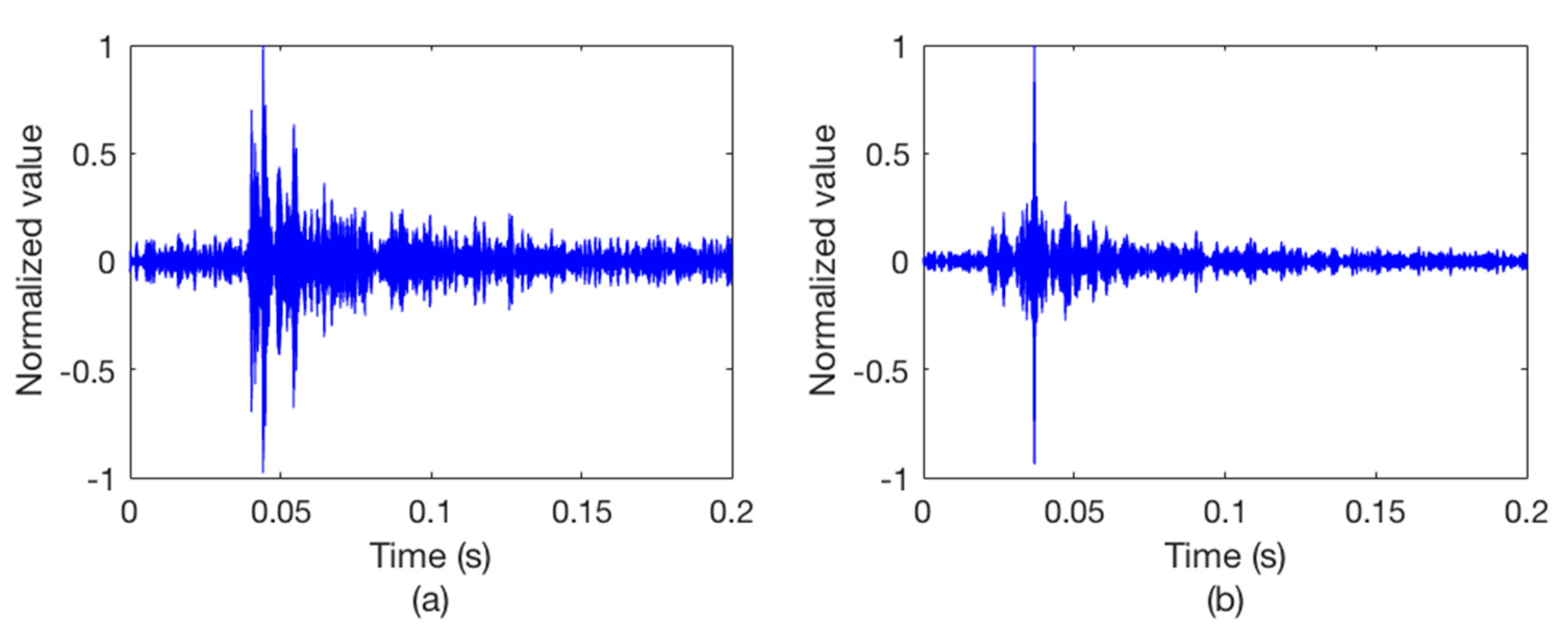
4. Experiments
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kilfoyle, D.B.; Baggeroer, A.B. The state of the art in underwater acoustic telemetry. IEEE J. Ocean. Eng. 2000, 25, 4–27. [Google Scholar] [CrossRef]
- Song, H.C.; Hodgkiss, W.S.; Kuperman, W.A.; Stevenson, M.; Akal, T. Improvement of time-reversal communications using adaptive channel equalizers. IEEE J. Ocean. Eng. 2006, 31, 487–496. [Google Scholar] [CrossRef]
- Song, H.C. An overview of underwater time-reversal communication. IEEE J. Ocean. Eng. 2016, 41, 644–655. [Google Scholar] [CrossRef]
- Edelmann, G.F.; Akal, T.; Hodgkiss, W.S.; Kim, S.; Kuperman, W.A.; Song, H.C. An initial demonstration of underwater acoustic communication using time reversal. IEEE J. Ocean. Eng. 2002, 27, 602–609. [Google Scholar] [CrossRef] [Green Version]
- Yang, T.C. Temporal resolutions of time-reversal and passive-phase conjugation for underwater acoustic communications. IEEE J. Ocean. Eng. 2003, 28, 229–245. [Google Scholar] [CrossRef]
- Song, H.C. Time reversal communication with a mobile source. J. Acoust. Soc. Am. 2013, 134, 2623–2626. [Google Scholar] [CrossRef] [PubMed]
- Shimura, T.; Watanabe, Y.; Ochi, H.; Song, H.C. Long-range time reversal communication in deep water: Experimental results. J. Acoust. Soc. Am. 2012, 132, EL49–EL53. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shimura, T.; Ochi, H.; Song, H.C. Experimental demonstration of multiuser communication in deep water using time reversal. J. Acoust. Soc. Am. 2013, 134, 3223–3229. [Google Scholar] [CrossRef] [PubMed]
- Song, H.C.; Howe, B.; Brown, M.; Andrew, R. Diversity-based acoustic communication with a glider in deep water. J. Acoust. Soc. Am. 2014, 135, 1023–1026. [Google Scholar] [CrossRef] [PubMed]
- Shimura, T.; Kida, Y.; Deguchi, M. Basic research on MIMO underwater communication using adaptive time reversal. In Proceedings of the 2017 IEEE Oceans, Aberdeen, UK, 19–22 June 2017. [Google Scholar]
- Song, A.; Badiey, M.; Song, H.C.; Hodgkiss, W.S.; Poter, M.B. Impact of ocean variability on coherent underwater acoustic communications during the Kauai experiment. J. Acoust. Soc. Am. 2008, 123, 856–865. [Google Scholar] [CrossRef] [PubMed]
- Song, H.C.; Hodgkiss, W.S. Self-synchronization and spatial diversity of passive time reversal communication. J. Acoust. Soc. Am. 2015, 137, 2974–2977. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yin, J.W.; Du, P.Y.; Shen, J.W.; Guo, L.X. Study on time reverse mirror in underwater acoustic communication. In Proceedings of the Meetings on Acoustics, Montreal, QC, Canada, 2–7 June 2013. [Google Scholar]
- Han, X.; Yin, J.W.; Du, P.Y.; Zhang, X. Experimental demonstration of underwater acoustic communication using bionic signals. Appl. Acoust. 2014, 78, 7–10. [Google Scholar] [CrossRef]
- Zhao, A.B.; Zeng, C.G.; Hui, J.; Ma, L.; Bi, X.J. Experimental Demonstration of Long-Range Underwater Acoustic Communication Using a Vertical Sensor Array. Sensors 2017, 17, 1516. [Google Scholar] [CrossRef] [PubMed]
- Johnson, M.; Freitag, L.; Stojanovic, M. Improved Doppler tracking and correction for underwater acoustic communications. In Proceedings of the 1997 IEEE International Conference on Acoustics, Speech and Signal Processing, Munich, Germany, 21–24 April 1997. [Google Scholar]
- Tu, K.; Duman, T.M.; Stojanovic, M.; Proakis, J.G. Multiple-resampling receiver design for OFDM over Doppler-distorted underwater acoustic channels. IEEE J. Ocean. Eng. 2013, 38, 333–345. [Google Scholar] [CrossRef] [Green Version]
- Song, H.C.; Hodgkiss, W.S.; Kuperman, W.A.; Higley, W.J.; Raghukumar, K.; Akal, T.; Stevenson, M. Spatial diversity in passive time reversal communications. J. Acoust. Soc. Am. 2006, 120, 2067–2076. [Google Scholar] [CrossRef]
- Kim, Y.H.; Song, I.; Yoon, S.; Park, S.R. An efficient frequency offset estimator for OFDM systems and its performance characteristics. IEEE Trans. Veh. Technol. 2001, 50, 1307–1312. [Google Scholar] [CrossRef] [Green Version]
- Tufvesson, F.; Edfors, O.; Faulkner, M. Time and frequency synchronization for OFDM using PN-sequence preambles. In Proceedings of the IEEE VTS 50th Vehicular Technology Conference, Amsterdam, The Netherlands, 19–22 September 1999. [Google Scholar]
- Sharif, B.S.; Neasham, J.; Hinton, O.R.; Adams, A.E. A computationally efficient Doppler compensation system for underwater acoustic communications. IEEE J. Ocean. Eng. 2000, 25, 52–61. [Google Scholar] [CrossRef]
- Luan, Y.F.; Yan, S.F.; Qin, Y.; Xu, L.J. Doppler Estimation Using Time Reversal Mirror for Underwater Acoustic Time-varying Multipath Channel. In Proceedings of the 2017 IEEE International Conference on Signal Processing, Communications and Computing (ICSPCC), Xiamen, China, 22–25 October 2017. [Google Scholar]
- Hui, J.Y.; Liu, L.; Liu, H.; Feng, H.H. A study on pattern time delay coding underwater acoustic communication. Acta Acust. 1999, 24, 561–573. [Google Scholar]
- Yin, J.W.; Hui, J.Y.; Hui, J.; Yao, Z.X.; Wang, Y.L. Underwater acoustic communication based on pattern time delay shift coding scheme. Ocean. Eng. 2006, 20, 499–508. [Google Scholar]
- Yin, J.W.; Zhang, X.; Zhu, G.P.; Tang, S.Y.; Sun, H. Parametric array differential pattern time delay shift coding underwater acoustic communication in the under-ice environment. Chin. J. Acoust. 2016, 35, 431–439. [Google Scholar] [CrossRef]
- van Walree, P.A.; Socheleau, F.-X.; Otnes, R.; Jenserud, T. The watermark benchmark for underwater acoustic modulation schemes. IEEE J. Ocean. Eng. 2017, 42, 1007–1018. [Google Scholar] [CrossRef]

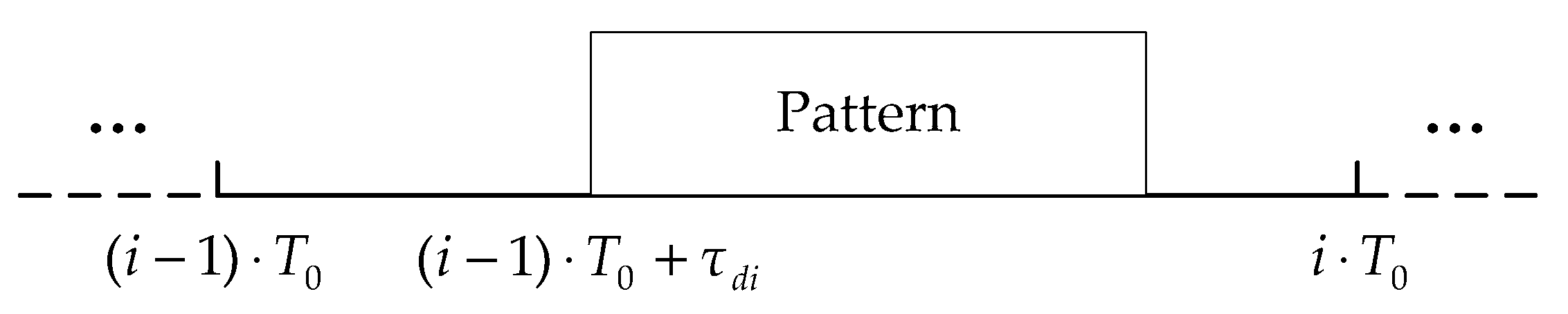







| Parameter | Value |
|---|---|
| Ts (ms) | 100 |
| TISI (ms) | 200 |
| Tp (ms) | 16 |
| Tc (ms) | 24 |
| Bandwidth (kHz) | 2(5.5–7.5) |
| n (bit) | 4 |
| Information size (bit) | 400 |
| Communication rate (bit/s) | 100 |
| Hydrophone Channels of KAU2 | BER (%) | |
|---|---|---|
| TRM | TRM-SBDC | |
| Hydrophone channel 4 | 34.000 | 0 |
| Hydrophone channel 8 | 30.893 | 0.321 |
| Hydrophone channel 12 | 34.036 | 1.429 |
| Experimental Scene | Initial Distance (m) | Status of the Sending Boat |
|---|---|---|
| 1 | 450 | moored |
| 2 | 705 | drifting |
| 3 | 640 | drifting |
| 4 | 690 | drifting |
| Experimental Scene | BER (%) | |
|---|---|---|
| TRM | TRM-SBDC | |
| 1 | 0 | 0 |
| 2 | 15.325 | 0 |
| 3 | 26.550 | 0.050 |
| 4 | 24.025 | 0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, A.; Zeng, C.; Hui, J.; Ma, L.; Bi, X. An Underwater Time Reversal Communication Method Using Symbol-Based Doppler Compensation with a Single Sound Pressure Sensor. Sensors 2018, 18, 3279. https://doi.org/10.3390/s18103279
Zhao A, Zeng C, Hui J, Ma L, Bi X. An Underwater Time Reversal Communication Method Using Symbol-Based Doppler Compensation with a Single Sound Pressure Sensor. Sensors. 2018; 18(10):3279. https://doi.org/10.3390/s18103279
Chicago/Turabian StyleZhao, Anbang, Caigao Zeng, Juan Hui, Lin Ma, and Xuejie Bi. 2018. "An Underwater Time Reversal Communication Method Using Symbol-Based Doppler Compensation with a Single Sound Pressure Sensor" Sensors 18, no. 10: 3279. https://doi.org/10.3390/s18103279
APA StyleZhao, A., Zeng, C., Hui, J., Ma, L., & Bi, X. (2018). An Underwater Time Reversal Communication Method Using Symbol-Based Doppler Compensation with a Single Sound Pressure Sensor. Sensors, 18(10), 3279. https://doi.org/10.3390/s18103279




