Wheel Force Sensor-Based Techniques for Wear Detection and Analysis of a Special Road
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Principle of Road Load Acquisition
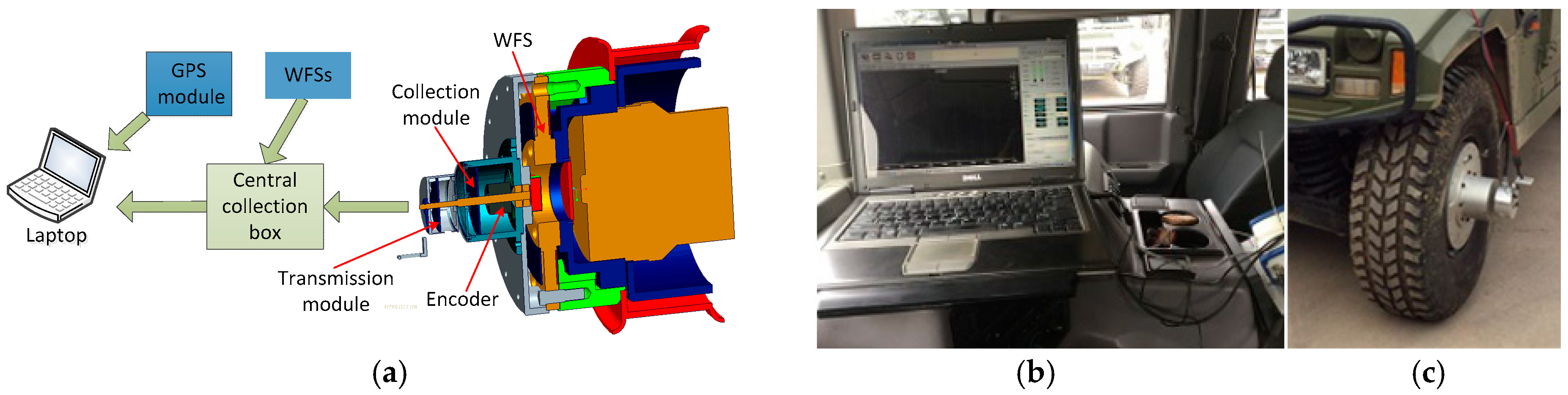
2.2. Road Load Acquisition System
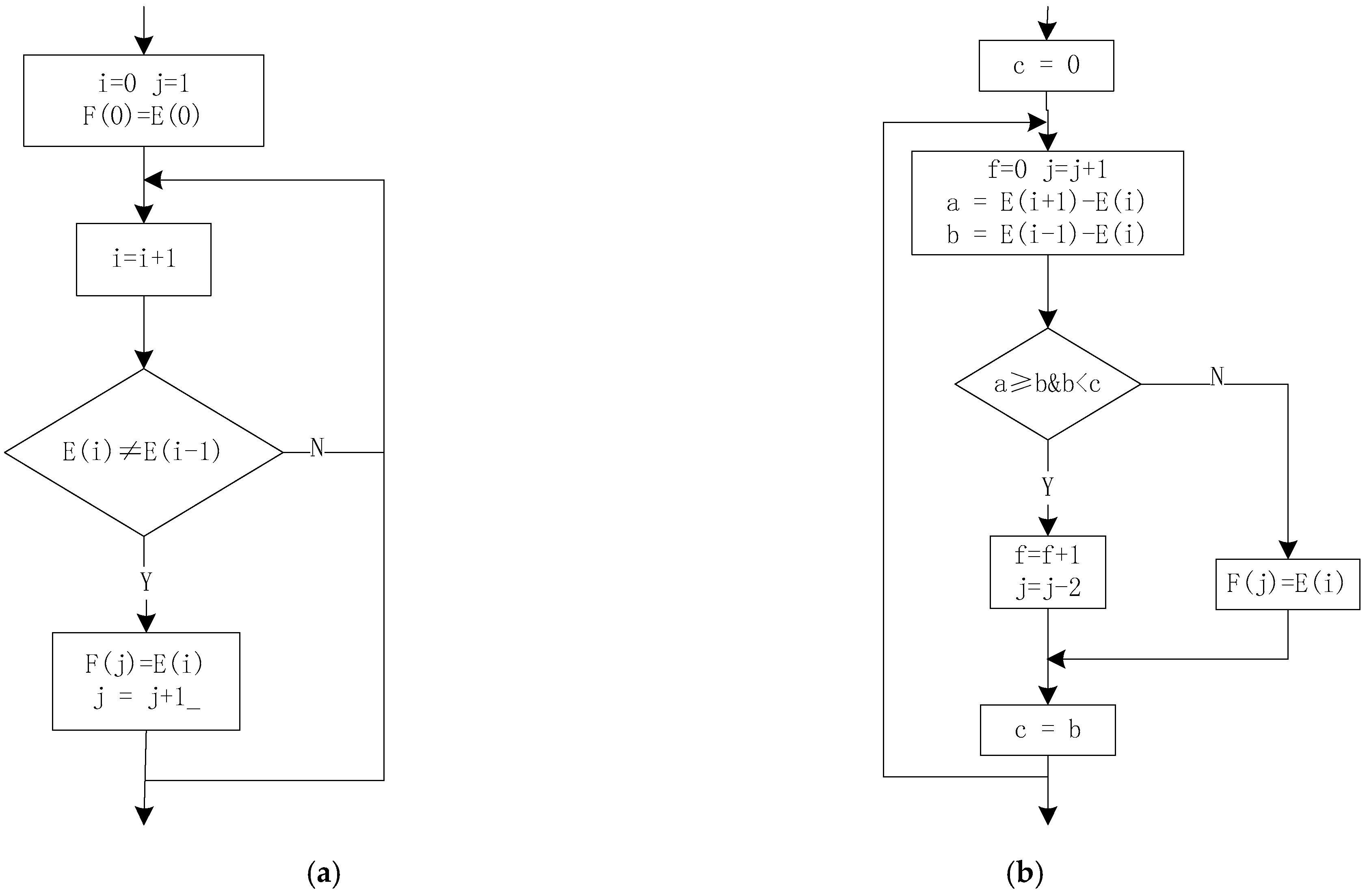
2.3. Road Load Processing for Rain flow Counting Method
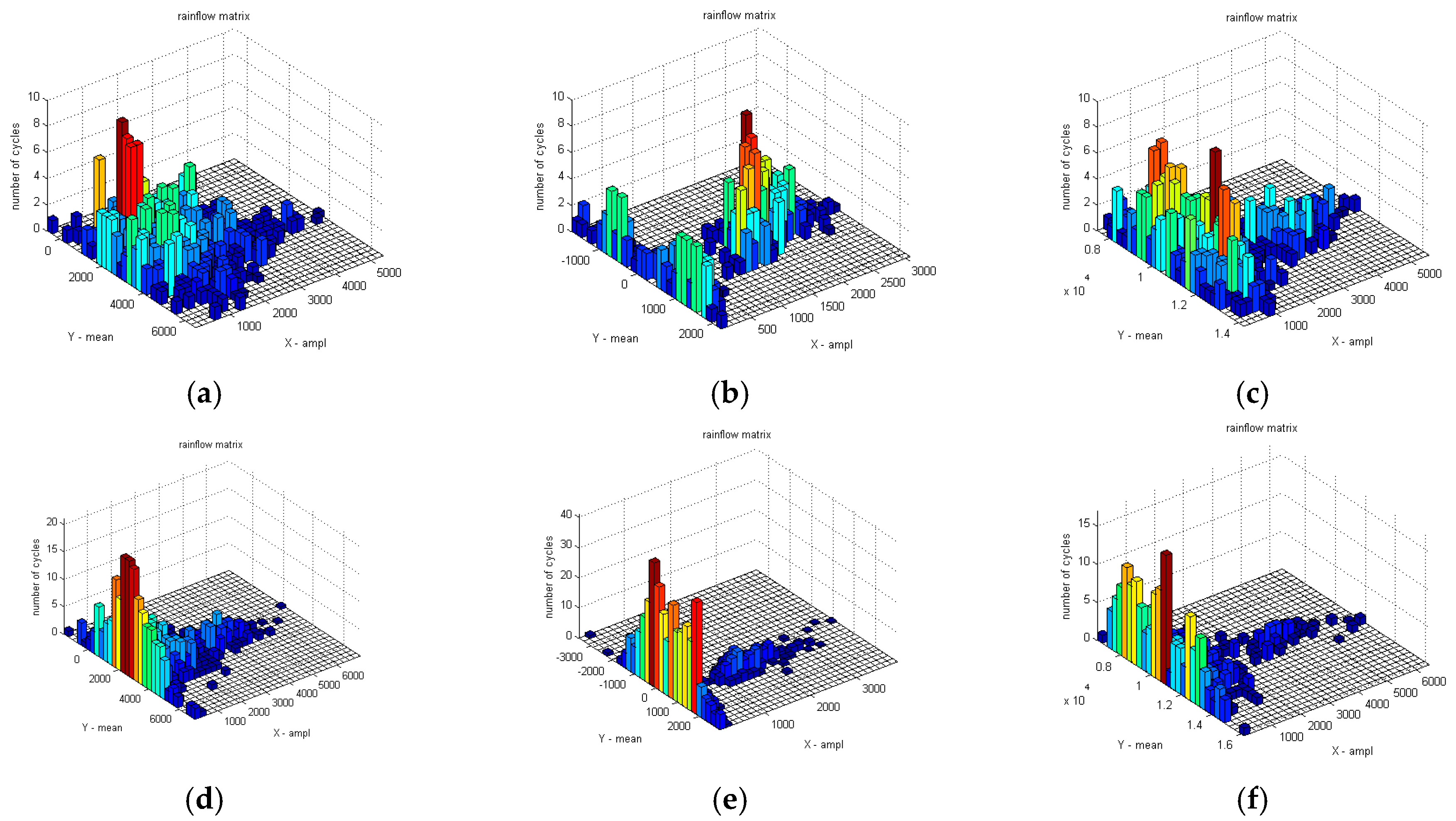
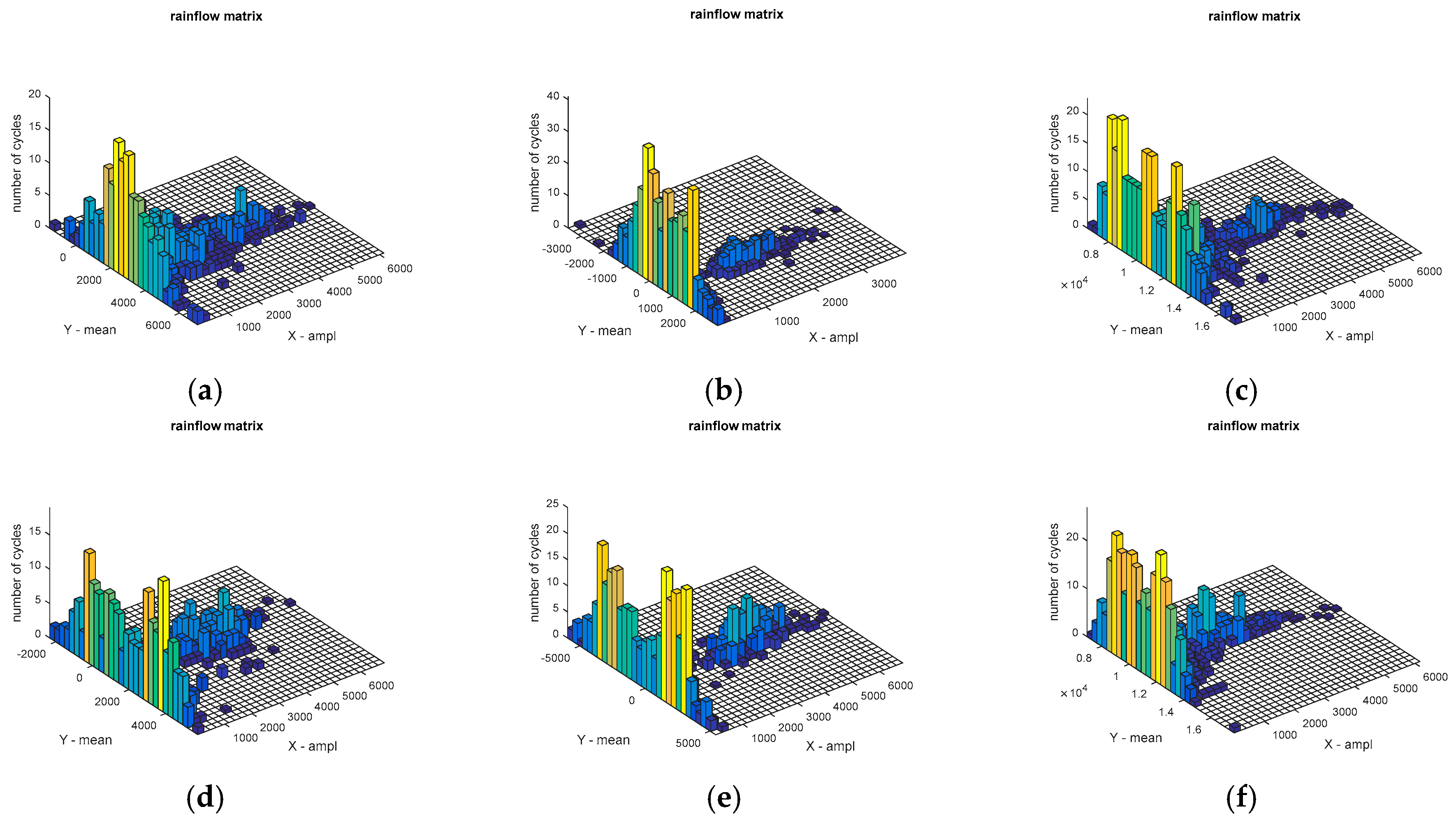
3. Results
4. Discussion
4.1. Multiple Road Load Collection Experiment
4.2. The Rain Flow Counting Model Improvement
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lin, C.L.; Yang, M.Y.; Chen, E.P.; Chen, Y.C.; Yu, W.C. Antilock braking control system for electric vehicles. J. Eng. 2018, 2018, 60–67. [Google Scholar] [CrossRef]
- He, W.; He, J.; Ren, W. Damage localization of beam structures using mode shape extracted from moving vehicle response. Measurement 2018, 121, 276–285. [Google Scholar] [CrossRef]
- Xu, L.; Reimer, U.; Li, J.; Huang, H.; Hu, Z.; Jiang, H.; Janßen, H.; Ouyang, M.; Lehnert, W. Design of durability test protocol for vehicular fuel cell systems operated in power-follow mode based on statistical results of on-road data. J. Power Sources 2017, 377, 59–69. [Google Scholar] [CrossRef]
- Li, Z.; Mitsuoka, M.; Inoue, E.; Okayasu, T.; Hirai, Y.; Zhu, Z. Parameter sensitivity for tractor lateral stability against Phase I overturn on random road surfaces. Biosyst. Eng. 2016, 150, 10–23. [Google Scholar] [CrossRef]
- Yan, D.; Luo, Y.; Lu, N.; Yuan, M.; Beer, M. Fatigue Stress Spectra and Reliability Evaluation of Short- to Medium-Span Bridges under Stochastic and Dynamic Traffic Loads. J. Bridge Eng. 2017, 22, 04017102. [Google Scholar] [CrossRef]
- Hassan, R.; Evans, R. Road roughness characteristics in car and truck wheel tracks. Int. J. Pavement Eng. 2013, 14, 736–745. [Google Scholar] [CrossRef]
- Cheng, G.; Guo, W.; Lei, Y. Research on the Airport Pavement Roughness Evaluation Based on Full Aircraft Model. Highw. Eng. 2016, 4, 006. [Google Scholar]
- Katicha, S.W.; Khoury, J.E.; Flintsch, G.W. Assessing the effectiveness of probe vehicle acceleration measurements in estimating road roughness. Int. J. Pavement Eng. 2016, 17, 698–708. [Google Scholar] [CrossRef]
- Radopoulou, S.C.; Brilakis, I. Automated Detection of Multiple Pavement Defects. J. Comput. Civ. Eng. 2016, 31, 04016057. [Google Scholar] [CrossRef] [Green Version]
- Kim, R.E.; Kang, S.; Spencer, B.F.; Ozer, H.; Al-Qadi, I.L. Stochastic Analysis of Energy Dissipation of a Half-Car Model on Nondeformable Rough Pavement. J. Transp. Eng. Part B Pavements 2017, 143, 04017016. [Google Scholar] [CrossRef]
- Alleva, L. Road Surface Roughness Measuring System and Method. WO2012029026A1, 8 March 2012. [Google Scholar]
- Ramasubramanian, M.K.; Jackson, S.D. A sensor for measurement of frition coefficient on moving flexible surfaces. IEEE Sens. J. 2005, 5, 844–849. [Google Scholar] [CrossRef]
- Kim, J.H.; Kang, D.I.; Shin, H.H.; Park, Y.K. Design and analysis of a column type multi-component force/moment sensor. Measurement 2003, 3, 213–219. [Google Scholar] [CrossRef]
- Lin, G.; Pang, H.; Zhang, W.; Wang, D.; Feng, L. A self-decoupled three-axis force sensor for measuring the wheel force. Proc. Inst. Mech. Eng. Part D J. Autom. Eng. 2014, 228, 319–334. [Google Scholar] [CrossRef]
- Shinde, V.; Jha, J.; Tewari, A.; Miashra, S. Modified Rain Flow Counting Algorithm for Fatigue Life Calculation; Springer: Singapore, 2018. [Google Scholar]
- Chen, C.; Yang, Z.; He, J.; Tian, H.; Li, S.; Wang, D. Load spectrum generation of machining center based on rainflowrain flow counting method. J. Vibroeng. 2017, 19, 5767–5779. [Google Scholar] [CrossRef]
- Sadowski, N.; Lajoie-Mazenc, M.; Bastos, J.P.; da Luz, M.F.; Kuo-Peng, P. Evaluation and analysis of iron losses in electrical machines using the rain flow method. IEEE Trans. Magn. 2000, 36, 1923–1926. [Google Scholar] [CrossRef]
- Liu, G.; Wang, D.; Hu, Z. Application of the Rain-flowrain flow Counting Method in Fatigue. In Proceedings of the International Conference on Electronics, Network and Computer Engineering, Anaheim, CA, USA, 16–19 February 2016. [Google Scholar]
- Shinde, V.; Jha, J.; Tewari, A.; Miashra, S. Fatigue life assessment for large components based on rainflowrain flow counted local strains using the damage domain. Int. J. Fatigue 2014, 68, 150–158. [Google Scholar]








| Mean Range | The Number of Mean Cycles | Amplitude Range | The Number of Amplitude Cycles | The Number of Cycles That Satisfy Both the Mean Range and the Amplitude Range | |||
|---|---|---|---|---|---|---|---|
| The standard road | The right WFS | Fx | 1000 N–3000 N | 836 | >1000 N | 775 | 553 |
| Fy | −1000 N–1000 N | 845 | >1000 N | 712 | 587 | ||
| Fz | 8 KN–14 KN | 1205 | >1000 N | 1031 | 844 | ||
| The left WFS | Fx | 1000 N–3000 N | 822 | >1000 N | 745 | 576 | |
| Fy | −1000 N–1000 N | 876 | >1000 N | 732 | 565 | ||
| Fz | 8 KN–14 KN | 1188 | >1000 N | 989 | 812 | ||
| The target road | The right WFS | Fx | 1000 N–3000 N | 755 | >1000 N | 703 | 489 |
| Fy | −1000 N–1000 N | 765 | >1000 N | 683 | 534 | ||
| Fz | 8 KN–14 KN | 1033 | >1000 N | 897 | 776 | ||
| The left WFS | Fx | 1000 N–3000 N | 769 | >1000 N | 715 | 508 | |
| Fy | −1000 N–1000 N | 778 | >1000 N | 682 | 515 | ||
| Fz | 8 KN–14 KN | 994 | >1000 N | 917 | 749 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, H.; Zhang, W.; Wang, D. Wheel Force Sensor-Based Techniques for Wear Detection and Analysis of a Special Road. Sensors 2018, 18, 2493. https://doi.org/10.3390/s18082493
Yan H, Zhang W, Wang D. Wheel Force Sensor-Based Techniques for Wear Detection and Analysis of a Special Road. Sensors. 2018; 18(8):2493. https://doi.org/10.3390/s18082493
Chicago/Turabian StyleYan, Huawen, Weigong Zhang, and Dong Wang. 2018. "Wheel Force Sensor-Based Techniques for Wear Detection and Analysis of a Special Road" Sensors 18, no. 8: 2493. https://doi.org/10.3390/s18082493
APA StyleYan, H., Zhang, W., & Wang, D. (2018). Wheel Force Sensor-Based Techniques for Wear Detection and Analysis of a Special Road. Sensors, 18(8), 2493. https://doi.org/10.3390/s18082493





