A Flow Velocity Measurement Method Based on a PVDF Piezoelectric Sensor
Abstract
:1. Introduction
2. Theory
2.1. Piezoelectric Principle of PVDF Film
2.2. Acoustic Principle of Turbulent Fluctuating Pressure
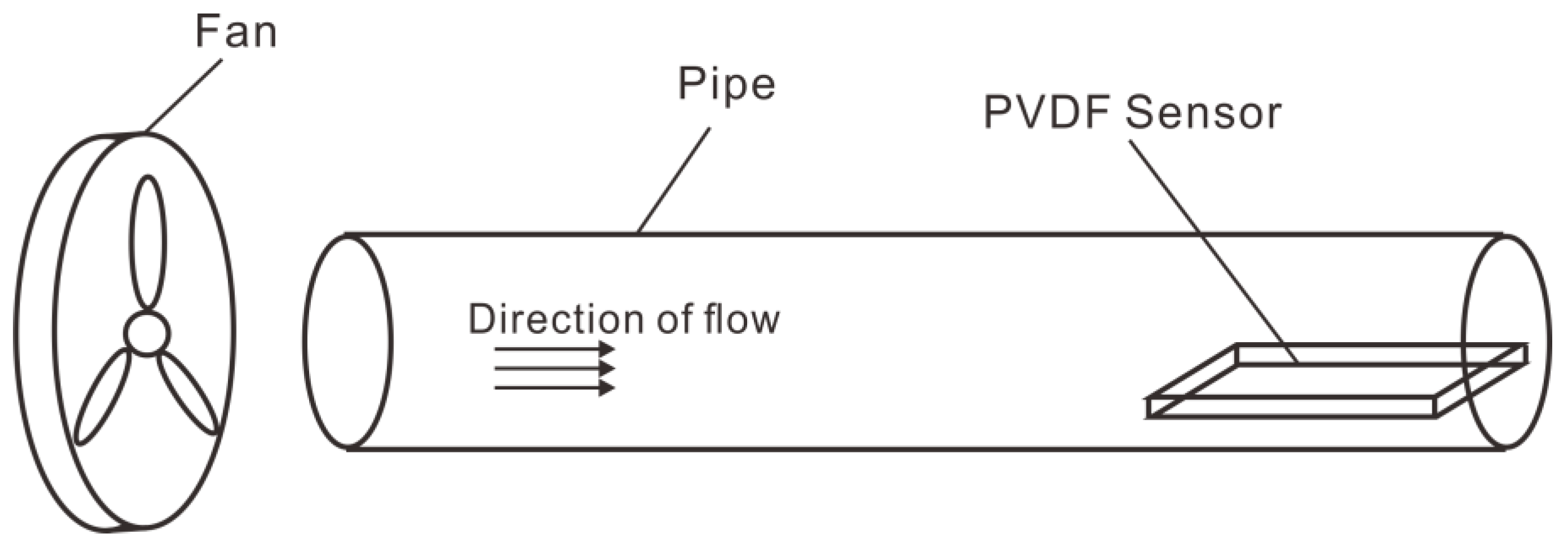
2.3. Experimental Principle
3. Finite-Element Simulation of Flow Excitation Noise
3.1. Flow-Field Modeling and Meshing
3.2. Numerical Simulation of Flow Field
3.3. Numerical Simulation of Sound Field
4. Flow-Rate Measurement Experiment and Analysis of Results
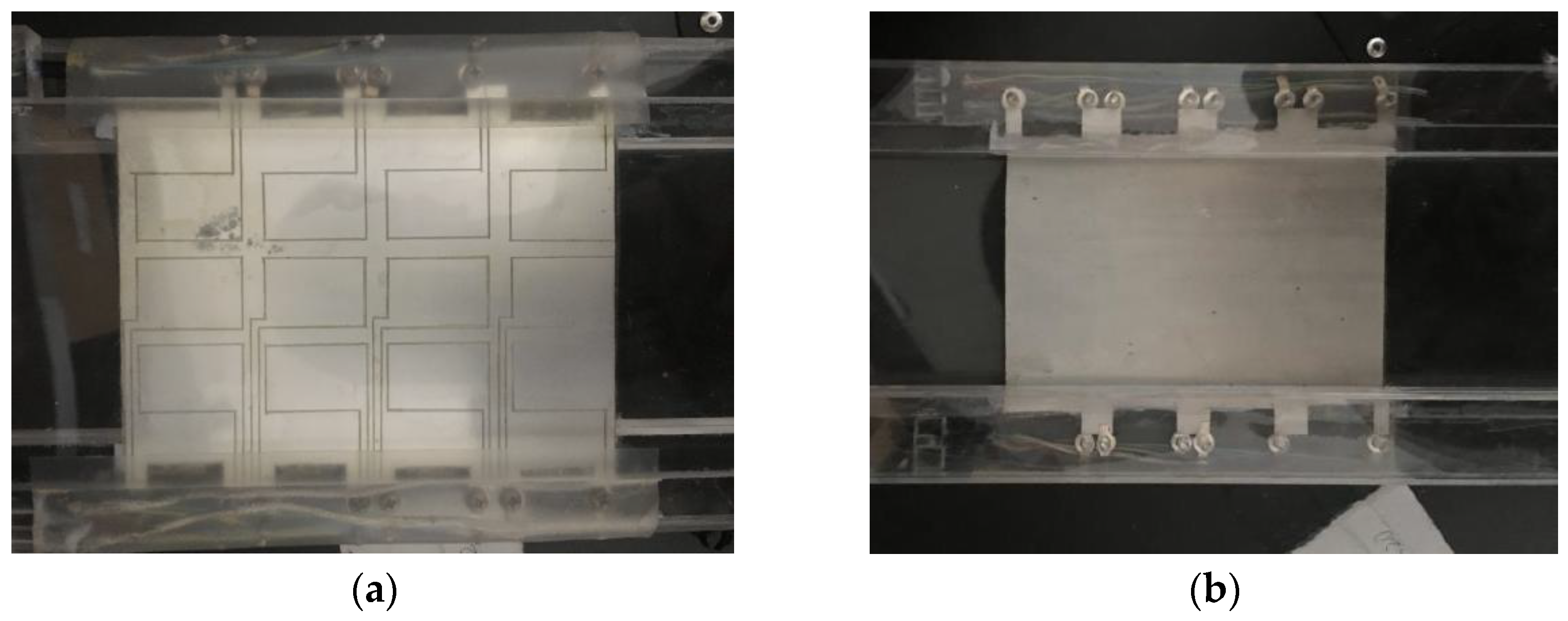
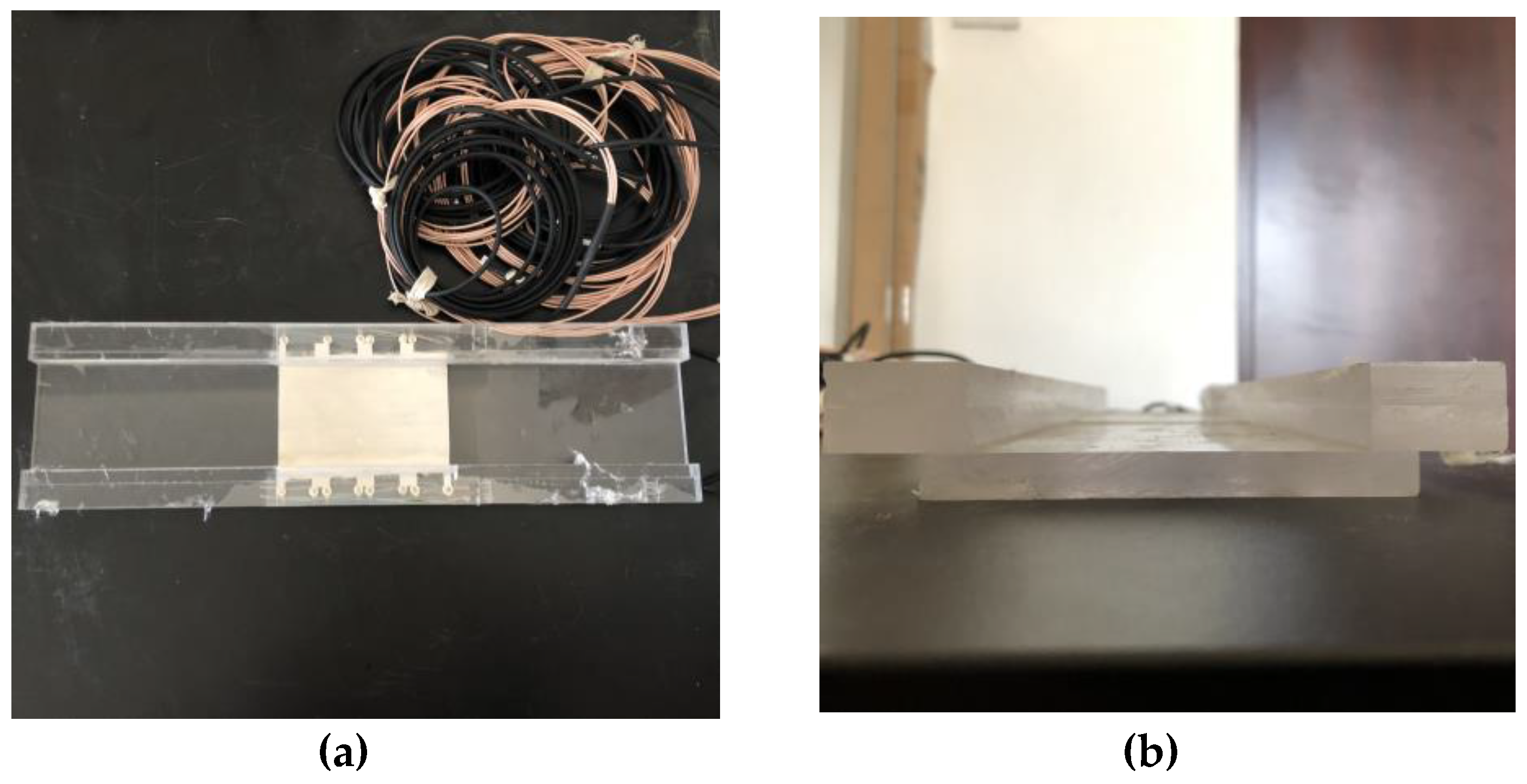
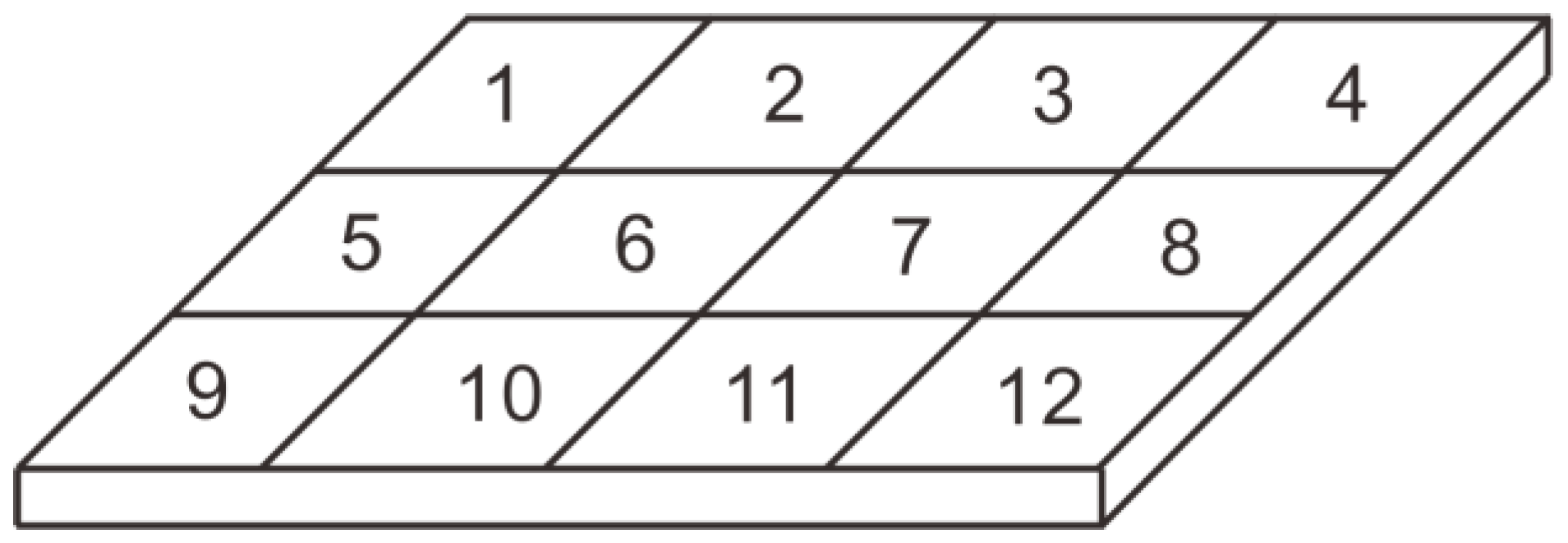
4.1. Production of PVDF Sensor
4.2. Experimental Steps
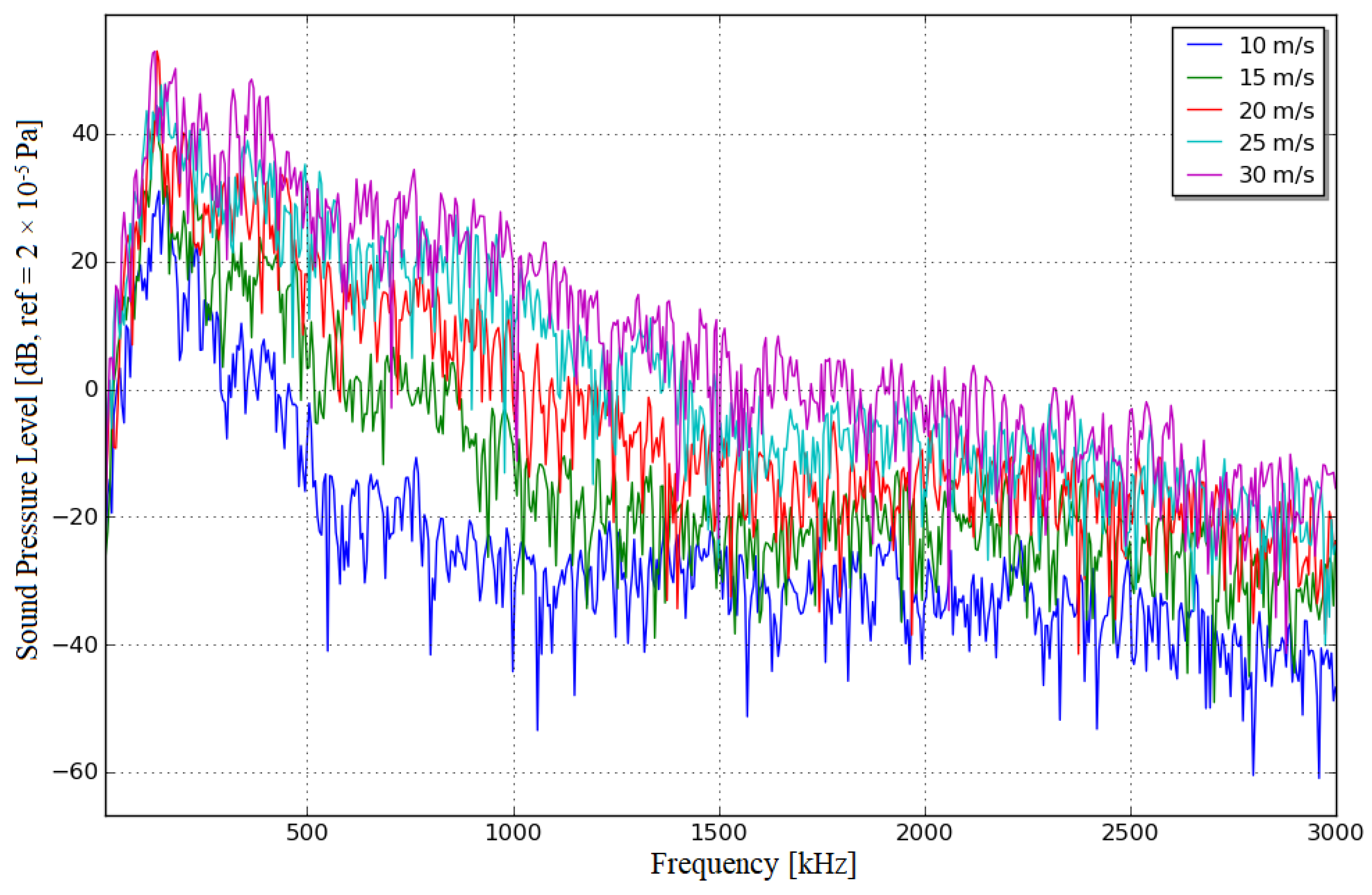
4.3. Experimental Results
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Werkmann, K.H.; Sauerschell, W. Flow Meter Having a Rotary Body. U.S. Patent 4,393,724, 19 June 1983. [Google Scholar]
- Ma, Y. The improvement about transducer and circuit of rotational flow velocity instrument. Autom. Instrum. 1995, 10, 7–8. (In Chinese) [Google Scholar]
- Voulgaris, G.; Trowbridge, J.H. Evaluation of the acoustic Doppler velocimeter (ADV) for turbulence measurements. J. Atmos. Ocean. Technol. 1998, 15, 272–289. [Google Scholar] [CrossRef]
- Lane, S.N.; Biron, P.M.; Bradbrook, K.F.; Butler, J.B.; Chandler, J.H.; Crowell, M.; Mclelland, S.J.; Richards, K. Three-dimensional measurement of river channel flow processes using acoustic Doppler velocimetry. Earth Surf. Process. Landf. J. Br. Geomorph. Group 1998, 23, 1247–1267. [Google Scholar]
- Liu, D. Research on the Key Technology of Acoustic Doppler Current Velocity Measurement; Harbin Engineering University: Harbin, China, 2010. (In Chinese) [Google Scholar]
- Kawai, H. The piezoelectricity of polyvinylidene fluoride. Jpn. J. Appl. Phys. 1969, 8, 975. [Google Scholar]
- Kowbel, W.; Xia, X.; Champion, W.; Withers, J.C.; Wada, B.K. PZT/polymer flexible composites for embedded actuator and sensor applications. SPIE 1999, 3675, 32–43. [Google Scholar]
- van Tol, D.; Hughes, W.J. Underwater PVDF acoustic intensity probe. J. Acoust. Soc. Am. 1993, 93, 2273. [Google Scholar] [CrossRef]
- Lu, Z.; Dorantes-Gonzalez, D.J.; Chen, K.; Yang, F.; Jin, B.; Li, Y.; Chen, Z.; Hu, X. A four-quadrant PVDF transducer for surface acoustic wave detection. Sensors 2012, 12, 16641–16659. [Google Scholar] [CrossRef]
- Yuan, R.; Sui, S. Dynamic response of PVDF thin film under pulse pressure. Instrum. Technol. Sens. 2011, 8, 4–6. (In Chinese) [Google Scholar]
- Ge, H.; He, Z.; Yuan, W. The self-noise response of a large-planar PVDF hydrophone to turbulent boundary layer pressure fluctuation. Acta Acust. 1999, 2, 155–166. (In Chinese) [Google Scholar]
- Wang, Y.C.; Huang, C.H.; Lee, Y.C.; Tsai, H.H. Development of a PVDF sensor array for measurement of the impulsive pressure generated by cavitation bubble collapse. Exp. Fluids 2006, 41, 365–373. [Google Scholar]
- Yang, L.-J.; Hsu, C.-K.; Ho, J.-Y.; Feng, C.-K. Flapping wings with PVDF sensors to modify the aerodynamic forces of a micro aerial vehicle. Sens. Actuators A Phys. 2007, 139, 95–103. [Google Scholar] [CrossRef]
- Tanaka, Y.; Nguyen, D.P.; Fukuda, T.; Sano, A. Wearable skin vibration sensor using a PVDF film. In Proceedings of the 2015 IEEE World Haptics Conference (WHC), Evanston, IL, USA, 22–26 June 2015; pp. 146–151. [Google Scholar]
- Dung, C.; Sasaki, E. Numerical simulation of output response of PVDF sensor attached on a cantilever beam subjected to impact loading. Sensors 2016, 16, 601. [Google Scholar] [CrossRef]
- Li, H. Research on Piezoelectric Intelligent Materials for Real-Time Monitoring of Flow Velocity and Pressure. Master’s Thesis, Harbin Engineering University, Harbin, China, 2011. (In Chinese). [Google Scholar]
- Kline, S.J.; Reynolds, W.C.; Schraub, F.A.; Runstadler, P.W. The structure of turbulent boundary layers. J. Fluid Mech. 1967, 30, 741–773. [Google Scholar] [CrossRef]
- Abu-Ghannam, B.J.; Shaw, R. Natural transition of boundary layers—The effects of turbulence, pressure gradient, and flow history. J. Mech. Eng. Sci. 1980, 22, 213–228. [Google Scholar] [CrossRef]
- Willmarth, W.W. Wall pressure fluctuations in a turbulent boundary layer. J. Acoust. Soc. Am. 1958, 28, 776. [Google Scholar] [CrossRef]
- Ling, F. Vibration and Noise of Submarine Model Excited by TBL Fluctuating Pressure. Master’s Thesis, Dalian University of Technology, Dalian, China, 2013. (In Chinese). [Google Scholar]
- Shang, D.; Li, Q.; Shang, D. Experimental investigation on flow-induced noise of the underwater hydrofoil structure. Acta Acust. 2012, 37, 416–423. (In Chinese) [Google Scholar]
- Abshagen, J.; Kuter, D.; Nejedl, V. Flow-induced interior noise from a turbulent boundary layer of a towed body. Adv. Aircr. Spacecr. Sci. 2016, 3, 259–269. [Google Scholar] [CrossRef]
- Chu, X.; Xu, Y.; Yuan, S.; Wang, L.; Liu, M.; Li, L. Vibration, testing and diagnosis. J. Vib. Meas. Diagn. 2013, 33, 351–356. (In Chinese) [Google Scholar]
- Youn, J.P.; Yong, S.K.; Cheolmin, P. Micropatterning of semicrystalline poly (vinylidene fluoride) solutions. Eur. Polym. J. 2005, 41, 1002–1012. [Google Scholar]
- Yu, M.; Wu, Y.; Pang, Y. A review of progress for hydrodynamic noise of ships. J. Ship Mech. 2007, 11, 152–158. (In Chinese) [Google Scholar]
- Song, H. Study on Noise Mechanism of Underwater Open Airfoil and Nozzle Flow. Master’s Thesis, Harbin Engineering University, Harbin, China, 2015. (In Chinese). [Google Scholar]
- Zhang, Y.; Zhang, T.; Liu, J.; Zhao, W.; Li, Q. Three-dimensional simulation and validation of the flow-induced noise based on Lighthill’s acoustic analogy theory. Ship Sci. Technol. 2014, 36, 55–59. (In Chinese) [Google Scholar]
- Wang, Z.; Zhang, J. Analysis of underwater jet noise prediction method based on large eddy simulation. Noise Vib. Control 2017, 37, 47–51. (In Chinese) [Google Scholar]










| Inlet | Velocity Inlet |
|---|---|
| Outlet | Pressure outlet |
| Pipe Surface | Symmetry |
| PVDF film and others | Non-slip wall |
| Wind Speed (m/s) | 10 | 15 | 20 | 25 | 30 |
|---|---|---|---|---|---|
| Total sound level (dB) | 57.66128 | 66.90901 | 74.39316 | 78.6987 | 83.82377 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Q.; Xing, J.; Shang, D.; Wang, Y. A Flow Velocity Measurement Method Based on a PVDF Piezoelectric Sensor. Sensors 2019, 19, 1657. https://doi.org/10.3390/s19071657
Li Q, Xing J, Shang D, Wang Y. A Flow Velocity Measurement Method Based on a PVDF Piezoelectric Sensor. Sensors. 2019; 19(7):1657. https://doi.org/10.3390/s19071657
Chicago/Turabian StyleLi, Qi, Junhua Xing, Dajing Shang, and Yilin Wang. 2019. "A Flow Velocity Measurement Method Based on a PVDF Piezoelectric Sensor" Sensors 19, no. 7: 1657. https://doi.org/10.3390/s19071657
APA StyleLi, Q., Xing, J., Shang, D., & Wang, Y. (2019). A Flow Velocity Measurement Method Based on a PVDF Piezoelectric Sensor. Sensors, 19(7), 1657. https://doi.org/10.3390/s19071657




