InAs/InAsSb Strain-Balanced Superlattices for Longwave Infrared Detectors
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
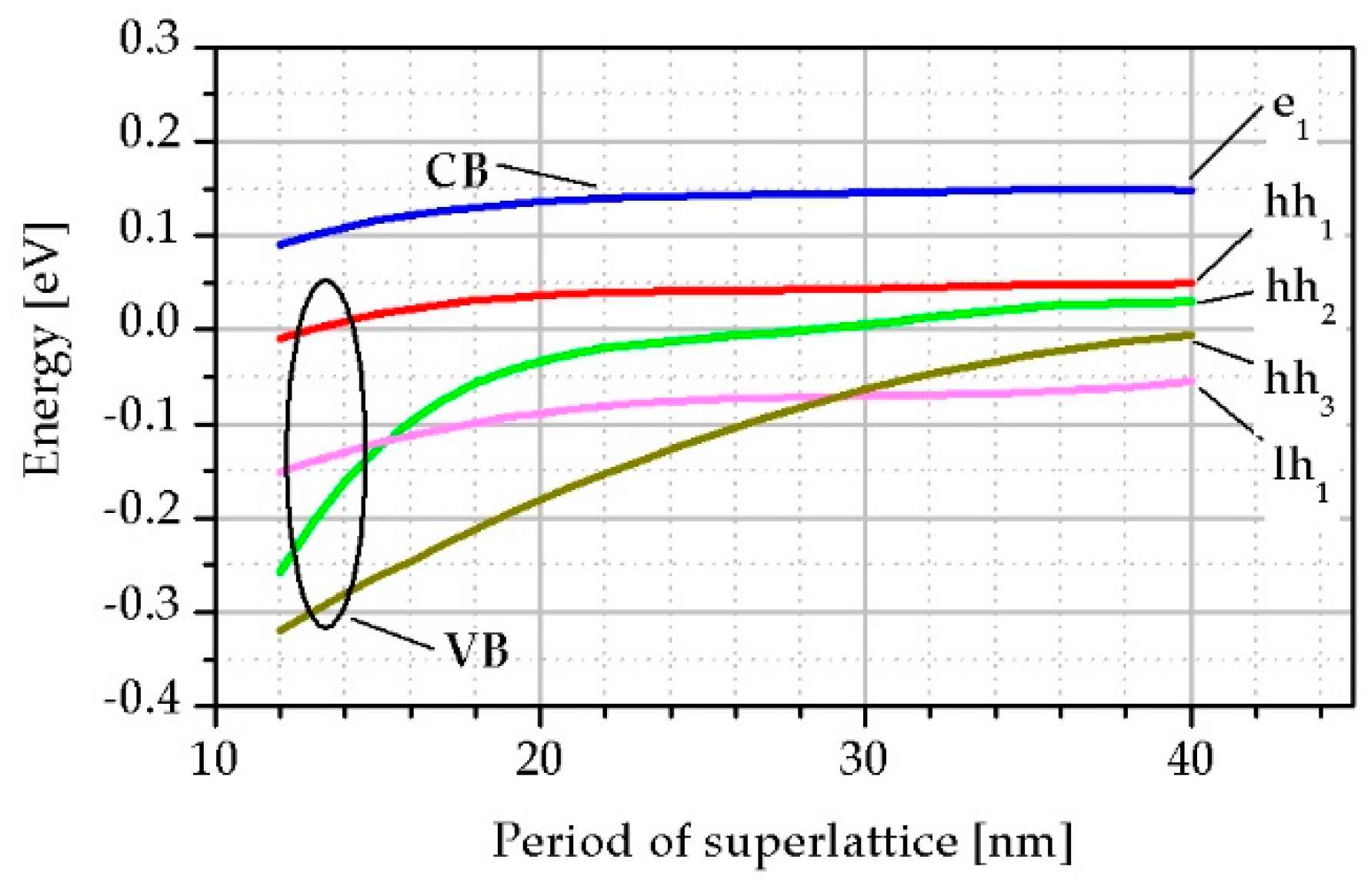
3.1. Influence of the Period on the Superlattice (SL) Parameters
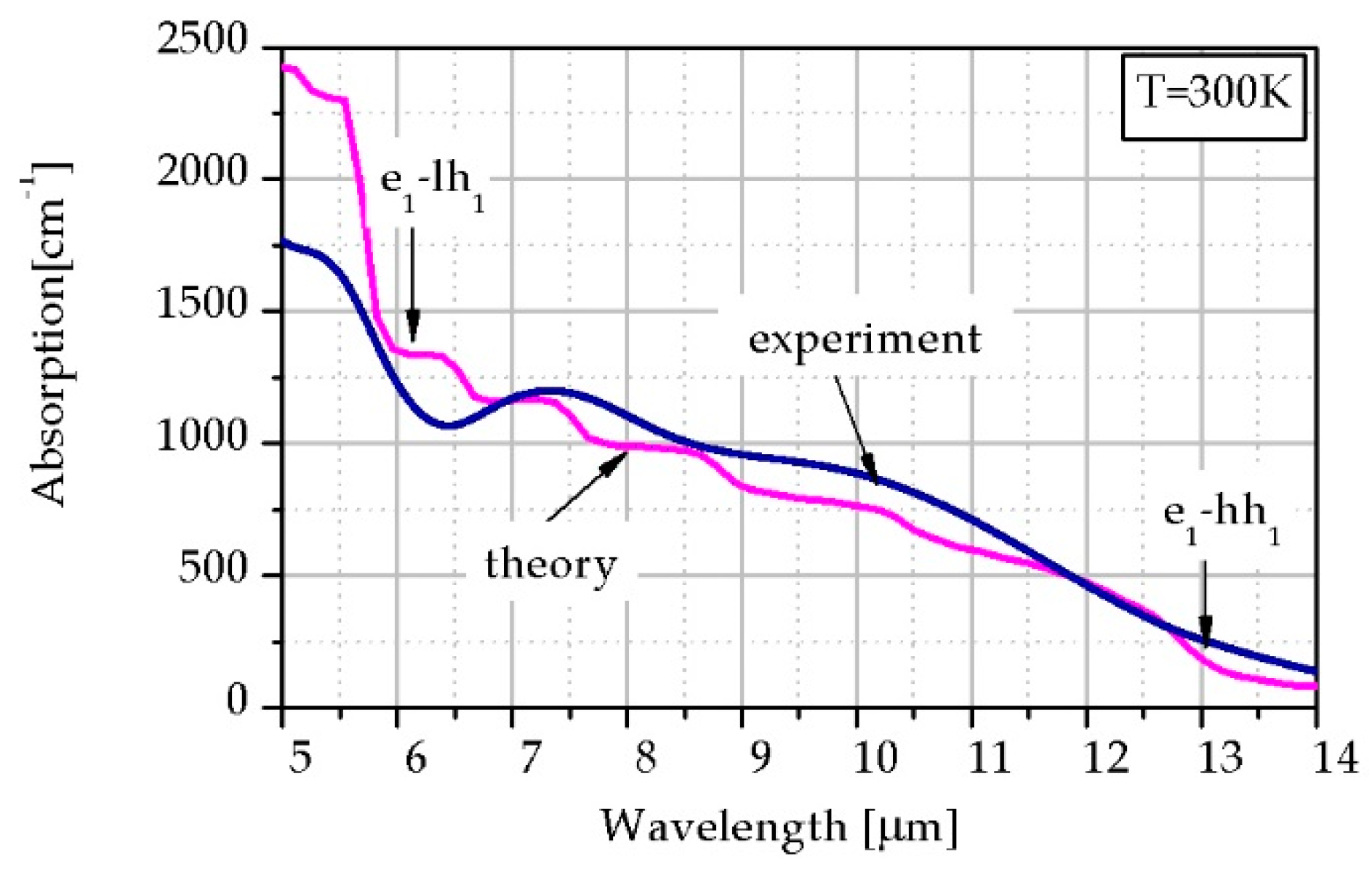
3.2. Comparison of the Theoretical Simulation with the Experimental Data
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Smith, L.D.; Mailhiot, C. Proposal for strained type II superlattice infrared detectors. J. Appl. Phys. 1987, 62, 2545–2548. [Google Scholar] [CrossRef]
- Rogalski, A.; Martyniuk, P.; Kopytko, M. InAs/GaSb type-II superlattice infrared detectors: Future prospect. Appl. Phys. Rev. 2017, 4, 031304. [Google Scholar] [CrossRef]
- Steenbergen, E.H.; Connelly, B.C.; Metcalfe, G.D.; Shen, H.; Wraback, M.; Lubyshev, D.; Qiu, Y.; Fastenau, J.M.; Liu, A.W.K.; Elhamri, S.; et al. Significantly improved minority carrier lifetime observed in a long-wavelength infrared III-V type-II superlattice comprised of InAs/InAsSb. Appl. Phys. Lett. 2011, 99, 251110. [Google Scholar] [CrossRef]
- Rogalski, A.; Kopytko, M.; Martyniuk, P. Antimonide-Based Infrared Detectors: A New Perspective; SPIE Press: Bellingham, WA, USA, 2018. [Google Scholar]
- Webster, P.T.; Riordan, N.A.; Liu, S.; Steenbergen, E.H.; Synowicki, R.A.; Zhang, Y.-H.; Johnson, S.R. Measurement of InAsSb bandgap energy and InAs/InAsSb band edge positions using spectroscopic ellipsometry and photoluminescence spectroscopy. J. Appl. Phys. 2015, 118, 245706. [Google Scholar] [CrossRef]
- Svensson, S.P.; Sarney, W.L.; Hier, H.; Lin, Y.; Wang, D.; Donetsky, D.; Shterengas, L.; Kipshidze, G.; Belenky, G. Band gap of InAs1−xSbx with native lattice constant. Phys. Rev. B 2012, 86, 245205. [Google Scholar] [CrossRef]
- Kim, H.S.; Cellek, O.O.; Lin, Z.-Y.; He, Z.-Y.; Zhao, X.-H.; Liu, S.; Li, H.; Zhang, Y.-H. Long-wave infrared nBn photodetectors based on InAs/InAsSb type-II superlattices. Appl. Phys. Lett. 2012, 101, 161114. [Google Scholar] [CrossRef]
- Haddadi, A.; Chen, G.; Chevallier, R.; Hoang, A.M.; Razeghi, M. InAs/InAs1−xSbx type-II superlattices for high performance long wavelength infrared detection. Appl. Phys. Lett. 2014, 105, 121104. [Google Scholar] [CrossRef]
- Lotfi, H.; Li, L.; Ye, H.; Hinkey, R.T.; Lei, L.; Yang, R.Q.; Keay, J.C.; Mishima, T.D.; Santos, M.B.; Johnson, M.B. Interband cascade infrared photodetectors with long and very-long cutoff wavelengths. Infrared Phys. Technol. 2015, 70, 162–167. [Google Scholar] [CrossRef]
- Lei, L.; Lia, L.; Yea, H.; Lotfia, H.; Yang, R.Q.; Johnsonc, M.B.; Massengalea, J.A.; Mishimab, T.D.; Santosb, M.B. Long-wavelength interband cascade infrared photodetectors towards high temperature operation. In Proceedings of the SPIE OPTO, the Optoelectronics and Photonic Materials and Devices Conference, San Francisco, CA, USA, 28 January–2 February 2017; p. 1011113. [Google Scholar]
- Haddadi, A.; Dehzangi, A.; Chevallier, R.; Adhikary, R.; Razeghi, M. Bias-selectable nBn dual-band long-/very long-wavelength infrared photodetectors based on InAs/InAs1−xSbx/AlAs1−xSbx type-II superlattices. Sci. Rep. 2017, 7, 3379. [Google Scholar] [CrossRef] [PubMed]
- Wojtas, J.; Gluszek, A.; Hudzikowski, A.; Tittel, K.F. Mid-infrared trace gas sensors technology based on intracavity quartz-enhanced photoacustic spectroscopy. Sensors 2017, 17, 513. [Google Scholar] [CrossRef] [PubMed]
- Bielecki, Z.; Stacewicz, T.; Wojtas, J.; Mikołajczyk, J.; Szabra, D.; Prokopiuk, A. Selected optoelectronic sensors in medical applications. Opto-Electron. Rev. 2018, 26, 122–133. [Google Scholar] [CrossRef]
- Lestrade, M.; Li, Z.Q.; Li, Z.S. Finite difference k.p modeling of type II MQWs. Opt. Quantum Electron. 2014, 46, 1345–1352. [Google Scholar] [CrossRef]
- Birner, S. Modeling of semiconductor nanostructures and semiconductor-electrolyte interfaces. Available online: https://inis.iaea.org/search/search.aspx?orig_q=RN:43026032 (accessed on 21 April 2019).
- Vurgaftman, I.; Meyer, J.R.; Ram-Mohan, L.R. Band parameters for III–V compound semiconductors and their alloys. J. Appl. Phys. 2001, 89, 5815–5875. [Google Scholar] [CrossRef]
- Wei, S.-H.; Zunger, A. Calculated natural band offsets of all II–VI and III–V semiconductors: Chemical trends and the role of cation d orbitals. Appl. Phys. Lett. 1998, 72, 2011–2013. [Google Scholar] [CrossRef]
- Yu, P.Y.; Cardona, M. Fundamentals of Semiconductors: Physics and Materials Properties, 4th ed.; Springer: Berlin, Germany, 2010. [Google Scholar]
- Steenbergen, E.H. Strain-balanced InAs-InAsSb Type-II Superlattices on GaSb Substrates for Infrared Photodetector Applications. Ph.D. Thesis, Arizona state university, Tempe, AZ, USA, May 2012. [Google Scholar]
- Harrison, J.W.; Hauser, J.R. Alloy scattering in ternary III-V compounds. Phys. Rev. B 1976, 13, 5347–5350. [Google Scholar] [CrossRef]
- Manyk, T.; Michalczewski, K.; Murawski, K.; Grodecki, K.; Rutkowski, J.; Martyniuk, P. Electronic band structure of InAs/InAsSb type-II superlattice for HOT LWIR detectors. Results Phys. 2018, 11, 1119–1123. [Google Scholar] [CrossRef]
- Lackner, D.; Steger, M.; Thewalt, M.L.W.; Pitts, O.J.; Cherng, Y.T.; Watkins, S.P.; Plis, E.; Krishna, S. InAs/InAsSb strain balanced superlattices for optical detectors: Material properties and energy band simulations. J. Appl. Phys. 2012, 111, 034507. [Google Scholar] [CrossRef]
- Chuang, S.L. Physics of Optoelectronics Devises; Wiley-Interscience publication: Hoboken, NJ, USA, 1995; p. 716. [Google Scholar]
- Dong, H.M.; Li, L.L.; Xu, W.; Han, K. Effect of microscopic interface asymmetry on optical properties of short-period InAs/GaSb type-II superlattices. Thin Solid Film. 2015, 589, 388–395. [Google Scholar] [CrossRef]
- Michalczewski, K.; Kubiszyn, Ł.; Martyniuk, P.; Wu, C.H.; Jureńczyk, J.; Grodecki, K.; Benyahia, D.; Rogalski, A.; Piotrowski, J. Demonstration of HOT LWIR T2SLs InAs/InAsSb photodetectors grown on GaAs substrate. Infrared Phys. Technol. 2018, 95, 222–226. [Google Scholar] [CrossRef]








| Parameter | InAs | InSb | GaSb |
|---|---|---|---|
| E0(Γ) [eV] | 0.417 | 0.235 | 0.812 |
| E0(X) [eV] | 1.433 | 0.63 | 1.141 |
| E0(L) [eV] | 1.133 | 0.93 | 0.875 |
| ∆so [eV] | 0.39 | 0.81 | 0.76 |
| me/m0 (0K) | 0.026 | 0.014 | 0.039 |
| Ev, vac | 1.39 | 1.75 | 1.78 |
| a = f (T) [Å] |
| Bowing Coefficient | Bowing Parameter (bbow) |
|---|---|
| bg [eV] | 0.72 |
| b∆so [eV] | 1.2 |
| bme/m0 | 0.035 |
| bv, vac [eV] | – 0.47 |
| Sample | L [nm] | LInAsSb [nm] | LInAs [nm] | xSb | Eg [eV] | τ [ns] |
|---|---|---|---|---|---|---|
| A | 13.20 | 2.8 | 10.4 | 0.38 | 0.1048 | 24 |
| B | 13.55 | 4.2 | 9.35 | 0.38 | 0.0996 | 1.2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Manyk, T.; Michalczewski, K.; Murawski, K.; Martyniuk, P.; Rutkowski, J. InAs/InAsSb Strain-Balanced Superlattices for Longwave Infrared Detectors. Sensors 2019, 19, 1907. https://doi.org/10.3390/s19081907
Manyk T, Michalczewski K, Murawski K, Martyniuk P, Rutkowski J. InAs/InAsSb Strain-Balanced Superlattices for Longwave Infrared Detectors. Sensors. 2019; 19(8):1907. https://doi.org/10.3390/s19081907
Chicago/Turabian StyleManyk, Tetiana, Krystian Michalczewski, Krzysztof Murawski, Piotr Martyniuk, and Jaroslaw Rutkowski. 2019. "InAs/InAsSb Strain-Balanced Superlattices for Longwave Infrared Detectors" Sensors 19, no. 8: 1907. https://doi.org/10.3390/s19081907
APA StyleManyk, T., Michalczewski, K., Murawski, K., Martyniuk, P., & Rutkowski, J. (2019). InAs/InAsSb Strain-Balanced Superlattices for Longwave Infrared Detectors. Sensors, 19(8), 1907. https://doi.org/10.3390/s19081907





