Numerical Sensitivity Analysis for Dielectric Characterization of Biological Samples by Open-Ended Probe Technique
Abstract
:1. Introduction
2. Materials and Methods
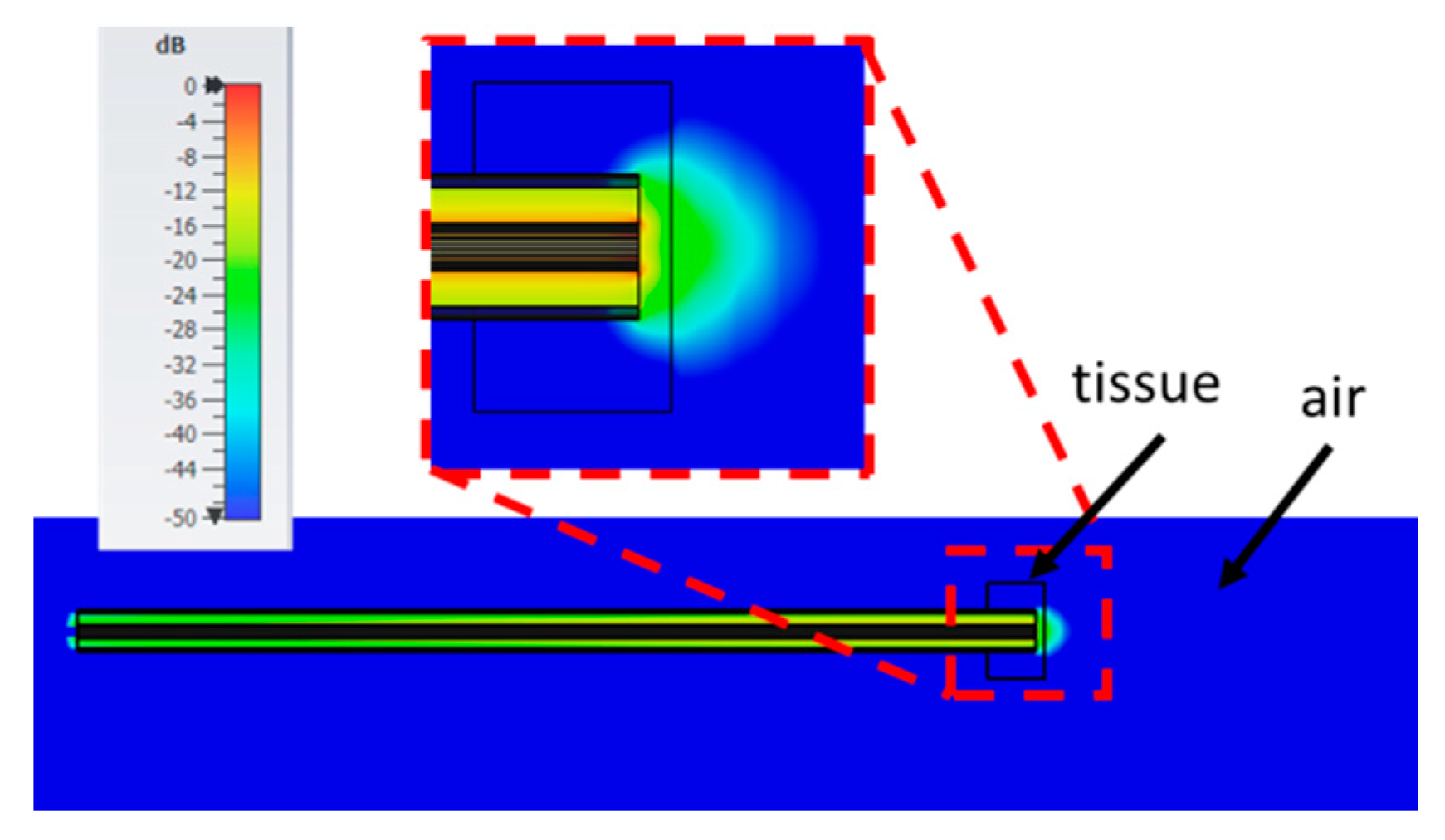
2.1. Numerical Method and Model of the Open-Ended Probe Technique Experimental Set-Up
2.2. Sensitivity Analysis
3. Results
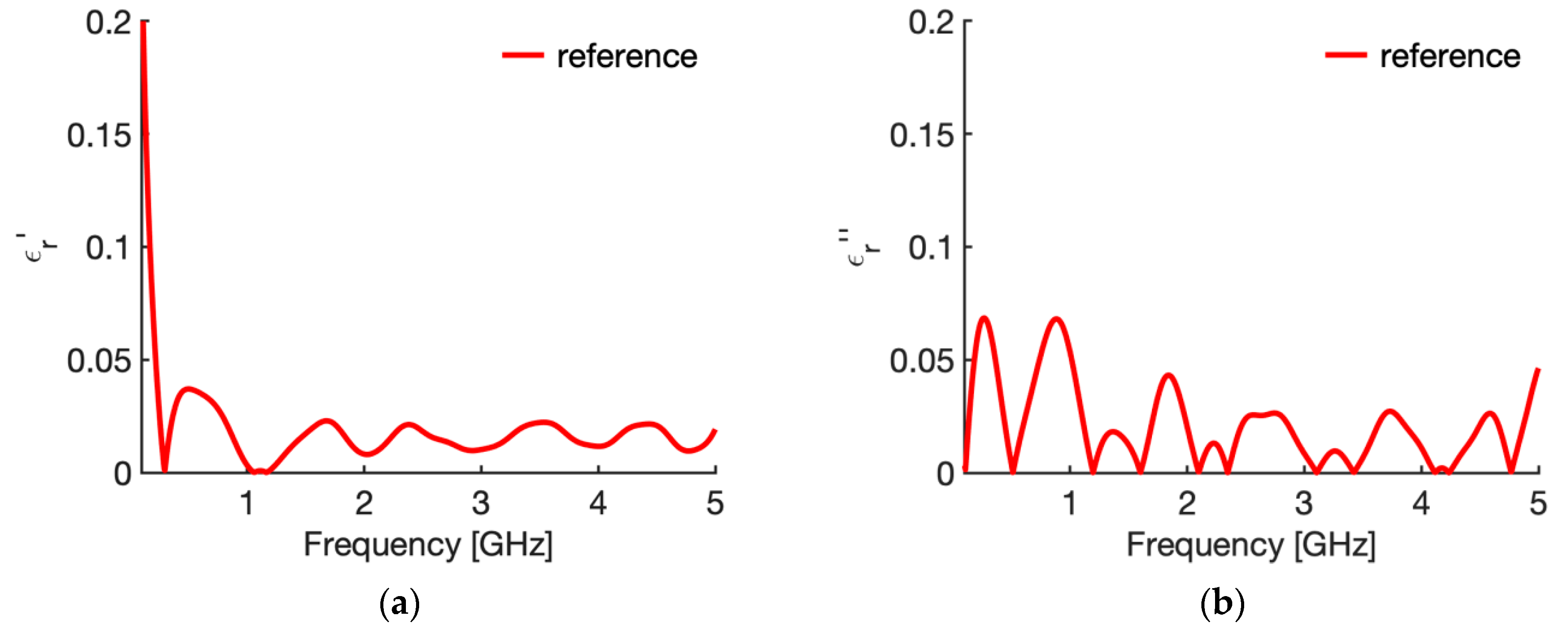
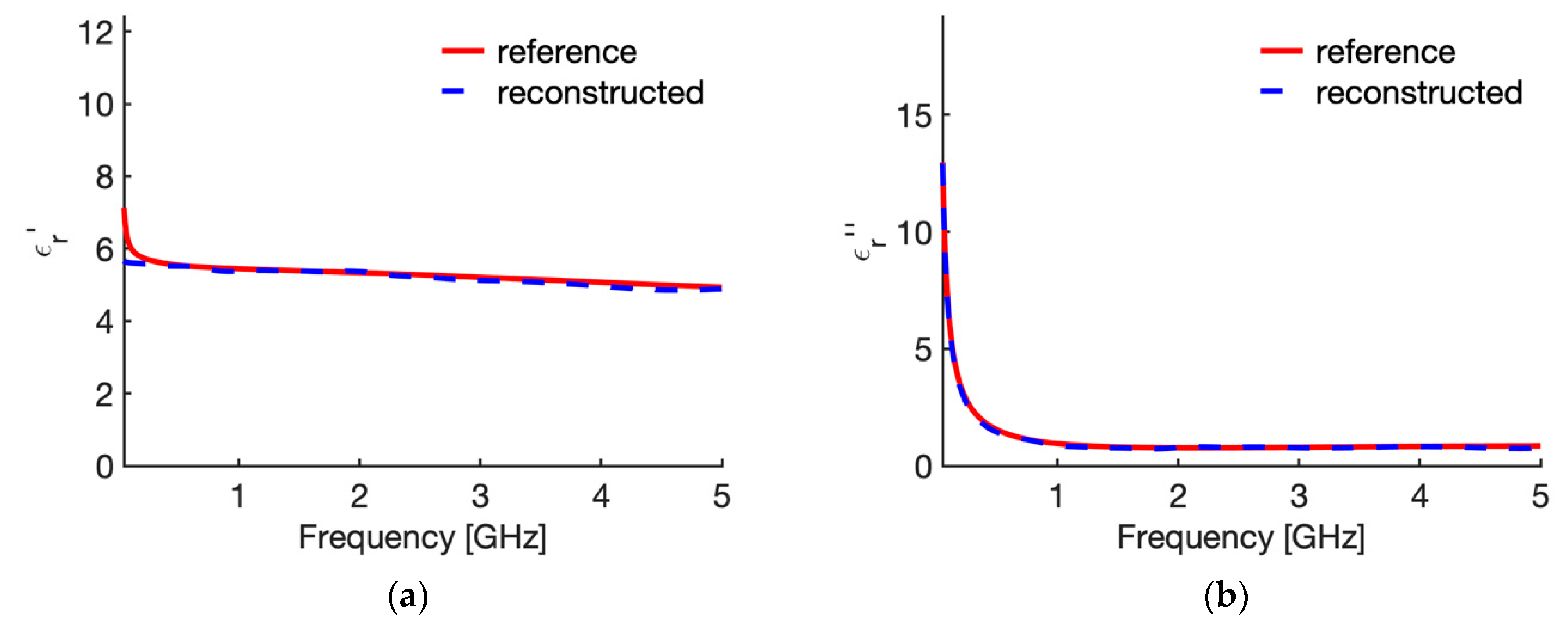
3.1. Reference Condition
3.2. Sensitivity Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Datta, N.R.; Ordóñez, S.G.; Gaipl, U.S.; Paulides, M.M.; Crezee, H.; Gellermann, J.; Marder, D.; Puric, E.; Bodis, S. Local hyperthermia combined with radiotherapy and-/or chemotherapy: Recent advances and promises for the future. Canc. Treat. Rev. 2015, 41, 742–753. [Google Scholar] [CrossRef] [PubMed]
- Lubner, M.G.; Brace, C.L.; Hinshaw, J.L.; Lee, F.T., Jr. Microwave tumor ablation: Mechanism of action, clinical results, and devices. J. Vasc. Interv. Radiol. 2010, 21 (Suppl. 8), S192–S203. [Google Scholar] [CrossRef] [Green Version]
- Brace, C.L. Radiofrequency and microwave ablation of the liver, lung, kidney, and bone: What are the differences? Curr. Probl. Diagn. Radiol. 2009, 38, 135–143. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rubaek, T.; Mohr, J.J. Microwave tomography. In An Introduction to Microwave Imaging for Breast Cancer Detection; Conceição, R.C., Mohr, J.J., O’Halloran, M., Eds.; Springer International Publishing: Cham, Switzerland, 2016; pp. 17–45. [Google Scholar] [CrossRef]
- Chandra, R.; Zhou, H.; Balasingham, I.; Narayanan, R.M. On the Opportunities and Challenges in Microwave Medical Sensing and Imaging. IEEE Trans. Biomed. Eng. 2015, 62, 1667–1682. [Google Scholar] [CrossRef] [PubMed]
- Scapaticci, R.; Lopresto, V.; Pinto, R.; Cavagnaro, M.; Crocco, L. Monitoring Thermal Ablation via Microwave Tomography: An Ex Vivo Experimental Assessment. Diagnostics 2018, 8, 81. [Google Scholar] [CrossRef] [Green Version]
- Lopresto, V.; Pinto, R.; Farina, L.; Cavagnaro, M. Microwave thermal ablation: Effects of tissue properties variations on predictive models for treatment planning. Med. Eng. Phys. 2017, 46, 63–70. [Google Scholar] [CrossRef]
- Kok, H.P.; Kotte, A.N.T.J.; Crezee, J. Planning, optimisation and evaluation of hyperthermia treatments. Int. J. Hyperth. 2017, 33, 593–607. [Google Scholar] [CrossRef] [Green Version]
- Prasad, B.; Kim, S.; Cho, W.; Kim, S.; Kim, J.K. Effect of tumor properties on energy absorption, temperature mapping, and thermal dose in 13.56-MHz radiofrequency hyperthermia. J. Therm. Biol. 2018, 74, 281–289. [Google Scholar] [CrossRef]
- Joines, W. Frequency dependent absorption of electromagnetic energy in biological tissue. IEEE Trans. Biomed. Eng. 1984, 31, 17–20. [Google Scholar] [CrossRef]
- Peyman, A. Dielectric properties of tissues and their applications; state of knowledge. In Proceedings of the 2014 IEEE MTT-S International Microwave Workshop Series on RF and Wireless Technologies for Biomedical and Healthcare Applications (IMWS-Bio2014), London, UK, 8–10 December 2014. [Google Scholar] [CrossRef]
- Kraszewski, A.; Stuchly, M.; Stuchly, S. ANA calibration method for measurements of dielectric properties. IEEE Trans. Instrum. Meas. 1983, 32, 385–387. [Google Scholar] [CrossRef]
- Gabriel, C.; Gabriel, S.; Corthout, E. The dielectric properties of biological tissues: I. Literature survey. Phys. Med. Biol. 1996, 41, 2231–2249. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gabriel, S.; Lau, R.W.; Gabriel, C. The dielectric properties of biological tissues: II. Measurements in the frequency range 10 Hz to 20 GHz. Phys. Med. Biol. 1996, 41, 2251–2269. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peyman, A.; Holden, S.; Gabriel, C. Dielectric properties of tissues at microwave frequencies, Mobile Telecommunications and Health Research Programme. Final Rep. Rum 2005, 3, 1–46. [Google Scholar]
- Gabriel, C. Compilation of the Dielectric Properties of Body Tissues at RF and Microwave Frequencies U.S. Air Force Report AFOSR-TR-96. 1996. Available online: https://www.fcc.gov/general/body-tissue-dielectric-parameters (accessed on 22 April 2020).
- Andreuccetti, D. An Internet Resource for the Calculation of the Dielectric Properties of Body Tissues in the Frequency Range 10 Hz–100 GHz. 2012. Available online: http://niremf.ifac.cnr.it/tissprop/ (accessed on 22 April 2020).
- Hasgall, P.A.; Di Gennaro, F.; Baumgartner, C.; Neufeld, E.; Lloyd, B.; Gosselin, M.C.; Payne, D.; Klingenböck, A.; Kuster, N. IT’IS Database for Thermal and Electromagnetic Parameters of Biological Tissues, Version 4.0. 2018. Available online: https://itis.swiss/virtual-population/tissue-properties/database/dielectric-properties/ (accessed on 22 April 2020). [CrossRef]
- Brady, M.M.; Symons, S.A.; Stuchly, S.S. Dielectric behaviour of selected animal tissues in vivo at frequencies from 2 to 4 GHz. IEEE Trans. Biomed. Eng. 1981, 28, 305–307. [Google Scholar] [CrossRef]
- Farrugia, L.; Wismayer, P.S.; Mangion, L.Z.; Sammut, C.V. Accurate in vivo dielectric properties of liver from 500 MHz to 40 GHz and their correlation to ex vivo measurements. Electromagn. Biol. Med. 2016, 35, 365–373. [Google Scholar] [CrossRef]
- Lopresto, V.; Pinto, R.; Lovisolo, G.A.; Cavagnaro, M. Changes in the dielectric properties of ex vivo bovine liver during microwave thermal ablation at 2.45 GHz. Phys. Med. Biol. 2012, 57, 2309–2327. [Google Scholar] [CrossRef]
- Meaney, P.M.; Gregory, A.P.; Seppälä, J.; Lahtinen, T. Open-Ended Coaxial Dielectric Probe Effective Penetration Depth Determination. IEEE Trans. Microw. Theory Tech. 2016, 64, 915–923. [Google Scholar] [CrossRef] [Green Version]
- La Gioia, A.; Porter, E.; Salahuddin, S.; O’Halloran, M. Impact of Radial Heterogeneities of Biological Tissues on Dielectric Measurements. In Proceedings of the International Conference on Electromagnetics in Advanced Applications (ICEAA), Verona, Italy, 11–15 September 2017. [Google Scholar] [CrossRef]
- Weir, W.B. Automatic measurement of complex dielectric constant and permeability at microwave frequencies. Proc. IEEE 1974, 62, 33–36. [Google Scholar] [CrossRef]
- Campbell, A. Measurements and Analysis of the Microwave Dielectric Properties of Tissues. Ph.D. Thesis, University of Glasgow, Glasgow, UK, October 1990. [Google Scholar]
- Karki, B.; Wi, H.; McEwan, A.; Kwon, H.; Oh, T.I.; Woo, E.J.; Seo, J.K. Evaluation of a Multi-Electrode Bioimpedance Spectroscopy Tensor Probe to Detect the Anisotropic Conductivity Spectra of Biological Tissues. Meas. Sci. Techno. 2014, 25, 075702. [Google Scholar] [CrossRef]
- Lazebnik, M.; McCartney, L.; Popovic, D.; Watkins, C.B.; Lindstrom, M.J.; Harter, J.; Sewall, S.; Magliocco, A.; Booske, J.H.; Okoniewski, M.; et al. A Large-Scale Study of the Ultrawideband Microwave Dielectric Properties of Normal Breast Tissue Obtained from Reduction Surgeries. Phys. Med. Biol. 2007, 52, 2637–2656. [Google Scholar] [CrossRef] [Green Version]
- Sugitani, T.; Kubota, S.; Kuroki, S.; Sogo, K.; Arihiro, K.; Okada, M.; Kadoya, T.; Hide, M.; Oda, M.; Kikkawa, T. Complex Permittivities of Breast Tumor Tissues Obtained from Cancer Surgeries. Appl. Phys. Lett. 2014, 104, 253702. [Google Scholar] [CrossRef]
- Belhadj-Tahar, N.; Fourrier-Lamer, A. Broad-Band Analysis of a Coaxial Discontinuity Used for Dielectric Measurements. IEEE Trans. Microw. Theory Tech. 1986, 34, 346–350. [Google Scholar] [CrossRef]
- De Langhe, P.; Martens, L.; De Zutter, D. Design rules for an experimental setup using an open-ended coaxial probe based on theoretical modelling. IEEE Trans. Instrum. Meas. 1994, 43, 810–817. [Google Scholar] [CrossRef]
- Gregory, A.P.; Clarke, R.N. Dielectric metrology with coaxial sensors. Meas. Sci. Technol. 2007, 18, 1372–1386. [Google Scholar] [CrossRef]
- Marais, J.I.F. A Permittivity Measurement System for High Frequency. Master’s thesis, Laboratories. Thesis for the Master of Science in Engineering. University of Stellenbosh, South Africa, 2006. Available online: https://core.ac.uk/download/pdf/37319818.pdf (accessed on 14 June 2020).
- Agilent 85070E Dielectric Probe Kit. Available online: http://na.support.keysight.com/materials/help/85070.pdf (accessed on 23 February 2020).
- Keysight N1501A Dielectric Probe Kit 10 MHz to 50 GHz, Technical Overview. Available online: https://www.keysight.com/it/en/assets/7018-04631/technical-overviews/5992-0264.pdf (accessed on 18 May 2020).
- Stuchly, M.; Stuchly, S. Coaxial line reflection method for measuring dielectric properties of biological substances at radio and microwave frequencies—A Review. IEEE Trans. Instrum. Meas. 1980, 29, 176–182. [Google Scholar] [CrossRef]
- Reinoso, R.F.; Telfer, B.A.; Rowland, M. Tissue Water Content in Rats Measured by Desiccation. J. Pharmacol. Toxicol. Meth. 1997, 38, 87–92. [Google Scholar] [CrossRef]
- Marklein, R. The Finite Integration Technique as a General Tool to Compute Acoustic, Electromagnetic, Elastodynamic, and Coupled Wave Fields. In Review of Radio Science: 1999–2002; Ross Stone, W., Ed.; John Wiley and Sons: Hoboken, NJ, USA, 2002; pp. 201–244. ISBN 978-0471268666. [Google Scholar]
- Electromagnetic Simulation Solvers, CST Studio Suite. Available online: https://www.3ds.com/products-services/simulia/products/cst-studio-suite/solvers/ (accessed on 18 May 2020).
- Marsland, T.; Evans, S. Dielectric measurements with an openended coaxial probe. IEE Proc. H 1987, 134, 341–3490. [Google Scholar] [CrossRef]
- Ruvio, G.; Vaselli, M.; Lopresto, V.; Pinto, R.; Farina, L.; Cavagnaro, M. Comparison of different methods for dielectric properties measurements in liquid sample media. Int. J. RF Microw. Comput. Aided Eng. 2018, 28, e21215. [Google Scholar] [CrossRef]
- Piuzzi, E.; Merla, C.; Cannazza, G.; Zambotti, A.; Apollonio, F.; Cataldo, A.; D’Atanasio, A.; De Benedetto, E.; Liberti, M. A comparative analysis between customized and commercial systems for complex permittivity measurements on liquid samples at microwave frequencies. IEEE Trans. Instrum. Meas. 2013, 62, 1034–1045. [Google Scholar] [CrossRef]
- Nyshadham, A.; Sibbald, C.; Stuchly, S. Permittivity measurements using open-ended sensors and reference liquid calibration—An uncertainty analysis. IEEE Trans. Microw. Theory Tech. 1992, 40, 305–314. [Google Scholar] [CrossRef]
- Popovic, D.; McCartney, L.; Beasley, C.; Lazebnik, M.; Okoniewski, M.; Hagness, S.C.; Booske, J.H. Precision open-ended coaxial probes for in vivo and ex vivo dielectric spectroscopy of biological tissues at microwave frequencies. IEEE Trans. Microw. Theory Tech. 2005, 53, 1713–1722. [Google Scholar] [CrossRef] [Green Version]
- Shahzad, A.; Khan, S.; Jones, M.; MDwyer, R.; O’Halloran, M. Investigation of the effect of dehydration on tissue dielectric properties in ex vivo measurements. Biomed. Phys. Eng. Express (BPEX) 2017, 3, 045001. [Google Scholar] [CrossRef] [Green Version]
- Ley, S.; Schilling, S.; Fiser, O.; Vrba, J.; Sachs, J.; Helbig, M. Ultra-Wideband Temperature Dependent Dielectric Spectroscopy of Porcine Tissue and Blood in the Microwave Frequency Range. Sensors 2019, 19, 1707. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gabriel, C.; Chan, T.Y.A.; Grant, E.H. Admittance models for open ended coaxial probes and their place in dielectric spectroscopy. Phys. Med. Biol. 1994, 39, 2183–2200. [Google Scholar] [CrossRef] [PubMed]
- Martellosio, A.; Pasian, M.; Bozzi, M.; Perregrini, L.; Mazzanti, A.; Svelto, F.; Summers, P.E.; Renne, G.; Preda, L.; Bellomi, M. Dielectric properties characterization from 0.5 to 50 GHz of breast cancer tissues. IEEE Trans. Microw. Theory Tech. 2017, 65, 998–1011. [Google Scholar] [CrossRef]
- Peyman, A.; Kos, B.; Djokić, M.; Trotovšek, B.; Limbaeck-Stokin, C.; Serša, G.; Miklavčič, D. Variation in dielectric properties due to pathological changes in human liver. Bioelectromagnetics 2015, 36, 603–612. [Google Scholar] [CrossRef]
- Gabriel, C.; Peyman, A. Dielectric measurement: Error analysis and assessment of uncertainty. Phys. Med. Biol. 2007, 51, 6033–6046. [Google Scholar] [CrossRef]
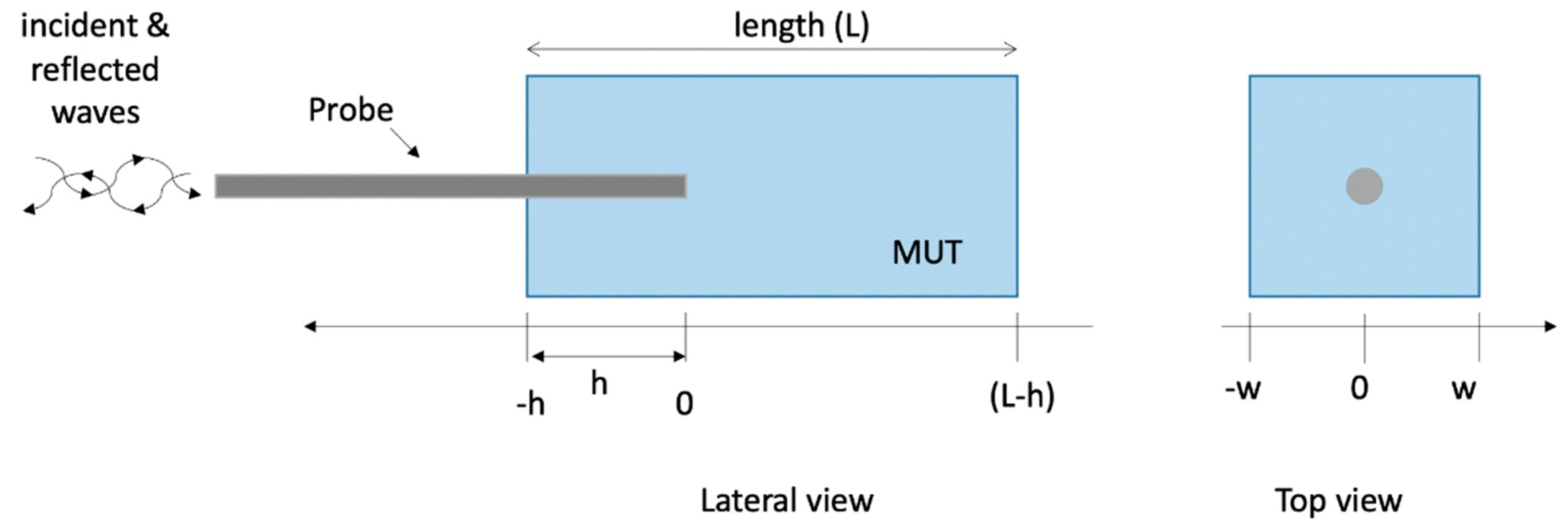
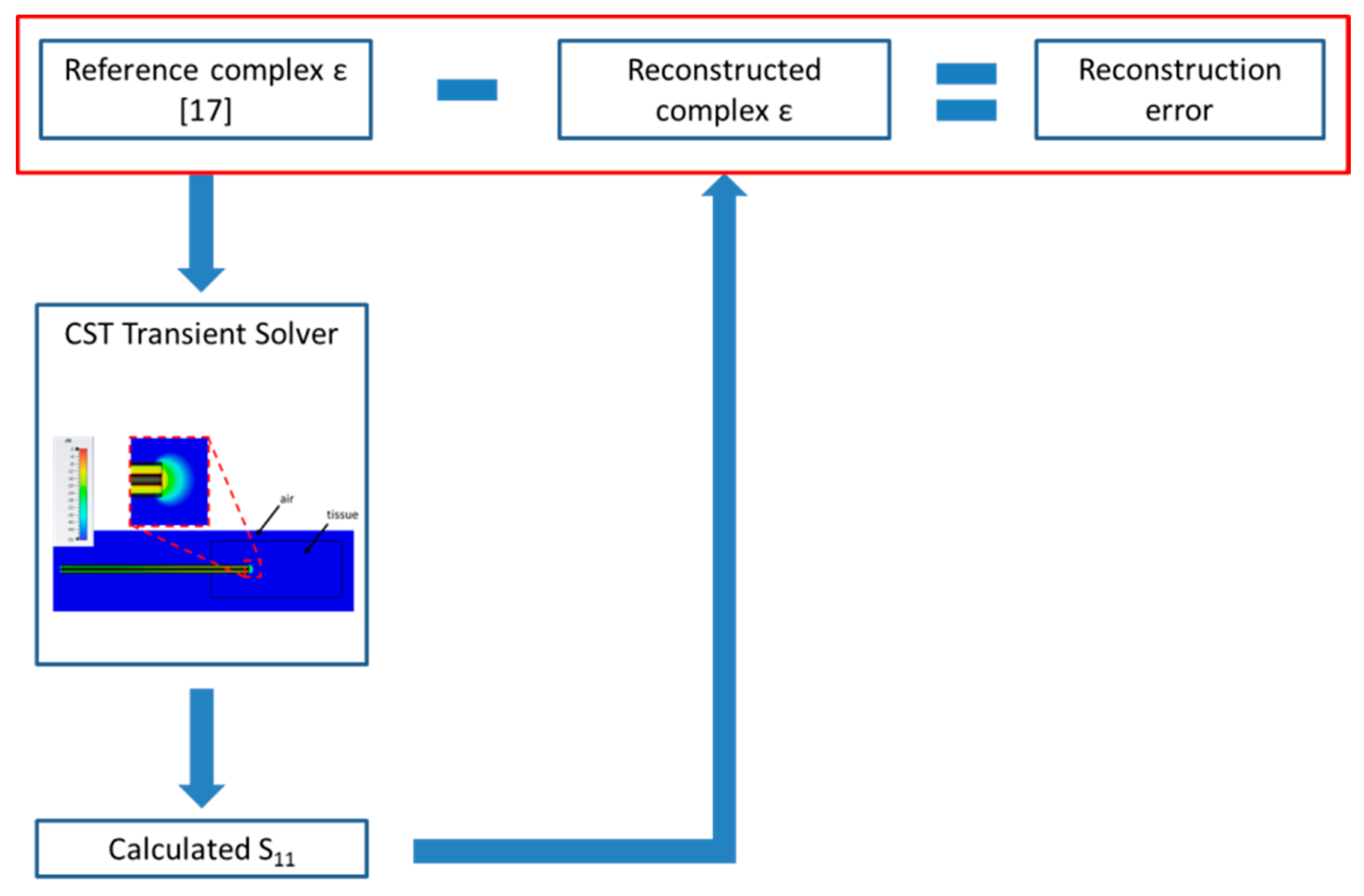


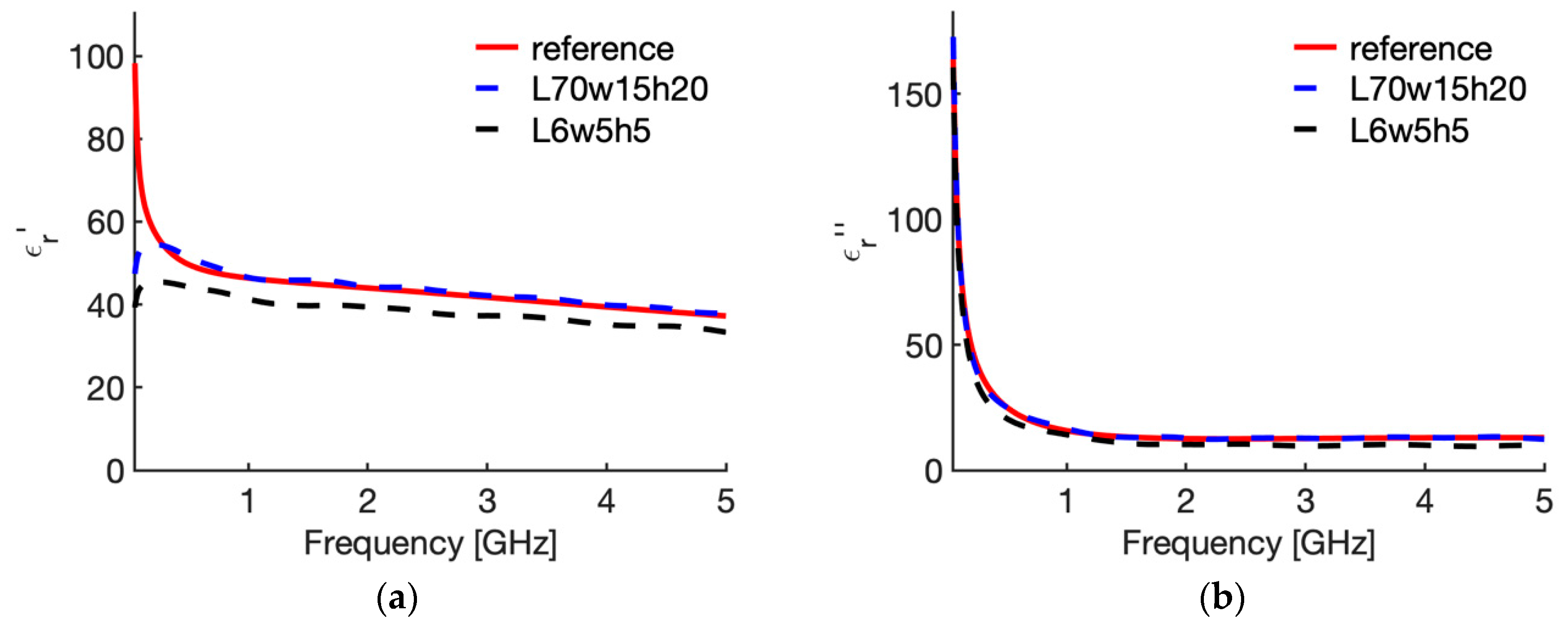
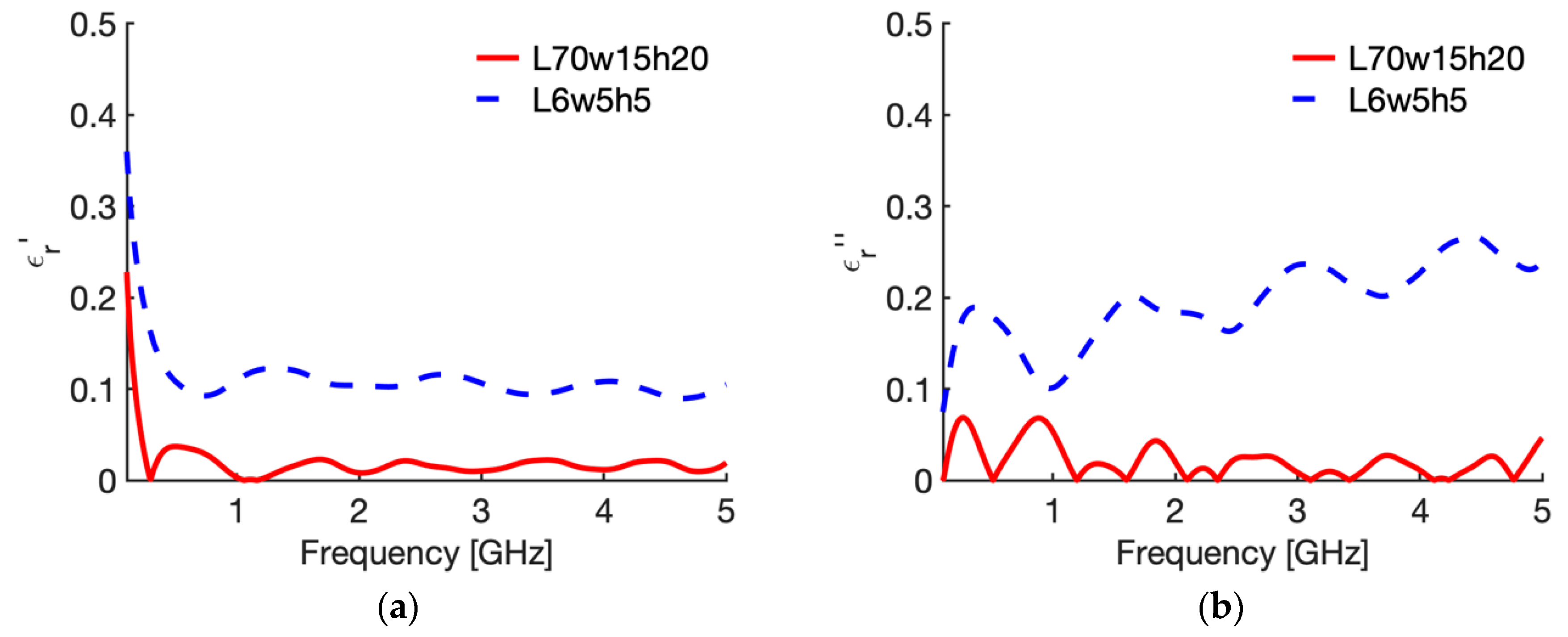
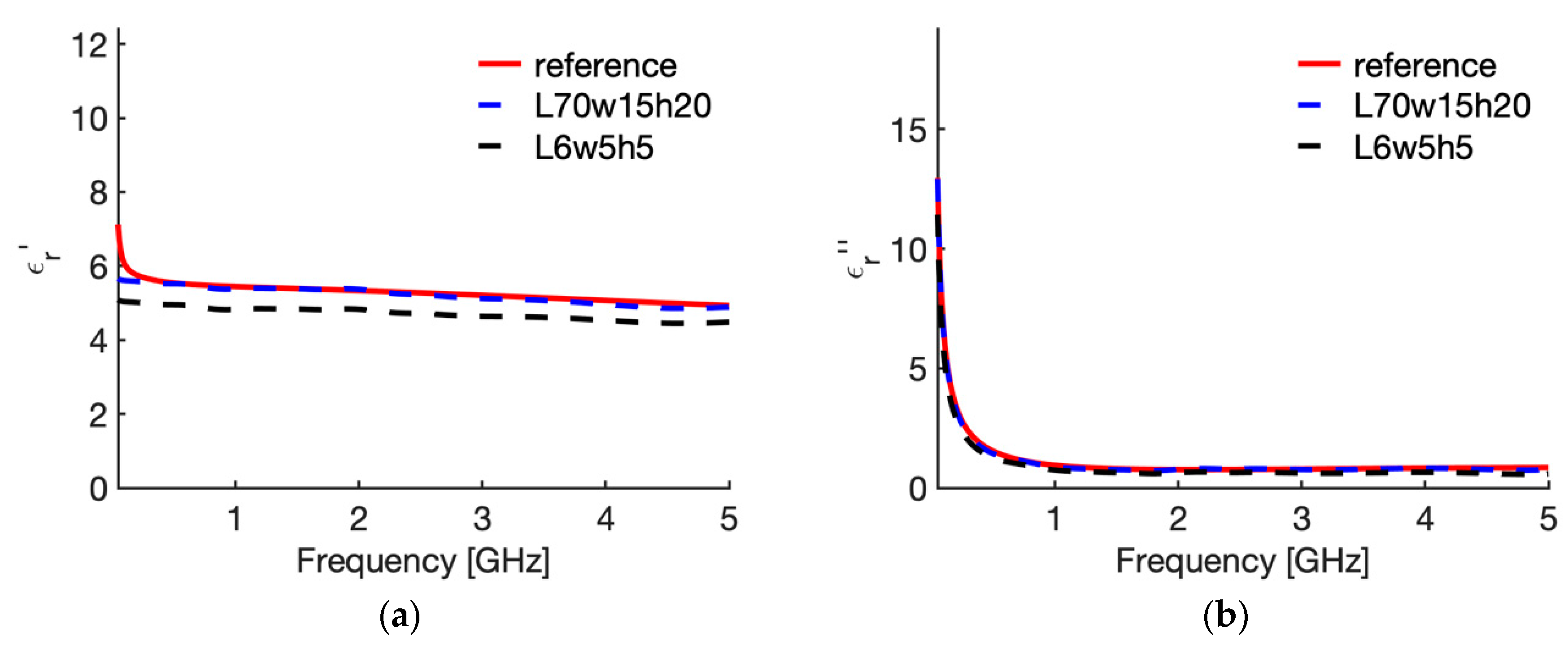
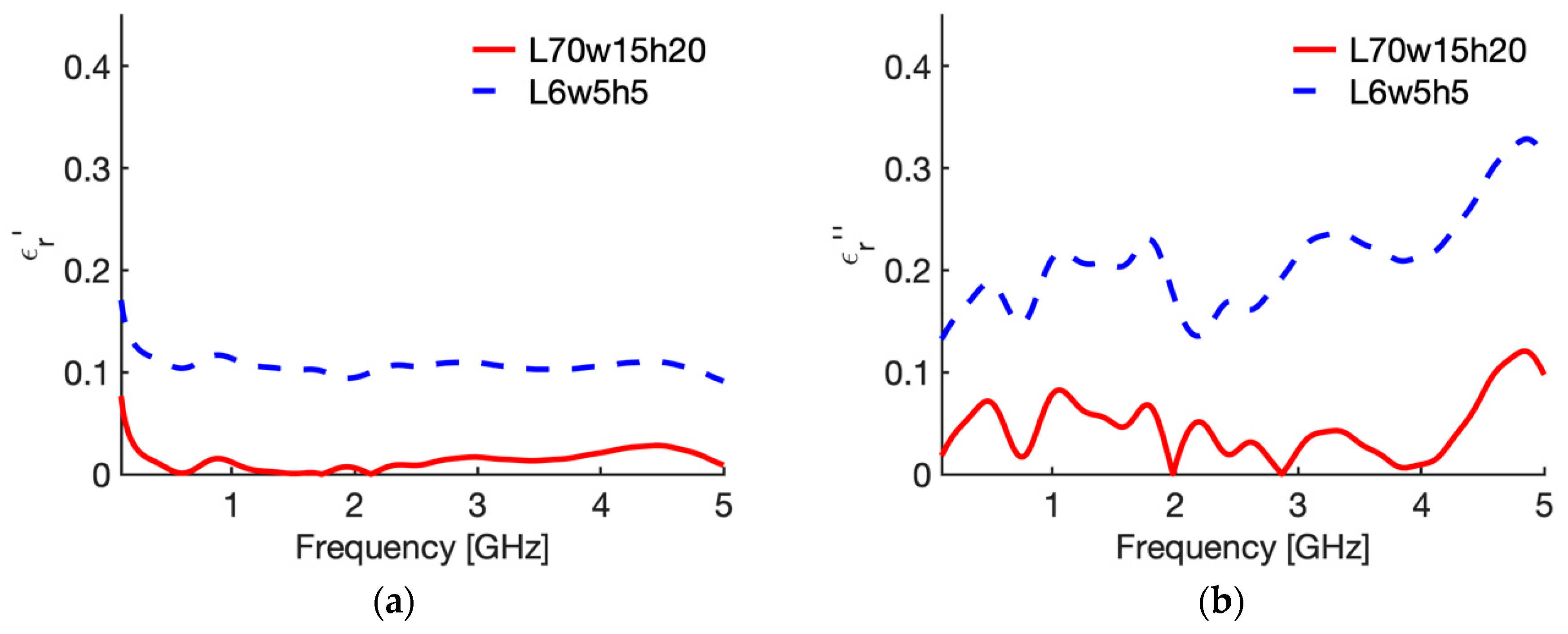

| N | Case | Height (L mm) | Width (w, mm) | Insertion Depth (h, mm) | Backing |
|---|---|---|---|---|---|
| 1 | reference | 70 | 15 | 20 | air |
| 2 | shorter | 25 | 15 | 20 | air |
| 3 | narrow | 70 | 5 | 20 | air |
| 4 | 0 insertion | 70 | 15 | 0 | air |
| 5 | shorter + narrow + 0 ns. | 25 | 5 | 0 | air |
| 6 | influence of the height (narrow, small insertion depth) | 10 | 5 | 5 | air |
| 7 | 9 | 5 | 5 | air | |
| 8 | 8 | 5 | 5 | air | |
| 9 | 7 | 5 | 5 | air | |
| 10 | 6 | 5 | 5 | air | |
| 11 | influence of the width (short, small insertion depth) | 10 | 4 | 5 | air |
| 12 | 10 | 3 | 5 | air | |
| 13 | 10 | 2 | 5 | air | |
| 14 | influence of the insertion depth (short, narrow) | 9 | 5 | 4 | air |
| 15 | 8 | 5 | 3 | air | |
| 16 | 7 | 5 | 2 | air | |
| 17 | 6 | 5 | 1 | air | |
| 18 | 5 | 5 | 0 | air | |
| 19 | influence of the support | 10 | 5 | 5 | PEC 1 |
| 20 | 8 | 5 | 3 | PEC 1 |
| N | Case Name (L; w; h; Backing) | Average (st. dev) | Average (st. dev) |
|---|---|---|---|
| 1 | reference (70; 15; 20; air) | 1.9 (2.0) | 2.1 (1.7) |
| 2 | shorter (25; 15; 20; air) | 2.1 (2.1) | 2.4 (1.8) |
| 3 | narrow (70; 5; 20; air) | 2.6 (2.5) | 3.3 (2.5) |
| 4 | 0 insertion (70; 15; 0; air) | 2.8 (2.2) | 9.4 (3.9) |
| 5 | s + n + 0 ins (25; 5; 0; air) | 2.9 (2.7) | 10.3 (3.7) |
| 6 | height (10; 5; 5; air) | 2.8 (2.8) | 4.8 (2.9) |
| 7 | height (9; 5; 5; air) | 2.8 (2.8) | 4.6 (2.7) |
| 8 | height (8; 5; 5; air) | 2.5 (2.8) | 4.3 (2.7) |
| 9 | height (7; 5; 5; air) | 1.7 (3.0) | 6.8 (3.1) |
| 10 | height (6; 5; 5; air) | 11.1 (3.0) | 19.6 (4.3) |
| 11 | width (10; 4; 5; air) | 2.9 (2.9) | 4.9 (2.6) |
| 12 | width (10; 3; 5; air) | 1.8 (3.0) | 9.0 (3.7) |
| 13 | width (10; 2; 5; air) | 8.8 (3.1) | 17.6 (4.2) |
| 14 | insertion (9; 5; 4; air) | 3.1 (2.9) | 5.2 (3.9) |
| 15 | insertion (8; 5; 3; air) | 3.4 (2.9) | 5.0 (3.2) |
| 16 | insertion (7; 5; 2; air) | 3.2 (3.0) | 4.7 (2.3) |
| 17 | insertion (6; 5; 1; air) | 2.5 (3.0) | 6.1 (2.6) |
| 18 | insertion (5; 5; 0; air) | 3.0 (3.3) | 10.7 (3.4) |
| 19 | support (10; 5; 5; PEC) | 1.9 (2.6) | 4.6 (2.8) |
| 20 | support (8; 5; 3; PEC) | 2.1 (2.9) | 4.9 (3.3) |
| N | Case Name (L; w; h; Backing) | Average (st. dev) | Average (st. dev) |
|---|---|---|---|
| 1 | reference (70; 15; 20; air) | 1.3 (1.0) | 4.6 (2.9) |
| 2 | shorter (25; 15; 20; air) | 1.5 (1.0) | 5.2 (3.5) |
| 3 | narrow (70; 5; 20; air) | 1.8 (1.0) | 6.5 (3.8) |
| 4 | 0 insertion (70; 15; 0; air) | 2.8 (1.0) | 4.6 (3.5) |
| 5 | s + n + 0 ins (25; 5; 0; air) | 3.0 (1.0) | 5.6 (3.6) |
| 6 | height (10; 5; 5; air) | 1.6 (0.9) | 8.1 (4.5) |
| 7 | height (9; 5; 5; air) | 0.9 (0.7) | 8.5 (4.6) |
| 8 | height (8; 5; 5; air) | 2.0 (0.9) | 9.2 (4.7) |
| 9 | height (7; 5; 5; air) | 3.4 (0.9) | 11.4 (4.8) |
| 10 | height (6; 5; 5; air) | 10.7 (0.8) | 21.0 (4.7) |
| 11 | width (10; 4; 5; air) | 2.0 (0.9) | 8.6 (4.5) |
| 12 | width (10; 3; 5; air) | 2.8 (0.9) | 9.4 (4.4) |
| 13 | width (10; 2; 5; air) | 6.6 (1.1) | 12.9 (3.4) |
| 14 | insertion (9; 5; 4; air) | 1.6 (0.9) | 8.1 (4.5) |
| 15 | insertion (8; 5; 3; air) | 1.7 (0.9) | 8.1 (4.6) |
| 16 | insertion (7; 5; 2; air) | 1.9 (0.9) | 8.0 (4.5) |
| 17 | insertion (6; 5; 1; air) | 2.1 (0.8) | 7.7 (3.7) |
| 18 | insertion (5; 5; 0; air) | 3.1 (1.0) | 6.9 (3.4) |
| 19 | support (10; 5; 5; PEC) | 1.2 (0.7) | 6.2 (3.4) |
| 20 | support (8; 5; 3; PEC) | 1.3 (0.7) | 6.2 (3.8) |
| N | Case L; w; h; Backing | Average (st. dev) | Average (st. dev) |
|---|---|---|---|
| 1 | 70; 10; 20; air | 0.8 (0.8) | 4.5 (3.3) |
| 2 | 30; 15; 20; air | 0.9 (0.8) | 4.7 (2.9) |
| 3 | 30; 10; 20; air | 0.9 (0.8) | 4.6 (3.3) |
| 4 | 10; 10; 0; air | 2.8 (1.2) | 6.1 (4.7) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cavagnaro, M.; Ruvio, G. Numerical Sensitivity Analysis for Dielectric Characterization of Biological Samples by Open-Ended Probe Technique. Sensors 2020, 20, 3756. https://doi.org/10.3390/s20133756
Cavagnaro M, Ruvio G. Numerical Sensitivity Analysis for Dielectric Characterization of Biological Samples by Open-Ended Probe Technique. Sensors. 2020; 20(13):3756. https://doi.org/10.3390/s20133756
Chicago/Turabian StyleCavagnaro, Marta, and Giuseppe Ruvio. 2020. "Numerical Sensitivity Analysis for Dielectric Characterization of Biological Samples by Open-Ended Probe Technique" Sensors 20, no. 13: 3756. https://doi.org/10.3390/s20133756
APA StyleCavagnaro, M., & Ruvio, G. (2020). Numerical Sensitivity Analysis for Dielectric Characterization of Biological Samples by Open-Ended Probe Technique. Sensors, 20(13), 3756. https://doi.org/10.3390/s20133756






