A Wearable Device Based on a Fiber Bragg Grating Sensor for Low Back Movements Monitoring †
Abstract
:1. Introduction
2. Design and Development of the Wearable System
2.1. Sensing Element Based on FBG
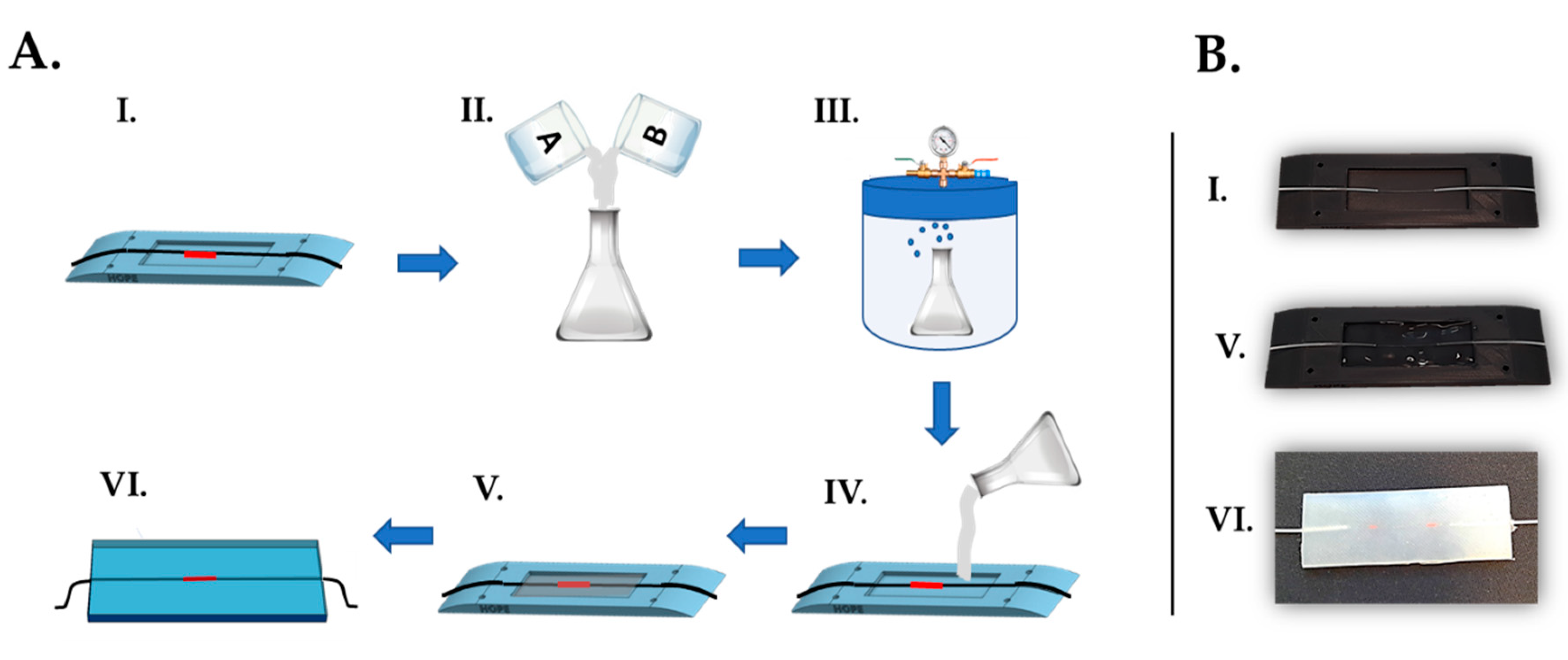
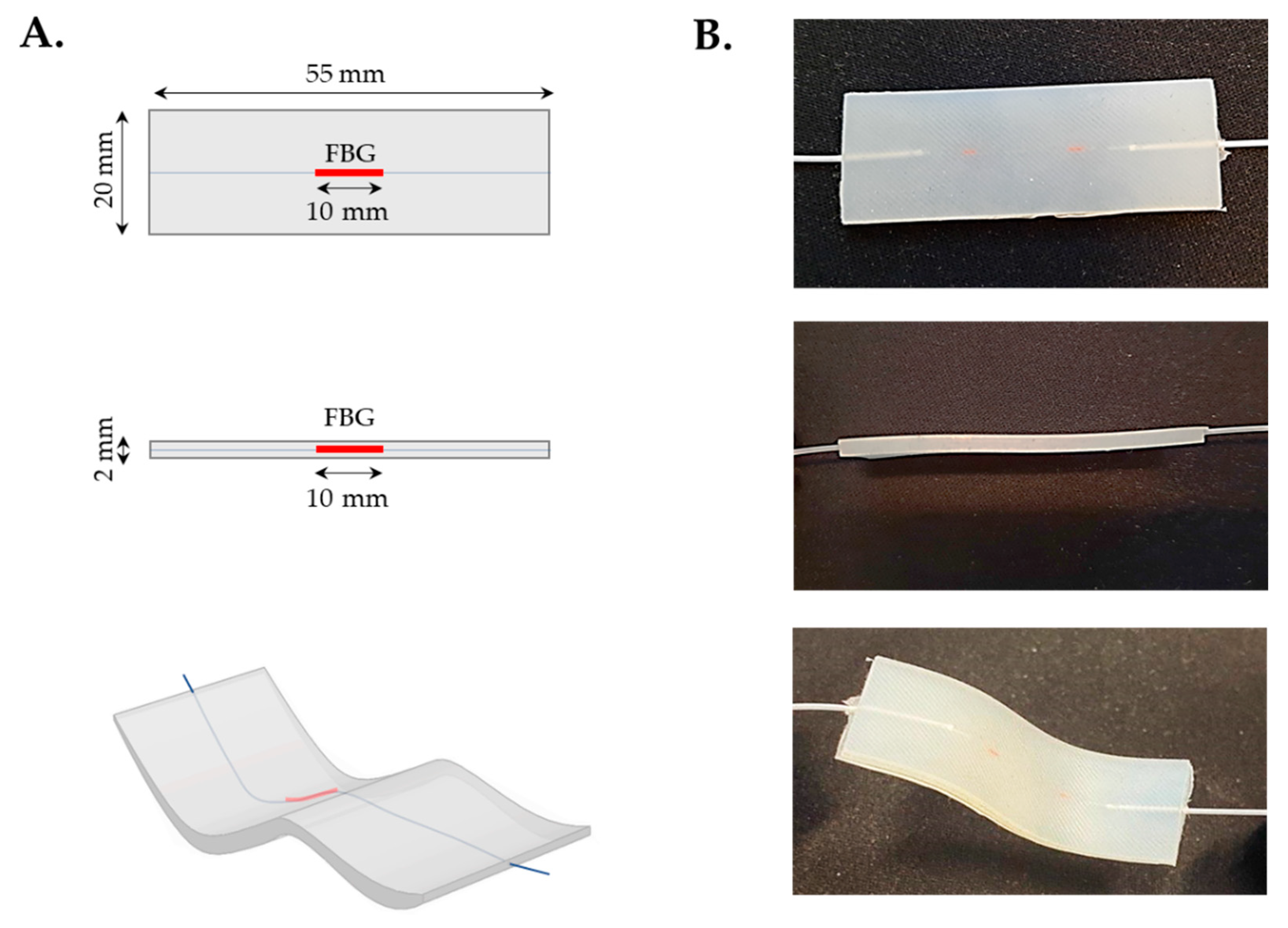
2.1.1. Design and Manufacturing
- The FBG was placed at the midsection of the custom-made plastic mold. The extremities of the optical fiber were then passed inside the lateral grooves and delicately fixed with the help of some adhesive tape in order to keep the fiber adequately tight;
- Dragon SkinTM20 silicone rubber parts A and B were mixed 1A:1B by volume ratio (as indicated in the technical bulletin [31]). Then, an amount of 10% in volume of liquid thinner was added to reduce the viscosity of the compound. The mixture was well stirred in order to allow the complete blending of all the components;
- The compound was put into a vacuum chamber and let degas for few minutes in order to obtain an opalescent fluid with no presence of gas bubbles;
- The degassed mixture was slowly poured into the mold until its full filling;
- The mixture was let polymerize for a curing time of four hours at room temperature (as indicated in the technical bulletin [31]);
- Once solidified, the flexible rectangular-shaped (i.e., 55 mm × 20 mm × 2 mm) sensing element was extracted from the mold. The excess of polymeric material was removed by means of a cutter and the edges were refined.
2.1.2. Working Principle
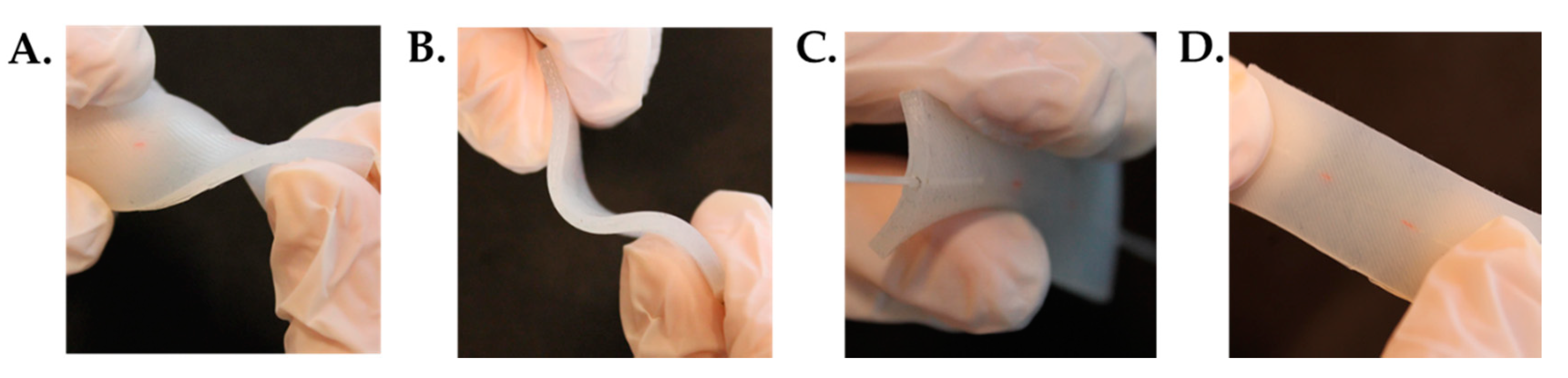
2.1.3. Mechanical Characterization
2.2. Wearable Device
3. Feasibility Assessment for Monitoring Low Back Movements
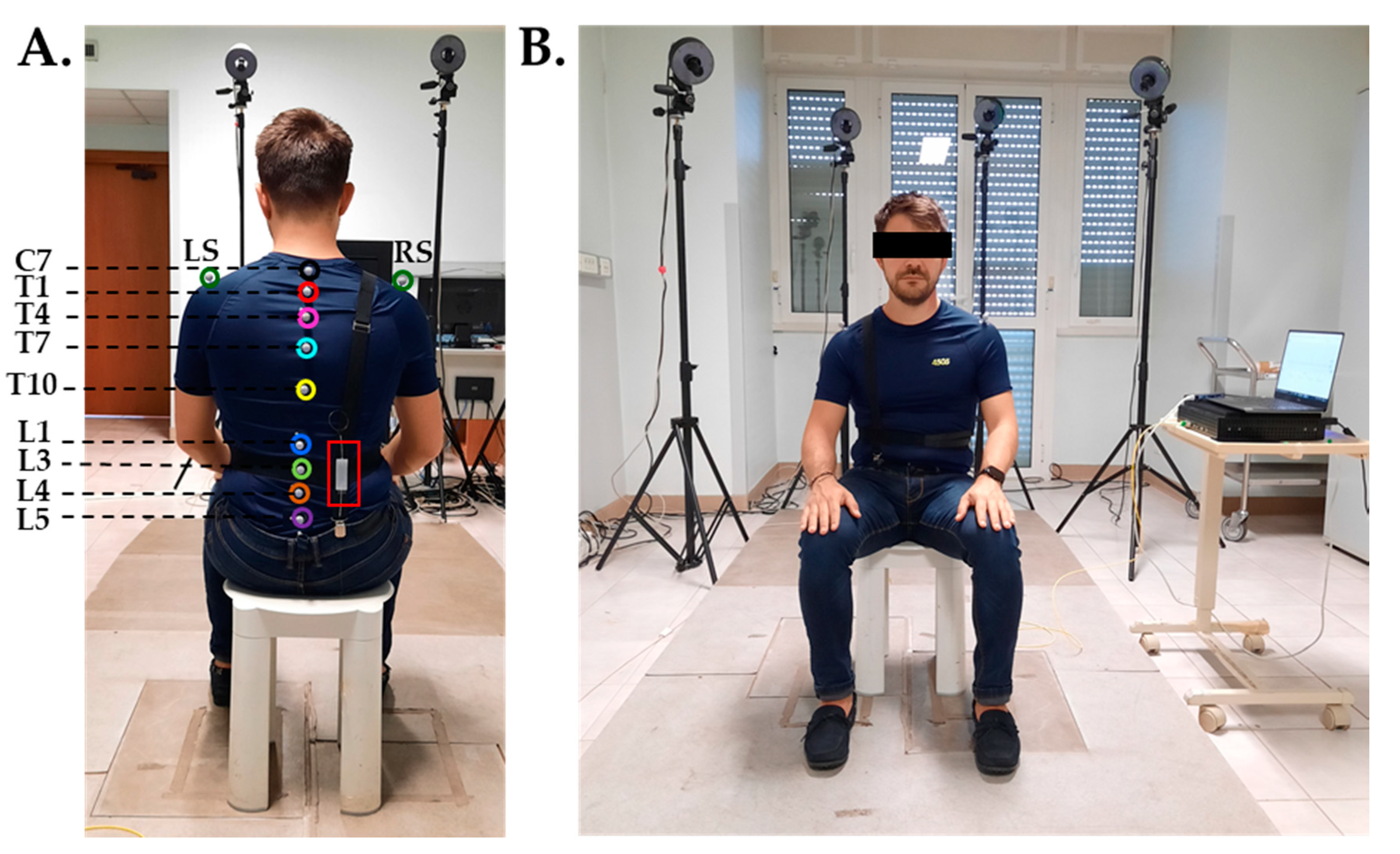
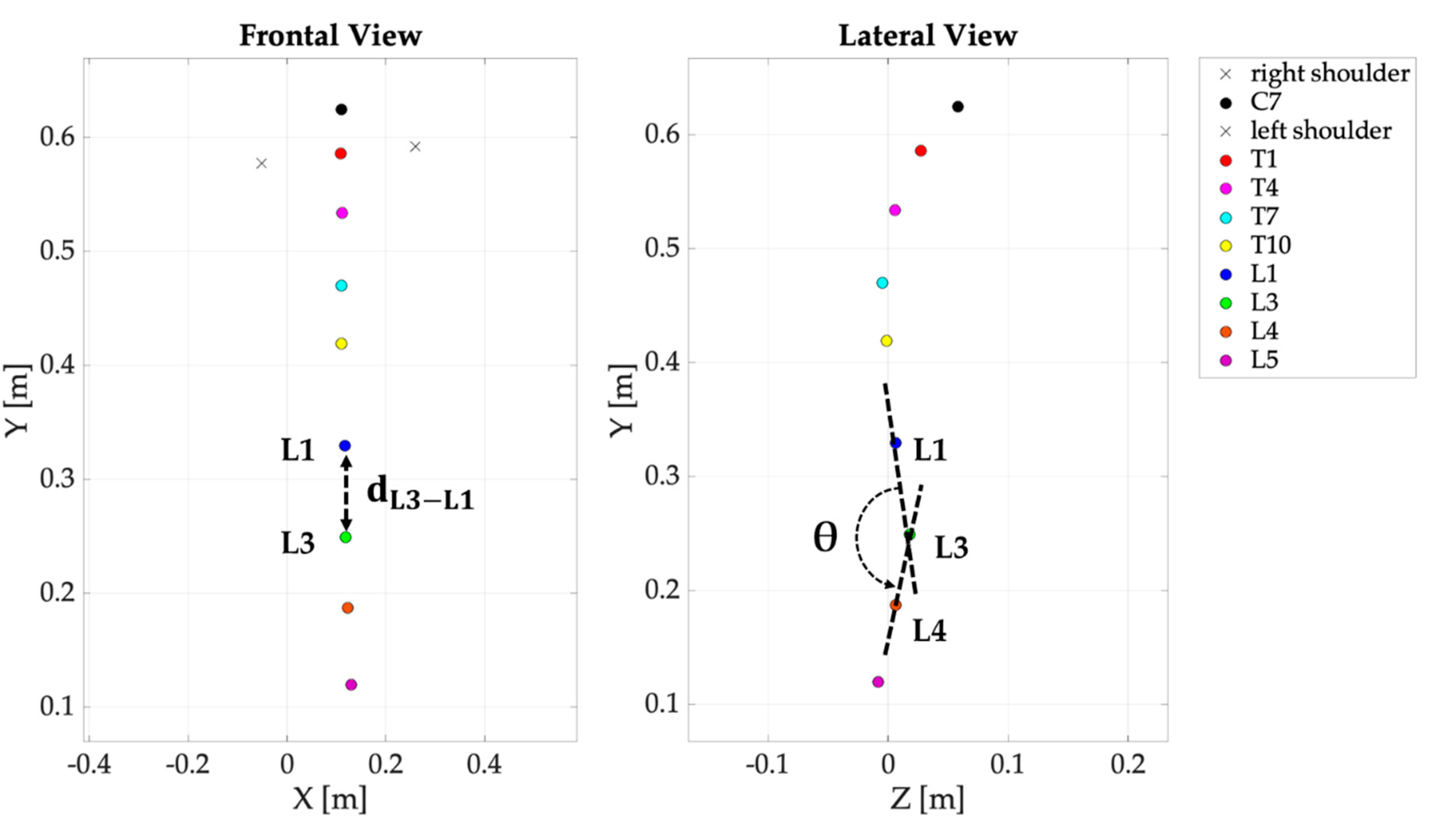
3.1. Population and Experimental Design
3.2. Data Analysis
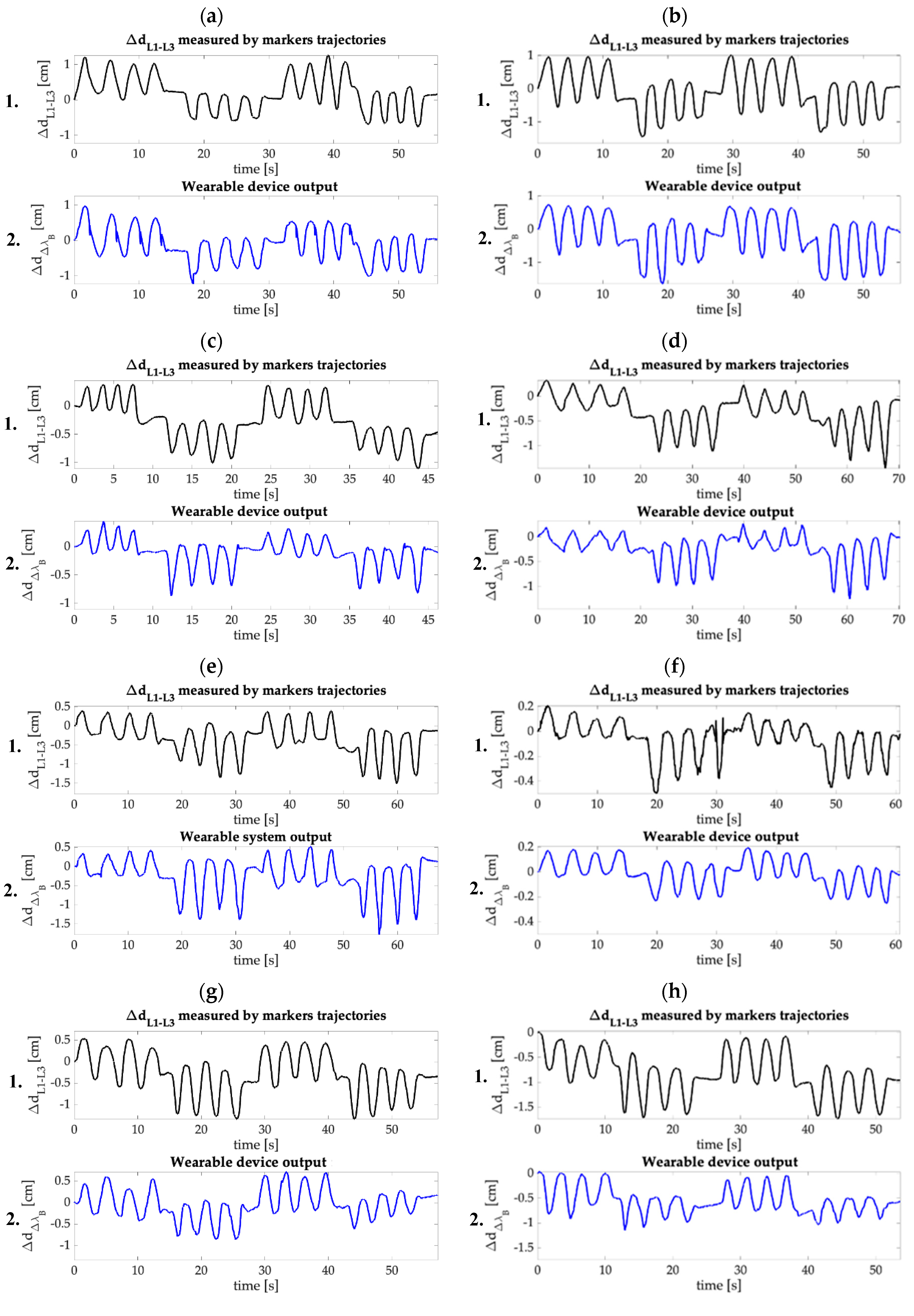
3.3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Lasi, H.; Fettke, P.; Kemper, H.-G.; Feld, T.; Hoffmann, M. Industry 4.0. Bus Inf. Syst. Eng. 2014, 6, 239–242. [Google Scholar] [CrossRef]
- Gerr, F.; Marcus, M.; Ortiz, D.J. Methodological limitations in the study of video display terminal use and upper extremity musculoskeletal disorders. Am. J. Ind. Med. 1996, 29, 649–656. [Google Scholar] [CrossRef]
- Schuster, K.; Groß, K.; Vossen, R.; Richert, A.; Jeschke, S. Preparing for industry 4.0—Collaborative virtual learning environments in engineering education. In Engineering Education 40; Springer: New York, NY, USA, 2016; pp. 477–487. [Google Scholar]
- Woods, V. Musculoskeletal disorders and visual strain in intensive data processing workers. Occup. Med. (Chic Ill). 2005, 55, 121–127. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cho, C.-Y.; Hwang, Y.-S.; Cherng, R.-J. Musculoskeletal symptoms and associated risk factors among office workers with high workload computer use. J. Manip. Physiol. Ther. 2012, 35, 534–540. [Google Scholar] [CrossRef] [PubMed]
- Spyropoulos, P.; Papathanasiou, G.; Georgoudis, G.; Chronopoulos, E.; Koutis, H.; Koumoutsou, F. Prevalence of low back pain in Greek public office workers. Pain Physician 2007, 10, 651. [Google Scholar]
- Koes, B.W.; van Tulder, M.W.; Thomas, S. Diagnosis and treatment of low back pain. BMJ 2006, 332, 1430–1434. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Maetzel, A.; Li, L. The economic burden of low back pain: A review of studies published between 1996 and 2001. Best Pract. Res. Clin. Rheumatol. 2002, 16, 23–30. [Google Scholar] [CrossRef]
- Ricci, J.A.; Stewart, W.F.; Chee, E.; Leotta, C.; Foley, K.; Hochberg, M.C. Back pain exacerbations and lost productive time costs in United States workers. Spine (Phila Pa 1976) 2006, 31, 3052–3060. [Google Scholar] [CrossRef]
- Guo, H.R.; Tanaka, S.; Cameron, L.L.; Seligman, P.J.; Behrens, V.J.; Ger, J.; Wild, D.K.; Putz-Anderson, V. Back pain among workers in the United States: National estimates and workers at high risk. Am. J. Ind. Med. 1995, 28, 591–602. [Google Scholar] [CrossRef]
- Kaliniene, G.; Ustinaviciene, R.; Skemiene, L.; Vaiciulis, V.; Vasilavicius, P. Associations between musculoskeletal pain and work-related factors among public service sector computer workers in Kaunas County, Lithuania. BMC Musculoskelet. Disord. 2016, 17, 420. [Google Scholar] [CrossRef] [Green Version]
- Kachingwe, A.F.; Phillips, B.J. Inter-and intrarater reliability of a back range of motion instrument. Arch. Phys. Med. Rehabil. 2005, 86, 2347–2353. [Google Scholar] [CrossRef]
- Ma, H.T.; Yang, Z.; Griffith, J.F.; Leung, P.C.; Lee, R.Y.W. A new method for determining lumbar spine motion using Bayesian belief network. Med. Biol. Eng. Comput. 2008, 46, 333–340. [Google Scholar] [CrossRef] [PubMed]
- Lee, R. Measurement of movements of the lumbar spine. Physiother. Theory Pract. 2002, 18, 159–164. [Google Scholar] [CrossRef]
- Pearcy, M. Measurement of back and spinal mobility. Clin. Biomech. 1986, 1, 44–51. [Google Scholar] [CrossRef]
- Bartalesi, R.; Lorussi, F.; De Rossi, D.; Tesconi, M.; Tognetti, A. Wearable monitoring of lumbar spine curvature by inertial and e-textile sensory fusion. In Proceedings of the 2010 Annual International Conference of the IEEE Engineering in Medicine and Biology, IEEE, Buenos Aires, Argentina, 31 August–4 September 2010; pp. 6373–6376. [Google Scholar]
- Suter, M.; Eichelberger, P.; Frangi, J.; Simonet, E.; Baur, H.; Schmid, S. Measuring lumbar back motion during functional activities using a portable strain gauge sensor-based system: A comparative evaluation and reliability study. J. Biomech. 2020, 100, 1–14. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Williams, J.M.; Haq, I.; Lee, R.Y. Dynamic measurement of lumbar curvature using fibre-optic sensors. Med. Eng. Phys. 2010, 32, 1043–1049. [Google Scholar] [CrossRef]
- Cloud, B.A.; Zhao, K.D.; Breighner, R.; Giambini, H.; An, K.-N. Agreement between fiber optic and optoelectronic systems for quantifying sagittal plane spinal curvature in sitting. Gait Posture 2014, 40, 369–374. [Google Scholar] [CrossRef] [Green Version]
- Dunne, L.E.; Walsh, P.; Smyth, B.; Caulfield, B. Design and evaluation of a wearable optical sensor for monitoring seated spinal posture. In Proceedings of the 2006 10th IEEE International Symposium on Wearable Computers, Broomfield, CO, USA, 11–14 October 2006; pp. 65–68. [Google Scholar]
- Yin, S.; Ruffin, P. Fiber optic sensors. In Wiley Encyclopedia of Biomedical Engineering; Metin, A., Ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2006. [Google Scholar]
- Krohn, D.A.; MacDougall, T.; Mendez, A. Fiber Optic Sensors: Fundamentals and Applications; SPIE Press: Bellingham, WA, USA, 2014. [Google Scholar]
- Anwar Zawawi, M.; O’Keffe, S.; Lewis, E. Intensity-modulated fiber optic sensor for health monitoring applications: A comparative review. Sens. Rev. 2013, 33, 57–67. [Google Scholar] [CrossRef]
- Presti, D.L.; Romano, C.; Massaroni, C.; D’Abbraccio, J.; Massari, L.; Caponero, M.A.; Oddo, C.M.; Formica, D.; Schena, E. Cardio-Respiratory Monitoring in Archery Using a Smart Textile Based on Flexible Fiber Bragg Grating Sensors. Sensors 2019, 19, 3581. [Google Scholar] [CrossRef] [Green Version]
- Presti, D.L.; Massaroni, C.; Saccomandi, P.; Caponero, M.A.; Formica, D.; Schena, E. A wearable textile for respiratory monitoring: Feasibility assessment and analysis of sensors position on system response. In Proceedings of the Annual International Conference of the IEEE Engineering in Medicine and Biology Society, Jeju Island, Korea, 11–15 July 2017; pp. 4423–4426. [Google Scholar]
- Massaroni, C.; Presti, D.L.; Saccomandi, P.; Caponero, M.A.; D’Amato, R.; Schena, E. Fiber Bragg grating probe for relative humidity and respiratory frequency estimation: Assessment during mechanical ventilation. IEEE Sens. J. 2018, 18, 2125–2130. [Google Scholar] [CrossRef]
- Massaroni, C.; Venanzi, C.; Silvatti, A.P.; Presti, D.L.; Saccomandi, P.; Formica, D.; Giurazza, F.; Caponero, M.A.; Schena, E. Smart textile for respiratory monitoring and thoraco-abdominal motion pattern evaluation. J. Biophotonics 2018, 11, e201700263. [Google Scholar] [CrossRef] [Green Version]
- Presti, D.L.; Massaroni, C.; D’Abbraccio, J.; Massari, L.; Caponero, M.A.; Longo, U.G.; Oddo, C.M.; Formica, D.; Schena, E. Wearable system based on flexible FBG for respiratory and cardiac monitoring. IEEE Sens. J. 2019. [Google Scholar] [CrossRef]
- Presti, D.L.; Carnevale, A.; D’Abbraccio, J.; Massari, L.; Massaroni, C.; Sabbadini, R.; Zaltieri, M.; Tocco, J.D.; Bravi, M.; Miccinilli, S.; et al. A Multi-Parametric Wearable System to Monitor Neck Movements and Respiratory Frequency of Computer Workers. Sensors 2020, 20, 536. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- User Manual 3D printer Ultimaker 2. Available online: https://support.ultimaker.com/hc/en-us/articles/360011811480-The-Ultimaker-2-user-manuals (accessed on 8 July 2020).
- Dragon Skin Technical Bullettin. Available online: https://www.smooth-on.com/products/dragon-skin-20/ (accessed on 8 July 2020).
- Hill, K.O.; Meltz, G. Fiber Bragg grating technology fundamentals and overview. J. Light Technol. 1997, 15, 1263–1276. [Google Scholar] [CrossRef] [Green Version]
- Doyle, C. Fibre Bragg Grating Sensors-An Introduction to Bragg gratings and interrogation techniques. Smart Fibres Ltd 2003, 1, 1–5. [Google Scholar]
- JCGM. Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement; The International Organization for Standardization: Geneva, Switzerland, 2008. [Google Scholar]
- Chockalingam, N.; Dangerfield, P.H.; Giakas, G.; Cochrane, T. Study of Marker Placements in the Back for Opto-electronic Motion Analysis. Stud. Health Technol. Inform. 2002, 88, 105–109. [Google Scholar] [PubMed]
- Tang, L.; Tao, X.; Choy, C. Effectiveness and optimization of fiber Bragg grating sensor as embedded strain sensor. Smart Mater. Struct. 1999, 8, 154. [Google Scholar] [CrossRef] [Green Version]


| Trial | #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 |
| β [cm·nm−1] | 1.38 | 2.10 | 2.78 | 1.08 | 1.43 | 0.32 | 1.45 | 1.84 |
| R2 | 0.92 | 0.78 | 0.89 | 0.66 | 0.91 | 0.93 | 0.88 | 0.88 |
| Trial | #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 |
| MAEΔd [cm] | 0.33 | 0.18 | 0.21 | 0.14 | 0.14 | 0.07 | 0.28 | 0.29 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zaltieri, M.; Massaroni, C.; Lo Presti, D.; Bravi, M.; Sabbadini, R.; Miccinilli, S.; Sterzi, S.; Formica, D.; Schena, E. A Wearable Device Based on a Fiber Bragg Grating Sensor for Low Back Movements Monitoring. Sensors 2020, 20, 3825. https://doi.org/10.3390/s20143825
Zaltieri M, Massaroni C, Lo Presti D, Bravi M, Sabbadini R, Miccinilli S, Sterzi S, Formica D, Schena E. A Wearable Device Based on a Fiber Bragg Grating Sensor for Low Back Movements Monitoring. Sensors. 2020; 20(14):3825. https://doi.org/10.3390/s20143825
Chicago/Turabian StyleZaltieri, Martina, Carlo Massaroni, Daniela Lo Presti, Marco Bravi, Riccardo Sabbadini, Sandra Miccinilli, Silvia Sterzi, Domenico Formica, and Emiliano Schena. 2020. "A Wearable Device Based on a Fiber Bragg Grating Sensor for Low Back Movements Monitoring" Sensors 20, no. 14: 3825. https://doi.org/10.3390/s20143825
APA StyleZaltieri, M., Massaroni, C., Lo Presti, D., Bravi, M., Sabbadini, R., Miccinilli, S., Sterzi, S., Formica, D., & Schena, E. (2020). A Wearable Device Based on a Fiber Bragg Grating Sensor for Low Back Movements Monitoring. Sensors, 20(14), 3825. https://doi.org/10.3390/s20143825











