CSNOMA: Carrier Sense Non-Orthogonal Multiple Access
Abstract
1. Introduction
- We propose a new multiple access protocol, carrier sense non-orthogonal multiple access (CSNOMA), which utilizes the interference cancellation technique in the sense of cross-layer design with considering physical (PHY) and multiple access control (MAC) layers.
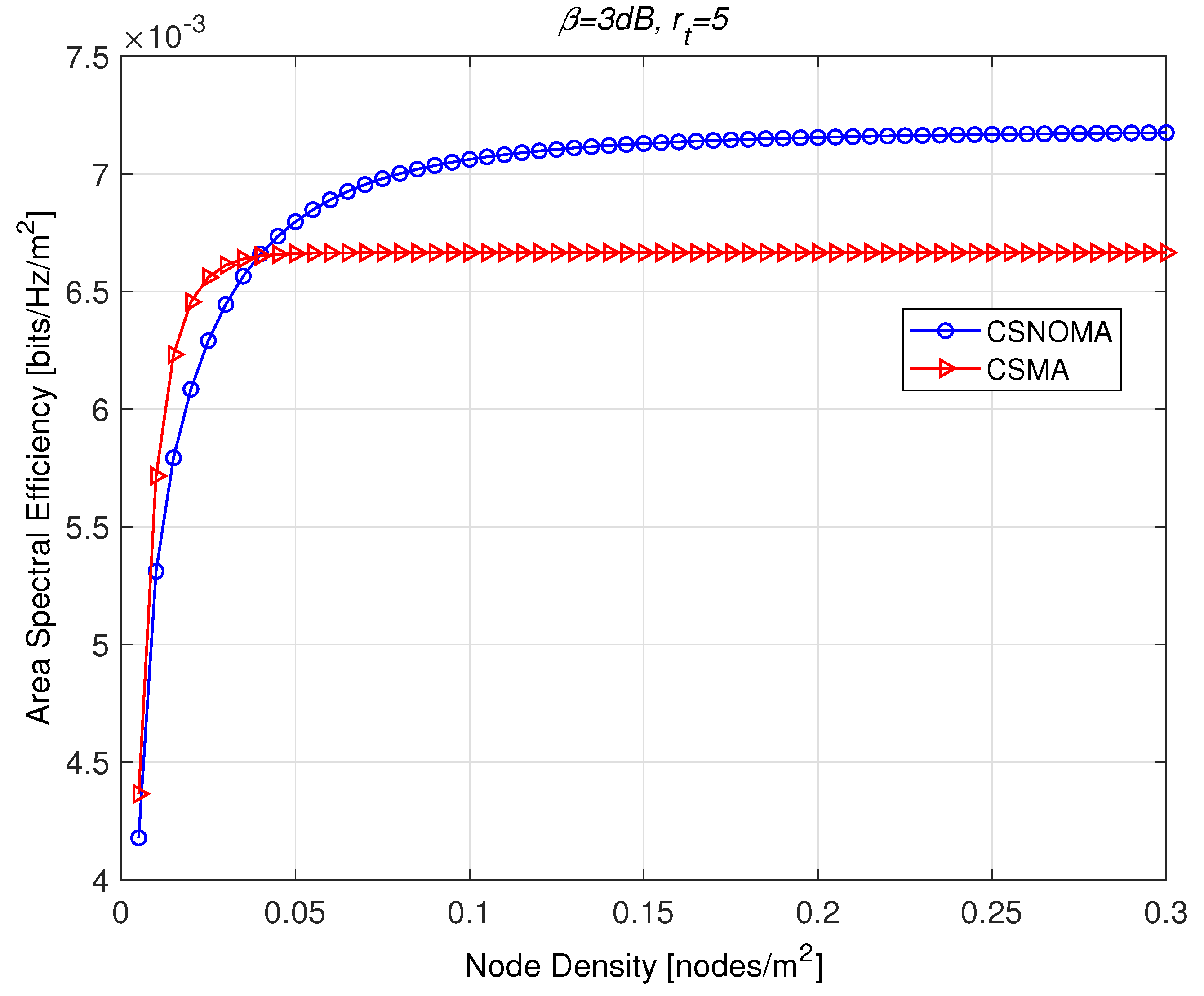
- We show the area spectral efficiency performance of the proposed scheme is better than CSMA/CA, as the node density increases.
- We present a practical signaling design which is compatible with IEEE 802.11 DCF mode.
2. Related Work
3. System Model
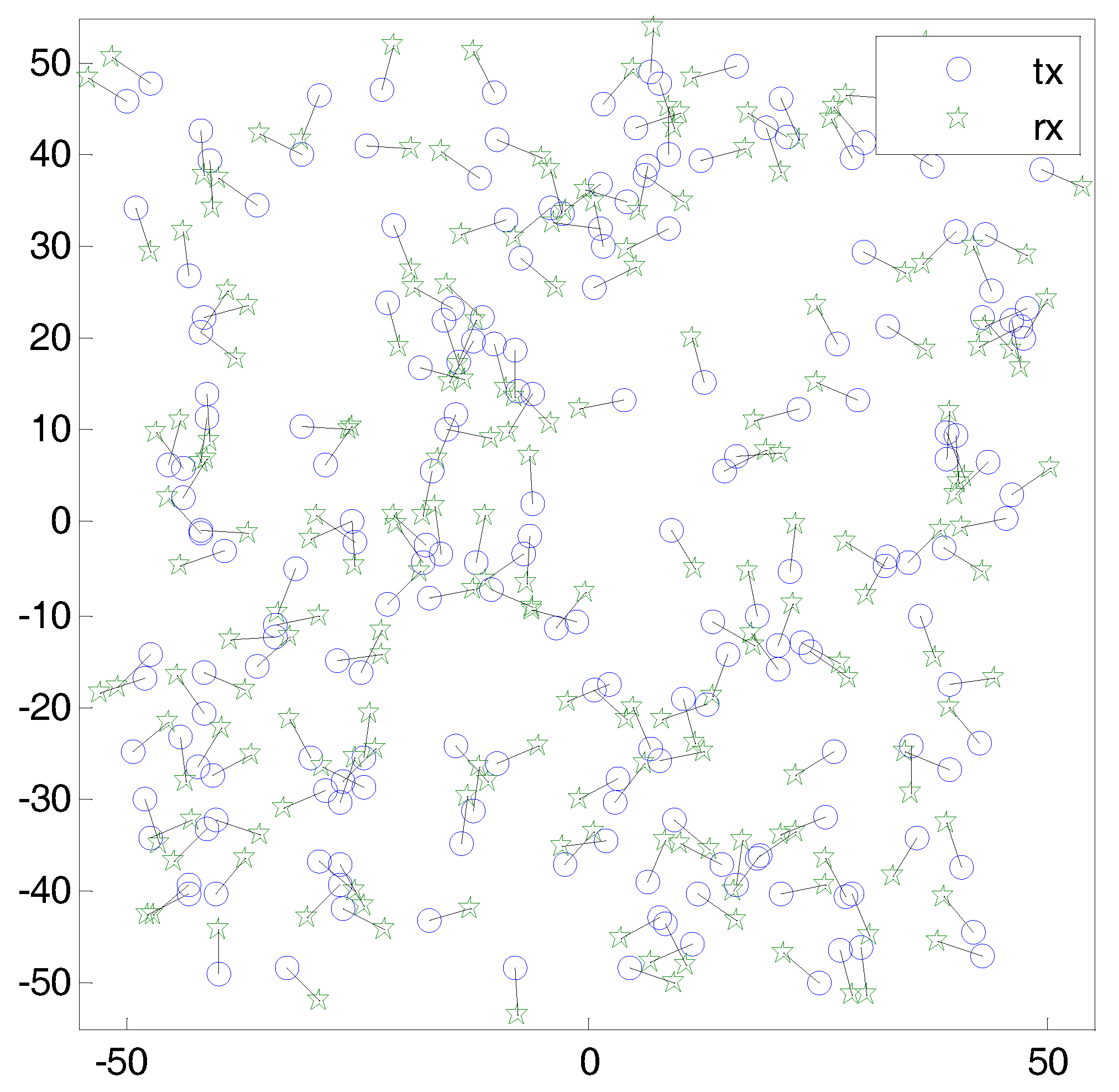
3.1. Network Model
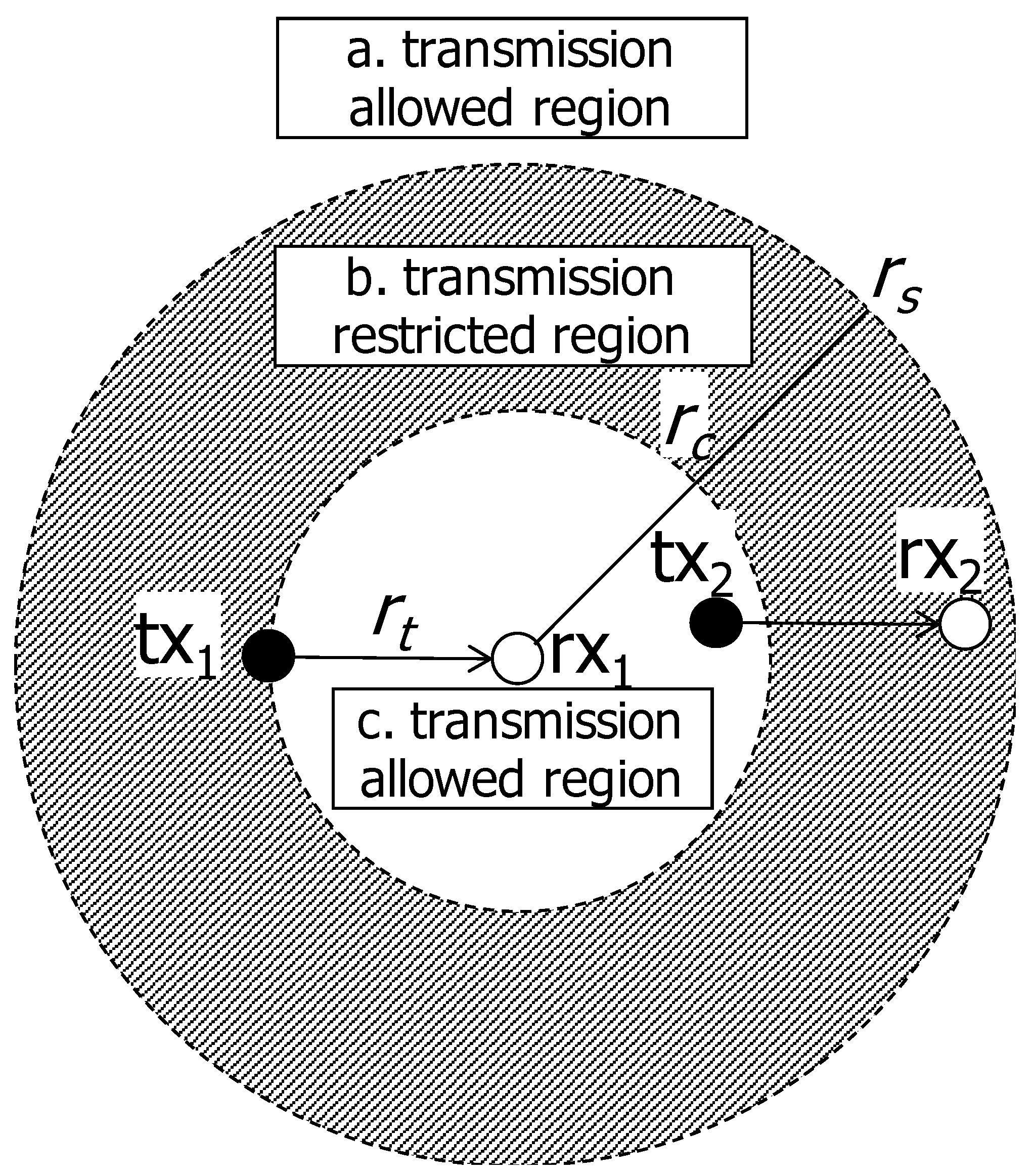
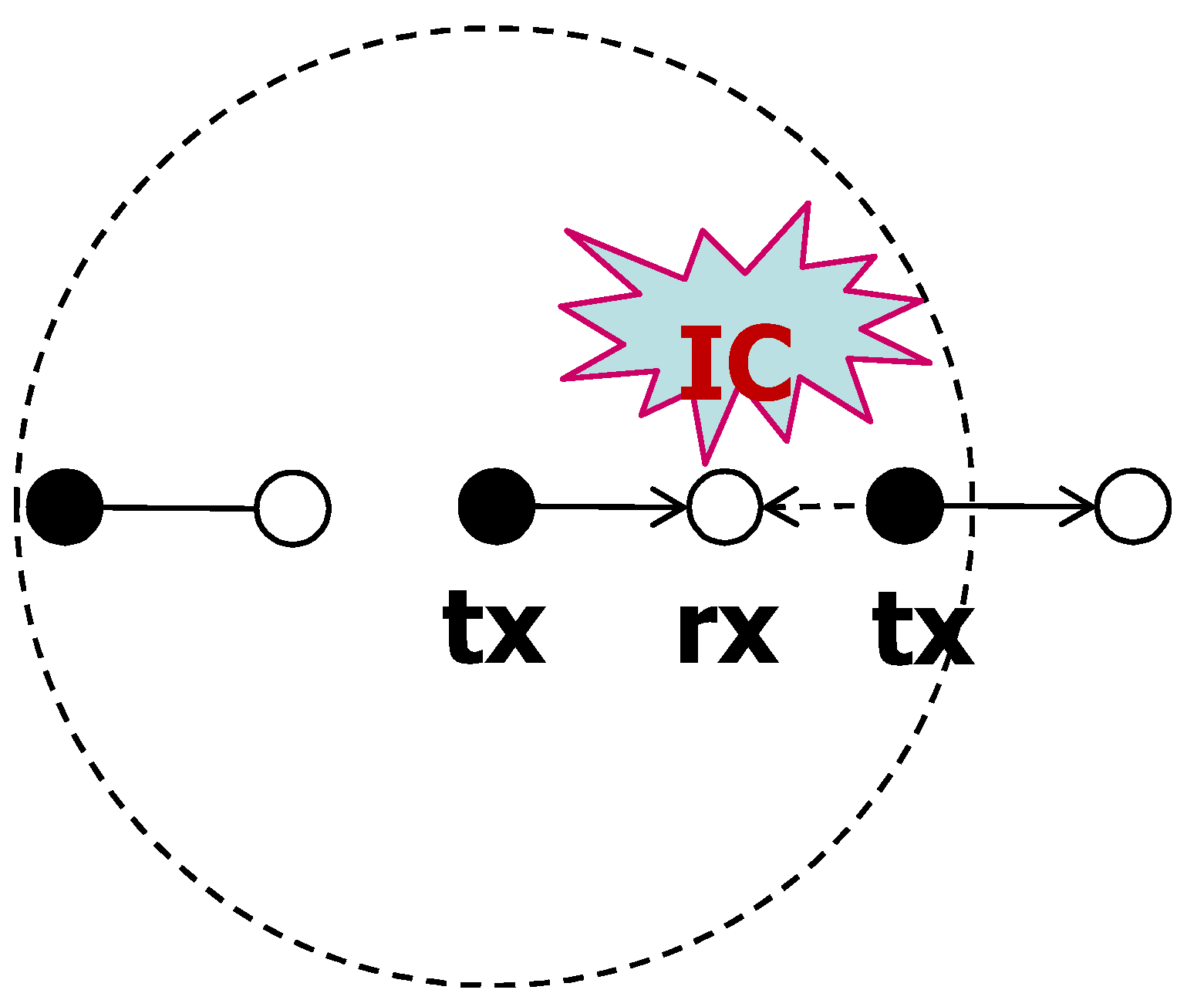
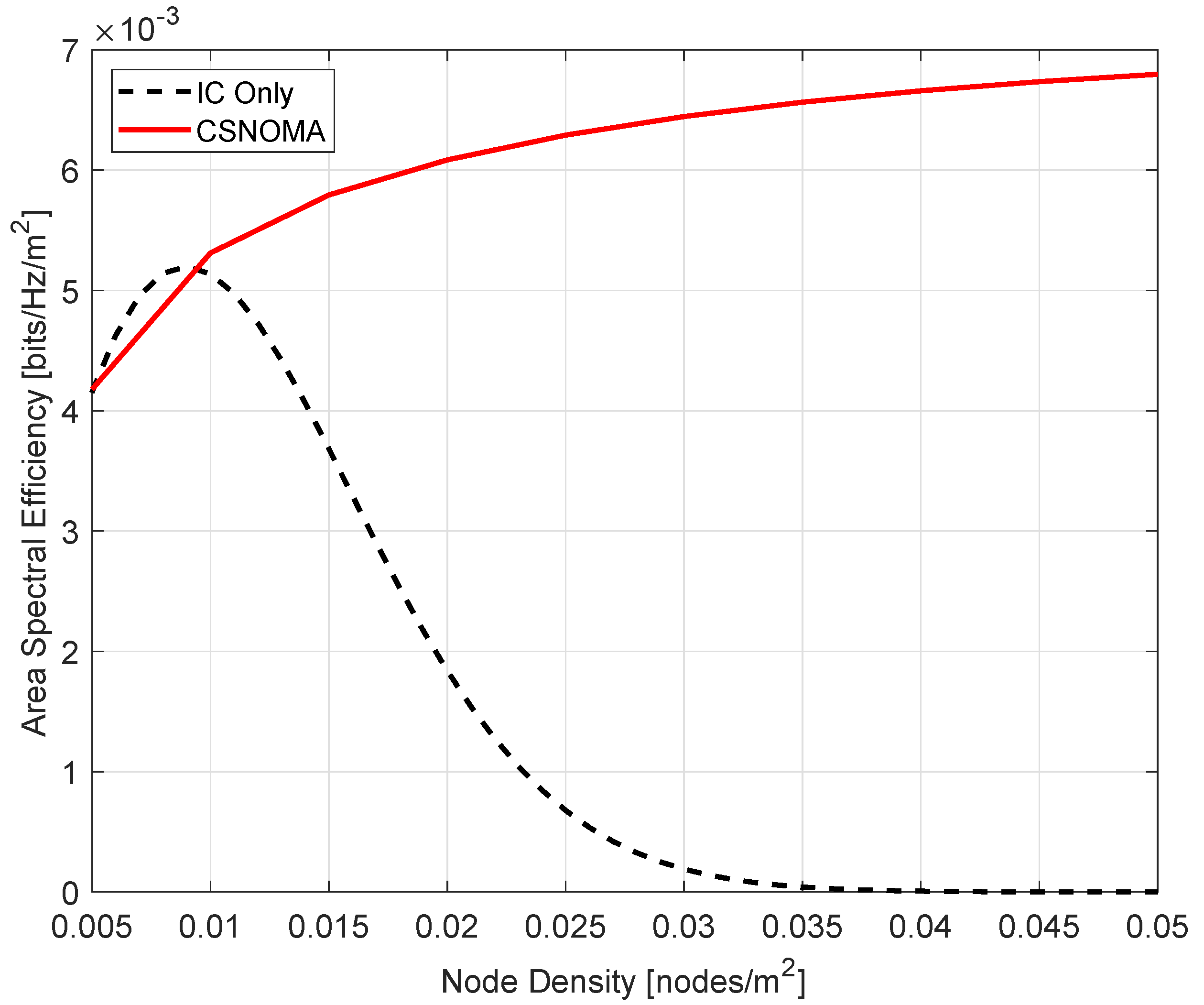
3.2. Interference Cancellation Model
4. Carrier Sense Non-Orthogonal Multiple Access (CSNOMA)
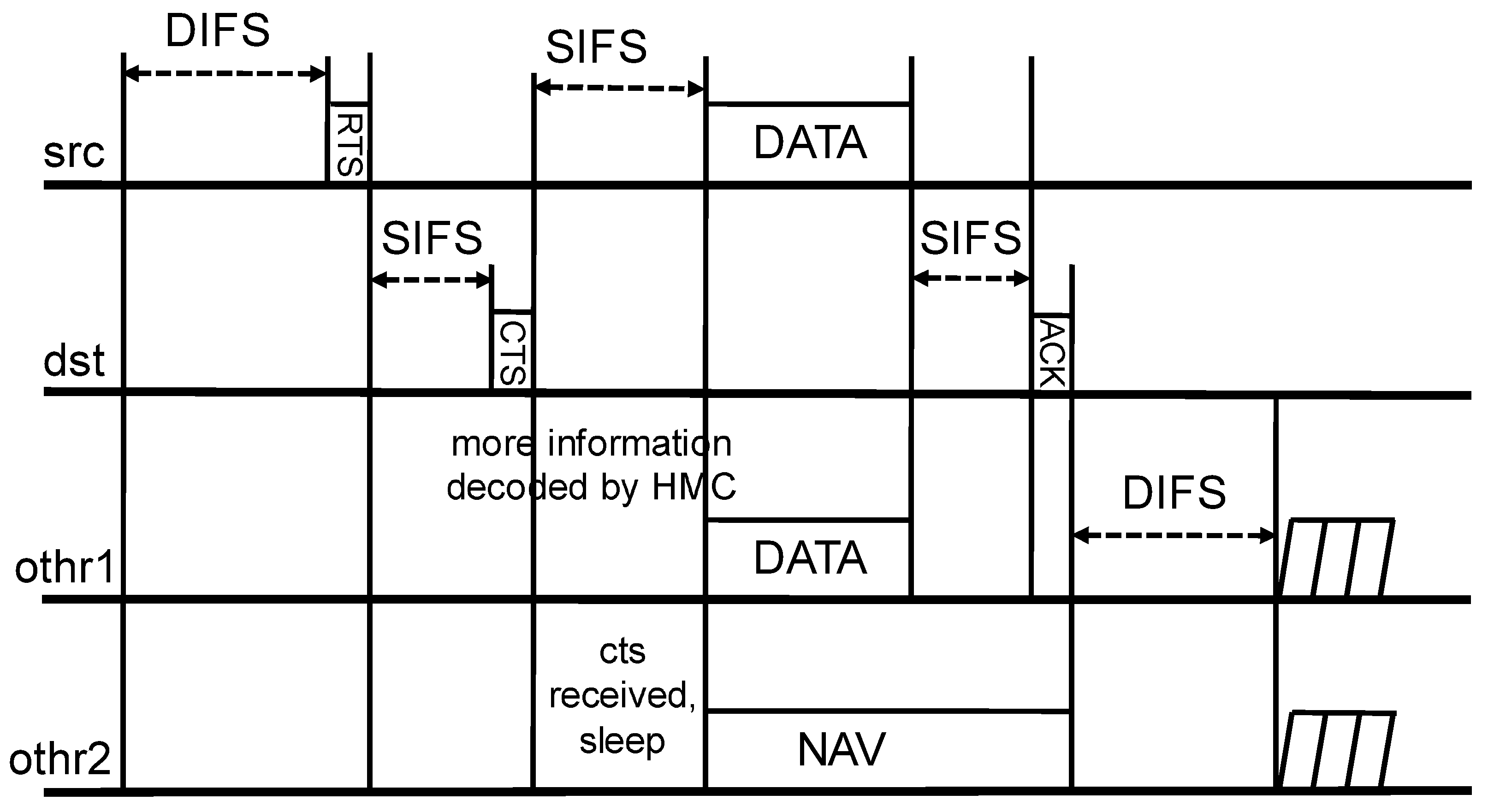
4.1. Proposed Protocol
4.2. Area Spectral Efficiency Analysis
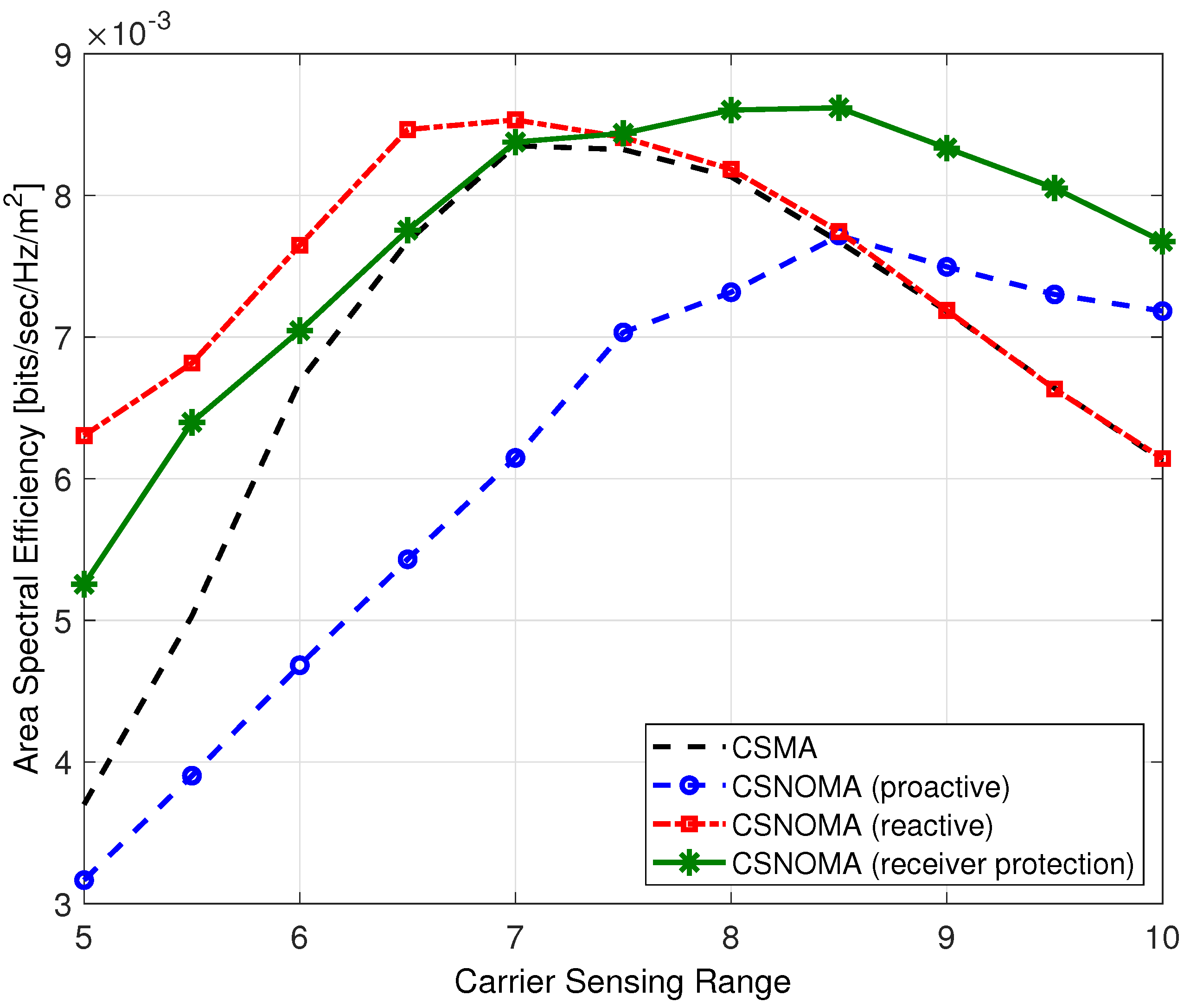
4.3. Optimal Carrier Sensing Range
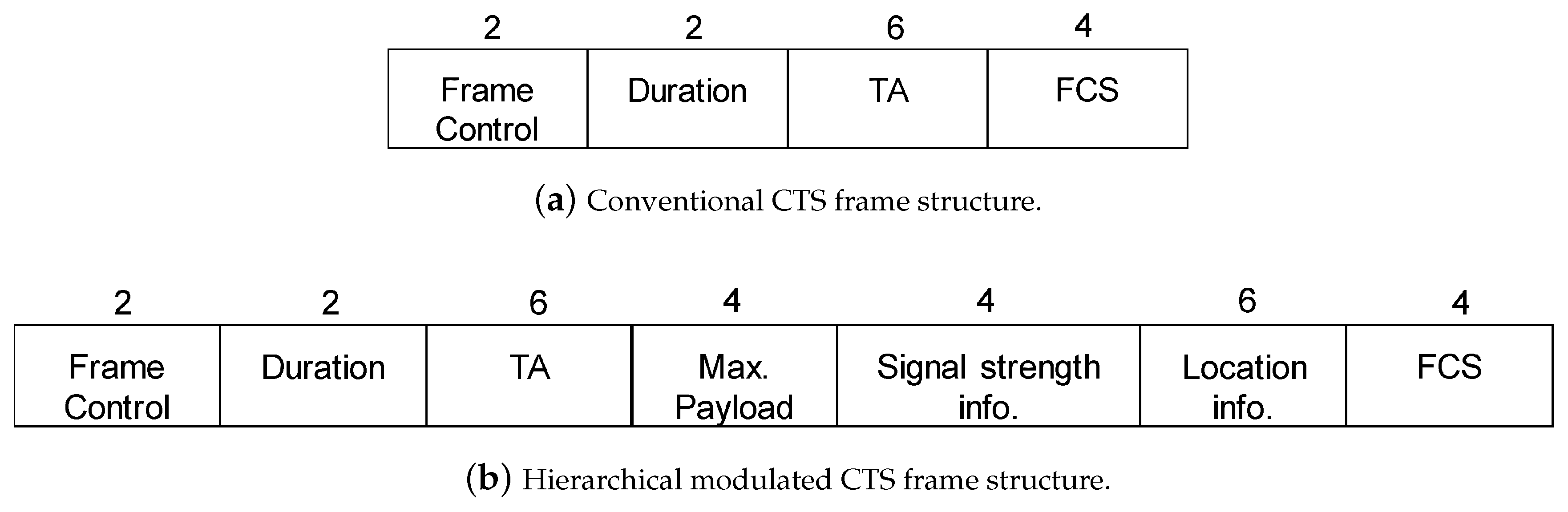
4.4. Signaling Design
CTS Frame Format
4.5. Reactive/Proactive Mode
4.6. Receiver Protection Mode
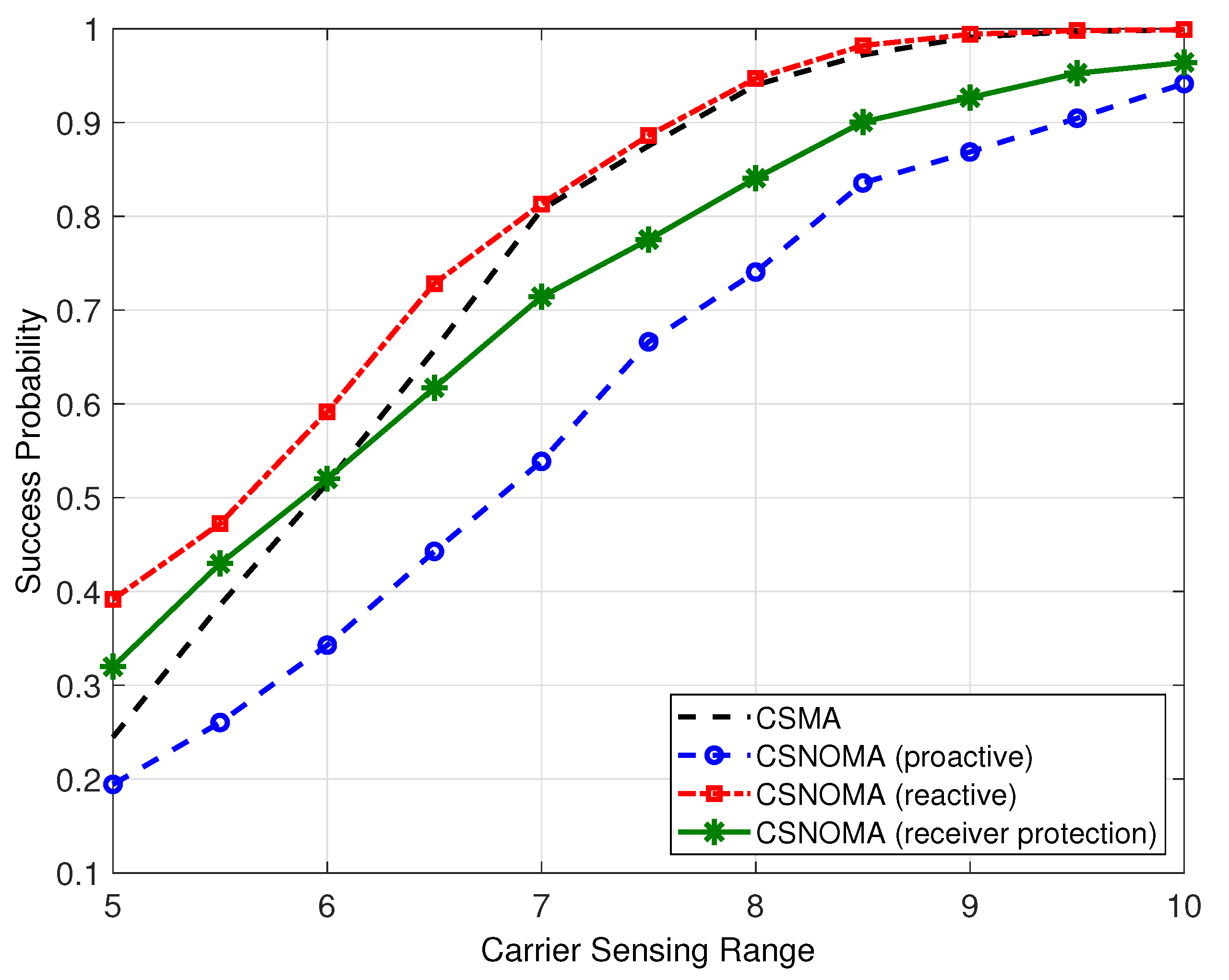
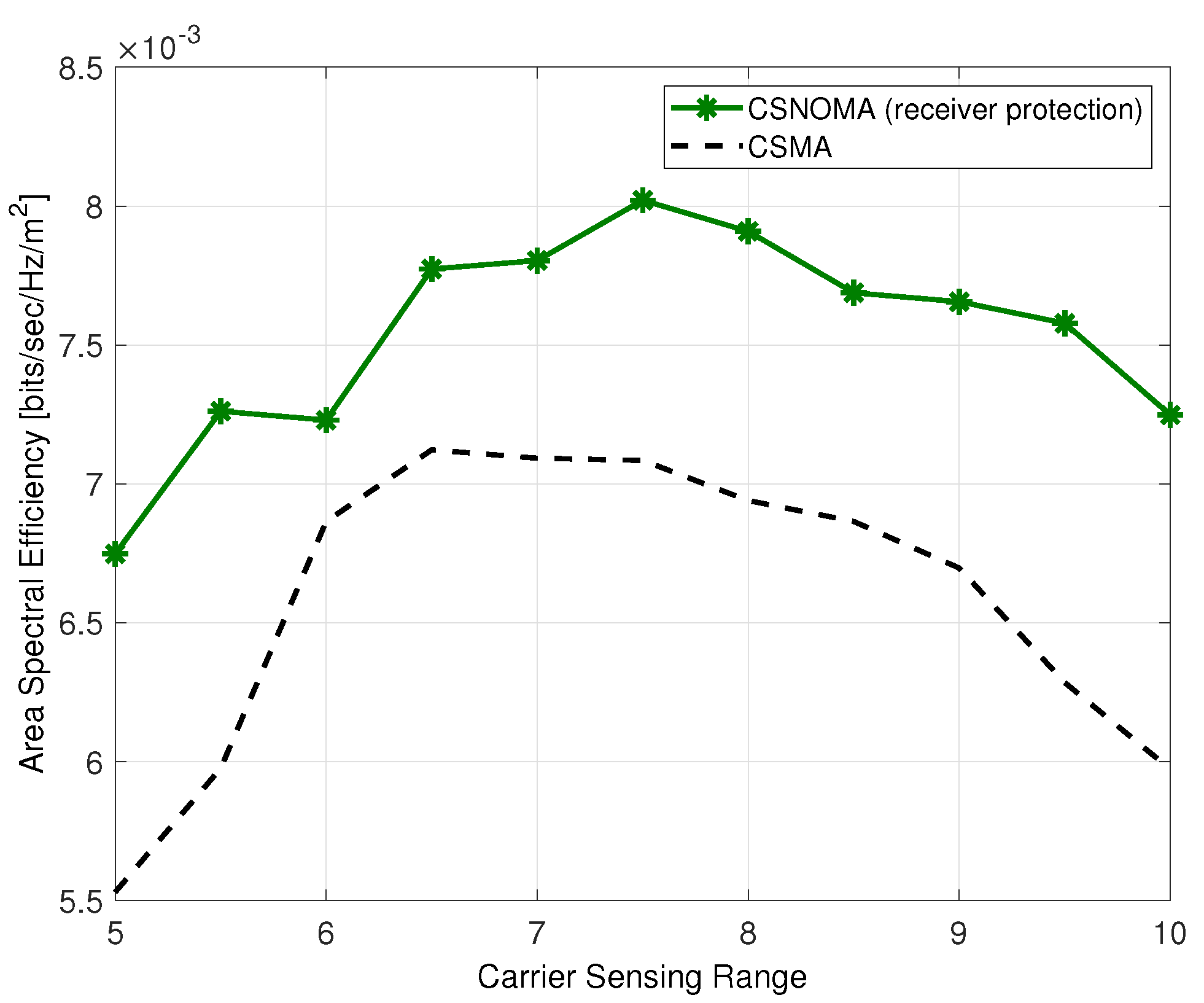
5. Numerical Results
6. Concluding Remarks
Author Contributions
Funding
Conflicts of Interest
Appendix A
Appendix B
References
- Cisco. Cisco Annual Internet Report (2018–2023) White Paper; Cisco: San Jose, CA, USA, 2020. [Google Scholar]
- Yu, H.; Afzal, M.K.; Zikria, Y.B.; Rachedi, A.; Fitzek, F.H. Tactile Internet: Technologies, test platforms, trials, and applications. Future Gener. Comput. Syst. 2020, 106, 685–688. [Google Scholar] [CrossRef]
- Alouini, M.S.; Goldsmith, A.J. Area spectral efficiency of cellular mobile radio systems. IEEE Trans. Veh. Technol. 1999, 48, 1047–1066. [Google Scholar] [CrossRef]
- Baccelli, F.; Błaszczyszyn, B.; Mühlethaler, P. An Aloha protocol for multihop mobile wireless networks. IEEE Trans. Inf. Theory 2006, 52, 421–436. [Google Scholar] [CrossRef]
- Kim, D.M.; Kim, S.L. An iterative algorithm for optimal carrier sensing threshold in random CSMA/CA wireless networks. IEEE Commun. Lett. 2013, 17, 2076–2079. [Google Scholar] [CrossRef]
- Bianchi, G. Performance analysis of the IEEE 802.11 distributed coordination function. IEEE J. Sel. Areas Commun. 2000, 18, 535–547. [Google Scholar] [CrossRef]
- Saito, Y.; Kishiyama, Y.; Benjebbour, A.; Nakamura, T.; Li, A.; Higuchi, K. Non-orthogonal multiple access (NOMA) for cellular future radio access. In Proceedings of the IEEE Vehicular Technology Conference (VTC Spring), Dresden, Germany, 2–5 June 2013; pp. 1–5. [Google Scholar]
- 3GPP. Study on Non-Orthogonal Multiple Access (NOMA) for NR. TR 38.812. 2018. Available online: https://portal.3gpp.org/desktopmodules/Specifications/SpecificationDetails.aspx?specificationId=3236 (accessed on 7 August 2020).
- Vaezi, M.; Schober, R.; Ding, Z.; Poor, H.V. Non-orthogonal multiple access: Common myths and critical questions. IEEE Wirel. Commun. 2019, 26, 174–180. [Google Scholar] [CrossRef]
- Del-Valle-Soto, C.; Mex-Perera, C.; Orozco-Lugo, A.; Lara, M.; Galván-Tejada, G.M.; Olmedo, O. On the MAC/Network/Energy performance evaluation of wireless sensor networks: Contrasting MPH, AODV, DSR and ZTR routing protocols. Sensors 2014, 14, 22811–22847. [Google Scholar] [CrossRef]
- Yan, J.; Zhou, M.; Ding, Z. Recent advances in energy-efficient routing protocols for wireless sensor networks: A review. IEEE Access 2016, 4, 5673–5686. [Google Scholar] [CrossRef]
- Andrews, J.G. Interference cancellation for cellular systems: A contemporary overview. IEEE Wirel. Commun. 2005, 12, 19–29. [Google Scholar] [CrossRef]
- Jafar, S.A. Interference Alignment: A New Look at Signal Dimensions in a Communication Network; Now Publishers Inc.: Boston, MA, USA, 2011. [Google Scholar]
- Costa, M. Writing on dirty paper. IEEE Trans. Inf. Theory 1983, 29, 439–441. [Google Scholar] [CrossRef]
- Katti, S.; Gollakota, S.; Katabi, D. Embracing wireless interference: Analog network coding. ACM SIGCOMM Comput. Commun. Rev. 2007, 37, 397–408. [Google Scholar] [CrossRef]
- Popovski, P.; Yomo, H. Physical network coding in two-way wireless relay channels. In Proceedings of the 2007 IEEE International Conference on Communications, Glasgow, UK, 24–28 June 2007; pp. 707–712. [Google Scholar]
- Jain, M.; Choi, J.I.; Kim, T.; Bharadia, D.; Seth, S.; Srinivasan, K.; Levis, P.; Katti, S.; Sinha, P. Practical, real-time, full duplex wireless. In Proceedings of the 17th Annual International Conference on Mobile Computing and Networking, Las Vegas, NV, USA, 19–23 September 2011; pp. 301–312. [Google Scholar]
- Bharadia, D.; McMilin, E.; Katti, S. Full duplex radios. In Proceedings of the ACM SIGCOMM 2013 Conference on SIGCOMM, Hong Kong, China, 12–16 August 2013; pp. 375–386. [Google Scholar]
- Hong, S.; Brand, J.; Choi, J.I.; Jain, M.; Mehlman, J.; Katti, S.; Levis, P. Applications of self-interference cancellation in 5G and beyond. IEEE Commun. Mag. 2014, 52, 114–121. [Google Scholar] [CrossRef]
- Jiang, Z.; Zhou, M. Spread spectrum MAC protocol with dynamic rate and collision avoidance for mobile ad hoc network. IEEE Trans. Veh. Technol. 2007, 56, 3149–3158. [Google Scholar] [CrossRef]
- Zheng, M.; Lin, J.; Liang, W.; Yu, H. A priority-aware frequency domain polling MAC protocol for OFDMA-based networks in cyber-physical systems. IEEE/CAA J. Autom. Sin. 2015, 2, 412–421. [Google Scholar]
- Lv, S.; Wang, X.; Zhou, X. Scheduling under SINR Model in Ad Hoc Networks with successive interference cancellation. In Proceedings of the 2010 IEEE Global Telecommunications Conference GLOBECOM 2010, Miami, FL, USA, 6–10 December 2010; pp. 1–5. [Google Scholar]
- Lv, S.; Zhuang, W.; Wang, X.; Zhou, X. Scheduling in wireless ad hoc networks with successive interference cancellation. In Proceedings of the IEEE INFOCOM 2011, Shanghai, China, 10–15 April 2011; pp. 1287–1295. [Google Scholar]
- Lv, S.; Zhuang, W.; Wang, X.; Zhou, X. Link scheduling in wireless networks with successive interference cancellation. Comput. Netw. 2011, 55, 2929–2941. [Google Scholar] [CrossRef][Green Version]
- Mollanoori, M.; Ghaderi, M. Fair and efficient scheduling in wireless networks with successive interference cancellation. In Proceedings of the 2011 IEEE Wireless Communications and Networking Conference, Cancun, Mexico, 28–31 March 2011; pp. 221–226. [Google Scholar]
- Mollanoori, M.; Ghaderi, M. On the performance of successive interference cancellation in random access networks. In Proceedings of the 2012 9th Annual IEEE Communications Society Conference on Sensor, Mesh and Ad Hoc Communications and Networks (SECON), Seoul, Korea, 18–21 June 2012; pp. 461–469. [Google Scholar]
- Sadjadpour, H.R.; Wang, Z.; Garcia-Luna-Aceves, J.J. The capacity of wireless ad hoc networks with multipacket reception. IEEE Trans. Commun. 2010, 58, 600–610. [Google Scholar] [CrossRef]
- Blomer, J.; Jindal, N. Transmission capacity of wireless ad hoc networks: Successive interference cancellation vs. joint detection. In Proceedings of the IEEE International Conference on Communications (ICC), Dresden, Germany, 14–18 June 2009; pp. 1–5. [Google Scholar] [CrossRef]
- Weber, S.P.; Andrews, J.G.; Yang, X.; de Veciana, G. Transmission capacity of wireless ad hoc networks with successive interference cancellation. IEEE Trans. Inf. Theory 2007, 53, 2799–2814. [Google Scholar] [CrossRef]
- Weber, S.; Andrews, J.G.; Jindal, N. An overview of the transmission capacity of Wireless Networks. IEEE Trans. Commun. 2010, 58, 3593–3604. [Google Scholar] [CrossRef]
- Tong, L.; Zhao, Q.; Mergen, G. Multipacket reception in random access wireless networks: From signal processing to optimal medium access control. IEEE Commun. Mag. 2001, 39, 108–112. [Google Scholar] [CrossRef]
- Sato, N.; Fujii, T. A MAC protocol for multi-packet ad-hoc wireless network utilizing multi-antenna. In Proceedings of the 6th IEEE Consumer Communications and Networking Conference (CCNC), Las Vegas, NV, USA, 11–13 January 2009; pp. 1–5. [Google Scholar] [CrossRef]
- Celik, G.D.; Zussman, G.; Khan, W.F.; Modiano, E. MAC for networks with multipacket reception capability and spatially distributed nodes. IEEE Trans. Mob. Comput. 2010, 9, 226–240. [Google Scholar] [CrossRef]
- Lee, H.; Lim, C.C.; Choi, J. A refined MAC protocol with multipacket reception for wireless networks. Wirel. Commun. Mob. Comput. 2011, 11, 1275–1286. [Google Scholar] [CrossRef]
- Ghosh, A.; Mangalvedhe, N.; Ratasuk, R.; Mondal, B.; Cudak, M.; Visotsky, E.; Thomas, T.A.; Andrews, J.G.; Xia, P.; Jo, H.S.; et al. Heterogeneous cellular networks: From theory to practice. IEEE Commun. Mag. 2012, 50, 54–64. [Google Scholar] [CrossRef]
- Patel, P.; Holtzman, J. Analysis of a simple successive interference cancellation scheme in a DS/CDMA system. IEEE J. Sel. Areas Commun. 1994, 12, 796–807. [Google Scholar] [CrossRef]
- Andrews, J.G.; Meng, T.H. Optimum power control for successive interference cancellation with imperfect channel estimation. IEEE Trans. Wirel. Commun. 2003, 2, 375–383. [Google Scholar] [CrossRef]
- Halperin, D.; Anderson, T.; Wetherall, D. Taking the sting out of carrier sense: Interference cancellation for wireless LANs. In Proceedings of the 14th ACM International Conference on Mobile Computing and Networking (MOBICOM ’08), San Francisco, CA, USA, 14–19 September 2008; pp. 339–350. [Google Scholar]
- Lv, S.; Zhuang, W.; Wang, X.; Liu, C.; Hu, X.; Sun, Y.; Zhou, X. A performance study of CSMA in wireless networks with successive interference cancellation. In Proceedings of the 2012 IEEE International Conference on Communications (ICC), Ottawa, ON, Canada, 10–15 June 2012; pp. 466–471. [Google Scholar]
- Baccelli, F.; Błaszczyszyn, B. Stochastic Geometry and Wireless Networks, Volume I: Theory; Now Publishers Inc.: Boston, MA, USA, 2009; Volume 1. [Google Scholar]
- Haenggi, M.; Ganti, R.K. Interference in large wireless networks. Found. Trends Netw. 2009, 3, 127–248. [Google Scholar] [CrossRef]
- Jiang, H.; Wilford, P.A. A hierarchical modulation for upgrading digital broadcast systems. IEEE Trans. Broadcast. 2005, 51, 223–229. [Google Scholar] [CrossRef]
- Park, J.M.; Kim, S.L.; Choi, J. Hierarchically modulated network coding for asymmetric two-way relay systems. IEEE Trans. Veh. Technol. 2010, 59, 2179–2184. [Google Scholar] [CrossRef]
- Nguyen, H.Q.; Baccelli, F.; Kofman, D. A stochastic geometry analysis of dense IEEE 802.11 networks. In Proceedings of the IEEE INFOCOM 2007—26th IEEE International Conference on Computer Communications, Barcelona, Spain, 6–12 May 2007; pp. 1199–1207. [Google Scholar]
- Stoyan, D.; Kendall, W.; Mecke, J. Stochastic Geometry and Its Applications, 2nd ed.; Wiley: Hoboken, NJ, USA, 1995. [Google Scholar]
- Yuan, Y.X. Step-sizes for the gradient method. AMS IP Stud. Adv. Math. 2008, 42, 785. [Google Scholar]






| Paper | Aim | Proposed Solution | Pros | Cons |
|---|---|---|---|---|
| [22] | Concurrent transmissions, multipacket reception (MPR) at the receiver to combat interference | Conflict set graph for the interference with successive interference cancellation (SIC) | Improve MPR | Difficult to characterize the link interface under SINR model |
| [23,24] | Concurrent transmissions, MPR at the receiver to combat interference | Scheduling scheme to improve bound | Improve the bound | Not efficient scheme to achieve good scheduling |
| [25] | Concurrent transmissions, MPR at the receiver to combat interference | Fair uplink scheduling algorithm | Increase network throughput | Low density network |
| [26] | Design SIC-aware MAC | Novel SIC-aware MAC protocol | Increase throughput by 1.5 | Subjected to selfish behavior of users |
| [27] | Close the gap between throughput capacity in the physical model | Investigate network scaling law | Low power is attained to achieve higher throughput | Simplified the interference cancellation condition |
| [28] | Comparison on successive interference cancellation and Joint detection (JD) | Benefits of JD surpass those of SIC | JD provide significant outage benefit regardless of the SIR threshold | JD depend largely on good code schemes and more complicated hardware |
| [29] | Study the improvement of transmission capacity obtained with SIC | Closed-form upper and lower bounds for Transmission Capacity (TC) of ad hoc network with SIC receivers | It showed imperfections in the interference cancellation rapidly degrade its usefulness | The network density largely determines the efficacy of SIC |
| [30] | Summarize new analytical tools of TC (Survey paper) | Contributions developed metric for transmission capacity | Show improvement in transmission capacity | Limited information in MAC design issue |
| [31] | Examine interaction between the physical and medium access control layers | Cross-layer design between PHY and MAC | Quantify the effectiveness of signal processing on multipacket reception | Less practical |
| [32] | A MAC Protocol for Multi-Packet Ad-hoc Wireless Network Utilizing Multi-Antenna | A novel multi-antenna utilization scheme for avoiding the packet congestion | Improve throughput, decreases transmission delay in the relay node | Only works with multiple antennas |
| [33] | MAC for MPR | Markovian analysis was used to evaluate performance | Ability to capture few packets simultaneously | Can lead to unfairness when nodes are spatially distributed |
| [34] | Enable the coexistence utilize the MPR capability to maximize throughput | New MPR MAC protocol | Improves throughput | Spatially random topology is not considered |
| [35] | To construct a femtocell cluster sharing the same frequency band | Interference management scheme | Reduce cross-tier interference in the downlink | The performance of FUEs decreased |
| [36] | Analysis of IC scheme in a DS/CDMA system | Analyze a simple successive interference cancellation scheme for coherent BPSK modulation | Extend the analysis for a noncoherent modulation scheme | Limited consideration on analyzing the processing delay involved and the practical implementation of the interference canceler |
| [37] | Maximize the overall capacity to estimate error | Power control strategy | Power control is a key element in allowing a SIC system to achieve high performance in practice | Requires highly accurate estimates for the amplitude and phase of each user’s signal |
| [38] | High packet loss rate and poor spatial reuse | Algorithm for interference cancellation | Reduced packet loss and increase spatial reuse | Not fully combined IC into MAC |
| [39] | Performance study of CSMA | CSMA for single packet reception is designed | SIC provides many new transmission opportunities | Not suitable to combining IC capability |
| Notation | Description |
|---|---|
| path loss exponent, | |
| target SIR threshold | |
| communication distance | |
| carrier sensing distance | |
| strong interferer distance | |
| maximum cancellable distance | |
| a ball of radius r centered at origin | |
| an annulus between and | |
| interference at typical receiver from , | |
| area of region x | |
| number of points in region x | |
| node density | |
| transmitting node density | |
| additional transmitting node density | |
| a Poisson point process of intensity | |
| a set of points in | |
| euclidian distance between and origin | |
| transmission success probability | |
| transmission success probability with k-times interference cancellation |
| Distance | Type of Region |
|---|---|
| r > | Transmission allowed region (a) |
| < r < | Transmission restricted region (b) |
| r < | Transmission allowed region (c) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, D.M.; Kim, S.-L. CSNOMA: Carrier Sense Non-Orthogonal Multiple Access. Sensors 2020, 20, 5024. https://doi.org/10.3390/s20185024
Kim DM, Kim S-L. CSNOMA: Carrier Sense Non-Orthogonal Multiple Access. Sensors. 2020; 20(18):5024. https://doi.org/10.3390/s20185024
Chicago/Turabian StyleKim, Dong Min, and Seong-Lyun Kim. 2020. "CSNOMA: Carrier Sense Non-Orthogonal Multiple Access" Sensors 20, no. 18: 5024. https://doi.org/10.3390/s20185024
APA StyleKim, D. M., & Kim, S.-L. (2020). CSNOMA: Carrier Sense Non-Orthogonal Multiple Access. Sensors, 20(18), 5024. https://doi.org/10.3390/s20185024





