Effect of Loading Rate on Tensile and Failure Behavior of Concrete
Abstract
:1. Introduction
2. Experimental Setup
2.1. Test Specimens
2.2. Material Properties
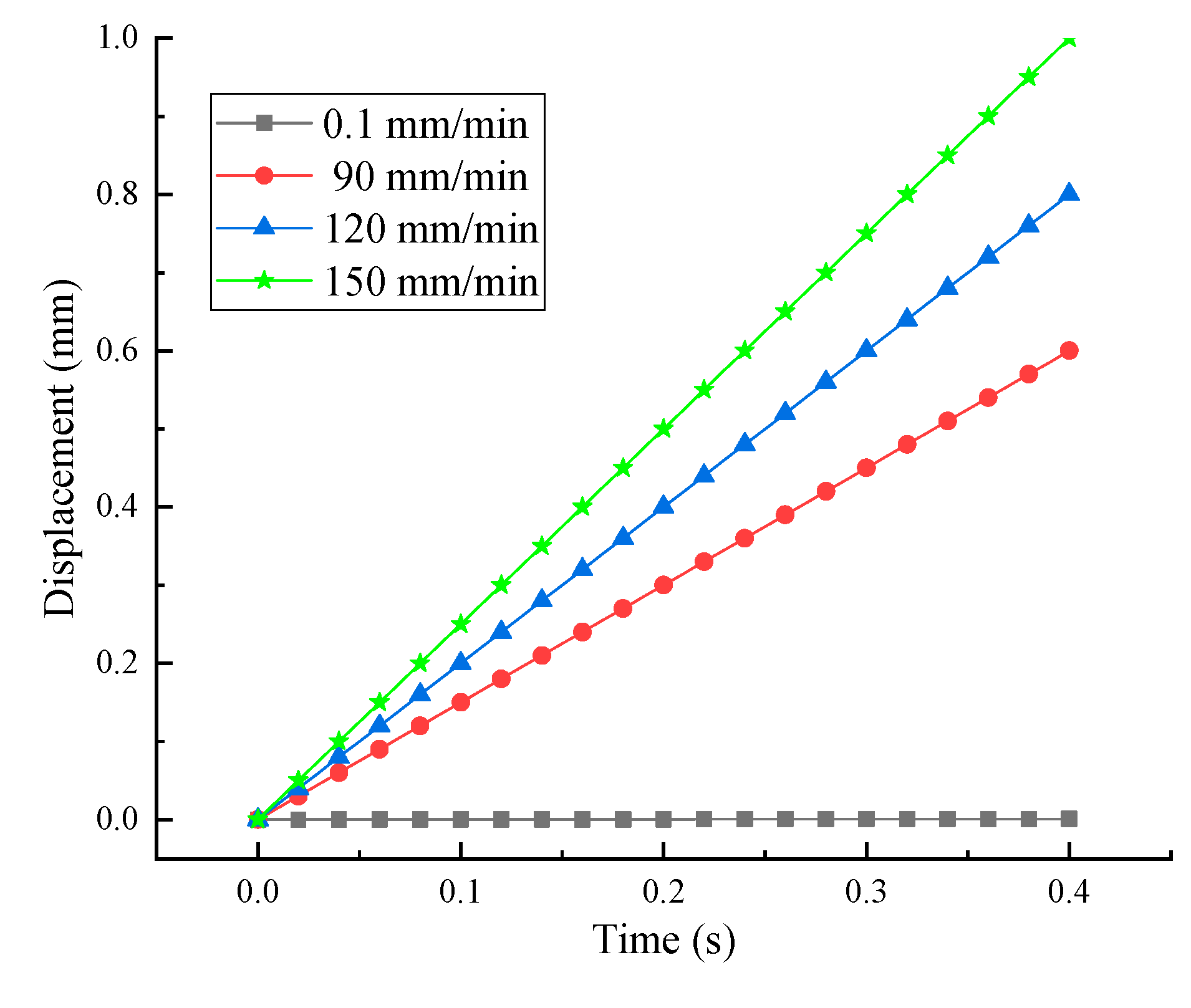
2.3. Loading and Measures
3. Experimental Results under Static Loading and Dynamic Loading
3.1. Static Loading Condition
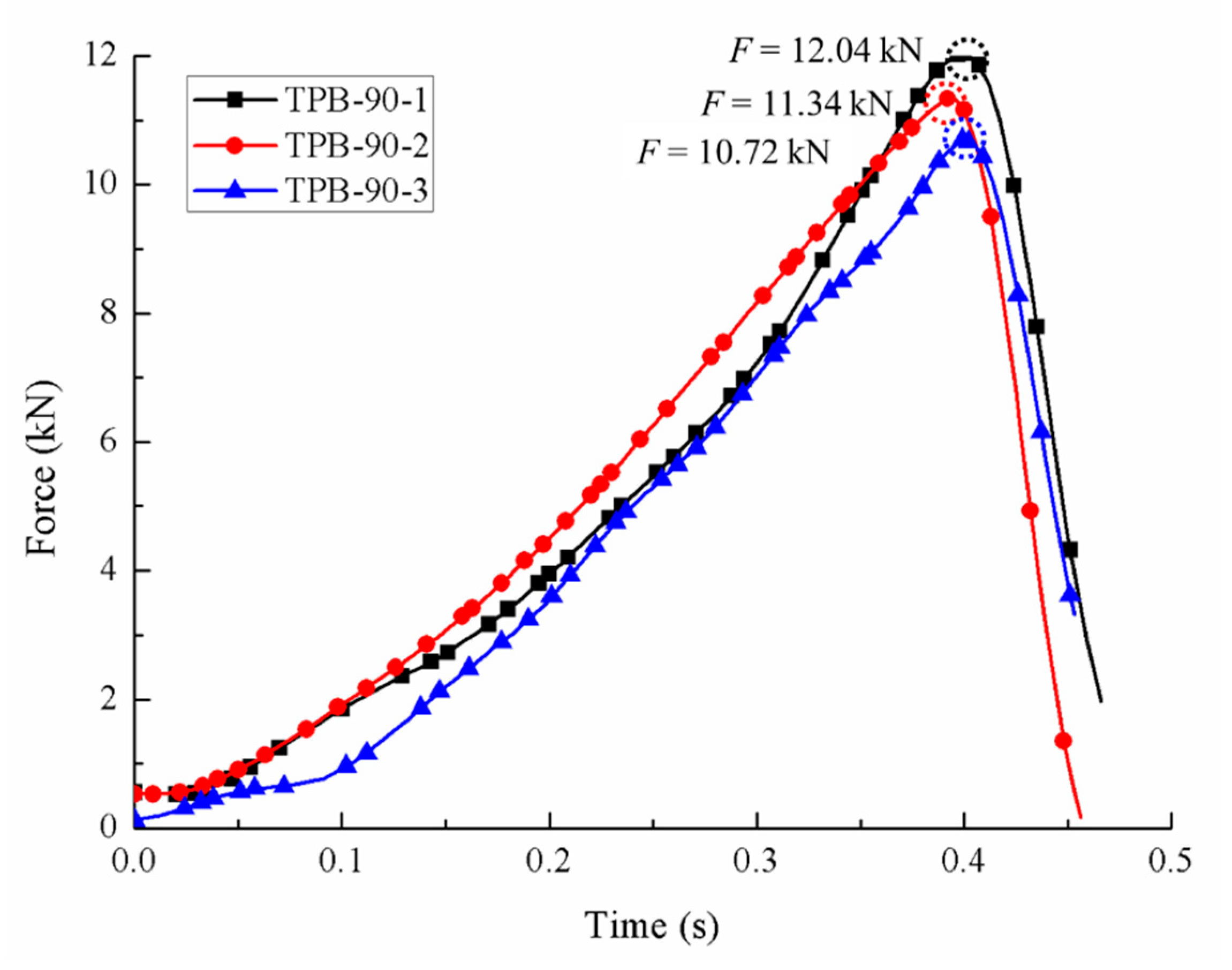
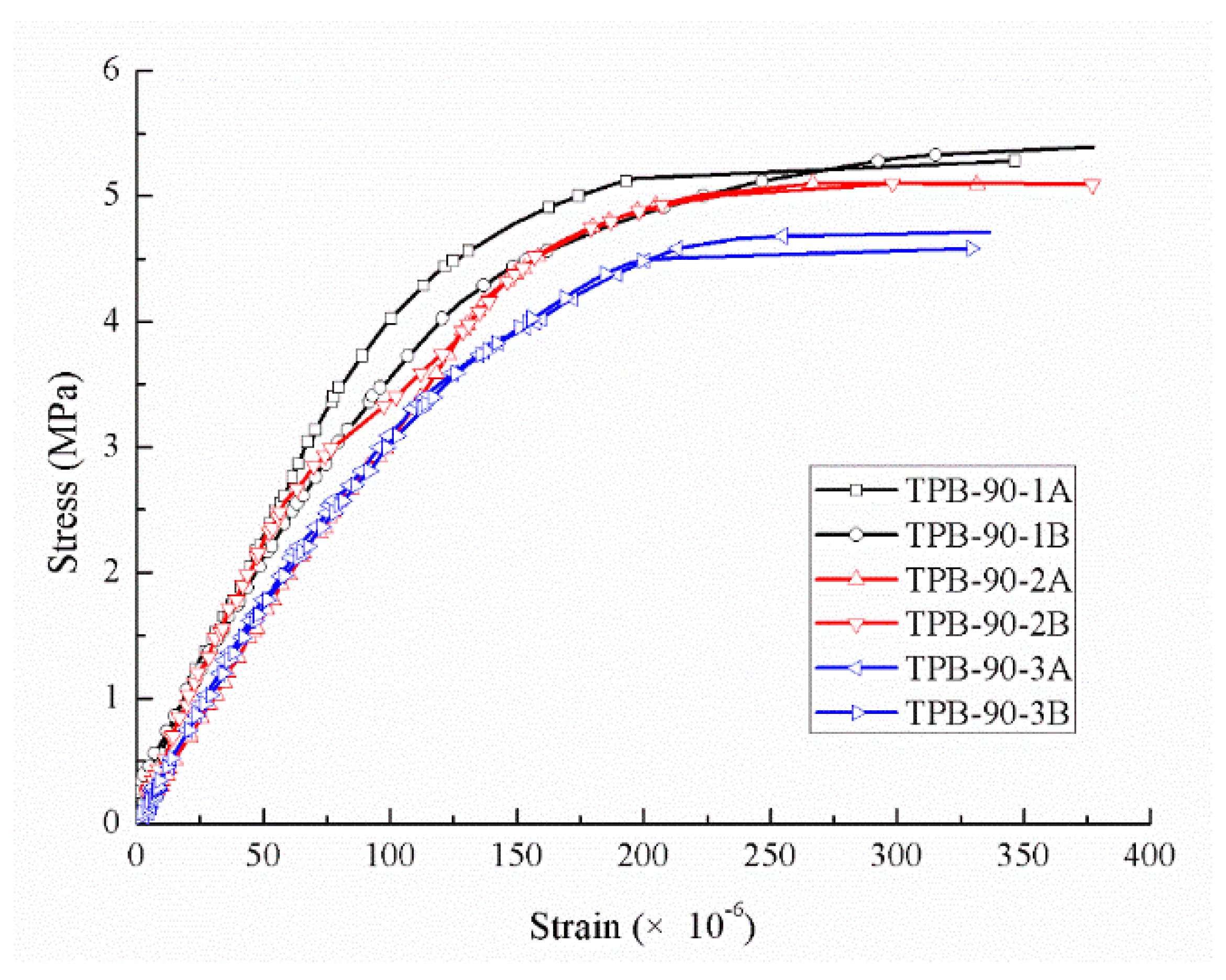
3.2. Dynamic Loading Condition
4. Results and Discussion
4.1. Dynamic Increase Factor
4.2. Effect of Aggregate on Dynamic Strength
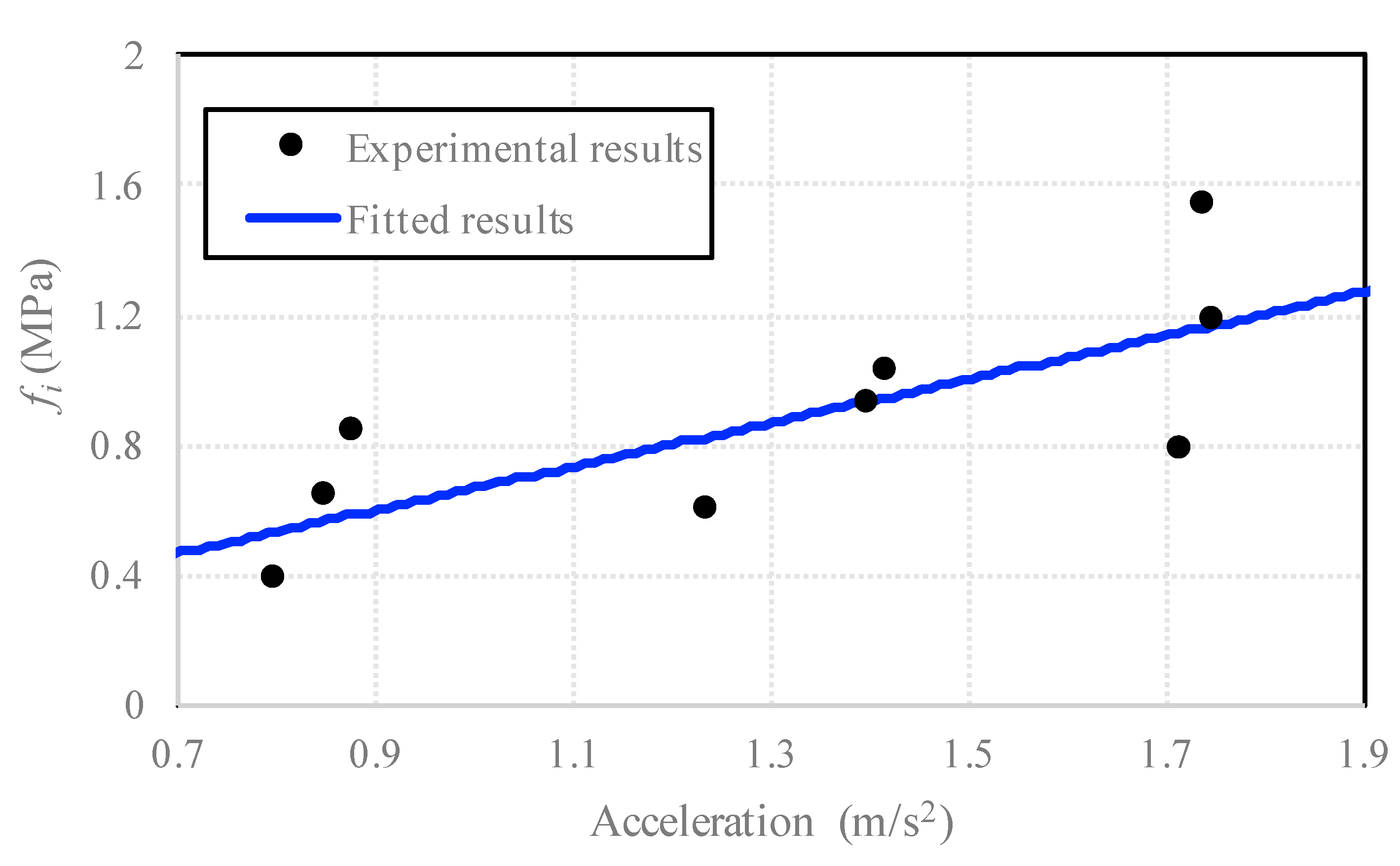
4.3. Effect of Inertia on Dynamic Strength
5. Numerical Simulation on the Mesoscopic Scale
6. Conclusions
- (1)
- The inherent inhomogeneity of the materials and the inertial effects were considered as the main factors responsible for the strength enhancement of the concrete. According to the experimental results of the stress-strain curves, the tensile strength and stiffness appeared distinctly sensitive to the strain rate.
- (2)
- A relation between DIF in the tension and strain rate was proposed to predict the increase in strength with the increasing strain rate, especially under the range of 10−6 s−1 and 1.5 × 10−3 s−1.
- (3)
- The aggregate played an important role in the dynamic strength enhancement; the higher the strain rate, the greater the destruction of the aggregates. The inertia effect was quantified and increased linearly with the acceleration.
- (4)
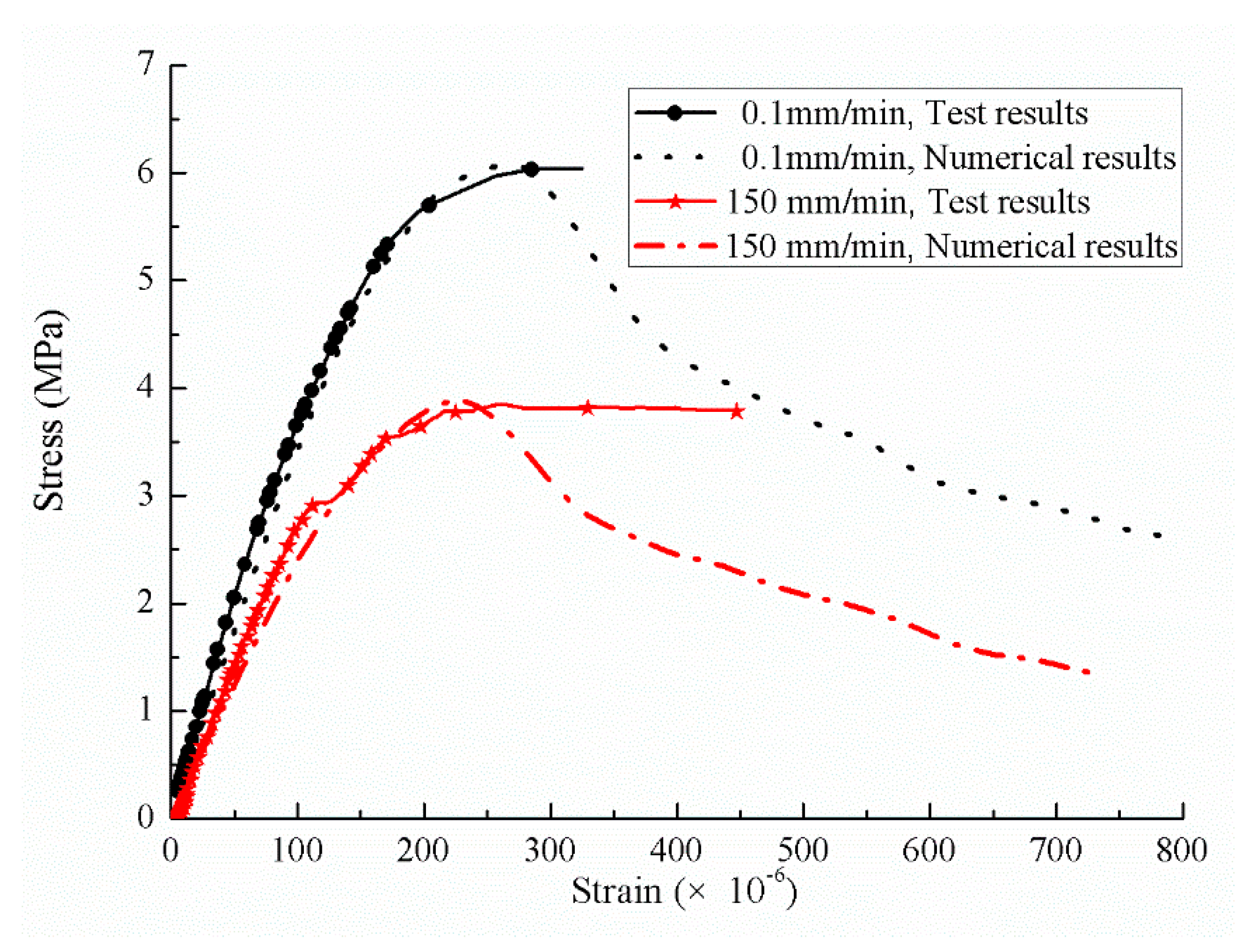
- The obtained experimental results were employed in the finite element analysis of the concrete beams, and the numerical results consisted well with the experimental results.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bischoff, P.H.; Perry, S.H. Impact behavior of plain concrete loaded in uniaxial compression. J. Eng. Mech. 1995, 121, 685–693. [Google Scholar] [CrossRef]
- Zhang, Y.; Xu, Y.; Zheng, Y.; Fernandez-Rodriguez, E.; Sun, A.; Yang, C.; Wang, J. Multi objective Optimization Design and Experimental Investigation on the Axial Flow Pump with Orthogonal Test Approach. Complexity 2019, 2019, 1–14. [Google Scholar] [CrossRef] [Green Version]
- Chen, X.; Wu, S.; Zhou, J. Experimental and modeling study of dynamic mechanical properties of cement paste, mortar and concrete. Constr. Build. Mater. 2013, 43, 419–430. [Google Scholar] [CrossRef]
- Bischoff, P.H.; Perry, S.H. Compressive behavior of concrete at high strain rates. Mater. Struct. 1991, 24, 425–450. [Google Scholar] [CrossRef]
- Mazars, J.; Millard, A. Dynamic Behavior of Concrete and Seismic Engineering; Wiley ISTE: London, UK, 2010; Volume 132, pp. 782–783. [Google Scholar]
- Abrams, D.A. Effect of rate of application of load on the compressive strength of concrete. ASTM J. 1917, 17, 70–78. [Google Scholar]
- Ross, C.A.; Tedesco, J.W.; Kuennen, S.T. Effects of strain rate on concrete strength. Mater. J. 1995, 92, 37–47. [Google Scholar]
- Guo, Y.; Gao, G.; Lin, J.; Shim, V.P.W. Quasi-static and Dynamic Splitting of High-strength Concretes-Tensile Stress-strain Response and Effects of Strain Rate. Int. J. Impact Eng. 2019, 125, 188–211. [Google Scholar] [CrossRef]
- Li, Q.; Meng, H. About the dynamic strength enhancement of concrete-like materials in a split Hopkinson pressure bar test. Int. J. Solids Struct. 2003, 40, 343–360. [Google Scholar] [CrossRef]
- Chen, J.J.; Guo, B.; Liu, H.; Liu, H.B. Dynamic Brazilian Test of Brittle Materials Using the Split Hopkinson Pressure Bar and Digital Image Correlation. Strain 2014, 50, 563–570. [Google Scholar] [CrossRef]
- Zhou, X.; Hao, H. Modelling of compressive behaviour of concrete-like materials at high strain rate. Int. J. Solids Struct. 2008, 45, 4648–4661. [Google Scholar] [CrossRef] [Green Version]
- Lv, T.; Chen, X.; Chen, G. Analysis on the waveform features of the split Hopkinson pressure bar tests of plain concrete specimen. Int. J. Impact Eng. 2017, 103, 107–123. [Google Scholar] [CrossRef]
- Zhao, H.; Gary, G. A new method for the separation of waves. Application to the SHPB technique for an unlimited duration of measurement. J. Mech. Phys. Solids 1997, 45, 1185–1202. [Google Scholar] [CrossRef]
- Reinhardt, H.W. Testing and monitoring techniques for impact and impulse loading of concrete structures. In Proceedings of the RILEM Symposium on Impact and Impulsive Loading of Concrete Structures, Berlin, Germany, 2–4 June 1982; Volume 1, pp. 65–88. [Google Scholar]
- Comité Euro-International du Béton. CEB-FIP Model Code 1990; Redwood Books: Wiltshire, UK, 1993. [Google Scholar]
- Malvar, L.J.; Ross, C.A. Review of strain rate effects for concrete in tension. Mater. J. 1998, 95, 735–739. [Google Scholar]
- Grote, D.L.; Park, S.W.; Zhou, M. Dynamic behavior of concrete at high strain rates and pressures: I. experimental characterization. Int. J. Impact Eng. 2001, 25, 869–886. [Google Scholar] [CrossRef]
- Brace, W.F.; Jones, A.H. Comparison of uniaxial deformation in shock and static loading of three rocks. J. Geophys. Res. 1971, 76, 4913–4921. [Google Scholar] [CrossRef]
- Qi, C.Z.; Qian, Q.H. Physical mechanism of dependence of material strength on strain rate for rock-like material. Chin. J. Rock Mech. Eng. 2003, 22, 177–181. [Google Scholar]
- Lu, Y.; Li, Q. About the dynamic uniaxial tensile strength of concrete-like materials. Int. J. Impact Eng. 2011, 38, 170–180. [Google Scholar] [CrossRef]
- Hentz, S.; Donze, F.V.; Daudeville, L. Discrete element modelling of concrete submitted to dynamic loading at high strain rates. Comput. Struct. 2004, 82, 2509–2524. [Google Scholar] [CrossRef]
- Kim, D.J.; Sirijaroonchai, K.; El-Tawil, S.; Naaman, A.E. Numerical simulation of the split hopkinson pressure bar test technique for concrete under compression. Int. J. Impact Eng. 2010, 37, 141–149. [Google Scholar] [CrossRef]
- Weerheijm, J. Concrete under Impact Tensile Loading and Lateral Compression. Ph.D. Thesis, Delft University, Delft, The Netherlands, 1992. [Google Scholar]
- Dang, F.; Pan, F.; Jiao, K.; Shi, J. Mechanism for enhancement of dynamic strength and failure model of nonuniform brittle materials. Earthq. Eng. Eng. Dyn. 2015, 35, 111–118. [Google Scholar]
- Li, W.; Mitchell, L. Laser scanning system testing-Errors and improvements. Measurement 1995, 16, 91–101. [Google Scholar] [CrossRef]
- Posiadala, B. Free vibrations of uniform Timoshenko beams with attachments. J. Sound Vib. 1997, 25, 155–160. [Google Scholar] [CrossRef]
- Betonbau, F. Fib Model Code for Concrete Structures 2010; Ernst & Sohn: Wollerau, Switzerland, 2013. [Google Scholar]
- Du, C.; Sun, L. Numerical simulation of concrete aggregates with arbitrary shapes and its application. J. Hydraul. Eng. 2006, 37, 662–667. [Google Scholar]
- Hao, O.; Chen, X. 3D meso scale modeling of concrete with a local background grid method. Constr. Build. Mater. 2020, 257, 119382. [Google Scholar]
- Lubliner, J.; Oliver, J.; Oller, S.; Oñate, E. A plastic-damage model for concrete. Int. J. Solids Struct. 1989, 25, 299–326. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G. Plastic-Damage Model for Cyclic Loading of Concrete Structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
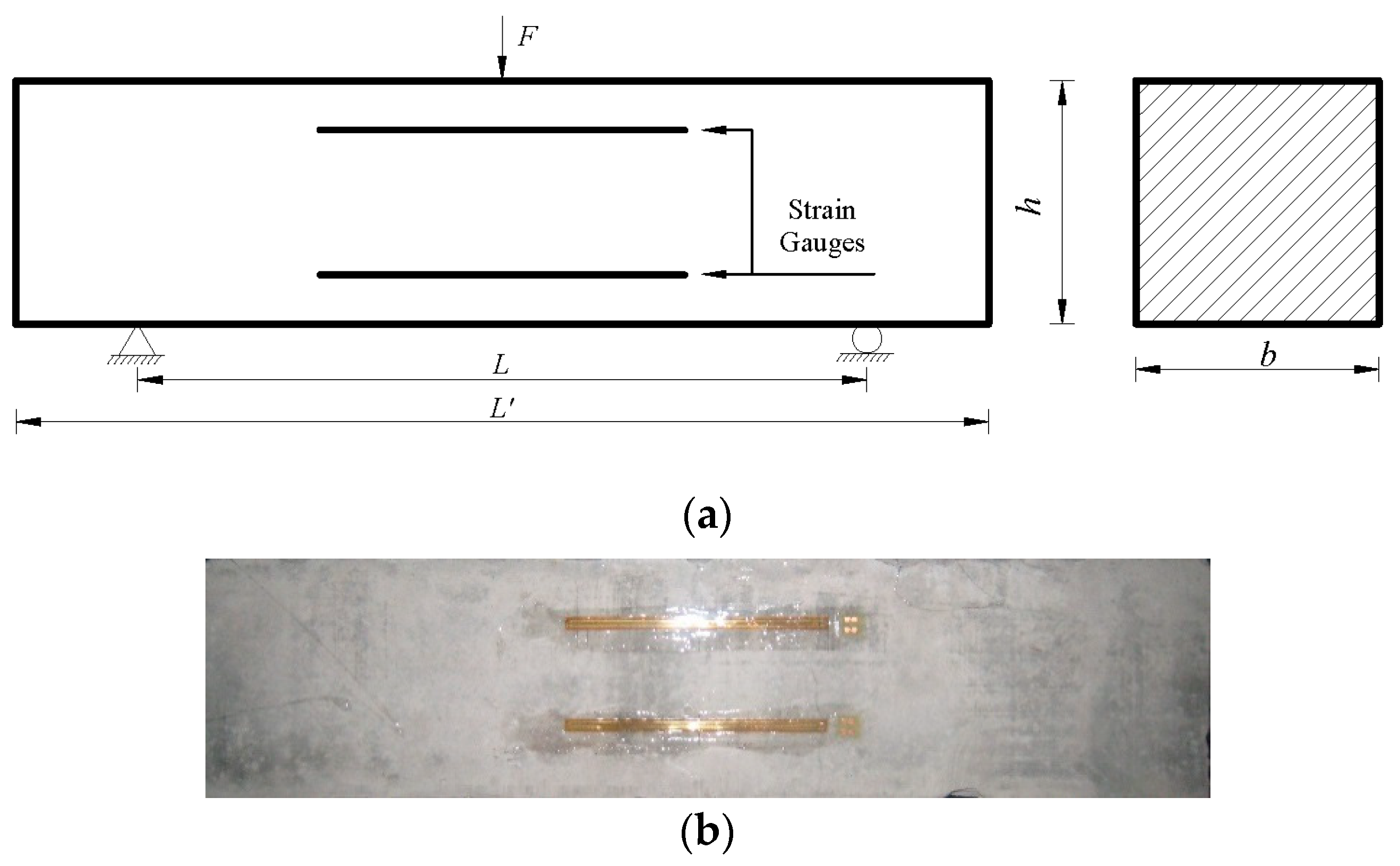













| Water | Cement | Sand | Aggregate (Representative Particle Size: 25 mm) |
|---|---|---|---|
| 129 | 430 | 482 | 1170 |
| Nominal Strength (MPa) | Compressive Strength (MPa) | Tensile Strength (MPa) | Young’s Modulus (MPa) | |||
|---|---|---|---|---|---|---|
| Sample Value | Representative Value | Sample Value | Representative Value | Sample Value | Representative value | |
| 30 | 30.6 | 30.2 | 3.56 | 3.54 | 36,400 | 35,900 |
| 28.9 | 3.48 | 35,200 | ||||
| 31.1 | 3.59 | 36,100 | ||||
| Specimen | Strain Rate (× 10−6 s−1) | Area (mm2) | Percentage of Area (%) | ||||
|---|---|---|---|---|---|---|---|
| Aggregate | Interface | Mortar | Aggregate | Interface | Mortar | ||
| TPB-0.1-1 | 25 | 1811.06 | 3992.07 | 5930.86 | 15.43 | 34.02 | 50.54 |
| TPB-0.1-2 | 28 | 1966.26 | 3712.09 | 6261.85 | 16.46 | 31.10 | 52.44 |
| TPB-0.1-3 | 31 | 2085.44 | 3984.22 | 6053.09 | 17.20 | 32.87 | 49.93 |
| Specimen | Strain Rate (× 10−6 s−1) | Area (mm2) | Percentage of Area (%) | Ultimate Load (kN) | ||||
|---|---|---|---|---|---|---|---|---|
| Aggregate | Interface | Mortar | Aggregate | Interface | Mortar | |||
| TPB-90-1 | 590 | 2346.18 | 3816.82 | 6221.44 | 18.94 | 30.82 | 50.24 | 12.04 |
| TPB-90-2 | 650 | 2140.36 | 3844.54 | 5898.61 | 18.01 | 32.35 | 49.64 | 11.34 |
| TPB-90-3 | 600 | 2158.75 | 3592.93 | 6421.50 | 17.73 | 29.52 | 52.75 | 10.72 |
| TPB-120-1 | 910 | 2589.93 | 2952.06 | 6776.41 | 21.02 | 23.96 | 55.01 | 12.97 |
| TPB-120-2 | 890 | 2392.52 | 3602.42 | 6134.63 | 19.72 | 29.70 | 50.58 | 12.72 |
| TPB-120-3 | 930 | 2239.58 | 3998.46 | 5163.36 | 18.98 | 34.73 | 46.29 | 11.51 |
| TPB-150-1 | 1140 | 2696.96 | 4221.81 | 5259.12 | 22.15 | 34.67 | 43.19 | 14.66 |
| TPB-150-2 | 1050 | 2355.97 | 2871.46 | 6068.22 | 20.86 | 25.42 | 53.72 | 13.40 |
| TPB-150-3 | 1150 | 2502.51 | 3662.71 | 4921.66 | 22.57 | 33.04 | 44.39 | 12.86 |
| Load Velocity (mm/min) | Experimental DIF | Strain Rate (× 10−6 s−1) | (MPa) | (MPa) | (m/s2) | (MPa) |
|---|---|---|---|---|---|---|
| 90 | 1.30 | 590 | 5.42 | 2.91 | 0.88 | 0.83 |
| 1.22 | 650 | 5.10 | 2.77 | 0.85 | 0.64 | |
| 1.15 | 600 | 4.82 | 2.73 | 0.80 | 0.39 | |
| 120 | 1.40 | 910 | 5.80 | 3.23 | 1.40 | 0.92 |
| 1.37 | 890 | 5.72 | 3.03 | 1.42 | 1.03 | |
| 1.24 | 930 | 5.18 | 2.92 | 1.24 | 0.60 | |
| 150 | 1.38 | 1140 | 6.60 | 3.41 | 1.72 | 0.79 |
| 1.44 | 1050 | 6.03 | 3.21 | 1.75 | 1.18 | |
| 1.58 | 1150 | 5.79 | 3.47 | 1.74 | 1.54 |
| Specimen | T1 | T2 | T3 | T4 | T5 | T6 | T7 | T8 | T9 | T10 | T11 | T12 |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Test | 0.92 | 0.97 | 1.11 | 1.15 | 1.22 | 1.24 | 1.30 | 1.37 | 1.38 | 1.40 | 1.44 | 1.58 |
| Model code 2010 | 1.06 | 1.06 | 1.05 | 1.29 | 1.12 | 1.13 | 1.12 | 1.13 | 1.14 | 1.13 | 1.13 | 1.13 |
| Malvar | 1.14 | 1.14 | 1.13 | 1.26 | 1.29 | 1.31 | 1.29 | 1.31 | 1.33 | 1.31 | 1.32 | 1.33 |
| This paper | 1.01 | 1.02 | 1.01 | 1.20 | 1.22 | 1.34 | 1.20 | 1.33 | 1.47 | 1.33 | 1.41 | 1.47 |
| Material | Young’s Modulus (MPa) | Poisson’s Ratio | Fracture Energy (N/m) | Tensile Strength (MPa) |
|---|---|---|---|---|
| Aggregate | 80 | 0.16 | 140.4 | 15.38 |
| Mortar | 30 | 0.22 | 71.3 | 2.16 |
| Interface | 22 | 0.16 | 46.8 | 1.73 |
| Concrete | 30 | 0.17 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Sun, L.; Zhao, W.; Zheng, Y. Effect of Loading Rate on Tensile and Failure Behavior of Concrete. Sensors 2020, 20, 5994. https://doi.org/10.3390/s20215994
Chen X, Sun L, Zhao W, Zheng Y. Effect of Loading Rate on Tensile and Failure Behavior of Concrete. Sensors. 2020; 20(21):5994. https://doi.org/10.3390/s20215994
Chicago/Turabian StyleChen, Xiaocui, Liguo Sun, Wenhu Zhao, and Yuan Zheng. 2020. "Effect of Loading Rate on Tensile and Failure Behavior of Concrete" Sensors 20, no. 21: 5994. https://doi.org/10.3390/s20215994
APA StyleChen, X., Sun, L., Zhao, W., & Zheng, Y. (2020). Effect of Loading Rate on Tensile and Failure Behavior of Concrete. Sensors, 20(21), 5994. https://doi.org/10.3390/s20215994





