Compact Double-Layer FR4-Based Focusing Lens Using High-Efficiency Huygens’ Metasurface Unit Cells
Abstract
1. Introduction
2. Huygens’ Metasurface Unit Cell Design
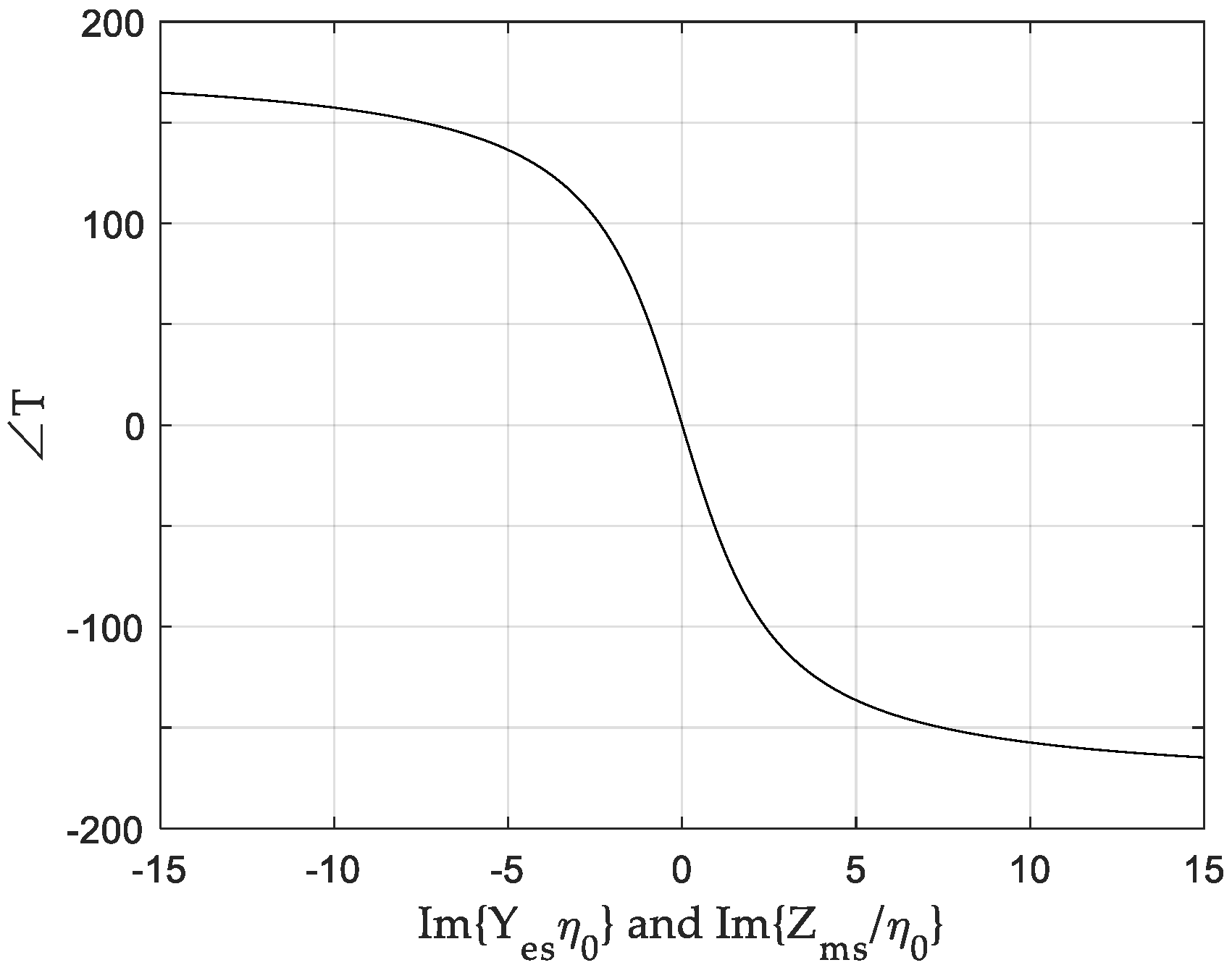
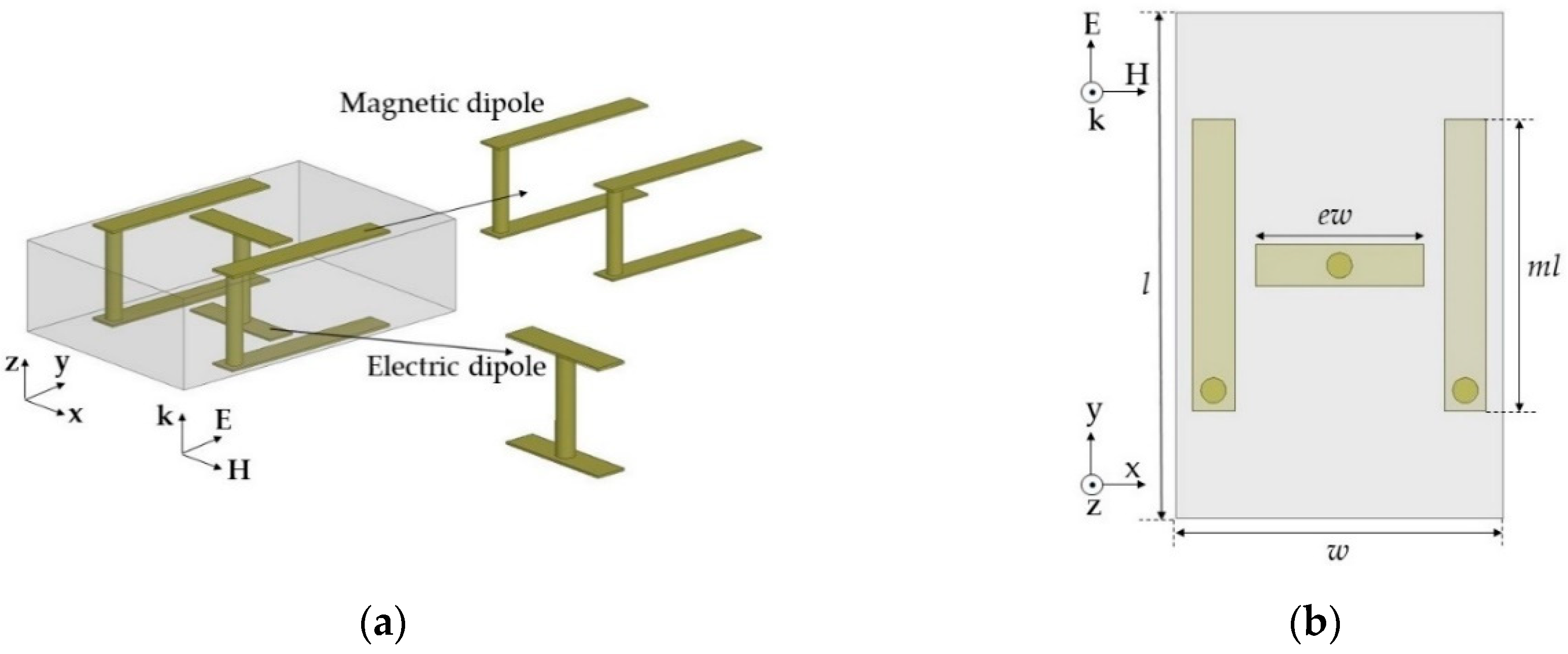
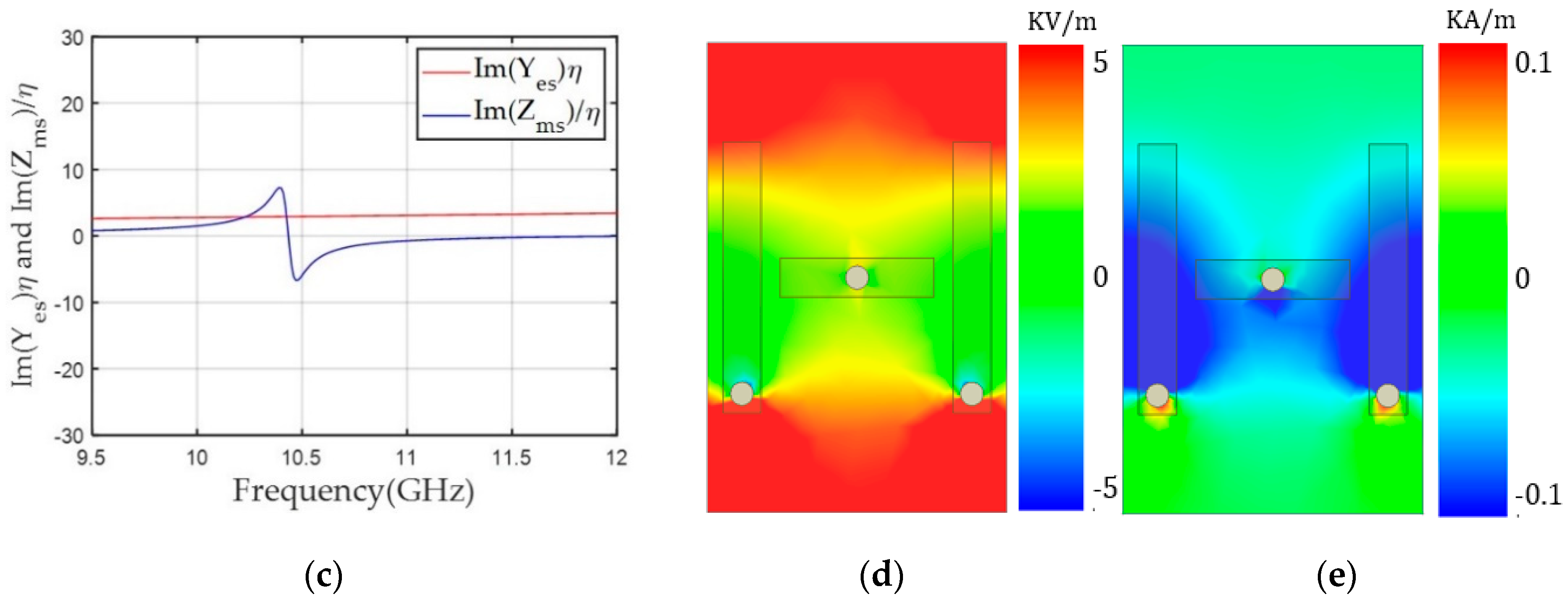
2.1. Unit Cell Design Method
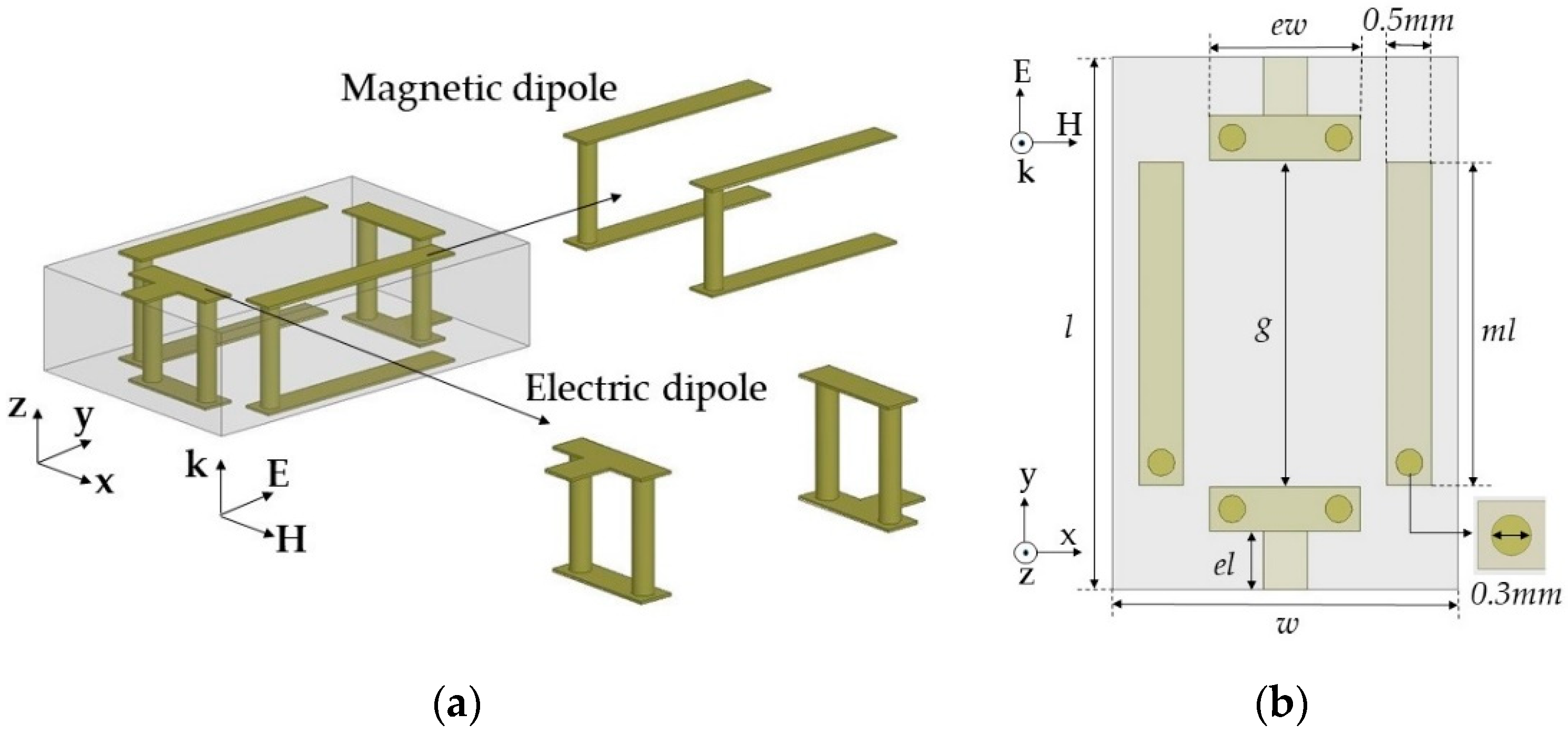
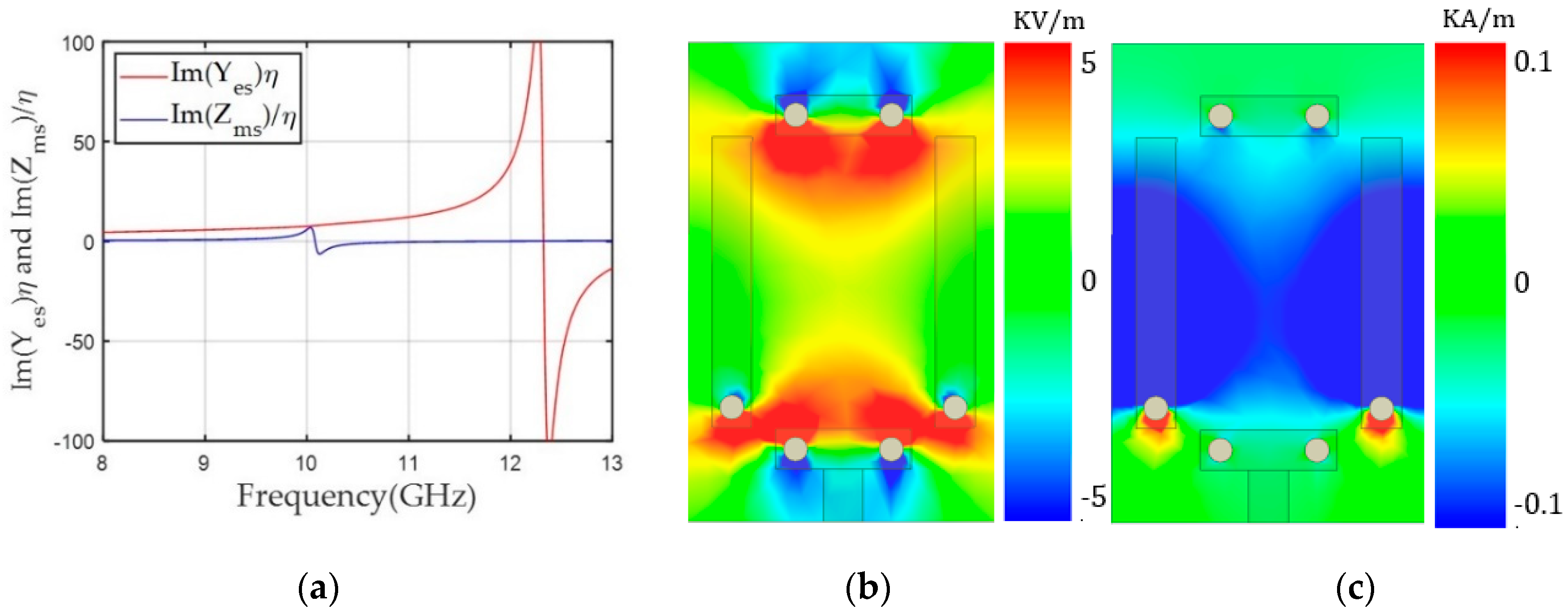
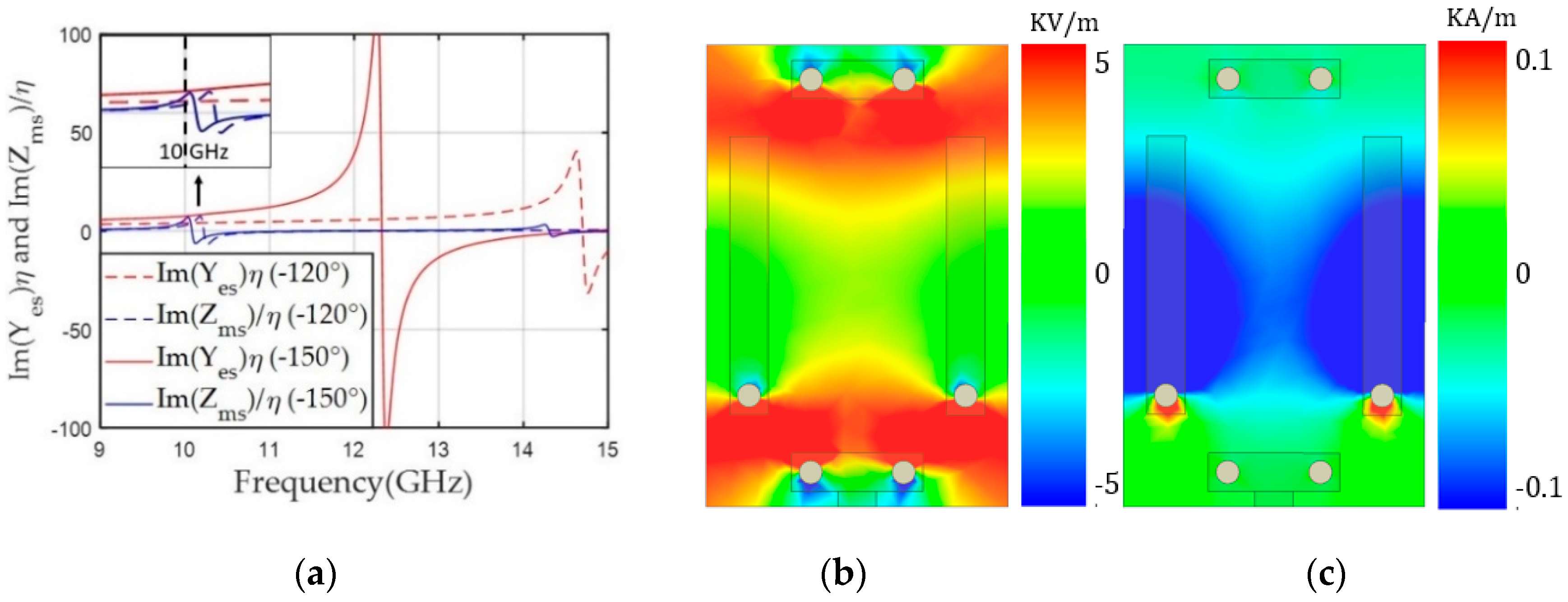
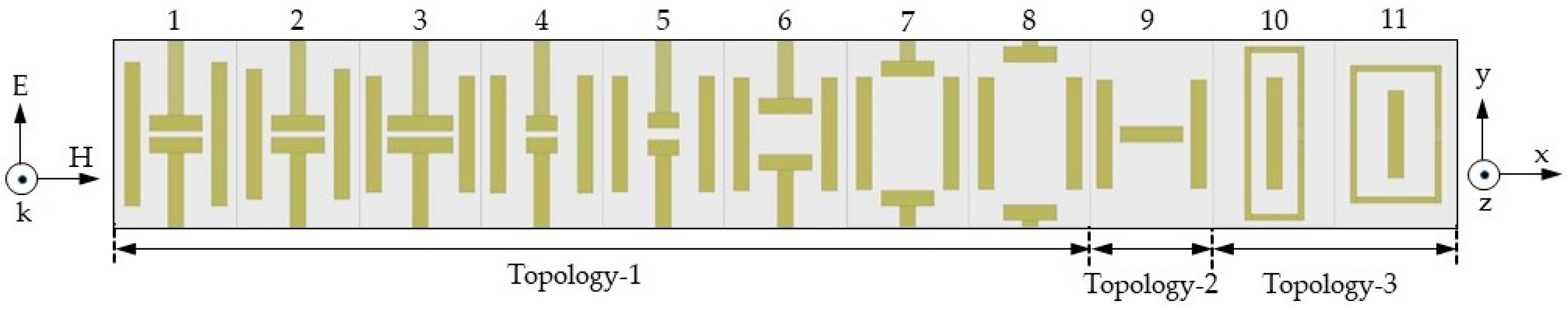
2.2. Unit Cell Topology Design and Analysis
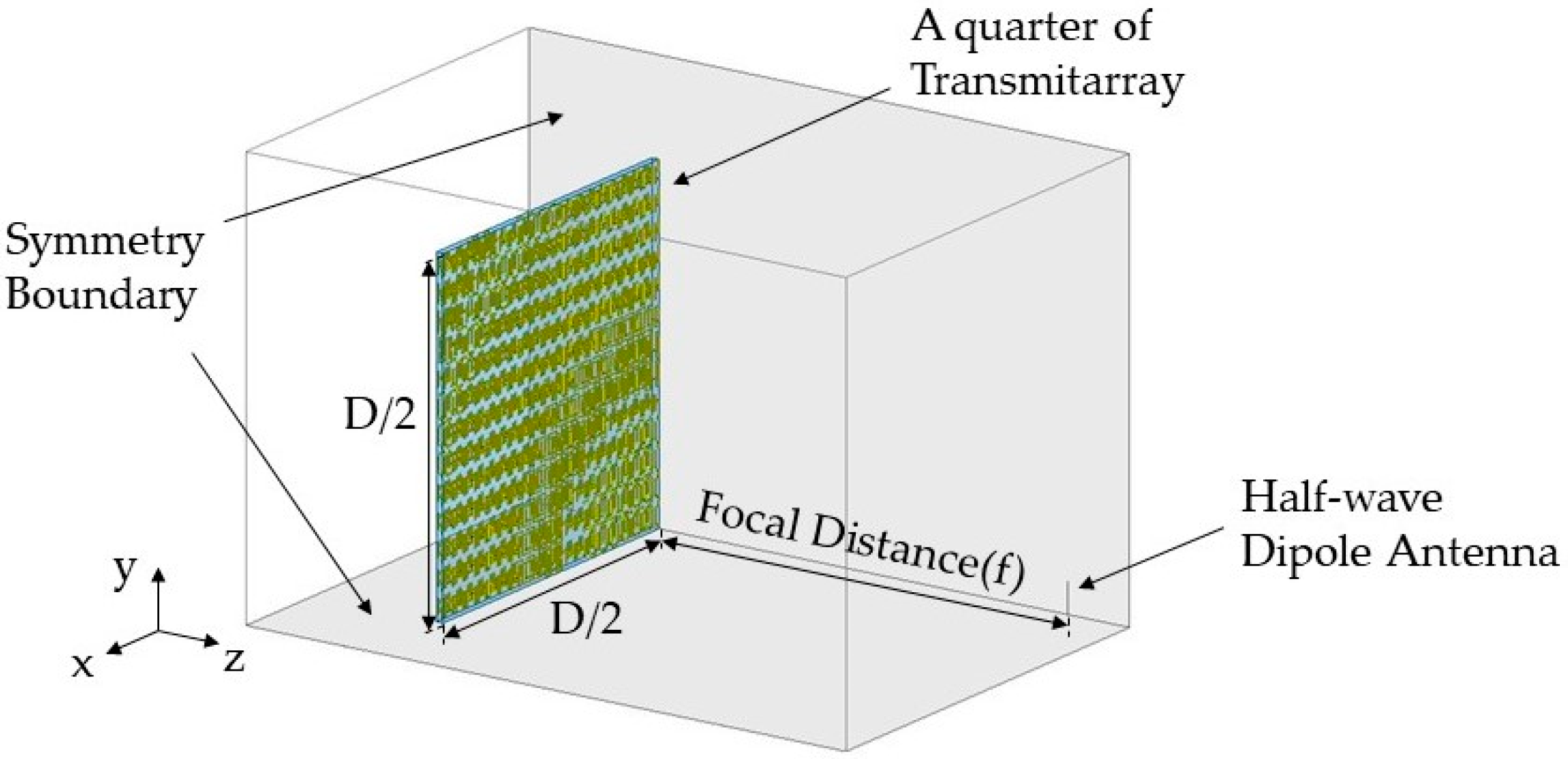
2.3. Focusing Lens Design
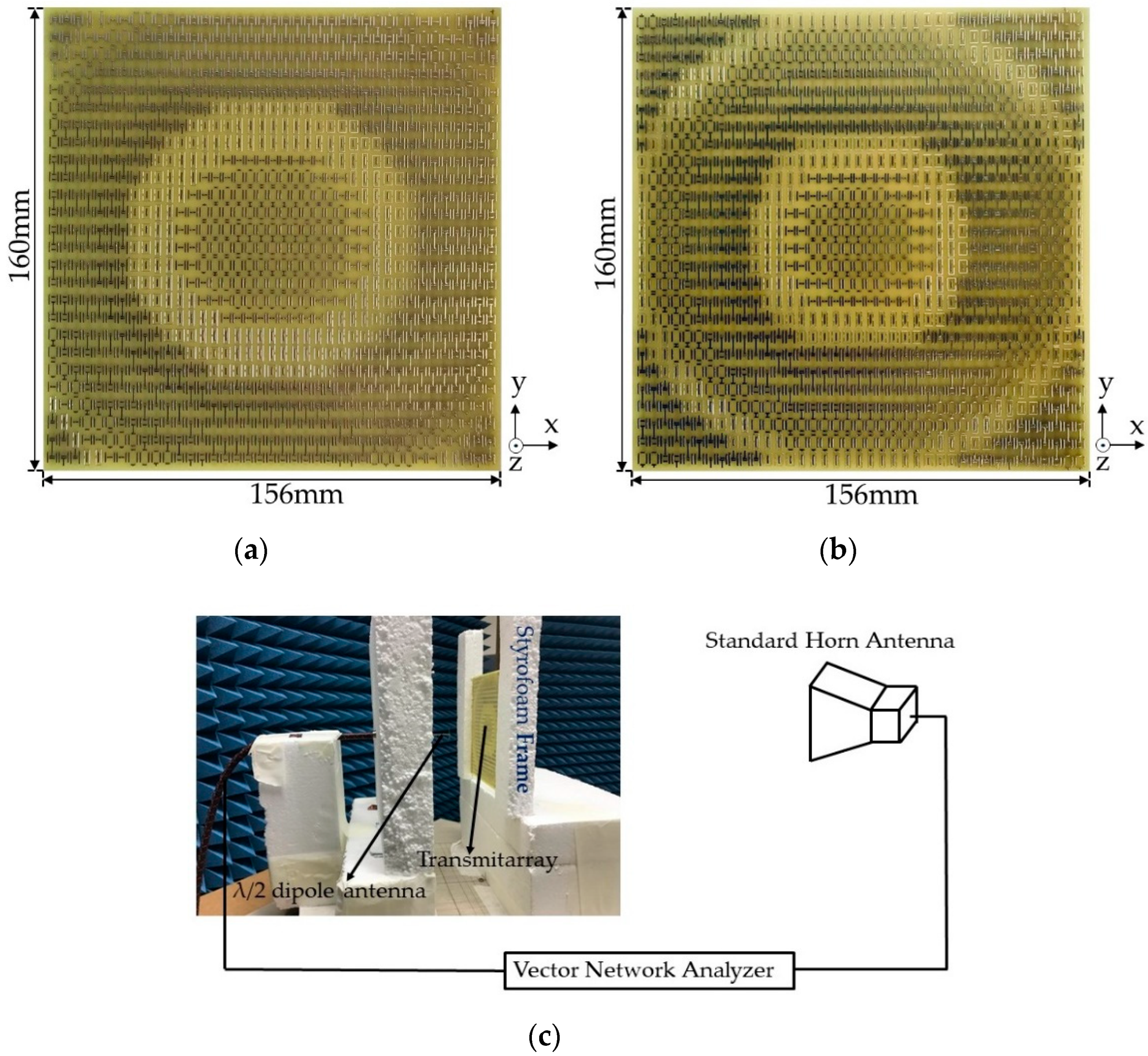
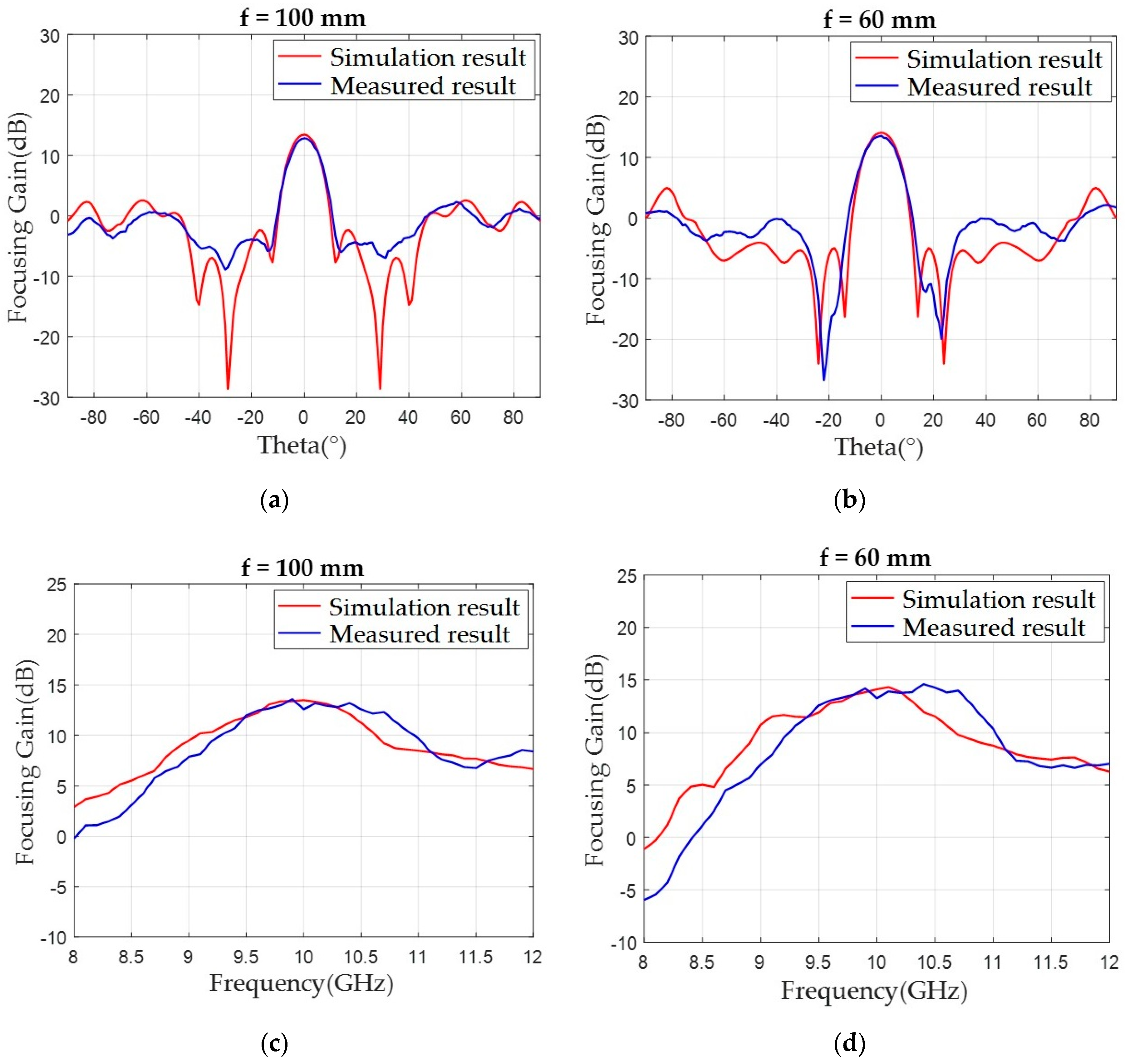
3. Fabrication and Measurement
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yu, N.; Genevet, P.; Kats, M.A.; Aieta, F.; Tetienne, J.-P.; Capasso, F.; Gaburro, Z. Light Propagation with Phase Discontinuities: Generalized Laws of Reflection and Refraction. Science 2011, 334, 333–337. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Grbic, A. Metamaterial Huygens’ surfaces: Tailoring wave fronts with reflectionless sheets. Phys. Rev. Lett. 2013, 110, 197401. [Google Scholar] [CrossRef] [PubMed]
- Yu, N.; Genevet, P.; Aieta, F.; Kats, M.A.; Blanchard, R.; Aoust, G.; Tetienne, J.-P.; Gaburro, Z.; Capasso, F. Flat Optics: Controlling Wavefronts with Optical Antenna Metasurfaces. IEEE J. Sel. Top. Quantum Electron. 2013, 19, 4700423. [Google Scholar] [CrossRef]
- Liu, S.; Cheng, Q.; Xu, Q.; Wang, T.Q.; Du, L.L.; Luan, K.; Xu, Y.H.; Bao, D.; Fu, X.J.; Han, J.G.; et al. Free-Standing Metasurfaces for High-Efficiency Transmitarrays for Controlling Terahertz Waves. Adv. Opt. Mater. 2016, 4, 384–390. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Emani, N.K.; Shaltout, A.M.; Boltasseva, A.; Shalaev, V.M.; Grbic, A. Efficient Light Bending with Isotropic Metamaterial Huygens’ Surfaces. Nano Lett. 2014, 14, 2491–2497. [Google Scholar] [CrossRef] [PubMed]
- Pfeiffer, C.; Grbic, A. Bianisotropic Metasurfaces for Optimal Polarization Control: Analysis and Synthesis. Phys. Rev. Appl. 2014, 2, 044011. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Grbic, A. Cascaded metasurfaces for complete phase and polarization control. Appl. Phys. Lett. 2013, 102, 231116. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Zhang, C.; Ray, V.; Guo, L.J.; Grbic, A. High Performance Bianisotropic Metasurfaces: Asymmetric Transmission of Light. Phys. Rev. Lett. 2014, 113, 023902. [Google Scholar] [CrossRef] [PubMed]
- Jia, S.L.; Wan, X.; Bao, D.; Zhao, Y.J.; Cui, T.J. Independent controls of orthogonally polarized transmitted waves using a Huygens metasurface. Laser Photonics Rev. 2015, 9, 545–553. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Grbic, A. Controlling Vector Bessel Beams with Metasurfaces. Phys. Rev. Appl. 2014, 2, 044012. [Google Scholar] [CrossRef]
- Pfeiffer, C.; Zhang, C.; Ray, V.; Guo, L.J.; Grbic, A. Polarization rotation with ultra-thin bianisotropic metasurfaces. Optica 2016, 3, 427. [Google Scholar] [CrossRef]
- Qin, F.; Wan, L.; Li, L.; Zhang, H.; Wei, G.; Gao, S. A Transmission Metasurface for Generating OAM Beams. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 1793–1796. [Google Scholar] [CrossRef]
- Jiang, S.; Chen, C.; Zhang, H.; Chen, W. Achromatic electromagnetic metasurface for generating a vortex wave with orbital angular momentum (OAM). Opt. Express 2018, 26, 6466. [Google Scholar] [CrossRef]
- Chen, M.L.N.; Jiang, L.J.; Sha, W.E.I. Ultrathin Complementary Metasurface for Orbital Angular Momentum Generation at Microwave Frequencies. IEEE Trans. Antennas Propag. 2017, 65, 396–400. [Google Scholar] [CrossRef]
- Zhang, K.; Wang, Y.; Yuan, Y.; Burokur, S.N. A Review of Orbital Angular Momentum Vortex Beams Generation: From Traditional Methods to Metasurfaces. Appl. Sci. 2020, 10, 1015. [Google Scholar] [CrossRef]
- Holloway, C.L.; Kuester, E.F.; Gordon, J.A.; O’Hara, J.; Booth, J.; Smith, D.R. An Overview of the Theory and Applications of Metasurfaces: The Two-Dimensional Equivalents of Metamaterials. IEEE Antennas Propag. Mag. 2012, 54, 10–35. [Google Scholar] [CrossRef]
- Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Planar Photonics with Metasurfaces. Science 2013, 339, 1232009. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.-T.; Taylor, A.J.; Yu, N. A review of metasurfaces: Physics and applications. Rep. Prog. Phys. 2016, 79, 076401. [Google Scholar] [CrossRef]
- Glybovski, S.B.; Tretyakov, S.A.; Belov, P.A.; Kivshar, Y.S.; Simovski, C.R. Metasurfaces: From microwaves to visible. Phys. Rep. 2016, 634, 1–72. [Google Scholar] [CrossRef]
- He, Q.; Sun, S.; Xiao, S.; Zhou, L. High-Efficiency Metasurfaces: Principles, Realizations, and Applications. Adv. Opt. Mater. 2018, 6, 1800415. [Google Scholar] [CrossRef]
- Azad, A.K.; Efimov, A.V.; Ghosh, S.; Singleton, J.; Taylor, A.J.; Chen, H.-T. Ultra-thin metasurface microwave flat lens for broadband applications. Appl. Phys. Lett. 2017, 110, 224101. [Google Scholar] [CrossRef] [PubMed]
- Lin, D.; Holsteen, A.L.; Maguid, E.; Fan, P.; Kik, P.G.; Hasman, E.; Brongersma, M.L. Polarization-independent metasurface lens employing the Pancharatnam-Berry phase. Opt. Express 2018, 26, 24835. [Google Scholar] [CrossRef]
- Ho, J.S.; Qiu, B.; Tanabe, Y.; Yeh, A.J.; Fan, S.; Poon, A.S.Y. Planar immersion lens with metasurfaces. Phys. Rev. B 2015, 91, 125145. [Google Scholar] [CrossRef]
- Epstein, A.; Eleftheriades, G.V. Arbitrary Antenna Arrays Without Feed Networks Based on Cavity-Excited Omega-Bianisotropic Metasurfaces. IEEE Trans. Antennas Propag. 2017, 65, 1749–1756. [Google Scholar] [CrossRef]
- Epstein, A.; Wong, J.P.; Eleftheriades, G.V. Cavity-excited Huygens’ metasurface antennas for near-unity aperture illumination efficiency from arbitrarily large apertures. Nat. Commun. 2016, 7, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Epstein, A.; Eleftheriades, G.V. Design and Experimental Verification of a Passive Huygens’ Metasurface Lens for Gain Enhancement of Frequency-Scanning Slotted-Waveguide Antennas. IEEE Trans. Antennas Propag. 2019, 67, 4678–4692. [Google Scholar] [CrossRef]
- Olk, A.E.; Powell, D.A. Huygens Metasurface Lens for W-Band Switched Beam Antenna Applications. IEEE Open J. Antennas Propag. 2020, 1, 290–299. [Google Scholar] [CrossRef]
- Wang, Z.; Ding, X.; Zhang, K.; Ratni, B.; Burokur, S.N.; Gu, X.; Wu, Q. Huygens Metasurface Holograms with the Modulation of Focal Energy Distribution. Adv. Opt. Mater. 2018, 6, 1800121. [Google Scholar] [CrossRef]
- Wan, W.; Gao, J.; Yang, X. Metasurface Holograms for Holographic Imaging. Adv. Opt. Mater. 2017, 5, 1700541. [Google Scholar] [CrossRef]
- Liu, G.; Wang, H.; Jiang, J.; Xue, F.; Yi, M. A High-Efficiency Transmitarray Antenna Using Double Split Ring Slot Elements. IEEE Antennas Wirel. Propag. Lett. 2015, 14, 1415–1418. [Google Scholar] [CrossRef]
- Li, H.; Wang, G.; Liang, J.; Gao, X.; Hou, H.; Jia, X. Single-Layer Focusing Gradient Metasurface for Ultrathin Planar Lens Antenna Application. IEEE Trans. Antennas Propag. 2017, 65, 1452–1457. [Google Scholar] [CrossRef]
- Wu, L.W.; Ma, H.F.; Gou, Y.; Wu, R.Y.; Wang, Z.X.; Wang, M.; Gao, X.; Cui, T.J. High-transmission ultrathin huygens’ metasurface with 360 phase control by using double-layer transmitarray elements. Phys. Rev. Appl. 2019, 12, 024012. [Google Scholar] [CrossRef]
- Xue, C.; Lou, Q.; Chen, Z.N. Broadband Double-Layered Huygens’ Metasurface Lens Antenna for 5G Millimeter-Wave Systems. IEEE Trans. Antennas Propag. 2020, 68, 1468–1476. [Google Scholar] [CrossRef]
- Kou, N.; Yu, S.; Ding, Z.; Zhang, Z. One-Dimensional Beam Scanning Transmitarray Lens Antenna Fed by Microstrip Linear Array. IEEE Access 2019, 7, 90731–90740. [Google Scholar] [CrossRef]
- An, W.; Xu, S.; Yang, F.; Li, M. A Double-Layer Transmitarray Antenna Using Malta Crosses with Vias. IEEE Trans. Antennas Propag. 2016, 64, 1120–1125. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, L.; Yu, J.-F.; Shi, X.-W. A C-Band Flat Lens Antenna with Double-Ring Slot Elements. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 341–344. [Google Scholar] [CrossRef]
- Xue, C.; Sun, J.; Niu, L.; Lou, Q. Ultrathin Dual-Polarized Huygens’ Metasurface: Design and Application. Ann. Phys. 2020, 532, 2000151. [Google Scholar] [CrossRef]
- Wong, J.P.; Selvanayagam, M.; Eleftheriades, G.V. Design of unit cells and demonstration of methods for synthesizing Huygens metasurfaces. Photonics Nanostruct.-Fundam. Appl. 2014, 12, 360–375. [Google Scholar] [CrossRef]
- Wong, J.P.S.; Selvanayagam, M.; Eleftheriades, G.V. Polarization Considerations for Scalar Huygens Metasurfaces and Characterization for 2-D Refraction. IEEE Trans. Microw. Theory Tech. 2015, 63, 913–924. [Google Scholar] [CrossRef]
- Wan, X.; Jia, S.L.; Cui, T.J.; Zhao, Y.J. Independent modulations of the transmission amplitudes and phases by using Huygens metasurfaces. Sci. Rep. 2016, 6, 25639. [Google Scholar] [CrossRef]
- Epstein, A.; Eleftheriades, G.V. Arbitrary power-conserving field transformations with passive lossless omega-type bianisotropic metasurfaces. IEEE Trans. Antennas Propag. 2016, 64, 3880–3895. [Google Scholar] [CrossRef]
- Chen, M.; Abdo-Sánchez, E.; Epstein, A.; Eleftheriades, G.V. Theory, design, and experimental verification of a reflectionless bianisotropic Huygens’ metasurface for wide-angle refraction. Phys. Rev. B 2018, 97, 125433. [Google Scholar] [CrossRef]
- Jouanlanne, C.; Clemente, A.; Huchard, M.; Keignart, J.; Barbier, C.; Le Nadan, T.; Petit, L. Wideband Linearly Polarized Transmitarray Antenna for 60 GHz Backhauling. IEEE Trans. Antennas Propag. 2017, 65, 1440–1445. [Google Scholar] [CrossRef]
- Luo, Q.; Gao, S.; Sobhy, M.; Yang, X. Wideband Transmitarray with Reduced Profile. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 450–453. [Google Scholar] [CrossRef]
- Holloway, C.L.; Mohamed, M.A.; Kuester, E.F.; Dienstfrey, A. Reflection and transmission properties of a metafilm: With an application to a controllable surface composed of resonant particles. IEEE Trans. Electromagn. Compat. 2005, 47, 853–865. [Google Scholar] [CrossRef]
- Epstein, A.; Eleftheriades, G.V. Huygens’ metasurfaces via the equivalence principle: Design and applications. J. Opt. Soc. Am. B 2016, 33, A31. [Google Scholar] [CrossRef]
- Al-Joumayly, M.A.; Behdad, N. Wideband Planar Microwave Lenses Using Sub-Wavelength Spatial Phase Shifters. IEEE Trans. Antennas Propag. 2011, 59, 4542–4552. [Google Scholar] [CrossRef]
- Li, M.; Behdad, N. Wideband True-Time-Delay Microwave Lenses Based on Metallo-Dielectric and All-Dielectric Lowpass Frequency Selective Surfaces. IEEE Trans. Antennas Propag. 2013, 61, 4109–4119. [Google Scholar] [CrossRef]
- Oh, J. Millimeter-Wave Thin Lens Employing Mixed-Order Elliptic Filter Arrays. IEEE Trans. Antennas Propag. 2016, 64, 3222–3227. [Google Scholar] [CrossRef]
- Wang, Z.X.; Wu, J.W.; Wan, X.; Wu, L.W.; Xiao, Q.; Cheng, Q.; Cui, T.J. Broadband and ultrathin Huygens metasurface with high transmittance. J. Phys. D Appl. Phys. 2020, 53, 455102. [Google Scholar] [CrossRef]






| Cell | Topology | g (mm) | g1 (mm) | g2 (mm) | ml (mm) | ew (mm) | Im (Yes) | Im (Zms) | Trans. Phase (°) | Trans. Loss (dB) |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 1 | 0.20 | 4.60 | 1.7 | 0 | 0 | 0 | −0.4 | ||
| 2 | 1 | 0.20 | 4.15 | 1.7 | −1.1 | −0.2 | 30 | −1.1 | ||
| 3 | 1 | 0.20 | 3.72 | 2.1 | −1.5 | −0.9 | 60 | −1.0 | ||
| 4 | 1 | 0.20 | 3.76 | 1.0 | −2.4 | −1.7 | 90 | −1.0 | ||
| 5 | 1 | 0.37 | 3.70 | 1.0 | −3.5 | −3.3 | 120 | −1.1 | ||
| 6 | 1 | 1.30 | 3.61 | 1.7 | −7.19 | −7.15 | 150 | −1.1 | ||
| 7 | 1 | 3.68 | 3.64 | 1.7 | 7.55 | 5.87 | −150 | −0.96 | ||
| 8 | 1 | 4.60 | 3.61 | 1.7 | 3.9 | 2.8 | −120 | −0.72 | ||
| 9 | 2 | 3.47 | 2.0 | 2.8 | 1.4 | −90 | −0.72 | |||
| 10 | 3 | 0.5 | 0.8 | 3.6 | 1.4 | 0.88 | −60 | −0.59 | ||
| 11 | 3 | 1 | 0.6 | 2.8 | 0.7 | 0.4 | −30 | −0.34 |
| Ref. | Layer # | Freq. (GHz) | Loss Tangent | Max. Loss of Unit Cell (dB) | Lens Size (mm × mm) | Thickness (mm) | Bandwidth (3 dB/1 dB) | f/D | Focusing Gain (dB) |
|---|---|---|---|---|---|---|---|---|---|
| [35] | 2 | 20 | 0.0014 | −1.75 | 338 × 338 (22.5λ × 22.5λ) | 1.575 (λ/9.5) | -/5.9 | 1.24 | 14.9 1 |
| [37] | 2 | 28 | 0.0027 | −1.63 | 165 × 165 (15.4λ × 15.4λ) | 1.524 (λ/7.0) | 13.3/- | 0.95 | 16.4 |
| [33] | 2 | 26.2 | 0.001 | −1.56 | 171.6 × 171.6 (15λ × 15λ) | 1.5 (λ/7.6) | 15.7/- | 0.99 | 15.7 1 |
| [32] | 2 | 13 | 0.0037 | −2.5 | 328 × 328 (14.2λ × 14.2λ) | 0.762 (λ/30) | 3/- | 0.8 | 11.5 |
| [34] | 2 | 10 | 0.005 | −3 | 360 × 500 (12λ × 16.6λ) | 2 (λ/15) | 6/- | 0.3 | 7.65 |
| [50] | 3 | 10.2 | 0.004 | −2.75 | 376.5 × 376.5 (12.8λ × 12.8λ) | 1.1 (λ/26.7) | 9.8/- | 0.8 | 14.4 |
| [36] | 2 | 6 | - | −1.4 | 210 × 210 (4.2λ × 4.2λ) | 2 (λ/25) | 15/- | 0.8 | 9 |
| [31] | 2 | 10 | 0.001 | −1.4 | 104 × 104 (3.46λ × 3.46λ) | 3 (λ/10) | -/- | 0.29 | 8.2 |
| This Work | 2 | 10 | 0.008 | −1.1 | 156 × 160 (5.1λ × 5.2λ) | 1.6 (λ/18.8) | 20/10 | 0.65/0.39 | 12.87/13.58 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, K.M.R.; Choi, S. Compact Double-Layer FR4-Based Focusing Lens Using High-Efficiency Huygens’ Metasurface Unit Cells. Sensors 2020, 20, 6142. https://doi.org/10.3390/s20216142
Islam KMR, Choi S. Compact Double-Layer FR4-Based Focusing Lens Using High-Efficiency Huygens’ Metasurface Unit Cells. Sensors. 2020; 20(21):6142. https://doi.org/10.3390/s20216142
Chicago/Turabian StyleIslam, Kd M. Raziul, and Sangjo Choi. 2020. "Compact Double-Layer FR4-Based Focusing Lens Using High-Efficiency Huygens’ Metasurface Unit Cells" Sensors 20, no. 21: 6142. https://doi.org/10.3390/s20216142
APA StyleIslam, K. M. R., & Choi, S. (2020). Compact Double-Layer FR4-Based Focusing Lens Using High-Efficiency Huygens’ Metasurface Unit Cells. Sensors, 20(21), 6142. https://doi.org/10.3390/s20216142






