Three-Dimensional Imaging by Frequency-Comb Spectral Interferometry
Abstract
1. Introduction
2. Methods
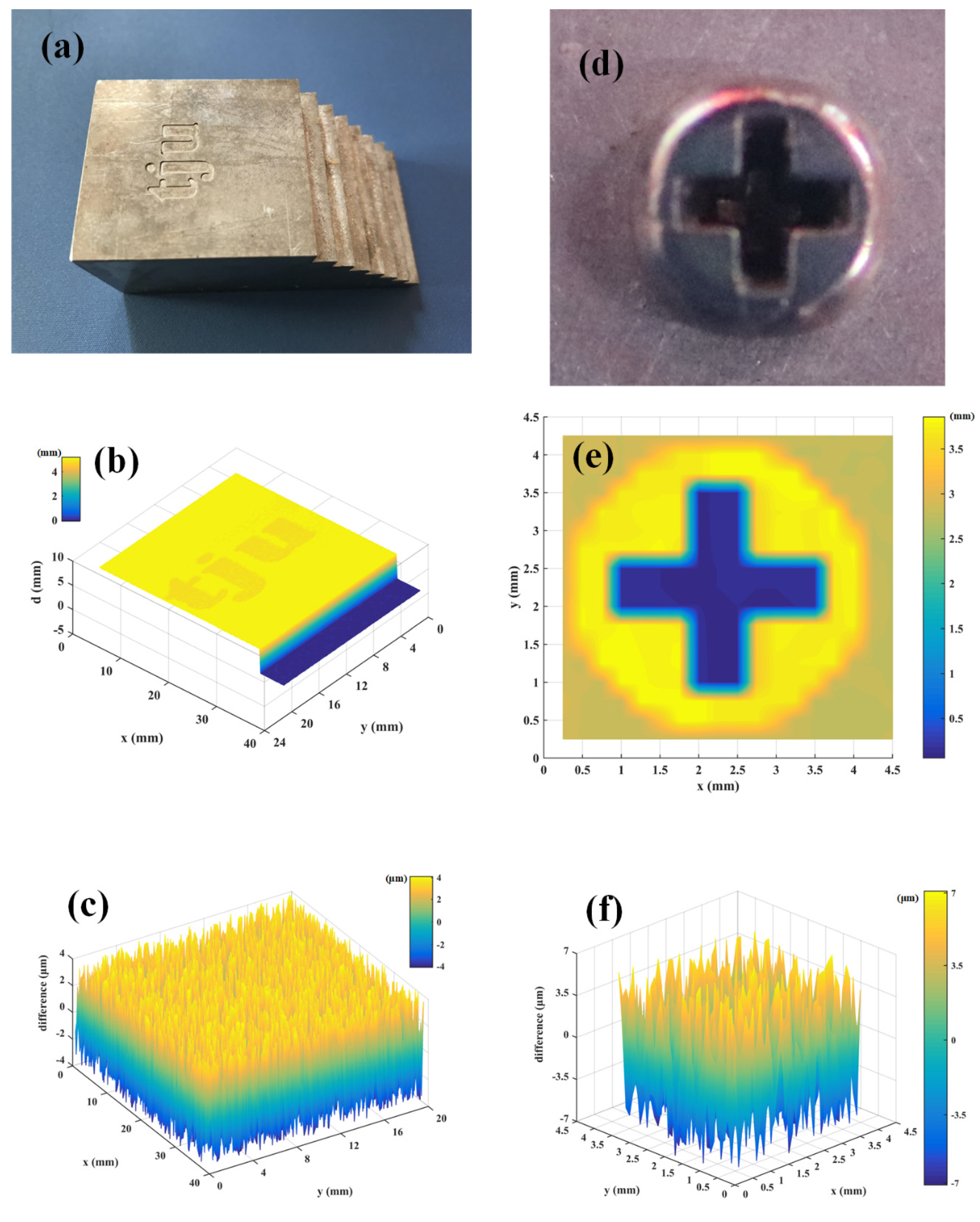
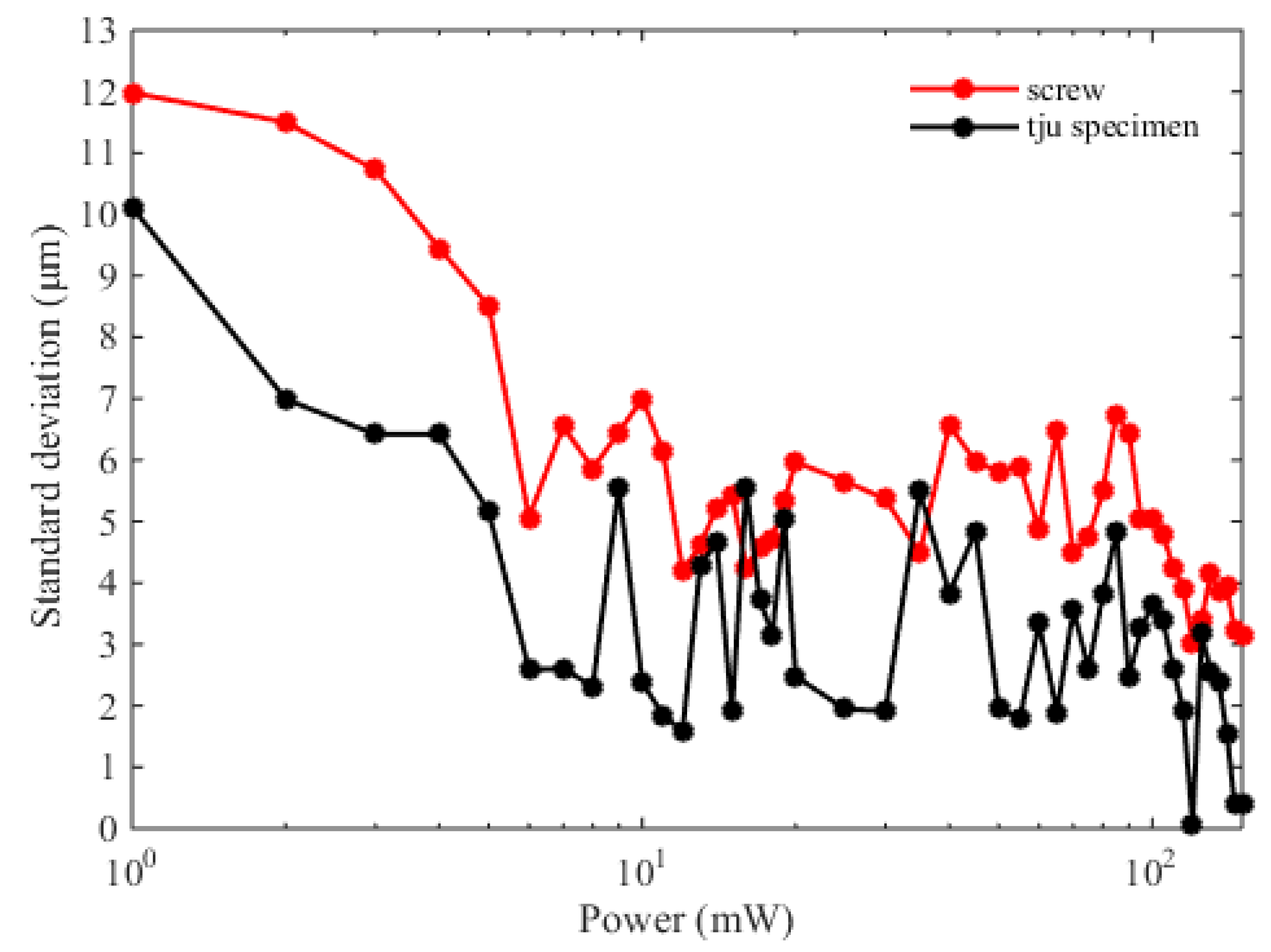
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Appendix A. Stabilization of the Repetition Frequency


References
- Newbury, N.R. Searching for applications with a fine-tooth comb. Nat. Photonics 2011, 5, 186–188. [Google Scholar] [CrossRef]
- John, L.H. Nobel Lecture: Defining and measuring optical frequencies. Rev. Mod. Phys. 2006, 78, 1279–1295. [Google Scholar]
- Theodor, W.H. Nobel Lecture: Passion for precision. Rev. Mod. Phys. 2006, 78, 1297–1309. [Google Scholar]
- Scott, A.D.; David, J.J.; Jun, Y.; Steven, T.C.; John, L.H.; Jinendra, K.R.; Robert, S.W.; Ronald, H.; Thomas, U.; Hänsch, T.W. Direct Link between Microwave and Optical Frequencies with a 300 THz Femtosecond Laser Comb. Phys. Rev. Lett. 2000, 84, 5102–5105. [Google Scholar]
- Nathalie, P.; Theodor, W.H. Frequency comb spectroscopy. Nat. Photonics 2019, 13, 146–157. [Google Scholar]
- Daryl, T.S.; Tara, D.; Travis, C.B.; Jordan, S.; Laura, C.S.; Connor, F.; Qing, L.; Daron, W.; Robert, I.B.; Aaron, B.; et al. An optical-frequency synthesizer using integrated photonics. Nature 2018, 557, 81–85. [Google Scholar]
- Victor, T.-C.; Jochen, S.; Mikael, M.; Lars, L.; Magnus, K.; Peter, A.A. Laser Frequency Combs for Coherent Optical Communications. J. Lightwave Technol. 2019, 37, 1663–1670. [Google Scholar]
- Wu, H.Z.; Zhao, T.; Wang, Z.Y.; Zhang, K.; Xue, B.; Li, J.S.; He, M.Z.; Qu, X.H. Long distance measurement up to 1.2 km by electro-optic dual-comb interferometry. Appl. Phys. Lett. 2017, 111, 251901. [Google Scholar]
- Zhang, J.; Zhu, J.; Deng, H.X.; Chai, Z.W.; Ma, M.; Zhong, X. Multi-camera calibration method based on a multi-plane stereo target. Appl. Opt. 2019, 58, 9353–9359. [Google Scholar] [CrossRef] [PubMed]
- Feng, X.G.; Zhu, J.Q.; Zhang, J.Y. The length error compensation method of articulated arm coordinate measuring machine. Rev. Sci. Instrum. 2019, 90, 105106. [Google Scholar] [CrossRef]
- Jia, L.C.; Xue, B.; Chen, S.L.; Wu, H.Z.; Yang, X.X.; Zhai, J.S.; Zeng, Z.M. A High-Resolution Ultrasonic Ranging System Using Laser Sensing and a Cross-Correlation Method. Appl. Sci. 2019, 9, 1483. [Google Scholar] [CrossRef]
- Petr, B.; Pavel, M.; Petr, K.; Miroslav, D. Length and refractive index measurement by Fourier transform interferometry and frequency comb spectroscopy. Meas. Sci. Technol. 2012, 23, 094001. [Google Scholar]
- Jonghan, J.; Young-Jin, K.; Yunseok, K.; Seung-Woo, K. Absolute length calibration of gauge blocks using optical comb of a femtosecond pulse laser. Opt. Express 2006, 14, 5968–5974. [Google Scholar]
- Woo-Deok, J.; Seungman, K.; Jiyong, P.; Keunwoo, L.; Joohyung, L.; Seungchul, K.; Young-Jin, K.; Seung-Woo, K. Femtosecond laser pulses for fast 3-D surface profilometry of microelectronic step-structures. Opt. Express 2013, 21, 15323. [Google Scholar]
- Baumann, E.; Giorgetta, F.R.; Deschênes, J.-D.; Swann, W.C.; Coddington, I.; Newbur, N.R. Comb-calibrated laser ranging for three-dimensional surface profiling with micrometer-level precision at a distance. Opt. Express 2014, 22, 24914. [Google Scholar] [CrossRef]
- Eric, W.M.; Matthew, S.H.; Fabrizio, R.G.; Torrey, H.; Gregory, B.R.; Nathan, R.N.; Esther, B. Coherent laser ranging for precision imaging through flames. Optica 2018, 5, 2334–2536. [Google Scholar]
- Weimann, C.; Messner, A.; Baumgartner, T.; Wolf, S.; Hoeller, F.; Freude, W.; Koos, C. Fast high-precision distance metrology using a pair of modulator-generated dual-color frequency combs. Opt. Express 2018, 26, 34305. [Google Scholar] [CrossRef]
- Weimann, C.; Lauermann, M.; Hoeller, F.; Freude, W.; Koos, C. Silicon photonic integrated circuit for fast and precise dual-comb distance metrology. Opt. Express 2017, 25, 30091. [Google Scholar] [CrossRef]
- Trocha, P.; Karpov, M.; Ganin, D.; Pfeiffer, M.H.P.; Kordts, A.; Wolf, S.; Krockenberger, J.; Marin-Palomo, P.; Weimann, C.; Randel, S.; et al. Ultrafast optical ranging using microresonator soliton frequency combs. Science 2018, 359, 887–891. [Google Scholar] [CrossRef]
- Yang, L.; Duan, L.Z. Depth-Resolved Imaging Based on Optical Sampling by Cavity Tuning. IEEE Photonic Technol. Lett. 2015, 27, 1761–1764. [Google Scholar] [CrossRef]
- Takashi, K.; Megumi, U.; Kaoru, M. No-scanning 3D measurement method using ultrafast dimensional conversion with a chirped optical frequency com. Sci. Rep. 2017, 7, 3670. [Google Scholar]
- Kaoru, M.; Hirokazu, M.; Zhang, Z.G.; Takashi, Y. Simultaneous 3-D Imaging Using Chirped Ultrashort Optical Pulses. Jpn. J. Appl. Phys. 1994, 33, 1348–1351. [Google Scholar]
- Ki-Nam, J.; Seung-Woo, K. Absolute distance measurement by dispersive interferometry using a femtosecond pulse laser. Opt. Express 2006, 14, 5954–5960. [Google Scholar]
- Philip, E.C. Refractive index of air: New equations for the visible and near infrared. Appl. Opt. 1996, 35, 1566–1573. [Google Scholar]
- Wu, G.H.; Xiong, S.L.; Ni, K.; Zhu, Z.B.; Zhou, Q. Parameter optimization of a dual-comb ranging system by using a numerical simulation method. Opt. Express 2015, 23, 32044–32053. [Google Scholar] [CrossRef]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Zhang, Z.; Xu, X.; Zhang, H.; Zhai, J.; Wu, H. Three-Dimensional Imaging by Frequency-Comb Spectral Interferometry. Sensors 2020, 20, 1743. https://doi.org/10.3390/s20061743
Zhao H, Zhang Z, Xu X, Zhang H, Zhai J, Wu H. Three-Dimensional Imaging by Frequency-Comb Spectral Interferometry. Sensors. 2020; 20(6):1743. https://doi.org/10.3390/s20061743
Chicago/Turabian StyleZhao, Haihan, Ziqiang Zhang, Xinyang Xu, Haoyun Zhang, Jingsheng Zhai, and Hanzhong Wu. 2020. "Three-Dimensional Imaging by Frequency-Comb Spectral Interferometry" Sensors 20, no. 6: 1743. https://doi.org/10.3390/s20061743
APA StyleZhao, H., Zhang, Z., Xu, X., Zhang, H., Zhai, J., & Wu, H. (2020). Three-Dimensional Imaging by Frequency-Comb Spectral Interferometry. Sensors, 20(6), 1743. https://doi.org/10.3390/s20061743



