Mechanical, Electrical, and Piezoresistive Sensing Characteristics of Epoxy-Based Composites Incorporating Hybridized Networks of Carbon Nanotubes, Graphene, Carbon Nanofibers, or Graphite Nanoplatelets
Abstract
:1. Introduction
2. Materials and Methods
3. Results
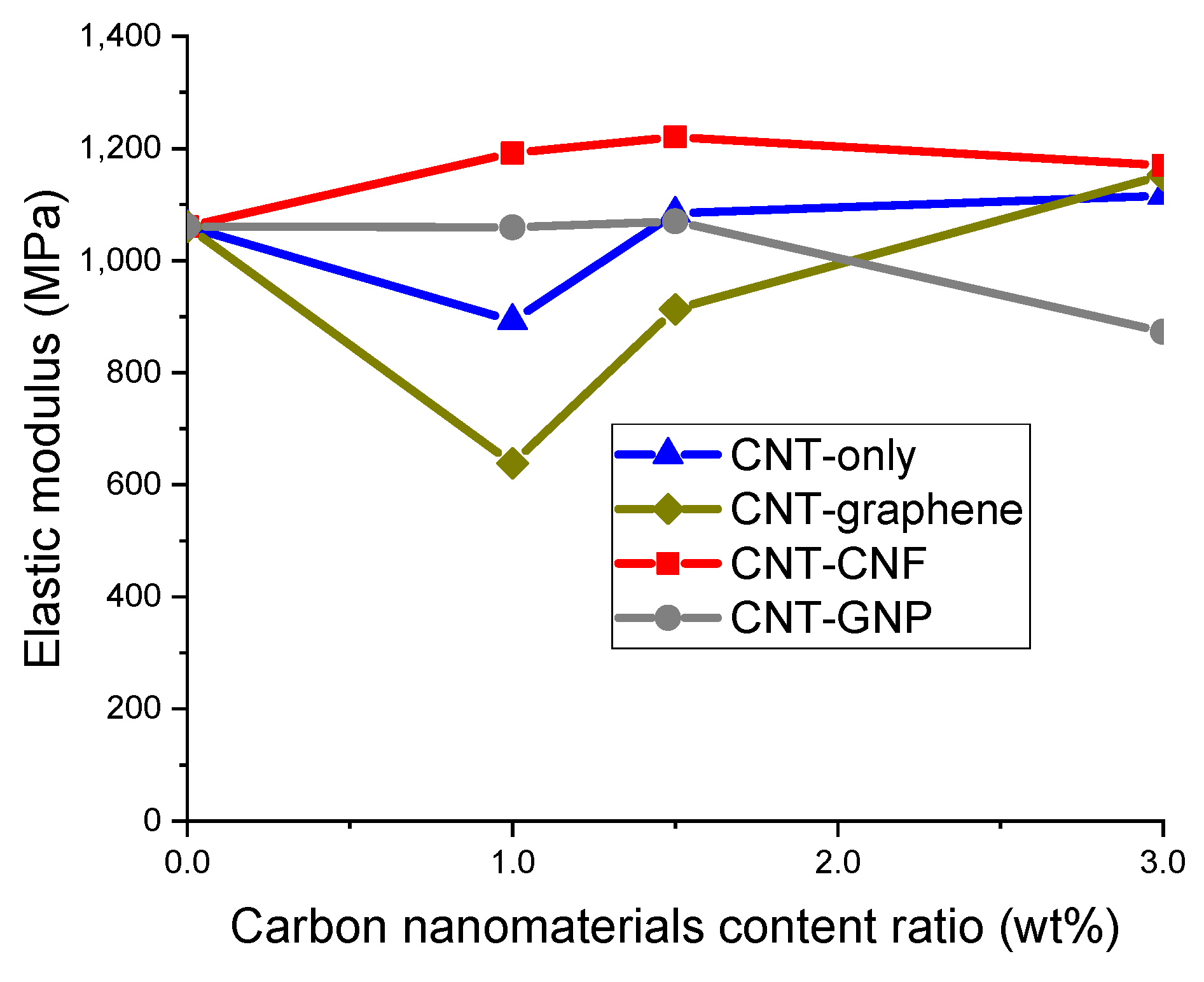
3.1. Elastic Modulus under Tensile Loading
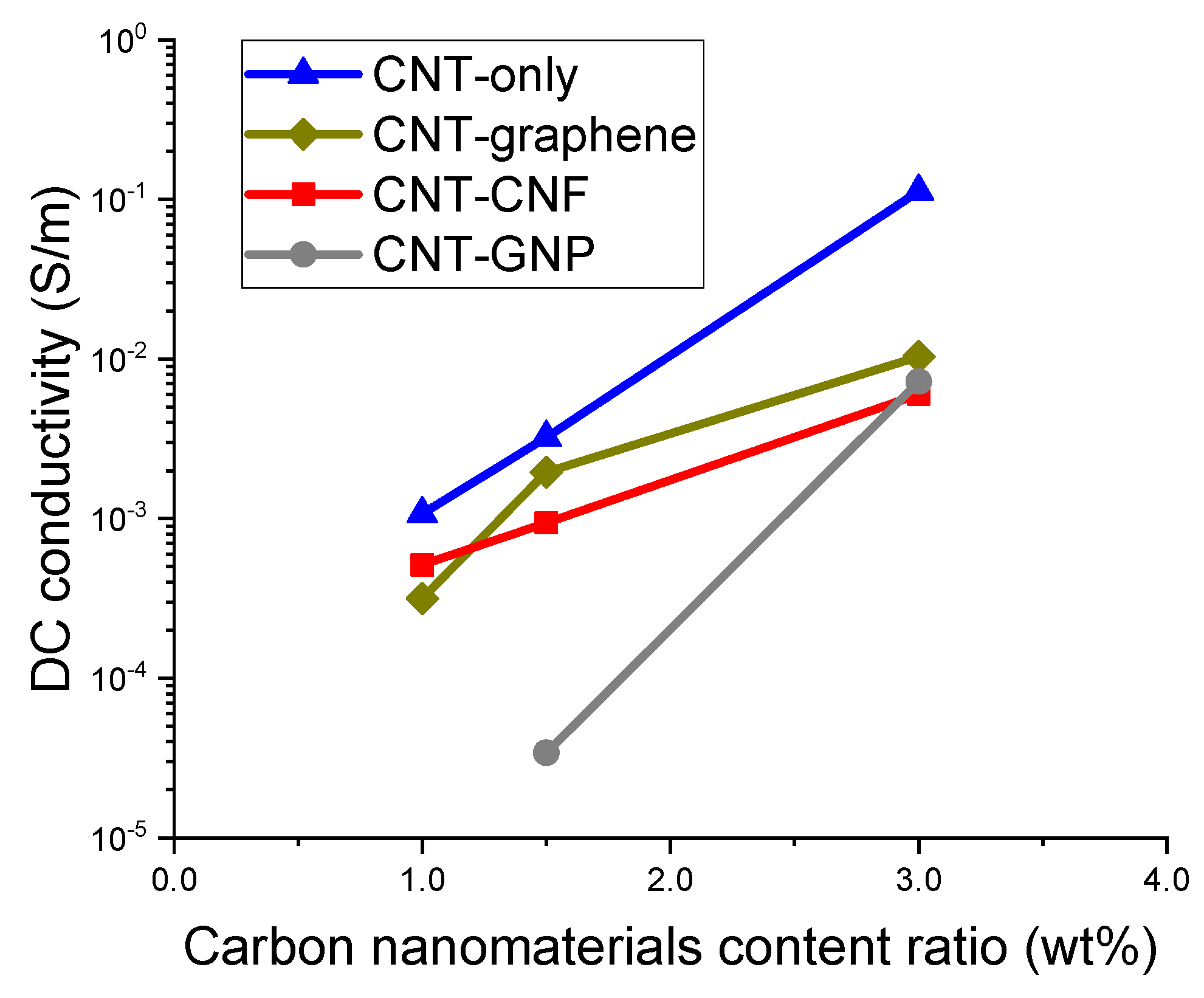
3.2. Electrical Conductivity
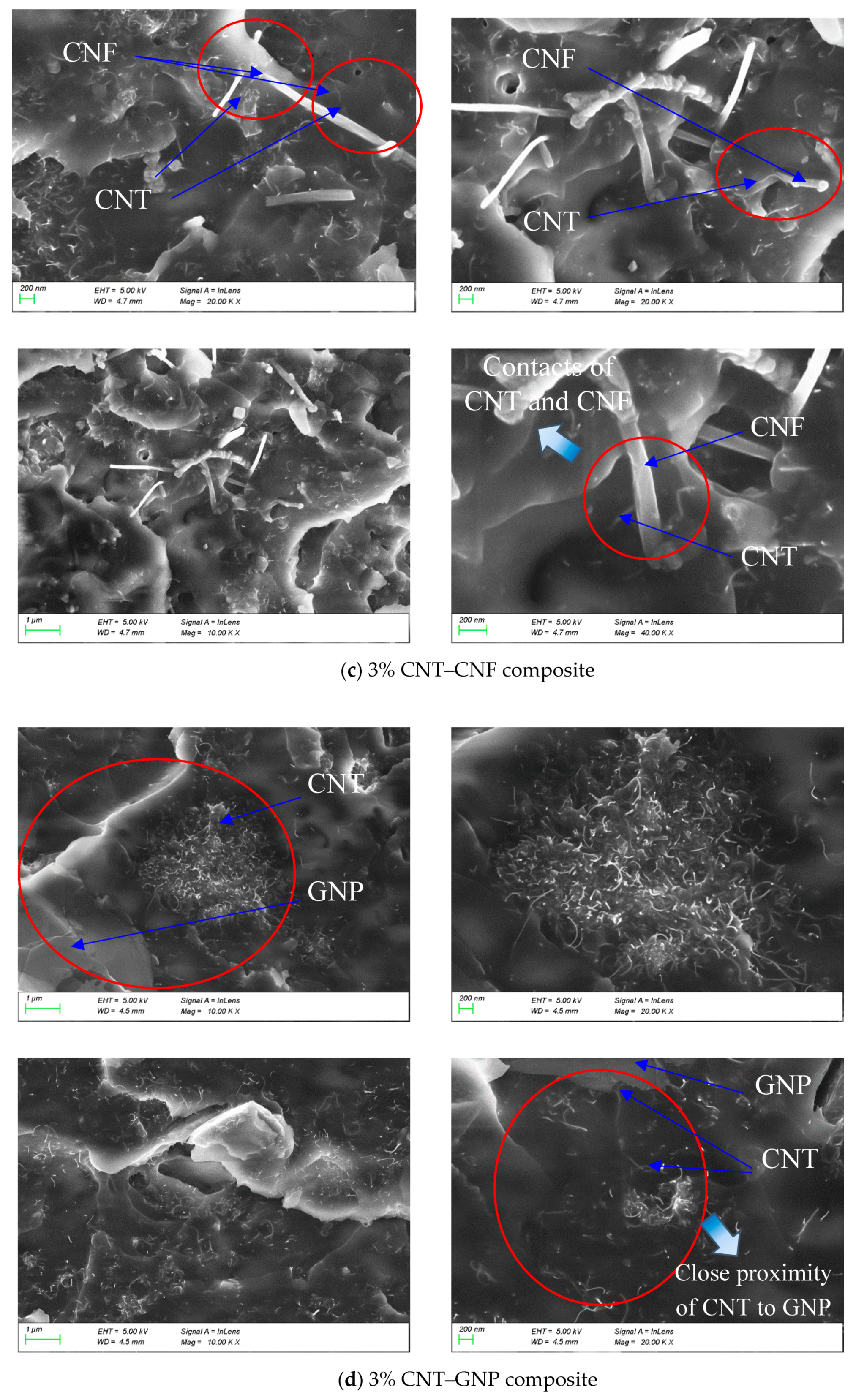
3.3. SEM Images
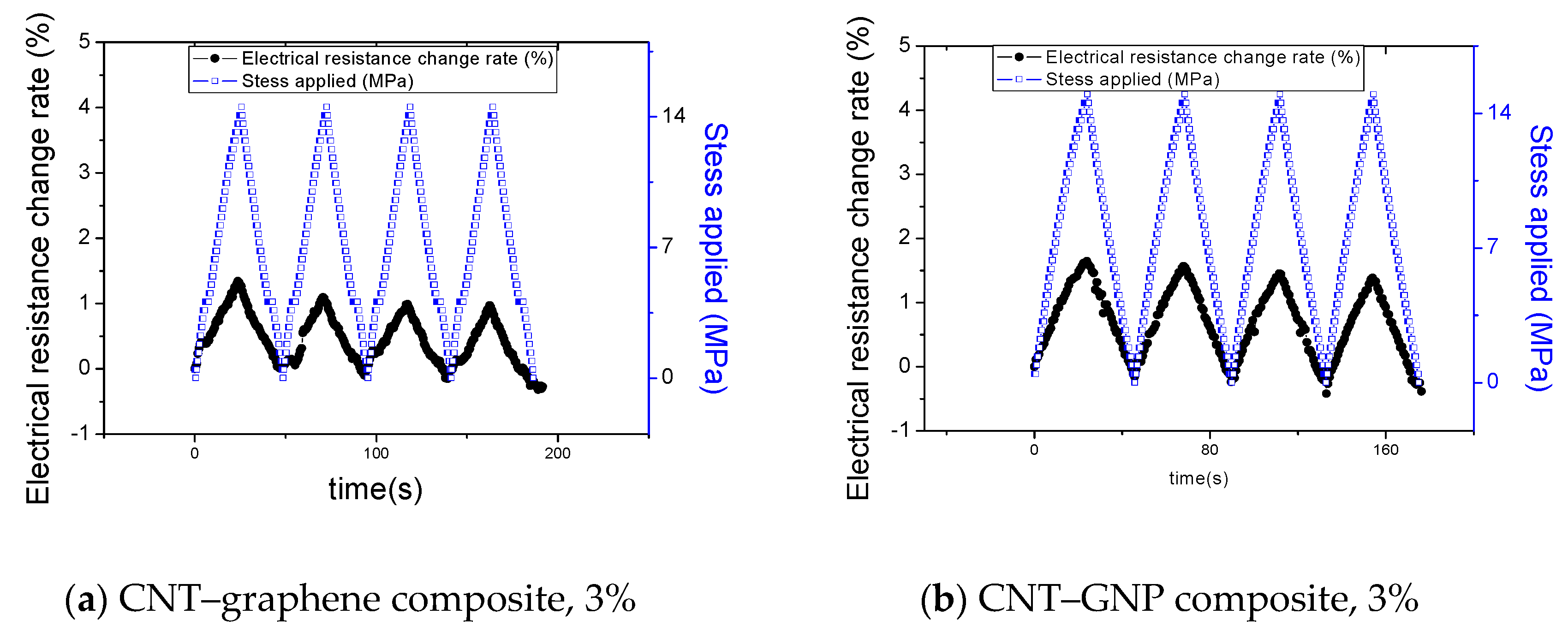
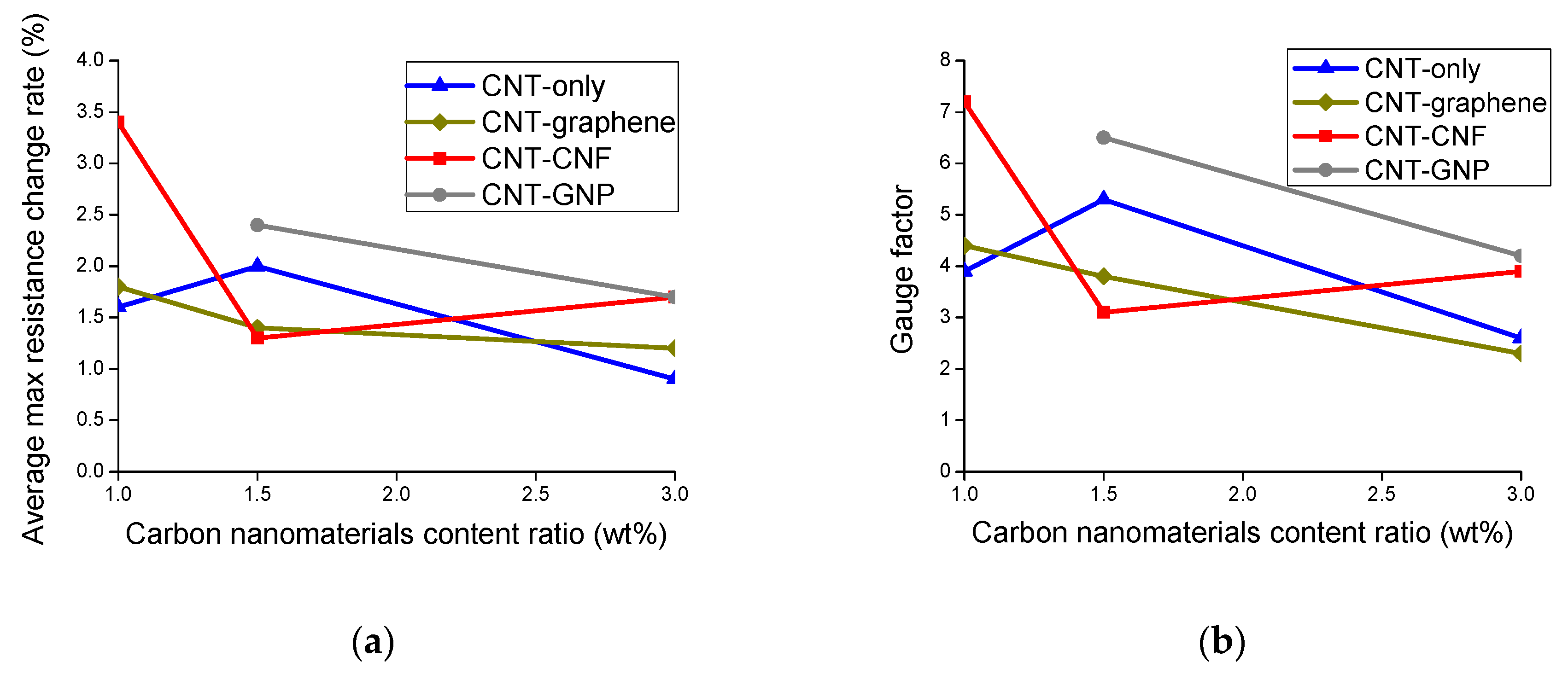
3.4. Piezoresistive Characteristics of the Composites Subjected to Repetitive Tensile Loadings
4. Concluding Remarks
- In the cases of composites with CNMs of 3%, elastic modulus of the CNT–CNF composite and CNT–graphene composite were greater than that of the CNT-only composite, which indicated synergistic effects attributed to the hybridized CNMs network.
- In the results of electrical conductivity of the composites, the percolation threshold of the CNT-only, CNT–graphene, and CNT–CNF composites ranged from 0% to 1%, but the CNT–GNP composite had a percolation threshold at a content ratio between 0% and 1.5%, which is larger than that of the other types of composites.
- SEM images showed that CNMs were distributed in the epoxy matrix and demonstrated interconnections of CNT–graphene and CNT–CNF, which led to enhancements in mechanical/electrical characteristics.
- It was found that gauge factor tended to decrease as the CNM content increased further beyond the percolation threshold. For the composites where the CNM networks were sufficiently formed with a content ratio of 3%, the greatest gauge factor was accomplished by the CNT–GNP composite, followed by the CNT–CNF composite, and this could not be achieved by the CNT-only composite. The superiority of the two types of composites in gauge factor was explained with the excluded volume theory.
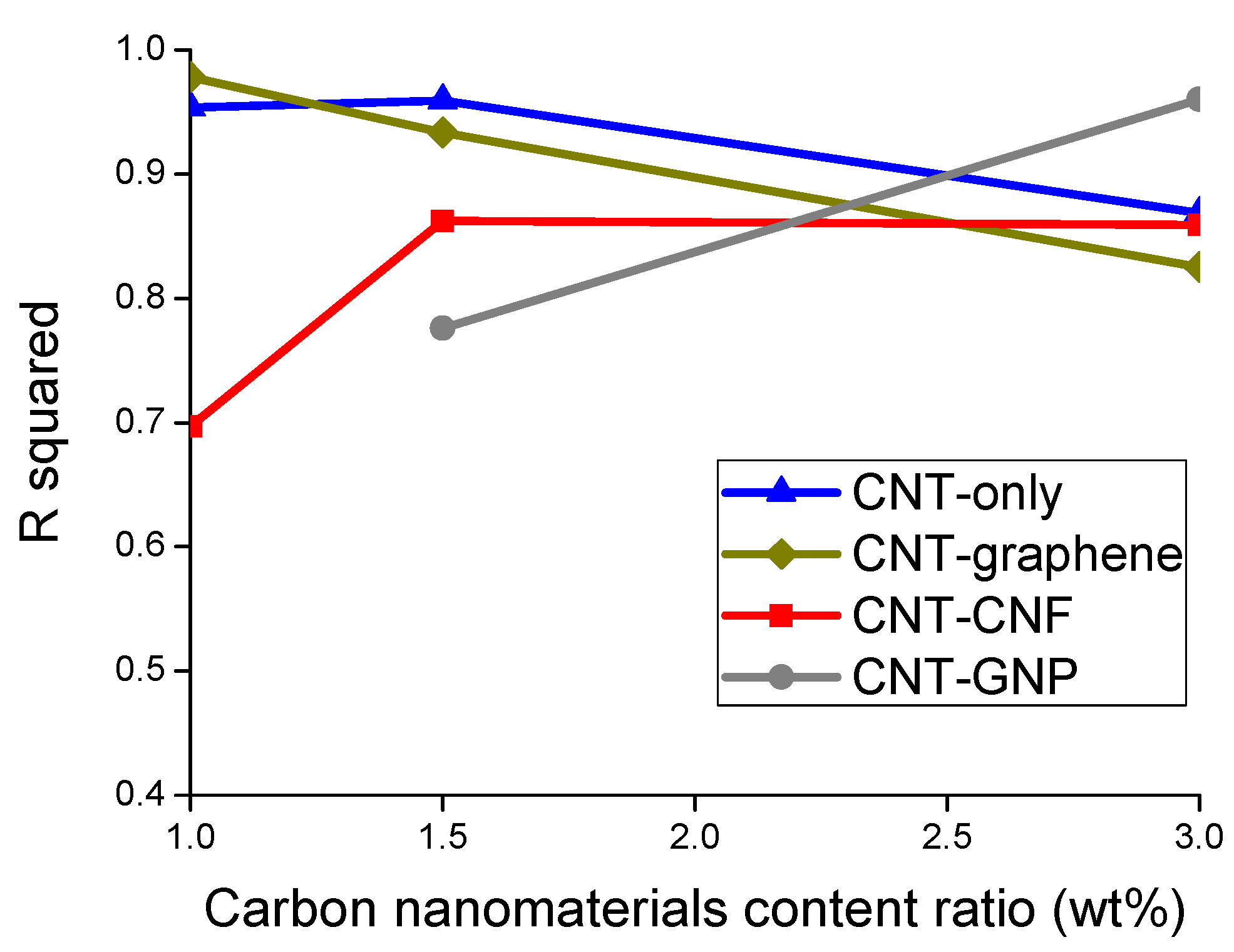
- The 3% CNT–GNP composite and 1.5% CNT-only composite exhibited relatively high R2 values, which was related to sensing stability, as well as a relatively high gauge factor.
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Li, W.; Dichiara, A.; Bai, J. Carbon nanotube-graphene nanoplatelet hybrids as high-performance multifunctional reinforcements in epoxy composites. Compos. Sci. Technol. 2013, 74, 221–227. [Google Scholar] [CrossRef]
- Luan, C.; Yao, X.; Shen, H.; Fu, J. Self-sensing of position-related loads in continuous carbon fibers-embedded 3D-printed polymer structures using electrical resistance measurement. Sensors 2018, 18, 994. [Google Scholar] [CrossRef] [Green Version]
- Park, J.M.; Kim, D.S.; Kim, S.J.; Kim, P.G.; Yoon, D.J.; DeVries, K.L. Inherent sensing and interfacial evaluation of carbon nanofiber and nanotube/epoxy composites using electrical resistance measurement and micromechanical technique. Compos. Part B Eng. 2007, 38, 847–861. [Google Scholar] [CrossRef]
- Sun, X.; Liu, X.; Shen, X.; Wu, Y.; Wang, Z.; Kim, J.K. Graphene foam/carbon nanotube/poly(dimethyl siloxane) composites for exceptional microwave shielding. Compos. Part A Appl. Sci. Manuf. 2016, 85, 199–206. [Google Scholar] [CrossRef]
- Punetha, V.D.; Rana, S.; Yoo, H.J.; Chaurasia, A.; McLeskey, J.T.; Ramasamy, M.S.; Sahoo, N.G.; Cho, J.W. Functionalization of carbon nanomaterials for advanced polymer nanocomposites: A comparison study between CNT and graphene. Prog. Polym. Sci. 2017, 67, 1–47. [Google Scholar] [CrossRef]
- Lee, S.H.; Lee, D.H.; Lee, W.J.; Kim, S.O. Tailored assembly of carbon nanotubes and graphene. Adv. Funct. Mater. 2011, 21, 1338–1354. [Google Scholar] [CrossRef]
- Tamburrano, A.; Sarasini, F.; De Bellis, G.; D’Aloia, A.G.; Sarto, M.S. The piezoresistive effect in graphene-based polymeric composites. Nanotechnology 2013, 24, 465702. [Google Scholar] [CrossRef] [PubMed]
- Chung, D.D.L. A review of exfoliated graphite. J. Mater. Sci. 2015, 51, 554–568. [Google Scholar] [CrossRef]
- Wang, X.; Meng, S.; Tebyetekerwa, M.; Li, Y.; Pionteck, J.; Sun, B.; Qin, Z.; Zhu, M. Highly sensitive and stretchable piezoresistive strain sensor based on conductive poly(styrene-butadiene-styrene)/few layer graphene composite fiber. Compos. Part A Appl. Sci. Manuf. 2018, 105, 291–299. [Google Scholar] [CrossRef]
- Nardelli, M.B. Electronic transport in extended systems: Application to carbon nanotubes. Phys. Rev. B Condens. Matter Mater. Phys. 1999, 60, 7828–7833. [Google Scholar] [CrossRef] [Green Version]
- Charlier, J.C.; Issi, J.P. Electrical conductivity of novel forms of carbon. J. Phys. Chem. Solids 1996, 57, 957–965. [Google Scholar] [CrossRef]
- Marsden, A.J.; Papageorgiou, D.G.; Vallés, C.; Liscio, A.; Palermo, V.; Bissett, M.A.; Young, R.J.; Kinloch, I.A. Electrical percolation in graphene-polymer composites. 2D Mater. 2018, 5, 032003. [Google Scholar] [CrossRef] [Green Version]
- Boland, C.S.; Khan, U.; Ryan, G.; Barwich, S.; Charifou, R.; Harvey, A.; Backes, C.; Li, Z.; Ferreira, M.S.; Möbius, M.E.; et al. Sensitive electromechanical sensors using viscoelastic graphene-polymer nanocomposites. Science 2016, 354, 1257–1260. [Google Scholar] [CrossRef] [PubMed]
- Avilés, F.; May-Pat, A.; Canché-Escamilla, G.; Rodríguez-Uicab, O.; Ku-Herrera, J.J.; Duarte-Aranda, S.; Uribe-Calderon, J.; Gonzalez-Chi, P.I.; Arronche, L.; La Saponara, V. Influence of carbon nanotube on the piezoresistive behavior of multiwall carbon nanotube/polymer composites. J. Intell. Mater. Syst. Struct. 2016, 27, 92–103. [Google Scholar] [CrossRef]
- Schütt, F.; Signetti, S.; Krüger, H.; Röder, S.; Smazna, D.; Kaps, S.; Gorb, S.N.; Mishra, Y.K.; Pugno, N.M.; Adelung, R. Hierarchical self-entangled carbon nanotube tube networks. Nat. Commun. 2017, 8, 1–10. [Google Scholar] [CrossRef] [PubMed]
- Garlof, S.; Mecklenburg, M.; Smazna, D.; Mishra, Y.K.; Adelung, R.; Schulte, K.; Fiedler, B. 3D carbon networks and their polymer composites: Fabrication and electromechanical investigations of neat Aerographite and Aerographite-based PNCs under compressive load. Carbon N. Y. 2017, 111, 103–112. [Google Scholar] [CrossRef]
- Garlof, S.; Fukuda, T.; Mecklenburg, M.; Smazna, D.; Mishra, Y.K.; Adelung, R.; Schulte, K.; Fiedler, B. Electro-mechanical piezoresistive properties of three dimensionally interconnected carbon aerogel (Aerographite)-epoxy composites. Compos. Sci. Technol. 2016, 134, 226–233. [Google Scholar] [CrossRef]
- Muhulet, A.; Miculescu, F.; Voicu, S.I.; Schütt, F.; Thakur, V.K.; Mishra, Y.K. Fundamentals and scopes of doped carbon nanotubes towards energy and biosensing applications. Mater. Today Energy 2018, 9, 154–186. [Google Scholar] [CrossRef]
- Avilés, F.; May-Pat, A.; López-Manchado, M.A.; Verdejo, R.; Bachmatiuk, A.; Rümmeli, M.H. A comparative study on the mechanical, electrical and piezoresistive properties of polymer composites using carbon nanostructures of different topology. Eur. Polym. J. 2018, 99, 394–402. [Google Scholar] [CrossRef]
- Nam, I.W.; Park, S.M.; Lee, H.K.; Zheng, L. Mechanical properties and piezoresistive sensing capabilities of FRP composites incorporating CNT fibers. Compos. Struct. 2017, 178, 1–8. [Google Scholar] [CrossRef]
- Huang, J.; Yang, X.; Her, S.C.; Liang, Y.M. Carbon nanotube/graphene nanoplatelet hybrid film as a flexible multifunctional sensor. Sensors 2019, 19, 317. [Google Scholar] [CrossRef] [Green Version]
- Luo, S.; Liu, T. SWCNT/graphite nanoplatelet hybrid thin films for self-temperature- compensated, highly sensitive, and extensible piezoresistive sensors. Adv. Mater. 2013, 25, 5650–5657. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Bai, J. Highly sensitive piezo-resistive graphite nanoplatelet-carbon nanotube hybrids/polydimethylsilicone composites with improved conductive network construction. ACS Appl. Mater. Interfaces 2015, 7, 9652–9659. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Li, W.; Huang, W.; Zhang, G.; Sun, R.; Wong, C.P. Fabrication of highly reinforced and compressible graphene/carbon nanotube hybrid foams via a facile self-assembly process for application as strain sensors and beyond. J. Mater. Chem. C 2017, 5, 2723–2730. [Google Scholar] [CrossRef]
- Kumar, S.; Sun, L.L.; Caceres, S.; Li, B.; Wood, W.; Perugini, A.; Maguire, R.G.; Zhong, W.H. Dynamic synergy of graphitic nanoplatelets and multi-walled carbon nanotubes in polyetherimide nanocomposites. Nanotechnology 2010, 21, 105702. [Google Scholar] [CrossRef]
- Aguilar-Bolados, H.; Yazdani-Pedram, M.; Contreras-Cid, A.; Lopez-Manchado, M.A.; May-Pat, A.; Avilés, F. Influence of the morphology of carbon nanostructures on the piezoresistivity of hybrid natural rubber nanocomposites. Compos. Part B Eng. 2017, 109, 147–154. [Google Scholar] [CrossRef]
- Nam, I.W.; Souri, H.; Lee, H.K. Percolation threshold and piezoresistive response of multi-wall carbon nanotube/cement composites. Smart Struct. Syst. 2016, 18, 217–231. [Google Scholar] [CrossRef]
- Mora, A.; Han, F.; Lubineau, G. Computational modeling of electrically conductive networks formed by graphene nanoplatelet-carbon nanotube hybrid particles. Model. Simul. Mater. Sci. Eng. 2018, 26, 035010. [Google Scholar] [CrossRef] [Green Version]
- Kim, Y.J.; Cha, J.Y.; Ham, H.; Huh, H.; So, D.S.; Kang, I. Preparation of piezoresistive nano smart hybrid material based on graphene. Curr. Appl. Phys. 2011, 11, 350–352. [Google Scholar] [CrossRef]
- Wang, Y.; Serrano, S.; Santiago-Aviles, J.J. Conductivity measurement of electrospun PAN-based carbon nanofiber. J. Mater. Sci. Lett. 2002, 21, 1055–1057. [Google Scholar] [CrossRef]
- Nam, I.W.; Choi, J.H.; Kim, C.G.; Lee, H.K. Fabrication and design of electromagnetic wave absorber composed of carbon nanotube-incorporated cement composites. Compos. Struct. 2018, 206, 439–447. [Google Scholar] [CrossRef]
- Ferreira, A.; Cardoso, P.; Klosterman, D.; Covas, J.A.; Van Hattum, F.W.J.; Vaz, F.; Lanceros-Mendez, S. Effect of filler dispersion on the electromechanical response of epoxy/vapor-grown carbon nanofiber composites. Smart Mater. Struct. 2012, 21, 075008. [Google Scholar] [CrossRef]
- Gupta, T.K.; Choosri, M.; Varadarajan, K.M.; Kumar, S. Self-sensing and mechanical performance of CNT/GNP/UHMWPE biocompatible nanocomposites. J. Mater. Sci. 2018, 53, 7939–7952. [Google Scholar] [CrossRef]
- Rathi, P.; Jha, M.K.; Hata, K.; Subramaniam, C. Real-time, wearable, biomechanical movement capture of both humans and robots with metal-free electrodes. ACS Omega 2017, 2, 4132–4142. [Google Scholar] [CrossRef]
- Souri, H.; Nam, I.W.; Lee, H.K. Electrical properties and piezoresistive evaluation of polyurethane-based composites with carbon nano-materials. Compos. Sci. Technol. 2015, 121, 41–48. [Google Scholar] [CrossRef]
- Philipse, A.P. The random contact equation and its implications for (colloidal) rods in packings, suspensions, and anisotropic powders. Langmuir 1996, 12, 5971. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.K.; Park, I.S.; Lee, H.K. Improved piezoresistive sensitivity and stability of CNT/cement mortar composites with low water-binder ratio. Compos. Struct. 2014, 116, 713–719. [Google Scholar] [CrossRef]






| CNM | Diameter or Thickness | Layers | Purity | Specific Surface Area (m2/g) | True Density (g/cm3) | Length or Width (μm) | Electrical Conductivity (S/cm) |
|---|---|---|---|---|---|---|---|
| CNT | <8 nm (outer diameter) 2–5 nm (inner diameter) | >98% | >350 | ~2.1 | 10–30 | >100 | |
| Graphene | 0.55–1.2 nm (thickness) | 1–5 | >99% | >500 | 0.5–3 | 184.8 | |
| CNF | 0.15–0.2 μm | 99.9% | 300 | ~2.0 | 10–30 | ||
| GNP | <30 | >90% | 2–16 | 6.67 |
| Order | Gap I Distance (μm) | Gap II Distance (μm) |
|---|---|---|
| 1 | 60 | 40 |
| 2 | 40 | 20 |
| 3 | 20 | 15 |
| 4 | 15 | 10 |
| 5 | 10 | 5 |
| 6 | 5 | 5 |
| 7 | 10 | 5 |
| 8 | 5 | 5 |
| 9 | 10 | 5 |
| 10 | 5 | 5 |
| 11 | 3 | 2 |
| 12 | 2 | 1 |
| d (μm) | L (μm) | Excluded Volume (μm3) | |
|---|---|---|---|
| CNT | 0.008 | 20 | 5.0 |
| Graphene | 1.75 | 6.6 | |
| CNF | 0.17 | 20 | 110.4 |
| GNP | 9 | 898.5 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, X.; Wang, J.; Biswas, S.; Kim, H.; Nam, I. Mechanical, Electrical, and Piezoresistive Sensing Characteristics of Epoxy-Based Composites Incorporating Hybridized Networks of Carbon Nanotubes, Graphene, Carbon Nanofibers, or Graphite Nanoplatelets. Sensors 2020, 20, 2094. https://doi.org/10.3390/s20072094
Wang X, Wang J, Biswas S, Kim H, Nam I. Mechanical, Electrical, and Piezoresistive Sensing Characteristics of Epoxy-Based Composites Incorporating Hybridized Networks of Carbon Nanotubes, Graphene, Carbon Nanofibers, or Graphite Nanoplatelets. Sensors. 2020; 20(7):2094. https://doi.org/10.3390/s20072094
Chicago/Turabian StyleWang, XiaoDong, JianChao Wang, Swarup Biswas, Hyeok Kim, and IlWoo Nam. 2020. "Mechanical, Electrical, and Piezoresistive Sensing Characteristics of Epoxy-Based Composites Incorporating Hybridized Networks of Carbon Nanotubes, Graphene, Carbon Nanofibers, or Graphite Nanoplatelets" Sensors 20, no. 7: 2094. https://doi.org/10.3390/s20072094
APA StyleWang, X., Wang, J., Biswas, S., Kim, H., & Nam, I. (2020). Mechanical, Electrical, and Piezoresistive Sensing Characteristics of Epoxy-Based Composites Incorporating Hybridized Networks of Carbon Nanotubes, Graphene, Carbon Nanofibers, or Graphite Nanoplatelets. Sensors, 20(7), 2094. https://doi.org/10.3390/s20072094






