Research on the Frequency Response and Dynamic Range of the Quadrature Fiber Optic Fabry–Perot Cavity Microphone Based on the Differential Cross Multiplication Demodulation Algorithm
Abstract
:1. Introduction
2. Fundamentals of the Q–FFPM–DCM
2.1. Theoretical Model of the Quadrature Fiber Optic F–P Cavity Microphone
2.2. DCM Signal Demodulation Algorithm
3. Analysis of the Frequency Response and Dynamic Range
3.1. Simulation of the Effect of the Signal Intensity on Demodulated Signals
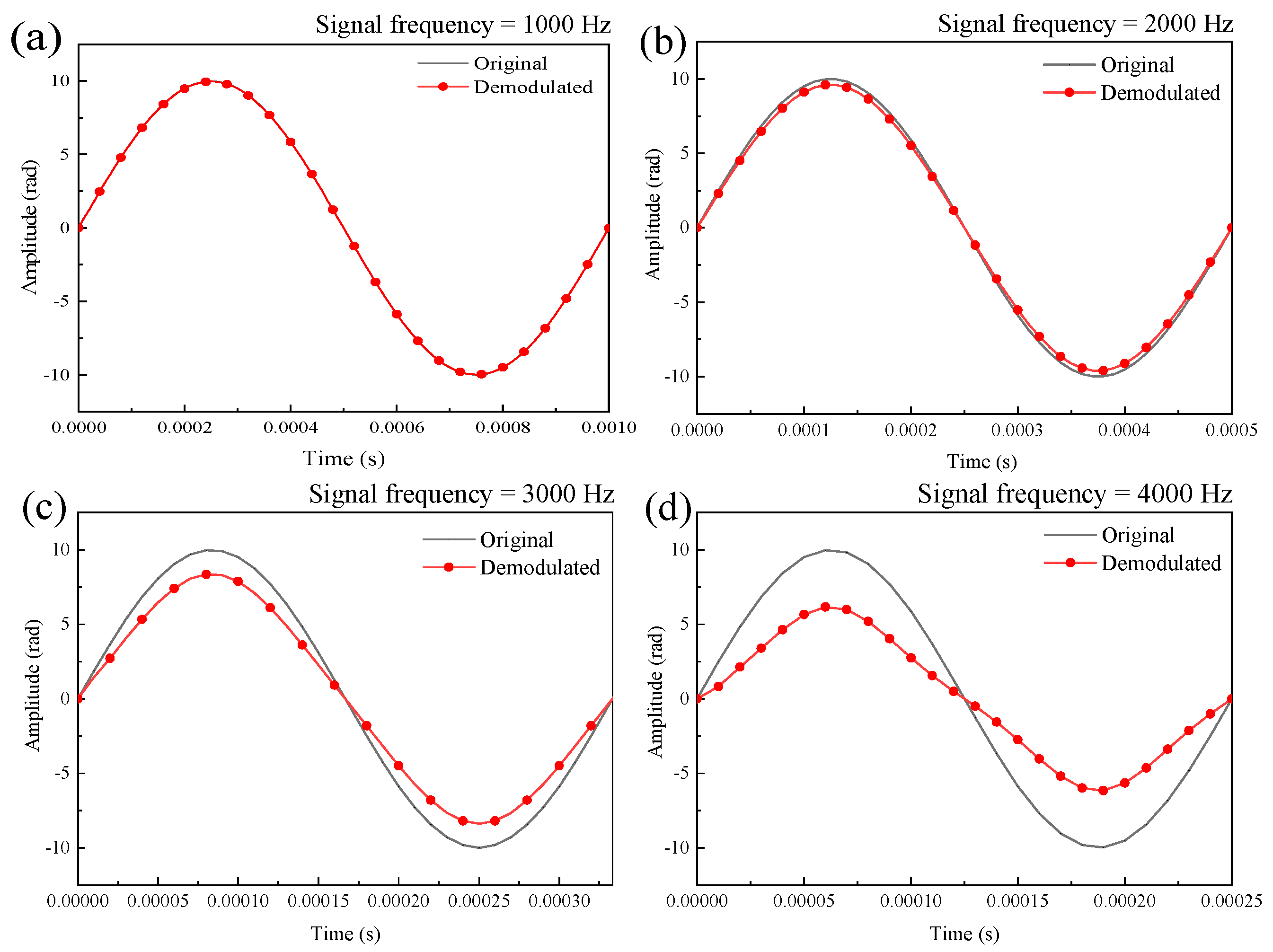
3.2. Simulation of the Effect of Signal Frequency on the Demodulated Signals
3.3. Simulation of the Effect of the Data Sampling Rate on the Demodulated Signals
3.4. Relationship between the Upper Limit of the Dynamic Range of the Signal, the Upper Limit of the Frequency Response Range, and the Data Sampling Rate
4. Effect of Quadrature Phase Deviation on Frequency Response and Dynamic Range
4.1. Simulation of the Effect of the Quadrature Phase Deviation on the Frequency Response
4.2. Simulation of the Effect of the Quadrature Phase Deviation on the Dynamic Range
4.3. A Method to Suppress the Quadrature Phase Deviation
5. Experiment
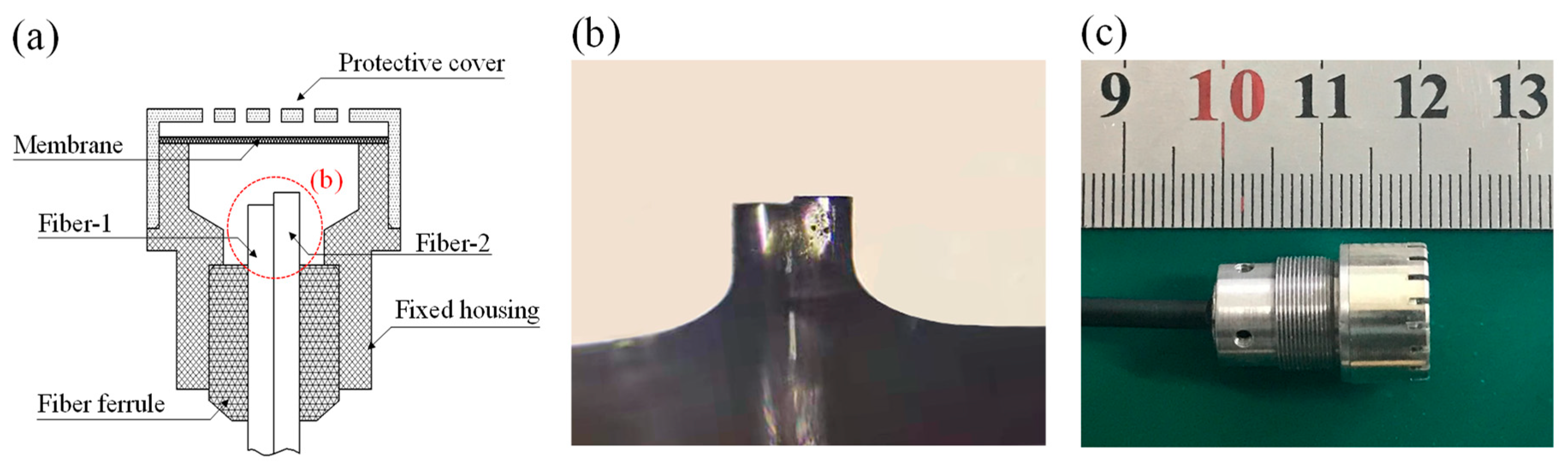
5.1. Q–FFPM–DCM Probe Construction and System Components
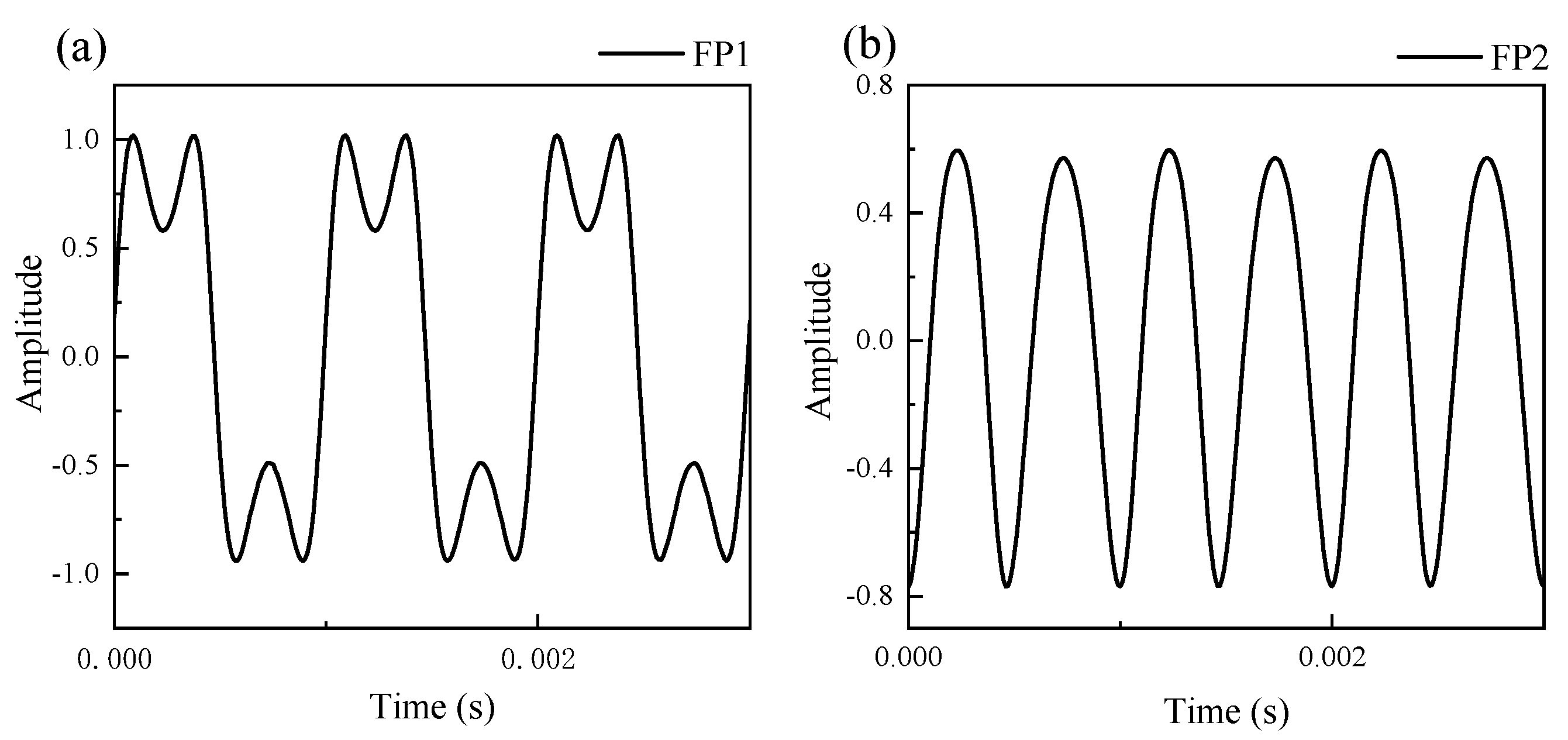
5.2. A Method for Adjusting the Q–FFPM–DCM into Quadrature State
6. Experimental Results and Discussion
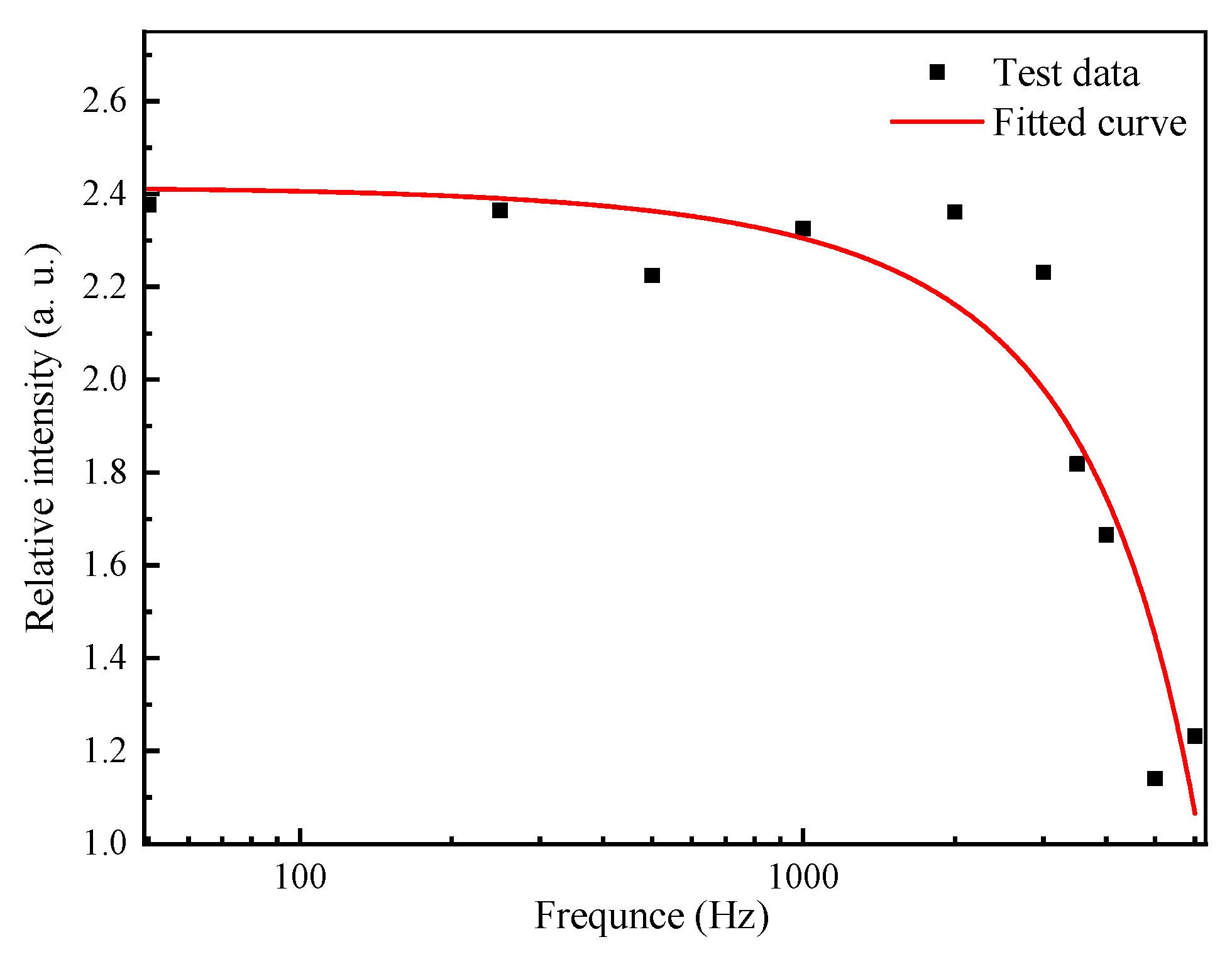
6.1. Experimental Results at Different Signal Frequencies
6.2. Experimental Results at Different Signal Intensities
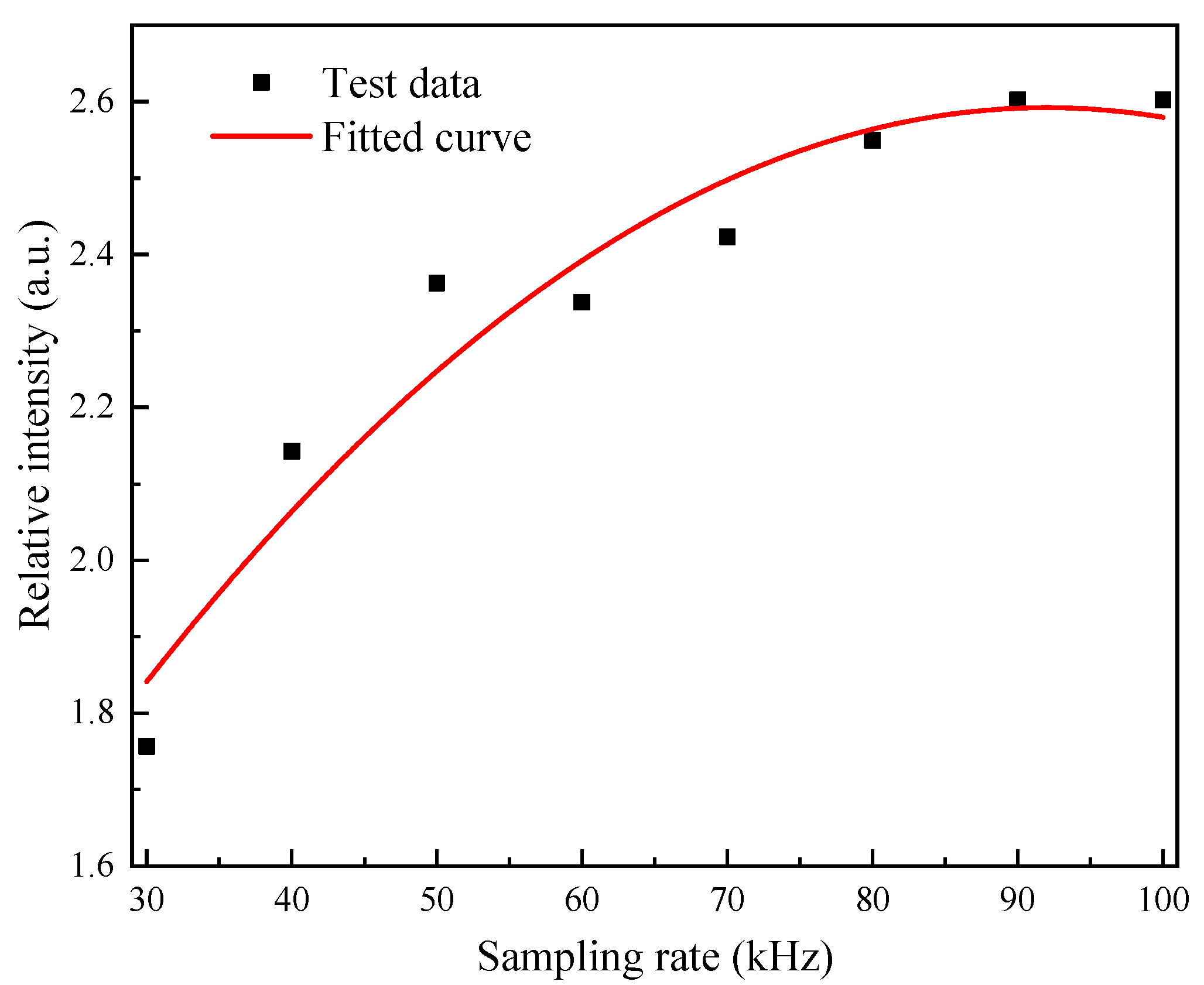
6.3. Experimental Results at Different Data Sampling Rates
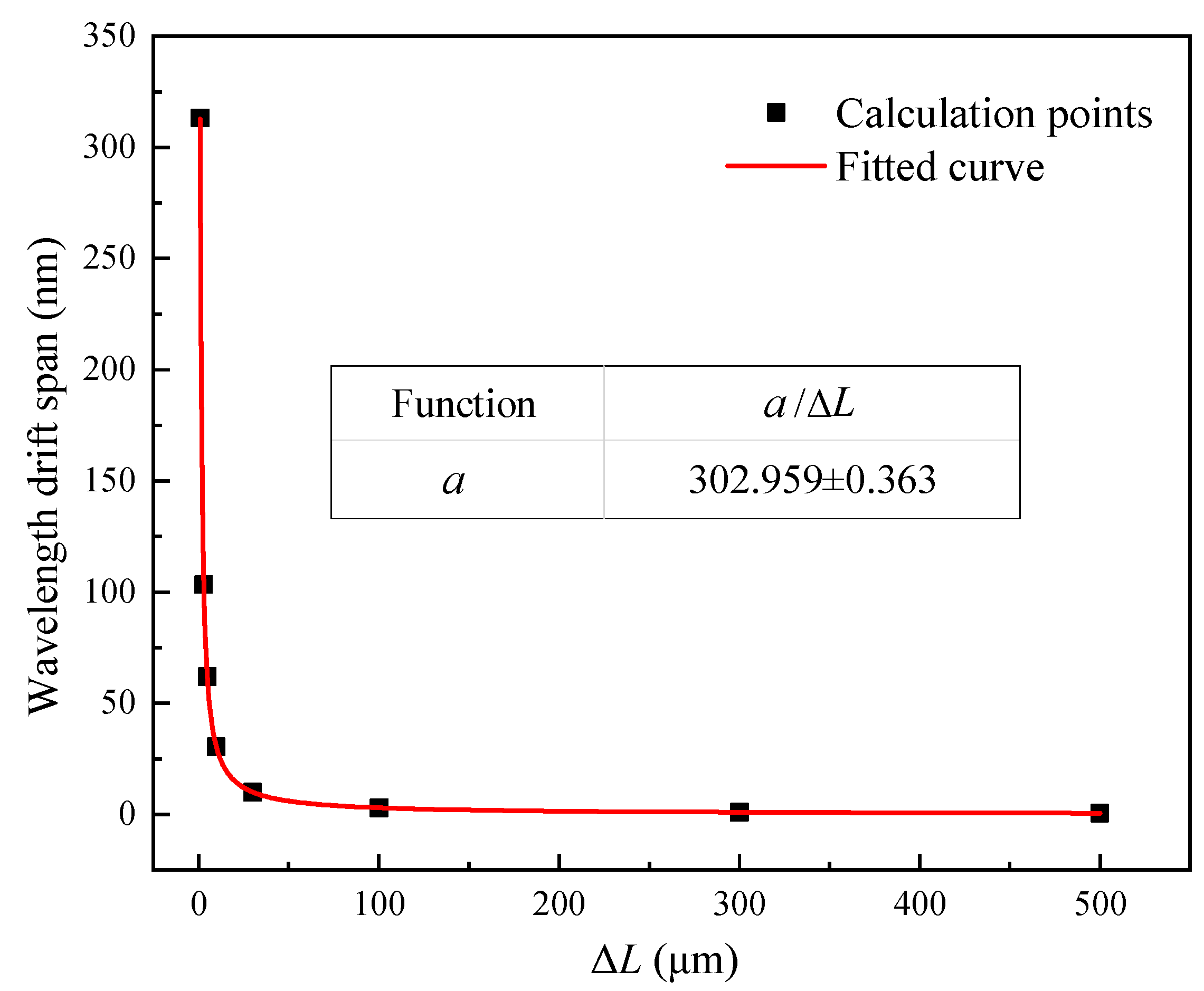
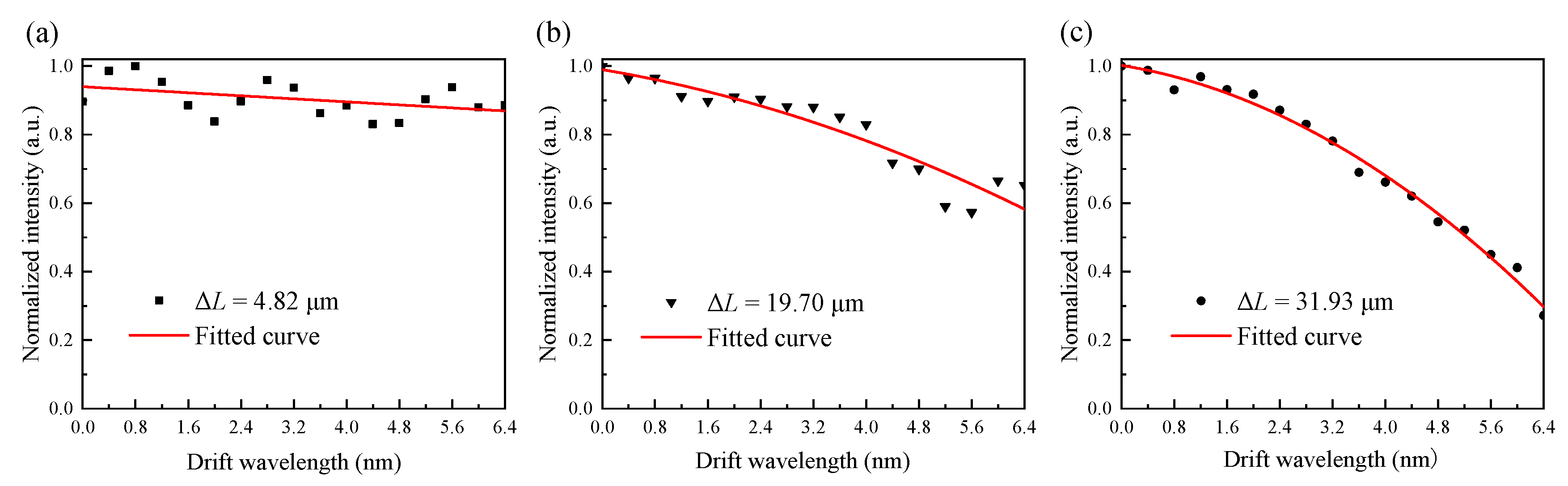
6.4. Results of the Suppression of the Quadrature Phase Deviation by Fiber Step Height
6.5. Discussion
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bado, M.F.; Casas, J.R. A Review of Recent Distributed Optical Fiber Sensors Applications for Civil Engineering Structural Health Monitoring. Sensors 2021, 21, 1818. [Google Scholar] [CrossRef] [PubMed]
- Barrias, A.; Casas, J.R.; Villalba, S. A review of distributed optical fiber sensors for civil engineering applications. Sensors 2016, 16, 748. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, K.; Yang, B.; Deng, H.; Guo, M.; Zhang, B.; Yang, Y.; Liu, S.; Zhao, Y.; Peng, W.; Yu, Q. Simultaneous measurement of acoustic pressure and temperature using a Fabry-Perot interferometric fiber-optic cantilever sensor. Opt. Express 2020, 28, 15050–15061. [Google Scholar] [CrossRef] [PubMed]
- Kumar, S.S.; Khansa, C.; Praveen, T.; Sreehari, C.; Santhanakrishnan, T.; Rajesh, R. Assessment of Dynamic Range in Interferometric Fiber Optic Hydrophones Based on Homodyne PGC Interrogator. IEEE Sens. J. 2020, 20, 13418–13425. [Google Scholar] [CrossRef]
- Deng, J.; Xiao, H.; Huo, W.; Luo, M.; May, R.; Wang, A.; Liu, Y. Optical fiber sensor-based detection of partial discharges in power transformers. Opt. Laser Technol. 2001, 33, 305–311. [Google Scholar] [CrossRef]
- Abuelma’atti, M.T. Harmonic and intermodulation performance of optical-fiber Mach–Zehnder microphone. Appl. Acoust. 2011, 72, 305–309. [Google Scholar] [CrossRef]
- Li, C.; Peng, X.; Zhang, H.; Wang, C.; Fan, S.; Cao, S. A sensitivity-enhanced flexible acoustic sensor using side-polished fiber Bragg grating. Measurement 2018, 117, 252–257. [Google Scholar] [CrossRef]
- Ma, J.; Yu, Y.; Jin, W. Demodulation of diaphragm based acoustic sensor using Sagnac interferometer with stable phase bias. Opt. Express 2015, 23, 29268–29278. [Google Scholar] [CrossRef]
- Acharya, A.; Kawade, N. A Fabry–Perot Interferometer-Based Fiber Optic Dynamic Displacement Sensor With an Analog in-Phase/Quadrature Generator. IEEE Sens. J. 2020, 20, 14764–14771. [Google Scholar] [CrossRef]
- Chen, J.; Chen, D.; Geng, J.; Cai, H.; Fang, Z.J.S. Stabilization of optical Fabry–Perot sensor by active feedback control of diode laser. Sens. Actuat. A-Phys. 2008, 148, 376–380. [Google Scholar] [CrossRef]
- Islam, M.; Ali, M.M.; Lai, M.-H.; Lim, K.-S.; Ahmad, H. Chronology of Fabry-Perot interferometer fiber-optic sensors and their applications: A review. Sensors 2014, 14, 7451–7488. [Google Scholar] [CrossRef] [Green Version]
- Coote, J.M.; Torii, R.; Desjardins, A.E. Dynamic Characterisation of Fibre-Optic Temperature Sensors for Physiological Monitoring. Sensors 2021, 21, 221. [Google Scholar] [CrossRef] [PubMed]
- Gao, X.-X.; Cui, J.-M.; Ai, M.-Z.; Huang, Y.-F.; Li, C.-F.; Guo, G.-C. An Acoustic Sensor Based on Active Fiber Fabry–Pérot Microcavities. Sensors 2020, 20, 5760. [Google Scholar] [CrossRef] [PubMed]
- Thurner, K.; Braun, P.-F.; Karrai, K. Fabry-Pérot interferometry for long range displacement sensing. Rev. Sci. Instrum. 2013, 84, 095005. [Google Scholar] [CrossRef]
- Chen, D.S.; Gao, H.Y.; Xiao, L. Novel optical fiber sound sensor based on differentiating interferometer. J. Ptoelectron. Laser 2006, 17, 942–944. [Google Scholar] [CrossRef] [Green Version]
- Ran, Z.; Li, C.; Ni, M.; Lu, E.; Rao, Y. Laser-machined cascaded micro cavities for simultaneous measurement of dual parameters. In Proceedings of the 2012 22nd International Conference on Optical Fiber Sensors, Beijing, China, 15–19 October 2012; p. 842187. [Google Scholar]
- Thurner, K.; Braun, P.-F.; Karrai, K. Absolute distance sensing by two laser optical interferometry. Rev. Sci. Instrum. 2013, 84, 115002. [Google Scholar] [CrossRef]
- Zhao, J.; Shi, Y.; Zhao, J.; Shan, N. Dual-Wavelength Quadrature Phase-Shifted Demodulation of Low-Finesse Short FP Cavity. J. Ptoelectron. Laser 2007, 18, 942. [Google Scholar] [CrossRef]
- Fang, G.; Xu, T.; Feng, S.; Li, F. Phase-sensitive optical time domain reflectometer based on phase-generated carrier algorithm. J. Lightwave Technol. 2015, 33, 2811–2816. [Google Scholar] [CrossRef]
- Huang, S.-C.; Lin, H. Modified phase-generated carrier demodulation compensated for the propagation delay of the fiber. Appl. Opt. 2007, 46, 7594–7603. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Shao, S.; Mei, H.; Hao, Q.; Rao, R. Analysis and mitigation of the carrier phase delay effect of the digital phase generated carrier algorithm. Appl. Opt. 2017, 56, 731–738. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Lin, J.; Liu, H.; Ma, Y.; Yan, L.; Jin, P. Diaphragm based long cavity Fabry–Perot fiber acoustic sensor using phase generated carrier. Opt. Commun. 2017, 382, 514–518. [Google Scholar] [CrossRef]
- Liu, Q.; Jing, Z.; Liu, Y.; Li, A.; Zhang, Y.; Huang, Z.; Han, M.; Peng, W. Quadrature phase-stabilized three-wavelength interrogation of a fiber-optic Fabry–Perot acoustic sensor. Opt. Lett. 2019, 44, 5402–5405. [Google Scholar] [CrossRef]
- Schmidt, M.; Fürstenau, N. Fiber-optic extrinsic Fabry–Perot interferometer sensors with three-wavelength digital phase demodulation. Opt. Lett. 1999, 24, 599–601. [Google Scholar] [CrossRef]
- Liao, H.; Lu, P.; Liu, L.; Wang, S.; Ni, W.; Fu, X.; Liu, D.; Zhang, J. Phase demodulation of short-cavity Fabry–Perot interferometric acoustic sensors with two wavelengths. IEEE Photonics J. 2017, 9, 1–9. [Google Scholar] [CrossRef]
- Chen, H.; Chen, Q.; Wang, W.; Zhang, X.; Ma, Z.; Li, Y.; Jing, X.; Yuan, S. Fiber-optic, extrinsic Fabry–Perot interferometric dual-cavity sensor interrogated by a dual-segment, low-coherence Fizeau interferometer for simultaneous measurements of pressure and temperature. Opt. Express 2019, 27, 38744–38758. [Google Scholar] [CrossRef]
- Gao, H.; Jiang, Y.; Cui, Y.; Zhang, L.; Jia, J.; Hu, J. Dual-cavity Fabry–Perot interferometric sensors for the simultaneous measurement of high temperature and high pressure. IEEE Sens. J. 2018, 18, 10028–10033. [Google Scholar] [CrossRef]
- Zhang, W.; Lu, P.; Qu, Z.; Fan, P.; Sima, C.; Liu, D.; Zhang, J. High Sensitivity and High Stability Dual Fabry-Perot Interferometric Fiber-Optic Acoustic Sensor Based on Sandwich-Structure Composite Diaphragm. IEEE Photonics J. 2021, 13, 1–13. [Google Scholar]
- Cheng, J.; Lu, D.-f.; Gao, R.; Qi, Z.-m. Fiber optic microphone with large dynamic range based on bi-fiber Fabry-Perot cavity. In Proceedings of the AOPC 2017: Fiber Optic Sensing and Optical Communications, Beijing, China, 4–6 June 2017; p. 104642C. [Google Scholar]
- Shi, Q.; Tian, Q.; Wang, L.; Tian, C.; Zhang, H.; Zhang, M.; Liao, Y.; Wang, H.; Zeng, X.; Huang, L. Performance improvement of phase-generated carrier method by eliminating laser-intensity modulation for optical seismometer. Opt. Eng. 2010, 49, 024402. [Google Scholar] [CrossRef]
- Wang, F.; Xie, J.; Hu, Z.; Xiong, S.; Luo, H.; Hu, Y. Interrogation of miniature extrinsic Fabry-Perot sensor using path matched differential interferometer and phase generated carrier scheme. In Proceedings of the 23rd International Conference on Optical Fibre Sensors, Santander, Spain, 2–6 June 2014; p. 915718. [Google Scholar]
- He, J.; Wang, L.; Li, F.; Liu, Y. An ameliorated phase generated carrier demodulation algorithm with low harmonic distortion and high stability. J. Lightwave Technol. 2010, 28, 3258–3265. [Google Scholar] [CrossRef]
- Jia, P.; Wang, D. Self-calibrated non-contact fibre-optic Fabry–Perot interferometric vibration displacement sensor system using laser emission frequency modulated phase generated carrier demodulation scheme. Meas. Sci. Technol. 2012, 23, 115201. [Google Scholar] [CrossRef]
- Egan, P.F.; Stone, J.A.; Hendricks, J.H.; Ricker, J.E.; Scace, G.E.; Strouse, G.F. Performance of a dual Fabry–Perot cavity refractometer. Opt. Lett. 2015, 40, 3945–3948. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chen, B.; Zhu, R.; Wu, Z.; Li, D.; Guo, S. Nanometer measurement with a dual Fabry–Perot interferometer. Appl. Opt. 2001, 40, 5632–5637. [Google Scholar] [CrossRef] [PubMed]
- Giovanni, D. Flat and Corrugated Diaphragm Design Handbook; CRC Press: Boca Raton, FL, USA, 1982; Volume 11. [Google Scholar]








| System Features | Q–FFPM/DCM [26,27,28,29,30,31] | Q–FFPM/Arctan [32,33] | PGC/DCM [19,20,21,22,28] | PGC/Arctan [28,32,33] | Multiwavelength/DCM [23,24,25,28] |
|---|---|---|---|---|---|
| Number of light sources | 1 | 1 | 1 | 1 | ≥2 |
| Acquisition of quadrature signals | Simple | Simple | More complex | More complex | Complex |
| System costs | Low | Low | High | High | High |
| Algorithm complexity | Low | High | Low | High | Low |
| Whether a carrier is required | No | No | Yes | Yes | No |
| Symbol | Value | Description |
|---|---|---|
| λ | 1550 nm | Wavelength |
| L1 | 375.18 μm | Initial length of short F–P cavity |
| L2 | 380 μm | Initial length of long F–P cavity |
| ΔL | 4.82 μm | Fiber optic step height |
| 0.95 A/W | Photoelectric conversion factor | |
| 0.98 | Contrast of interference spectrum | |
| R | 100 kΩ | Resistance value of the photoelectric conversion circuit |
| I0 | 10.7 μw | Central light intensity of the interference spectrum |
| ψ0 | 0 | Initial phase of the sound signal |
| n | 1 | Refractive index of air |
| 10 k | 20 k | 50 k | 79 k | 200 k | |||
|---|---|---|---|---|---|---|---|
| 10 (62.26 Pa) | 0.0258 | (Hz) | 258 | 697 | 1292 | 2038 | 5160 |
| 20 (124.52 Pa) | 0.0129 | (Hz) | 129 | 348 | 645 | 1019 | 2580 |
| 30 (186.78 Pa) | 0.0086 | (Hz) | 86 | 232 | 430 | 679 | 1720 |
| 40 (249.04 Pa) | 0.0064 | (Hz) | 64 | 174 | 322 | 509 | 1290 |
| 50 (311.30 Pa) | 0.0051 | (Hz) | 51 | 139 | 258 | 407 | 1032 |
| ΔL (μm) | Lower Limit of Wavelength Drift (nm) | Working Wavelength λ (nm) | Upper Limit of WaveLength Drift (nm) | Wavelength Drift Span (nm) |
|---|---|---|---|---|
| 0.96 | 1409.091 | 1550 | 1722.222 | 313.131 |
| 4.84 | 1519.607 | 1550 | 1581.632 | 62.024 |
| 9.88 | 1534.951 | 1550 | 1565.346 | 30.395 |
| 30.03 | 1545.016 | 1550 | 1555.016 | 9.999 |
| 100.16 | 1548.502 | 1550 | 1551.500 | 2.997 |
| 500.06 | 1549.699 | 1550 | 1550.300 | 0.600 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, B.; Cheng, J.; Zhao, L.; Zhu, Z.; Zou, X.; Qin, L.; Wang, Y. Research on the Frequency Response and Dynamic Range of the Quadrature Fiber Optic Fabry–Perot Cavity Microphone Based on the Differential Cross Multiplication Demodulation Algorithm. Sensors 2021, 21, 6152. https://doi.org/10.3390/s21186152
Ren B, Cheng J, Zhao L, Zhu Z, Zou X, Qin L, Wang Y. Research on the Frequency Response and Dynamic Range of the Quadrature Fiber Optic Fabry–Perot Cavity Microphone Based on the Differential Cross Multiplication Demodulation Algorithm. Sensors. 2021; 21(18):6152. https://doi.org/10.3390/s21186152
Chicago/Turabian StyleRen, Baokai, Jin Cheng, Longjiang Zhao, Zhenghou Zhu, Xiaoping Zou, Lei Qin, and Yifei Wang. 2021. "Research on the Frequency Response and Dynamic Range of the Quadrature Fiber Optic Fabry–Perot Cavity Microphone Based on the Differential Cross Multiplication Demodulation Algorithm" Sensors 21, no. 18: 6152. https://doi.org/10.3390/s21186152
APA StyleRen, B., Cheng, J., Zhao, L., Zhu, Z., Zou, X., Qin, L., & Wang, Y. (2021). Research on the Frequency Response and Dynamic Range of the Quadrature Fiber Optic Fabry–Perot Cavity Microphone Based on the Differential Cross Multiplication Demodulation Algorithm. Sensors, 21(18), 6152. https://doi.org/10.3390/s21186152







