Design of a Multi-Point Kinematic Coupling for a High Precision Telescopic Simultaneous Measurement System
Abstract
:1. Introduction
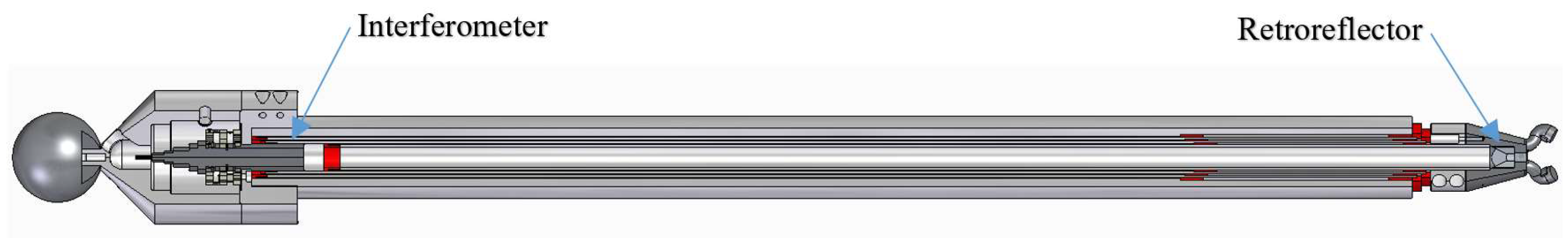
2. The High Precision Telescopic System
3. The Multi-Point Kinematic Coupling
3.1. Working Principle
3.2. Requirements of the System
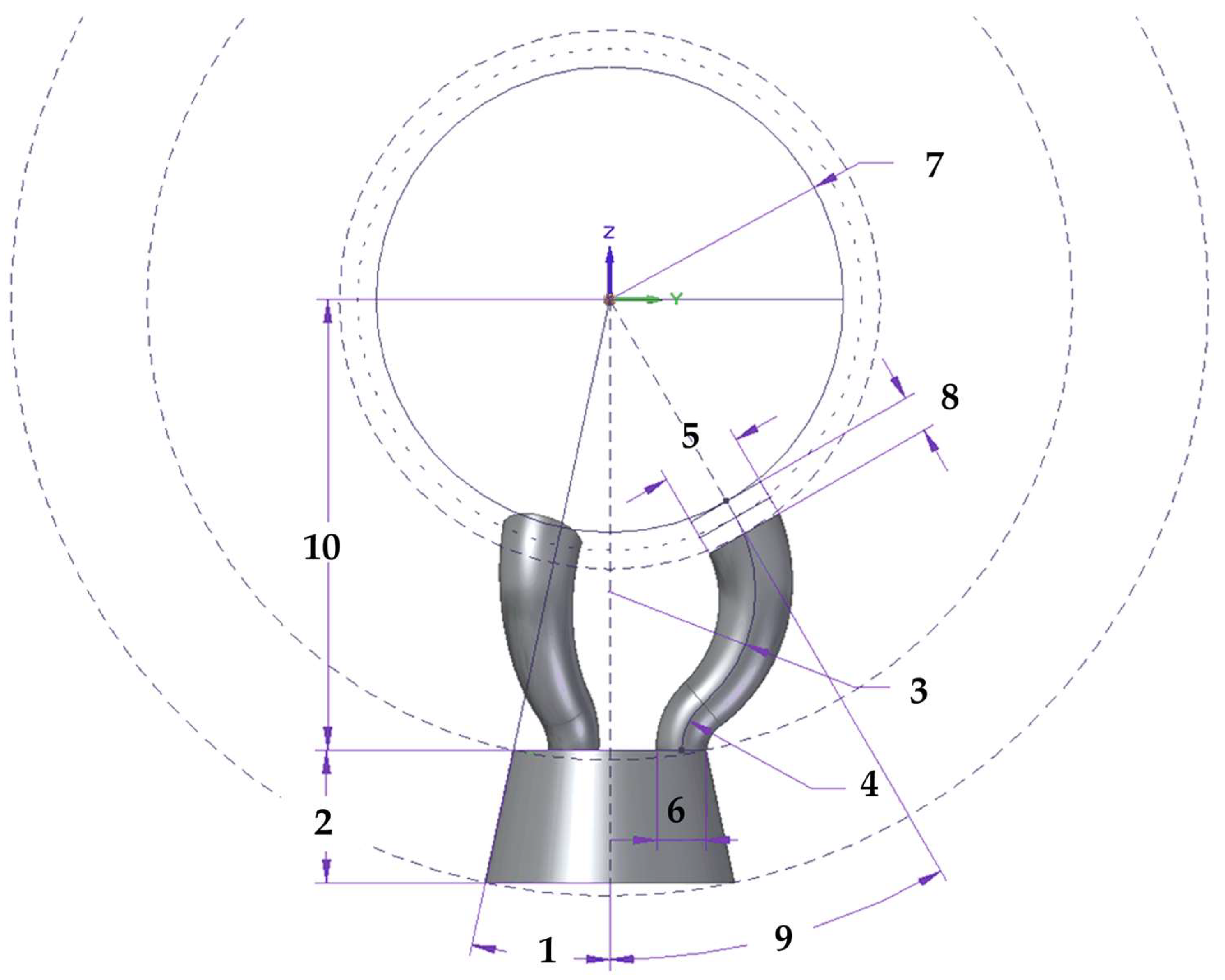
3.3. Kinematic Coupling Design Parameters
3.3.1. Tridents Magnets and Sphere Diameter Relation
3.3.2. Contact Point Angle
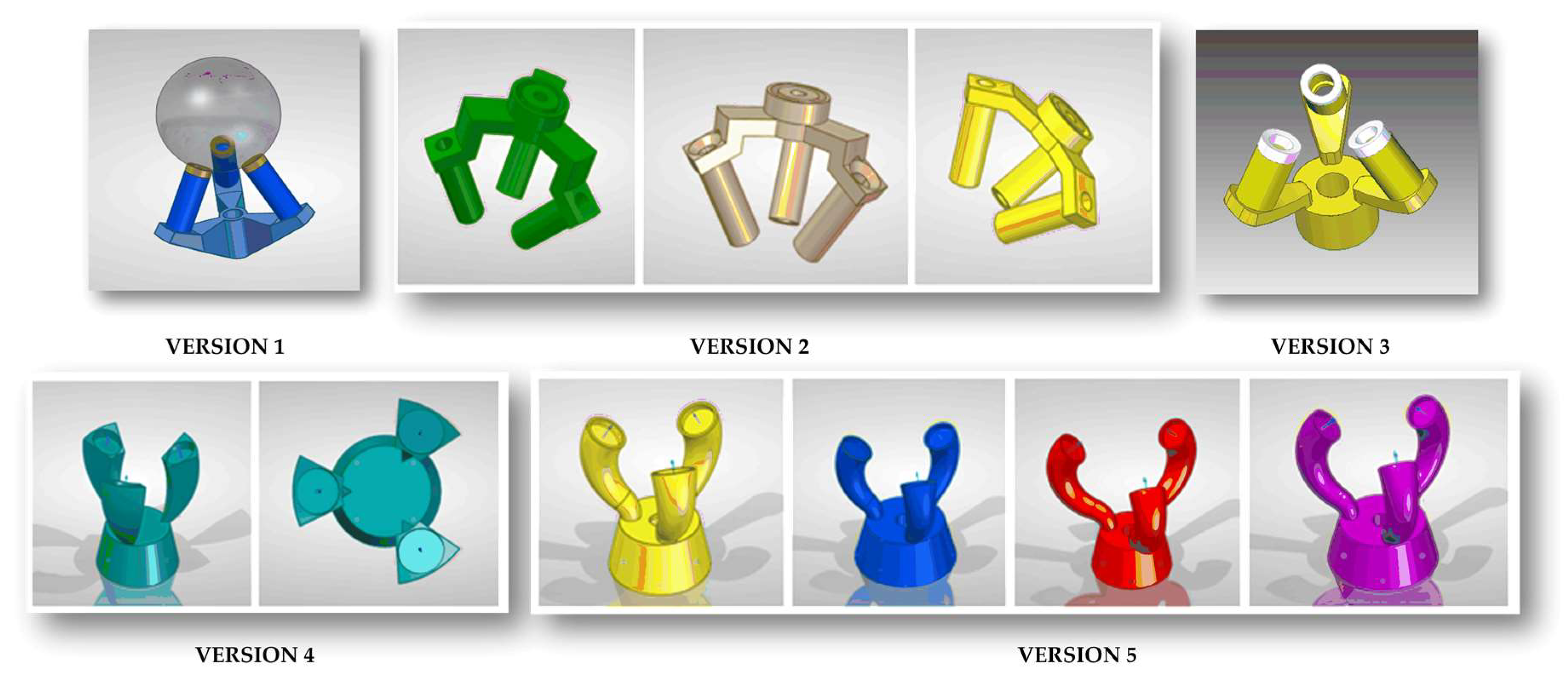
3.4. Kinematic Coupling Design Versions
4. Prototyping and Experimental Results
5. Discussion
- The trident has three fingers that generate a multi-point contact with the fixed sphere attached to the MT spindle nose. This was achieved with three magnets located at the ends of the fingers. They have enough force not to be detached from the fixed sphere along the MT travel in the different axis at high feed rates (up to 20 m/min). It also generates repulsion forces among the other trident’s fingers to avoid collisions or interferences that would hinder the correct working of the telescopic arms set.
- The design of the fingers assures their interlacing and the body allows the fingers not to collide up to a certain angle depending on the range of the MT to be verified. The fingers are short to minimize the distance between the fixed sphere attached to the MT spindle’s nose and the retroreflector. This allows maximizing the accuracy of the distance measurement.
- The design process took into account experimental studies to define the optimal contact point angle of the fingers, concluding that under the hypothesis that the system works in intermediate lubrication conditions and looking for a compact design, an angle of 30 degrees was chosen.
- The trident design was modified during the development considering the functional and dimensional requirements throughout different design versions, analyzing its main features and drawbacks.
- The final design also minimizes the deformation of the trident that could lead to a relative movement between the center of the sphere and the trident, as this would include an error in the laser measurement of the sphere-to-sphere distance.
- The final design of the kinematic coupling was mechanized by lathe machining and five-axis milling in stainless steel grade 304 L in a single part. Finally, repeatability tests were performed in the laboratory and in the workshop of the trident assembled on the telescopic arm. The results obtained showed repeatability values lower than 0.5 µm for the laboratory test, and 1.2 µm for the workshop test.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Slocum, A.H. Precision Machine Design; Prentice Hall: Hoboken, NJ, USA, 1992; ISBN 0872634922. [Google Scholar]
- Blanding, D.L. Exact Constraint: Machine Design Using Kinematic Principles; Asme Press: New York, NY, USA, 1999. [Google Scholar]
- Hale, L.C. Principles and Techniques for Designing Precision Machines; Massachusetts Institute of Technology: Cambridge, MA, USA, 1999. [Google Scholar]
- Evans, C.J. Precision engineering: An evolutionary perspective. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2012, 370, 3835–3851. [Google Scholar] [CrossRef] [PubMed]
- Slocum, A. Kinematic couplings: A review of design principles and applications. Int. J. Mach. Tools Manuf. 2010, 50, 310–327. [Google Scholar] [CrossRef] [Green Version]
- Hale, L.C.; Slocum, A.H. Optimal design techniques for kinematic couplings. Precis. Eng. 2001, 25, 114–127. [Google Scholar] [CrossRef]
- Schmiechen, P.; Slocum, A. Analysis of kinematic systems: A generalized approach. Precis. Eng. 1996, 19, 11–18. [Google Scholar] [CrossRef]
- William, S.; Thomson; Tait, P.G. Treatise on Natural Philosophy. 1879. Reprinted as Principles of Mechanics and Dynamics; Dover Publications: Mineola, NY, USA, 1962. [Google Scholar]
- Culpepper, M.L. Design and Application of Compliant Quasi-Kinematic Couplings; Massachsetts Institute of Technology: Cambridge, MA, USA, 2000. [Google Scholar]
- Culpepper, M.L.; Slocum, A.H.; DiBiasio, C.M. Design of detachable precision fixtures which utilize hard and lubricant coatings to mitigate wear and reduce friction hysteresis. In Proceedings of the 2003 Annual Meeting of the American Society for Precision Engineering, Portland, OR, USA, 26–31 October 2003; pp. 47–50. [Google Scholar]
- Slocum, A.H. Kinematic couplings for precision fixturing-Part I: Formulation of design parameters. Precis. Eng. 1988, 10, 85–91. [Google Scholar] [CrossRef]
- Slocum, A.H.; Donmez, A. Kinematic couplings for precision fixturing-Part 2: Experimental determination of repeatability and stiffness. Precis. Eng. 1988, 10, 115–121. [Google Scholar] [CrossRef]
- Esmaeili, S.M.; Mayer, J.R.R.; Sanders, M.P.; Dahlem, J.P.; Xing, K. Five-Axis Machine Tool Volumetric and Geometric Error Reduction by Indirect Geometric Calibration and Lookup Tables. J. Manuf. Sci. Eng. 2021, 143, 1–14. [Google Scholar] [CrossRef]
- Jiang, X.; Wang, L.; Liu, C. Geometric accuracy evaluation during coordinated motion of rotary axes of a five-axis machine tool. Meas. J. Int. Meas. Confed. 2019, 146, 403–410. [Google Scholar] [CrossRef]
- Huang, Y.B.; Fan, K.C.; Lou, Z.F.; Sun, W. A novel modeling of volumetric errors of three-axis machine tools based on Abbe and Bryan principles. Int. J. Mach. Tools Manuf. 2020, 151, 103527. [Google Scholar] [CrossRef]
- Deng, M.; Li, H.; Xiang, S.; Liu, P.; Feng, X.; Du, Z.; Yang, J. Geometric errors identification considering rigid-body motion constraint for rotary axis of multi-axis machine tool using a tracking interferometer. Int. J. Mach. Tools Manuf. 2020, 158, 103625. [Google Scholar] [CrossRef]
- Aguado, S.; Santolaria, J.; Samper, D.; Velazquez, J.; Aguilar, J.J. Empirical analysis of the efficient use of geometric error identification in a machine tool by tracking measurement techniques. Meas. Sci. Technol. 2016, 27, 035002. [Google Scholar] [CrossRef]
- Aguado, S.; Santolaria, J.; Samper, D.; Aguilar, J.J. Protocol for machine tool volumetric verification using commercial laser tracker. Int. J. Adv. Manuf. Technol. 2014, 75, 425–444. [Google Scholar] [CrossRef]
- Wang, S.; Cheung, C.F.; Kong, L. A Fiducial-Aided Recon gurable Artefact for the Estimation of Volumetric Errors of Multi-axis Ultra- Precision Machine Tools. Available online: https://www.researchsquare.com/article/rs-581329/v1 (accessed on 22 July 2021).
- Qiao, Y.; Chen, Y.; Yang, J.; Chen, B. A five-axis geometric errors calibration model based on the common perpendicular line (CPL) transformation using the product of exponentials (POE) formula. Int. J. Mach. Tools Manuf. 2017, 118–119, 49–60. [Google Scholar] [CrossRef]
- Miller, J.E.; Longstaff, A.P.; Parkinson, S.; Fletcher, S. Improved machine tool linear axis calibration through continuous motion data capture. Precis. Eng. 2017, 47, 249–260. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Zhang, P.; Deng, M.; Xiang, S.; Du, Z.; Yang, J. Volumetric error measurement and compensation of three-axis machine tools based on laser bidirectional sequential step diagonal measuring method. Meas. Sci. Technol. 2020, 31, 055201. [Google Scholar] [CrossRef]
- Wan, A.; Wang, Y.; Xue, G.; Chen, K.; Xu, J. Accurate kinematics calibration method for a large-scale machine tool. IEEE Trans. Ind. Electron. 2020, 68, 9832–9843. [Google Scholar] [CrossRef]
- Xiang, S.; Altintas, Y. Modeling and compensation of volumetric errors for five-axis machine tools. Int. J. Mach. Tools Manuf. 2016, 101, 65–78. [Google Scholar] [CrossRef]
- Aguilar, J.J.; Acero, R.; Brosed, F.J.; Santolaria, J. Development of a High Precision Telescopic Instrument Based on Simultaneous Laser Multilateration for Machine Tool Volumetric Verification. Sensors 2020, 20, 3798. [Google Scholar] [CrossRef] [PubMed]






| Device | Item | ID | Design Parameter | Unit |
|---|---|---|---|---|
| Group 1 | Trident’s body | 1 | Trident’s body angle | degree |
| 2 | Trident’s body height | mm | ||
| Trident’s finger | 3 | Finger’s upper arc radii | mm | |
| 4 | Finger’s lower arc radii | mm | ||
| 5 | Finger’s upper diameter | mm | ||
| 6 | Finger’s lower diameter | mm | ||
| Group 2 | Fixed sphere | 7 | Fixed sphere diameter | mm |
| Magnet | 5 | Magnet diameter | mm | |
| 8 | Magnet height | mm | ||
| Trident–Sphere | 9 | Contact point angle | degree | |
| 10 | Fixed sphere–trident body distance | mm |
| Contact Point Angle (Degrees) | 25 | 30 | 35 | 40 | 45 | 50 | 54.74 |
| Force (kg) | 1.04 | 1.00 | 0.96 | 0.90 | 0.83 | 0.74 | 0.68 |
| Prototype Version | Features/Advantages | Drawbacks |
|---|---|---|
| 1 | Trident divided in two parts, bolted body and fingers Simple finger and body geometry easy to machine | Interferences detected in the elements Collisions among fingers and tridents’ bodies |
| 2 | Trident divided in two parts, bolted body and fingers Simple finger and body geometry easy to machine Three different trident heights for easy interlacing Body with rectangular sections easy to machine | Collisions due to rectangular sections Rotation is avoided No tridents interlacing as they are at different angles |
| 3 | Trident divided in two parts, bolted body and fingers Smooth and curved design lines in the trident body Design of the fingers support above the body trying to avoid collision between the fingers and the bodies | Interference between elements Collisions among fingers and not between tridents’ bodies Lightweight finger support design |
| Difficult machining Lack of rigidity of the assembly | ||
| 4 | Trident in one single part (body and fingers) Smoother, curved design lines in the trident body | Collisions due to straight lateral finger surfaces Lightweight finger-to-body design |
| Fingers come out vertically from the body to reduce collisions | Lack of rigidity of the assembly Medium difficulty in machining | |
| Simple design for easy turning and milling | ||
| 5 | Same trident concept as version 4 The fingers came out completely vertical from the body thanks to an arch at the bottom Reduction in the finger lower radius versus its upper radius Rounded finger’s lateral surface Trident fingers interlace easily in pairs | Limited miniaturization to enable the three trident’s fingers interlacing simultaneously Trident fingers interlace in pairs, but triple interlacing is hardly needed in operation Complex lathe machining and 5-axis milling for trident manufacturing are required |
 |  |  |  |  |  |  |  | |
| Version | 5.1 | 5.2 | 5.3 | 5.4 | 5.5 | 5.6 | 5.7 | 5.8 |
| Fixed sphere diameter (mm) | 38.1 | 38.1 | 38.1 | 38.1 | 38.1 | 38.1 | 38.1 | 38.1 |
| Magnet diameter (mm) | 6 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 |
| Magnet height (mm) | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 |
| Contact point angle (°) | 30 | 30 | 30 | 30 | 30 | 30 | 30 | 30 |
| Sphere–body distance (mm) | 36.9 | 36.9 | 36.9 | 36.9 | 36.9 | 36.9 | 29.1 | 29.1 |
| Finger arc radii (mm) | 9.4/5 | 5.6/15 | 8.6/5 | 4.5/15 | 8.7/5 | 3.5/15 | 5.7/4 | 3.5/8.5 |
| Trident body angle (°) | 12 | 12 | 12 | 12 | 12 | 12 | 15 | 15 |
| Finger upper diameter (mm) | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 | 6.5 |
| Finger lower diameter (mm) | 2 | 2 | 4 | 4 | 6 | 6 | 5.1 | 5.1 |
| Trident body height (mm) | 12 | 12 | 12 | 12 | 12 | 12 | 12 | 12 |
| Deformation (µm) | 43.2 | 45.5 | 5.87 | 6.2 | 1.92 | 2.07 | 1.31 | 1.36 |
| Telescopic Arm | Laboratory | Workshop | ||||
|---|---|---|---|---|---|---|
| Mean (mm) | Std (mm) | Semi-Range (mm) | Mean (mm) | Std (mm) | Semi-Range (mm) | |
| 1 | 470.8224 | 0.0003 | 0.00045 | 572.3533 | 0.0008 | 0.0012 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acero, R.; Aguilar, J.J.; Brosed, F.J.; Santolaria, J.; Aguado, S.; Pueo, M. Design of a Multi-Point Kinematic Coupling for a High Precision Telescopic Simultaneous Measurement System. Sensors 2021, 21, 6365. https://doi.org/10.3390/s21196365
Acero R, Aguilar JJ, Brosed FJ, Santolaria J, Aguado S, Pueo M. Design of a Multi-Point Kinematic Coupling for a High Precision Telescopic Simultaneous Measurement System. Sensors. 2021; 21(19):6365. https://doi.org/10.3390/s21196365
Chicago/Turabian StyleAcero, Raquel, Juan José Aguilar, Francisco Javier Brosed, Jorge Santolaria, Sergio Aguado, and Marcos Pueo. 2021. "Design of a Multi-Point Kinematic Coupling for a High Precision Telescopic Simultaneous Measurement System" Sensors 21, no. 19: 6365. https://doi.org/10.3390/s21196365
APA StyleAcero, R., Aguilar, J. J., Brosed, F. J., Santolaria, J., Aguado, S., & Pueo, M. (2021). Design of a Multi-Point Kinematic Coupling for a High Precision Telescopic Simultaneous Measurement System. Sensors, 21(19), 6365. https://doi.org/10.3390/s21196365







