Analysis and Validation of Ultrasonic Probes in Liquid Level Monitoring Systems
Abstract
:1. Introduction
2. Theory and Methods
2.1. Reflection and Transmission of Ultrasonic Waves
2.2. Design of Liquid Level Monitoring System
2.3. Selection of Ultrasonic Probe
3. Results and Discussion
3.1. Sensor Diameter Analysis
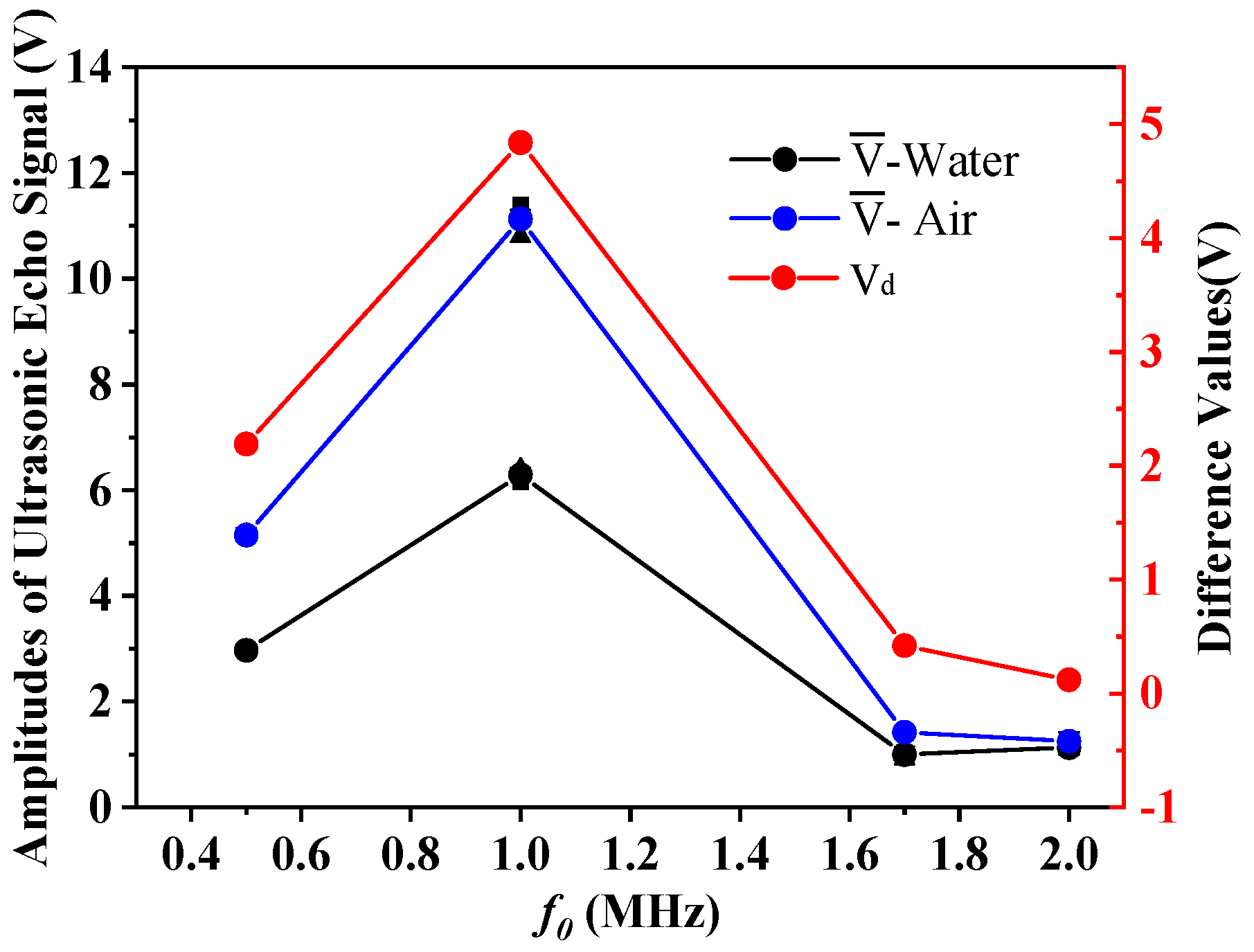
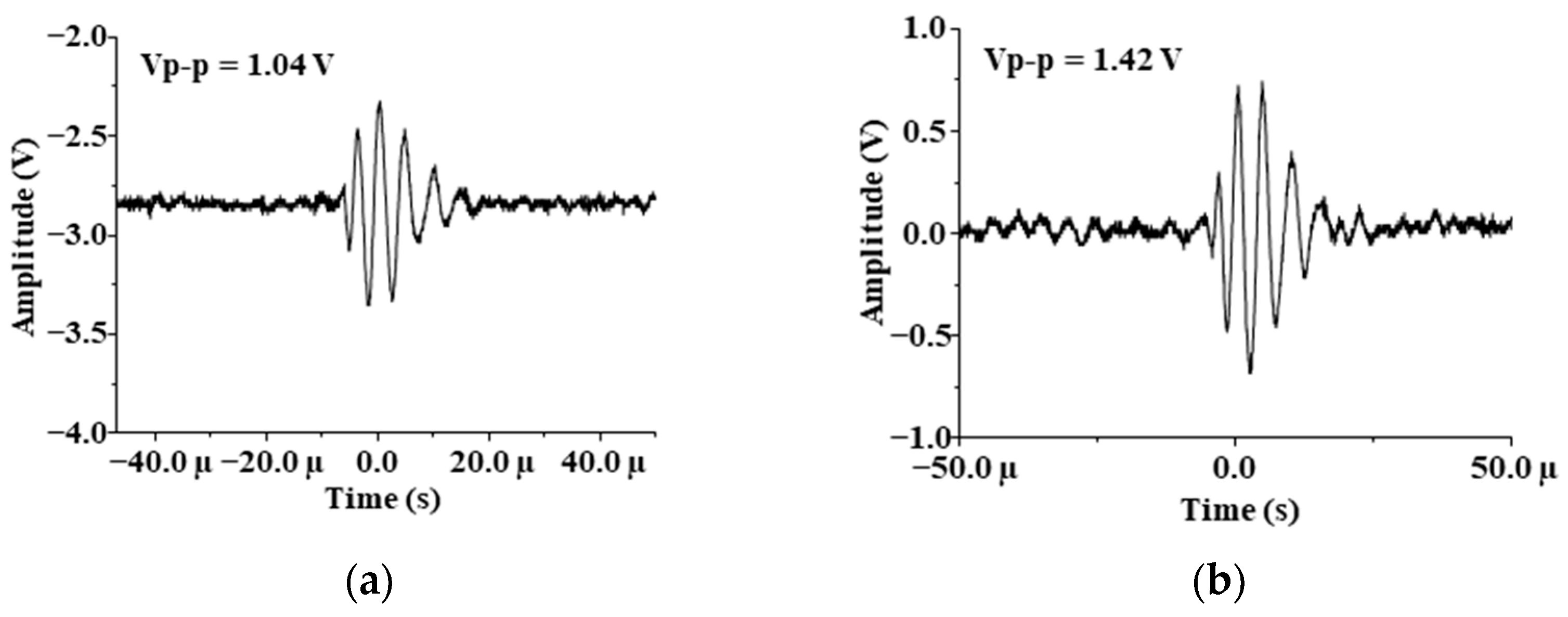
3.2. Sensor Natural Frequency Analysis
3.3. Emitted Excitation Signal Amplitude Analysis
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yumei, L. Research and Design on an Intelligent Level Measurement System. J. Sichuan Univ. Sci. Eng. 2009, 22, 108–110. [Google Scholar]
- Sakharov, V.E.; Kuznetsov, S.A.; Zaitsev, B.D.; Kuznetsova, I.E.; Joshi, S.G. Liquid level sensor using ultrasonic Lamb waves. Ultrasonics 2003, 41, 319–322. [Google Scholar] [CrossRef]
- Pal, A.; Kalyan, U.P.; Harika, C.M.; Vasuki, B. Capacitive Sensor for Level Measurement in Hopper/Silos—Experimental Evaluation. In Proceedings of the International Conference on Intelligent Computing, Instrumentation and Control Technologies (ICICICT), Kannur, India, 5–6 July 2019. [Google Scholar]
- Ren, K. The influence by temperature and product density to accuracy of magnetostrictive level gauge. In Proceedings of the IEEE International Conference on Information Acquisition, Hefei, China, 21–25 June 2004; pp. 248–251. [Google Scholar]
- Lee, C.; Zheng, Y.; Chang, H. Directional fiber-optic level meter based on a micro-air-bubble drifted in a liquid core fiber Fabry-Pérot interferometer. In Proceedings of the Conference on Lasers and Electro Optics, San Jose, CA, USA, 9–14 June 2013; pp. 1–2. [Google Scholar]
- Camposmartin, J.M.; Capelsanchez, M.C.; Perezpresas, P.; Fierro, J.L.G. Oxidative processes of desulfurization of liquid fuels. J. Chem. Technol. Biotechnol. 2010, 85, 879–890. [Google Scholar] [CrossRef] [Green Version]
- Khairi, M.T.M.; Ibrahim, S.; Yunus, M.A.M.; Faramarzi, M. Contact and non-contact ultrasonic measurement in the food industry: A review. Meas. Sci. Technol. 2016, 27, 012001. [Google Scholar] [CrossRef]
- Berketis, K.; Tzetzis, D.; Hogg, P.J. Noncontact ultrasonics used for impact damage detection on long-term water-immersed GFRP composites. Int. J. Microstruct. Mater. Prop. 2009, 4, 34–44. [Google Scholar] [CrossRef] [Green Version]
- Tenoudji, F.C.; Citerne, J.M.; Dutilleul, H.; Busquet, D. Non-contact ultrasonic defect imaging in composites. In AIP Conference Proceedings; AIP Publishing LLC: Melville, NY, USA, 2016; Volume 1706, p. 080003. [Google Scholar]
- Alvarenga, A.V.; Silva, C.E.R.; Costafelix, R.P.B. Monte Carlo uncertainty assessment of ultrasonic beam parameters from immersion transducers used to non-destructive testing. Ultrasonics 2016, 69, 144–151. [Google Scholar] [CrossRef]
- Zhang, M.; Li, S. A method of the untouched ultrasonic liquid level measurement with high precision. In Proceedings of the 2010 International Conference on Computer Application and System Modeling (ICCASM 2010), Taiyuan, China, 22–24 October 2010; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2010; Volume 7, pp. V7–V144. [Google Scholar]
- Gan, T.H.; Pallav, P.; Hutchins, D.A. Non-contact ultrasonic quality measurements of food products. J. Food Eng. 2006, 77, 239–247. [Google Scholar] [CrossRef]
- Zakaria, Z.; Idroas, M.; Samsuri, A.; Adam, A.A. Ultrasonic instrumentation system for Liquefied Petroleum Gas level monitoring. J. Nat. Gas Sci. Eng. 2017, 45, 428–435. [Google Scholar] [CrossRef]
- Haohao, H.; Junqiao, X. A method of liquid level measurement based on ultrasonic echo characteristics. In Proceedings of the International Conference on Computer Application and System Modeling (ICCASM 2010), Taiyuan, China, 22–24 October 2010; Institute of Electrical and Electronics Engineers (IEEE): Piscataway, NJ, USA, 2010; Volume 11, pp. V11–V682. [Google Scholar]
- Hauptmann, P.; Hoppe, N.; Püttmer, A. Application of ultrasonic sensors in the process industry. Meas. Sci. Technol. 2002, 13, R73. [Google Scholar] [CrossRef]
- Yanjun, Z.; Bin, Z.; Liang, Z.; Yunchao, L.; Xiaolong, G.; Zhaojun, L. Liquid Level Measurement Model Outside of Closed Containers Based on Continuous Sound Wave Amplitude. Sensors 2018, 18, 2516. [Google Scholar]
- Danilov, V.N. Selection of the parameters of a probe during immersion ultrasonic inspection. Russ. J. Nondestruct. Test. 2007, 43, 336–343. [Google Scholar] [CrossRef]
- Lanoye, R.; Vermeir, G.; Lauriks, W.; Kruse, R.; Mellert, V. Measuring the free field acoustic impedance and absorption coefficient of sound absorbing materials with a combined particle velocity-pressure sensor. J. Acoust. Soc. Am. 2006, 119, 2826–2831. [Google Scholar] [CrossRef]
- Chen, L.; Dong, X.; Han, J.; Ye, P. Development of a Ultrasonic Instrument for the Sealed Container’s Liquid Level Measurement. In Proceedings of the World Congress on Intelligent Control & Automation, Dalian, China, 21–23 June 2006; IEEE: Piscataway, NJ, USA, 2006. [Google Scholar]
- Ohbuchi, T.; Mizutani, K.; Wakatsuki, N.; Nishimiya, K.; Masuyama, H. Reconstruction of Three-Dimensional Sound Field from Two-Dimensional Sound Field Using Optical Computerized Tomography and Near-Field Acoustical Holography. Jpn. J. Appl. Phys. 2009, 48, 07GC03. [Google Scholar] [CrossRef]
- Gao, W.; Liu, W.; Hu, Y.; Wang, J. Study of Ultrasonic Near-Field Region in Ultrasonic Liquid-Level Monitoring System. Micromachines 2020, 11, 763. [Google Scholar] [CrossRef] [PubMed]
- Gunawan, A.I.; Saijo, Y.; Hozumi, N.; Yoshida, S.; Kobayashi, K.; Yamamoto, S. Acoustic impedance estimation using calibration curve for scanning acoustic impedance microscope. In Proceedings of the 2016 International Conference on Knowledge Creation and Intelligent Computing (KCIC), Manado, Indonesia, 15–17 November 2016; pp. 240–245. [Google Scholar]
- Pilch, A.; Kamisiński, T.; Zastawnik, M. Comparison of Pressure and Intensity Methods in Evaluating the Directional Diffusion Coefficient. Acta Phys. Pol. A 2013, 123, 1054–1058. [Google Scholar] [CrossRef]
- Liu, Y.; Li, H. Wave reflection and transmission by porous breakwaters: A new analytical solution. Coast. Eng. 2013, 78, 46–52. [Google Scholar] [CrossRef]
- Arnold, F.J.; Mühlen, S.S. The influence of the thickness of non-piezoelectric pieces on pre-stressed piezotransducers. Ultrasonics 2003. [Google Scholar] [CrossRef]
- Fei, L.; Zhang, S.; Luo, J.; Geng, X.; Xu, Z.; Shrout, T.R. [111]-oriented PIN-PMN-PT crystals with ultrahigh dielectric permittivity and high frequency constant for high-frequency transducer applications. J. Appl. Phys. 2016, 120, 074105. [Google Scholar]
- Mace, B.R.; Halkyard, C.R.; El-Khatib, H.M. Real-time measurement of wave components and intensity in a beam in the presence of a near field. J. Sound Vib. 2005, 286, 507–527. [Google Scholar] [CrossRef]
- Oberhardt, B.J.; Smith, S.W.; Zara, J.M. Systems and Methods for Improving the Performance of Sensing Devices Using Oscillatory Devices. U.S. Patent No. 6,849,910, 1 February 2005. [Google Scholar]
- Moghanizadeh, A.; Farzi, A. Effect of heat treatment on an AISI 304 austenitic stainless steel evaluated by the ultrasonic attenuation coefficient. Materialpruefung: Werkstoffe und Bautle. Forsch. Pruf. Anwend. 2016, 58, 448–452. [Google Scholar]
- Bin, Z.; Yue-Juan, W.; Wen-Yi, L.; Yan-Jun, Z.; Zong, Y.; Li-Hui, Z.; Ji-Jun, X. A Liquid Level Measurement Technique Outside a Sealed Metal Container Based on Ultrasonic Impedance and Echo Energy. Sensors 2017, 17, 185. [Google Scholar]
- Tasinkevych, Y.; Danicki, E.J. Wave generation and scattering by periodic baffle system in application to beam-forming analysis. Wave Motion 2011, 48, 130–145. [Google Scholar] [CrossRef]






| Z1 (g/cm2·s) | ZW (g/cm2·s) | ZA (g/cm2·s) |
|---|---|---|
| 32 × 105 | 1.48 × 105 | 40 |
| D (mm) | f0 (MHz) |
|---|---|
| 10 | 1 |
| 15 | 0.5, 1, 1.7, 2 |
| 25 | 1 |
| 38 | 1 |
| D (mm) | Medium | V1 (V) | V2 (V) | V3 (V) | Vd (V) | ||
|---|---|---|---|---|---|---|---|
| 10 | Water | 14.4 | 14.2 | 14 | 14.2 | 0.13 | 4.2 |
| Air | 18.4 | 18.6 | 18.2 | 18.4 | 0.13 | ||
| 15 | Water | 6.16 | 6.40 | 6.32 | 6.29 | 0.09 | 4.84 |
| Air | 11.40 | 10.8 | 11.20 | 11.13 | 0.22 | ||
| 25 | Water | 1.52 | 1.14 | 1.12 | 1.26 | 0.17 | 0.13 |
| Air | 1.72 | 1.24 | 1.2 | 1.39 | 0.22 | ||
| 38 | Water | 0.432 | 0.504 | 0.448 | 0.46 | 0.03 | 0.09 |
| Air | 0.648 | 0.528 | 0.488 | 0.55 | 0.06 |
| f0 (MHz) | Medium | V1 (V) | V2 (V) | V3 (V) | Vd (V) | ||
|---|---|---|---|---|---|---|---|
| 0.5 | Water | 2.94 | 2.98 | 2.98 | 2.97 | 0.02 | 2.19 |
| Air | 5.12 | 5.16 | 5.18 | 5.15 | 0.02 | ||
| 1 | Water | 6.16 | 6.4 | 6.32 | 6.29 | 0.09 | 4.84 |
| Air | 11.4 | 10.8 | 11.2 | 11.13 | 0.22 | ||
| 1.7 | Water | 1.04 | 0.92 | 1.04 | 1.00 | 0.05 | 0.42 |
| Air | 1.44 | 1.42 | 1.4 | 1.42 | 0.01 | ||
| 2 | Water | 1.16 | 1.1 | 1.14 | 1.13 | 0.02 | 0.12 |
| Air | 1.2 | 1.24 | 1.32 | 1.25 | 0.04 |
| VT (V) | Medium | V1 (V) | V2 (V) | V3 (V) | Vd (V) | ||
|---|---|---|---|---|---|---|---|
| 5 | Water | 3.60 | 3.52 | 3.28 | 3.47 | 0.12 | 2.13 |
| Air | 5.92 | 5.56 | 5.32 | 5.60 | 0.21 | ||
| 10 | Water | 5.12 | 5.60 | 5.36 | 5.36 | 0.16 | 3.92 |
| Air | 9.28 | 9.36 | 9.20 | 9.28 | 0.05 | ||
| 15 | Water | 6.16 | 6.40 | 6.32 | 6.29 | 0.09 | 4.84 |
| Air | 11.40 | 10.80 | 11.20 | 11.13 | 0.22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, W.; Liu, W.; Li, F.; Hu, Y. Analysis and Validation of Ultrasonic Probes in Liquid Level Monitoring Systems. Sensors 2021, 21, 1320. https://doi.org/10.3390/s21041320
Gao W, Liu W, Li F, Hu Y. Analysis and Validation of Ultrasonic Probes in Liquid Level Monitoring Systems. Sensors. 2021; 21(4):1320. https://doi.org/10.3390/s21041320
Chicago/Turabian StyleGao, Wanjia, Wenyi Liu, Fei Li, and Yanjun Hu. 2021. "Analysis and Validation of Ultrasonic Probes in Liquid Level Monitoring Systems" Sensors 21, no. 4: 1320. https://doi.org/10.3390/s21041320
APA StyleGao, W., Liu, W., Li, F., & Hu, Y. (2021). Analysis and Validation of Ultrasonic Probes in Liquid Level Monitoring Systems. Sensors, 21(4), 1320. https://doi.org/10.3390/s21041320






