Research on Rainfall Monitoring Based on E-Band Millimeter Wave Link in East China
Abstract
:1. Introduction
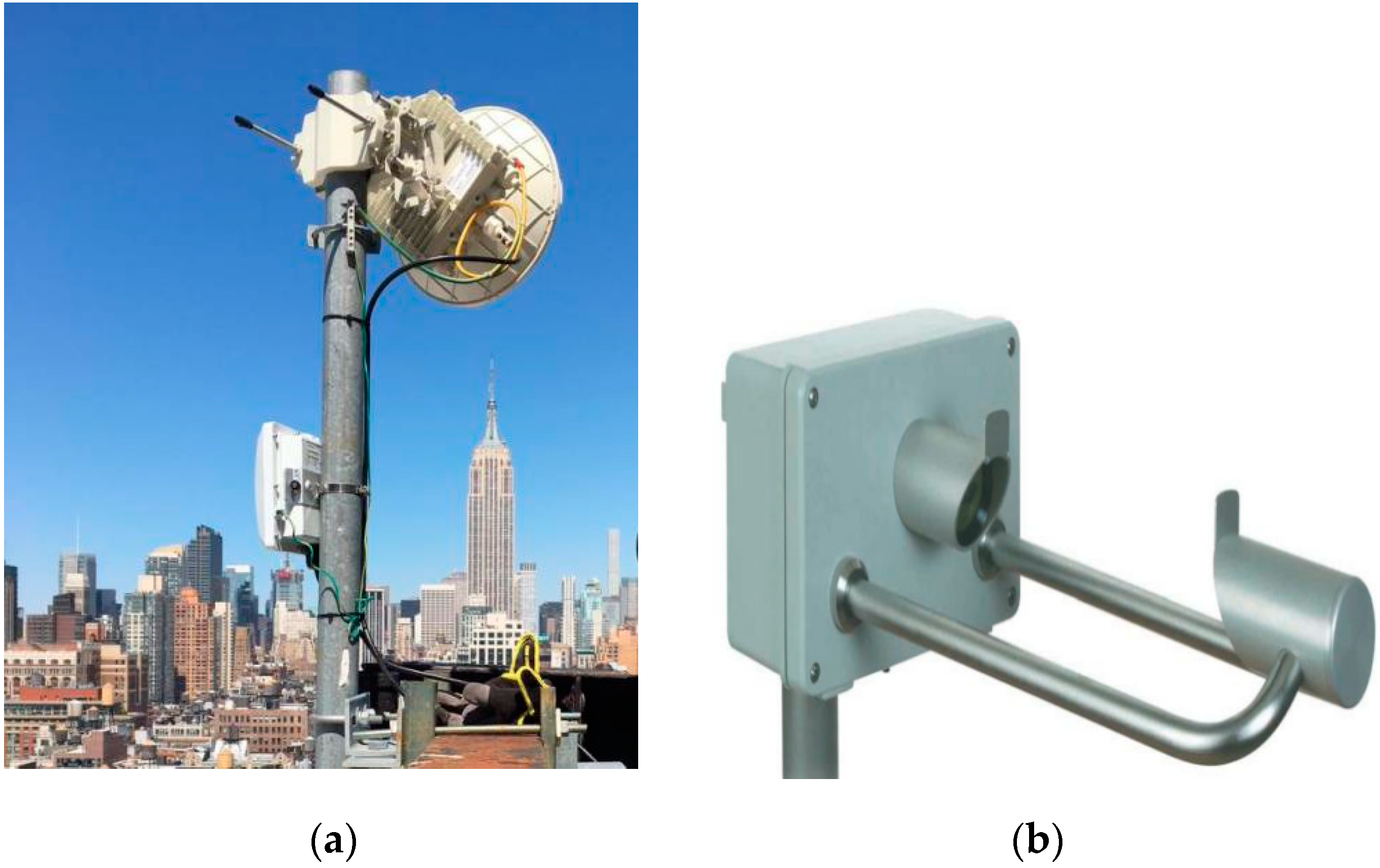
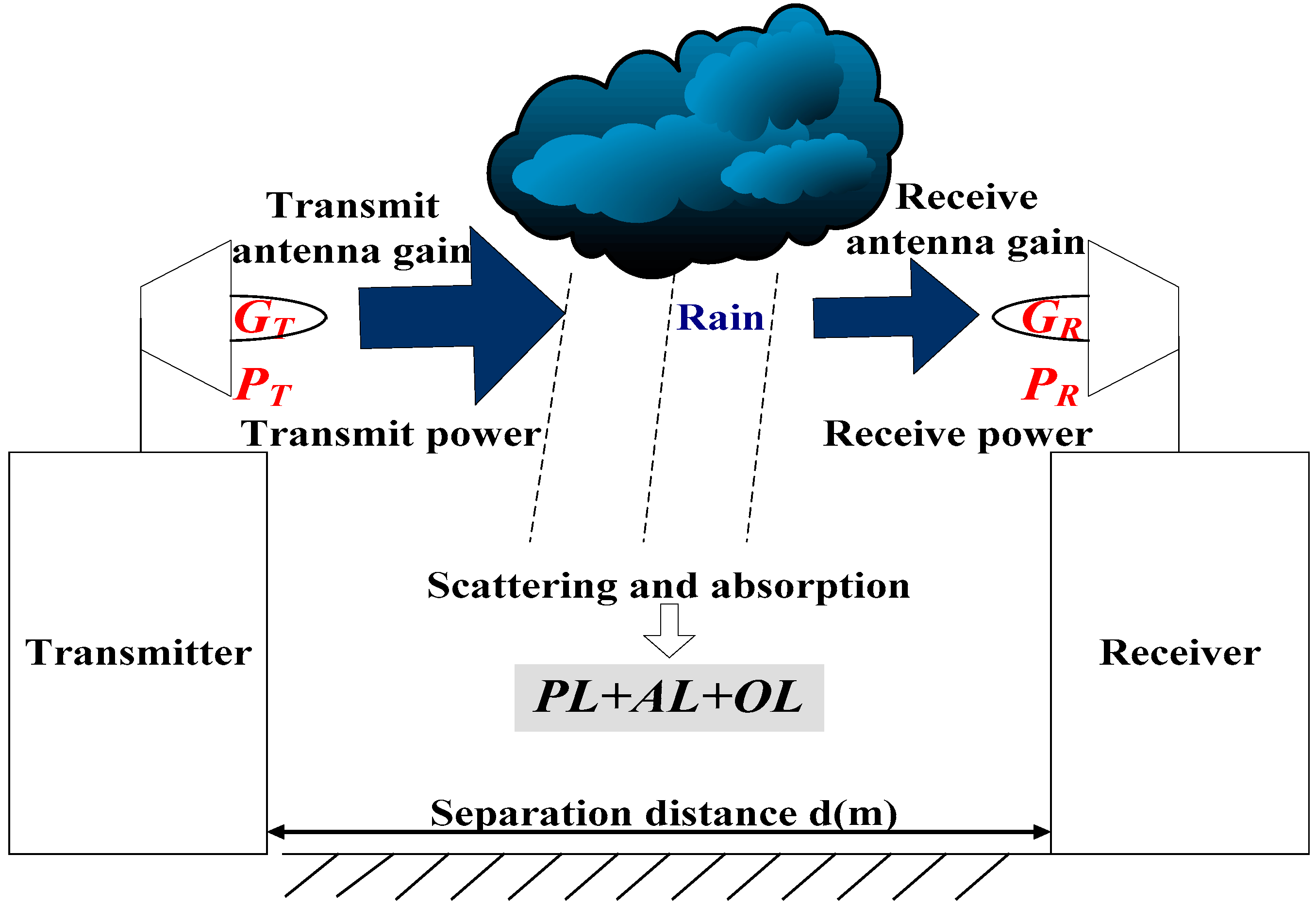
2. Experimental Equipment and Link Propagation Characteristics
3. Data Processing
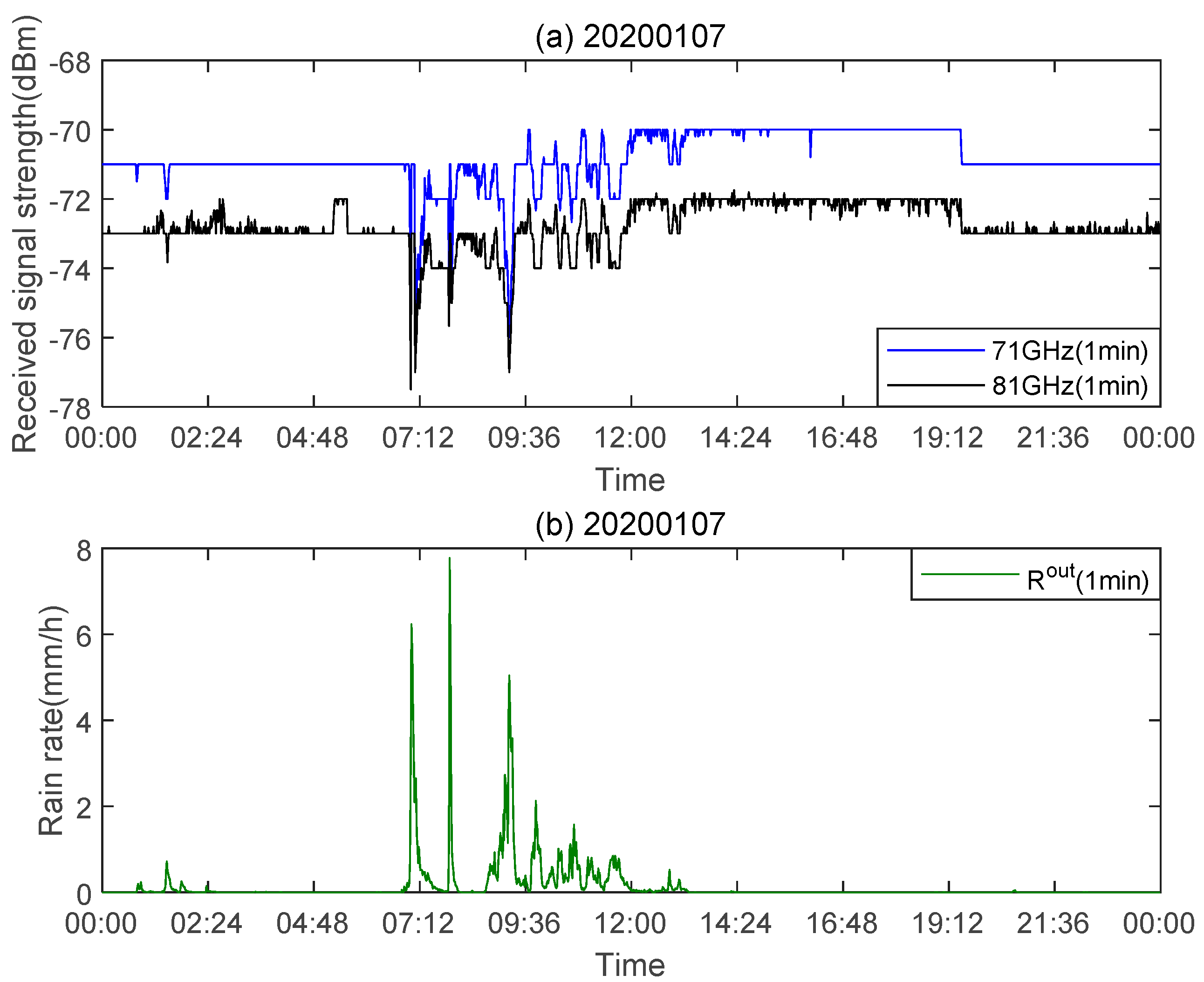
3.1. Post-Processing
3.2. Attenuation Baseline Calculation
4. Rainfall Rate Inversion
4.1. Raindrop Size Distribution
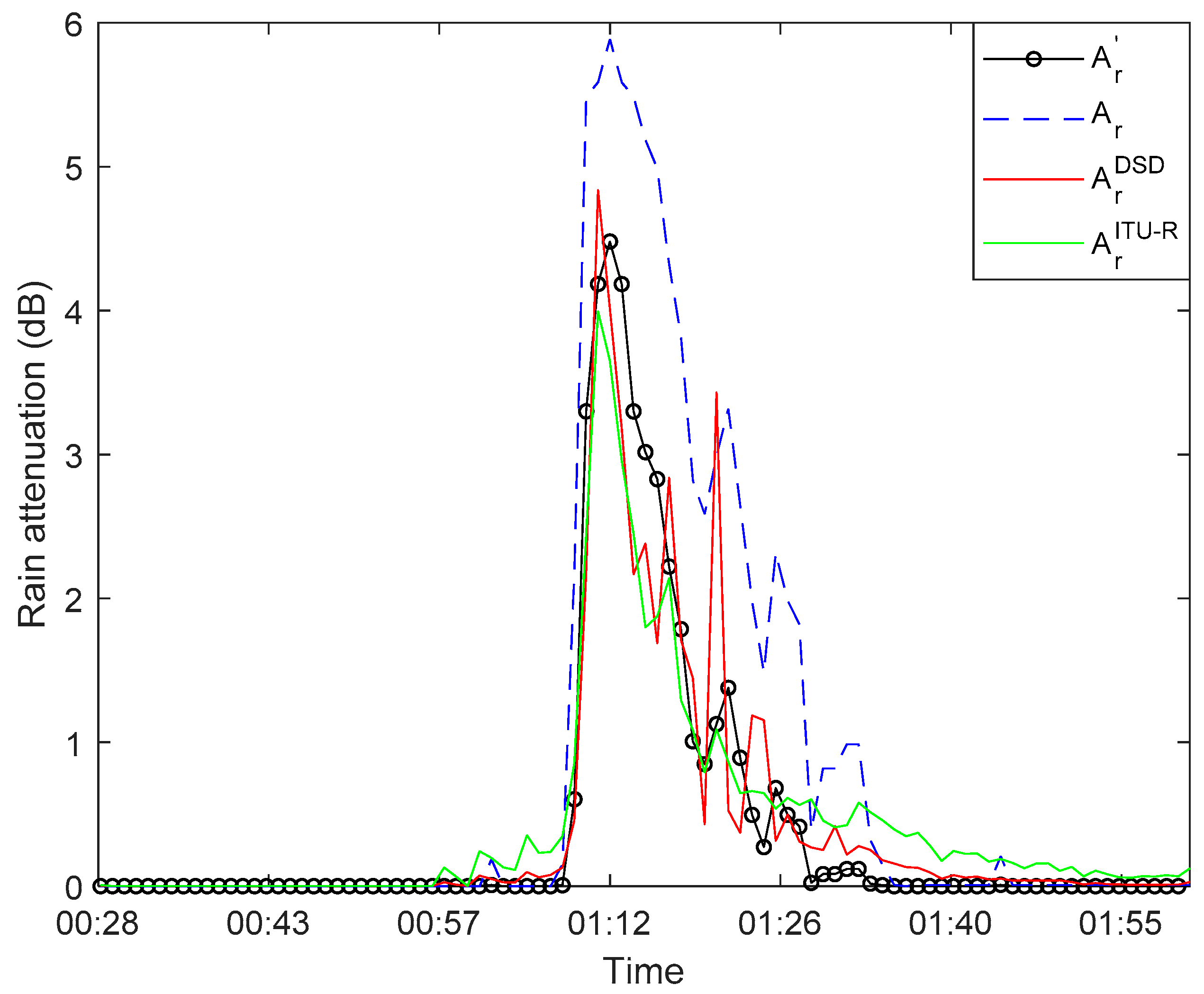
4.2. Wet Antenna Correction
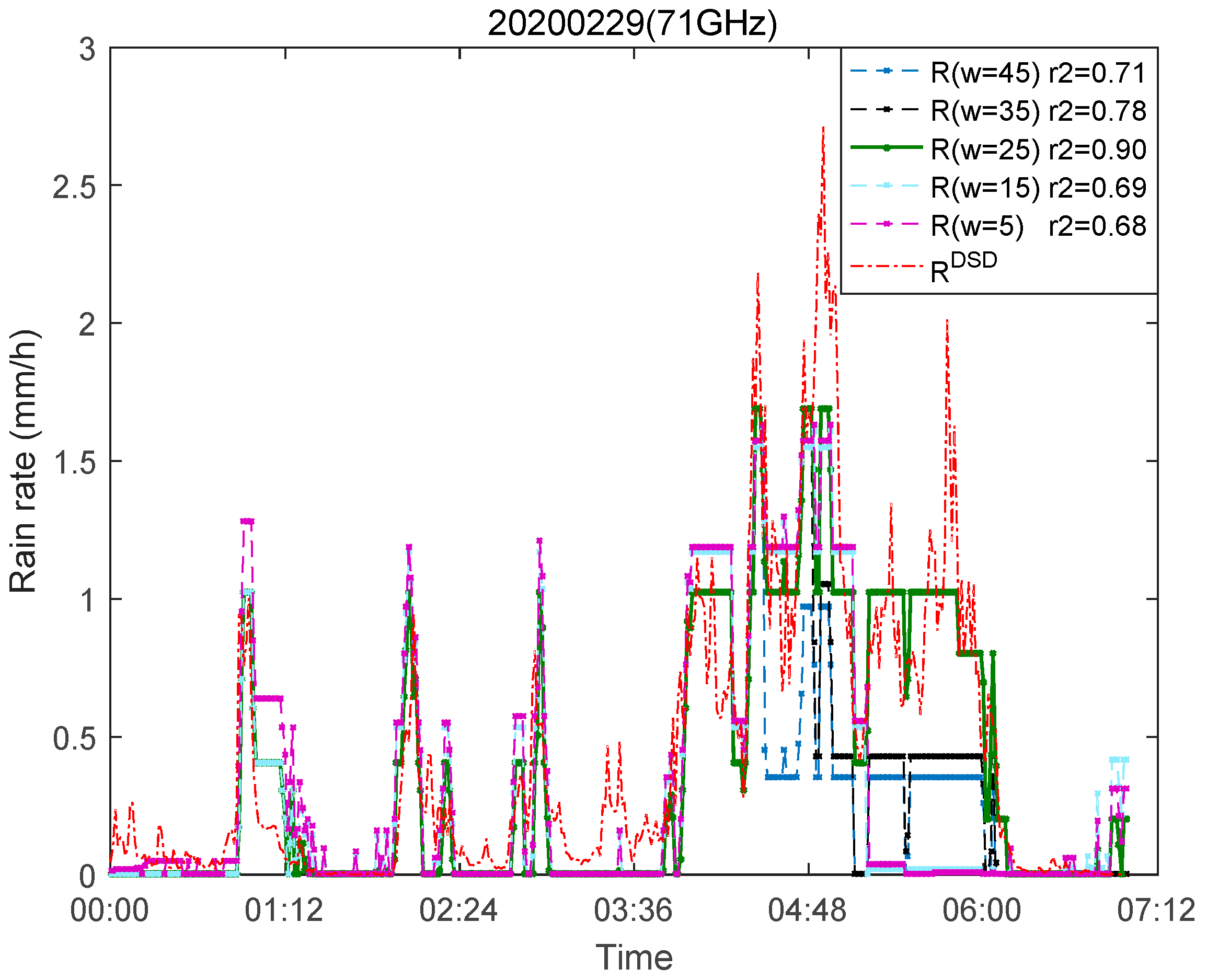
4.3. Rainfall Rate Inversion Result
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hou, A.Y.; Kakar, R.K.; Neeck, S.; Azarbarzin, A.A.; Kummerow, C.D.; Kojima, M.; Oki, R.; Nakamura, K.; Iguchi, T. The global precipitation measurement mission. Bull. Am. Meteorol. Soc. 2014, 95, 701–722. [Google Scholar] [CrossRef]
- Sevruk, B. Rainfall measurement: Gauges. In Encyclopedia of Hydrological Sciences; Wiley & Sons Ltd.: Chichester, UK, 2005; pp. 529–536. [Google Scholar]
- Berne, A.; Krajewski, W.F. Radar for hydrology: Unful-filled promise or unrecognized potential. Adv. Water Resour. 2013, 51, 357–366. [Google Scholar] [CrossRef]
- Huang, J.; Cao, Y.; Raimundo, X.; Cheema, A.; Salous, S. Rain statistics investigation and rain attenuation modeling for millimeter wave short-range fixed links. IEEE Access 2019, 7, 156110–156120. [Google Scholar] [CrossRef]
- Han, C.; Duan, S. Impact of atmospheric parameters on the propagated signal power of millimeter-wave bands based on real measurement data. IEEE Access 2019, 7, 113626–113641. [Google Scholar] [CrossRef]
- Rappaport, T.S. Millimeter wave mobile communications for 5G cellular: It will work! IEEE Access 2013, 1, 335–349. [Google Scholar] [CrossRef]
- Messer, H.A.; Zinevich, A.; Alpert, P. Environmental monitoring by wireless communication networks. Science 2006, 312, 713. [Google Scholar] [CrossRef] [Green Version]
- Imhoff, R.O.; Overeem, A.; Brauer, C.C.; Leijnse, H.; Weerts, A.H.; Uijlenhoet, R. Rainfall nowcasting using commercial microwave links. Geophys. Res. Lett. 2020, 47, e2020GL089365. [Google Scholar] [CrossRef]
- Han, C.; Bi, Y.; Duan, S.; Lu, G. Rain rate retrieval test from 25-GHz, 28-GHz, and 38-GHz millimeter-wave link measurement in Beijing. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2019, 12, 2835–2847. [Google Scholar] [CrossRef]
- Graf, M.; Chwala, C.; Polz, J.; Kunstmann, H. Rainfall estimation from a German-wide commercial microwave link network:Optimized processing and validation for 1 year of data. Hydrol. Earth Syst. Sci. 2020, 24, 2931–2950. [Google Scholar] [CrossRef]
- Zinevich, A.; Messer, H.; Alpert, P. Prediction of rainfall intensity measurement errors using commercial microwave communication links. Atmos. Meas. Tech. 2010, 3, 1385–1402. [Google Scholar] [CrossRef] [Green Version]
- Messer, H.; Zinevich, A.; Alpert, P. Environmental sensor networks using existing wireless communication systems for rainfall and wind velocity measurements. IEEE Instrum. Meas. Mag. 2012, 15, 32–38. [Google Scholar] [CrossRef]
- Uijlenhoet, R.; Overeem, A.; Leijnse, H. Opportunistic remote sensing of rainfall using microwave links from cellular communication networks. Wiley Interdiscip. Rev. Water 2018, 5, e1289. [Google Scholar]
- Chwala, C.; Gmeiner, A.; Qiu, W.; Hipp, S.; Nienaber, D.; Siart, U. Precipitation observation using microwave backhaul links in the alpine and pre-alpine region of Southern Germany. Hydrol. Earth Syst. Sci. 2012, 16, 2647–2661. [Google Scholar] [CrossRef] [Green Version]
- Ahuna, L.N.; Afullo, T.J.; Alonge, A.A. 30-second and one-minute rainfall rate modelling and conversion for millimetric wave propagation in South Africa. SAIEE Afr. Res. J. 2016, 107, 17–29. [Google Scholar] [CrossRef]
- Ostrometzky, J.; Raich, R.; Bao, L.; Hansryd, J.; Messer, H. The wet-antenna effect-a factor to be considered in future communication networks. IEEE Trans. Antennas Propag. 2018, 66, 315–322. [Google Scholar] [CrossRef]
- Han, C.Z.; Huo, J.; Gao, Q.Q.; Su, G.Y.; Wang, H. Rainfall monitoring based on next-generation millimeter-wave backhaul technologies in a dense urban environment. Remote Sens. 2020, 12, 1045. [Google Scholar] [CrossRef] [Green Version]
- Pu, K.; Liu, X.; He, H. Wet antenna attenuation model of E-band microwave links based on the LSTM Algorithm. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 1586–1590. [Google Scholar] [CrossRef]
- Al-Samman, A.M.; Mohamed, M.; Ai, Y.; Cheffena, M.; Azmi, M.H.; Rahman, T.A. Rain attenuation measurements and analysis at 73 GHz E-band link in tropical region. IEEE Commun. Lett. 2020, 24, 1368–1372. [Google Scholar] [CrossRef]
- Luini, L.; Roveda, G.; Zaffaroni, M.; Costa, M.; Riva, C.G. The impact of rain on short E-band radio links for 5G mobile systems: Experimental results and prediction models. IEEE Trans. Antennas Propag. 2020, 68, 3124–3134. [Google Scholar] [CrossRef]
- Shrestha, S.; Choi, D.Y. Rain attenuation statistics over millimeter wave bands in south korea. J. Atmos. Sol. Terr. Phys. 2017, 152, 1–10. [Google Scholar] [CrossRef] [Green Version]
- Luini, L.; Roveda, G.; Zaffaroni, M.; Costa, M.; Riva, C. EM wave propagation experiment at E band and D band for 5G wireless systems: Preliminary results. In Proceedings of the 12th European Conference on Antennas and Propagation (EuCAP 2018), London, UK, 9–13 April 2018; pp. 1–5. [Google Scholar]
- Norouzian, F.; Marchetti, E.; Gashinova, M.; Hoare, E.; Constantinou, C.; Gardner, P.; Cherniakov, M. Rain attenuation at millimetre wave and low-thz frequencies. IEEE Trans. Antennas Propag. 2019, 68, 421–431. [Google Scholar] [CrossRef]
- Wu, C.L.; Chau, K.W. Prediction of rainfall time series using modular soft computingmethods. Eng. Appl. Artif. Intell. 2013, 26, 997–1007. [Google Scholar] [CrossRef] [Green Version]
- Siklu Carrier-Grade 1000Mbps E-Band radio Datasheet. Available online: https://go.siklu.com/eh-1200-series-datasheet-new-lp (accessed on 6 February 2020).
- Thies Clima Laser Precipitation Monitor. Available online: https://thiesclima.cn/cnweb/index.php/products/precipitation. (accessed on 6 February 2020).
- Daniels, R.C.; Heath, R.W.; Murdock, J.N.; Rappaport, T.S. Millimeter Wave Wireless Communications: Systems and Circuits; Prentice Hall: Upper Saddle River, NJ, USA, 2014; pp. 4–7. [Google Scholar]
- ITU-R. P. 838–3 (International Telecommunication Union Radio Communication Bureau Propagation Recommendation). In Specific Attenuation Model. for Rain for Use in Prediction Methods; ITU-R: Geneva, Switzerland, 2005. [Google Scholar]
- Ostrometzky, J.; Messer, H. Dynamic Determination of the Baseline Level in Microwave Links for Rain Monitoring from Minimum Attenuation Values. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 24–33. [Google Scholar] [CrossRef]
- Schleiss, M.; Berne, A. Identification of dry and rainy periods using telecommunication microwave links. IEEE Geosci. Remote Sens. Lett. 2010, 7, 611–615. [Google Scholar] [CrossRef]
- Lam, H.Y.; Luini, L.; Din, J.; Capsoni, C.; Panagopoulos, A.D. Investigation of rain attenuation in equatorial Kuala Lumpur. IEEE Antennas Wirel. Propag. Lett. 2012, 11, 1002–1005. [Google Scholar] [CrossRef]
- Marshall, J.S.; Palmer, W.M. The distribution of raindrops with size. J. Atmos. Sci. 1948, 5, 165–166. [Google Scholar] [CrossRef]
- Chwala, C.; Kunstmann, H. Commercial microwave link networks for rainfall observation: Assessment of the current status and future challenges. Wiley Interdiscipl. Rev. Water 2019, 6, e1337. [Google Scholar] [CrossRef] [Green Version]
- Mie, G. Beiträge zur Optik trüber Medien, speziell kolloidaler Metal-lösungen. Ann. Phy. 1908, 25, 377–445. [Google Scholar] [CrossRef]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; Wiley: New York, NY, USA, 1998; pp. 381–428. [Google Scholar]
- Liebe, H.J.; Hufford, G.A.; Cotton, M.G. Propagation modeling of moist air and suspended water/ice particles at frequencies below 1000 GHz. In Proceedings of the Electromagnetic Wave Propagation Panel Symposium, AGARD Conference Proceedings, Palma, Spain, 17–20 May 1993. [Google Scholar]
- Hansryd, J.; Li, Y.; Chen, J.; Ligander, P. Long term path attenuation measurement of the 71-76 GHz band in a 70/80 GHz microwave link. In Proceedings of the Fourth European Conference on Antennas and Propagation, Barcelona, Spain, 12–16 April 2010; pp. 1–4. [Google Scholar]
- Crane, R.K. Analysis of the effects of water on the ACTS propagation terminal antenna. IEEE Trans. Antennas Propag. 2002, 50, 954–965. [Google Scholar] [CrossRef]
- Jacobson, M.D.; Hogg, D.C.; Snider, J.B. Wet reflectors in millimeter-wave radiometry-experiment and theory. IEEE Trans. Geosci. Remote Sens. 1986, 24, 784–791. [Google Scholar] [CrossRef]
- Islam, M.; Tharek, A.; Din, J.; Chebil, J. Measurement of wet antenna effect on microwave propagation at 23, 26 and 38 GHz. In Proceedings of the IEEE Antennas and Propagation Society International Symposium, Transmitting Waves of Progress to the Next Millennium, 2000, Held in conjunction with: USNC/URSI National Radio Science Meeting, Salt Lake City, UT, USA, 16–21 July 2000; pp. 2094–2098. [Google Scholar]
- Minda, H.; Nakamura, K. High temporal resolution path-average rain gauge with 50-GHz band microwave. J. Atmos. Ocean. Technol. 2005, 22, 165–179. [Google Scholar] [CrossRef]
- Kharadly, M.M.Z.; Ross, R. Effect of wet antenna attenuation on propagation data statistics. IEEE Trans. Antennas Propag. 2001, 49, 1183–1191. [Google Scholar] [CrossRef]
- Garcia-Rubia, J.M.; Riera, J.M.; Garcia-del-Pino, P.; Bernarroch, A. Attenuation measurements and propagation modeling in the W-band. IEEE Trans. Antennas Propag. 2013, 61, 1860–1867. [Google Scholar] [CrossRef]
- Messer, H. Capitalizing on cellular technology-opportunities and challenges for near ground weather monitoring. Environments 2018, 5, 73. [Google Scholar] [CrossRef] [Green Version]




| Parameter | 71–76/81–86 GHz |
|---|---|
| Transmit power | +7 dBm |
| Level resolution | 0.1 dB |
| Tx and Rx antenna gain | 50 dBi |
| Antenna polarization | vertical |
| Antenna size | 0.65 m |
| Bandwidth | 250 MHz |
| Throughput | 1 Gbps full duplex |
| Link budget (BER (Bit Error Rate) = 10−6) | 196 dB (including 2ft antennas’ gain) |
| Modulation | QPSK (Quadrature Phase Shift Keying) |
| Date | Duration (h) | Accumulated Rainfall Rates (mm/h) | Maximum Rainfall Rates (mm/h) |
|---|---|---|---|
| 7 January 2020 | 6.5 | 196.7 | 7.7 |
| 22 January 2020 | 4.5 | 274 | 3.6 |
| 24 January 2020 | 5.5 | 20 | 0.4 |
| 25 January 2020 | 9 | 154 | 1 |
| 28 February 2020 | 17 | 407 | 9.4 |
| 29 February 2020 | 7 | 248 | 3.6 |
| 9 March 2020 | 8 | 337 | 4.2 |
| 10 March 2020 | 3 | 64 | 2.7 |
| 13 March 2020 | 7 | 13 | 0.4 |
| 21 March 2020 | 5 | 220 | 5.6 |
| Date (Dry) | Frequency | ||
|---|---|---|---|
| 31 December 2019 | 71 GHz | 69.2 | 0.03 |
| 81 GHz | 70.5 | 0.05 | |
| 19 January 2020 | 71 GHz | 67.8 | 0.03 |
| 81 GHz | 69.9 | 0.04 | |
| 24 February 2020 | 71 GHz | 68.6 | 0.08 |
| 81 GHz | 70.1 | 0.08 | |
| 7 March 2020 | 71 GHz | 68.4 | 0.09 |
| 81 GHz | 70.4 | 0.09 | |
| 11 March 2020 | 71 GHz | 67.9 | 0.05 |
| 81 GHz | 69.9 | 0.06 | |
| 18 March 2020 | 71 GHz | 68.5 | 0.08 |
| 81 GHz | 69.9 | 0.08 |
| Date | Frequency | ||||
|---|---|---|---|---|---|
| 28 February 2020 | 71 GHz | 0.90 | 4.02 | 0.89 | 3.44 |
| 81 GHz | 0.91 | 0.99 | 0.89 | 0.68 | |
| 29 February 2020 | 71 GHz | 0.88 | 0.57 | 0.86 | 0.40 |
| 81 GHz | 0.89 | 3.69 | 0.87 | 2.21 | |
| 9 March 2020 | 71 GHz | 0.59 | 2.94 | 0.61 | 1.95 |
| 81 GHz | 0.57 | 0.78 | 0.60 | 0.53 | |
| 10 March 2020 | 71 GHz | 0.55 | 0.35 | 0.53 | 0.26 |
| 81 GHz | 0.67 | 0.24 | 0.65 | 0.23 | |
| 13 March 2020 | 71 GHz | 0.59 | 0.58 | 0.61 | 0.47 |
| 81 GHz | 0.63 | 0.48 | 0.63 | 0.40 | |
| 21 March 2020 | 71 GHz | 0.87 | 0.52 | 0.87 | 0.36 |
| 81 GHz | 0.84 | 3.14 | 0.84 | 2.22 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, S.; Han, C.; Huo, J.; Cai, W.; Zhang, Y.; Li, P.; Zhang, G.; Ji, B.; Zhou, J. Research on Rainfall Monitoring Based on E-Band Millimeter Wave Link in East China. Sensors 2021, 21, 1670. https://doi.org/10.3390/s21051670
Zheng S, Han C, Huo J, Cai W, Zhang Y, Li P, Zhang G, Ji B, Zhou J. Research on Rainfall Monitoring Based on E-Band Millimeter Wave Link in East China. Sensors. 2021; 21(5):1670. https://doi.org/10.3390/s21051670
Chicago/Turabian StyleZheng, Siming, Congzheng Han, Juan Huo, Wenbing Cai, Yinhui Zhang, Peng Li, Gaoyuan Zhang, Baofeng Ji, and Jiafeng Zhou. 2021. "Research on Rainfall Monitoring Based on E-Band Millimeter Wave Link in East China" Sensors 21, no. 5: 1670. https://doi.org/10.3390/s21051670
APA StyleZheng, S., Han, C., Huo, J., Cai, W., Zhang, Y., Li, P., Zhang, G., Ji, B., & Zhou, J. (2021). Research on Rainfall Monitoring Based on E-Band Millimeter Wave Link in East China. Sensors, 21(5), 1670. https://doi.org/10.3390/s21051670









