Characteristics of an Eight-Quadrant Corner Reflector Involving a Reconfigurable Active Metasurface
Abstract
:1. Introduction
2. Proposed Miniaturized Active Frequency-Selective Surface
3. Electromagnetic Scattering Characteristics of the Proposed Eight-Quadrant Corner Reflector
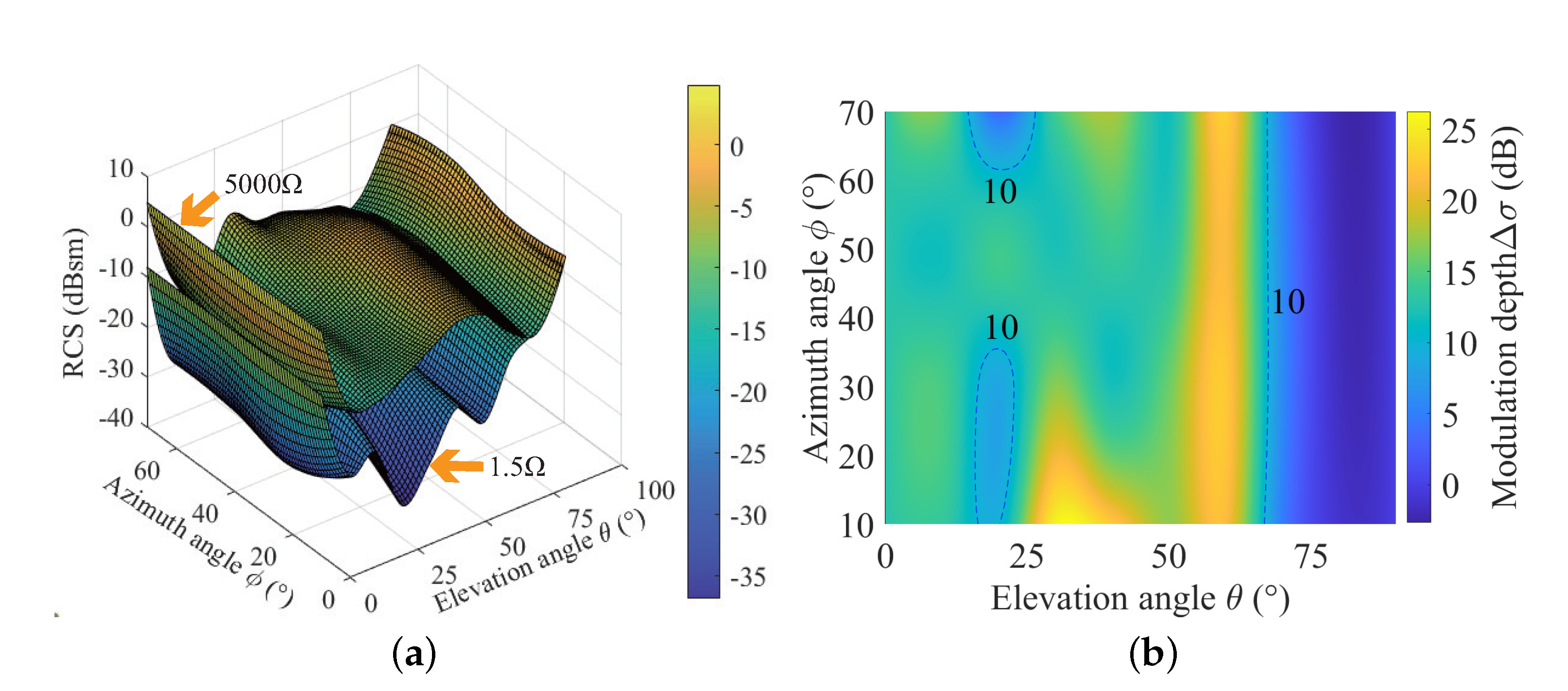
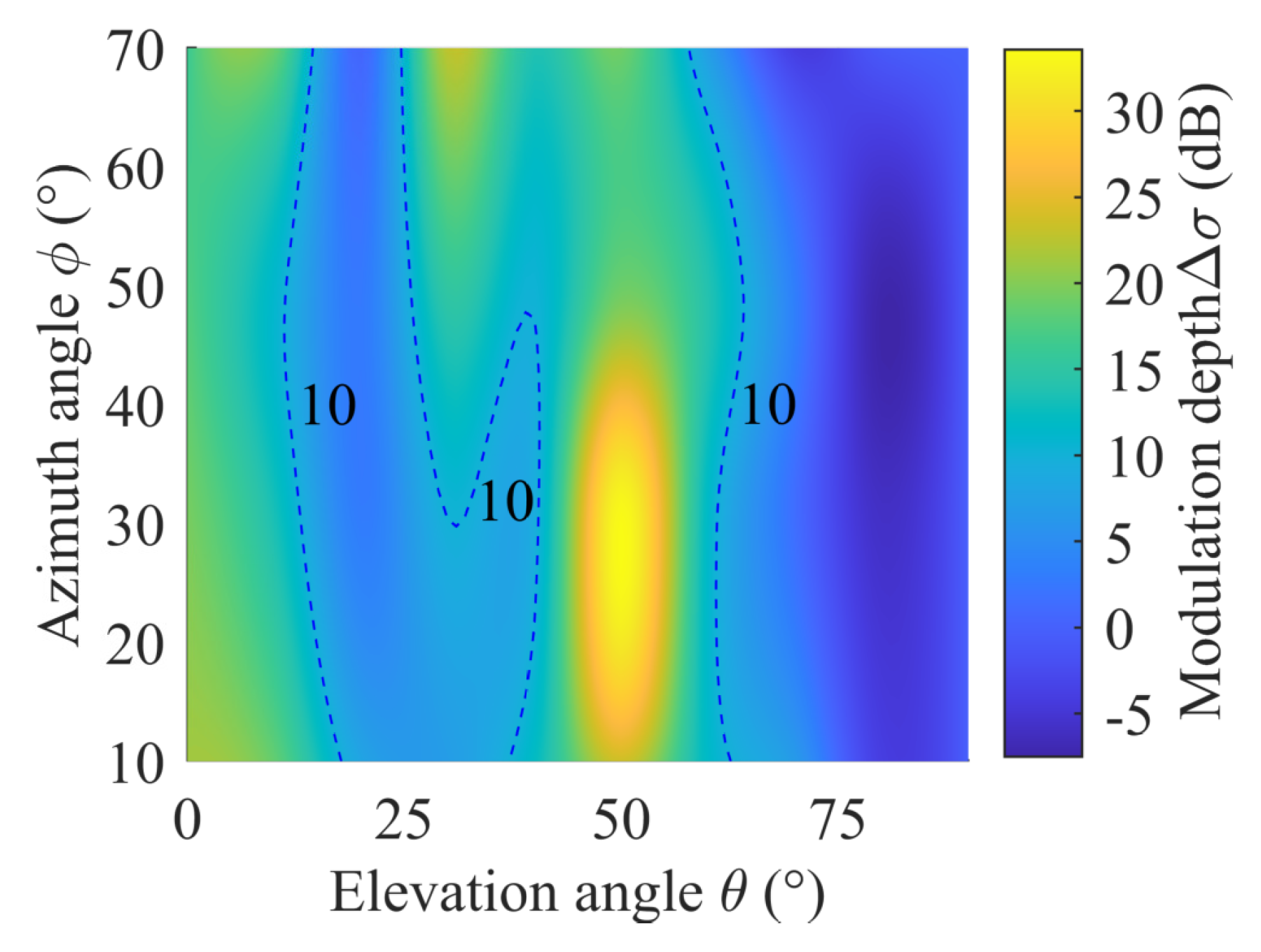
3.1. RCS Modulation Depth
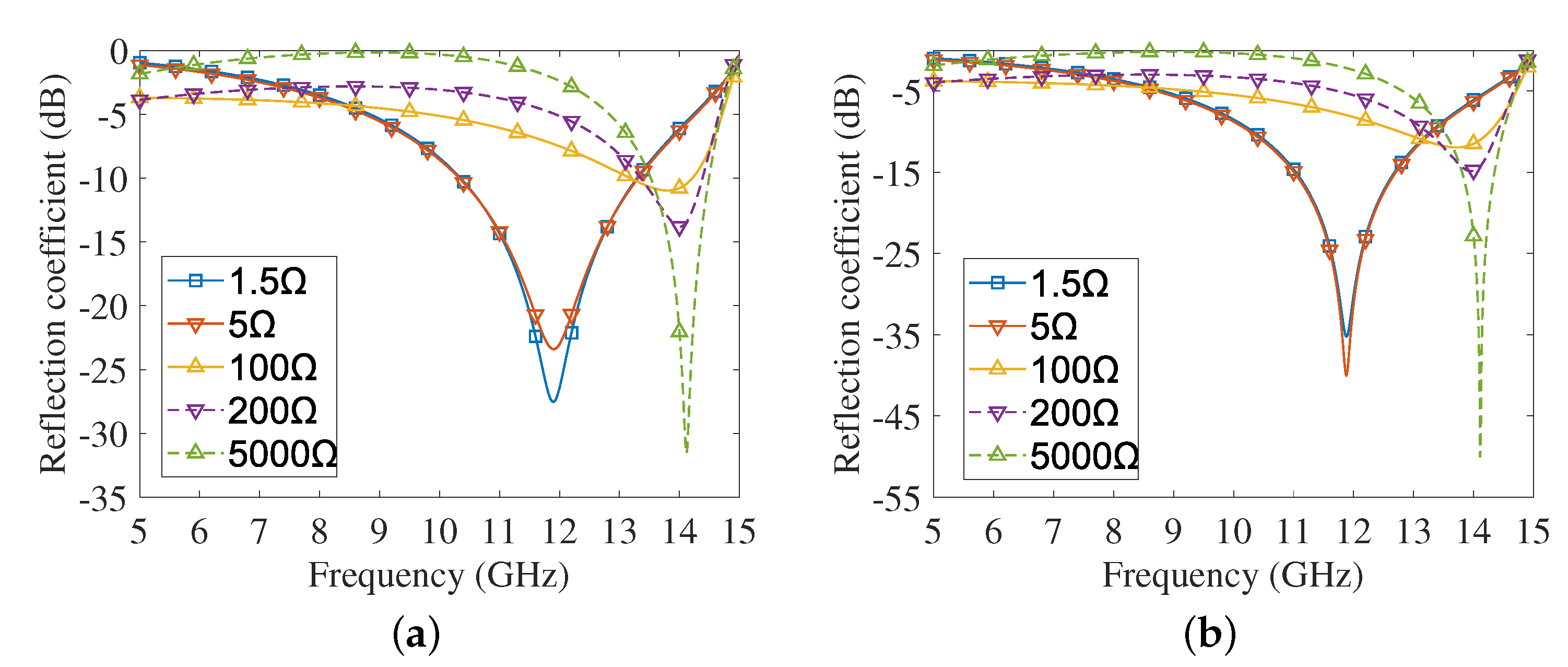
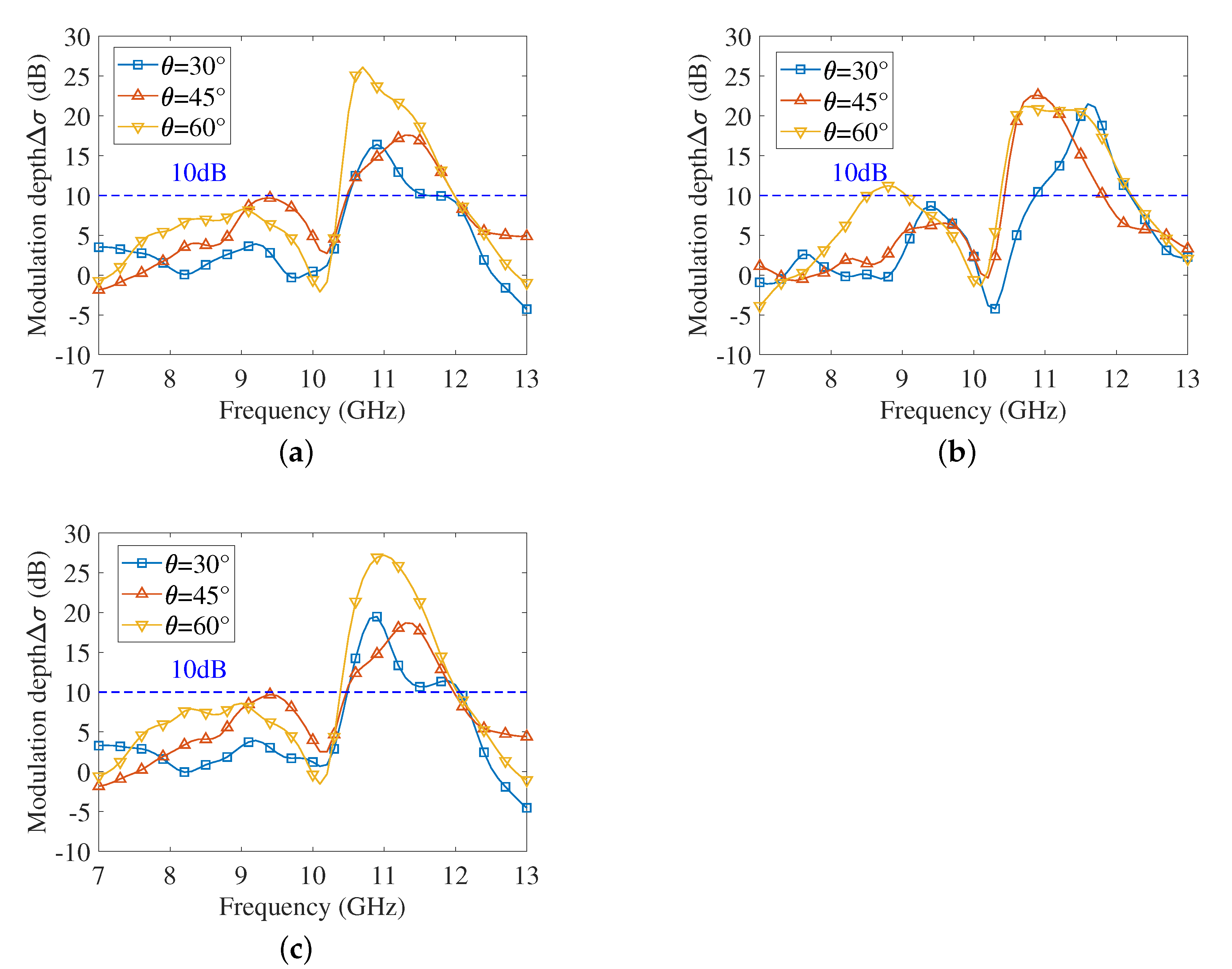
3.2. Adjustable Bandwidth
3.3. Comparison with the Traditional Eight-Quadrant Corner Reflector
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kraus, J.D. The corner-reflector antenna. Proc. IRE 1940, 28, 513–519. [Google Scholar] [CrossRef]
- Kraus, J.D.; Marhefka, R.J. Corner Reflectors, 3rd ed.; McGraw-Hill: New York, NY, USA, 2002; pp. 352–365. [Google Scholar]
- Skolnik, M.I. Introduction to Radar Systems, 2nd ed.; McGraw-Hill: Singapore, 1981; pp. 553–558. [Google Scholar]
- Sterr, U.O.; Olver, A.D.; Clarricoats, P.J.B. Variable beamwidth corner reflector antenna. Electron. Lett. 1998, 34, 1050–1051. [Google Scholar] [CrossRef]
- Sazegar, M.; Zheng, Y.; Kohler, C.; Maune, H.; Nikfalazar, M.; Binder, J.R.; Jakoby, R. Beam Steering Transmitarray Using Tunable Frequency Selective Surface with Integrated Ferroelectric Varactors. IEEE Trans. Antennas Propag. 2012, 60, 5690–5699. [Google Scholar] [CrossRef]
- Li, M.; Behdad, N. A Third-Order Bandpass Frequency Selective Surface with a Tunable Transmission Null. IEEE Trans. Antennas Propag. 2012, 60, 2109–2113. [Google Scholar] [CrossRef]
- Yang, Y.; Li, W.; Salama, K.N.; Shamim, A. Polarization Insensitive and Transparent Frequency Selective Surface for Dual Band GSM Shielding. IEEE Trans. Antennas Propag. 2021, 69, 2779–2789. [Google Scholar] [CrossRef]
- Xu, G.; Hum, S.V.; Eleftheriades, G.V. A Technique for Designing Multilayer Multistopband Frequency Selective Surfaces. IEEE Trans. Antennas Propag. 2018, 66, 780–789. [Google Scholar] [CrossRef]
- Ghosh, S.; Srivastava, K.V. An Angularly Stable Dual-Band FSS with Closely Spaced Resonances Using Miniaturized Unit Cell. IEEE Microwave Wireless Compon. Lett. 2017, 27, 218–220. [Google Scholar] [CrossRef]
- Li, D.; Li, T.W.; Li, E.P.; Zhang, Y.J. A 2.5-D Angularly Stable Frequency Selective Surface Using Via-Based Structure for 5G EMI Shielding. IEEE Trans. Electromagn. Compat. 2018, 60, 768–775. [Google Scholar] [CrossRef]
- Mao, M.; Zhang, C.R.; Yang, Y.; He, X.X. Active Frequency Selective Surface with Wide Reconfigurable Passband. In Proceedings of the 2020 Cross Strait Radio Science & Wireless Technology Conference (CSRSWTC), Fuzhou, China, 13–16 December 2020; pp. 1–3. [Google Scholar]
- Mamedes, D.F.; Neto, A.G.; Silva, J.C.; Bornemann, J. Design of reconfigurable frequency-selective surfaces including the PIN diode threshold region. IET Microw Antennas Propag. 2018, 60, 768–775. [Google Scholar] [CrossRef] [Green Version]
- Bouslama, M.; Traii, M.; Denidni, T.A.; Gharsallah, A. Beam-Switching Antenna with a New Reconfigurable Frequency Selective Surface. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 1159–1162. [Google Scholar] [CrossRef]
- Gu, C.; Izquierdo, B.S.; Gao, S.; Batchelor, J.C.; Parker, E.A.; Qin, F.; Wei, G.; Li, J.; Xu, J. Dual-Band Electronically Beam-Switched Antenna Using Slot Active Frequency Selective Surface. IEEE Trans. Antennas Propag. 2017, 65, 1393–1398. [Google Scholar] [CrossRef]
- So, H.; Ando, A.; Seki, T.; Kawashima, M.; Sugiyama, T. Multiband sector antenna with the same beamwidth employing multiple woodpile metamaterial reflectors. IEICE Trans. Electron. 2014, 97, 976–985. [Google Scholar] [CrossRef] [Green Version]
- Decena, B.A.; Luzon, J.R.; Purisima, M.C.L. 24 GHz pattern reconfigurable corner reflector antennas using frequency selective conductor loops and strips. In Proceedings of the TENCON 2017-2017 IEEE Region 10 Conference, Penang, Malaysia, 5–8 November 2017; pp. 2914–2919. [Google Scholar]
- Tanizawa, Y.; Cho, K.; So, H.; Ando, A. Radiation characteristics of corner reflector antenna employing frequency selective surface. In Proceedings of the 2016 URSI Asia-Pacific Radio Science Conference (URSI AP-RASC), Seoul, Korea, 21–25 August 2016; pp. 1–3. [Google Scholar]
- Chatterjee, A.; Parui, S.K. Beamwidth control of omnidirectional antenna using conformal frequency selective surface of different curvatures. IEEE Trans. Antennas Propag. 2018, 66, 3225–3230. [Google Scholar] [CrossRef]
- Chatterjee, A.; Parui, S.K. Performance Enhancement of a Dual-Band Monopole Antenna by Using a Frequency-Selective Surface-Based Corner Reflector. IEEE Trans. Antennas Propag. 2016, 64, 2165–2171. [Google Scholar] [CrossRef]
- Elzwawi, G.H.; Kesavan, A.; Alwahishi, R.; Denidni, T.A. A New Corner-Reflector Antenna with Tunable Gain Based on Active Frequency Selective Surfaces. IEEE Open J. Antennas Propag. 2020, 1, 88–94. [Google Scholar] [CrossRef]
- Mamedes, D.F.; Neto, A.G.; Bornemann, J. Reconfigurable Corner Reflector Using PIN-Diode-Switched Frequency Selective Surfaces. In Proceedings of the 2020 IEEE International Symposium on Antennas and Propagation and North American Radio Science Meeting, Toronto, ON, Canada, 5–10 July 2020; pp. 127–128. [Google Scholar]
- Lazaro, A.; Lorenzo, J.; Villarino, R.; Girbau, D. Modulated corner reflector using frequency selective surfaces for FMCW radar applications. In Proceedings of the 2015 European Microwave Conference (EuMC), Paris, France, 7–10 September 2015; pp. 111–114. [Google Scholar]
- Lazaro, A.; Lorenzo, J.; Villarino, R.; Girbau, D. Backscatter transponder based on frequency selective surface for FMCW radar applications. Radioengineering 2014, 23, 632–641. [Google Scholar]
- Jiménez-Sáez, A.; Schüßler, M.; El-Absi, M.; Abbas, A.A.; Solbach, K.; Kaiser, T.; Jakoby, R. Frequency Selective Surface Coded Retroreflectors for Chipless Indoor Localization Tag Landmarks. IEEE Antennas Wirel. Propag. Lett. 2020, 19, 726–730. [Google Scholar] [CrossRef]
- Sarabandi, K.; Behdad, N. A Frequency Selective Surface with Miniaturized Elements. IEEE Trans. Antennas Propag. 2007, 55, 1239–1245. [Google Scholar] [CrossRef]
- Behdad, N.; Sarabandi, K. A Miniaturized Band-Pass Frequency Selective Surface. In Proceedings of the 2006 IEEE Antennas and Propagation Society International Symposium, Albuquerque, NM, USA, 9–14 July 2006; pp. 4171–4174. [Google Scholar]
- Al-Joumayly, M.; Behdad, N. A New Technique for Design of Low-Profile, Second-Order, Bandpass Frequency Selective Surfaces. IEEE Trans. Antennas Propag. 2009, 57, 452–459. [Google Scholar] [CrossRef]
- Huang, F.C.; Chiu, C.N.; Wu, T.L.; Chiou, Y.P. A Circular-Ring Miniaturized-Element Metasurface with Many Good Features for Frequency Selective Shielding Applications. IEEE Trans. Electromagn. Compat 2015, 57, 365–374. [Google Scholar] [CrossRef]
- Liu, N.; Sheng, X.; Fan, J.; Guo, D. A miniaturized FSS based on tortuous structure design. IEICE Electron. Express 2017, 14, 1–7. [Google Scholar] [CrossRef] [Green Version]
- Sheng, X.-J.; Fan, J.-J.; Liu, N.; Zhang, C.-B. A miniaturized dual-band FSS with controllable frequency resonances. IEEE Microwave Wireless Compon. Lett. 2017, 27, 915–917. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gan, L.; Sun, G.; Feng, D.; Li, J. Characteristics of an Eight-Quadrant Corner Reflector Involving a Reconfigurable Active Metasurface. Sensors 2022, 22, 4715. https://doi.org/10.3390/s22134715
Gan L, Sun G, Feng D, Li J. Characteristics of an Eight-Quadrant Corner Reflector Involving a Reconfigurable Active Metasurface. Sensors. 2022; 22(13):4715. https://doi.org/10.3390/s22134715
Chicago/Turabian StyleGan, Lin, Guang Sun, Dejun Feng, and Jianbing Li. 2022. "Characteristics of an Eight-Quadrant Corner Reflector Involving a Reconfigurable Active Metasurface" Sensors 22, no. 13: 4715. https://doi.org/10.3390/s22134715
APA StyleGan, L., Sun, G., Feng, D., & Li, J. (2022). Characteristics of an Eight-Quadrant Corner Reflector Involving a Reconfigurable Active Metasurface. Sensors, 22(13), 4715. https://doi.org/10.3390/s22134715





