3D-Printable Piezoelectric Composite Sensors for Acoustically Adapted Guided Ultrasonic Wave Detection †
Abstract
:1. Introduction
2. Materials and Methods
2.1. Theoretical Background on GUW Detection and Wave Interaction at Piezocomposite Sensors
2.2. Sensor Manufacturing
2.3. Sensor Geometry Selection
2.4. Experimental Setup for the Determination of Detectable GUW Signals
2.5. Experimental Setup for the Determination of GUW Reflections at Applied Sensors
3. Results
3.1. GUW Detection Using Tape Casted Piezocomposite Sensors
3.2. GUW Reflections at Applied Piezocomposite Sensors
4. Conclusions
- Optimize the material properties to increase the piezoelectric sensitivity and further minimize reflections when interacting with GUW.
- Replace PZT by lead-free particles such as barium titanate or potassium sodium niobate.
- Consider geometry rather than only the estimated effective sensor length as a criterion for sensor performance. This can be done, providing an analytical model to link the sensor response to an excited GUW wave field, e.g., a 3D linear elasticity model as presented in [33].
- Realizing a defined characterization environment to extract the sensor behavior in a sort of frequency response function that is independent from the sensor’s excitation.
- Design a concept for variable, direction-sensitive, and mode-selective sensors.
- Address mode conversion in reflection analysis for further insight in wave propagation interference by applied sensors.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Giurgiutiu, V. Structural Health Monitoring with Piezoelectric Wafer Active Sensors; Academic Press/Elsevier: Amsterdam, The Netherlands, 2008. [Google Scholar]
- Su, Z.; Ye, L. (Eds.) Identification of Damage Using Lamb Waves: From Fundamentals to Applications; Lecture Notes in Applied and Computational Mechanics; Springer: Berlin, Germany, 2009; Volume 48. [Google Scholar]
- Balageas, D.; Fritzen, C.P.; Güemes, A. Structural Health Monitoring; ISTE Ltd and ISTE: London, UK; Newport Beach, CA, USA, 2006. [Google Scholar] [CrossRef]
- Lammering, R.; Gabbert, U.; Sinapius, M.; Schuster, T.; Wierach, P. (Eds.) Lamb-Wave Based Structural Health Monitoring in Polymer Composites; Springer eBook Collection; Springer: Cham, Switzerland, 2018. [Google Scholar] [CrossRef]
- Yu, L.; Giurgiutiu, V. In situ 2-D piezoelectric wafer active sensors arrays for guided wave damage detection. Ultrasonics 2008, 48, 117–134. [Google Scholar] [CrossRef] [PubMed]
- Yoo, B.; Purekar, A.S.; Zhang, Y.; Pines, D.J. Piezoelectric-paint-based two-dimensional phased sensor arrays for structural health monitoring of thin panels. Smart Mater. Struct. 2010, 19, 1–17. [Google Scholar] [CrossRef]
- Schmidt, D. Modenselektive Übertragung von Lambwellen in Faserverbundstrukturen. Ph.D. Thesis, Technische Universität Braunschweig, Braunschweig, Germany, 2014. [Google Scholar]
- Wang, W.; Zhang, H.; Lynch, J.P.; Cesnik, C.E.; Li, H. Experimental and numerical validation of guided wave phased arrays integrated within standard data acquisition systems for structural health monitoring. Struct. Control Health Monit. 2018, 25, e2171. [Google Scholar] [CrossRef]
- Rose, J.L. Ultrasonic Guided Waves in Solid Media; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar] [CrossRef]
- Lamb, H. On waves in an elastic plate. Proc. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1917, 93, 114–128. [Google Scholar] [CrossRef]
- PI Ceramic GmbH. Datasheet—Material Data of Piezoelectric Materials: Specific Parameters of the Standard Materials. Available online: https://www.piceramic.de/fileadmin/user_upload/physik_instrumente/files/datasheets/PI_Ceramic_Material_Data.pdf (accessed on 6 September 2022).
- Giurgiutiu, V.; Lin, B. In-Situ Fabrication of Composite Piezoelectric Wafer Active Sensors for Structural Health Monitoring. In Proceedings of the ASME 2004 International Mechanical Engineering Congress and Exposition, Anaheim, CA, USA, 13–19 November 2004; pp. 89–95. [Google Scholar] [CrossRef]
- Banquart, A.; Callé, S.; Levassort, F.; Fritsch, L.; Ossant, F.; Toffessi Siewe, S.; Chevalliot, S.; Capri, A.; Grégoire, J.M. Piezoelectric P(VDF-TrFE) film inkjet printed on silicon for high-frequency ultrasound applications. J. Appl. Phys. 2021, 129, 195107. [Google Scholar] [CrossRef]
- Zhou, P.; Yang, X.; Su, Y.; Yang, J.; Xu, L.; Wang, K.; Zhou, L.-M.; Su, Z. Direct-write nanocomposite sensor array for ultrasonic imaging of composites. Compos. Commun. 2021, 28, 100937. [Google Scholar] [CrossRef]
- Chen, Z.; Song, X.; Lei, L.; Chen, X.; Fei, C.; Chiu, C.T.; Qian, X.; Ma, T.; Yang, Y.; Shung, K.; et al. 3D printing of piezoelectric element for energy focusing and ultrasonic sensing. Nano Energy 2016, 27, 78–86. [Google Scholar] [CrossRef]
- Cheng, J.; Chen, Y.; Wu, J.W.; Ji, X.R.; Wu, S.H. 3D Printing of BaTiO3 Piezoelectric Ceramics for a Focused Ultrasonic Array. Sensors 2019, 19, 4078. [Google Scholar] [CrossRef] [PubMed]
- Narita, F.; Fox, M. A Review on Piezoelectric, Magnetostrictive, and Magnetoelectric Materials and Device Technologies for Energy Harvesting Applications. Adv. Eng. Mater. 2018, 20, 1700743. [Google Scholar] [CrossRef]
- Sakamoto, W.K.; Higuti, R.T.; Crivelini, E.B.; Nagashima, H.N. Polymer matrix-based piezoelectric composite for structural health monitoring. In Proceedings of the 2013 Joint IEEE International Symposium on Applications of Ferroelectric and Workshop on Piezoresponse Force Microscopy (ISAF/PFM), Prague, Czech Republic, 21–25 July 2013; pp. 295–297. [Google Scholar] [CrossRef]
- Fang, X.; He, J.; Zhang, Y. Preparation and Characterization of Large-Area and Flexible Lead Zirconate Titanate/Polyvinyl-Butyral/Additives Composite Films for Piezoelectric Sensor Application. Sens. Mater. 2016, 28, 681–688. [Google Scholar] [CrossRef]
- Pan, H.H.; Huang, M.W. Piezoelectric cement sensor-based electromechanical impedance technique for the strength monitoring of cement mortar. Constr. Build. Mater. 2020, 254, 119307. [Google Scholar] [CrossRef]
- Sirohi, J.; Chopra, I. Fundamental Understanding of Piezoelectric Strain Sensors. J. Intell. Mater. Syst. Struct. 2000, 11, 246–257. [Google Scholar] [CrossRef]
- Bergman, D.R. Computational Acoustics—Theory and Implementation, 1st ed.; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Kaltenbacher, M. Computational Acoustics, 1st ed.; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Sinapius, M. Adaptronik; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar] [CrossRef]
- Mitkus, R.; Pierou, A.; Feder, J.; Sinapius, M. Investigation and Attempt to 3D Print Piezoelectric 0-3 Composites Made of Photopolymer Resins and PZT. In Proceedings of the ASME 2020 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Virtual, 15 September 2020. [Google Scholar] [CrossRef]
- Mitkus, R.; Taleb Alashkar, A.; Sinapius, M. An Attempt to Topology Optimize 3D Printed Piezoelectric Composite Sensors for Highest D31 Output. In Proceedings of the ASME 2021 Conference on Smart Materials, Adaptive Structures and Intelligent Systems, Virtual, 14–15 September 2021. [Google Scholar] [CrossRef]
- Roloff, T.; Mitkus, R.; Lion, J.N.; Sinapius, M. 3D Printable Piezoelectric Composite Sensors for Guided Ultrasonic Wave Detection. Eng. Proc. 2021, 10, 36. [Google Scholar] [CrossRef]
- Marwitz, S.; Zabel, V. Operational Modal Analysis with a 3D Laser Vibrometer Without External Reference. In Rotating Machinery, Hybrid Test Methods, Vibro-Acoustics & Laser Vibrometry; de Clerck, J., Epp, D.S., Eds.; Springer International Publishing: Cham, Switzerland, 2016; Volume 8, pp. 75–85. [Google Scholar] [CrossRef]
- Apetre, N.; Ruzzene, M.; Hanagud, S.; Sharma, V.; Gopalakrishnan, S. Damage Measure Formulation Based on the Filtered Spectral Approximation of the Structural Response. In Proceedings of the 48th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, Honolulu, HI, USA, 23–26 April 2007; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2007; pp. 1–14. [Google Scholar] [CrossRef]
- Tian, Z.; Yu, L.; Leckey, C. Delamination detection and quantification on laminated composite structures with Lamb waves and wavenumber analysis. J. Intell. Mater. Syst. Struct. 2015, 26, 1723–1738. [Google Scholar] [CrossRef]
- Moll, J.; Golub, M.V.; Glushkov, E.; Glushkova, N.; Fritzen, C.P. Non-axisymmetric Lamb wave excitation by piezoelectric wafer active sensors. Sens. Actuators A Phys. 2012, 174, 173–180. [Google Scholar] [CrossRef]
- Pohl, J.; Willberg, C.; Gabbert, U.; Mook, G. Experimental and Theoretical Analysis of Lamb Wave Generation by Piezoceramic Actuators for Structural Health Monitoring. Exp. Mech. 2012, 52, 429–438. [Google Scholar] [CrossRef]
- Raghavan, A.; Cesnik, C.E.S. Finite-dimensional piezoelectric transducer modeling for guided wave based structural health monitoring. Smart Mater. Struct. 2005, 14, 1448–1461. [Google Scholar] [CrossRef]
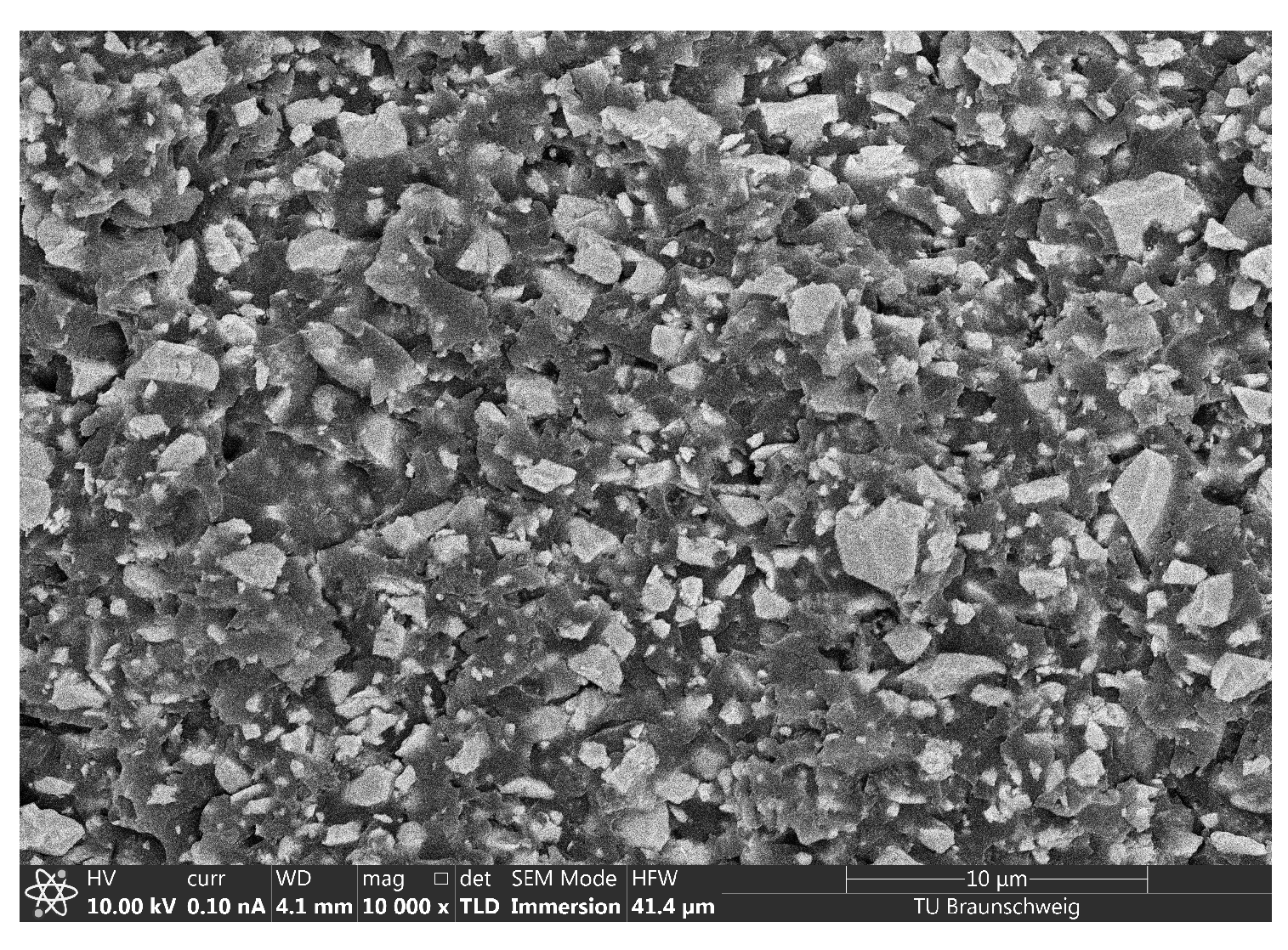


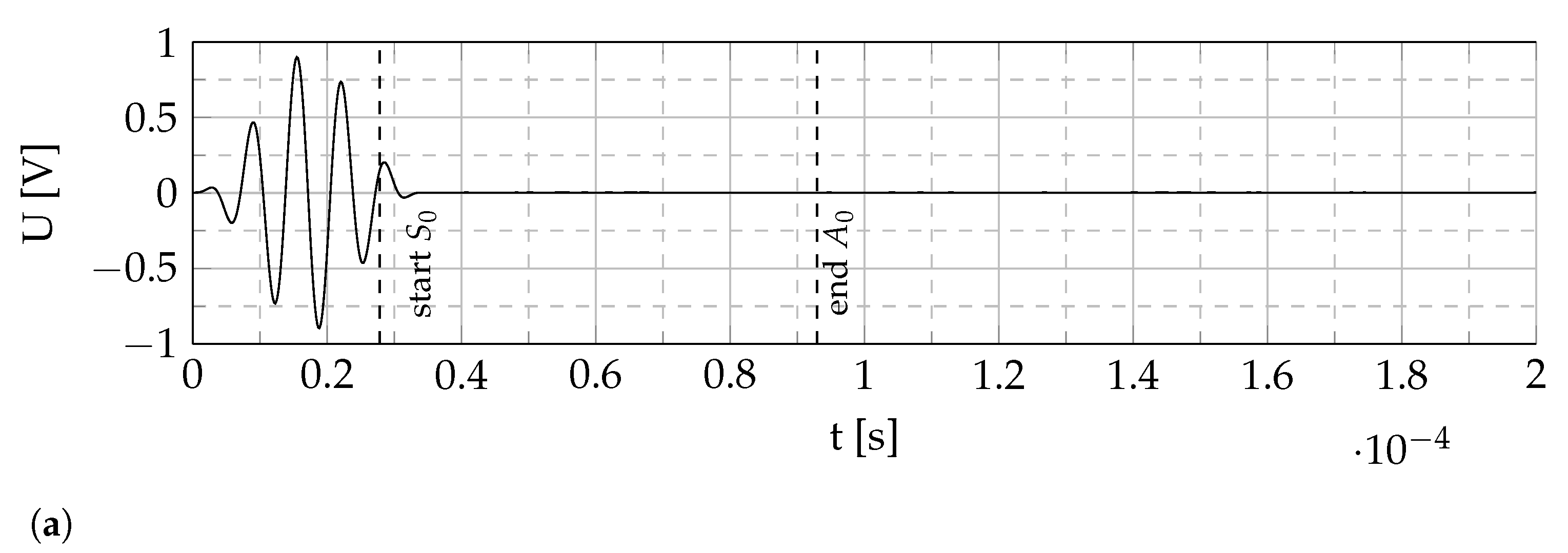

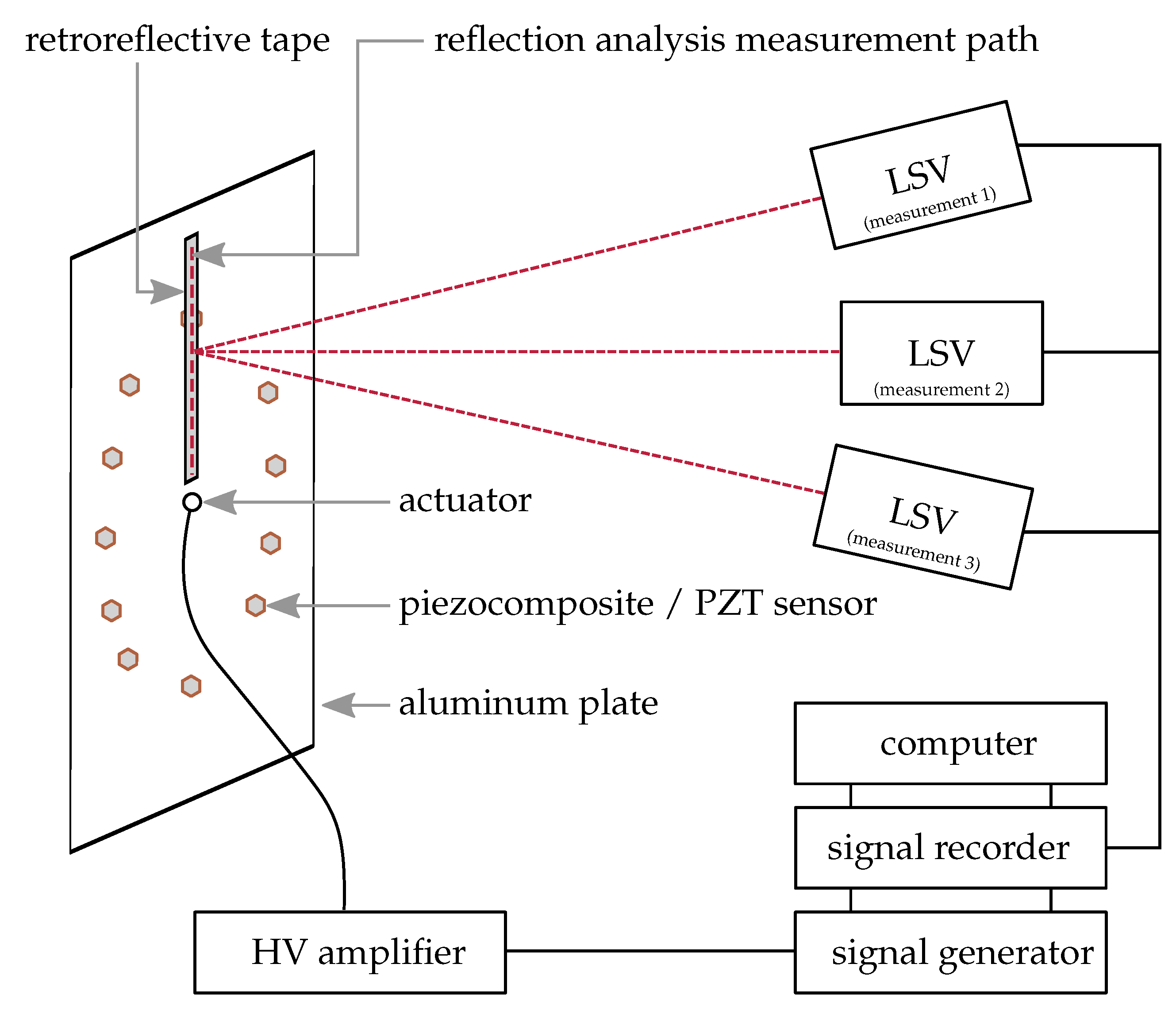


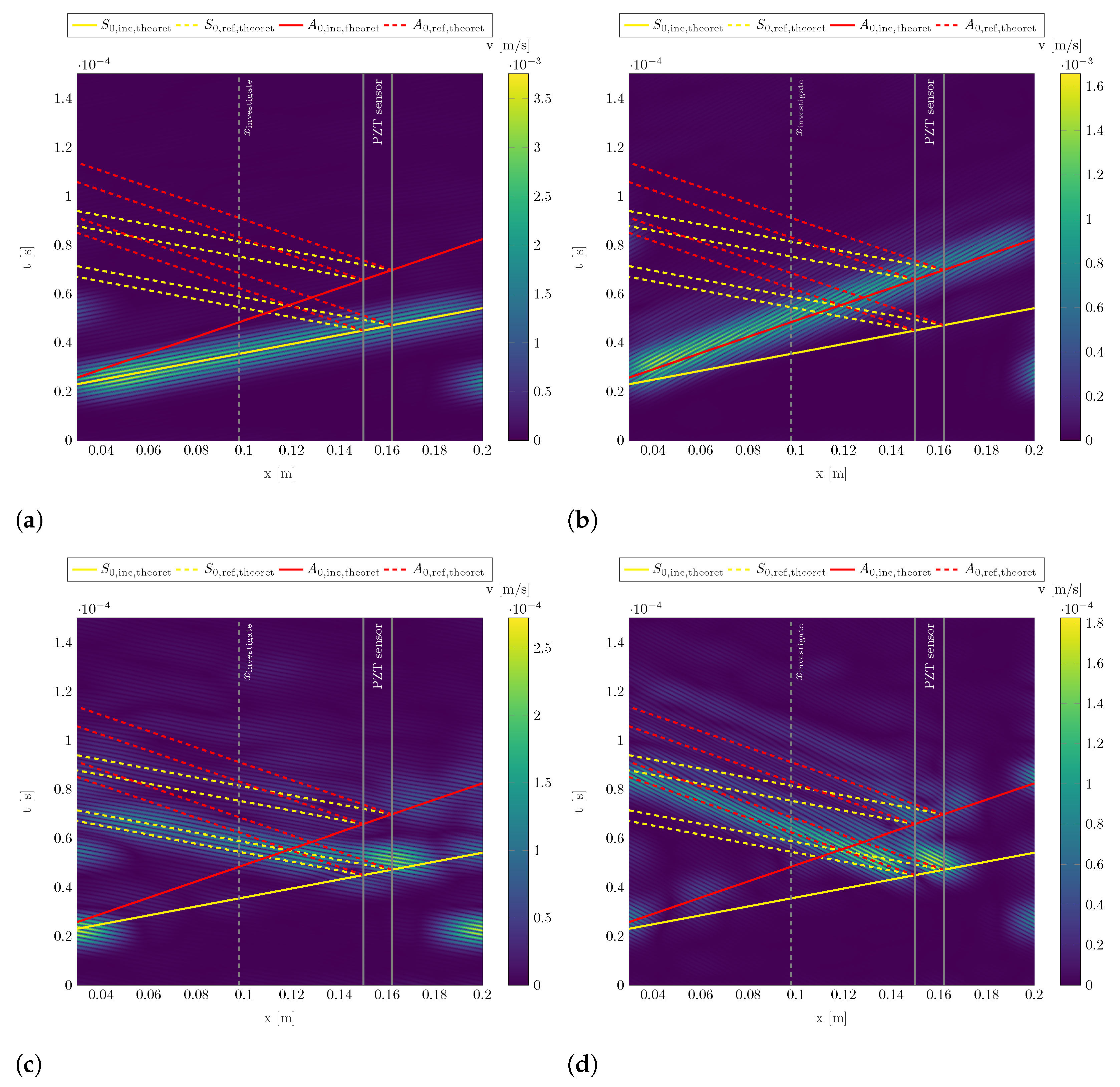

| Material | Young’s Modulus (perp. to pol. dir.) Y [GPa] | Dielectric Permittivity at 1 kHz [nF m] | Piezoelectric Charge Constant [pC N] | Electromechanical Coupling Factor [-] |
|---|---|---|---|---|
| Custom piezocomposite | 1.8 ± 0.2 | 0.08 ± 0.002 | −0.92 ± 0.13 | 0.0044 ± 0.00091 |
| Commercial ceramic (PIC255) [11] | 62.5 | 15.9 | −180 | 0.35 |
| Measured Variable | Parameter | Dimension | -Mode (num.) | -Mode (exp.) | -Mode (num.) | -Mode (exp.) |
|---|---|---|---|---|---|---|
| Group velocity | mean | [m s−1] | 5368.71 | 5498.4 | 3022.04 | 2987.0 |
| standard dev. | [%] | - | 2.10 | - | 1.06 | |
| Wavelength | mean | [mm] | 0.0181 | 0.0182 | 0.0067 | 0.0067 |
| standard dev. | [%] | - | 1.42 | - | 0.74 |
| Type of Reflection | Data Base | Dimension | PZT | Square (Orien. 1) | Square (Orien. 2) | Circle |
|---|---|---|---|---|---|---|
| – | in-plane data | [%] | 4.60 | 2.09 | 1.58 | 0.95–2.50 (*) |
| – | out-of-plane data | [%] | 1.76 | 1.17 | 1.22 | 1.75 |
| - | [mm] | 98 | 96 | 92 | 95 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roloff, T.; Mitkus, R.; Lion, J.N.; Sinapius, M. 3D-Printable Piezoelectric Composite Sensors for Acoustically Adapted Guided Ultrasonic Wave Detection. Sensors 2022, 22, 6964. https://doi.org/10.3390/s22186964
Roloff T, Mitkus R, Lion JN, Sinapius M. 3D-Printable Piezoelectric Composite Sensors for Acoustically Adapted Guided Ultrasonic Wave Detection. Sensors. 2022; 22(18):6964. https://doi.org/10.3390/s22186964
Chicago/Turabian StyleRoloff, Thomas, Rytis Mitkus, Jann Niklas Lion, and Michael Sinapius. 2022. "3D-Printable Piezoelectric Composite Sensors for Acoustically Adapted Guided Ultrasonic Wave Detection" Sensors 22, no. 18: 6964. https://doi.org/10.3390/s22186964
APA StyleRoloff, T., Mitkus, R., Lion, J. N., & Sinapius, M. (2022). 3D-Printable Piezoelectric Composite Sensors for Acoustically Adapted Guided Ultrasonic Wave Detection. Sensors, 22(18), 6964. https://doi.org/10.3390/s22186964









