Meter Wave Polarization-Sensitive Array Radar for Height Measurement Based on MUSIC Algorithm
Abstract
:1. Introduction
2. Multipath Signal Model of Meter Wave PSA
3. PS-GMUSIC Algorithm
4. Proposed MPS-GMUSIC Algorithm
5. Proposed P-SSV-MUSIC Algorithm
6. Computational Complexity
7. Simulation
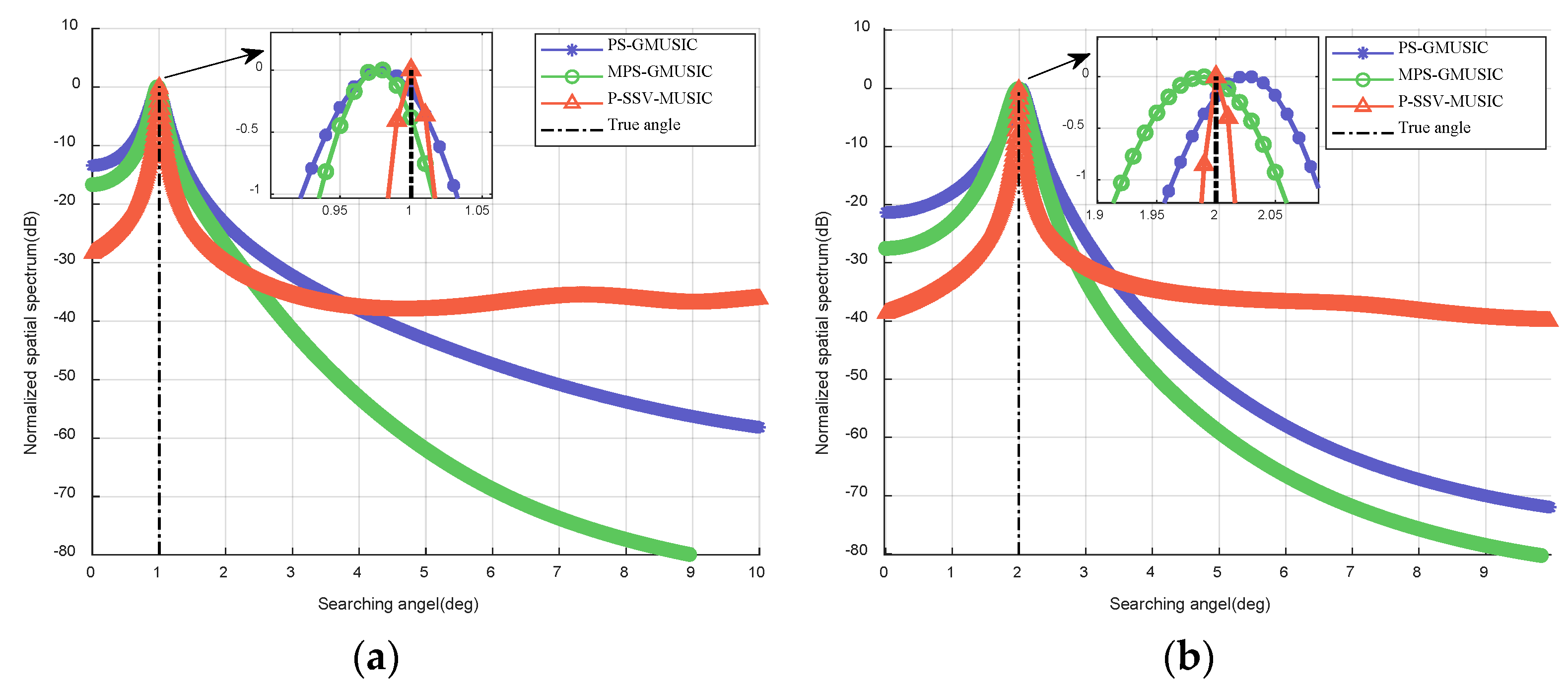
7.1. Spectrum Estimation of the Proposed Method
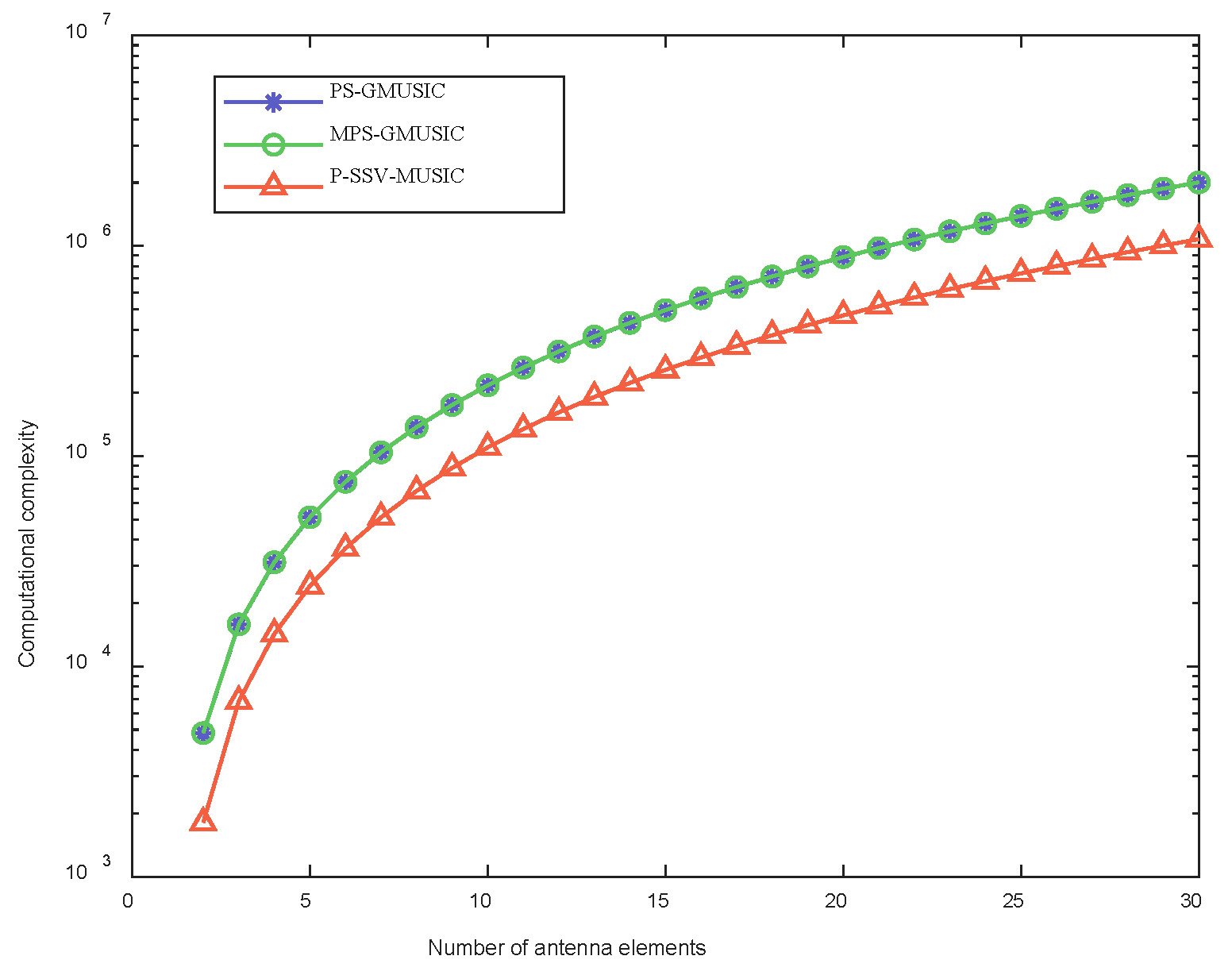
7.2. Computational Complexity of the Proposed Method
7.3. RMSE of the Proposed Method
7.3.1. RMSE with Respect to the SNR
7.3.2. RMSE with Respect to the Snapshot Number
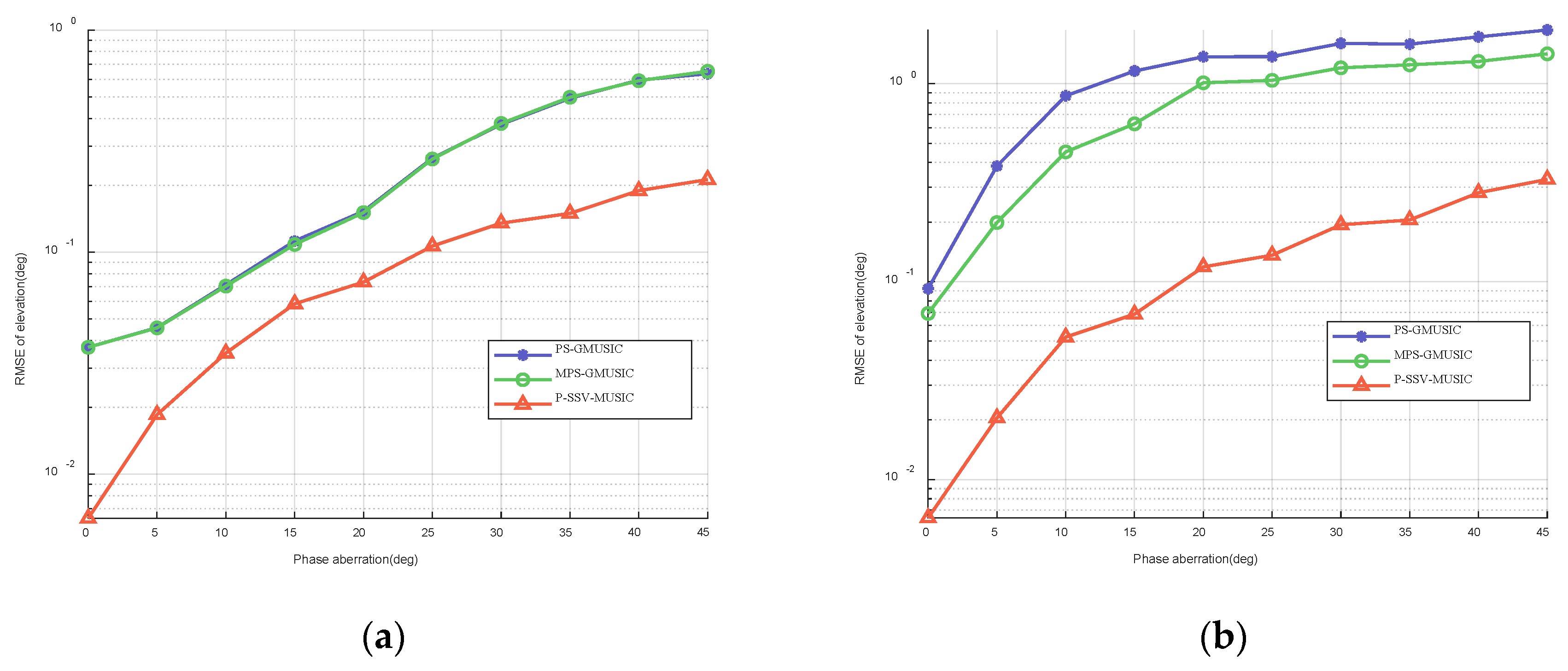
7.3.3. RMSE with Respect to the Phase Aberration
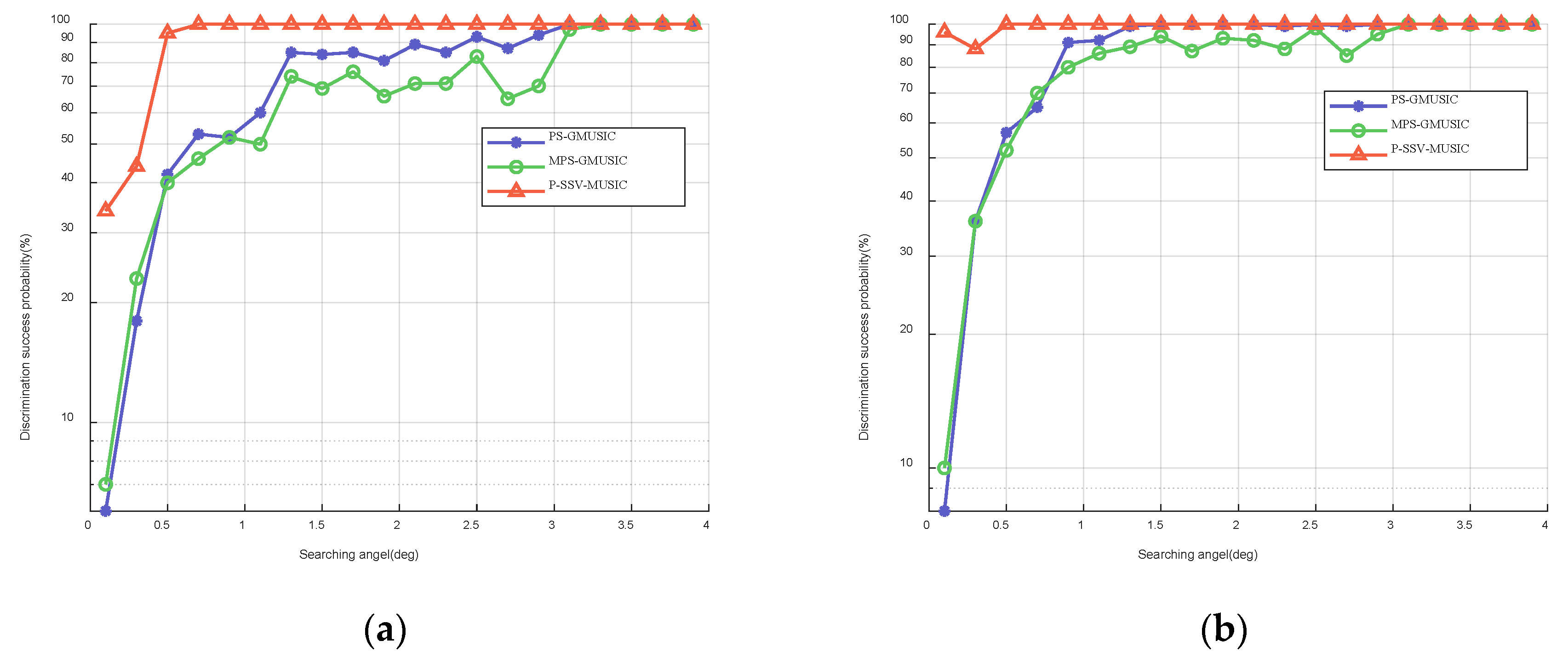
7.4. Discrimination Success Probability of the Proposed Method
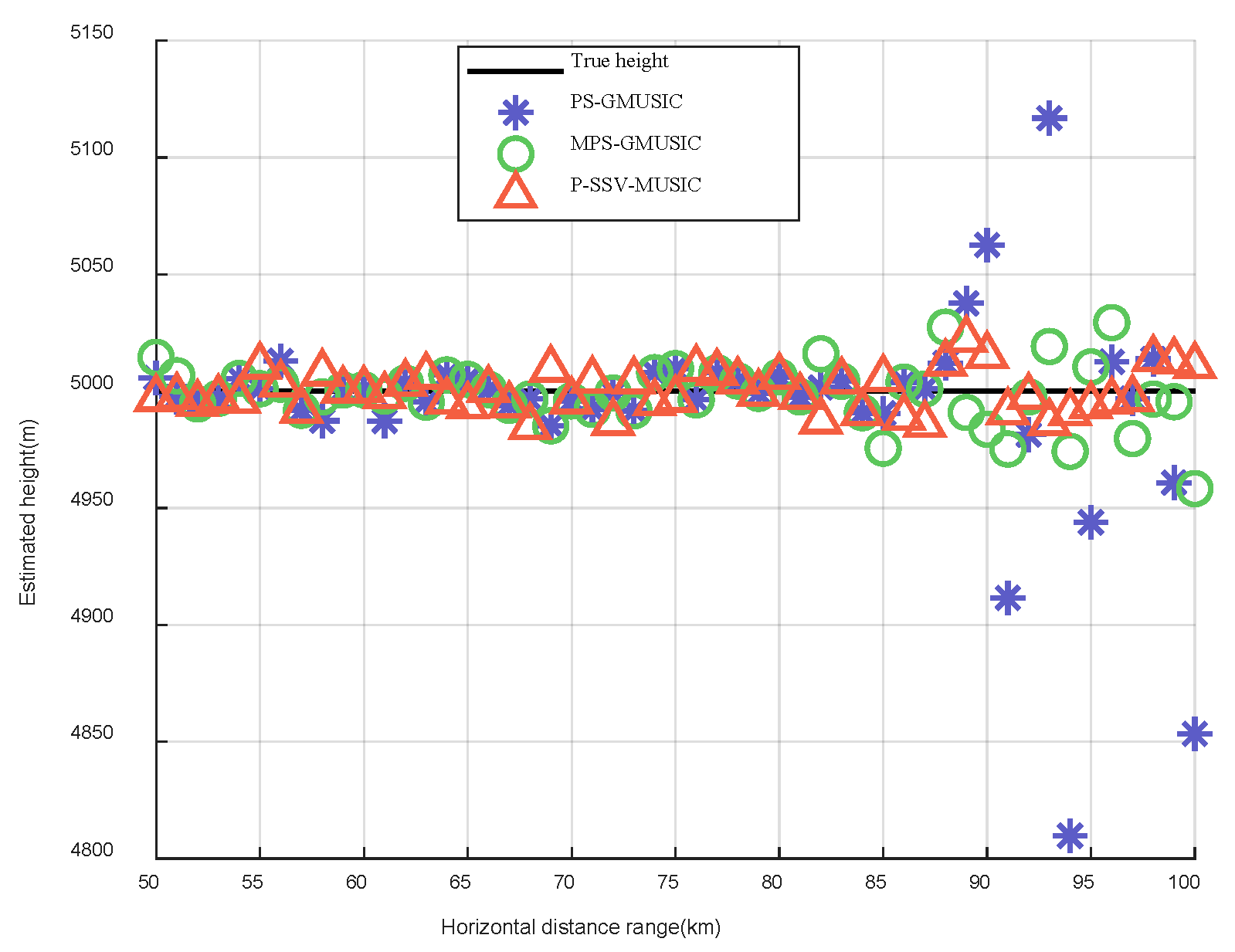
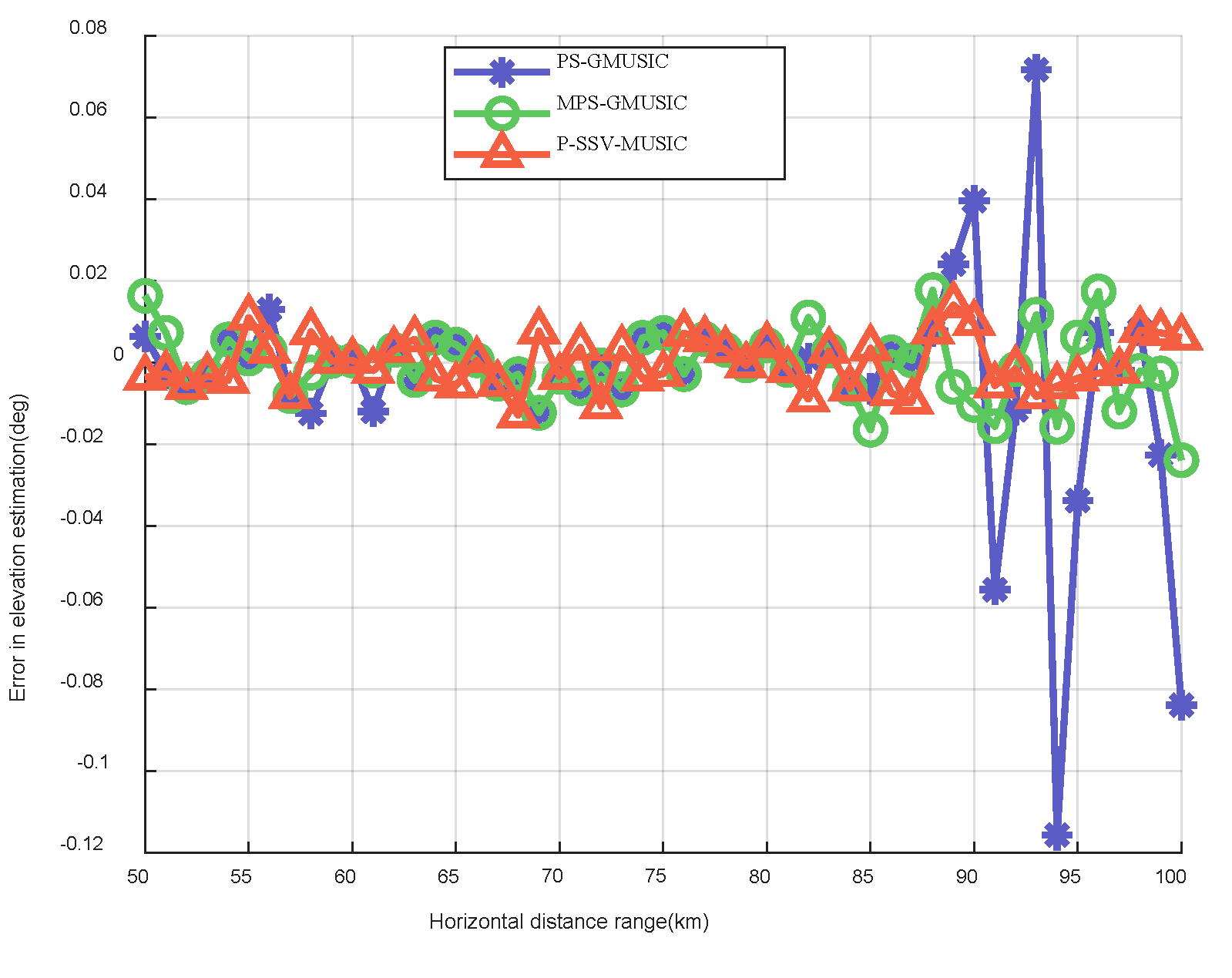
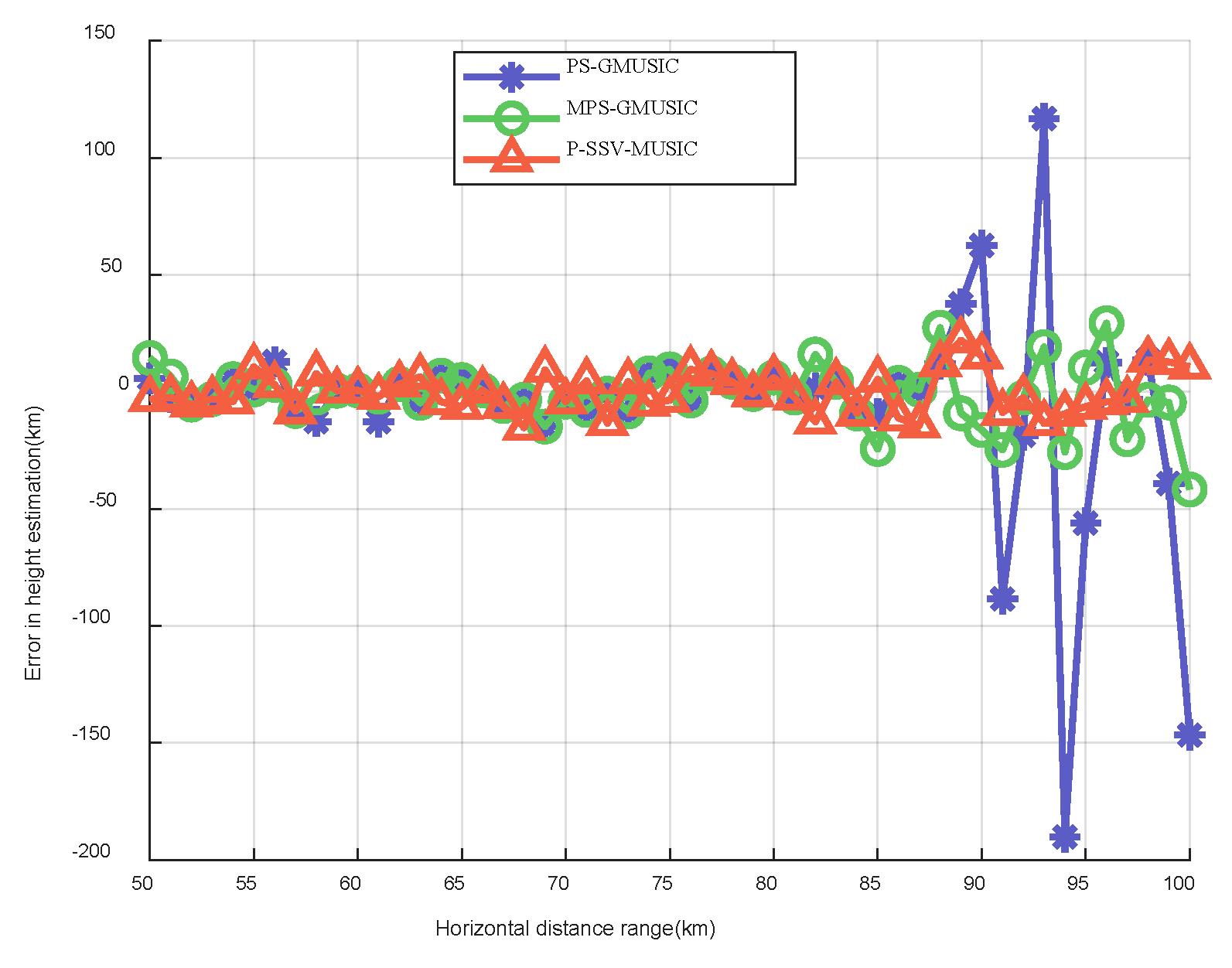
7.5. Tracking Measurement Results of Simulated Track
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Heiner, K. VHF/UHF radar Part 1: Characteristics. Electron. Commun. Eng. J. 2002, 14, 61–72. [Google Scholar]
- Heiner, K. VHF/UHF radar Part 2: Operational aspects and applications. Electron. Commun. Eng. J. 2002, 14, 101–111. [Google Scholar]
- Xu, Z.H.; Wu, J.; Xiong, Z. Low-angle tracking algorithm using polarisation sensitive array for very-high frequency radar. IET Radar Sonar Navig. 2014, 8, 1035–1041. [Google Scholar] [CrossRef]
- Rahamim, D.; Tabrikian, J.; Shavit, R. Source localization using vector sensor array in a multipath environment. IEEE Trans. Signal Process. 2004, 52, 3096–3103. [Google Scholar] [CrossRef]
- Shan, T.J.; Wax, M.; Kailath, T. On spatial smoothing for direction-of-arrival estimation of coherent signals. IEEE Trans. Acoust. Speech Signal Process. 1985, 33, 806–811. [Google Scholar] [CrossRef]
- Zheng, Y.S. Study on Some Issues of Low-Angle Altitude Measurement for VHF Array Radar. Ph.D. Thesis, Xidian University, Xi’an, China, 2017. [Google Scholar]
- Kwak, H.; Eunjung, Y.; Chun, J. Vector sensor arrays in DOA estimation for the low angle tracking. In Proceedings of the International Waveform Diversity and Design Conference, Pisa, Italy, 4–8 June 2007; pp. 183–187. [Google Scholar]
- Xu, Y.; Liu, Z. Polarimetric angular smoothing algorithm for an electromagnetic vector-sensor array. IET Radar Sonar Navig. 2007, 1, 230–240. [Google Scholar] [CrossRef]
- Zheng, G.M.; Chen, B.X.; Yang, M.L. Weighted polarization smoothing algorithm for electromagnetic vector-sensor array. Syst. Eng. Electron. 2012, 34, 637–643. [Google Scholar]
- Liu, Z.; He, J.; Liu, Z. Computationally efficient DOA and polarization estimation of coherent sources with linear electromagnetic vector-sensor array. EURASIP J. Adv. Signal Process. 2011, 2011, 490289. [Google Scholar] [CrossRef]
- Si, W.J.; Wang, Y.; Hou, C.B.; Wang, H. Real-valued 2D MUSIC algorithm based on modified forward/backward averaging using an arbitrary centrosymmetric polarization sensitive array. Sensors 2017, 17, 2241. [Google Scholar] [CrossRef]
- Steinwandt, J.; Roemer, F.; Haardt, M.; del Galdo, G. Performance analysis of multi-dimensional ESPRIT-type algorithms for arbitrary and strictly non-circular sources with spatial smoothing. IEEE Trans. Signal Process. 2017, 65, 2262–2276. [Google Scholar] [CrossRef]
- Qiu, S.; Sheng, W.X.; Ma, X.F.; Kirubarajan, T.A. Maximum Likelihood Method for Joint DOA and Polarization Estimation Based on Manifold Separation. IEEE Trans. Aerosp. Electron. Syst. 2021, 57, 2481–2500. [Google Scholar] [CrossRef]
- Cui, W.; Shen, Q.; Liu, W.; Wu, S.L. Low complexity DOA estimation for wideband off-grid sources based on re-focused compressive sensing with dynamic dictionary. IEEE J. Sel. Top. Signal Process. 2019, 13, 918–930. [Google Scholar] [CrossRef]
- Dai, M.H.; Ma, X.F.; Liu, W.; Sheng, W.X.; Han, Y.B.; Xu, H.W. Monopulse based DOA and polarization estimation with polarization sensitive arrays. Signal Process. 2022, 195, 108487. [Google Scholar] [CrossRef]
- Zhang, X.W.; Liao, G.S.; Yang, Z.W.; Chen, Y. Derivative ESPRIT for DOA and polarization estimation for UCA using tangential individually-polarized dipole. Digit. Signal Process. 2020, 96, 102599. [Google Scholar] [CrossRef]
- Chen, R.; Long, W.X.; Wang, X.D.; Li, J.D. Multi-Mode OAM Radio Waves: Generation, Angle of Arrival Estimation and Reception with UCAs. IEEE Trans. Wirel. Commun. 2020, 19, 6932–6947. [Google Scholar] [CrossRef]
- Long, W.X.; Chen, R.; Marco, M.; Li, J.D. AoA Estimation for OAM Communication Systems with Mode-Frequency Multi-Time ESPRIT Method. IEEE Trans. Veh. Technol. 2021, 70, 5094–5098. [Google Scholar] [CrossRef]
- Huan, S.; Zhang, M.; Dai, G. Low Elevation Angle Estimation with Range Super-Resolution in Wideband Radar. Sensors 2020, 20, 3104. [Google Scholar] [CrossRef]
- Zheng, Y.S.; Chen, B.X. Study on direction of arrival estimation method of low angle targets for double orthogonally oriented electromagnetic vector-sensor array. Radar Sci. Technol. 2017, 4, 131–136. [Google Scholar]
- Tan, J.; Nie, Z. Polarization smoothing generalized MUSIC algorithm with polarization sensitive array for low angle estimation. Sensors 2018, 18, 1534. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y. Research on Some Issues of Low-Angle Target Height Measurement for VHF Array Radar. Ph.D. Thesis, Xidian University, Xi’an, China, 2019. [Google Scholar]
- Wu, J.Q. Advanced Metric Wave Radar, 1st ed.; National Defense Industry Press: Beijing, China, 2020; pp. 45–47. [Google Scholar]
- Zhao, J.; Yang, J. Double-null pattern synthesis for low-angle tracking. In Proceedings of the International Conference on Microwave and Millimeter Wave Technology, Guilin, China, 18–21 April 2007; pp. 1–4. [Google Scholar]
- Zhang, X.D. Matrix Analysis and Applications, 1st ed.; Tsinghua University Press: Beijing, China, 2004; pp. 528–538. [Google Scholar]
- Liu, Y.; Liu, H.W.; X, X.G. Projection techniques for altitude estimation over complex multipath condition-based VHF radar. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2018, 11, 2362–2375. [Google Scholar] [CrossRef]





| Index | Dielectric | Relative Permittivity | Surface Material Conductivity |
|---|---|---|---|
| 1 | Good soil (wet soil) | 25 | 0.02 |
| 2 | General soil | 15 | 0.005 |
| 3 | Poor soil (dry soil) | 3 | 0.001 |
| 4 | Snow and ice | 3 | 0.001 |
| 5 | Freshwater | 81 | 0.7 |
| 6 | Seawater | 75 | 0.5 |
| Algorithm Name | Computational Complexity |
|---|---|
| PS-GMUSIC | |
| MPS-GMUSIC | |
| P-SSV-MUSIC |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, G.; Zheng, G.; Wang, H.; Chen, C. Meter Wave Polarization-Sensitive Array Radar for Height Measurement Based on MUSIC Algorithm. Sensors 2022, 22, 7298. https://doi.org/10.3390/s22197298
Wang G, Zheng G, Wang H, Chen C. Meter Wave Polarization-Sensitive Array Radar for Height Measurement Based on MUSIC Algorithm. Sensors. 2022; 22(19):7298. https://doi.org/10.3390/s22197298
Chicago/Turabian StyleWang, Guoxuan, Guimei Zheng, Hongzhen Wang, and Chen Chen. 2022. "Meter Wave Polarization-Sensitive Array Radar for Height Measurement Based on MUSIC Algorithm" Sensors 22, no. 19: 7298. https://doi.org/10.3390/s22197298
APA StyleWang, G., Zheng, G., Wang, H., & Chen, C. (2022). Meter Wave Polarization-Sensitive Array Radar for Height Measurement Based on MUSIC Algorithm. Sensors, 22(19), 7298. https://doi.org/10.3390/s22197298





