Photothermal Self-Excitation of a Phase-Controlled Microcantilever for Viscosity or Viscoelasticity Sensing
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Setup
2.1.1. Optomechanical Block
2.1.2. Demodulating and Control Electronics
2.1.3. Electrical Scheme of the PLL
2.2. General Modeling Equations of the PLL
2.3. General PLL Model Applied to Purely Viscous Newtonian Fluids
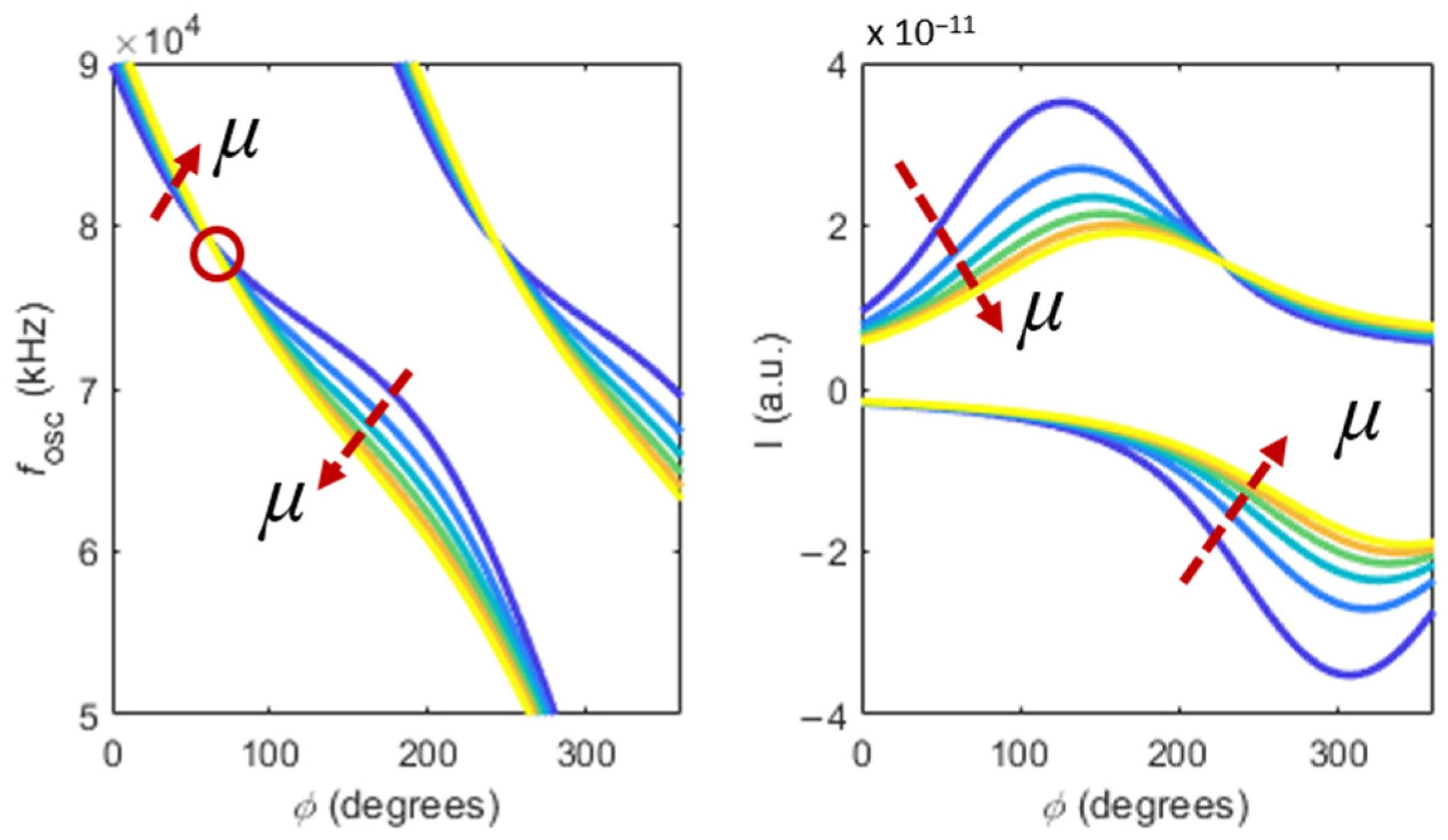
2.4. General PLL Model Applied to Viscoelastic Non-Newtonian Fluids
3. Results
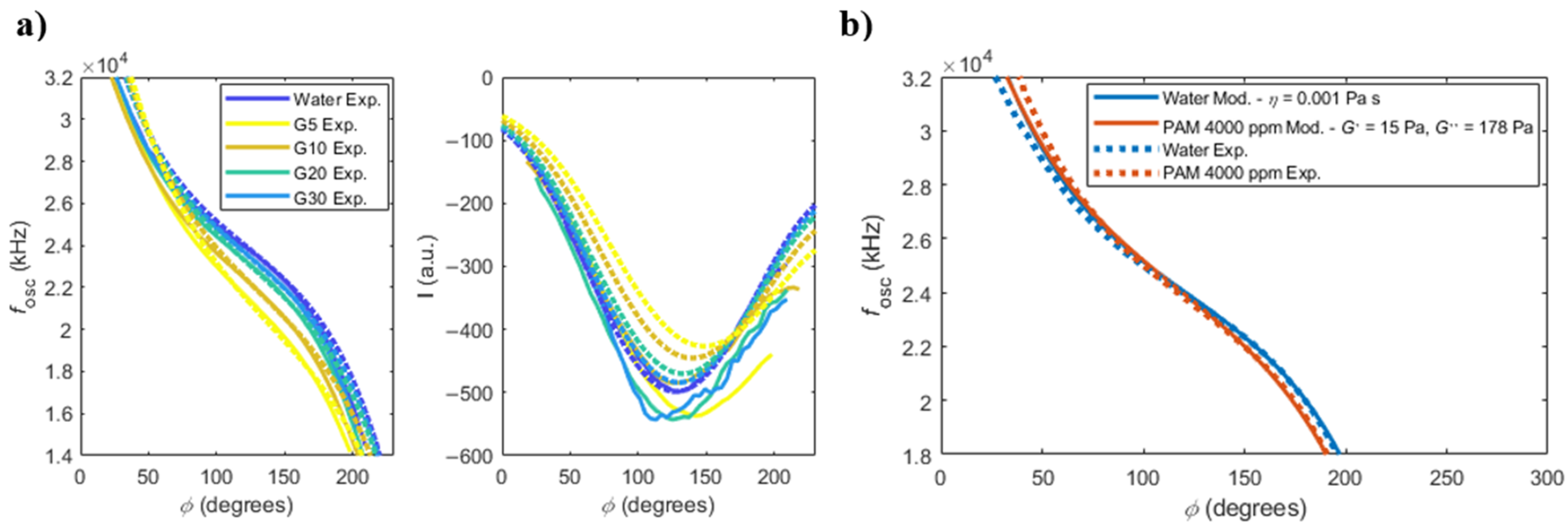
3.1. Newtonian Water/Glycerol Solutions
3.2. Non-Newtonian Fluid
4. Discussion
4.1. Sensitivity of the PLL Platform to Small Changes in Rheological Parameters
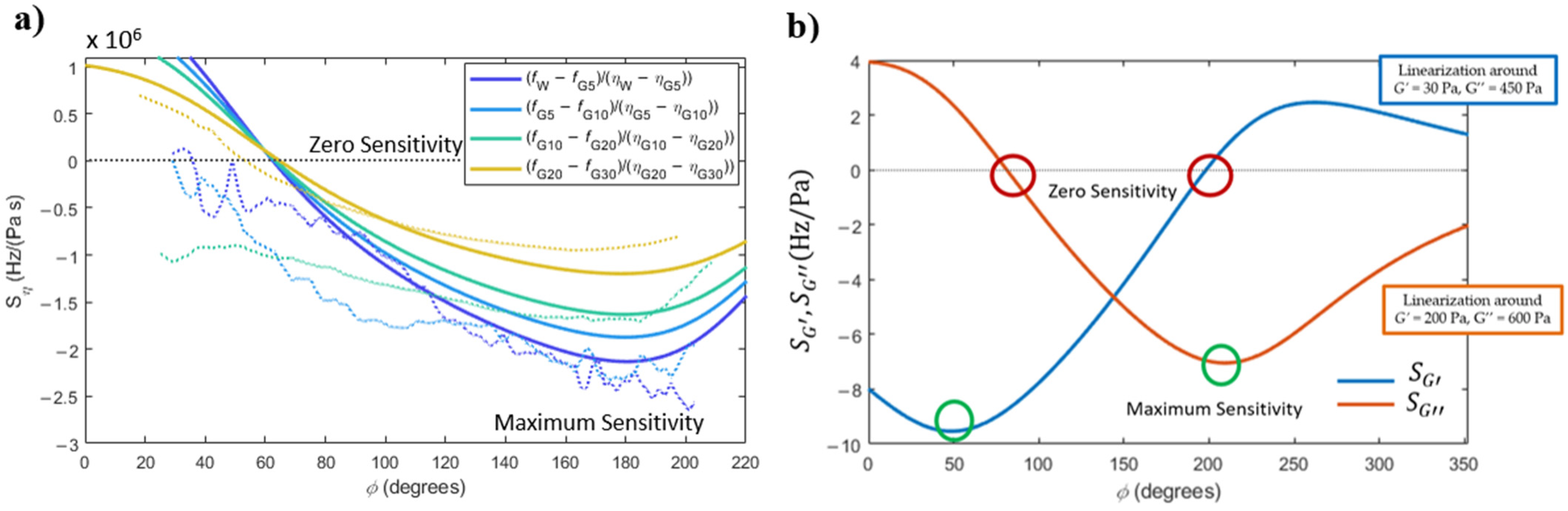
4.1.1. Sensitivity to Viscosity Variations in Newtonian Fluids
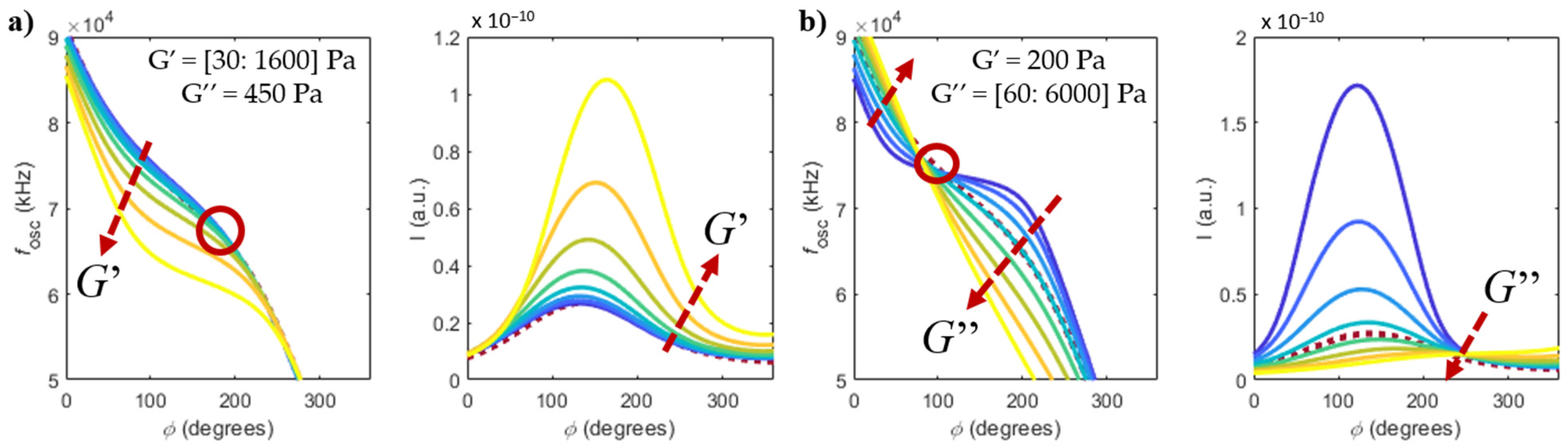
4.1.2. Sensitivity to Variations of Elastic and Viscous Modulus in Non-Newtonian Fluids
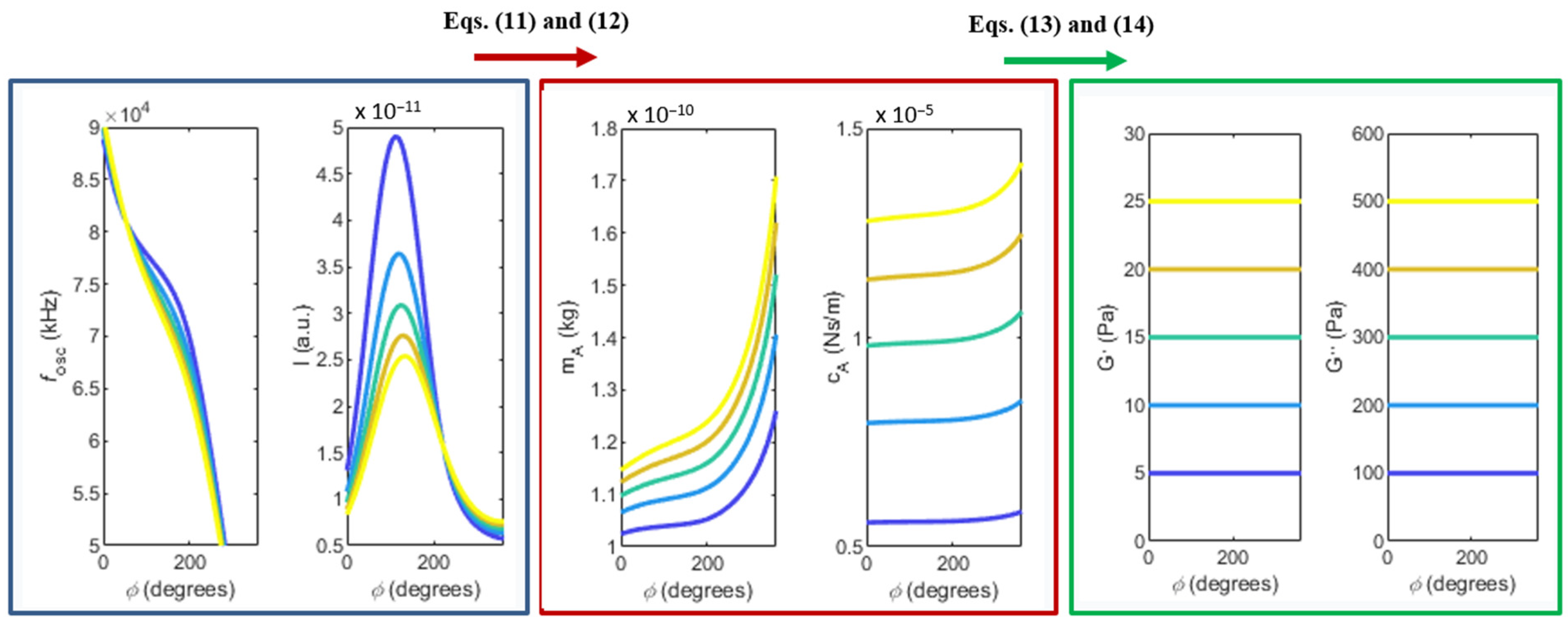
4.2. Inversion Problem to Extract G′ and G″ from the Measured Frequency and Amplitude of the Oscillations in the PLL
4.2.1. Analytical Method
4.2.2. Issues to Implement the Inversion Method
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cicuta, P.; Donald, A.M. Microrheology: A review of the method and applications. Soft Matter 2007, 3, 1449–1455. [Google Scholar] [CrossRef]
- Robertson-Anderson, R.M. Optical Tweezers Microrheology: From the Basics to Advanced Techniques and Applications. ACS Macro Lett. 2018, 7, 968–975. [Google Scholar] [CrossRef] [Green Version]
- Tassieri, M. Microrheology with optical tweezers: Peaks & troughs. Curr. Opin. Colloid Interface Sci. 2019, 43, 39–51. [Google Scholar] [CrossRef] [Green Version]
- Christopher, G.F.; Yoo, J.M.; Dagalakis, N.; Hudson, S.D.; Migler, K.B. Development of a MEMS based dynamic rheometer. Lab Chip 2010, 10, 2749–2757. [Google Scholar] [CrossRef]
- Dufour, I.; Maali, A.; Amarouchene, Y.; Ayela, C.; Caillard, B.; Darwiche, A.; Guirardel, M.; Kellay, H.; Lemaire, E.; Mathieu, F.; et al. The Microcantilever: A Versatile Tool for Measuring the Rheological Properties of Complex Fluids. J. Sens. 2012, 2012, 719898. [Google Scholar] [CrossRef] [Green Version]
- Sader, J.E. Frequency response of cantilever beams immersed in viscous fluids with applications to the atomic force microscope. J. Appl. Phys. 1998, 84, 64–76. [Google Scholar] [CrossRef] [Green Version]
- Green, C.P.; Sader, J.E. Torsional frequency response of cantilever beams immersed in viscous fluids with applications to the atomic force microscope. J. Appl. Phys. 2002, 92, 6262–6274. [Google Scholar] [CrossRef] [Green Version]
- Ahmed, N.; Nino, D.F.; Moy, V.T. Measurement of solution viscosity by atomic force microscopy. Rev. Sci. Instrum. 2001, 72, 2731–2734. [Google Scholar] [CrossRef]
- Boskovic, S.; Chon, J.; Mulvaney, P.; Sader, J. Rheological measurements using microcantilevers. J. Rheol. 2002, 46, 891. [Google Scholar] [CrossRef] [Green Version]
- Belmiloud, N.; Dufour, I.; Colin, A.; Nicu, L. Rheological behavior probed by vibrating microcantilevers. Appl. Phys. Lett. 2008, 92, 041907. [Google Scholar] [CrossRef]
- Vančura, C.; Dufour, I.; Heinrich, S.M.; Josse, F.; Hierlemann, A. Analysis of resonating microcantilevers operating in a viscous liquid environment. Sens. Actuators A Phys. 2008, 141, 43–51. [Google Scholar] [CrossRef]
- Ghatkesar, M.K.; Braun, T.; Barwich, V.; Ramseyer, J.-P.; Gerber, C.; Hegner, M.; Lang, H.P. Resonating modes of vibrating microcantilevers in liquid. Appl. Phys. Lett. 2008, 92, 043106. [Google Scholar] [CrossRef] [Green Version]
- Ghatkesar, M.K.; Rakhmatullina, E.; Lang, H.-P.; Gerber, C.; Hegner, M.; Braun, T. Multi-parameter microcantilever sensor for comprehensive characterization of Newtonian fluids. Sens. Actuators B Chem. 2008, 135, 133–138. [Google Scholar] [CrossRef]
- Castille, C.; Dufour, I.; Lucat, C. Longitudinal vibration mode of piezoelectric thick-film cantilever-based sensors in liquid media. Appl. Phys. Lett. 2010, 96, 154102. [Google Scholar] [CrossRef] [Green Version]
- Youssry, M.; Belmiloud, N.; Caillard, B.; Ayela, C.; Pellet, C.; Dufour, I. A straightforward determination of fluid viscosity and density using microcantilevers: From experimental data to analytical expressions. Sens. Actuators A Phys. 2011, 172, 40–46. [Google Scholar] [CrossRef]
- Belmiloud, N.; Dufour, I.; Nicu, L.; Colin, A.; Pistre, J. Vibrating Microcantilever used as Viscometer and Microrheometer. Proc. IEEE Sens. 2006, 4, 753–756. [Google Scholar] [CrossRef]
- Lemaire, E.; Caillard, B.; Youssry, M.; Dufour, I. High-frequency viscoelastic measurements of fluids based on microcantilever sensing: New modeling and experimental issues. Sens. Actuators A Phys. 2013, 201, 230–240. [Google Scholar] [CrossRef] [Green Version]
- Youssry, M.; Lemaire, E.; Caillard, B.; Colin, A.; Dufour, I. On-chip characterization of the viscoelasticity of complex fluids using microcantilevers. Meas. Sci. Technol. 2012, 23, 125306. [Google Scholar] [CrossRef]
- De Pastina, A.; Padovani, F.; Brunetti, G.; Rotella, C.; Niosi, F.; Usov, V.; Hegner, M. Multimodal real-time frequency tracking of cantilever arrays in liquid environment for biodetection: Comprehensive setup and performance analysis. Rev. Sci. Instrum. 2021, 92, 065001. [Google Scholar] [CrossRef]
- Labuda, A.; Kobayashi, K.; Kiracofe, D.; Suzuki, K.; Grütter, P.H.; Yamada, H. Comparison of photothermal and piezoacoustic excitation methods for frequency and phase modulation atomic force microscopy in liquid environments. AIP Adv. 2011, 1, 022136. [Google Scholar] [CrossRef]
- Asakawa, H.; Fukuma, T. Spurious-free cantilever excitation in liquid by piezoactuator with flexure drive mechanism. Rev. Sci. Instrum. 2009, 80, 103703. [Google Scholar] [CrossRef] [Green Version]
- Mouro, J.; Tiribilli, B.; Paoletti, P. Measuring viscosity with nonlinear self-excited microcantilevers. Appl. Phys. Lett. 2017, 111, 144101. [Google Scholar] [CrossRef] [Green Version]
- Mouro, J.; Paoletti, P.; Basso, M.; Tiribilli, B. Measuring Viscosity Using the Hysteresis of the Non-Linear Response of a Self-Excited Cantilever. Sensors 2021, 21, 5592. [Google Scholar] [CrossRef]
- Yabuno, H.; Higashino, K.; Kuroda, M.; Yamamoto, Y. Self-excited vibrational viscometer for high-viscosity sensing. J. Appl. Phys. 2014, 116, 124305. [Google Scholar] [CrossRef] [Green Version]
- Higashino, K.; Yabuno, H.; Aono, K.; Yamamoto, Y.; Kuroda, M. Self-Excited Vibrational Cantilever-Type Viscometer Driven by Piezo-Actuator. J. Vib. Acoust. 2015, 137, 061009. [Google Scholar] [CrossRef]
- Urasaki, S.; Yabuno, H.; Yamamoto, Y.; Matsumoto, S. Sensorless Self-Excited Vibrational Viscometer with Two Hopf Bifurcations Based on a Piezoelectric Device. Sensors 2021, 21, 1127. [Google Scholar] [CrossRef]
- Bircher, B.A.; Duempelmann, L.; Renggli, K.; Lang, H.P.; Gerber, C.; Bruns, N.; Braun, T. Real-Time Viscosity and Mass Density Sensors Requiring Microliter Sample Volume Based on Nanomechanical Resonators. Anal. Chem. 2013, 85, 8676–8683. [Google Scholar] [CrossRef] [Green Version]
- Ramos, D.; Tamayo, J.; Mertens, J.; Calleja, M. Photothermal excitation of microcantilevers in liquids. J. Appl. Phys. 2006, 99, 124904. [Google Scholar] [CrossRef] [Green Version]
- Ramos, D.; Mertens, J.; Calleja, M.; Tamayo, J. Phototermal self-excitation of nanomechanical resonators in liquids. Appl. Phys. Lett. 2008, 92, 173108. [Google Scholar] [CrossRef]
- Maali, A.; Hurth, C.; Boisgard, R.; Jai, C.; Cohen-Bouhacina, T.; Aimé, J.-P. Hydrodynamics of oscillating atomic force microscopy cantilevers in viscous fluids. J. Appl. Phys. 2005, 97, 074907. [Google Scholar] [CrossRef]
- Mouro, J.; Pinto, R.; Paoletti, P.; Tiribilli, B. Microcantilever: Dynamical Response for Mass Sensing and Fluid Characterization. Sensors 2020, 21, 115. [Google Scholar] [CrossRef]
- Mouro, J.; Tiribilli, B.; Paoletti, P. Nonlinear behaviour of self-excited microcantilevers in viscous fluids. J. Micromech. Microeng. 2017, 27, 095008. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mouro, J.; Paoletti, P.; Sartore, M.; Vassalli, M.; Tiribilli, B. Photothermal Self-Excitation of a Phase-Controlled Microcantilever for Viscosity or Viscoelasticity Sensing. Sensors 2022, 22, 8421. https://doi.org/10.3390/s22218421
Mouro J, Paoletti P, Sartore M, Vassalli M, Tiribilli B. Photothermal Self-Excitation of a Phase-Controlled Microcantilever for Viscosity or Viscoelasticity Sensing. Sensors. 2022; 22(21):8421. https://doi.org/10.3390/s22218421
Chicago/Turabian StyleMouro, João, Paolo Paoletti, Marco Sartore, Massimo Vassalli, and Bruno Tiribilli. 2022. "Photothermal Self-Excitation of a Phase-Controlled Microcantilever for Viscosity or Viscoelasticity Sensing" Sensors 22, no. 21: 8421. https://doi.org/10.3390/s22218421








