Development of an Accurate and Robust Air-Coupled Ultrasonic Time-of-Flight Measurement Technique
Abstract
:1. Introduction
2. Theory
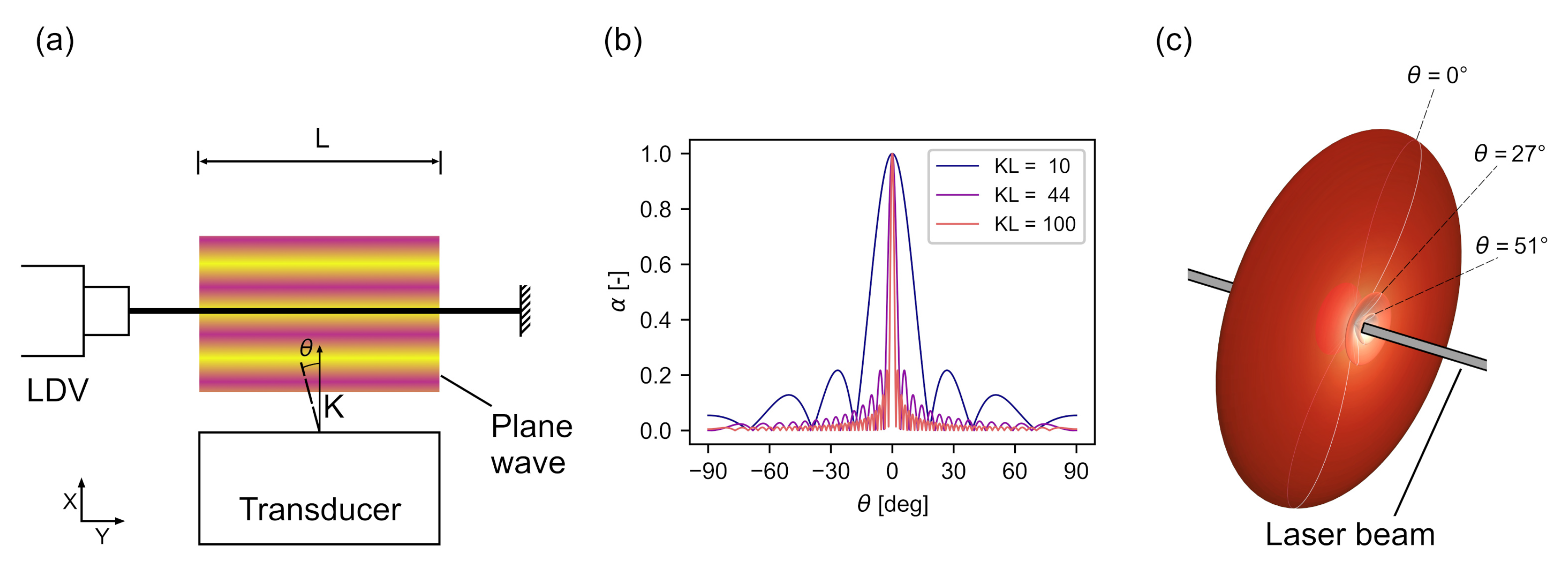
2.1. Refracto-Vibrometry
2.2. Time-of-Flight Measurements
2.3. Laser Positioning Error
3. Methods and Materials
4. Results and Discussion
4.1. Signal
4.2. Hardware Delay
4.3. Experimental Verification
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Josserand, T.; Wolley, J. A miniature high resolution 3-D imaging sonar. Ultrasonics 2011, 51, 275–280. [Google Scholar] [CrossRef] [PubMed]
- Jackson, J.C.; Summan, R.; Dobie, G.I.; Whiteley, S.M.; Pierce, S.G.; Hayward, G. Time-of-flight measurement techniques for airborne ultrasonic ranging. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2013, 60, 343–355. [Google Scholar] [CrossRef] [PubMed]
- Yi, D.; Jin, H.; Kim, M.C.; Kim, S.C. An Ultrasonic Object Detection Applying the ID Based on Spread Spectrum Technique for a Vehicle. Sensors 2020, 20, 414. [Google Scholar] [CrossRef] [Green Version]
- Verellen, T.; Kerstens, R.; Steckel, J. High-Resolution Ultrasound Sensing for Robotics Using Dense Microphone Arrays. IEEE Access 2020, 8, 190083–190093. [Google Scholar] [CrossRef]
- Stiefmeier, T.; Ogris, G.; Junker, H.; Lukowicz, P.; Troster, G. Combining Motion Sensors and Ultrasonic Hands Tracking for Continuous Activity Recognition in a Maintenance Scenario. In Proceedings of the 2006 10th IEEE International Symposium on Wearable Computers, Montreux, Switzerland, 11–14 October 2006; pp. 97–104. [Google Scholar] [CrossRef]
- Dahl, T.; Ealo, J.L.; Bang, H.J.; Holm, S.; Khuri-Yakub, P. Applications of airborne ultrasound in human–computer interaction. Ultrasonics 2014, 54, 1912–1921. [Google Scholar] [CrossRef]
- Pantea, C.; Rickel, D.G.; Migliori, A.; Leisure, R.G.; Zhang, J.; Zhao, Y.; El-Khatib, S.; Li, B. Digital ultrasonic pulse-echo overlap system and algorithm for unambiguous determination of pulse transit time. Rev. Sci. Instrum. 2005, 76, 1–9. [Google Scholar] [CrossRef]
- Philippidis, T.P.; Aggelis, D.G. Experimental study of wave dispersion and attenuation in concrete. Ultrasonics 2005, 43, 584–595. [Google Scholar] [CrossRef]
- Grohmann, M.; Niederleithinger, E.; Buske, S. Geometry Determination of a Foundation Slab Using the Ultrasonic Echo Technique and Geophysical Migration Methods. J. Nondestr. Eval. 2016, 35, 17. [Google Scholar] [CrossRef]
- Marhenke, T.; Neuenschwander, J.; Furrer, R.; Twiefel, J.; Hasener, J.; Niemz, P.; Sanabria, S.J. Modeling of delamination detection utilizing air-coupled ultrasound in wood-based composites. NDT E Int. 2018, 99, 1–12. [Google Scholar] [CrossRef]
- Si-Chaib, M.O.; Djelouah, H.; Boutkedjirt, T. Propagation of ultrasonic waves in materials under bending forces. NDT E Int. 2005, 38, 283–289. [Google Scholar] [CrossRef]
- Haslinger, S.G.; Lowe, M.J.S.; Wang, Z.; Shi, F. Time of flight diffraction for rough planar defects. NDT E Int. 2021, 124, 102521. [Google Scholar] [CrossRef]
- Liu, M.; Chen, S.; Wong, Z.Z.; Yao, K.; Cui, F. In situ disbond detection in adhesive bonded multi-layer metallic joint using time-of-flight variation of guided wave. Ultrasonics 2020, 102, 106062. [Google Scholar] [CrossRef]
- Landis, E.N.; Hassefras, E.; Oesch, T.S.; Niederleithinger, E. Relating ultrasonic signals to concrete microstructure using X-ray computed tomography. Constr. Build. Mater. 2021, 268, 121124. [Google Scholar] [CrossRef]
- Sampath, S.; Dhayalan, R.; Kumar, A.; Kishore, N.N.; Sohn, H. Evaluation of material degradation using phased array ultrasonic technique with full matrix capture. Eng. Fail. Anal. 2021, 120, 105118. [Google Scholar] [CrossRef]
- Bhadwal, N.; Torabi Milani, M.; Coyle, T.; Sinclair, A. Dry Coupling of Ultrasonic Transducer Components for High Temperature Applications. Sensors 2019, 19, 5383. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schickert, M.; Krause, M. Ultrasonic techniques for evaluation of reinforced concrete structures. In Non-Destructive Evaluation of Reinforced Concrete Structures; Maierhofer, C., Reinhardt, H.W., Dobmann, G., Eds.; Woodhead Publishing: Oxford, UK; Cambridge, UK; New Delhi, India, 2010; Volume 2, pp. 490–530. [Google Scholar] [CrossRef]
- Krautkrämer, J.; Krautkrämer, H. Coupling. In Ultrasonic Testing of Materials; Springer: Berlin/Heidelberg, Germany, 1990; pp. 266–278. [Google Scholar] [CrossRef]
- Mihaljević, M.; Markučič, D.; Runje, B.; Keran, Z. Measurement uncertainty evaluation of ultrasonic wall thickness measurement. Measurement 2019, 137, 179–188. [Google Scholar] [CrossRef]
- Klinger, C.; Bettge, D. Axle fracture of an ICE3 high speed train. Eng. Fail. Anal. 2013, 35, 66–81. [Google Scholar] [CrossRef]
- Alzuhiri, M.; Song, J.; Li, B.; Kumar, D.; Qiu, Z.; Qian, J.; Deng, Y. Enhanced pulsed thermoacoustic imaging by noncoherent pulse compression. J. Appl. Phys. 2021, 130, 174902. [Google Scholar] [CrossRef]
- Gan, T.H.; Hutchins, D.A.; Billson, D.R.; Schindel, D.W. The use of broadband acoustic transducers and pulse-compression techniques for air-coupled ultrasonic imaging. Ultrasonics 2001, 39, 181–194. [Google Scholar] [CrossRef]
- Chimenti, D.E. Review of air-coupled ultrasonic materials characterization. Ultrasonics 2014, 54, 1804–1816. [Google Scholar] [CrossRef]
- Fang, Y.; Lin, L.; Feng, H.; Lu, Z.; Emms, G.W. Review of the use of air-coupled ultrasonic technologies for nondestructive testing of wood and wood products. Comput. Electron. Agr. 2017, 137, 79–87. [Google Scholar] [CrossRef]
- Wang, X.; Gong, X.; Li, C.; Wu, R.; Chen, Z.; Wu, H.; Zhang, D.; Cao, X. Low insertion loss air-coupled ultrasonic transducer with parallel laminated piezoelectric structure. AIP Adv. 2020, 10, 105331. [Google Scholar] [CrossRef]
- Khyam, M.O.; Ge, S.S.; Li, X.; Pickering, M.R. Highly Accurate Time-of-Flight Measurement Technique Based on Phase-Correlation for Ultrasonic Ranging. IEEE Sens. J. 2017, 17, 434–443. [Google Scholar] [CrossRef]
- Fitch, J.P. Radar Processing. In Synthetic Aperture Radar; Springer: New York, NY, USA, 1988; pp. 1–32. [Google Scholar] [CrossRef]
- Bühling, B.; Strangfeld, C.; Maack, S.; Schweitzer, T. Experimental analysis of the acoustic field of an ultrasonic pulse induced by a fluidic switch. J. Acoust. Soc. Am. 2021, 149, 2150–2158. [Google Scholar] [CrossRef]
- Bühling, B.; Maack, S.; Schweitzer, T.; Strangfeld, C. Enhancing the spectral signatures of ultrasonic fluidic transducer pulses for improved time-of-flight measurements. Ultrasonics 2022, 119, 106612. [Google Scholar] [CrossRef] [PubMed]
- Esslinger, D.; Rapp, P.; Sawodny, O.; Tarin, C. High Precision Opto-Acoustic BPSK-CDMA Distance Measurement for Object Tracking. In Proceedings of the 2018 IEEE International Conference on Systems, Man, and Cybernetics (SMC), Miyazaki, Japan, 7–10 October 2018; pp. 2898–2905. [Google Scholar] [CrossRef]
- Suñol, F.; Ochoa, D.A.; Garcia, J.E. High-Precision Time-of-Flight Determination Algorithm for Ultrasonic Flow Measurement. IEEE Trans. Instrum. Meas. 2019, 68, 2724–2732. [Google Scholar] [CrossRef]
- Jia, L.; Xue, B.; Chen, S.; Wu, H.; Yang, X.; Zhai, J.; Zeng, Z. A High-Resolution Ultrasonic Ranging System Using Laser Sensing and a Cross-Correlation Method. Appl. Sci. 2019, 9, 1483. [Google Scholar] [CrossRef] [Green Version]
- Leetang, K.; Hachiya, H.; Hirata, S. Evaluation of ultrasonic target detection by alternate transmission of different codes in M-sequence pulse compression. In Proceedings of the 2020 IEEE International Ultrasonics Symposium (IUS), Las Vegas, NV, USA, 7–11 September 2020; pp. 1–4. [Google Scholar] [CrossRef]
- Gómez Álvarez-Arenas, T.E.; Benedito, J.; Corona, E. Non-contact ultrasonic assessment of the properties of vacuum-packaged dry-cured ham. In Proceedings of the 2009 IEEE International Ultrasonics Symposium, Rome, Italy, 20–23 September 2009; pp. 2541–2544. [Google Scholar] [CrossRef]
- Pallav, P.; Hutchins, D.A.; Gan, T.H. Air-coupled ultrasonic evaluation of food materials. Ultrasonics 2009, 49, 244–253. [Google Scholar] [CrossRef]
- Álvarez, F.J.; Kuc, R. Dispersion relation for air via Kramers-Kronig analysis. J. Acoust. Soc. Am. 2008, 124, EL57–EL61. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kandula, M. Sound propagation in saturated gas-vapor-droplet suspensions with droplet evaporation and nonlinear relaxation. J. Acoust. Soc. Am. 2012, 131, EL434–EL440. [Google Scholar] [CrossRef]
- Choi, D.W.; McIntyre, C.; Hutchins, D.A.; Billson, D.R. Gas jet as a waveguide for air-coupled ultrasound. Ultrasonics 2002, 40, 145–151. [Google Scholar] [CrossRef]
- Torras-Rosell, A.; Barrera-Figueroa, S.; Jacobsen, F. An acousto-optic beamformer. J. Acoust. Soc. Am. 2012, 132, 144–149. [Google Scholar] [CrossRef] [PubMed]
- Zipser, L.; Franke, H. Refracto-Vibrometry for Visualizing Ultrasound in Gases, Fluids and Condensed Matter. In Proceedings of the 2007 IEEE Ultrasonics Symposium, New York, NY, USA, 28–31 October 2007; pp. 395–398. [Google Scholar] [CrossRef]
- Zipser, L.; Franke, H. Visualization and measurement of acoustic and fluidic phenomena using a laser-scanning vibrometer. In Proceedings of the Fifth International Conference on Vibration Measurements by Laser Techniques, Ancona, Italy, 18–21 June 2002; Volume 4827, pp. 192–198. [Google Scholar] [CrossRef]
- Malkin, R.; Todd, T.; Robert, D. A simple method for quantitative imaging of 2D acoustic fields using refracto-vibrometry. J. Sound Vib. 2014, 333, 4473–4482. [Google Scholar] [CrossRef] [Green Version]
- Oikawa, Y.; Goto, M.; Ikeda, Y.; Takizawa, T.; Yamasaki, Y. Sound field measurements based on reconstruction from laser projections. In Proceedings of the IEEE International Conference on Acoustics, Speech, and Signal Processing (ICASSP ’05), Philadelphia, PA, USA, 23 March 2005; Volume 4, pp. iv/661–iv/664. [Google Scholar] [CrossRef] [Green Version]
- Torras-Rosell, A.; Barrera-Figueroa, S.; Jacobsen, F. Sound field reconstruction using acousto-optic tomography. J. Acoust. Soc. Am. 2012, 131, 3786–3793. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Martarelli, M.; Castellini, P.; Tomasini, E.P. Subsonic jet pressure fluctuation characterization by tomographic laser interferometry. Exp. Fluids 2013, 54, 1626. [Google Scholar] [CrossRef]
- Oikawa, Y.; Ishikawa, K.; Yatabe, K.; Onuma, T.; Niwa, H. Seeing the sound we hear: Optical technologies for visualizing sound wave. In Proceedings of the SPIE Three-Dimensional Imaging, Visualization, and Display 2018, Orlando, FL, USA, 16 May 2018; Volume 10666, pp. 106660C-1–106660C-3. [Google Scholar] [CrossRef]
- Torras-Rosell, A. New Measurements Techniques: Optical Methods for Characterizing Sound Fields. Ph.D. Thesis, Technical University of Denmark, Kongens Lyngby, Denmark, 2014. [Google Scholar]
- Solodov, I.; Döring, D.; Busse, G. Air-coupled laser vibrometry: Analysis and applications. Appl. Opt. 2009, 48, C33–C37. [Google Scholar] [CrossRef] [PubMed]
- Butler, J.L.; Sherman, C.H. Acoustic Radiation from Transducers. In Transducers and Arrays for Underwater Sound; Springer International Publishing: Cham, Switzerlands, 2016; pp. 517–553. [Google Scholar] [CrossRef]
- Maack, S. Untersuchungen zum Schallfeld Niederfrequenter Ultraschallprüfkopfe für Die Anwendung im Bauwesen [Investigations of the Sound Field of Low-Frequency Ultrasonic Probes for Use in Civil Engineering]. Ph.D. Thesis, Technische Universität Berlin, Berlin, Germany, 2012. [Google Scholar]
- Grohmann, M.; Müller, S.; Niederleithinger, E.; Sieber, S. Reverse time migration: Introducing a new imaging technique for ultrasonic measurements in civil engineering. Surf. Geophys. 2017, 15, 242–258. [Google Scholar] [CrossRef] [Green Version]
- Coelho Lima, I.; Grohmann, M.; Niederleithinger, E. Advanced Ultrasonic Imaging for Concrete: Alternative Imaging Conditions for Reverse Time Migration. In Proceedings of the 2018 DGZfP Jahrestagung, Leipzig, Germany, 7–9 May 2018; pp. 1–10. [Google Scholar]
- Hall, K.S. Air-Coupled Ultrasonic Tomographic Imaging of Concrete Elements. Ph.D. Thesis, University of Illinois at Urbana-Champaign, Champaign, IL, USA, 2011. [Google Scholar]
- Choi, H.; Popovics, J.S. NDE application of ultrasonic tomography to a full-scale concrete structure. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2015, 62, 1076–1085. [Google Scholar] [CrossRef]
- Virtanen, P.; Gommers, R.; Oliphant, T.E.; Haberl, M.; Reddy, T.; Cournapeau, D.; Burovski, E.; Peterson, P.; Weckesser, W.; Bright, J.; et al. SciPy 1.0: Fundamental Algorithms for Scientific Computing in Python. Nat. Methods 2020, 17, 261–272. [Google Scholar] [CrossRef] [Green Version]
- Bühling, B.; Maack, S.; Schönsee, E.; Schweitzer, T.; Strangfeld, C. Acoustic and flow data of fluidic and piezoelectric ultrasonic transducers. Data Brief 2021, 38, 107280. [Google Scholar] [CrossRef]
- Hutchins, D.; Burrascano, P.; Davis, L.; Laureti, S.; Ricci, M. Coded waveforms for optimised air-coupled ultrasonic nondestructive evaluation. Ultrasonics 2014, 54, 1745–1759. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Sheng Chen, G.; Liu, X. Vibrations and Advanced Dynamics. In Friction Dynamics; Woodhead Publishing: Duxford/ Kidlington, UK; Cambridge, MA, USA, 2016; pp. 7–90. [Google Scholar] [CrossRef]
- Nozato, H.; Shimoda, T.; Kokuyama, W. Dependence of frequency response on different velocity sensitivities of laser Doppler vibrometer. Meas. Sens. 2021, 18, 100301. [Google Scholar] [CrossRef]
- Zhu, Q.; Burtin, C.; Binetruy, C. Acoustoelastic effect in polyamide 6: Linear and nonlinear behaviour. Polym. Test. 2014, 40, 178–186. [Google Scholar] [CrossRef]
- Daschewski, M.; Boehm, R.; Prager, J.; Kreutzbruck, M.; Harrer, A. Physics of thermo-acoustic sound generation. J. Appl. Phys. 2013, 114, 1–12. [Google Scholar] [CrossRef]








Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bühling, B.; Küttenbaum, S.; Maack, S.; Strangfeld, C. Development of an Accurate and Robust Air-Coupled Ultrasonic Time-of-Flight Measurement Technique. Sensors 2022, 22, 2135. https://doi.org/10.3390/s22062135
Bühling B, Küttenbaum S, Maack S, Strangfeld C. Development of an Accurate and Robust Air-Coupled Ultrasonic Time-of-Flight Measurement Technique. Sensors. 2022; 22(6):2135. https://doi.org/10.3390/s22062135
Chicago/Turabian StyleBühling, Benjamin, Stefan Küttenbaum, Stefan Maack, and Christoph Strangfeld. 2022. "Development of an Accurate and Robust Air-Coupled Ultrasonic Time-of-Flight Measurement Technique" Sensors 22, no. 6: 2135. https://doi.org/10.3390/s22062135
APA StyleBühling, B., Küttenbaum, S., Maack, S., & Strangfeld, C. (2022). Development of an Accurate and Robust Air-Coupled Ultrasonic Time-of-Flight Measurement Technique. Sensors, 22(6), 2135. https://doi.org/10.3390/s22062135





