Human Gait Modeling, Prediction and Classification for Level Walking Using Harmonic Models Derived from a Single Thigh-Mounted IMU
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experimental Setup
2.2. Modeling of Thigh Angle
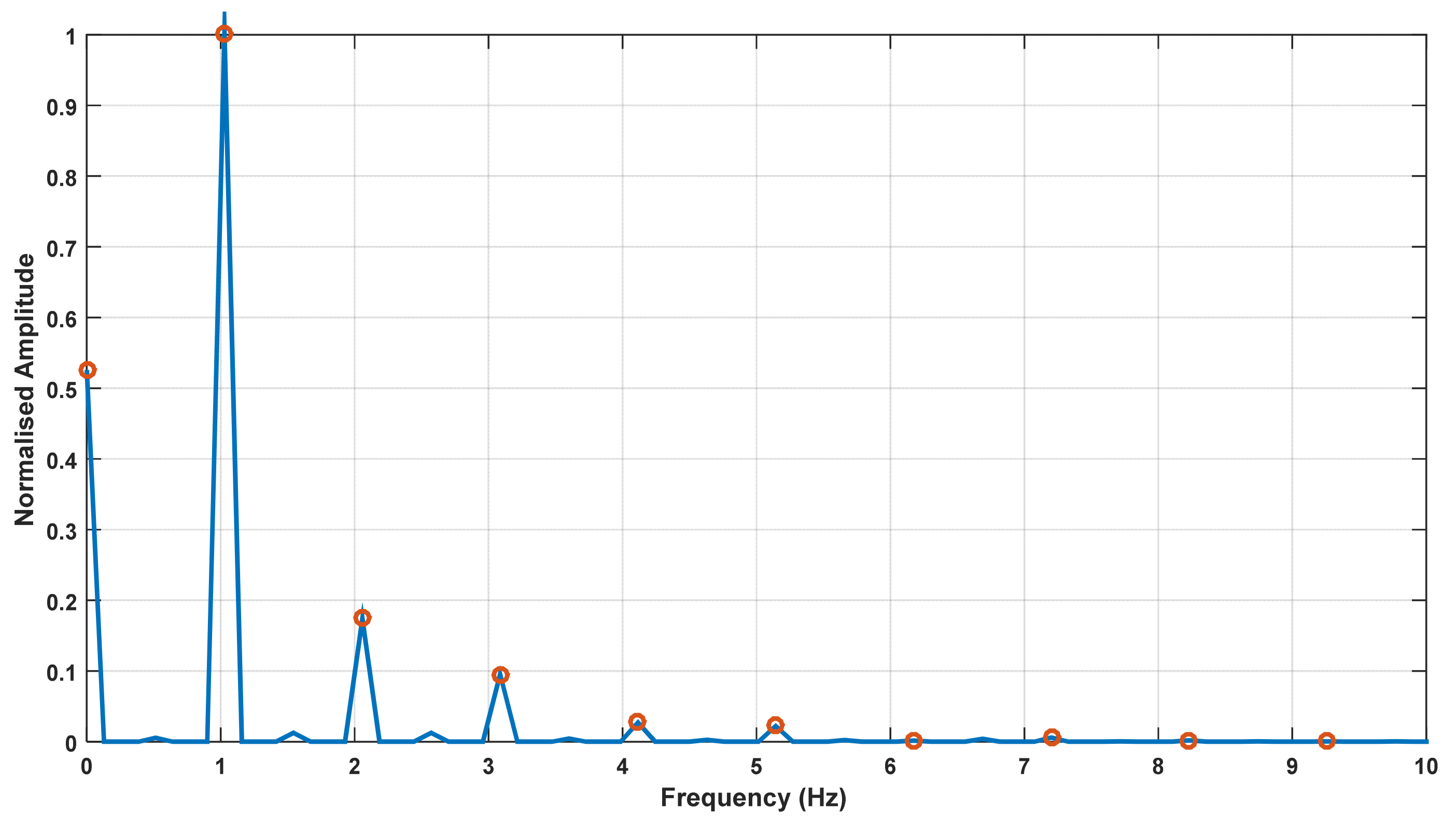
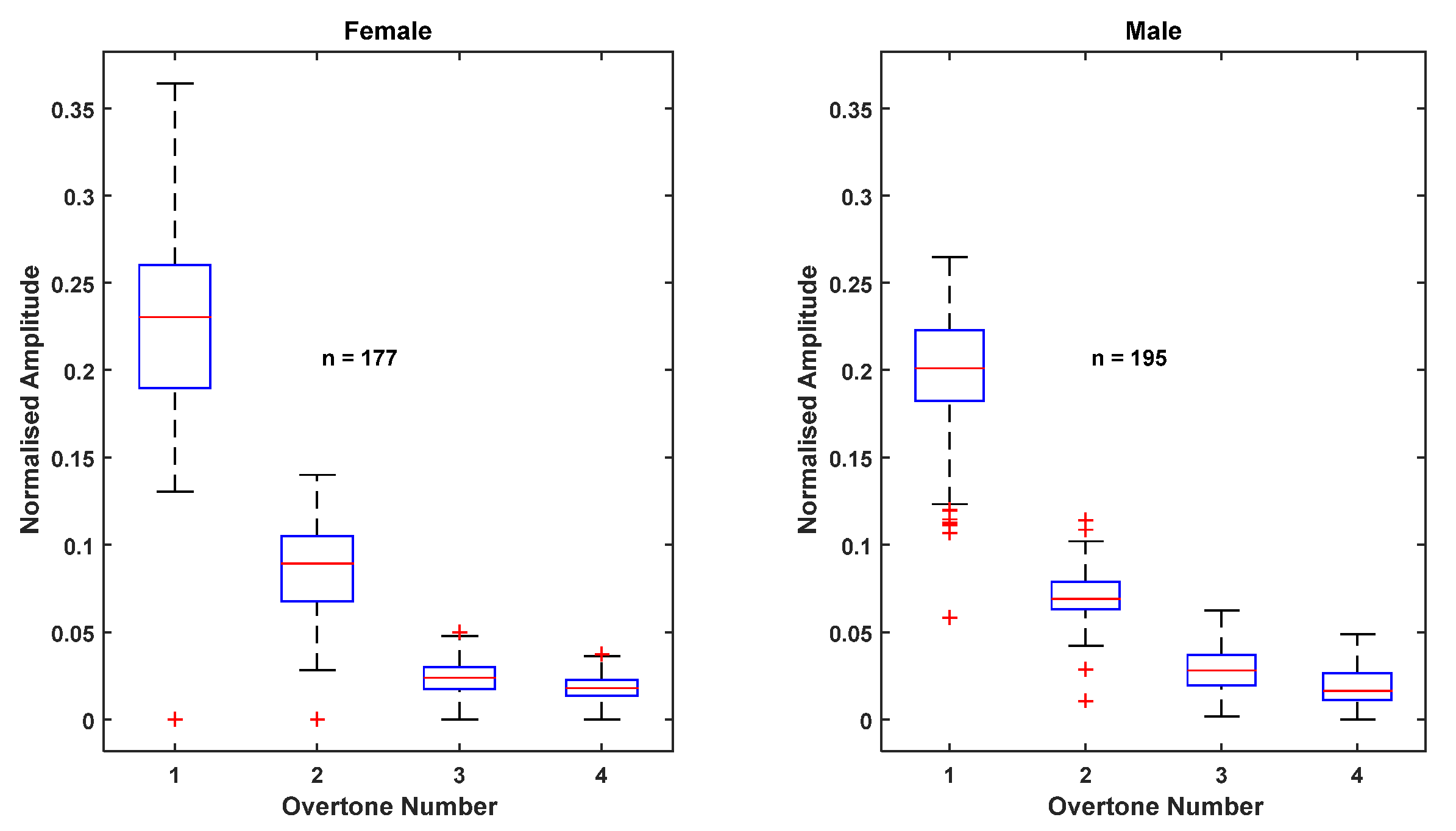
2.3. Harmonic Models for Thigh Flexion–Extension (Derived from IMU Data) and Gyro Signal
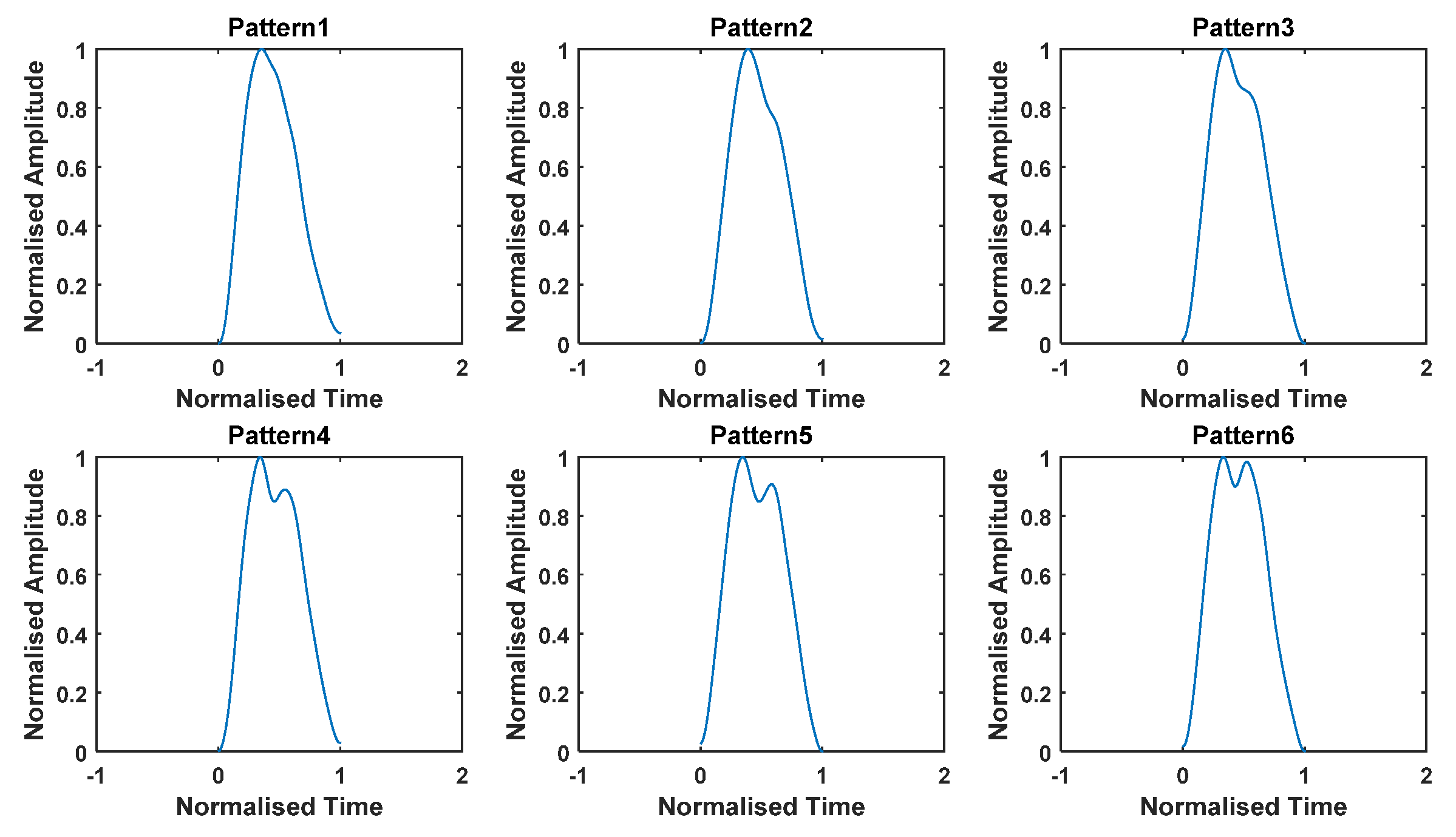
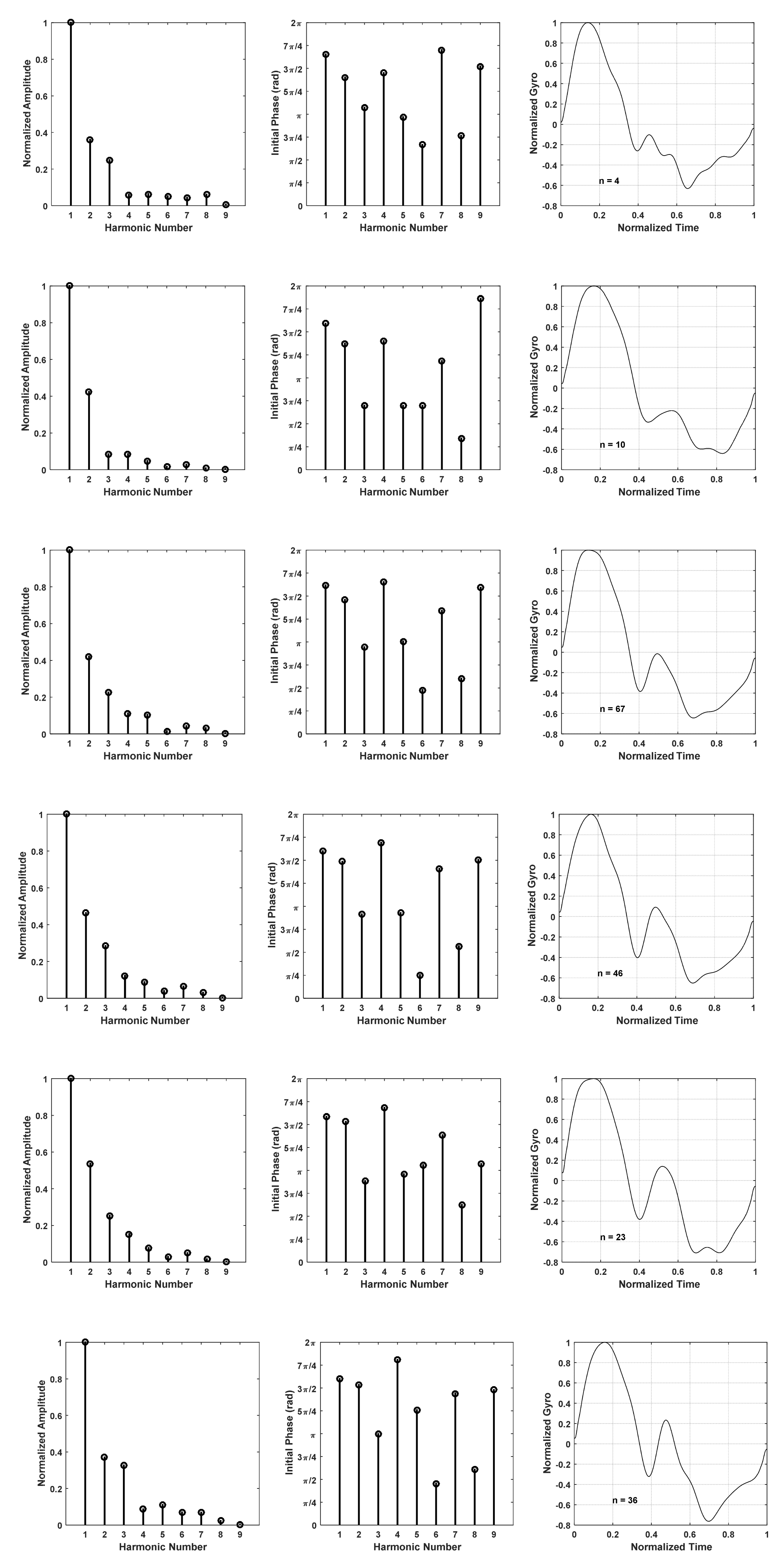
- Pattern 1—The heal contact is exactly at the end of the swing of the reference leg, and the foot is almost flat by the time of the heal contact, meaning that no oscillation of thigh is visible during the Loading Response;
- Pattern 2—The heal contact occurs slightly after the end of the swing of the reference leg, and there is a small angle between the foot and the ground, meaning that an angle change in the thigh is slightly visible during Loading Response;
- Pattern 3—The heal contact is slightly after the end of the swing of the reference leg, and there is a larger angle between the foot and the ground than in the previous case, meaning that a larger change of angle in the thigh is visible during the Loading Response;
- Pattern 4—The heal contact occurs after the end of the swing of the reference leg, so that the leg moves downwards before heal contact, and the foot is angled towards the ground by the time of the heal contact. Hence, an oscillation of the thigh is visible during Loading Response;
- Pattern 5—The heal contact occurs after the end of the swing of the reference leg, so that the leg moves downwards before heal contact and the foot is angled towards the ground by the time of the heal contact. More oscillation of the thigh is visible during Loading Response here compared to the previous case;
- Pattern 6—The heal contact occurs after the end of the swing of the reference leg, so that the leg moves downwards before heal contact and the foot is angled towards the ground by the time of the heal contact. A strong oscillation is visible during Loading Response, so that the secondary peak is comparable to the primary peak.
2.4. Predicting and Classifying Strides Using Harmonic Models
3. Results
3.1. Modeling of Thigh Angle
3.2. Harmonic Models for Thigh Flexion–Extension (Derived from IMU data) and Gyro Signal
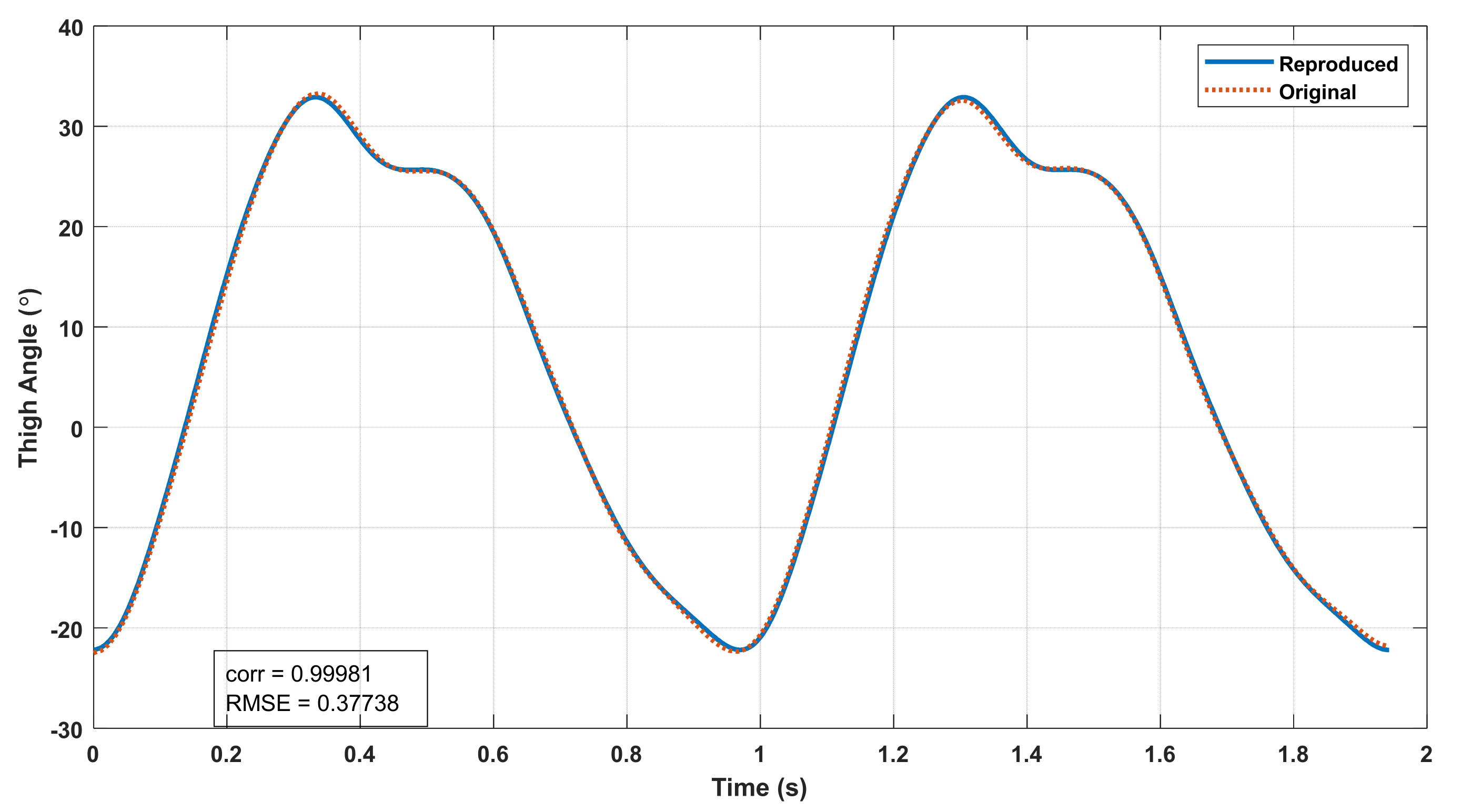
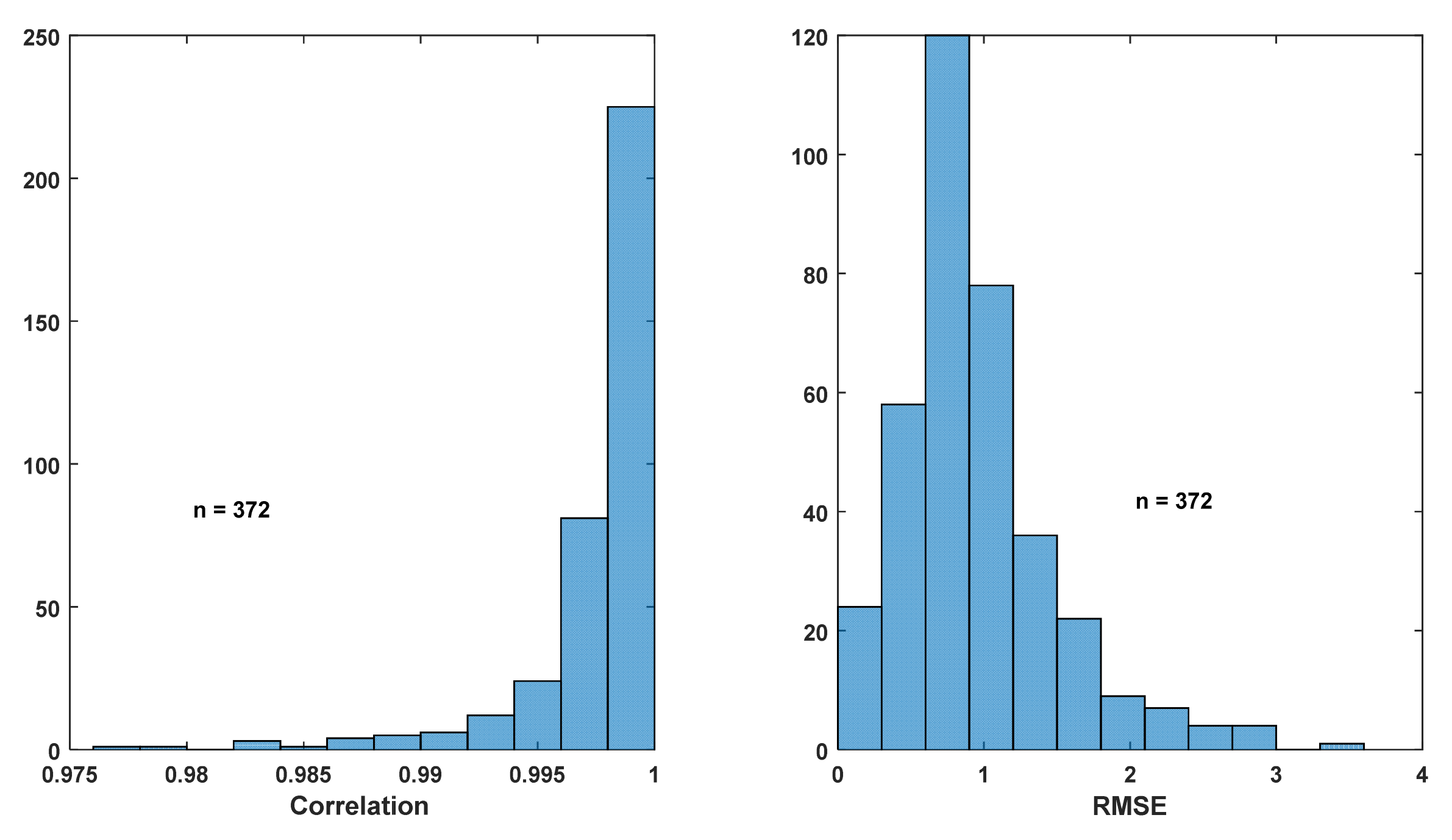
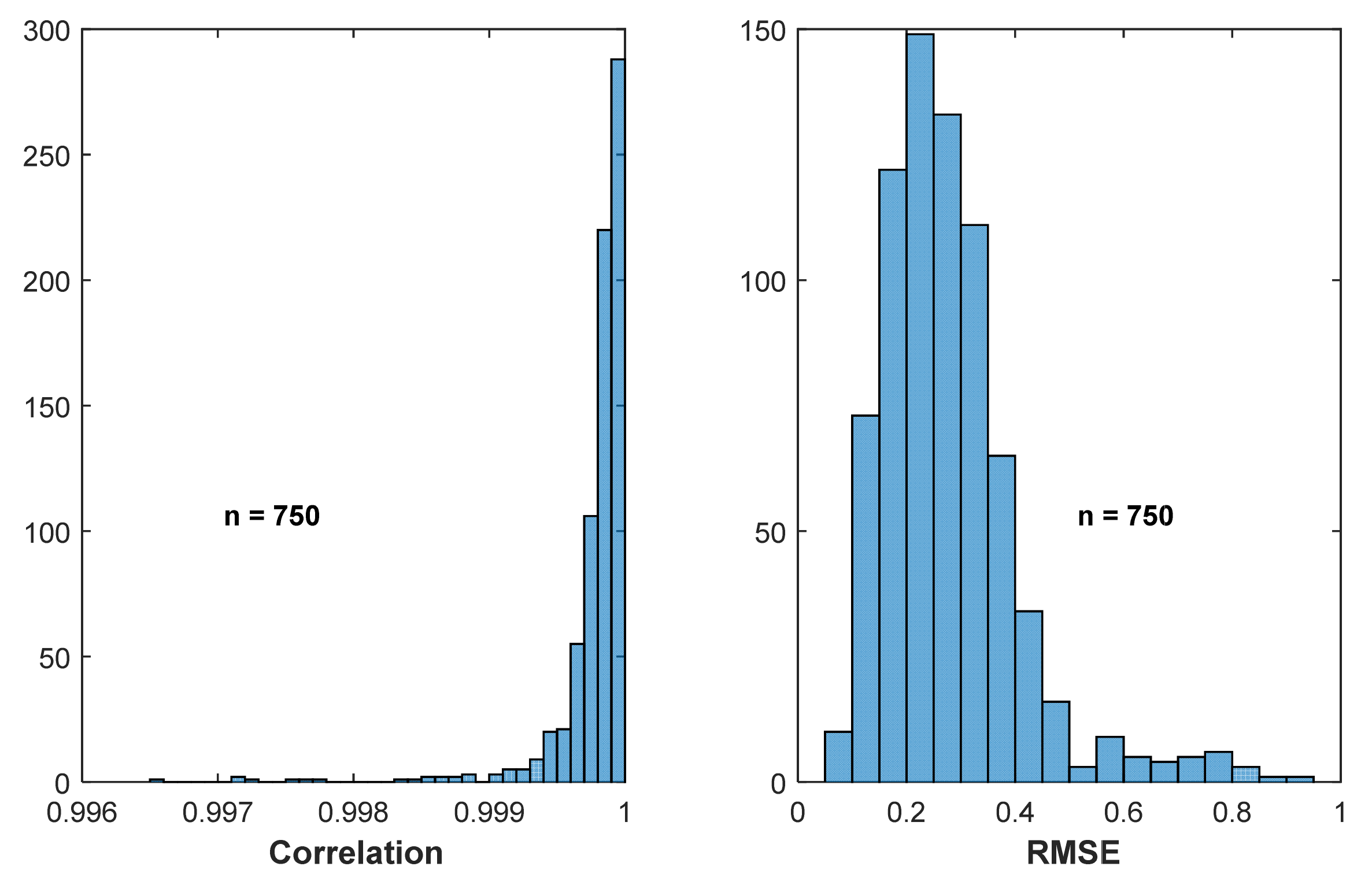
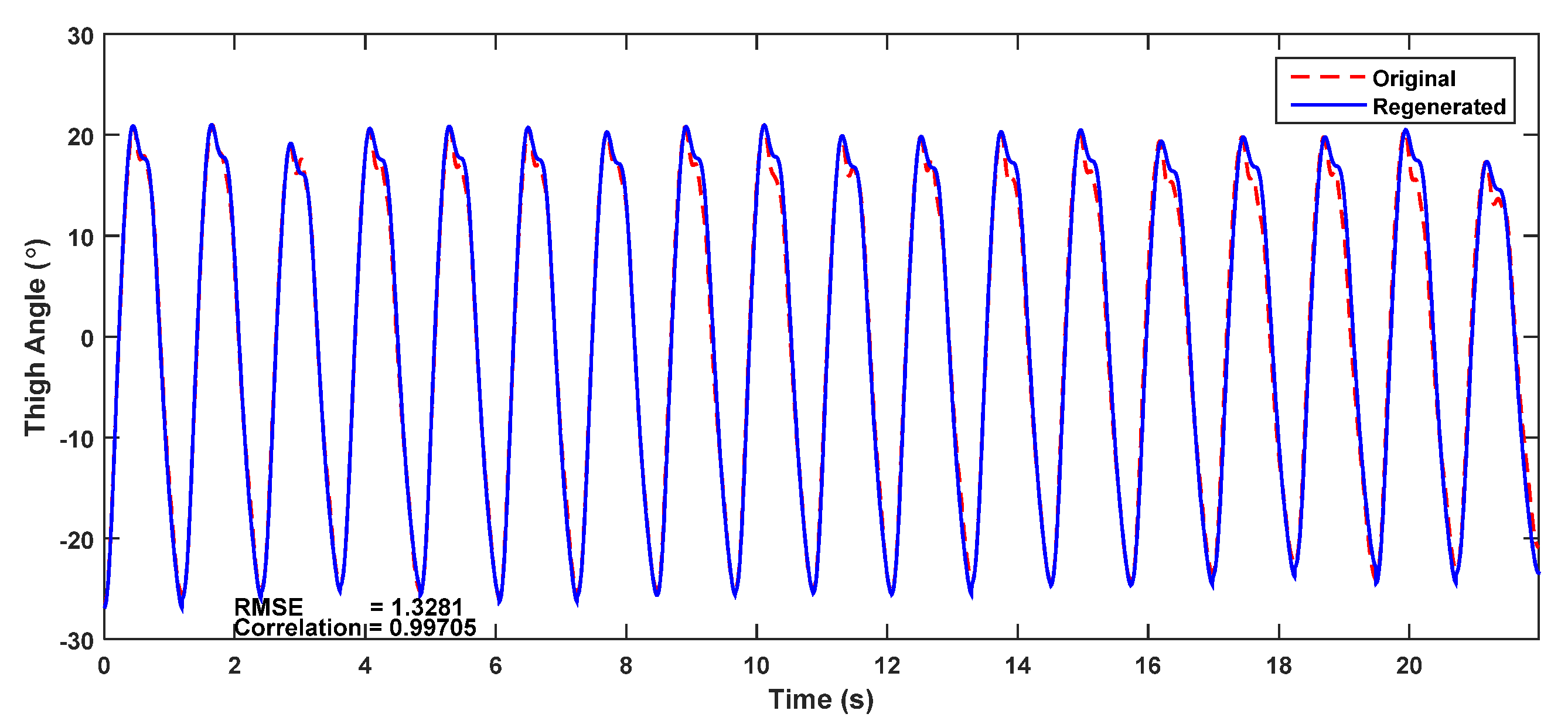
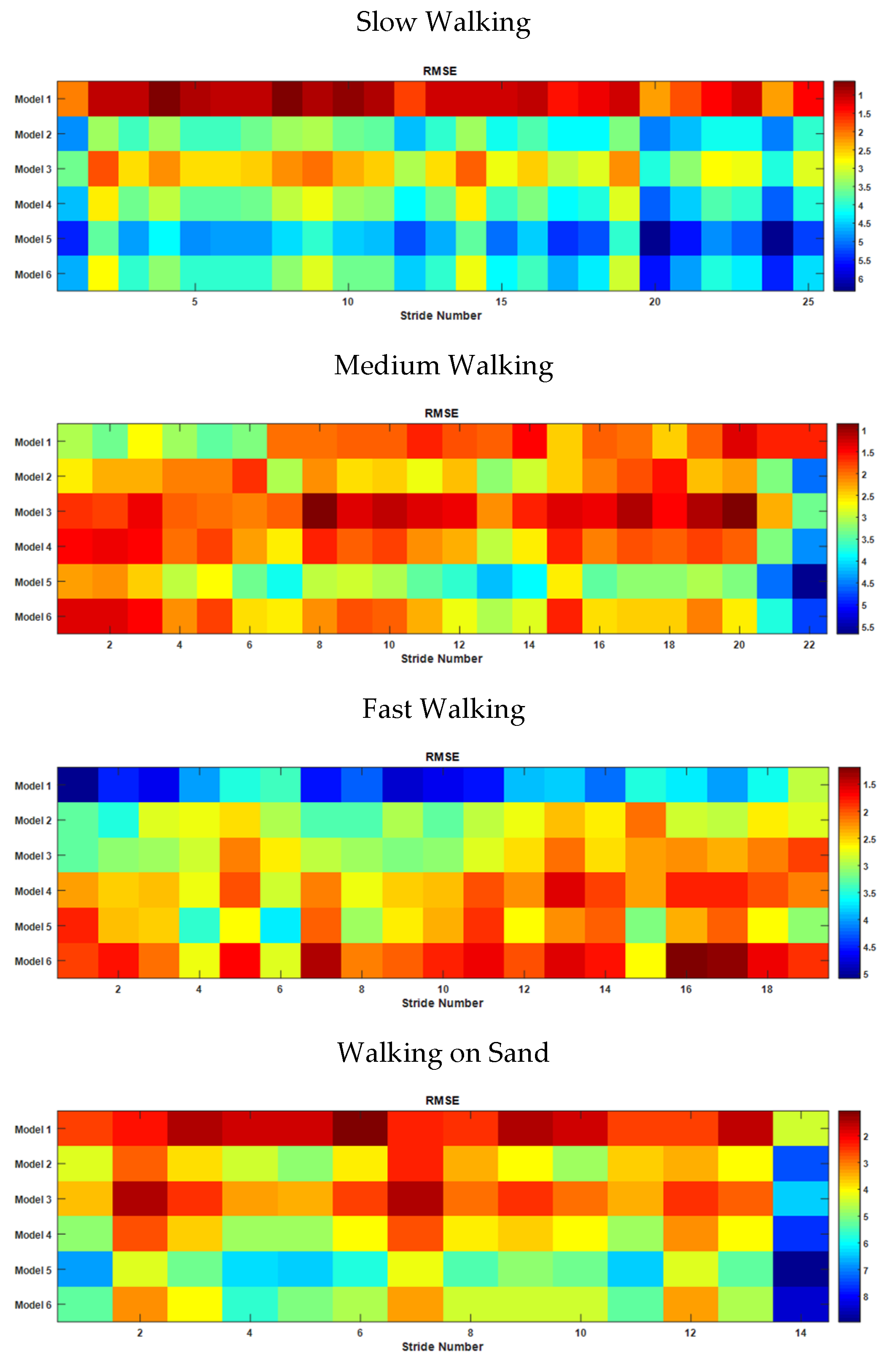
3.3. Predicting Thigh Angle Using the Harmonic Model
3.4. Classifying Strides Using the Harmonic Model
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Ethical Statements
References
- Bocksch, M.; Seitz, J.; Jahn, J. Pedestrian Activity Classification to Improve Human Tracking and Localization. In Proceedings of the Forth International Conference on Indoor Positioning and Indoor Navigation (IPIN2013), Montbeliard, France, 28–31 October 2013; Available online: http://ipin2013.sciencesconf.org/conference/ipin2013/eda_en.pdf (accessed on 1 January 2014).
- Ibrahim, R.K. Novel Gait Models and Features for Gait Patterns Classification. Ph.D. Thesis, School of Electrical Engineering and Telecommunication, The University of New South Wales, Sydney, Australia, 2011. [Google Scholar]
- Olsson, F.; Rantakokko, J.; Nygards, J. Cooperative Localization by Foot-Mounted Inertial Navigation Systems and Ultrawideband Ranging. In Proceedings of the Fifth International Conference on Indoor Positioning and Indoor Navigation (IPIN2014), Busan, Korea, 27–30 October 2014; Available online: http://www.ipin2014.org/wp/pdf/2A-4.pdf (accessed on 15 July 2015).
- Nilsson, J.-O.; Gupta, A.K.; Handel, P. Foot-mounted Inertial Navigation Made Easy. In Proceedings of the Fifth International Conference on Indoor Positioning and Indoor Navigation (IPIN2014), Busan, Korea, 27–30 October 2014; Available online: http://www.ipin2014.org/wp/pdf/1A-4.pdf (accessed on 1 July 2015).
- Abhayasinghe, N.; Murray, I. Human Gait Phase Recognition Based on Thigh Movement Computed using IMUs. In Proceedings of the 2014 IEEE Ninth International Conference on Intelligent Sensors, Sensor Networks and Information Processing (ISSNIP), Singapore, 21–24 April 2014; Available online: https://ieeexplore.ieee.org/document/6827604 (accessed on 19 June 2014).
- Diaz, E.M.; Gonzalez, A.L.M.; De Ponte Müller, F. Standalone Inertial Pocket Navigation System. In Proceedings of the Position, Location and Navigation Symposium—PLANS 2014, Monterey, CA, USA, 5–8 May 2014. [Google Scholar]
- Murray, M.P. Gait as a total pattern of movement. Am. J. Phys. Med. 1967, 46, 290–332. [Google Scholar] [PubMed]
- Geisheimer, J.L.; Greneker, E.F., III; Marshall, W.S. A high-resolution Doppler model of human gait. In Proceedings of the SPIE on Radar Technology, Orlando, FL, USA, 30 July 2002. [Google Scholar]
- Tong, K.; Granat, M.H. A practical gait analysis system using gyroscopes. Med. Eng. Phys. 1999, 21, 87–94. [Google Scholar] [CrossRef]
- Onyshko, S.; Winter, D.A. A mathematical model for the dynamics of human locomotion. J. Biomech. 1980, 13, 361–368. [Google Scholar] [CrossRef]
- Siegler, S.; Seliktar, R.; Hyman, W. Simulation of human gait with the aid of a simple mechanical model. J. Biomech. 1982, 15, 415–425. [Google Scholar] [CrossRef]
- Qiuyang, H.; Zaiyue, Y. Frequency-Estimation-Based Thigh Angle Prediction in Level-Ground Walking Using IMUs. In Proceedings of the 2013 International Conference on Cyber-Enabled Distributed Computing and Knowledge Discovery (CyberC), Beijing, China, 10–12 October 2013. [Google Scholar]
- Abhayasinghe, K.; Murray, I. A novel approach for indoor localization using human gait analysis with gyroscopic data. In Proceedings of the Third International Conference on Indoor Positioning and Indoor Navigation (IPIN2012), Sydney, Australia, 13–15 November 2012; Available online: http://www.surveying.unsw.edu.au/ipin2012/proceedings/submissions/22_Paper.pdf (accessed on 1 January 2014).
- Abhayasinghe, N.; Murray, I. The Application of “off-the-shelf” Components for Building IMUs for Navigation Research. In Proceedings of the Fifth International Conference on Indoor Positioning and Indoor Navigation (IPIN2014), Busan, Korea, 27–30 October 2014; Available online: http://www.ipin2014.org/wp/pdf/2A-3.pdf (accessed on 1 July 2015).
- Abhayasinghe, N.; Murray, I.; Sharif Bidabadi, S. Validation of Thigh Angle Estimation Using Inertial Measurement Unit Data against Optical Motion Capture Systems. Sensors 2019, 19, 596. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Albu, A.B.; Bergevin, R.; Quirion, S. Generic Temporal Segmentation of Cyclic Human Motion. Pattern Recognit. 2008, 41, 6–21. [Google Scholar] [CrossRef]
- Chang, C.; Ansari, R.; Khokha, A. Efficient tracking of cyclic human motion by component motion. IEEE Signal Process. Lett. 2004, 11, 941–944. [Google Scholar] [CrossRef]
- Quinn, B.G.; Hannan, E.J. The Estimation and Tracking of Frequency; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Chhoeum, V.; Kim, Y.; Min, S.-D. Estimation of Knee Joint Angle Using Textile Capacitive Sensor and Artificial Neural Network Implementing with Three Shoe Types at Two Gait Speeds: A Preliminary Investigation. Sensors 2021, 21, 5484. [Google Scholar] [CrossRef] [PubMed]
- Sarshar, M.; Polturi, S.; Schega, L. Gait Phase Estimation by Using LSTM in IMU-Based Gait Analysis—Proof of Concept. Sensors 2021, 21, 5749. [Google Scholar] [CrossRef] [PubMed]










| Model | 1st Harmonic | 2nd Harmonic | 3rd Harmonic | 4th Harmonic | 5th Harmonic | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | |
| 1 | 1 | 3.6133 | 0.18150 | 2.8125 | 0.08525 | 1.7860 | 0.015085 | 2.8816 | 0.013952 | 1.4683 |
| 2 | 1 | 3.4174 | 0.21348 | 2.7200 | 0.03001 | 0.6868 | 0.022038 | 2.7407 | 0.010615 | 0.7321 |
| 3 | 1 | 3.5088 | 0.20959 | 3.0176 | 0.07352 | 1.3860 | 0.028212 | 3.6534 | 0.019884 | 1.5846 |
| 4 | 1 | 3.4504 | 0.23148 | 3.0875 | 0.09581 | 1.2984 | 0.030199 | 3.727 | 0.018138 | 1.3387 |
| 5 | 1 | 3.4057 | 0.26781 | 3.2431 | 0.08322 | 1.1994 | 0.038494 | 3.7332 | 0.014827 | 1.4261 |
| 6 | 1 | 3.4515 | 0.18452 | 3.2409 | 0.10950 | 1.5581 | 0.021041 | 4.0952 | 0.022180 | 2.3650 |
| Model | 1st Harmonic | 2nd Harmonic | 3rd Harmonic | 4th Harmonic | 5th Harmonic | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | an | ϕn (rad) | |
| 1 | 1 | 5.19 | 0.36027 | 4.402 | 0.2489 | 3.3649 | 0.059486 | 4.5546 | 0.063368 | 3.0386 |
| 2 | 1 | 4.996 | 0.42343 | 4.3092 | 0.084499 | 2.1904 | 0.085638 | 4.3984 | 0.047704 | 2.1871 |
| 3 | 1 | 5.0759 | 0.42098 | 4.58 | 0.22486 | 2.9632 | 0.11145 | 5.1886 | 0.10383 | 3.1609 |
| 4 | 1 | 5.0243 | 0.46313 | 4.666 | 0.28408 | 2.8688 | 0.12266 | 5.3146 | 0.087658 | 2.9082 |
| 5 | 1 | 4.9736 | 0.53663 | 4.8087 | 0.25256 | 2.7775 | 0.15274 | 5.2844 | 0.077172 | 3.0107 |
| 6 | 1 | 5.0247 | 0.36973 | 4.8195 | 0.32584 | 3.1308 | 0.086019 | 5.6766 | 0.10968 | 3.9544 |
| Model | 6th Harmonic | 7th Harmonic | 8th Harmonic | 9th Harmonic | ||||||
| an | ϕn(rad) | an | ϕn(rad) | an | ϕn(rad) | an | ϕn(rad) | |||
| 1 | 0.051567 | 2.0871 | 0.043595 | 5.3394 | 0.06063 | 2.397 | 0.006469 | 4.7733 | ||
| 2 | 0.01632 | 2.187 | 0.028081 | 3.7139 | 0.008522 | 1.0669 | 0.00339 | 5.8446 | ||
| 3 | 0.014819 | 1.4992 | 0.042851 | 4.2084 | 0.031311 | 1.8894 | 0.002707 | 4.9969 | ||
| 4 | 0.039558 | 0.79304 | 0.064016 | 4.4247 | 0.030717 | 1.7806 | 0.002826 | 4.7229 | ||
| 5 | 0.029039 | 3.3201 | 0.049497 | 4.3447 | 0.015691 | 1.9591 | 0.000666 | 3.3624 | ||
| 6 | 0.069381 | 1.4251 | 0.067827 | 4.5243 | 0.022453 | 1.9092 | 0.000749 | 4.6469 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abhayasinghe, N.; Murray, I. Human Gait Modeling, Prediction and Classification for Level Walking Using Harmonic Models Derived from a Single Thigh-Mounted IMU. Sensors 2022, 22, 2164. https://doi.org/10.3390/s22062164
Abhayasinghe N, Murray I. Human Gait Modeling, Prediction and Classification for Level Walking Using Harmonic Models Derived from a Single Thigh-Mounted IMU. Sensors. 2022; 22(6):2164. https://doi.org/10.3390/s22062164
Chicago/Turabian StyleAbhayasinghe, Nimsiri, and Iain Murray. 2022. "Human Gait Modeling, Prediction and Classification for Level Walking Using Harmonic Models Derived from a Single Thigh-Mounted IMU" Sensors 22, no. 6: 2164. https://doi.org/10.3390/s22062164
APA StyleAbhayasinghe, N., & Murray, I. (2022). Human Gait Modeling, Prediction and Classification for Level Walking Using Harmonic Models Derived from a Single Thigh-Mounted IMU. Sensors, 22(6), 2164. https://doi.org/10.3390/s22062164







