Sensitivity-Tunable Terahertz Liquid/Gas Biosensor Based on Surface Plasmon Resonance with Dirac Semimetal
Abstract
:1. Introduction
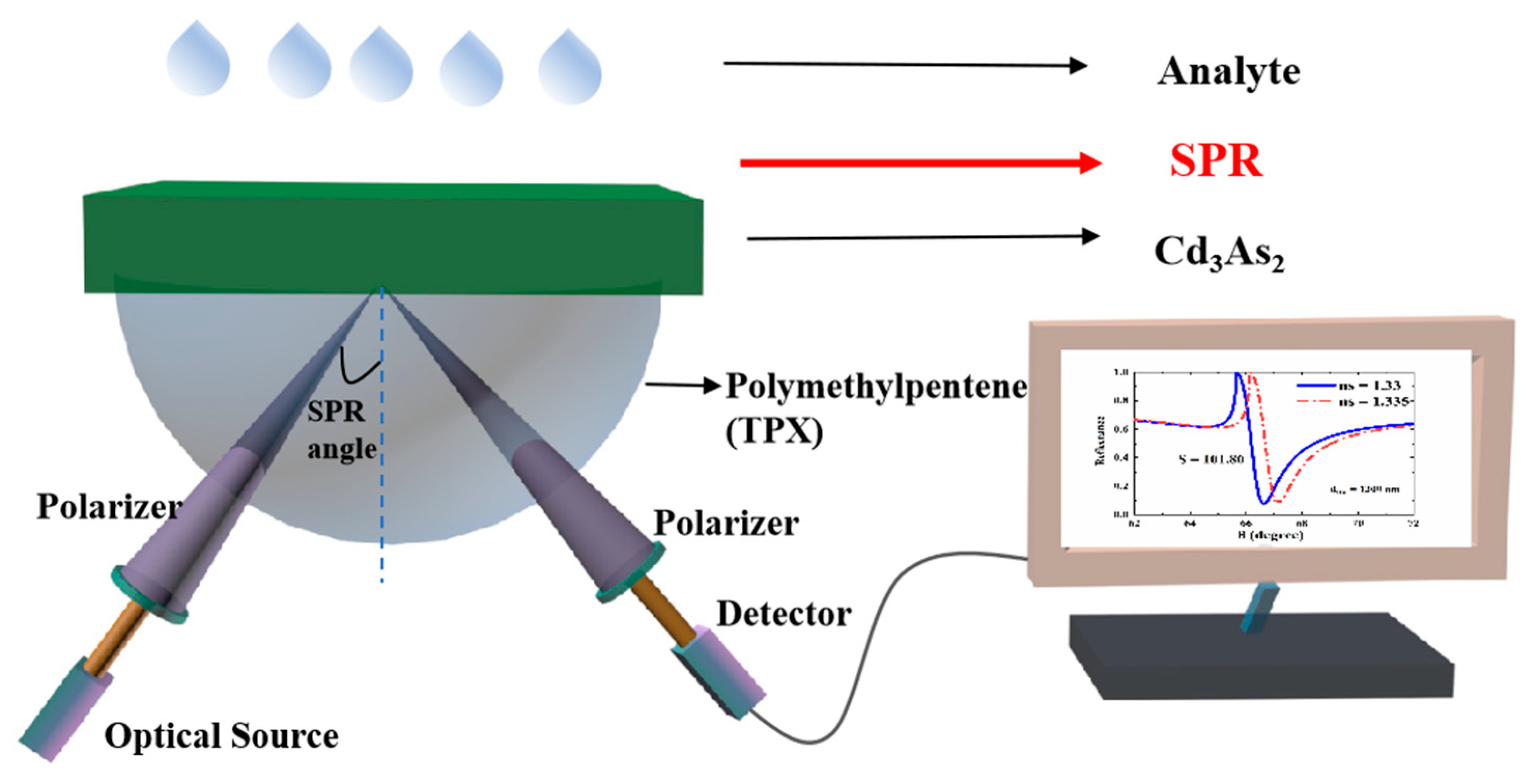
2. Materials and Methods
2.1. Theoretical Model
2.2. Methods
3. Results and Discussion
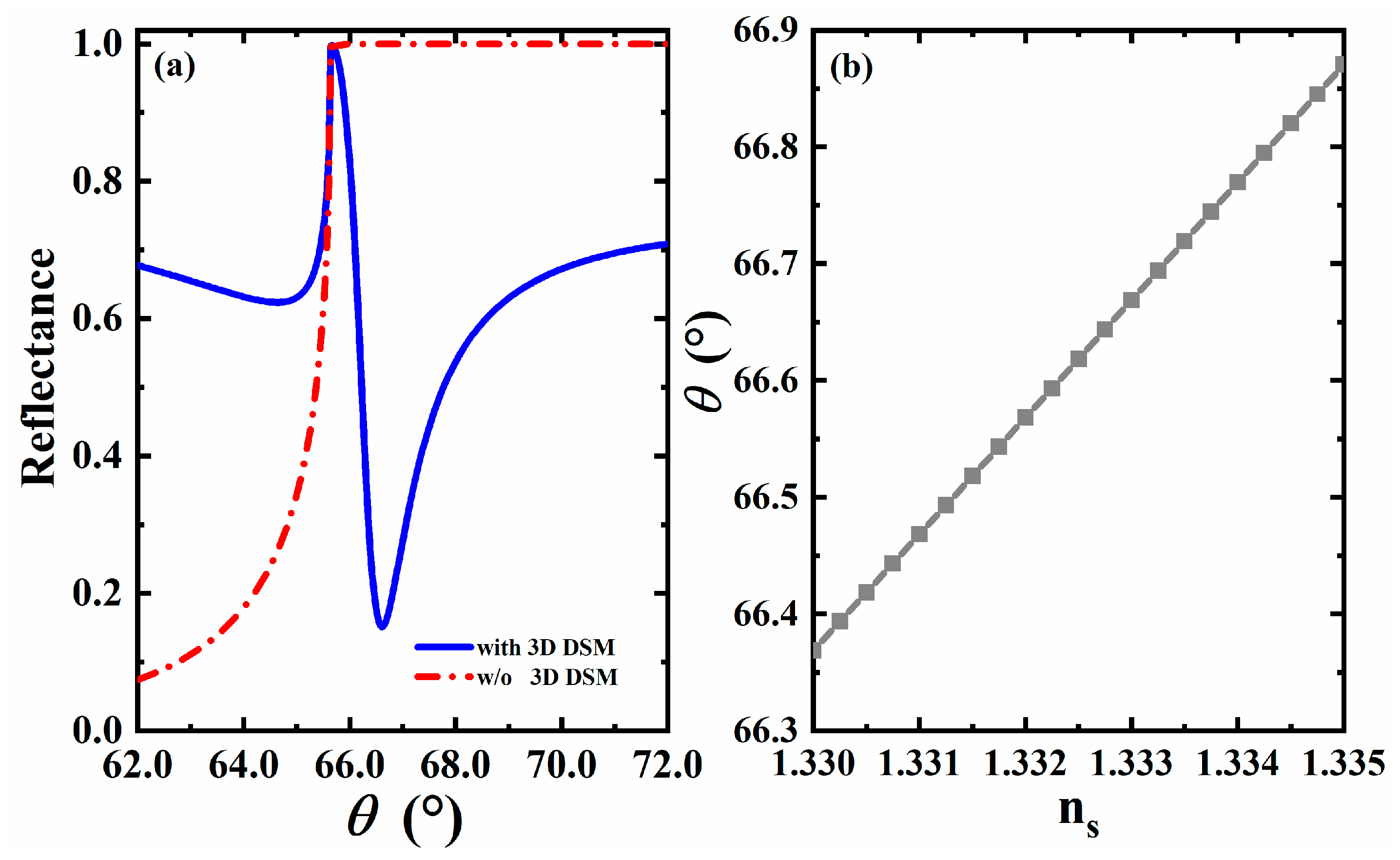
3.1. The Effect of 3D DSM
3.2. The Effect of Refractive Index on Formant Angle
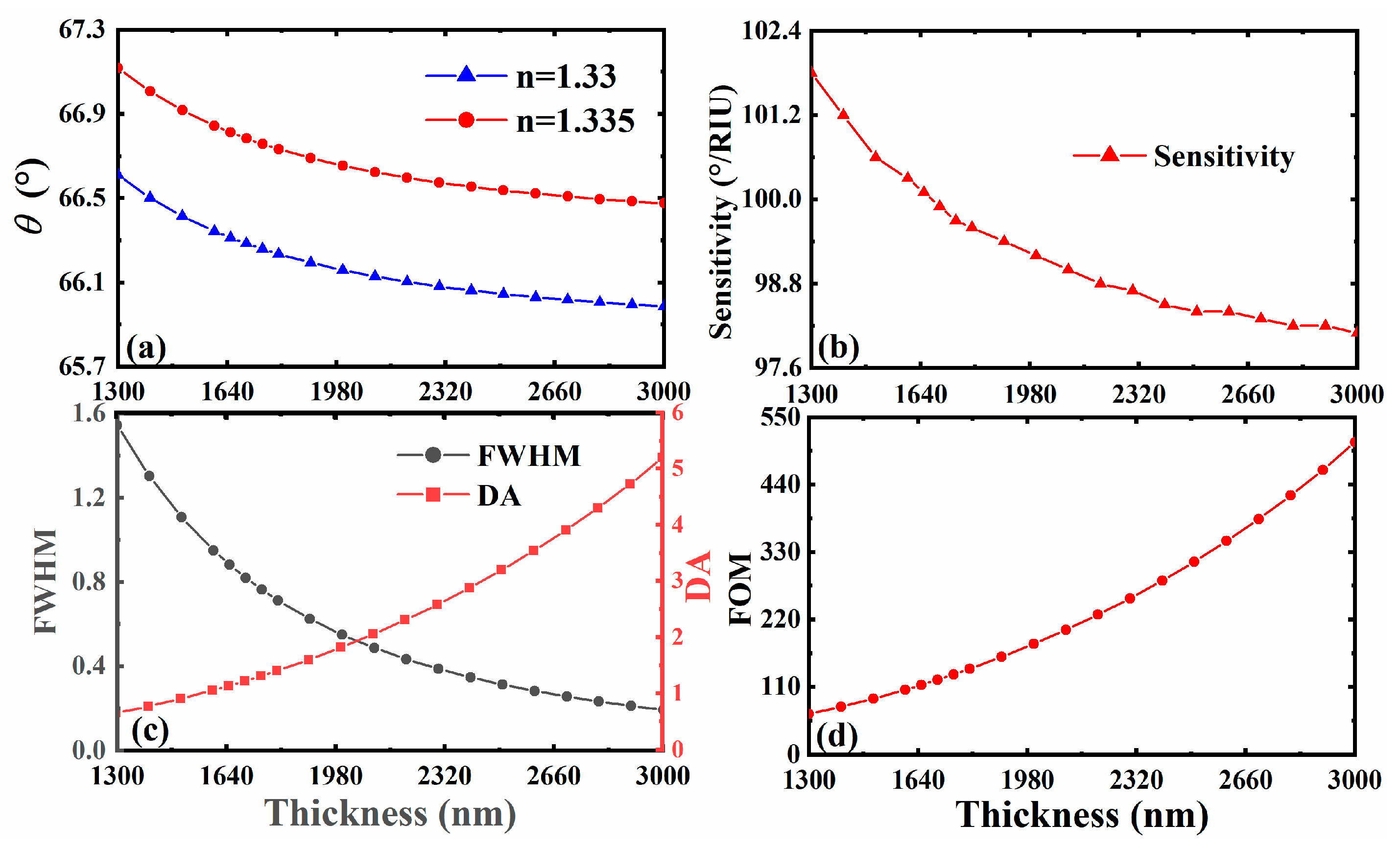
3.3. The Effect of Different Thickness of 3D DSM
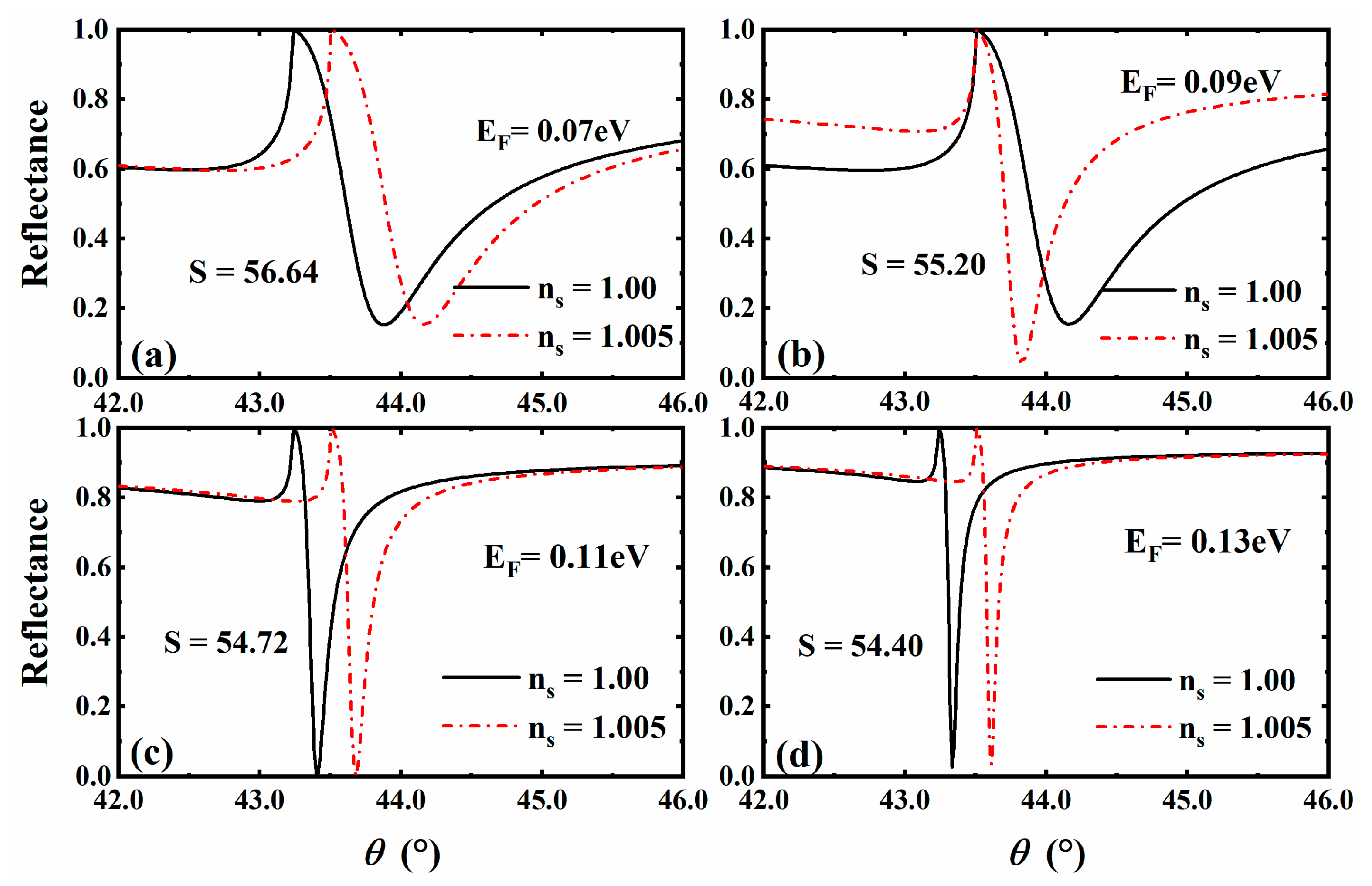
3.4. The Effect of Different Fermi Energy of 3D DSM
3.5. The Situation for Gas
3.6. Other Notes to This Article
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, Q.; Zhang, D.; Lu, Y.; Yao, Y.; Li, S.; Liu, Q. Graphene oxide-based optical biosensor functionalized with peptides for explosive detection. Biosens. Bioelectron. 2015, 68, 494–499. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.; Zhi, S.; Sun, X.; Lei, C.; Zhou, Y. Ultrasensitive detection of bioanalytes based on signal amplification of coil-integrated giant magnetoimpedance biosystems. Sens. Actuators B Chem. 2017, 247, 1–10. [Google Scholar] [CrossRef]
- Fan, X.; White, I.M.; Shopova, S.I.; Zhu, H.; Suter, J.D.; Sun, Y. Sensitive optical biosensors for unlabeled targets: A review. Anal. Chim. Acta 2008, 620, 8–26. [Google Scholar] [CrossRef] [PubMed]
- Son, M.H.; Park, S.W.; Sagong, H.Y.; Jung, Y.K. Recent Advances in Electrochemical and Optical Biosensors for Cancer Biomarker Detection. Biochip. J. 2022, 17, 44–67. [Google Scholar] [CrossRef]
- Servarayan, K.L.; Sundaram, E.; Manna, A.; Sivasamy, V.V. Label free optical biosensor for insulin using naturally existing chromene mimic synthesized receptors: A greener approach. Anal. Chim. Acta 2023, 1239, 340692. [Google Scholar] [CrossRef]
- Hamouleh-Alipour, A.; Forouzeshfard, M.; Baghbani, R.; Vafapour, Z. Blood Hemoglobin Concentration Sensing by Optical Nano Biosensor-Based Plasmonic Metasurface: A Feasibility Study. IEEE Trans. Nanotechnol. 2022, 21, 620–628. [Google Scholar] [CrossRef]
- Sanz, V.; Marcos, S.D.; Galbán, J. A reagentless optical biosensor based on the intrinsic absorption properties of peroxidase. Biosens. Bioelectron. 2007, 22, 956–964. [Google Scholar] [CrossRef]
- Sivakumar, R.; Lee, N.Y. Recent advances in airborne pathogen detection using optical and electrochemical biosensors. Anal. Chim. Acta 2022, 1234, 340297. [Google Scholar] [CrossRef]
- Pebdeni, A.B.; Roshani, A.; Mirsadoughi, E.; Behzadifar, S.; Hosseini, M. Recent advances in optical biosensors for specific detection of E. coli bacteria in food and water. Food Control 2022, 135, 108822. [Google Scholar] [CrossRef]
- Zhu, J.; Yang, B.; Hao, H.; Peng, L.; Lou, S. Gold nanoparticles-based colorimetric assay of pesticides: A critical study on aptamer’s role and another alternative sensor array strategy. Sens. Actuators B Chem. 2023, 381, 133439. [Google Scholar] [CrossRef]
- Yang, Y.; Lei, X.; Liu, B.; Liu, H.; Chen, J.; Fang, G.; Liu, J.; Wang, S. A ratiometric fluorescent sensor based on metalloenzyme mimics for detecting organophosphorus pesticides. Sens. Actuators B Chem. 2023, 377, 133031. [Google Scholar] [CrossRef]
- Huertas, C.S.; Calvo-Lozano, O.; Mitchell, A.; Lechuga, L.M. Advanced Evanescent-Wave Optical Biosensors for the Detection of Nucleic Acids: An Analytic Perspective. Front. Chem. 2019, 7, 724. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Gong, P.; Zhang, Y.; Zhou, X. Label-Free Micro Probe Optical Fiber Biosensor for Selective and Highly Sensitive Glucose Detection. IEEE Trans. Instrum. Meas. 2022, 7, 1–8. [Google Scholar] [CrossRef]
- Shumeiko, V.; Malach, E.; Helman, Y.; Paltiel, Y.; Bisker, G.; Hayouka, Z.; Shoseyov, O. A nanoscale optical biosensor based on peptide encapsulated SWCNTs for detection of acetic acid in the gaseous phase. Sens. Actuators B Chem. 2021, 327, 128832. [Google Scholar] [CrossRef]
- Malmir, K.; Habibiyan, H.; Ghafoorifard, H. Ultrasensitive optical biosensors based on microresonators with bent waveguides. Optik 2020, 216, 164906. [Google Scholar] [CrossRef]
- Konopsky, V.N.; Alieva, E.V. Imaging biosensor based on planar optical waveguide. Opt. Laser Technol. 2019, 115, 171–175. [Google Scholar] [CrossRef]
- Khani, S.; Hayati, M. Optical biosensors using plasmonic and photonic crystal band-gap structures for the detection of basal cell cancer. Sci. Rep. 2022, 12, 5246. [Google Scholar] [CrossRef]
- Xiao, X.; Gao, Y.; Xiang, J.; Zhou, F. Laser-induced thermal effect in surface plasmon resonance. Anal. Chim. Acta 2010, 676, 75–80. [Google Scholar] [CrossRef]
- Yang, Z.; Liu, C.; Gao, Y.; Wang, J.; Yang, W. Influence of surface roughness on surface plasmon resonance phenomenon of gold film. Chin. Opt. Lett. 2016, 14, 042401. [Google Scholar] [CrossRef] [Green Version]
- Bieker, P.; Schönhoff, M. Linear and Exponential Growth Regimes of Multilayers of Weak Polyelectrolytes in Dependence on pH. Macromolecules 2010, 43, 5052–5059. [Google Scholar] [CrossRef]
- Früh, A.; Rutkowski, S.; Akimchenko, I.O.; Tverdokhlebov, S.I.; Frueh, J. Orientation analysis of polymer thin films on metal surfaces via IR absorbance of the relative transition dipole moments. Appl. Surf. Sci. A J. Devoted Prop. Interfaces Relat. Synth. Behav. Mater. 2022, 594, 153476. [Google Scholar] [CrossRef]
- Puttharugsa, C.; Wangkam, T.; Houngkamhang, N.; Yodmongkol, S.; Gajanandana, O.; Himananto, O.; Sutapun, B.; Amarit, R.; Somboonkaew, A.; Srikhirin, T. A polymer surface for antibody detection by using surface plasmon resonance via immobilized antigen. Curr. Appl. Phys. 2013, 13, 1008–1013. [Google Scholar] [CrossRef]
- Biberoğlu, Ö. The Determination of Listeria monocytogenes in Foods with Optical Biosensors. Van. Vet. J. 2020, 31, 50–55. [Google Scholar] [CrossRef] [Green Version]
- Ermini, M.L.; Mariani, S.; Scarano, S.; Minunni, M. Bioanalytical approaches for the detection of single nucleotide polymorphisms by Surface Plasmon Resonance biosensors. Biosens. Bioelectron. 2014, 61, 28–37. [Google Scholar] [CrossRef] [PubMed]
- Homola, J.; Dostálek, J.; Chen, S.; Rasooly, A.; Jiang, S.; Yee, S.S. Spectral surface plasmon resonance biosensor for detection of staphylococcal enterotoxin B in milk. Int. J. Food. Microbiol. 2002, 75, 61–69. [Google Scholar] [CrossRef]
- Situ, C.; Crooks, S.R.; Baxter, A.G.; Ferguson, J.; Elliott, C.T. On-line detection of sulfamethazine and sulfadiazine in porcine bile using a multi-channel high-throughput SPR biosensor. Anal. Chim. Acta 2002, 473, 143–149. [Google Scholar] [CrossRef]
- Caldow, M.; Stead, S.L.; Day, J.; Sharman, M.; Situ, C.; Chris, E. Development and validation of an optical SPR biosensor assay for tylosin residues in honey. J. Agric. Food. Chem. 2005, 53, 7367–7370. [Google Scholar] [CrossRef]
- Riedel, T.; Rodriguez-Emmenegger, C.; Pereira, A.S.; Bědajánková, A.; Jinoch, P.; Boltovets, P.M.; Brynda, E. Diagnosis of Epstein–Barr virus infection in clinical serum samples by an SPR biosensor assay. Biosens. Bioelectron. 2014, 55, 278–284. [Google Scholar] [CrossRef]
- Li, Y.; Chen, H.; Chen, Q.; Li, H.; Gao, Z. Surface plasmon resonance induced methane gas sensor in hollow core anti-resonant fiber. Opt. Fiber. Technol. 2023, 78, 103293. [Google Scholar] [CrossRef]
- Lokman, N.F.; Bakar, A.; Ashrif, A.; Suja, F.; Abdullah, H.; Rahman, W.B.W.A.; Huang, N.; Yaacob, M.H. Highly sensitive SPR response of Au/chitosan/graphene oxide nanostructured thin films toward Pb (II) ions. Sens. Actuators B Chem. 2014, 195, 459–466. [Google Scholar] [CrossRef]
- Leong, K.H.; Gan, B.L.; Ibrahim, S.; Saravanan, P. Synthesis of surface plasmon resonance (SPR) triggered Ag/TiO2 photocatalyst for degradation of endocrine disturbing compounds. Appl. Surf. Sci. 2014, 319, 128–135. [Google Scholar] [CrossRef]
- Hu, M.; Li, M.; Li, M.Y.; Wen, X.; Deng, S.; Liu, S.; Lu, H. Sensitivity Enhancement of 2D Material-Based Surface Plasmon Resonance Sensor with an Al–Ni Bimetallic Structure. Sensors 2023, 23, 1714. [Google Scholar] [CrossRef]
- Zhang, L.; Wang, J.; Tian, Y. Electrochemical in-vivo sensors using nanomaterials made from carbon species, noble metals, or semiconductors. Microchim. Acta 2014, 181, 1471–1484. [Google Scholar] [CrossRef]
- Bhardwaj, R.; Hazra, A. Realization of ppb-level acetone detection using noble metals (Au, Pd, Pt) nanoparticles loaded GO FET sensors with simultaneous back-gate effect. Microelectron. Eng. 2022, 256, 111719. [Google Scholar] [CrossRef]
- Yang, Z.; Zhou, X.; Yin, Y.; Fang, W. Determination of Nitrite by Noble Metal Nanomaterial-Based Electrochemical Sensors: A Minireview. Anal. Lett. 2021, 4, 1–25. [Google Scholar] [CrossRef]
- Fares, H.; Almokhtar, M.; Almarashi, J.Q.M.; Rashad, M.; Moustafa, S. Tunable narrow-linewidth surface plasmon resonances of graphene-wrapped dielectric nanoparticles in the visible and near-infrared. Phys. E Low-Dimens. Syst. Nanostruct. 2022, 142, 115300. [Google Scholar] [CrossRef]
- Chen, S.; Chu, S.; Song, Y.; Wu, H.; Liu, Y.; Peng, W. Near-infrared surface plasmon resonance sensor with a graphene-gold surface architecture for ultra-sensitive biodetection. Anal. Chim. Acta 2022, 1205, 339692. [Google Scholar] [CrossRef]
- Liu, Z.K.; Jiang, J.; Zhou, B.; Wang, Z.J.; Zhang, Y.; Weng, H.M.; Prabhakaran, D.; Mo, S.K.; Peng, H.; Dudin, P. A stable three-dimensional topological Dirac semimetal Cd3As2. Nat. Mater. 2014, 13, 677–681. [Google Scholar] [CrossRef]
- Kotov, O.V.; Lozovik, Y.E. Dielectric response and novel electromagnetic modes in three-dimensional Dirac semimetal films. Phys. Rev. B 2016, 93, 235417. [Google Scholar] [CrossRef] [Green Version]
- Jiang, L.; Tang, J.; Wang, Q.; Wu, Y.; Zheng, Z.; Xiang, Y.; Dai, X. Manipulating optical Tamm state in the terahertz frequency range with graphene. Chin. Opt. Lett. 2019, 17, 20008. [Google Scholar] [CrossRef]
- Ooi, K.; Ang, Y.S.; Zhai, Q.; Tan, D.; Ang, L.K.; Ong, C.K. Nonlinear plasmonics of three-dimensional dirac semimetals. APL Photonics 2019, 4, 034402. [Google Scholar] [CrossRef]
- Wu, L.; You, Q.; Shan, Y.; Gan, S.; Zhao, Y.; Dai, X.; Xiang, Y. Few-layer ti3c2tx mxene: A promising surface plasmon resonance biosensing material to enhance the sensitivity. Sens. Actuators 2018, B277, 210–215. [Google Scholar] [CrossRef]
- Wu, L.; Guo, J.; Dai, X.; Xiang, Y.; Fan, D. Sensitivity Enhanced by MoS2–Graphene Hybrid Structure in Guided-Wave Surface Plasmon Resonance Biosensor. Plasmonics 2017, 13, 281–285. [Google Scholar] [CrossRef]
- Wu, L.; Jia, Y.; Jiang, L.; Guo, J.; Dai, X.; Xiang, Y.; Fan, D. Sensitivity Improved SPR Biosensor Based on the MoS2/Graphene–Aluminum Hybrid Structure. J. Light Technol. 2017, 35, 82–87. [Google Scholar] [CrossRef]
- Lin, Z.; Jiang, L.; Wu, L.; Guo, J.; Dai, X.; Xiang, Y.; Fan, D. Tuning and Sensitivity Enhancement of Surface Plasmon Resonance Biosensor with Graphene Covered Au-MoS 2-Au Films. IEEE Photonics J. 2016, 8, 1–8. [Google Scholar] [CrossRef]
- Mrozek, P.; Gorodkiewicz, E.; Falkowski, P.; Hościło, B. Sensitivity Analysis of Single- and Bimetallic Surface Plasmon Resonance Biosensors. Sensors 2021, 21, 4348. [Google Scholar] [CrossRef]
- Cheng, Y.-C.; Chang, Y.-J.; Chuang, Y.-C.; Huang, B.-Z.; Chen, C.-C. A plasmonic refractive index sensor with an ultrabroad dynamic sensing range. Sci. Rep. 2019, 9, 5134. [Google Scholar] [CrossRef] [Green Version]
- Xiang, Y.; Zhu, J.; Wu, L.; You, Q.; Ruan, B.; Dai, X. Highly Sensitive Terahertz Gas Sensor Based on Surface Plasmon Resonance with Graphene. IEEE Photonics J. 2018, 10, 1–7. [Google Scholar] [CrossRef]
- Tang, J.; Ye, Y.; Xu, J.; Zheng, Z.; Jin, X.; Jiang, L.; Jiang, J.; Xiang, Y. High-Sensitivity Terahertz Refractive Index Sensor in A Multilayered Structure with Graphene. Nanomaterials 2020, 10, 500. [Google Scholar] [CrossRef] [Green Version]
- Chiu, N.F.; Tu, Y.C.; Huang, T.Y. Enhanced sensitivity of anti-symmetrically structured surface plasmon resonance sensors with zinc oxide intermediate layers. Sensors 2014, 14, 170–187. [Google Scholar] [CrossRef] [Green Version]
- Fen, Y.W.; Yunus, W.M.M. Characterization of the Optical Properties of Heavy Metal Ions Using Surface Plasmon Resonance Technique. Opt. Photonics J. 2011, 1, 116–123. [Google Scholar] [CrossRef] [Green Version]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, M.; Ji, C.; Tang, X.; Tian, H.; Jiang, L.; Dai, X.; Wu, X.; Xiang, Y. Sensitivity-Tunable Terahertz Liquid/Gas Biosensor Based on Surface Plasmon Resonance with Dirac Semimetal. Sensors 2023, 23, 5520. https://doi.org/10.3390/s23125520
Ren M, Ji C, Tang X, Tian H, Jiang L, Dai X, Wu X, Xiang Y. Sensitivity-Tunable Terahertz Liquid/Gas Biosensor Based on Surface Plasmon Resonance with Dirac Semimetal. Sensors. 2023; 23(12):5520. https://doi.org/10.3390/s23125520
Chicago/Turabian StyleRen, Mengjiao, Chengpeng Ji, Xueyan Tang, Haishan Tian, Leyong Jiang, Xiaoyu Dai, Xinghua Wu, and Yuanjiang Xiang. 2023. "Sensitivity-Tunable Terahertz Liquid/Gas Biosensor Based on Surface Plasmon Resonance with Dirac Semimetal" Sensors 23, no. 12: 5520. https://doi.org/10.3390/s23125520
APA StyleRen, M., Ji, C., Tang, X., Tian, H., Jiang, L., Dai, X., Wu, X., & Xiang, Y. (2023). Sensitivity-Tunable Terahertz Liquid/Gas Biosensor Based on Surface Plasmon Resonance with Dirac Semimetal. Sensors, 23(12), 5520. https://doi.org/10.3390/s23125520






