Mach–Zehnder Modulators for Microwave Polarization Measurement in Astronomy Applications
Abstract
:1. Introduction
2. LiNbO3 Commercial MZMs
SSB-SC 15 GHz Bandwidth MZM
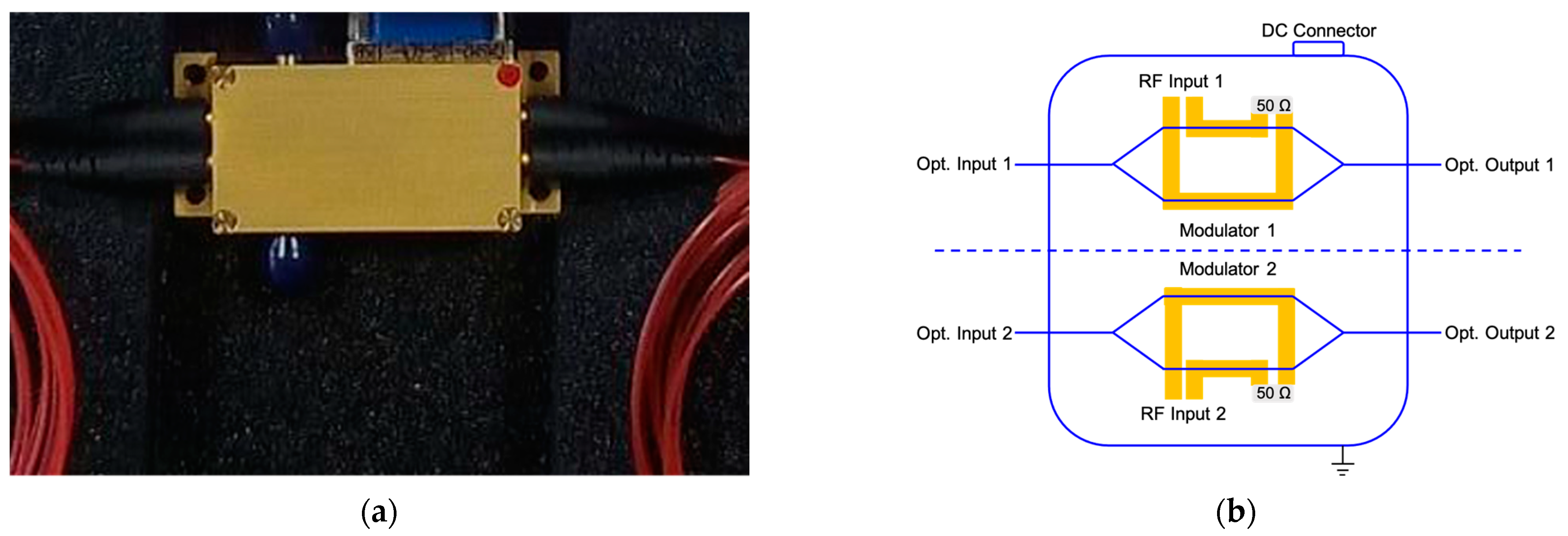
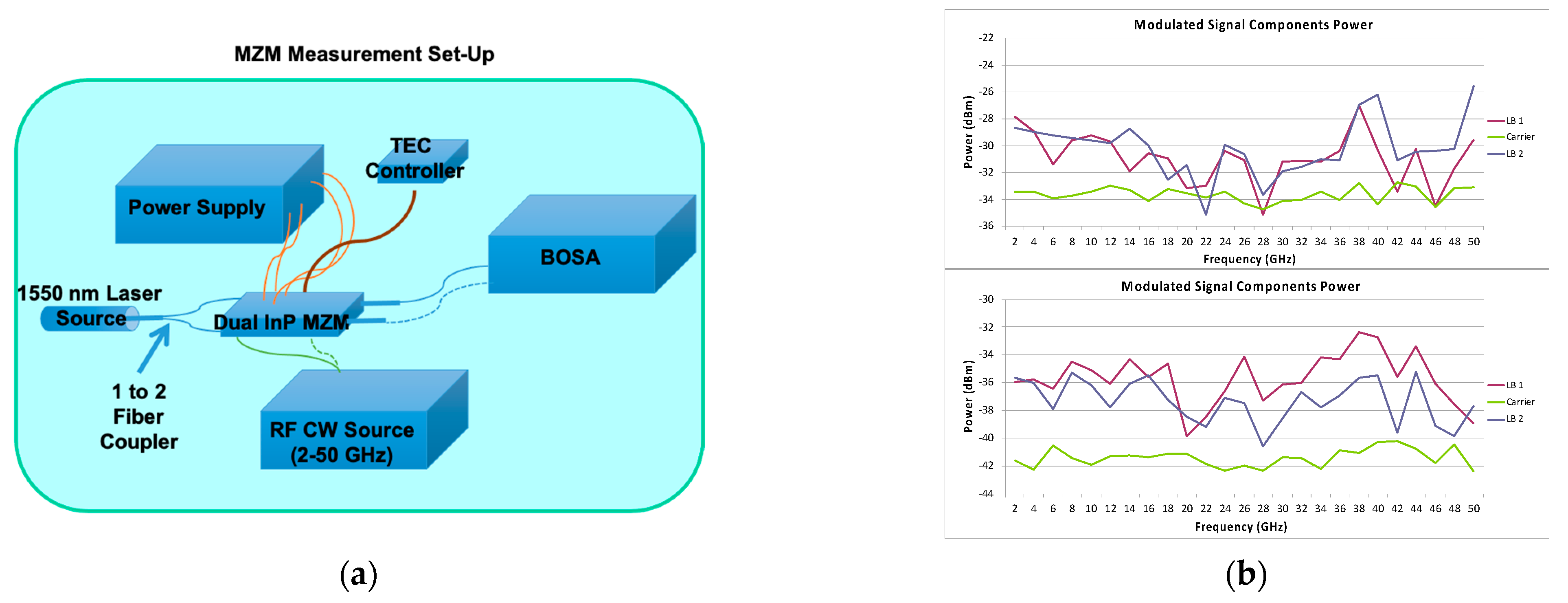
3. InP-Integrated Technology MZMs
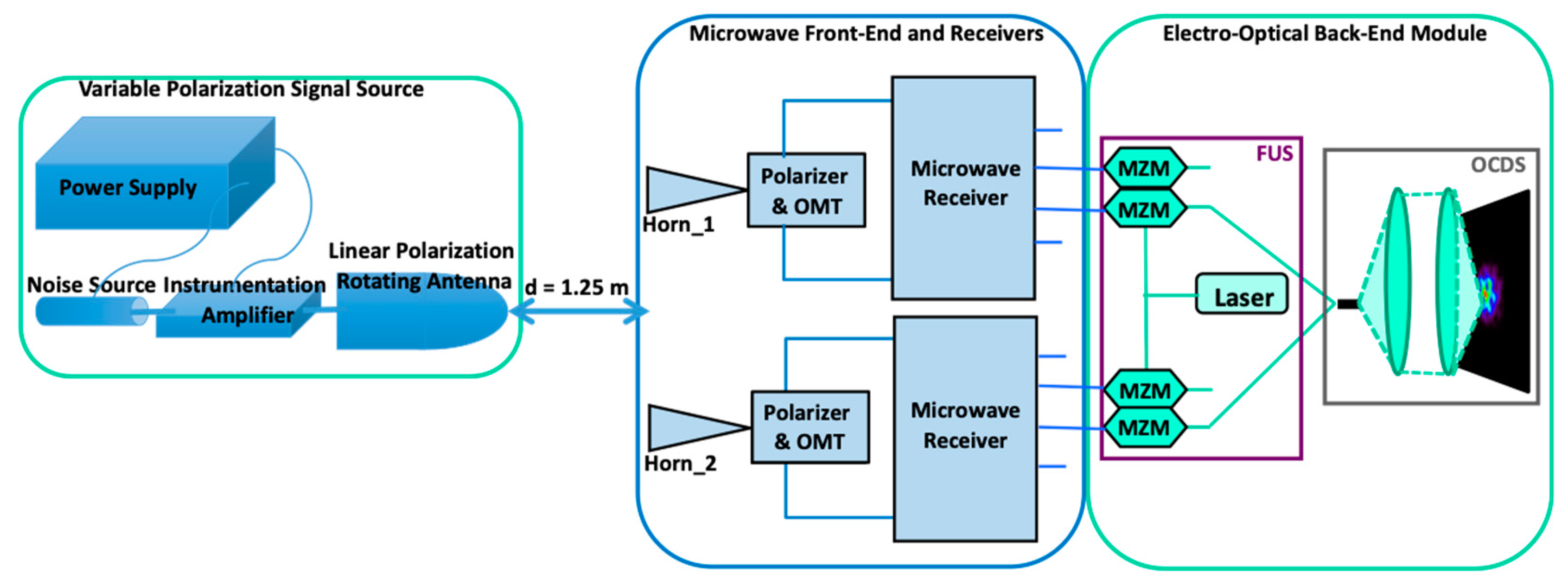
4. Application to a Microwave Polarimeter Demonstrator
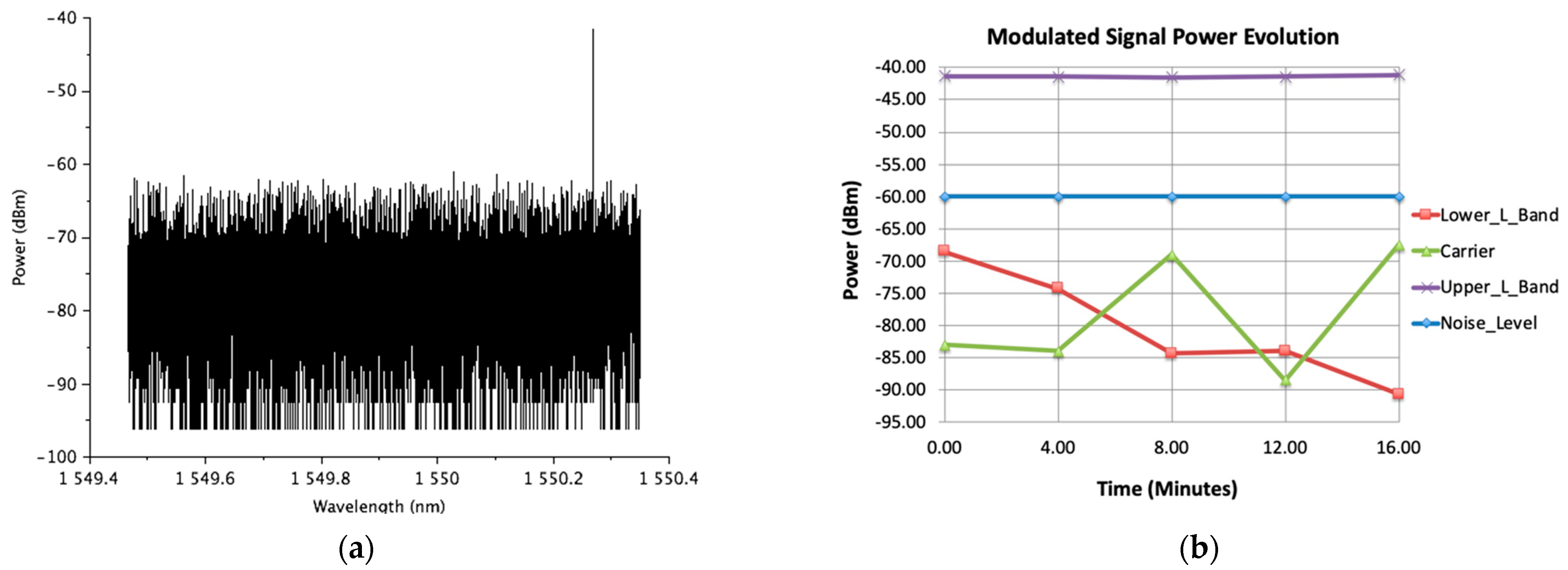
4.1. Polarization Measurement Results with LiNbO3 Commercial MZMs
4.2. Polarization Measurement Results with InP MZMs
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Penzias, A.A.; Wilson, R.W. A Measurement of Excess Antenna Temperature at 4080 Mc/s. Astrophys. J. 1965, 142, 419–421. [Google Scholar] [CrossRef]
- Alpher, R.A.; Bethe, H.; Gamow, G. The Origin of Chemical Elements. Phys. Rev. 1948, 73, 803–804. [Google Scholar] [CrossRef]
- Buder, I. Q/U Imaging Experiment (QUIET): A ground-based probe of cosmic microwave background polarization. In Proceedings of the Millimeter, Submillimeter, and Far-Infrared Detectors and Instrumentation for Astronomy V, San Diego, CA, USA, 27 June–2 July 2010; SPIE: Bellingham, WA, USA, 2010; Volume 7741. [Google Scholar]
- Barkats, D.; Aikin, R.; Bischoff, C.; Buder, I.; Kaufman, J.P.; Keating, B.G.; Kovac, J.M.; Su, M.; Ade, P.A.R.; Battle, J.O.; et al. Degree-Scale Cosmic Microwave Background Polarization Measurements from three years of BICEP1 data. Astrophys. J. Lett. 2014, 783, 67. [Google Scholar] [CrossRef] [Green Version]
- BICEP2 Collaboration; Keck Array Collaboration; Ade, P.A.R.; Ahmed, Z.; Aikin, R.W.; Alexander, K.D.; Barkats, D.; Benton, S.J.; Bischoff, C.A.; Bock, J.J.; et al. Constraints on Primordial Gravitational Waves using Planck, WMAP, and new BICEP2/Keck Observations through the 2015 season. Phys. Rev. Lett. 2018, 121, 221301. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leitch, E.M.; Kovac, J.M.; Halverson, N.W.; Carlstrom, J.E.; Pryke, C.; Smith, M.W.E. Degree Angular Scale Interferometer 3 Year Cosmic Microwave Background Polarization Results. Astrophys. J. Lett. 2005, 624, 10–20. [Google Scholar] [CrossRef] [Green Version]
- Ade, P.A.R.; Akiba, Y.; Anthony, A.E.; Arnold, K.; Atlas, M.; Barron, D.; Boettger, D.; Borrill, J.; Chapman, S.; Chinone, Y.; et al. Measurement of the Cosmic Microwave Polarization lensing power spectrum with the POLARBEAR experiment. Phys. Rev. Lett. 2014, 113, 021301. [Google Scholar] [CrossRef] [Green Version]
- Hanson, D.; Hoover, S.; Crites, A.; Ade, P.A.R.; Aird, K.A.; Austermann, J.E.; Beall, J.A.; Bender, A.N.; Benson, B.A.; Bleem, L.E.; et al. Detection of B-mode polarization in the Cosmic Microwave Background with data from the South Pole Telescope. Phys. Rev. Lett. 2013, 111, 141301. [Google Scholar] [CrossRef] [Green Version]
- Masi, S.; Ade, P.A.; Bock, J.J.; Bond, J.R.; Borrill, J.; Boscaleri, A.; Cabella, P.; Contaldi, C.R.; Crill, B.P.; de Bernardis, P.; et al. Instrument, method, brightness and polarization maps from the 2003 flight of BOOMERanG. Astron. Astrophys. 2006, 458, 687–716. [Google Scholar] [CrossRef]
- Rabii, B.; Winant, C.D.; Collins, J.S.; Lee, A.T.; Richards, P.L.; Abroe, M.E.; Hanany, S.; Johnson, B.R.; Ade, P.; Balbi, A.; et al. MAXIMA: A balloon-borne cosmic microwave background anisotropy experiment. Rev. Sci. Instrum. 2006, 77, 071101. [Google Scholar] [CrossRef]
- Bennett, C.L.; Banday, A.J.; Gorski, K.M.; Hinshaw, G.; Jackson, P.; Keegstra, P.; Kogut, A.; Smoot, G.F.; Wilkinson, D.T.; Wright, E.L. Four-Year COBE DMR Cosmic Microwave Background Observations: Maps and Basic Results. Astrophys. J. Lett. 1996, 464, L1–L4. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.L.; Larson, D.; Weiland, J.L.; Jarosik, N.; Hinshaw, G.; Odegard, N.; Smith, K.M.; Hill, R.S.; Gold, B.; Halpern, M.; et al. Nine-year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Final Maps and Results. Astrophys. J. Suppl. Ser. 2013, 208, 20. [Google Scholar] [CrossRef] [Green Version]
- Planck Collaboration; Akrami, Y.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.; Barreiro, R.; Bartolo, N.; et al. Planck Collaboration. Planck 2018 results. I. Overview, and the cosmological legacy of Planck. Astron. Astrophys. 2020, 641, A1. [Google Scholar]
- Planck Collaboration. Planck 2018 results—VI. Cosmological parameters. Astron. Astrophys. 2020, 641, A6. [Google Scholar] [CrossRef] [Green Version]
- Tristram, M.; Banday, A.J.; Górski, K.M.; Keskitalo, R.; Lawrence, C.R.; Andersen, K.J.; Barreiro, R.B.; Borrill, J.; Colombo, L.P.L.; Eriksen, H.K.; et al. Improved limits on the tensor-to-scalar ratio using BICEP and Planck. Phys. Rev. D 2022, 105, 083524. [Google Scholar] [CrossRef]
- Abazajian, K.; Crites, A.; Hui, H.; Moncelsi, L.; Schillaci, A.; Bock, J. CMB-S4 Decadal Survey APC White Paper. arXiv 2019, arXiv:1908.01062. [Google Scholar]
- Sugai, H.; Ade, P.A.R.; Akiba, Y.; Alonso, D.; Arnold, K.; Aumont, J.; Austermann, J.; Baccigalupi, C.; Banday, A.J.; Banerji, R.; et al. Updated Design of the CMB Polarization Experiment Satellite LiteBIRD. J. Low Temp. Phys. 2020, 199, 1107–1117. [Google Scholar] [CrossRef] [Green Version]
- Hanany, S.; Alvarez, M.; Artis, E.; Ashton, P.; Aumont, J.; Aurlien, R.; Banerji, R.; Barreiro, R.B.; Bartlett, J.G.; Basak, S.; et al. PICO: Probe of Inflation and Cosmic Origins. arXiv 2019, arXiv:1902.10541. [Google Scholar]
- Casas, F.J.; Ortiz, D.; Aja, D.; de la Fuente, L.; Artal, E.; Ruiz, R.; Mirapeix, J.M. A microwave polarimeter demonstrator for astronomy with near-infra-red up-conversion for optical correlation and detection. Sensors 2019, 19, 1870. [Google Scholar] [CrossRef] [Green Version]
- Casas, F.J.; Ortiz, D.; Villa, E.; Cano, J.L.; Cagigas, J.; Pérez, A.R.; Aja, B.; Terán, J.V.; de la Fuente, L.; Artal, E.; et al. The Thirty Gigahertz Instrument Receiver for the QUIJOTE Experiment: Preliminary Polarization Measurements and Systematic-Error Analysis. Sensors 2015, 15, 19124–19139. [Google Scholar] [CrossRef] [Green Version]
- Hamilton, J.-C.; Mousset, L.; Battistelli, E.; De Bernardis, P.; Bigot-Sazy, M.-A.; Chanial, P.; Charlassier, R.; D’Alessandro, G.; De Petris, M.; Lerena, M.G.; et al. Qubic i: Overview and science program. J. Cosmol. Astropart. Phys. 2022, 2022, 034. [Google Scholar] [CrossRef]
- Villa, E.; Cano, J.L.; Cagigas, J.; Ortiz, D.; Casas, F.J.; Pérez, A.R.; Aja, B.; Terán, J.V.; de la Fuente, L.; Artal, E.; et al. The thirty-gigahertz instrument receiver for the Q-U-I Joint Tenerife experiment: Concept and experimental results. Rev. Sci. Instrum. 2015, 86, 024702. [Google Scholar] [CrossRef] [PubMed]
- The LSPE collaboration; Addamo, G.; Ade PA, R.; Baccigalupi, C.; Baldini, A.M.; Battaglia, P.M.; Battistelli, E.S.; Baù, A.; de Bernardis, P.; Bersanelli, M.; et al. The large scale polarization explorer (LSPE) for CMB measurements: Performance forecast. J. Cosmol. Astropart. Phys. 2021, 2021, 008. [Google Scholar] [CrossRef]
- Collet, E. Field Guide to Polarization; SPIE Press: Bellingham, WA, USA, 2005. [Google Scholar]
- Pascual-Cisneros, G.; Casas, F.J.; Vielva, P. Optimization of a microwave polarimeter for astronomy with optical correlation and detection. Sensors 2023, 23, 2414. [Google Scholar] [CrossRef] [PubMed]
- Ortiz, D.; Casas, F.J.; Ruiz-Lombera, R.; Mirapeix, J. Electro-optic correlator for large-format microwave interferometry: Up-conversion and correlation stages performance analysis. Rev. Sci. Instrum. 2017, 88, 044702. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rubiño-Martín, J.A.; Guidi, F.; Génova-Santos, R.T.; Harper, S.E.; Herranz, D.; Hoyland, R.J.; Lasenby, A.N.; Poidevin, F.; Rebolo, R.; Ruiz-Granados, B.; et al. QUIJOTE scientific results—IV. A northern sky survey in intensity and polarization at 10–20 GHz with the multifrequency instrument. Mon. Not. R. Astron. Soc. 2023, 519, 3383–3431. [Google Scholar] [CrossRef]
- Thorlabs LN86S-FC MZM. Available online: https://www.thorlabs.com/thorproduct.cfm?partnumber=LN86S-FC&pn=LN86S-FC (accessed on 7 June 2023).
- Thorlabs LNQ4314 MZM. Available online: https://www.thorlabs.com/thorproduct.cfm?partnumber=LNQ4314 (accessed on 18 May 2023).
- Casas, F.J.; Vielva, P.; Barreiro, R.B.; Martínez-González, E.; Pascual-Cisneros, G. Polarization Calibration of a Microwave Polar- imeter with Near-Infrared Up-Conversion for Optical Correlation and Detection. Sensors 2022, 22, 8080. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Casas, F.J.; Pascual-Cisneros, G. Mach–Zehnder Modulators for Microwave Polarization Measurement in Astronomy Applications. Sensors 2023, 23, 6300. https://doi.org/10.3390/s23146300
Casas FJ, Pascual-Cisneros G. Mach–Zehnder Modulators for Microwave Polarization Measurement in Astronomy Applications. Sensors. 2023; 23(14):6300. https://doi.org/10.3390/s23146300
Chicago/Turabian StyleCasas, Francisco J., and Guillermo Pascual-Cisneros. 2023. "Mach–Zehnder Modulators for Microwave Polarization Measurement in Astronomy Applications" Sensors 23, no. 14: 6300. https://doi.org/10.3390/s23146300
APA StyleCasas, F. J., & Pascual-Cisneros, G. (2023). Mach–Zehnder Modulators for Microwave Polarization Measurement in Astronomy Applications. Sensors, 23(14), 6300. https://doi.org/10.3390/s23146300




