Optimal Frequency and Wireless Power Budget for Miniature Receivers in Obese People
Abstract
:1. Introduction and Objectives
1.1. Long-Term Monitoring with Implants and Ingestibles
1.2. Powering Miniature Medical Devices
1.3. Novelty and Objective
- Ultra-deep, miniature receivers: For the first time, mm sized receivers are investigated at depths up to 20 cm in the body, for the inclusion of all body types. Existing studies either focus on smaller depths, <10 cm, for deep implants [36,40,41,42,43,44], target larger receivers [45,46,47], or include tissue only for a small part of the Tx-Rx distance [48].
- Frequency methods and value: The approach of starting from the complete electromagnetic spectrum, from near-field inductive methods to far-field radiation and identifying the main losses at each frequency, allows us to find a maximum efficiency point. For the first time, this study explicitly focuses on the optimal efficiency for a depth of 20 cm. Interesting studies searching for the optimal frequency have been conducted already [40,49,50,51,52], although tissues are considered homogeneous, a far-field approximation is used, only tissue losses are considered for the efficiency, or the depth is limited to 10 cm while the optimal frequency for WPT significantly depends on depth and tissue characteristics.Secondly, the frequency of 13.56 MHz that will ultimately be used for the Tx and Rx design, has benefits compared to the more common mid-field and far-field approaches, as no focusing with the feedback loop is needed, the fields and power transfer are almost insensitive to variable tissue properties, and losses in electronics will we lower. The large wavelength gives this approach the benefit of transferring power to multiple receivers in the abdominal cavity more easily, undoubtedly a benefit for the application of long-term monitoring.
- User comfort: The limitations on Tx dimensions ensure that the hardware has a convenient form factor: A 10 cm coil can easily be worn on the body without hindering. A coil worn around the body as in [45,46] would be more cumbersome, needs an electrical connection to close the loop, and would require individual sizing. No need for contact gel as for US WPT also improves the user experience.

2. Models and Methods
2.1. Simulation Software and Models
2.2. PTE in a Two-Port Network
2.3. Optimal Frequency
2.3.1. Maximum Efficiency
2.3.2. Robustness
2.4. Transmitter and Receiver Design
2.4.1. Optimization Methodology
- , the number of windings in the axial direction;
- , the number of windings in the radial direction;
- d, the winding diameter;
- , the winding spacing in the axial direction;
- , the winding spacing in the radial direction.
2.4.2. Transmitter Optimization
2.4.3. Receiver Optimization
3. Results and Discussion
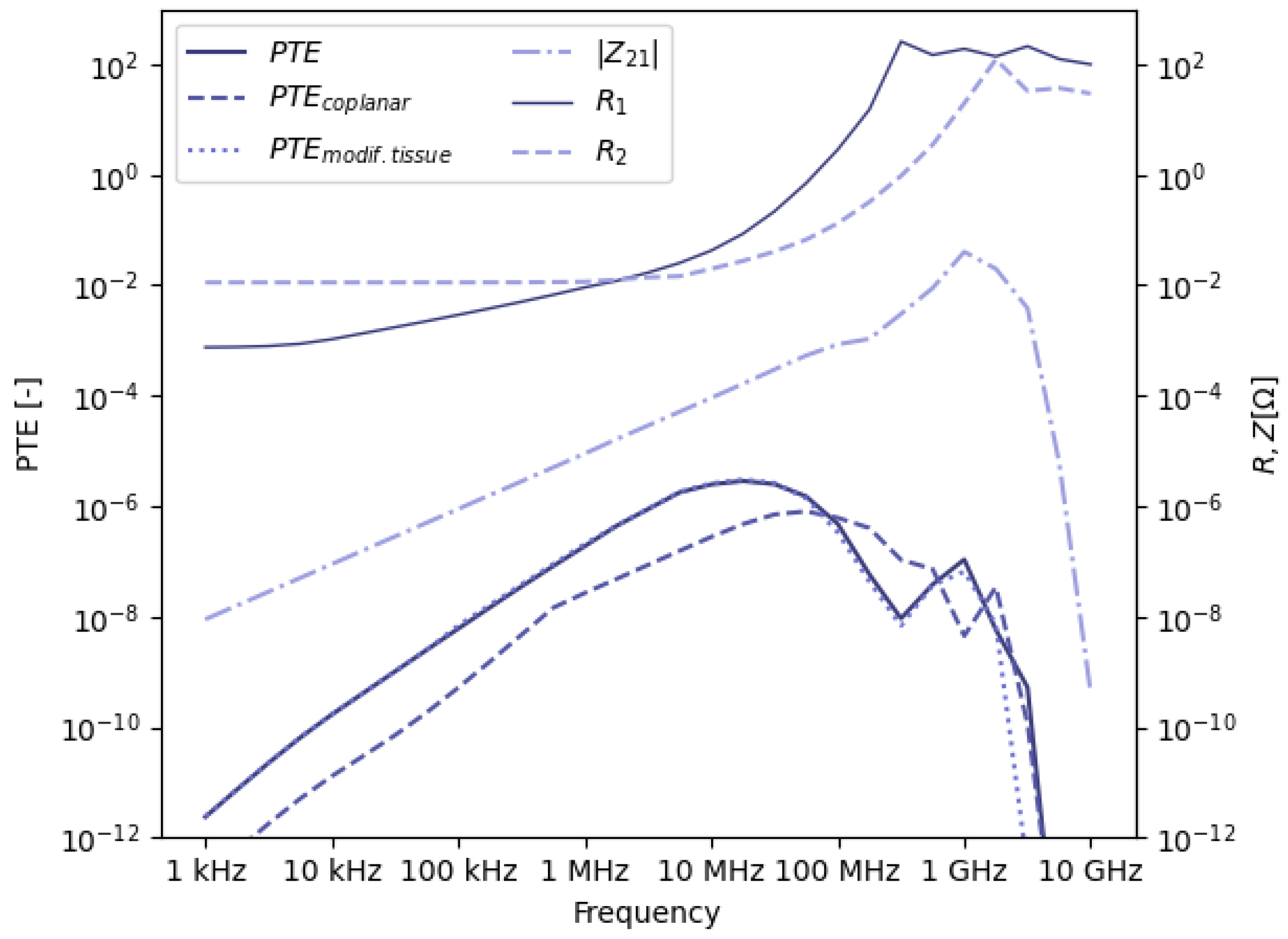
3.1. Optimal Frequency
3.1.1. Efficiency Values
3.1.2. Choice of Optimal Frequency Value
3.1.3. Robustness
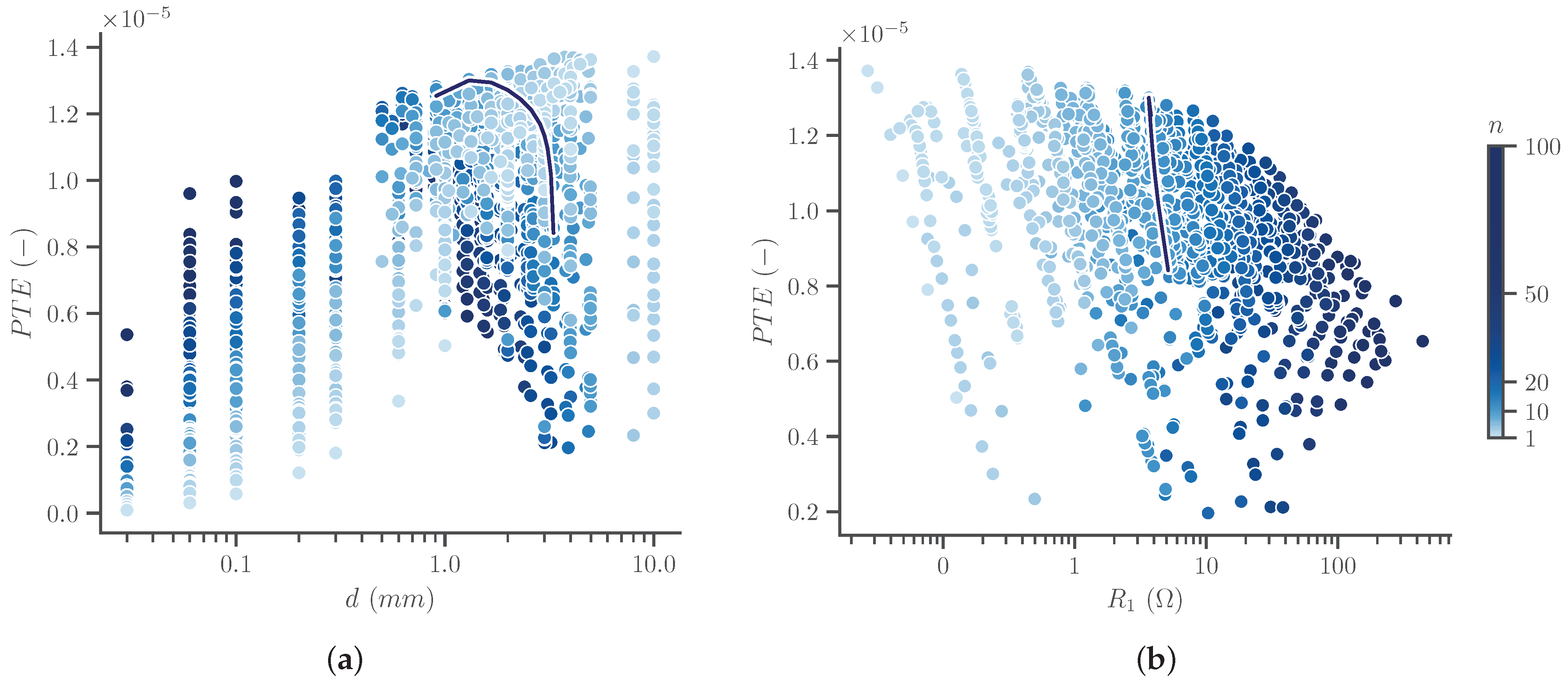
3.2. Transmitter Optimization
3.3. Receiver Optimization
3.4. Exposure and Output Power
3.4.1. Simulations of Exposure
3.4.2. Maximum Power Transfer
3.5. PTE Comparison and Applications
3.5.1. Comparison with Other Designs and Novelty
| Frequency (MHz) | Depth (mm) | Tx | Rx | PTE (%) | PTx | PRx | SAR (W/kg) | |
|---|---|---|---|---|---|---|---|---|
| [45] | 0.802 | 100 | 380 mm Ø (x2) a | 10 mm Ø | 9.1 | - | 367 mW | - |
| [47] | 1.0 | 100 | 200 mm Ø (x2) | 8.9 mm Ø | 1.0 b | 9.1 W | 91 mW | 0.66 |
| [62] | 13.56 | 50 | 45 mm Ø | mm2 | 0.03 | 0.3 W | - | 0.04 |
| [63] | 655 | 500 c | - | 10 mm Ø | 0.06 | 0.01 W | - | 0.078 |
| [64] | 915 | 1500 c | 500 mm | 10.8 mm Ø | 0.0001 | - | - | - |
| [36] | 1600 | 50 | 60 × 60 mm2 | 2 mm Ø | 0.04 | - | 195 µW | 0.89 |
| [36] | 1600 | 100 | 60 × 60 mm2 | 2 mm Ø | 0.002 | - | 10 µW | 0.89 |
| [65] | 2340 | 200 c | - | mm2 | 0.005 d | - | 100 µW | <0.1 |
| [48] | 2450 | 60 | mm3 | mm2 | 1.3 | 1 W | 13 mW | 0.90 |
| This work | 13.56 | 200 | 100 mm Ø × 10 mm | 4 mm Ø | 0.0027 | 0.7 W | 19 µW | 2.0 |
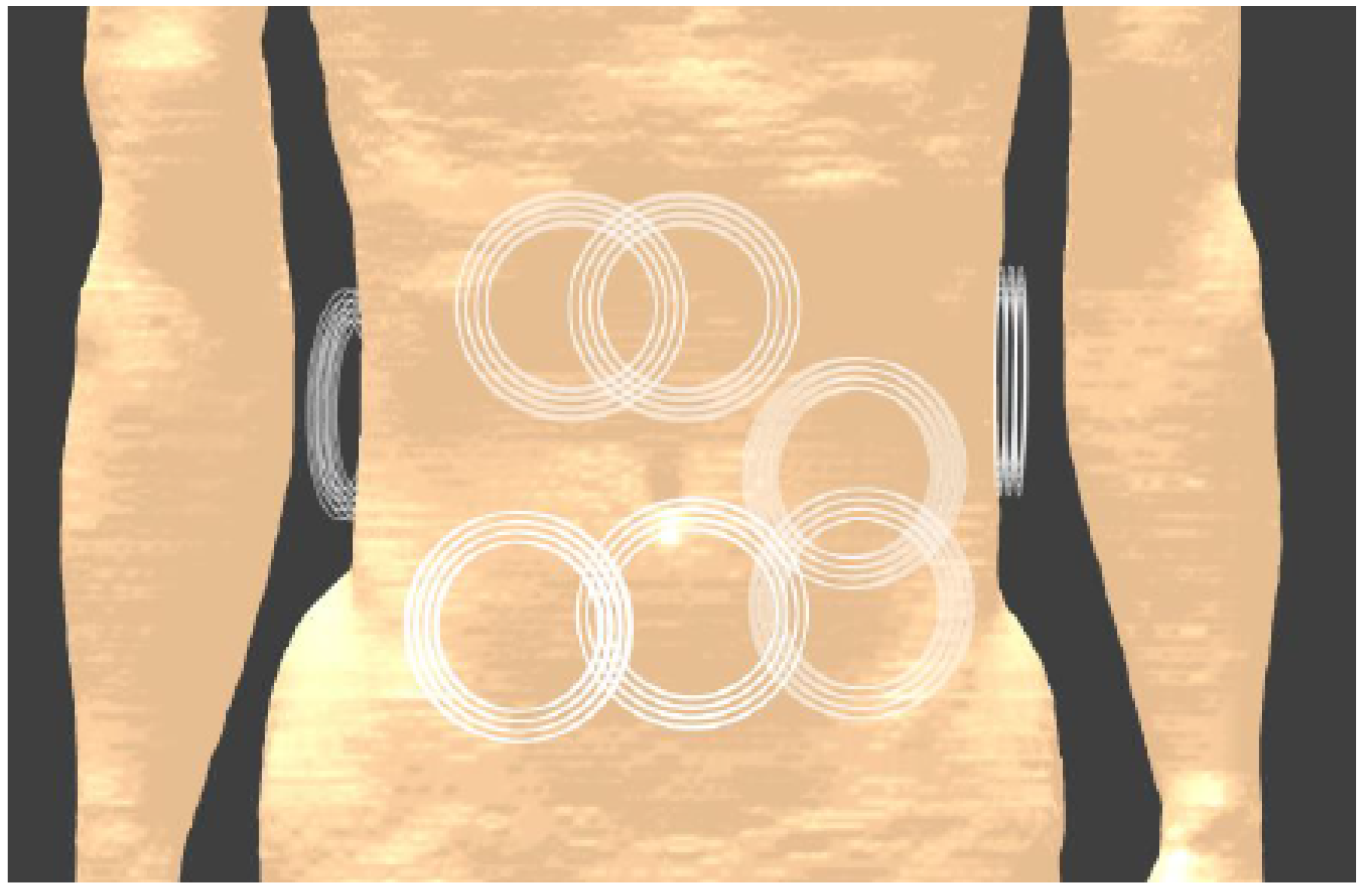
3.5.2. Applications
4. Validation
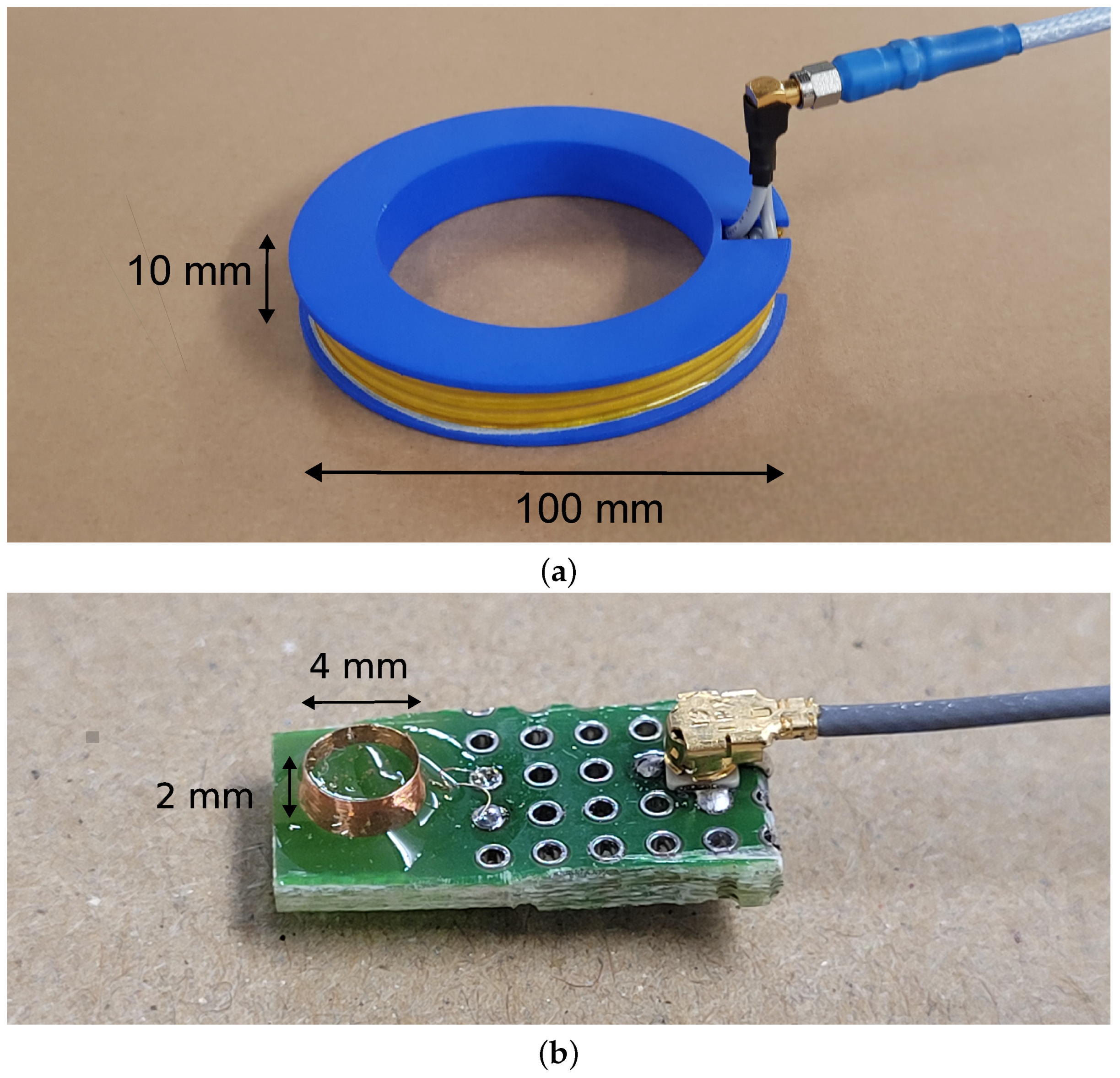
4.1. Transmitting Coil
4.2. Receiving Coil
4.3. Power Transfer
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Spaner, S.J.; Warnock, G.L. A Brief History of Endoscopy, Laparoscopy, and Laparoscopic Surgery. J. Laparoendosc. Adv. Surg. Tech. 2009, 7, 369–373. [Google Scholar] [CrossRef] [PubMed]
- Hopkins, H.H.; Kapany, N.S. A flexible fibrescope, using static scanning. Nature 1954, 173, 39–41. [Google Scholar] [CrossRef]
- Iddan, G.; Meron, G.; Glukhovsky, A.; Swain, P. Wireless capsule endoscopy. Nature 2000, 405, 417. [Google Scholar] [CrossRef] [PubMed]
- O’Grady, J.; Murphy, C.L.; Barry, L.; Shanahan, F.; Buckley, M. Defining gastrointestinal transit time using video capsule endoscopy: A study of healthy subjects. Endosc. Int. Open 2020, 8, E396. [Google Scholar] [CrossRef]
- Maqbool, S.; Parkman, H.P.; Friedenberg, F.K. Wireless capsule motility: Comparison of the smartPill® GI monitoring system with scintigraphy for measuring whole gut transit. Dig. Dis. Sci. 2009, 54, 2167–2174. [Google Scholar] [CrossRef]
- Van Helleputte, N.; Even, A.J.; Leonardi, F.; Stanzione, S.; Song, M.; Garripoli, C.; Sijbers, W.; Liu, Y.H.; Van Hoof, C. Miniaturized Electronic Circuit Design Challenges for Ingestible Devices. J. Microelectromech. Syst. 2020, 29, 645–652. [Google Scholar] [CrossRef]
- Mau, M.M.; Sarker, S.; Terry, B.S. Ingestible devices for long-term gastrointestinal residency: A review. Prog. Biomed. Eng. 2021, 3, 042001. [Google Scholar] [CrossRef]
- Liu, X.; Yang, Y.; Inda, M.E.; Lin, S.; Wu, J.; Kim, Y.; Chen, X.; Ma, D.; Lu, T.K.; Zhao, X. Magnetic Living Hydrogels for Intestinal Localization, Retention, and Diagnosis. Adv. Funct. Mater. 2021, 31, 2010918. [Google Scholar] [CrossRef]
- Palmer, A. Basal body temperature determinations in the management of menstrual disorders. Clin. Obstet. Gynecol. 1959, 2, 153–179. [Google Scholar] [CrossRef]
- Webster, W.W.; Smarr, B. Using Circadian Rhythm Patterns of Continuous Core Body Temperature to Improve Fertility and Pregnancy Planning. J. Circadian Rhythm. 2020, 18, 5. [Google Scholar] [CrossRef]
- Hasler, W.L. The use of SmartPill for gastric monitoring. Expert Rev. Gastroenterol. Hepatol. 2014, 8, 587–600. [Google Scholar] [CrossRef] [PubMed]
- Huang, F.; Magnin, C.; Brouqui, P. Ingestible sensors correlate closely with peripheral temperature measurements in febrile patients. J. Infect. 2020, 80, 161. [Google Scholar] [CrossRef] [PubMed]
- Tutuian, R.; Castell, D.O. Review article: Complete gastro-oesophageal reflux monitoring – combined pH and impedance. Aliment Pharmacol Ther 2006, 24, 27–37. [Google Scholar] [CrossRef] [PubMed]
- Liao, C.H.; Cheng, C.T.; Chen, C.C.; Jow, U.M.; Chen, C.H.; Lai, Y.L.; Chen, Y.C.; Ho, D.R. An Ingestible Electronics for Continuous and Real-Time Intraabdominal Pressure Monitoring. J. Pers. Med. 2020, 11, 12. [Google Scholar] [CrossRef]
- Mimee, M.; Nadeau, P.; Hayward, A.; Carim, S.; Flanagan, S.; Jerger, L.; Collins, J.; Mcdonnell, S.; Swartwout, R.; Citorik, R.J.; et al. An ingestible bacterial-electronic system to monitor gastrointestinal health. Science 2018, 360, 915–918. [Google Scholar] [CrossRef]
- Rezaei Nejad, H.; Oliveira, B.C.M.; Sadeqi, A.; Dehkharghani, A.; Kondova, I.; Langermans, J.A.M.; Guasto, J.S.; Tzipori, S.; Widmer, G.; Sonkusale, S.R. Ingestible Osmotic Pill for In Vivo Sampling of Gut Microbiomes. Adv. Intell. Syst. 2019, 1, 1900053. [Google Scholar] [CrossRef]
- Baltsavias, S.; Van Treuren, W.; Weber, M.J.; Charthad, J.; Baker, S.; Sonnenburg, J.L.; Arbabian, A. In Vivo Wireless Sensors for Gut Microbiome Redox Monitoring. IEEE Trans. Biomed. Eng. 2020, 67, 1821. [Google Scholar] [CrossRef]
- De la Paz, E.; Maganti, N.H.; Trifonov, A.; Jeerapan, I.; Mahato, K.; Yin, L.; Sonsa-ard, T.; Ma, N.; Jung, W.; Burns, R.; et al. A self-powered ingestible wireless biosensing system for real-time in situ monitoring of gastrointestinal tract metabolites. Nat. Commun. 2022, 13, 7405. [Google Scholar] [CrossRef]
- Sunwoo, S.H.; Lee, J.S.; Bae, S.J.; Shin, Y.J.; Kim, C.S.; Joo, S.Y.; Choi, H.S.; Suh, M.; Kim, S.W.; Choi, Y.J.; et al. Chronic and acute stress monitoring by electrophysiological signals from adrenal gland. Proc. Natl. Acad. Sci. USA 2019, 116, 1146–1151. [Google Scholar] [CrossRef]
- Lo, Y.K.; Wang, P.M.; Dubrovsky, G.; Wu, M.D.; Chan, M.; Dunn, J.C.; Liu, W. A Wireless Implant for Gastrointestinal Motility Disorders. Micromachines 2018, 9, 17. [Google Scholar] [CrossRef]
- Cigaina, V. Gastric pacing as therapy for morbid obesity: Preliminary results. Obes. Surg. 2002, 12 (Suppl. S1), S12–S16. [Google Scholar] [CrossRef] [PubMed]
- Xie, W.; Kothari, V.; Terry, B.S. A bio-inspired attachment mechanism for long-term adhesion to the small intestine. Biomed. Microdevices 2015, 17, 68. [Google Scholar] [CrossRef] [PubMed]
- AUTOCAPSULE—Autonomous Multimodal Implantable Endoscopic Capsule for the Gastrointestinal Tract. Available online: https://www.autocapsule.eu/ (accessed on 11 September 2023).
- Ghosh, A.; Li, L.; Xu, L.; Dash, R.P.; Gupta, N.; Lam, J.; Jin, Q.; Akshintala, V.; Pahapale, G.; Liu, W.; et al. Gastrointestinal-resident, shape-changing microdevices extend drug release in vivo. Sci. Adv. 2020, 6, 4133–4161. [Google Scholar] [CrossRef] [PubMed]
- Khan, S.R.; Pavuluri, S.K.; Cummins, G.; Desmulliez, M.P. Wireless Power Transfer Techniques for Implantable Medical Devices: A Review. Sensors 2020, 20, 3487. [Google Scholar] [CrossRef] [PubMed]
- Winters, C.; Subramanian, V.; Valdastri, P. Robotic, self-propelled, self-steerable, and disposable colonoscopes: Reality or pipe dream? A state of the art review. World J. Gastroenterol. 2022, 28, 5093. [Google Scholar] [CrossRef]
- Rao, K.M.P.; Alotaibi, F.M.; Alkanfery, H.M.; Mehedi, I.M.; Rao, K.P.; Alotaibi, F.M.; Alkanfery, H.M. Intelligent Wireless Capsule Endoscopy for the Diagnosis of Gastrointestinal Diseases. Diagnostics 2023, 13, 1445. [Google Scholar] [CrossRef]
- Yoshida, S.; Miyaguchi, H.; Nakamura, T. Development of Ingestible Thermometer with Built-in Coil Antenna Charged by Gastric Acid Battery and Demonstration of Long-Time in Vivo Telemetry. IEEE Access 2021, 9, 102368–102377. [Google Scholar] [CrossRef]
- Sheng, H.; Zhang, X.; Liang, J.; Shao, M.; Xie, E.; Yu, C.; Lan, W.; Sheng, H.; Zhang, X.; Liang, J.; et al. Recent Advances of Energy Solutions for Implantable Bioelectronics. Adv. Healthc. Mater. 2021, 10, 2100199. [Google Scholar] [CrossRef]
- Yoo, S.; Lee, J.; Joo, H.; Sunwoo, S.H.; Kim, S.; Kim, D.H. Wireless Power Transfer and Telemetry for Implantable Bioelectronics. Adv. Healthc. Mater. 2021, 10, 2100614. [Google Scholar] [CrossRef]
- Nadeau, P.; El-Damak, D.; Glettig, D.; Kong, Y.L.; Mo, S.; Cleveland, C.; Booth, L.; Roxhed, N.; Langer, R.; Chandrakasan, A.P.; et al. Prolonged energy harvesting for ingestible devices. Nat. Biomed. Eng. 2017, 1, 22. [Google Scholar] [CrossRef]
- Dinis, H.; Mendes, P.M. A comprehensive review of powering methods used in state-of-the-art miniaturized implantable electronic devices. Biosens. Bioelectron. 2021, 172, 112781. [Google Scholar] [CrossRef] [PubMed]
- Pinton, G.; Aubry, J.F.; Bossy, E.; Muller, M.; Pernot, M.; Tanter, M. Attenuation, scattering, and absorption of ultrasound in the skull bone. Med. Phys. 2012, 39, 299–307. [Google Scholar] [CrossRef] [PubMed]
- Agarwal, K.; Jegadeesan, R.; Guo, Y.X.; Thakor, N.V. Wireless Power Transfer Strategies for Implantable Bioelectronics. IEEE Rev. Biomed. Eng. 2017, 10, 136–161. [Google Scholar] [CrossRef]
- Lecluyse, C.; Minnaert, B.; Kleemann, M. A Review of the Current State of Technology of Capacitive Wireless Power Transfer. Energies 2021, 14, 5862. [Google Scholar] [CrossRef]
- Ho, J.S.; Yeh, A.J.; Neofytou, E.; Kim, S.; Tanabe, Y.; Patlolla, B.; Beygui, R.E.; Poon, A.S. Wireless power transfer to deep-tissue microimplants. Proc. Natl. Acad. Sci. USA 2014, 111, 7974–7979. [Google Scholar] [CrossRef] [PubMed]
- Huang, Y.; Yang, P.; Zhang, Z. An ECG Acquisition System with Piezoelectric Energy Harvesting for Low Power Healthcare Devices. In Proceedings of the International Conference on ASIC, Kunming, China, 26–29 October 2021. [Google Scholar] [CrossRef]
- Banks, E.; Lim, L.; Seubsman, S.A.; Bain, C.; Sleigh, A. Relationship of obesity to physical activity, domestic activities, and sedentary behaviours: Cross-sectional findings from a national cohort of over 70,000 Thai adults. BMC Public Health 2011, 11, 762. [Google Scholar] [CrossRef]
- Wilmet, G.; Verlinde, R.; Vandevoorde, J.; Carnol, L.; Devroey, D. Correlation between Body Mass Index and abdominal circumference in Belgian adults: A cross-sectional study. Rom. J. Intern. Med. 2017, 55, 28–35. [Google Scholar] [CrossRef]
- Soares, I.V.; Gao, M.; Cil, E.; Sipus, Z.; Skrivervik, A.K.; Ho, J.S.; Nikolayev, D. Wireless Powering Efficiency of Deep-Body Implantable Devices. IEEE Trans. Microw. Theory Tech. 2023, 71, 2680–2692. [Google Scholar] [CrossRef]
- Iqbal, A.; Al-Hasan, M.; Mabrouk, I.B.; Basir, A.; Nedil, M.; Yoo, H. Biotelemetry and Wireless Powering of Biomedical Implants Using a Rectifier Integrated Self-Diplexing Implantable Antenna. IEEE Trans. Microw. Theory Tech. 2021, 69, 3438–3451. [Google Scholar] [CrossRef]
- Mahmood, A.I.; Gharghan, S.K.; Eldosoky, M.A.; Soliman, A.M. Powering implanted sensors that monitor human activity using spider-web coil wireless power transfer. IET Power Electron. 2023, 16, 1339–1354. [Google Scholar] [CrossRef]
- Yu, Z.; Chen, J.C.; He, Y.; Alrashdan, F.T.; Avants, B.W.; Singer, A.; Robinson, J.T.; Yang, K. Magnetoelectric Bio-Implants Powered and Programmed by a Single Transmitter for Coordinated Multisite Stimulation. IEEE J. Solid-State Circuits 2022, 57, 818–830. [Google Scholar] [CrossRef]
- Singer, A.; Robinson, J.T. Wireless Power Delivery Techniques for Miniature Implantable Bioelectronics. Adv. Healthc. Mater. 2021, 10, 2100664. [Google Scholar] [CrossRef]
- Murliky, L.; Oliveira, G.; de Sousa, F.R.; Brusamarello, V.J. Tracking and Dynamic Tuning of a Wireless Powered Endoscopic Capsule. Sensors 2022, 22, 6924. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; De Santis, V.; Maradei, F.; Feliziani, M. Near field wireless powering of deep medical implants. Energies 2019, 12, 2721. [Google Scholar] [CrossRef]
- Khan, S.R.; Desmulliez, M.P. Towards a Miniaturized 3D Receiver WPT System for Capsule Endoscopy. Micromachines 2019, 10, 545. [Google Scholar] [CrossRef]
- Iqbal, A.; Sura, P.R.; Al-Hasan, M.; Mabrouk, I.B.; Denidni, T.A. Wireless power transfer system for deep-implanted biomedical devices. Sci. Rep. 2022, 12, 13689. [Google Scholar] [CrossRef]
- Poon, A.S.; O’driscoll, S.; Meng, T.H. Optimal frequency for wireless power transmission into dispersive tissue. IEEE Trans. Antennas Propag. 2010, 58, 1739–1750. [Google Scholar] [CrossRef]
- Kim, S.; Ho, J.S.; Poon, A.S. Wireless power transfer to miniature implants: Transmitter optimization. IEEE Trans. Antennas Propag. 2012, 60, 4838–4845. [Google Scholar] [CrossRef]
- Freeman, D.K.; Byrnes, S.J. Optimal Frequency for Wireless Power Transmission into the Body: Efficiency Versus Received Power. IEEE Trans. Antennas Propag. 2019, 67, 4073–4083. [Google Scholar] [CrossRef]
- Soares, I.V.; Gao, M.; Skrivervik, A.K.; Sipus, Z.; Zhadobov, M.; Sauleau, R.; Nikolayev, D. Physical bounds on implant powering efficiency using body-conformal WPT systems. In Proceedings of the 2021 IEEE Wireless Power Transfer Conference, WPTC, San Diego, CA, USA, 1–4 June 2021. [Google Scholar] [CrossRef]
- Gosselin, M.C.; Neufeld, E.; Moser, H.; Huber, E.; Farcito, S.; Gerber, L.; Jedensjo, M.; Hilber, I.; Gennaro, F.D.; Lloyd, B.; et al. Development of a new generation of high-resolution anatomical models for medical device evaluation: The Virtual Population 3.0. Phys. Med. Biol. 2014, 59, 5287. [Google Scholar] [CrossRef]
- COMSOL Multiphysics v5.6. COMSOL AB, Stockholm, Sweden. Available online: https://www.comsol.com (accessed on 13 September 2023).
- Gabriel, C. Compilation of the Dielectric Properties of Body Tissues at RF and Microwave Frequencies; Technical Report; Defense Technical Information Center: Fort Belvoir, VA, USA, 1996. [Google Scholar]
- Jayathurathnage, P.; Vilathgamuwa, M.; Simovski, C. Revisiting Two-Port Network Analysis for Wireless Power Transfer (WPT) Systems. In Proceedings of the 2018 IEEE 4th Southern Power Electronics Conference, SPEC 2018, Singapore, 10–13 December 2018. [Google Scholar] [CrossRef]
- Jiles, D.C. Modelling the Effects of Eddy Current Losses on Frequency Dependent Hysteresis in Electrically Conducting Media. IEEE Trans. Magn. 1994, 30, 4326–4328. [Google Scholar] [CrossRef]
- ASTM Standard No. B258-02; Standard Specification for Standard Nominal Diameters and Cross Sectional Areas of AWG Sizes of Solid Round Wires Used as Electrical Conductors. ASTM International: West Conshohocken, PA, USA, 2002.
- International Commission on Non-Ionizing Radiation Protection. Guidelines for limiting exposure to time-varying electric and magnetic fields (1 Hz–100 kHz). Health Phys. 2010, 99, 818–836. [Google Scholar] [CrossRef]
- IEEE. C95.1-2019—IEEE Standard for Safety Levels with Respect to Human Exposure to Electric, Magnetic, and Electromagnetic Fields, 0 Hz to 300 GHz; IEEE: Piscataway, NJ, USA, 2019. [Google Scholar] [CrossRef]
- Zurich MedTech. Sim4Life v6.2; Zurich MedTech: Zürich, Switzerland, 2018. [Google Scholar]
- Lyu, H.; John, M.; Burkland, D.; Greet, B.; Post, A.; Babakhani, A.; Razavi, M. Synchronized Biventricular Heart Pacing in a Closed-chest Porcine Model based on Wirelessly Powered Leadless Pacemakers. Sci. Rep. 2020, 10, 2067. [Google Scholar] [CrossRef]
- Abdi, A.; Aliakbarian, H. A Miniaturized UHF-Band Rectenna for Power Transmission to Deep-Body Implantable Devices. IEEE J. Transl. Eng. Health Med. 2019, 7, 1900311. [Google Scholar] [CrossRef]
- Ding, S.; Koulouridis, S.; Pichon, L. Implantable wireless transmission rectenna system for biomedical wireless applications. IEEE Access 2020, 8, 195551–195558. [Google Scholar] [CrossRef]
- Park, S.I.; Brenner, D.S.; Shin, G.; Morgan, C.D.; Copits, B.A.; Chung, H.U.; Pullen, M.Y.; Noh, K.N.; Davidson, S.; Oh, S.J.; et al. Soft, stretchable, fully implantable miniaturized optoelectronic systems for wireless optogenetics. Nat. Biotechnol. 2015, 33, 1280–1286. [Google Scholar] [CrossRef]
- Danneels, H.; Coddens, K.; Gielen, G. A fully-digital, 0.3V, 270 nW capacitive sensor interface without external references. In Proceedings of the European Solid-State Circuits Conference, Helsinki, Finland, 12–16 September 2011; pp. 287–290. [Google Scholar] [CrossRef]





| Parameter | Value (mm) |
|---|---|
| Tx–Rx distance | 200 |
| Tx diameter | 100 |
| Tx thickness | 10 |
| Rx diameter | 4 |
| Rx thickness | 2 |
| Tissue Layer | Thickness (mm) | Thickness (mm) |
|---|---|---|
| Original Model | Modified Model | |
| Skin | 2 | 2.5 |
| Subcutaneous fat | 42 | 56 |
| Muscle | 20 | 28 |
| Visceral fat | 124 | 96 |
| Small intestine | 12 | 17.5 |
| Parameter | Minimum Value | Maximum Value | |
|---|---|---|---|
| Tx | 1 | 10 | |
| 1 | 10 | ||
| d (mm) | 0.5 | 10 | |
| (mm) | 0.01 | 8 | |
| (mm) | 0.01 | 15 | |
| Rx | 1 | 40 | |
| 1 | 4 | ||
| d (mm) | 0.01 | 1.5 | |
| (mm) | 0.01 | 1.5 | |
| (mm) | 0.01 | 1.5 | |
| d (mm) | (mm) | (mm) | ||||
|---|---|---|---|---|---|---|
| 3 | 2 | 2.31 | 1.54 | 1.54 | 1.23 | |
| 3 | 3 | 1.67 | 2.50 | 2.50 | 2.41 | 1.32 |
| 3 | 4 | 1.30 | 3.04 | 3.04 | 3.66 | |
| 4 | 3 | 1.18 | 1.76 | 1.76 | 4.99 | |
| 4 | 4 | 1.18 | 1.76 | 1.76 | 7.95 | |
| 4 | 5 | 1.18 | 1.76 | 1.76 | 11.22 | |
| 5 | 4 | 1.11 | 1.11 | 1.11 | 14.29 | |
| 5 | 5 | 1.11 | 1.11 | 1.11 | 20.93 | |
| 5 | 6 | 1.11 | 1.11 | 1.11 | 28.30 | |
| 5 | 7 | 1.11 | 1.11 | 1.11 | 36.19 | |
| 5 | 8 | 1.11 | 1.11 | 1.11 | 44.47 | |
| 5 | 9 | 1.11 | 1.11 | 1.11 | 52.99 |
| Max. 10 g SAR (W/kg) for 1 W Input Power | |||
|---|---|---|---|
| Coil Next to Skin | Coil 1 mm away from Skin | Coil 5 mm away from Skin | |
| C1 | 4.3 | 4.0 | 2.9 |
| C2 | 4.1 | 3.8 | 2.8 |
| C3 | 4.2 | 3.9 | 3.0 |
| C4 | 4.2 | 3.8 | 2.9 |
| C5 | 4.3 | 3.9 | 2.9 |
| C6 | 3.9 | 3.6 | 2.6 |
| C7 | 3.8 | 3.4 | 2.5 |
| C8 | 4.0 | 3.7 | 2.6 |
| C9 | 2.8 | 2.5 | 1.9 |
| C10 | 3.9 | 3.6 | 2.6 |
| STD DEV | 0.4 | 0.4 | 0.3 |
| AVG | 3.9 | 3.6 | 2.7 |
| Quantity | Value |
|---|---|
| Tx Inductance L | 17.3 H |
| Tx Capacitance C | 12.4 pF |
| Rx Inductance L | 2.26 H |
| Rx Capacitance C | 1.52 pF |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Van de Steene, T.; Tanghe, E.; Martens, L.; Garripoli, C.; Stanzione, S.; Joseph, W. Optimal Frequency and Wireless Power Budget for Miniature Receivers in Obese People. Sensors 2023, 23, 8084. https://doi.org/10.3390/s23198084
Van de Steene T, Tanghe E, Martens L, Garripoli C, Stanzione S, Joseph W. Optimal Frequency and Wireless Power Budget for Miniature Receivers in Obese People. Sensors. 2023; 23(19):8084. https://doi.org/10.3390/s23198084
Chicago/Turabian StyleVan de Steene, Tom, Emmeric Tanghe, Luc Martens, Carmine Garripoli, Stefano Stanzione, and Wout Joseph. 2023. "Optimal Frequency and Wireless Power Budget for Miniature Receivers in Obese People" Sensors 23, no. 19: 8084. https://doi.org/10.3390/s23198084
APA StyleVan de Steene, T., Tanghe, E., Martens, L., Garripoli, C., Stanzione, S., & Joseph, W. (2023). Optimal Frequency and Wireless Power Budget for Miniature Receivers in Obese People. Sensors, 23(19), 8084. https://doi.org/10.3390/s23198084








