Mixing of Non-Collinear Lamb Wave Pulses in Plates with Material Nonlinearity
Abstract
1. Introduction
1.1. Nonlinear Guided Waves
1.2. Guided Wave Mixing
1.3. Lamb Wave Mixing
2. Theoretical Background
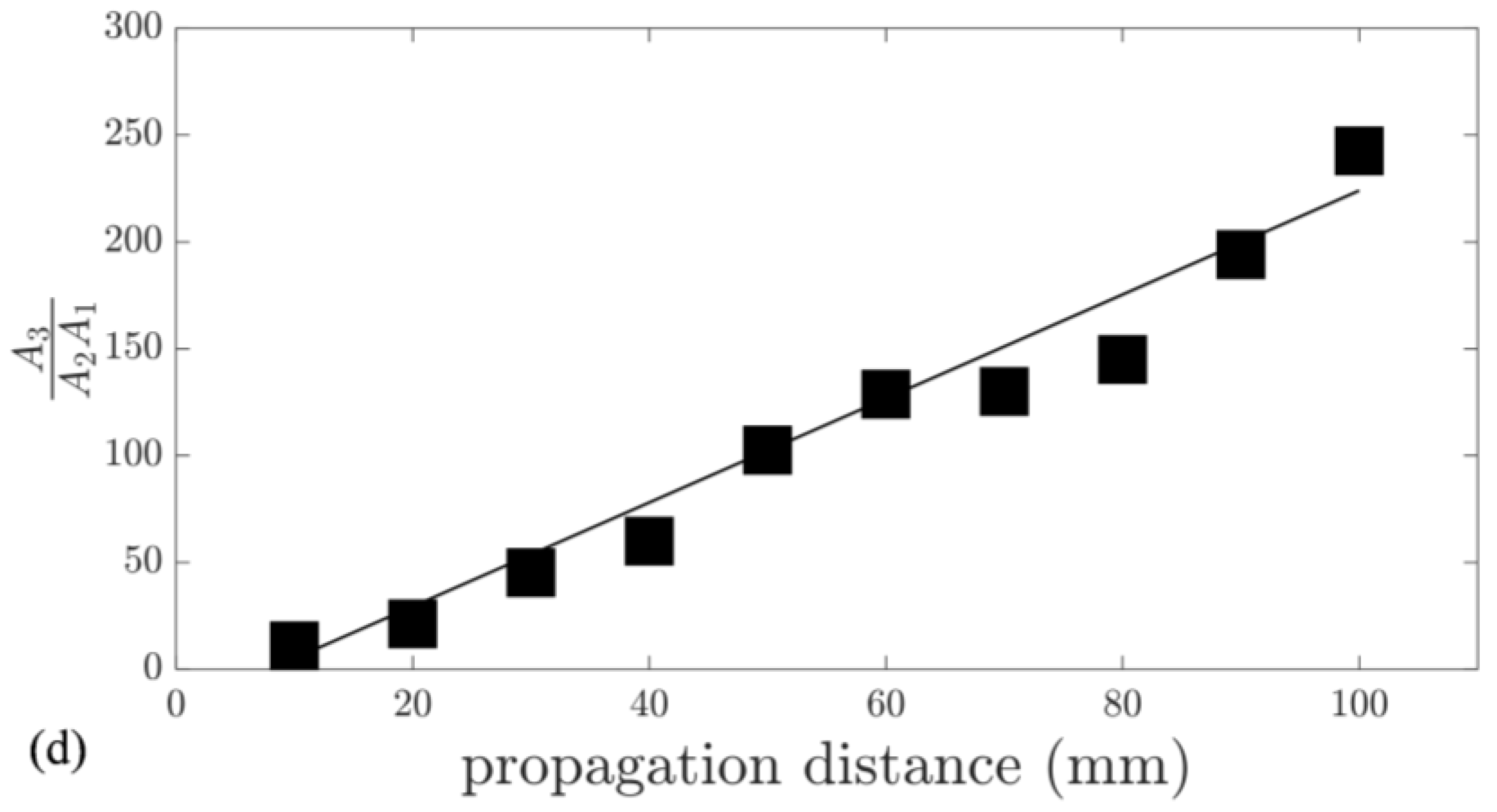
2.1. Internal Resonance Criteria for Harmonics Generation
2.2. Modelling of Material Nonlinearity
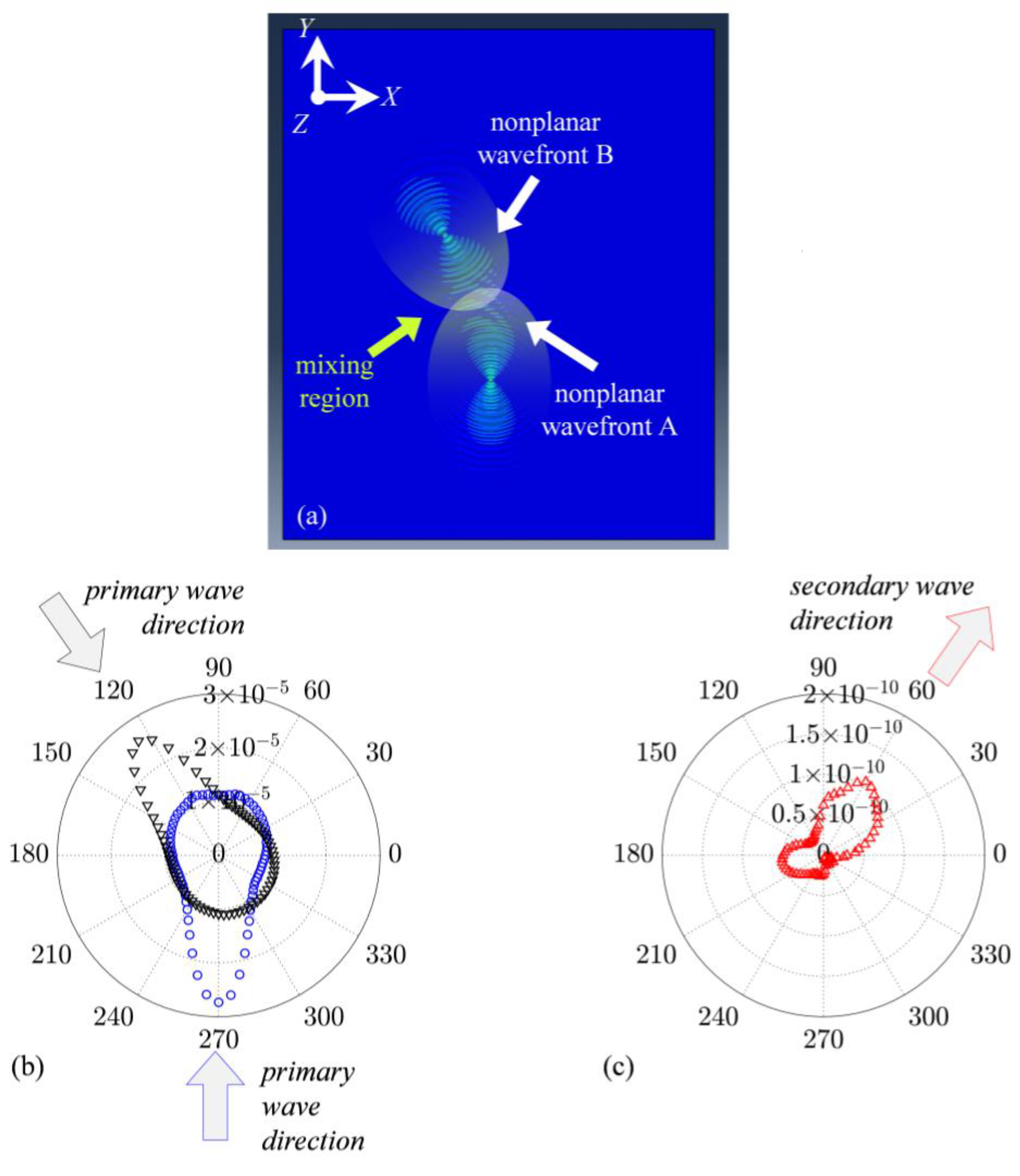
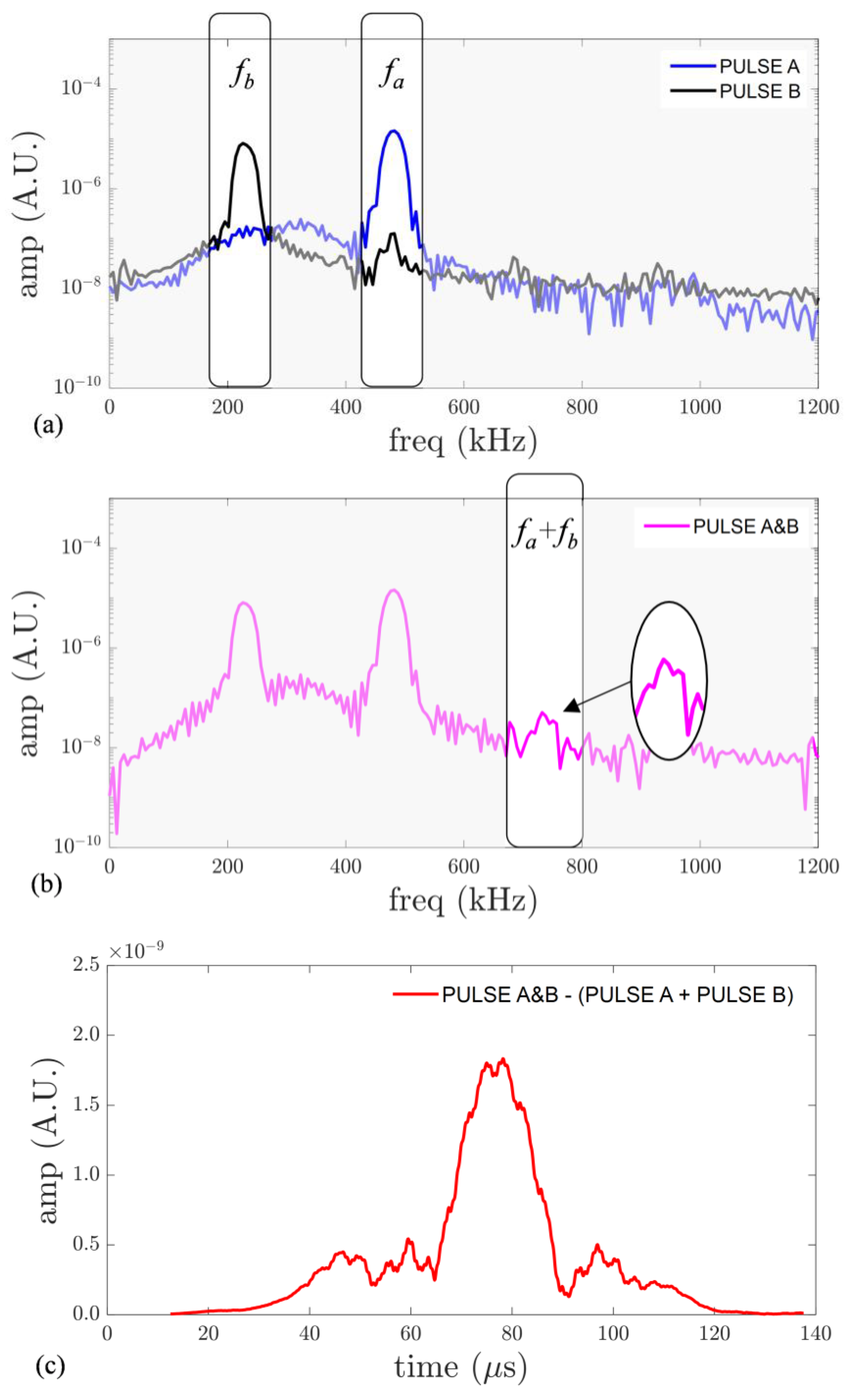
3. Finite Element Simulation
4. Experimental Study
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Chillara, V.; Lissenden, C. Review of nonlinear ultrasonic guided wave nondestructive evaluation: Theory, numerics, and experiments. Opt. Eng. 2015, 55, 011002. [Google Scholar] [CrossRef]
- Jhang, K.Y. Nonlinear ultrasonic techniques for nondestructive assessment of micro damage in material: A review. Int. J. Precis. Eng. Manuf. 2009, 10, 123–135. [Google Scholar] [CrossRef]
- Deng, M.; Pei, J. Assessment of accumulated fatigue damage in solid plates using nonlinear Lamb wave approach. Appl. Phys. Lett. 2007, 90, 121902. [Google Scholar] [CrossRef]
- Pruell, C.; Kim, J.-Y.; Qu, J.; Jacobs, L. A nonlinear-guided wave technique for evaluating plasticity-driven material damage in a metal plate. NDT E Int. 2009, 42, 199–203. [Google Scholar] [CrossRef]
- Soleimanpour, R.; Ng, C.T.; Wang, C. Higher harmonic generation of guided waves at delaminations in laminated composite beams. Struct. Health Monit. 2017, 16, 400–417. [Google Scholar] [CrossRef]
- Yang, Y.; Ng, C.T.; Kotousov, A. Influence of crack opening and incident wave angle on second harmonic generation of Lamb waves. Smart Mater. Struct. 2018, 27, 055013. [Google Scholar] [CrossRef]
- Van Den Abeele, K.E.A.; Johnson, P.A.; Sutin, A. Nonlinear Elastic Wave Spectroscopy (NEWS) techniques to discern material damage, part I: Nonlinear Wave Modulation Spectroscopy (NWMS). Res. Nondestruct. Eval. 2000, 12, 17–30. [Google Scholar] [CrossRef]
- Pineda Allen, J.C.; Ng, C.T. Debonding detection at adhesive joints using nonlinear Lamb waves mixing. NDT E Int. 2022, 125, 102552. [Google Scholar] [CrossRef]
- Croxford, A.J.; Wilcox, P.D.; Drinkwater, B.W.; Nagy, P.B. The use of non-collinear mixing for nonlinear ultrasonic detection of plasticity and fatigue. J. Acoust. Soc. Am. 2009, 126, EL117–EL122. [Google Scholar] [CrossRef]
- Liu, M.; Tang, G.; Jacobs, L.J.; Qu, J. Measuring acoustic nonlinearity parameter using collinear wave mixing. J. Appl. Phys. 2012, 112, 24908. [Google Scholar] [CrossRef]
- Tang, G.; Liu, M.; Jacobs, L.J.; Qu, J. Detecting localized plastic strain by a scanning collinear wave mixing method. J. Nondestruct. Eval. 2014, 33, 196–204. [Google Scholar] [CrossRef]
- Jiao, J.; Lv, H.; He, C.; Wu, B. Fatigue crack evaluation using the non-collinear wave mixing technique. Smart Mater. Struct. 2017, 26, 065005. [Google Scholar] [CrossRef]
- Jingpin, J.; Xiangji, M.; Cunfu, H.; Bin, W. Nonlinear Lamb wave-mixing technique for micro-crack detection in plates. NDT E Int. 2017, 85, 63–71. [Google Scholar] [CrossRef]
- Metya, A.; Tarafder, S.; Balasubramaniam, K. Nonlinear Lamb wave mixing for assessing localized deformation during creep. NDT E Int. 2018, 98, 89–94. [Google Scholar] [CrossRef]
- Zhu, H.; Ng, C.-T.; Kotousov, A. Frequency selection and time shifting for maximizing the performance of low-frequency guided wave mixing. NDT E Int. 2023, 133, 102735. [Google Scholar] [CrossRef]
- Hasanian, M.; Lissenden, C. Second order harmonic guided wave mutual interactions in plate: Vector analysis, numerical simulation, and experimental results. J. Appl. Phys. 2017, 122, 084901. [Google Scholar] [CrossRef]
- Hasanian, M.; Lissenden, C. Second order ultrasonic guided wave mutual interactions in plate: Arbitrary angles, internal resonance, and finite interaction region. J. Appl. Phys. 2018, 124, 164904. [Google Scholar] [CrossRef]
- Ishii, Y.; Hiraoka, K.; Adachi, T. Finite-element analysis of non-collinear mixing of two lowest-order antisymmetric Rayleigh–Lamb waves. J. Acoust. Soc. Am. 2018, 144, 53–68. [Google Scholar] [CrossRef]
- Li, W.; Deng, M.; Hu, N.; Xiang, Y. Theoretical analysis and experimental observation of frequency mixing response of ultrasonic Lamb waves. J. Appl. Phys. 2018, 124, 044901. [Google Scholar] [CrossRef]
- Sun, M.; Xiang, Y.; Deng, M.; Tang, B.; Zhu, W.; Xuan, F.-Z. Experimental and numerical investigations of nonlinear interaction of counter-propagating Lamb waves. Appl. Phys. Lett. 2019, 114, 011902. [Google Scholar] [CrossRef]
- Ishii, Y.; Biwa, S.; Adachi, T. Non-collinear interaction of guided elastic waves in an isotropic plate. J. Sound Vib. 2018, 419, 390–404. [Google Scholar] [CrossRef]
- De Lima, W.J.N.; Hamilton, M.F. Finite-amplitude waves in isotropic elastic plates. J. Sound Vib. 2003, 265, 819–839. [Google Scholar] [CrossRef]
- Chillara, V.; Lissenden, C. Interaction of guided wave modes in isotropic weakly nonlinear elastic plates: Higher harmonic generation. J. Appl. Phys. 2012, 111, 124909. [Google Scholar] [CrossRef]
- Vidler, J.; Kotousov, A.; Ng, C.T. Effect of randomly distributed voids on effective linear and nonlinear ealstic properties of isotropic materials. Int. J. Solids Struct. 2021, 216, 83–93. [Google Scholar] [CrossRef]
- Murnaghan, F.D. Finite deformations of an elastic soid. Am. J. Math. 1937, 52, 235–260. [Google Scholar] [CrossRef]
- Zhu, H.; Ng, C.T.; Kotousov, A. Low-frequency Lamb wave mixing for fatigue damage evaluation using phase-reversal approach. Ultrasonics 2022, 124, 106768. [Google Scholar] [CrossRef]
- Moser, F.; Jacobs, L.J.; Qu, J. Modeling elastic wave propagation in waveguides with the finite element method. NDT E Int. 1999, 32, 225–234. [Google Scholar] [CrossRef]
- Yang, Y.; Ng, C.T.; Mohabuth, M.; Kotousov, A. Finite element prediction of acoustoelastic effect associated with Lamb wave propagation in pre-stressed plates. Smart Mater. Struct. 2019, 28, 095007. [Google Scholar] [CrossRef]




| Density | l (GPa) | m (GPa) | n (GPa) | λ (GPa) | μ (GPa) |
|---|---|---|---|---|---|
| 2704 | −281.50 | −339 | −416 | 54.3 | 27.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pineda Allen, J.C.; Ng, C.T. Mixing of Non-Collinear Lamb Wave Pulses in Plates with Material Nonlinearity. Sensors 2023, 23, 716. https://doi.org/10.3390/s23020716
Pineda Allen JC, Ng CT. Mixing of Non-Collinear Lamb Wave Pulses in Plates with Material Nonlinearity. Sensors. 2023; 23(2):716. https://doi.org/10.3390/s23020716
Chicago/Turabian StylePineda Allen, Juan Carlos, and Ching Tai Ng. 2023. "Mixing of Non-Collinear Lamb Wave Pulses in Plates with Material Nonlinearity" Sensors 23, no. 2: 716. https://doi.org/10.3390/s23020716
APA StylePineda Allen, J. C., & Ng, C. T. (2023). Mixing of Non-Collinear Lamb Wave Pulses in Plates with Material Nonlinearity. Sensors, 23(2), 716. https://doi.org/10.3390/s23020716







